Optimal Design of the Laparoscopic Grasper Mechanism with Low Friction and Backlash
Abstract
1. Introduction
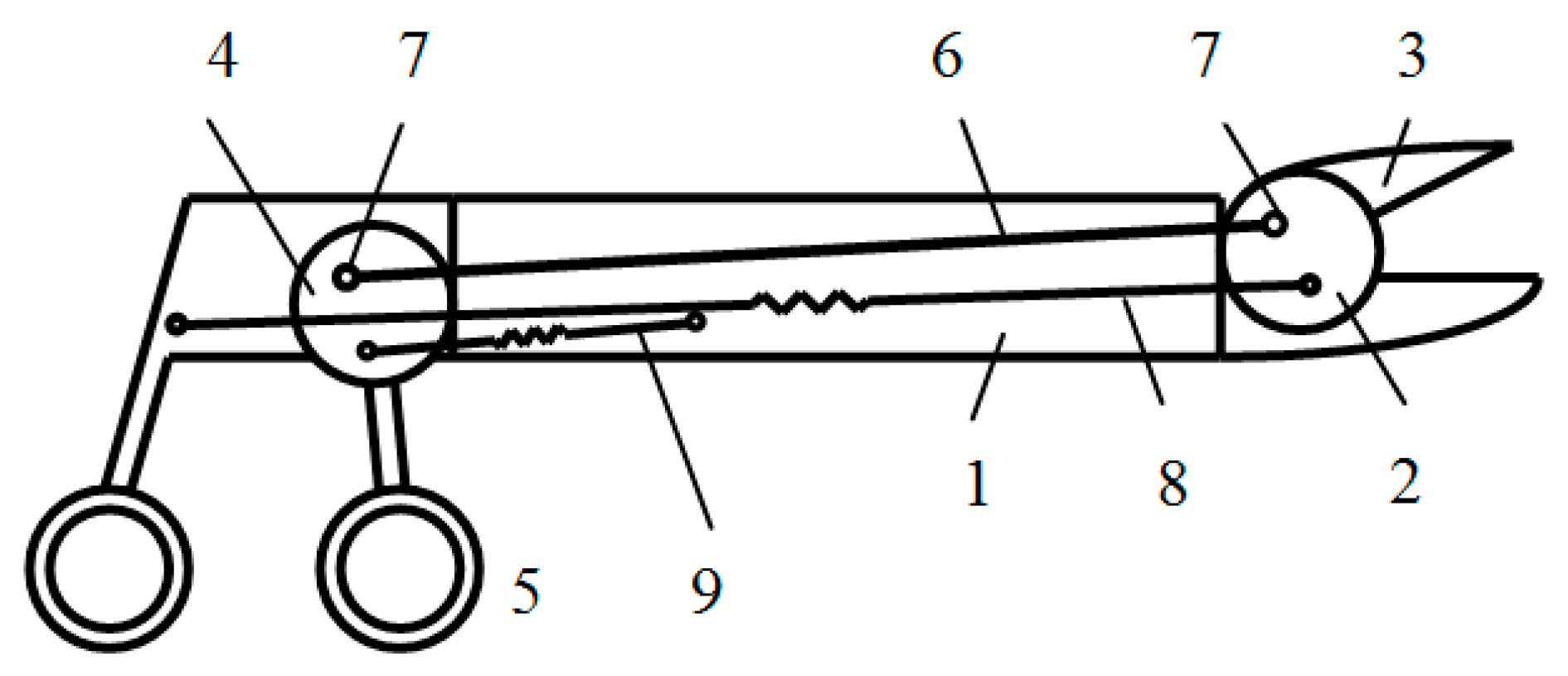
2. Kinematics of the Laparoscopic Grasper Mechanism
- —coordinate determining the position of the roller (2),
- —coordinate determining the position of the roller (4),
- and —quantities determining the position of point G on the roller (2),
- and —quantities determining the position of point H on the roller (4),
- —coordinate determining the initial position of the handle (5) for
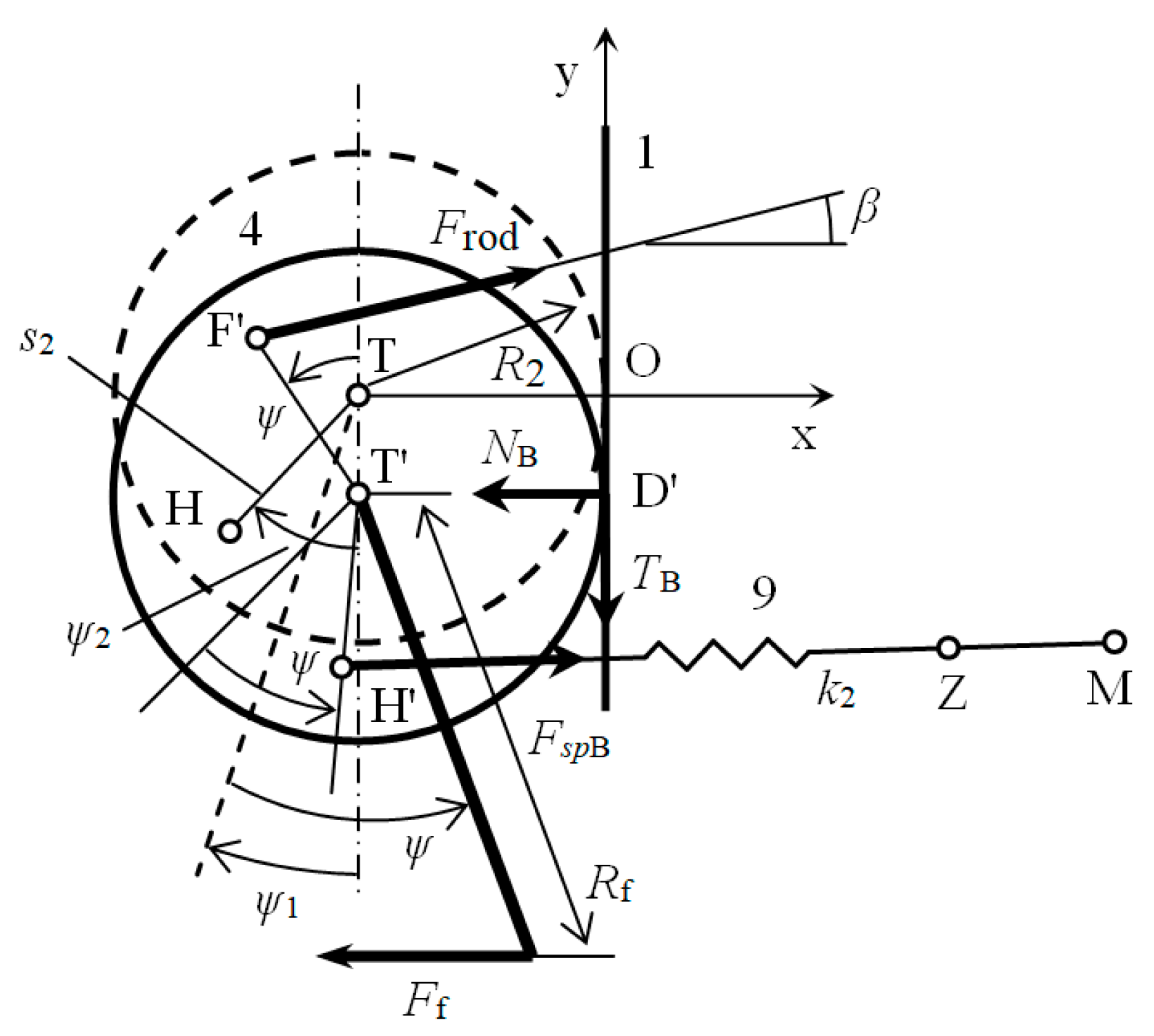
3. Statics of the Laparoscopic Grasper Mechanism
4. Elastic Potential Energy of the Springs of the Laparoscopic Grasper Mechanism
5. Formulation of the Optimization Problem
5.1. Constraints of the Optimization Problem
- (a)
- Constraint on the elastic potential energy of the mechanism springs:
- (b)
- Constraint on the axial force in the connecting rod:
5.2. Variables of the Optimization Problem
- —design variables determining the position of point G on the roller (2),
- —design variables determining the position of point H on the roller (4),
- —design variables determining the position of point K on the mechanism’s frame,
- —design variables determining the position of point M on the frame,
- —prestress coefficients of the driving and compensation springs.
- —stiffness coefficient of the driving spring,
- —stiffness coefficient of the compensation spring,
- —minimum value of the angular coordinate determining the position of the grasper jaw,
- —maximum value of the angular coordinate determining the position of the grasper jaw,
- —number of points of the movable jaw position interval
- —length,
- —radius of the rollers (2) and (4),
- —length,
- —length,
- —length of the handle,
- —force acting on the movable jaw,
- —free length of the driving spring,
- —free length of the compensation spring,
- —maximum allowable elastic potential energy in the springs,
- —maximum allowable compression force in the connecting rod,
- —angle determining the handle’s initial position.
6. Methods of Solution of the Optimization Problem
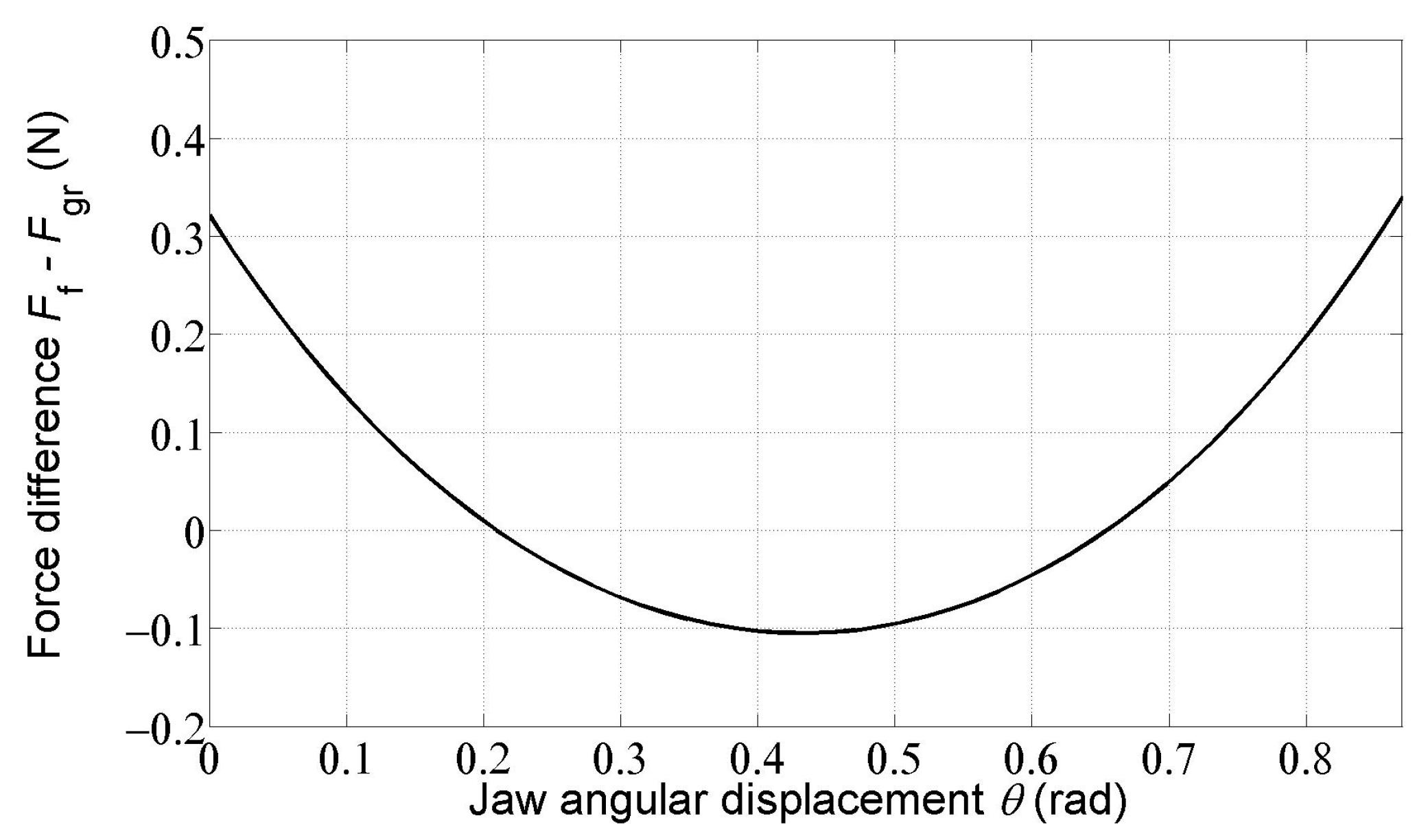
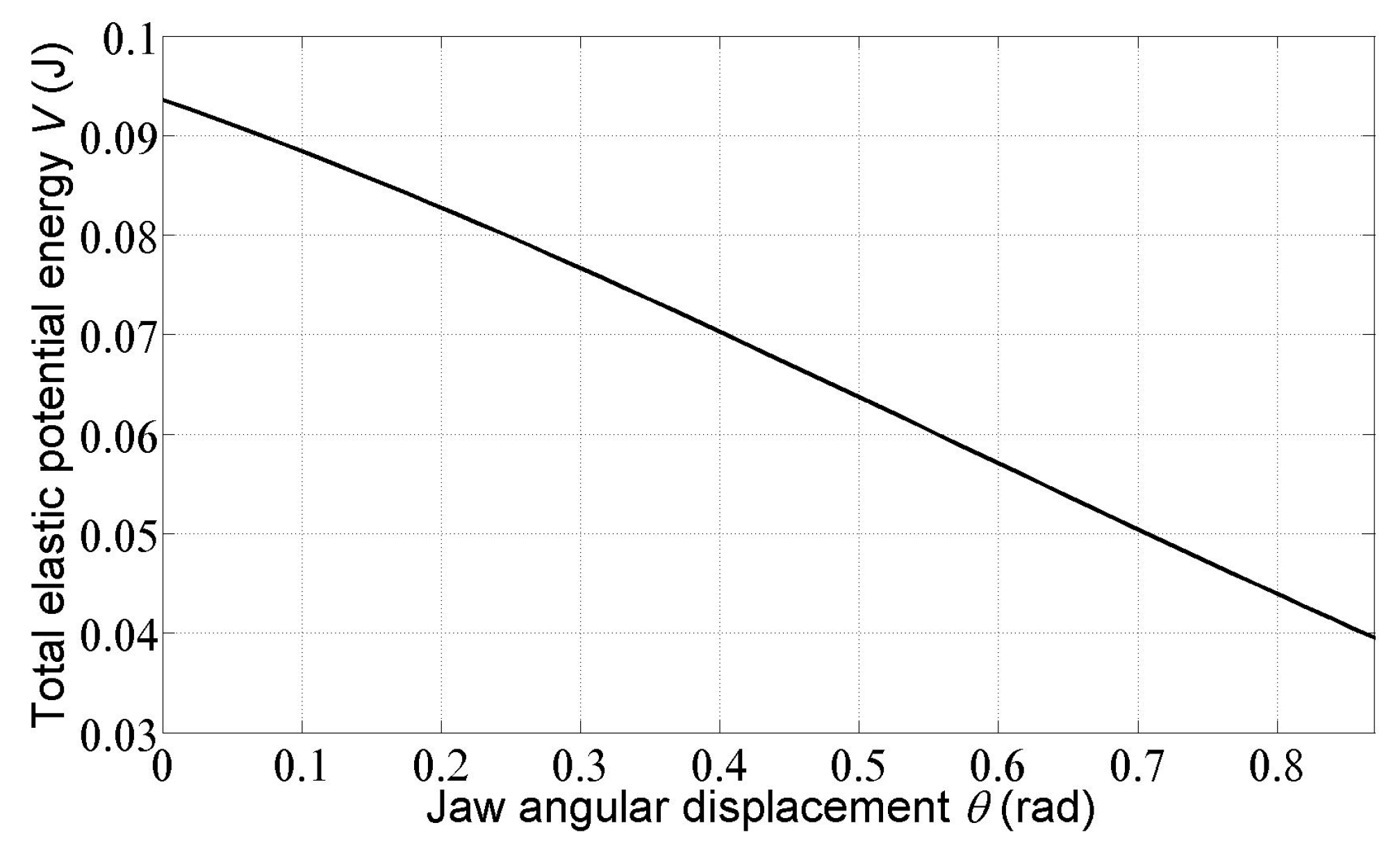
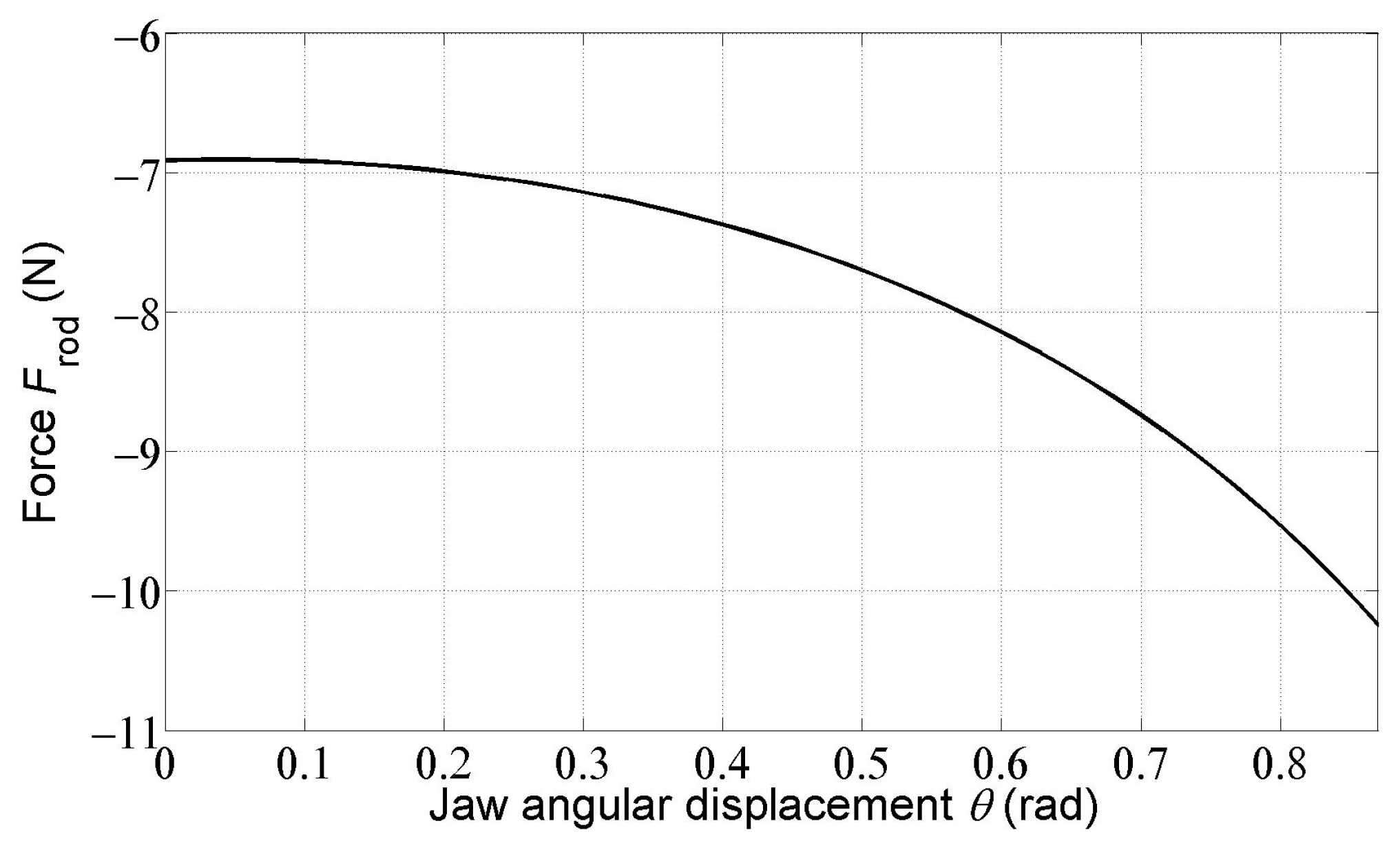
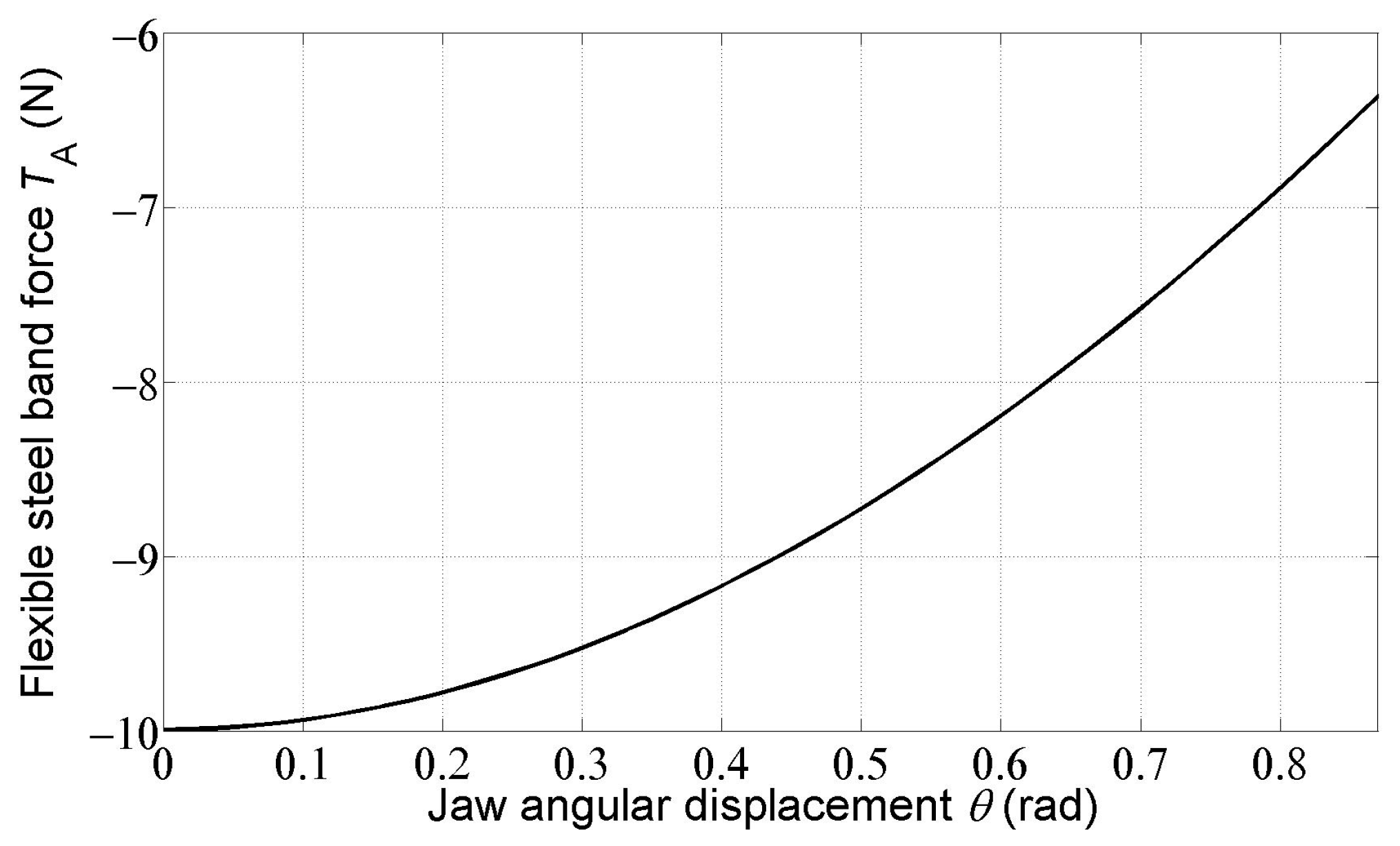
7. Results of Optimization and Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Himal, H.S. Minimally invasive (laparoscopic) surgery. Surg. Endosc. 2002, 16, 1647–1652. [Google Scholar] [CrossRef] [PubMed]
- Limb, C.; Rockall, T. Principles of laparoscopic surgery. In Reconstructive and Reproductive Surgery in Gynecology, 2nd ed.; Malcolm, G.M., Gomel, V., Eds.; CRC Press: Boca Raton, FL, USA, 2019; Volume 1, pp. 47–72. [Google Scholar] [CrossRef]
- Taylor, R.H.; Stoianovici, D. Medical robotics in computer-integrated surgery. IEEE Trans. Robot. Autom. 2003, 19, 765–781. [Google Scholar] [CrossRef]
- Ballantyne, G.H.; Leahy, P.F.; Modlin, I.M. Laparoscopic Surgery; WB Sounders Company: Philadelphia, PA, USA, 1994; p. 710. [Google Scholar]
- Herder, J.L.; Horward, M.J.; Sjoerdsma, W.A. Laparoscopic grasper with force perception. Minim. Invasive Ther. Allied Technol. 1997, 6, 279–286. [Google Scholar] [CrossRef]
- Sjoerdsma, W.A.; Herder, J.L.; Horward, M.J.; Jansen, A.; Bannenberg, J.J.G.; Grimbergen, C.A. Force transmission of laparoscopic grasping instruments. Minim Invasive Ther. Allied Technol. 1997, 6, 274–278. [Google Scholar] [CrossRef]
- Herder, J.L.; den Boer, K.T.; Sjoerdsma, W. Design and evaluation of laparoscopic forceps with accurate force feedback. In Proceedings of the 7th IFAC/IFIP/IFORS/IEA Symposium on Analysis, Design and Evaluation of Man-Machine Systems, Kyoto, Japan, 16–18 September 1998. [Google Scholar]
- Herder, J.L.; van den Berg, F.P.A. Statically Balanced Compliant Mechanisms (SBCMS): An Example and Prospects. In Proceedings of the ASME 2000 International Design Engineering Technical Conferences & Computers & Information in Engineering Conference, Baltimore, MD, USA, 10–13 September 2000. [Google Scholar]
- Hoetmer, K.; Herder, J.L.; Kim, C.J. A Building Block Approach for the Design of Statically Balanced Compliant Mechanisms. In Proceedings of the ASME 2009 International Design Engineering Technical Conferences & Computers & Information in Engineering Conference, IDETC/CIE 2009, San Diego, CA, USA, 30 August–2 September 2009. [Google Scholar]
- Tolou, N.; Herder, J.L. Concept and Modeling of a Statically Balanced Fully Compliant Grasper. In Proceedings of the ASME Design Engineering Technical Conference, San Diego, CA, USA, 30 August–2 September 2009. [Google Scholar]
- Tolou, N.; Henneken, W.A.; Herder, J.L. Statically Balanced Compliant Micro Mechanisms (SB-MEMS): Concepts and Simulation. In Proceedings of the ASME 2010 International Design Engineering Technical Conferences & Computers & Information in Engineering Conference, IDETC/CIE 2010, Montreal, QC, Canada, 15–18 August 2010. [Google Scholar]
- Gallego, J.A.; Herder, J.L. Criteria for the Static Balancing of Compliant Mechanisms. In Proceedings of the ASME 2010 International Design Engineering Technical Conferences & Computers & Information in Engineering Conference, IDETC/CIE 2010, Montreal, QC, Canada, 15–18 August 2010. [Google Scholar]
- Gallego Sánchez, J.A.G. Statically Balanced Compliant Mechanisms: Theory and Synthesis. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 9 October 2013. [Google Scholar]
- Lamers, A.J.; Sánchez, J.A.G.; Herder, J.L. Design of a statically balanced fully compliant grasper. Mech. Mach. Theory 2015, 92, 230–239. [Google Scholar] [CrossRef]
- Wang, S.; Yang, X.; Chen, Y.; Ma, J. A theoretical design of a bellow-shaped staticall balanced compliant mechanism. Mech. Mach. Theory 2021, 161, 163–172. [Google Scholar] [CrossRef]
- Puangmali, P.; Althoefer, K.; Seneviratne, L.D.; Murphy, D.; Dasgupta, P. State-of-the-Art in Force and Tactile Sensing for Minimally Invasive Surgery. IEEE Sens. J. 2008, 8, 371–380. [Google Scholar] [CrossRef]
- Faraz, A.; Payandeh, S.; Salvarinov, A. Design of a force control grasper through stiffness modulation for endosurgery: Theory and experiments. Mechatronics 2000, 10, 627–648. [Google Scholar] [CrossRef]
- Kianzad, S.; Karkouti, S.O.; Taghirad, H.D. Force control of intelligent laparoscopic forceps. J. Med. Imaging Health Inform. 2011, 1, 284–289. [Google Scholar] [CrossRef]
- Sümer, B.; Özin, M.C.; Eray, T.; Koç, I.M. The undamaged tissue grasping in a laparoscopic surgical grasper via distributed pressure measurement. Tribol. Int. 2017, 113, 330–337. [Google Scholar] [CrossRef]
- Koç, I.M.; Akça, E. Design of a piezoelectric based tactile sensor with bio-inspired micro/nano-pillars. Tribol. Int. 2013, 59, 321–331. [Google Scholar]
- Koç, I.M.; Eray, T.; Sümer, B.; Çerçi, N. An active force controlled laparoscopic grasper by using a smart material actuation. Tribol. Int. 2016, 100, 317–327. [Google Scholar] [CrossRef]
- Takizawa, T.; Kanno, T.; Miyzaki, R.; Tadano, K.; Kawashima, K. Grasping force estimation in robotic forceps using a soft pneumatic actuator with a built-in sensor. Sens. Actuators A Phys. 2018, 271, 124–130. [Google Scholar] [CrossRef]
- Xue, R.; Du, Z.; Yan, Z.; Ren, B. An estimation method of grasping force for laparoscope surgical robot based on the model of a cable-pulley system. Mech. Mach. Theory 2019, 134, 440–454. [Google Scholar] [CrossRef]
- Fukushima, K.; Miyazaki, T.; Kawase, T.; Kanno, T.; Sogabe, M.; Nakajima, Y.; Kawashima, K. A pneumatic rotary actuator for forceps tip rotation. Sens. Actuators A Phys. 2022, 333, 113222. [Google Scholar] [CrossRef]
- Chantal, C.J.A.; Vleugels, M.P.H.; Coppus, S.F.P.J.; Nieboer, T.E. The effects of laparoscopic graspers with enhanced haptic feedback on applied forces: A randomized comparison with conventional graspers. Surg. Endosc. 2017, 31, 5411–5417. [Google Scholar]
- Sancibrian, R.; Redondo-Figuero, C.; Gutierrez-Diez, M.C.; Gonzalez-Sarabia, E.; Manuel-Palazuelos, J.C. Ergonomic evaluation and performance of a new handle for laparoscopic tools in surgery. Appl. Ergon. 2020, 89, 103210. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Jia, Z.; Wang, J.; Shi, L.; Zhou, Z.R. Friction behavior at minimally invasive grasper/liver tissue interface. Tribol. Int. 2015, 81, 190–198. [Google Scholar] [CrossRef]
- Segla, S.; Kalker-Kalkman, C.M.; Schwab, A.L. Statical balancing of a robot mechanism with the aid of a genetic algorithm. Mech. Mach. Theory 1998, 33, 163–174. [Google Scholar] [CrossRef]
- Kalker-Kalkman, C.M. A design program based on the Monte Carlo method with applications. In Advances in Computer-Aided Engineering; Delft University Press: Delft, The Netherlands, 1994; pp. 61–70. [Google Scholar]
- Kalker-Kalkman, C.M.; Offermans, M.F. A general design program based on Genetic Algorithms with applications. In Proceedings of the 21st ASME Design Automation Conference, Boston, MA, USA, 14 September 1995. [Google Scholar]
- Segla, S. Static balancing of robot mechanisms and manipulation devices. SC-JME 2018, 68, 77–90. [Google Scholar] [CrossRef]
- Kalker-Kalkman, C.M. Optimal design with the aid of randomization methods. Eng. Comput. 1991, 7, 173–183. [Google Scholar] [CrossRef]
- Segla, S. Kinematic analysis and optimization of a wheel loader mechanism. Manuf. Technol. 2018, 18, 309–314. [Google Scholar] [CrossRef]
- Segla, S.; Musil, M. Comparison of passive and semi-active horizontal platform suspensions. Teh. Vjesn. 2018, 25, 1659–1666. [Google Scholar]
- Segla, S.; Kajaste, J.; Keski-Honkola, P. Optimization of Semi-active Seat Suspension with Magnetorheological Damper. In Proceedings of the 10th International Conference on Vibration Problems, Prague, Czech Republic, 7–9 September 2011. [Google Scholar]
- Optimization Toolbox. Available online: https://uk.mathworks.com/products/optimization.html (accessed on 3 November 2022).
- Global Optimization Toolbox User’s Guide. Available online: https://uk.mathworks.com/help/pdf_doc/gads/gads.pdf (accessed on 4 November 2022).
- Goldberg, D.E. Genetic Algorithms in Search, Optimization, and Machine Linearning; Addison-Wesley: Reading, MA, USA, 1989. [Google Scholar]
- Rao, S.S. Engineering Optimization Theory and Practice; Wiley: Hoboken, NJ, USA, 2020. [Google Scholar]



| Design Variable | Units | Lower Bound | Upper Bound |
|---|---|---|---|
| m | 0.0022 | 0.0034 | |
| ° | 1 | 40 | |
| m | 0.0028 | 0.0038 | |
| ° | 20 | 70 | |
| m | −0.07 | −0.02 | |
| m | −0.03 | −0.005 | |
| m | 0.12 | 0.18 | |
| m | −0.004 | 0.004 | |
| – | 0.15 | 1.8 | |
| – | 0.15 | 1.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Segla, S.; Svoboda, M. Optimal Design of the Laparoscopic Grasper Mechanism with Low Friction and Backlash. Appl. Sci. 2023, 13, 4997. https://doi.org/10.3390/app13084997
Segla S, Svoboda M. Optimal Design of the Laparoscopic Grasper Mechanism with Low Friction and Backlash. Applied Sciences. 2023; 13(8):4997. https://doi.org/10.3390/app13084997
Chicago/Turabian StyleSegla, Stefan, and Martin Svoboda. 2023. "Optimal Design of the Laparoscopic Grasper Mechanism with Low Friction and Backlash" Applied Sciences 13, no. 8: 4997. https://doi.org/10.3390/app13084997
APA StyleSegla, S., & Svoboda, M. (2023). Optimal Design of the Laparoscopic Grasper Mechanism with Low Friction and Backlash. Applied Sciences, 13(8), 4997. https://doi.org/10.3390/app13084997






