Crystal-Based X-ray Interferometry and Its Application to Phase-Contrast X-ray Imaging, Zeff Imaging, and X-ray Thermography
Abstract
:1. Introduction
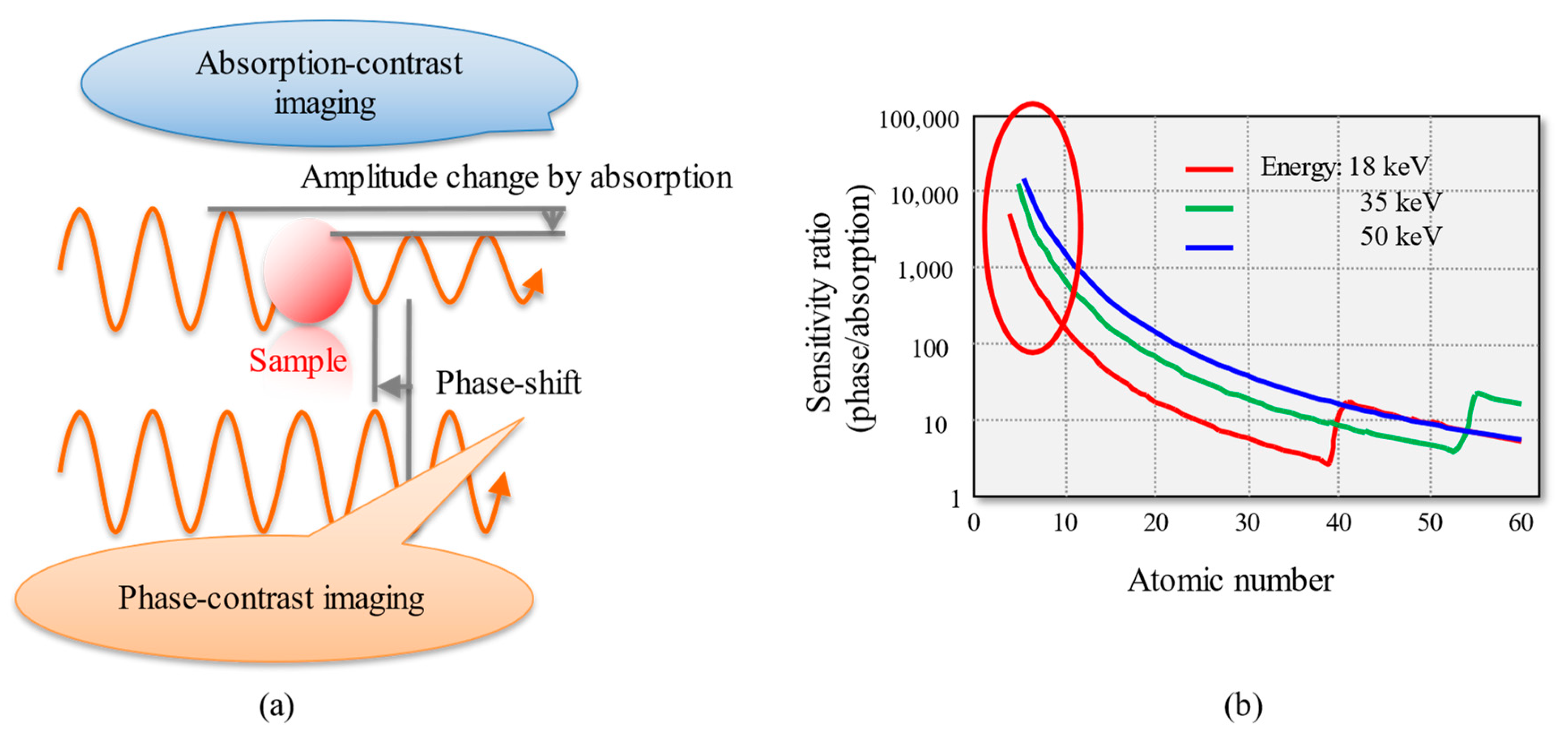
2. Principle of Phase-Contrast X-ray Imaging
- Diffraction-enhanced imaging (DEI), in which the refraction of X-rays generated by the sample (proportional to the spatial derivative of the phase shift) is detected on the basis of the X-ray diffraction (XRD) of an analyzer crystal placed downstream of the sample [4].
- Grating-based (Talbot) interferometry (GXI), in which the refraction of X-rays produced by the sample is detected with a grating interferometer (i.e., a Talbot interferometer) [5].
- Propagation-based imaging (PI), which detects phase shifts from the Fresnel fringes (a second-order derivative of the phase shifts) generated at a sufficient distance from the sample [6].
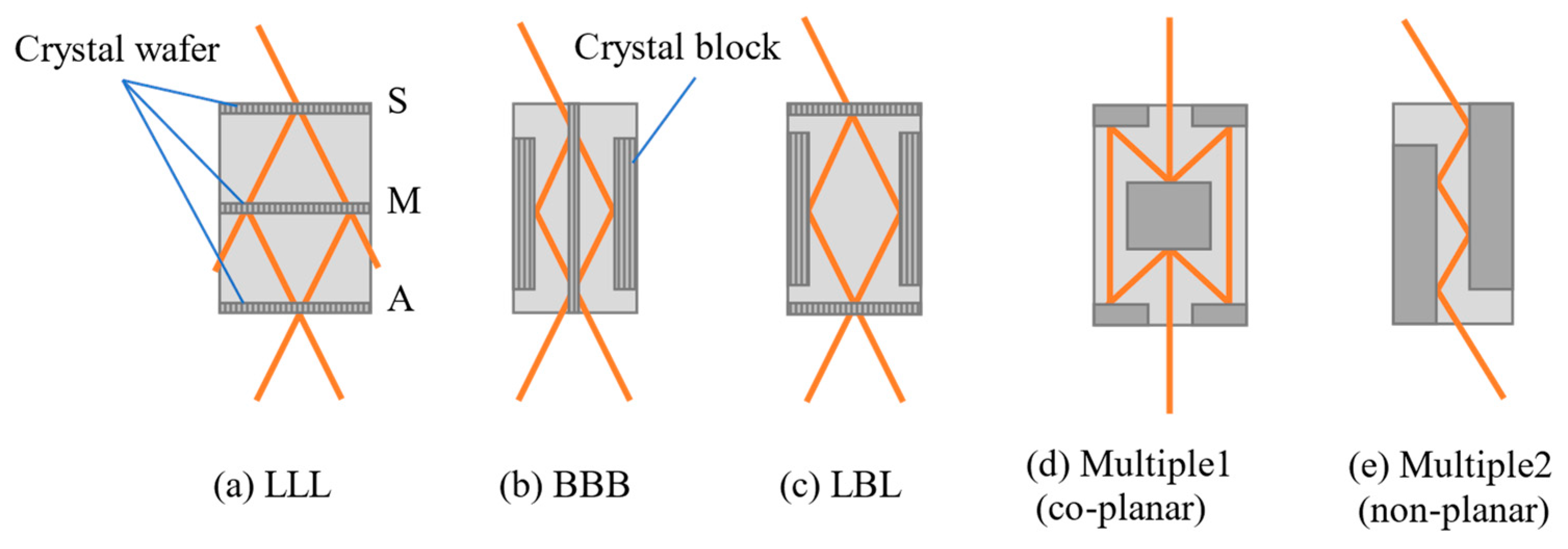
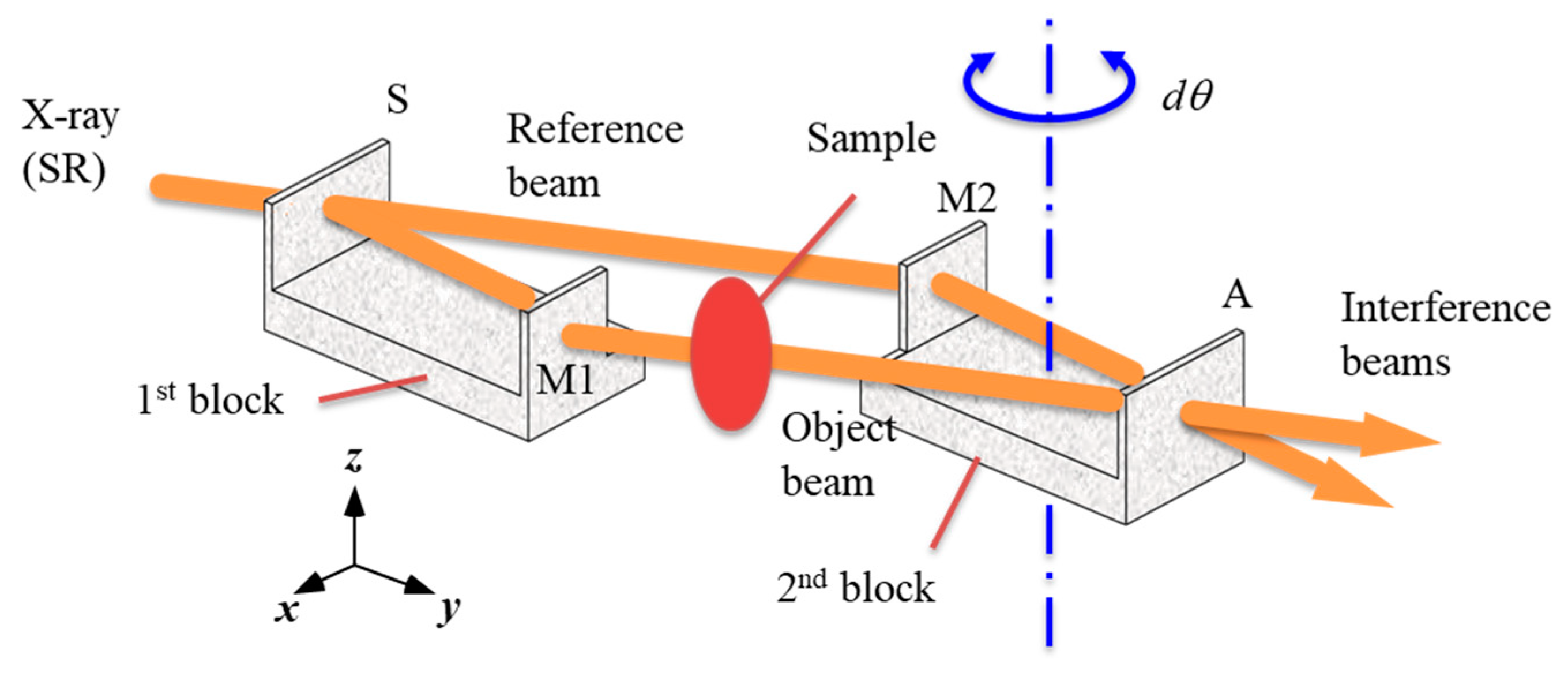
3. Crystal-Based X-ray Interferometer
4. History of CXI Development
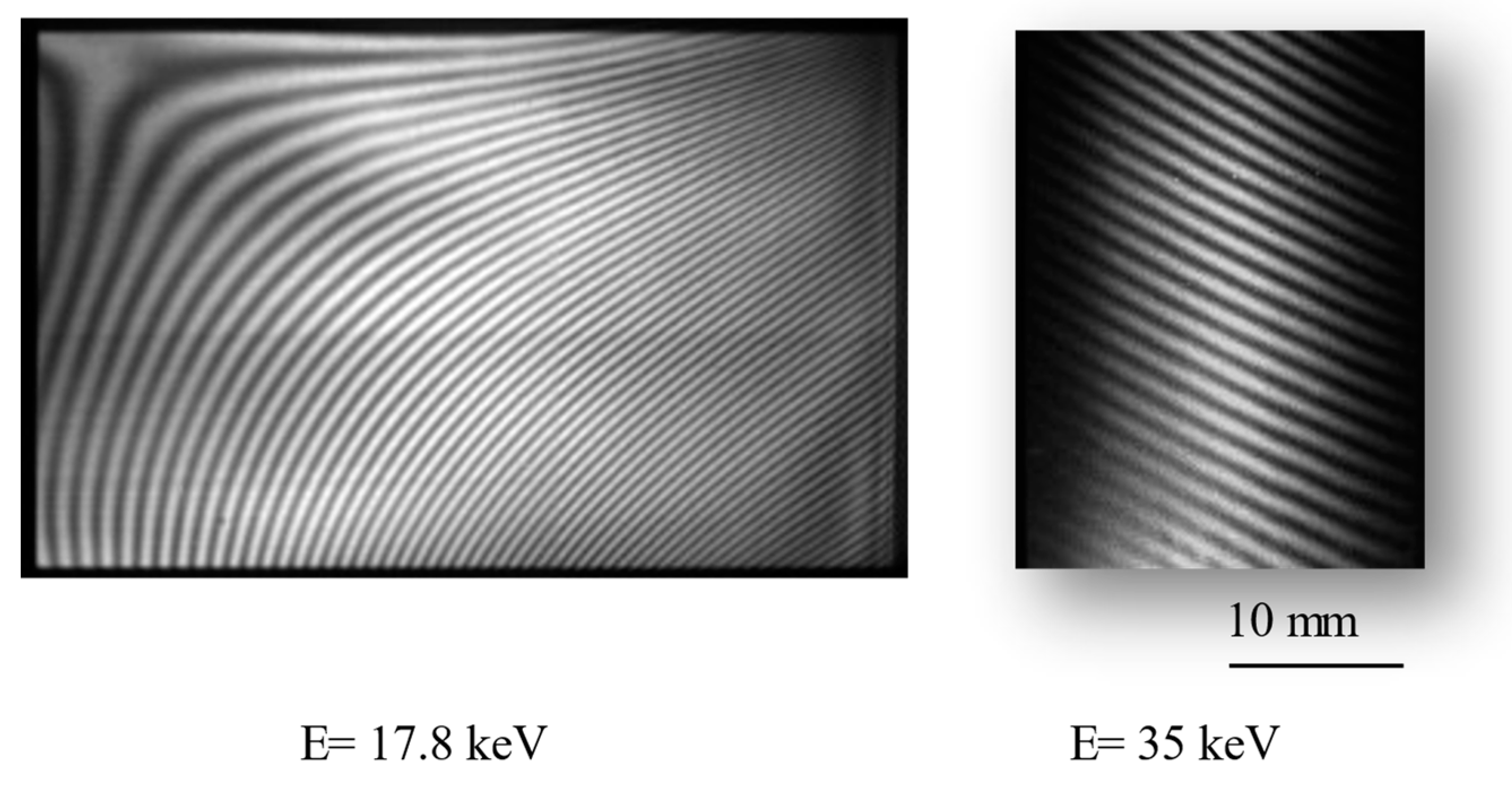
5. ST-CXI System and Example Observations
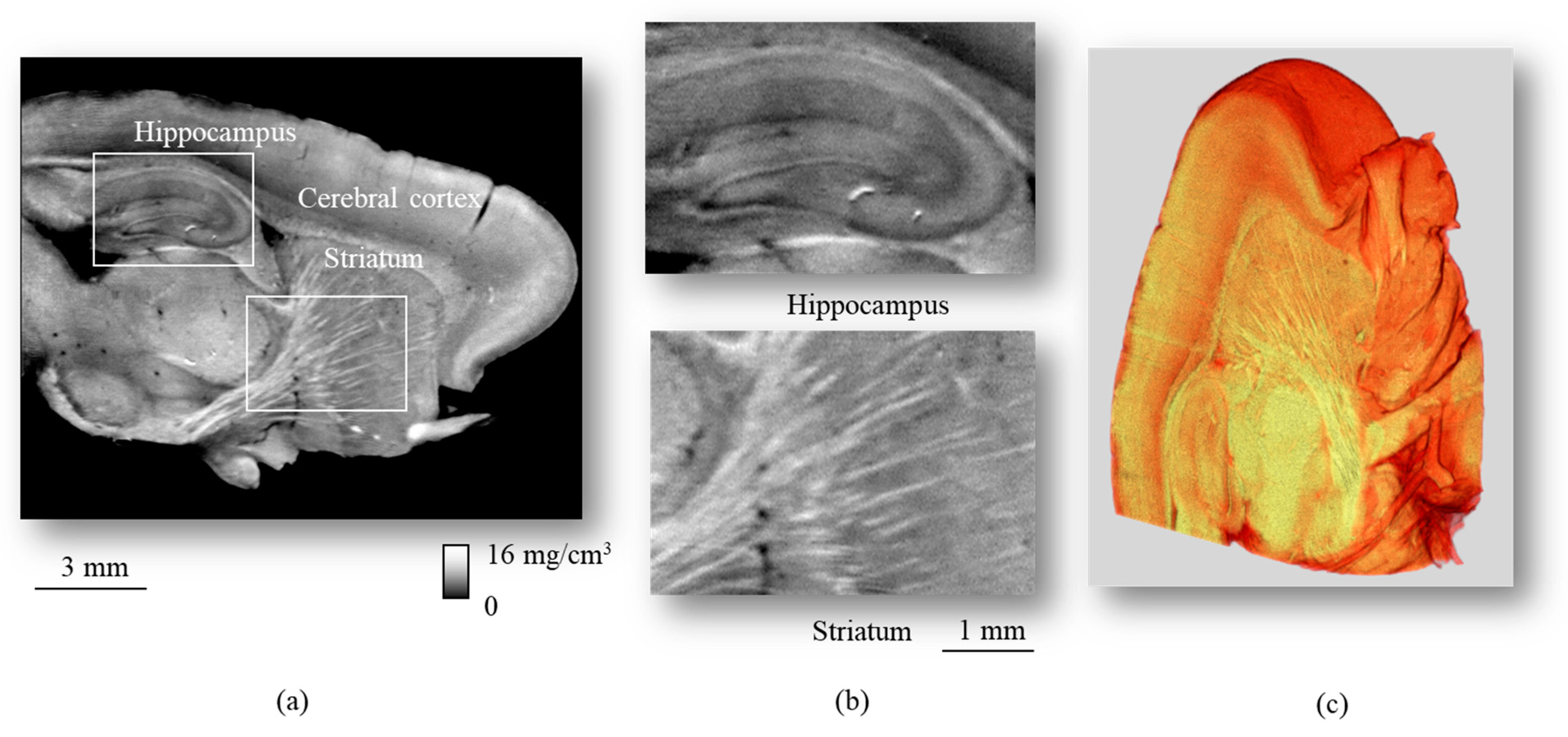
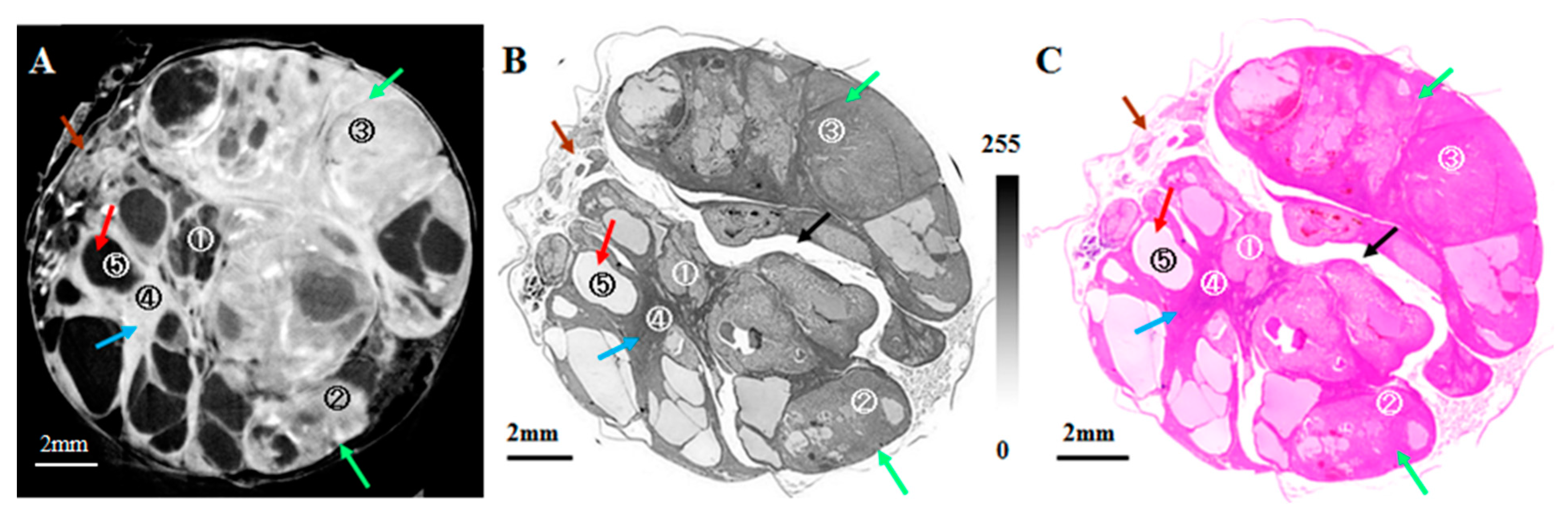
6. Application to Biomedical Research
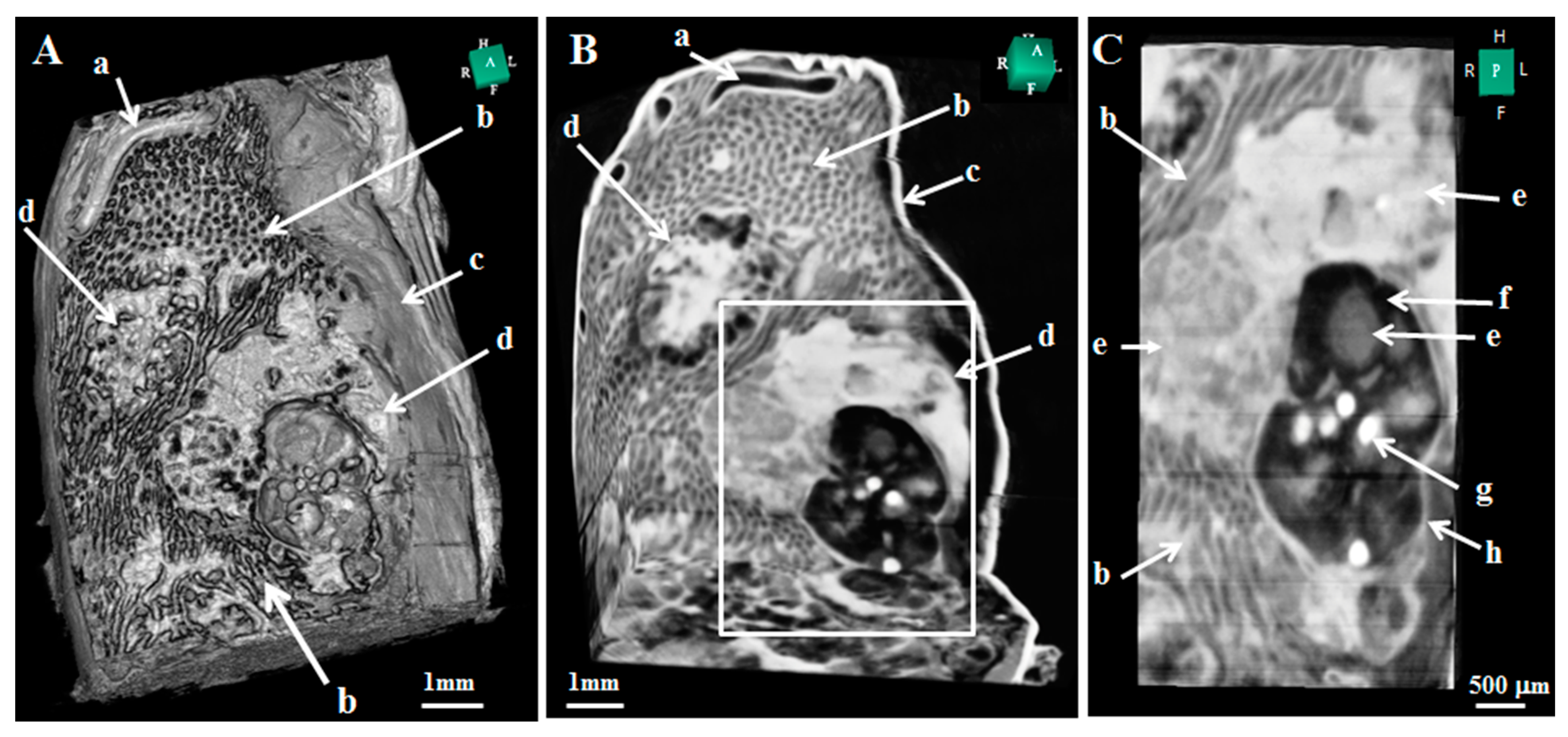
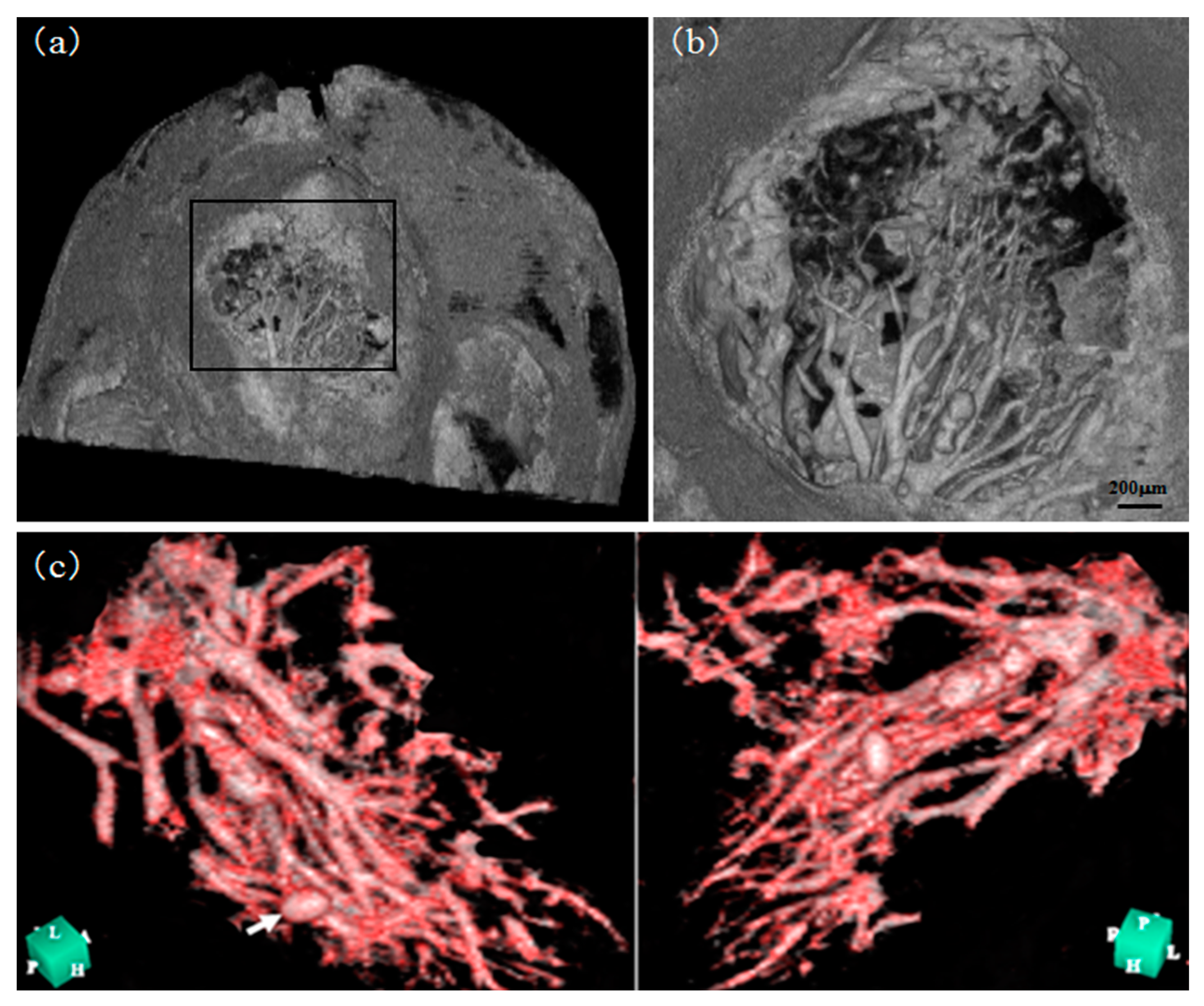
7. Embryonic Imaging by Phase-Contrast X-ray Imaging
8. Phase-Contrast X-ray Imaging in Various Thermal Environments
9. Industrial Applications (Operando Observation of Electrolyte Distribution)
10. Novel Imaging Using CXI
10.1. Coherence-Contrast Imaging
10.2. Effective Atomic Number (Zeff) Imaging
10.3. X-ray Thermography
11. Conclusions and Future Prospects
- Increasing SR energy (to 50 keV or higher) to increase X-ray transparency and thereby enable observation of thick and heavy samples such as biomedical samples, including calcification area, and semiconductor devices.
- Stabilization of phase detection by improvement of the image feedback system by installing a fast and sensitive X-ray imager to improve the density resolution below 0.1 mg/cm3. Improvement of the spatial resolution below 10 μm by thinning the crystal wafers to suppress the Borman fan effect (broaden X-ray beam in the wafer) [78].
- Biomedical: quantitative diagnosis based on absolute density changes with aging, disease, and drug administration, functional imaging using novel PCXI contrast agents, and quantitative evaluation of the effectiveness of thermotherapy by three-dimensional X-ray thermography.
- Embryonic imaging: given that the resolution of optical microscopy in the serial section is a few micrometers, CXI functions as a 3D microscope when the resolution of CXI reaches 10 μm. The whole body of a human embryo can be imaged with CXI, and 3D dynamic analysis of morphological changes during the embryonic stage is possible.
- Earth environment: observation of the decomposition process of clathrate hydrate, evaluation of the relationship between crystallinity and density combined with XRD, and three-dimensional observation of phase transitions due to temperature change.
- Industrial: Operand (in situ) observation of ion distribution in an electrolyte of various types of batteries for the optimization of their operation and charging/discharging conditions. Visualization of the internal temperature of devices such as LEDs and power semiconductor devices for optimal operation.
- Thermal engineering: visualization of dynamic thermal propagation (phonon propagation) in various materials such as thermoelectric conversion devices and laser ablation using a novel ps time-resolved pumps and probes method combining heating by pulsed laser irradiation and X-ray thermography using an X-ray free-electron laser [82].
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Momose, A.; Fukuda, J. Phase-contrast radiographs of nonstained rat cerebellar specimen. Med. Phys. 1995, 22, 375–379. [Google Scholar] [CrossRef]
- Bonse, U.; Hart, M. An X-ray Interferometer. Appl. Phys. Lett. 1965, 6, 155–156. [Google Scholar] [CrossRef]
- Momose, A. Demonstration of phase-contrast X-ray computed tomography using an X-ray interferometer. Nucl. Instrum. Methods Phys. Res. Sect. A 1995, 352, 622–628. [Google Scholar] [CrossRef]
- Davis, T.J.; Gao, D.; Gureyev, T.E.; Stevenson, A.W.; Wilkins, S.W. Phase-contrast imaging of weakly absorbing materials using hard X-rays. Nature 1995, 373, 595–598. [Google Scholar] [CrossRef]
- Momose, A.; Kawamoto, S.; Koyama, I.; Hamaishi, Y.; Takai, K.; Suzuki, Y. Demonstration of X-ray Talbot Interferometry. Jpn. J. Appl. Phys. 2003, 42, L866–L868. [Google Scholar] [CrossRef]
- Snigirev, A.; Snigireva, I.; Kohn, V.; Kuznetsov, S.; Schelokov, I. On the possibilities high-energy synchrotron of X-ray phase contrast microimaging radiation. Rev. Sci. Instrum. 1995, 66, 5486–5492. [Google Scholar] [CrossRef]
- Yoneyama, A.; Baba, R.; Lwin, T.T.; Kawamoto, M. Four-type phase-contrast X-ray imaging at SAGA Light Source. J. Phys. Conf. Ser. 2020, 2380, 012117. [Google Scholar] [CrossRef]
- Yoneyama, A.; Baba, R.; Hyodo, K.; Takeda, T. Quantitative comparison of performance of absorption, Talbot interferometric, and crystal X-ray interferometric imaging. In Proceedings of the European Congress of Radiology 2015, Vienna, Austria, 4–8 March 2015. [Google Scholar]
- Yoneyama, A.; Wu, J.; Hyodo, K.; Takeda, T. Quantitative comparison of imaging performance of X-ray interferometric imaging and diffraction enhanced imaging. Med. Phys. 2008, 35, 4724–4734. [Google Scholar] [CrossRef] [Green Version]
- Momose, A. X-ray phase imaging reaching clinical uses. Phys. Med. 2020, 79, 93–102. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Maikusa, H.; Takeda, Y. High-speed X-ray phase imaging and X-ray phase tomography with Talbot interferometer and white synchrotron radiation. Opt. Express 2009, 17, 12540–12545. [Google Scholar] [CrossRef] [PubMed]
- Yashiro, W.; Kamezawa, C.; Noda, D.; Kajiwara, K. Millisecond-order X-ray phase tomography with a fringe-scanning method. Appl. Phys. Express 2018, 11, 122501. [Google Scholar] [CrossRef]
- Vegso, K.; Wu, Y.; Takano, H.; Hoshino, M.; Momose, A. Development of pink-beam 4D phase CT for in-situ observation of polymers under infrared laser irradiation. Sci. Rep. 2019, 9, 7404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hagen, C.K.; Diemoz, P.C.; Endrizzi, M.; Rigon, L.; Dreossi, D.; Arfelli, F.; Lopez, F.C.; Longo, R.; Olivo, A. Theory and preliminary experimental verification of quantitative edge illumination X-ray phase contrast tomography. Opt. Express 2014, 22, 7989–8000. [Google Scholar] [CrossRef]
- Bonse, U.; Hart, M. An X-ray interferometer with Bragg case beam splitting and beam recombination. Z. Phys. 1966, 194, 1–17. [Google Scholar] [CrossRef]
- Koyama, I.; Yoshikawa, H.; Momose, A. Phase-Contrast X-ray Imaging with a Triple-Bragg-Case Interferometer. Jpn. J. Appl. Phys. 2003, 42, L80–L82. [Google Scholar] [CrossRef]
- Bonse, U.; Hart, M. Combined Laue–Bragg-case X-ray interferometers. Acta Crystallogr. Sect. A 1968, 24, 240–245. [Google Scholar] [CrossRef]
- Fezzaa, K.; Lee, W.K. An X-ray interferometer with a large and variable path length difference. J. Appl. Crystallogr. 2001, 34, 166–171. [Google Scholar] [CrossRef] [Green Version]
- Yoneyama, A.; Momose, A.; Koyama, I. Feasibility Test of a Nonplanar Three-Beam Case X-ray Interferometer for High-Resolution Phase-Contrast X-ray Imaging. Jpn. J. Appl. Phys. 2002, 41, L161–L163. [Google Scholar] [CrossRef]
- Becker, P.; Bonse, U. The skew-symmetric two-crystal X-ray interferometer. J. Appl. Crystallogr. 1974, 7, 593–598. [Google Scholar] [CrossRef]
- Takeda, M.; Ina, H.; Kobayashi, S. Fourier-transform method of fringe-pattern analysis for computer-based topography and interferometry. J. Opt. Soc. Am. 1982, 72, 156. [Google Scholar] [CrossRef]
- Bruning, J.H.; Herriott, D.R.; Gallagher, J.E.; Rosenfeld, D.P.; White, A.D.; Brangaccio, D.J. Digital Wavefront Measuring Interferometer for Testing Optical Surfaces and Lenses. Appl. Opt. 1974, 13, 2693–2703. [Google Scholar] [CrossRef] [PubMed]
- Yashiro, W.; Terui, Y.; Kawabata, K.; Momose, A. On the origin of visibility contrast in X-ray Talbot interferometry. Opt. Express 2010, 18, 16890–16901. [Google Scholar] [CrossRef] [PubMed]
- Momose, A.; Takeda, T.; Itai, Y.; Hirano, K. Phase-contrast X-ray computed tomography for observing biological soft tissues. Nat. Med. 1996, 2, 473–475. [Google Scholar] [CrossRef]
- Takeda, T.; Momose, A.; Ueno, E.; Itai, Y. Phase-contrast X-ray CT image of breast tumor. J. Synchrotron Radiat. 1998, 5, 1133–1135. [Google Scholar] [CrossRef]
- Yoneyama, A.; Takeya, S.; Lwin, T.T.; Takamatsu, D.; Baba, R.; Konishi, K.; Fujita, R.; Kobayashi, K.; Shima, A.; Kawamoto, M.; et al. Advanced X-ray imaging at beamline 07 of the SAGA Light Source. J. Synchrotron Radiat. 2021, 28, 1966–1977. [Google Scholar] [CrossRef] [PubMed]
- Bonse, U.; Te Kaat, E. A two-crystal X-ray interferometer. Z. Phys. A Hadron. Nucl. 1968, 214, 16–21. [Google Scholar] [CrossRef]
- Momose, A.; Yoneyama, A.; Hirano, K. Operation of a Two-Crystal X-ray Interferometer at the Photon Factory. J. Synchrotron Radiat. 1997, 4, 311–312. [Google Scholar] [CrossRef]
- Yoneyama, A.; Takeda, T.; Tsuchiya, Y.; Wu, J.; Thet Thet, L.; Koizumi, A.; Hyodo, K.; Itai, Y. A phase-contrast X-ray imaging system—With a 60 × 30 mm field of view—Based on a skew-symmetric two-crystal X-ray interferometer. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2004, 523, 217–222. [Google Scholar] [CrossRef]
- Yoneyama, A.; Takeda, T.; Wu, J.; Lwin, T.T.; Hyodo, K.; Hirai, Y. 60 × 40-mm X-ray Interference Pattern Generated by Two-Crystal X-ray Interferometer. PF Activity Report 2004. 2005, Volume 262. Available online: http://pfwww.kek.jp/acr2004pdf/part_b/pf04b262.pdf (accessed on 1 April 2023).
- Yoneyama, A.; Takeda, T.; Tsuchiya, Y.; Wu, J.; Lwin, T.T.; Hyodo, K.; Hirai, Y. High-energy phase-contrast X-ray imaging using a two-crystal X-ray interferometer. J. Synchrotron Radiat. 2005, 12, 534–536. [Google Scholar] [CrossRef]
- Yoneyama, A.; Nambu, A.; Ueda, K.; Yamada, S.; Takeya, S.; Hyodo, K.; Takeda, T. Phase-contrast X-ray imaging system with sub-mg/cm3 density resolution. J. Phys. Conf. Ser. 2013, 425, 192007. [Google Scholar] [CrossRef]
- Yoneyama, A.; Momose, A.; Seya, E.; Hirano, K.; Takeda, T.; Itai, Y. Operation of a separated-type X-ray interferometer for phase-contrast X-ray imaging. Rev. Sci. Instrum. 1999, 70, 4582. [Google Scholar] [CrossRef]
- Yoneyama, A.; Momose, A.; Koyama, I.; Seya, E.; Takeda, T.; Itai, Y.; Hirano, K.; Hyodo, K. Large-area phase-contrast X-ray imaging using a two-crystal X-ray interferometer. J. Synchrotron Radiat. 2002, 8, 277. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jud, C.; Schaff, F.; Zanette, I.; Wolf, J.; Fehringer, A.; Pfeiffer, F. Dentinal tubules revealed with X-ray tensor tomography. Dent. Mater. 2016, 32, 1189–1195. [Google Scholar] [CrossRef]
- Takeda, T.; Momose, A.; Hirano, K.; Haraoka, S.; Watanabe, T.; Ita, Y. Human Carcinoma: Early Experience with PhaseContrast X-ray CT with Synchrotron Radiation— Comparative Specimen Study with Optical Microscopy. Radiology 2000, 214, 298. [Google Scholar] [CrossRef]
- Stampanoni, M.; Groso, A.; Isenegger, A.; Mikuljan, G.; Chen, Q.; Bertrand, A.; Henein, S.; Betemps, R.; Frommherz, U.; Böhler, P.; et al. Trends in synchrotron-based tomographic imaging: The SLS experience. SPIE 2006, 6318, 193–206. [Google Scholar]
- Pfeiffer, F.; Weitkamp, T.; Bunk, O.; David, C. Phase retrieval and differential phase-contrast imaging with low-brilliance X-ray sources. Nat. Phys. 2006, 2, 258–261. [Google Scholar] [CrossRef] [Green Version]
- Takeda, T.; Wu, J.; Lwin, T.T.; Yoneyama, A.; Hyodo, K.; Matsuda, Y.; Kose, K. Interferometer-based phase-contrast X-ray computed tomography of colon cancer specimens: Comparative study with 4.74-T magnetic resonance imaging and optical microscopy. J. Comput. Assist. Tomogr. 2007, 31, 214–217. [Google Scholar] [CrossRef]
- Lwin, T.-T.; Yoneyama, A.; Hara, A.; Ohbu, M.; Maruyama, H.; Taguchi, M.; Esashi, S.; Matsushima, T.; Terazaki, K.; Hyodo, K.; et al. Spontaneous brain tumor imaging of aged rat by crystal X-ray interferometer-based phase-contrast X-ray CT. Acta Radiol. Open 2016, 5, 205846011562695. [Google Scholar] [CrossRef] [Green Version]
- Thet Thet, L.; Yoneyama, A.; Imai, M.; Maruyama, H.; Hyodo, K.; Takeda, T. Testicular seminoma in the aged rat visualized by phase-contrast X-ray computed tomography. Acta Radiol. Open 2018, 7, 205846011880665. [Google Scholar] [CrossRef] [Green Version]
- Lwin, T.T.; Yoneyama, A.; Maruyama, H.; Takeda, T. Visualization Ability of Phase-Contrast Synchrotron-Based X-ray Imaging Using an X-ray Interferometer in Soft Tissue Tumors. Technol. Cancer Res. Treat. 2021, 20, 15330338211010121. [Google Scholar] [CrossRef]
- Takeda, T.; Momose, A.; Wu, J.; Yu, Q.; Zeniya, T.; Lwin, T.-T.; Yoneyama, A.; Itai, Y. Vessel Imaging by Interferometric Phase-Contrast X-ray Technique. Circulation 2002, 105, 1708–1712. [Google Scholar] [CrossRef]
- Wu, J.; Takeda, T.; Lwin, T.T.; Momose, A.; Sunaguchi, N.; Fukami, T.; Yuasa, T.; Akatsuka, T. Imaging renal structures by X-ray phase-contrast microtomography. Kidney Int. 2009, 75, 945–951. [Google Scholar] [CrossRef] [Green Version]
- Esashi, S.; Yoneyama, A.; Thet-Thet-Lwin; Matsushima, T.; Maruyama, H.; Hyodo, K.; Takeda, T. Fine inner imaging of rat’s spleen by phase-contrast X-ray CT. Med. Imaging Technol. 2014, 32, 338–341. [Google Scholar] [CrossRef]
- Noda-Saita, K.; Yoneyama, A.; Shitaka, Y.; Hirai, Y.; Terai, K.; Wu, J.; Takeda, T.; Hyodo, K.; Osakabe, N.; Yamaguchi, T.; et al. Quantitative analysis of amyloid plaques in a mouse model of Alzheimer’s disease by phase-contrast X-ray computed tomography. Neuroscience 2006, 138, 1205–1213. [Google Scholar] [CrossRef]
- Takeda, T.; Yoneyama, A.; Wu, J.; Lwin, T.T.; Tsuchiya, Y.; Hyodo, K. In-Vivo Imaging of Cancer Implanted in Nude Mice by Two-Crystal Interferometer-Based Phase-Contrast X-ray Computed Tomography. Jpn. J. Appl. Phys. 2004, 43, L1144–L1146. [Google Scholar] [CrossRef]
- Yoneyama, A.; Amino, N.; Mori, M.; Kudoh, M.; Takeda, T.; Hyodo, K.; Hirai, Y. Non-invasive and Time-Resolved Observation of Tumors Implanted in Living Mice by Using Phase-Contrast X-ray Computed Tomography. Jpn. J. Appl. Phys. 2006, 45, 1864–1868. [Google Scholar] [CrossRef]
- Yamaguchi, Y.; Yamada, S. The Kyoto Collection of Human Embryos and Fetuses: History and Recent Advancements in Modern Methods. Cells Tissues Organs 2018, 205, 314–319. [Google Scholar] [CrossRef] [PubMed]
- Nohara, A.; Owaki, N.; Matsubayashi, J.; Katsube, M.; Imai, H.; Yoneyama, A.; Yamada, S.; Kanahashi, T.; Takakuwa, T. Morphometric analysis of secondary palate development in human embryos. J. Anat. 2022, 241, 1287–1302. [Google Scholar] [CrossRef] [PubMed]
- Osaka, M.; Ishikawa, A.; Yamada, S.; Uwabe, C.; Imai, H.; Matsuda, T.; Yoneyama, A.; Takeda, T.; Takakuwa, T. Positional Changes of the Ocular Organs During Craniofacial Development. Anat. Rec. 2017, 300, 2107–2114. [Google Scholar] [CrossRef] [Green Version]
- Toyoda, S.; Shiraki, N.; Yamada, S.; Uwabe, C.; Imai, H.; Matsuda, T.; Yoneyama, A.; Takeda, T.; Takakuwa, T. Morphogenesis of the Inner Ear at Different Stages of Normal Human Development. Anat. Rec. 2015, 298, 2081–2090. [Google Scholar] [CrossRef] [Green Version]
- Suzuki, Y.; Matsubayashi, J.; Ji, X.; Yamada, S.; Yoneyama, A.; Imai, H.; Matsuda, T.; Aoyama, T.; Takakuwa, T. Morphogenesis of the femur at different stages of normal human development. PLoS ONE 2019, 14, e0221569. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fujii, S.; Muranaka, T.; Matsubayashi, J.; Yamada, S.; Yoneyama, A.; Takakuwa, T. Bronchial tree of the human embryo: Categorization of the branching mode as monopodial and dipodial. PLoS ONE 2021, 16, e0245558. [Google Scholar] [CrossRef] [PubMed]
- Okuno, K.; Ishizu, K.; Matsubayashi, J.; Fujii, S.; Sakamoto, R.; Ishikawa, A.; Yamada, S.; Yoneyama, A.; Takakuwa, T. Rib Cage Morphogenesis in the Human Embryo: A Detailed Three-Dimensional Analysis. Anat. Rec. 2019, 302, 2211–2223. [Google Scholar] [CrossRef] [PubMed]
- Kanahashi, T.; Yamada, S.; Tanaka, M.; Hirose, A.; Uwabe, C.; Kose, K.; Yoneyama, A.; Takeda, T.; Takakuwa, T. A Novel Strategy to Reveal the Latent Abnormalities in Human Embryonic Stages from a Large Embryo Collection. Anat. Rec. 2016, 299, 8–24. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Takeya, S.; Honda, K.; Gotoh, Y.; Yoneyama, A.; Ueda, K.; Miyamoto, A.; Hondoh, T.; Hori, A.; Sun, D.; Ohmura, R.; et al. Diffraction-enhanced X-ray imaging under low-temperature conditions: Non-destructive observations of clathrate gas hydrates. J. Synchrotron Radiat. 2012, 19, 1038–1042. [Google Scholar] [CrossRef] [PubMed]
- Hassanpouryouzband, A.; Joonaki, E.; Vasheghani Farahani, M.; Takeya, S.; Ruppel, C.; Yang, J.; English, N.J.; Schicks, J.M.; Edlmann, K.; Mehrabian, H.; et al. Gas hydrates in sustainable chemistry. Chem. Soc. Rev. 2020, 49, 5225–5309. [Google Scholar] [CrossRef]
- Bender, M.; Sowers, T.; Brook, E. Gases in ice cores. Proc. Natl. Acad. Sci. 1997, 94, 8343–8349. [Google Scholar] [CrossRef] [Green Version]
- Takeya, S.; Nagaya, H.; Matsuyama, T.; Hondoh, T.; Lipenkov, V.Y. Lattice Constants and Thermal Expansion Coefficient of Air Clathrate Hydrate in Deep Ice Cores from Vostok, Antarctica. J. Phys. Chem. B 2000, 104, 668–670. [Google Scholar] [CrossRef]
- Takeya, S.; Honda, K.; Yoneyama, A.; Hirai, Y.; Okuyama, J.; Hondoh, T.; Hyodo, K.; Takeda, T. Observation of low-temperature object by phase-contrast X-ray imaging: Nondestructive imaging of air clathrate hydrates at 233K. Rev. Sci. Instrum. 2006, 77, 053705. [Google Scholar] [CrossRef] [Green Version]
- Takeya, S.; Honda, K.; Kawamura, T.; Yamamoto, Y.; Yoneyama, A.; Hirai, Y.; Hyodo, K.; Takeda, T. Imaging and density mapping of tetrahydrofuran clathrate hydrates by phase-contrast X-ray computed tomography. Appl. Phys. Lett. 2007, 90, 081920. [Google Scholar] [CrossRef]
- Takeya, S.; Gotoh, Y.; Yoneyama, A.; Hyodo, K.; Takeda, T. Observation of the growth process of icy materials in interparticle spaces: Phase-contrast X-ray imaging of clathrate hydrate. Can. J. Chem. 2015, 93, 983–987. [Google Scholar] [CrossRef]
- Takeya, S.; Muromachi, S.; Yoneyama, A. X-ray Imaging of Clathrate Hydrates as Gas Storage Materials: Absorption Contrast of Low-Density and Low-Absorption Materials Using Energy-Dependent X-ray Computed Tomography. Energy Fuels 2022, 36, 10659–10666. [Google Scholar] [CrossRef]
- Takeya, S.; Yoneyama, A.; Ueda, K.; Hyodo, K.; Takeda, T.; Mimachi, H.; Takahashi, M.; Iwasaki, T.; Sano, K.; Yamawaki, H.; et al. Nondestructive Imaging of Anomalously Preserved Methane Clathrate Hydrate by Phase Contrast X-ray Imaging. J. Phys. Chem. C 2011, 115, 16193–16199. [Google Scholar] [CrossRef]
- Takeya, S.; Yoneyama, A.; Ueda, K.; Mimachi, H.; Takahashi, M.; Sano, K.; Hyodo, K.; Takeda, T.; Gotoh, Y. Anomalously Preserved Clathrate Hydrate of Natural Gas in Pellet Form at 253 K. J. Phys. Chem. C 2012, 116, 13842–13848. [Google Scholar] [CrossRef]
- Mo, J.; Guo, E.; McCartney, D.G.; Eastwood, D.S.; Bent, J.; Van Dalen, G.; Schuetz, P.; Rockett, P.; Lee, P.D. Time-Resolved Tomographic Quantification of the Microstructural Evolution of Ice Cream. Materials 2018, 11, 2031. [Google Scholar] [CrossRef] [Green Version]
- Takeichi, Y.; Inami, N.; Suga, H.; Miyamoto, C.; Ueno, T.; Mase, K.; Takahashi, Y.; Ono, K. Design and performance of a compact scanning transmission X-ray microscope at the Photon Factory. Rev. Sci. Instrum. 2016, 87, 013704. [Google Scholar] [CrossRef]
- Chang, H.J.; Ilott, A.J.; Trease, N.M.; Mohammadi, M.; Jerschow, A.; Grey, C.P. Correlating Microstructural Lithium Metal Growth with Electrolyte Salt Depletion in Lithium Batteries Using 7Li MRI. J. Am. Chem. Soc. 2015, 137, 15209–15216. [Google Scholar] [CrossRef]
- Takamatsu, D.; Yoneyama, A.; Yabuuchi, S.; Hayakawa, J.; Hirano, T. Quantitative visualization of ion and thermal distributions in electrolytes during operation of electrochemical devices by Operando phase-contrast X-ray imaging. J. Mater. Res. 2022, 37, 1576–1590. [Google Scholar] [CrossRef]
- Takamatsu, D.; Yoneyama, A.; Asari, Y.; Hirano, T. Quantitative Visualization of Salt Concentration Distributions in Lithium-Ion Battery Electrolytes during Battery Operation Using X-ray Phase Imaging. J. Am. Chem. Soc. 2018, 140, 1608–1611. [Google Scholar] [CrossRef]
- Takamatsu, D.; Hirano, T.; Yoneyama, A.; Kimura, T.; Harada, M.; Terada, M.; Okoshi, T. In operando visualization of electrolyte stratification dynamics in lead-acid battery using phase-contrast X-ray imaging. Chem. Commun. 2020, 56, 9553–9556. [Google Scholar] [CrossRef]
- Yoneyama, A.; Takeda, T.; Tsuchiya, Y.; Wu, J.; Lwin, T.-T.; Hyodo, K. Coherence-contrast X-ray imaging based on X-ray interferometry. Appl. Opt. 2006, 44, 3258. [Google Scholar] [CrossRef] [PubMed]
- Yoneyama, A.; Hyodo, K.; Takeda, T. Feasibility test of Zeff imaging using X-ray interferometry. Appl. Phys. Lett. 2013, 103, 204108. [Google Scholar] [CrossRef]
- Yoneyama, A. Phase-contrast X-ray Imaging and Its Applications. In Advances in X-ray Chemical Analysis; The Japan Society for Analytical Chemistry: Tokyo, Japan, 2018; Volume 49. [Google Scholar]
- Yoneyama, A.; Iizuka, A.; Fujii, T.; Hyodo, K.; Hayakawa, J. Three-dimensional X-ray thermography using phase-contrast imaging. Sci. Rep. 2018, 8, 12674. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yoneyama, A.; Baba, R.; Hyodo, K. Visualization of thermal flow inside a biomedical sample using novel X-ray thermography. In Proceedings of the ECR 2020, Vienna, Austria, 11–15 March 2020. [Google Scholar] [CrossRef]
- Hirano, K.; Momose, A. Development of an X-ray Interferometer for High-Resolution Phase-Contrast X-ray Imaging. Jpn. J. Appl. Phys. 1999, 38, L1556. [Google Scholar] [CrossRef] [Green Version]
- Miki, H.; Yoneyama, A.; Hirano, K. Visualizing morphological structures of rice grains in precooked products using synchrotron radiation X-ray phase-contrast computed tomography. Food Funct. 2023, 14, 87–93. [Google Scholar] [CrossRef]
- Kamezawa, C.; Numano, T.; Kawabata, Y.; Kanetaka, H.; Furuya, M.; Yokota, K.; Kato, H.; Yoneyama, A.; Hyodo, K.; Yashiro, W. X-ray elastography by visualizing propagating shear waves. Appl. Phys. Express 2020, 13, 042004. [Google Scholar] [CrossRef]
- Takeya, S.; Nakano, K.; Thammawong, M.; Umeda, H.; Yoneyama, A.; Takeda, T.; Hyodo, K.; Matsuo, S. CO2 processing and hydration of fruit and vegetable tissues by clathrate hydrate formation. Food Chem. 2016, 205, 122–128. [Google Scholar] [CrossRef]
- Yoneyama, A.; Baba, R.; Takamatsu, D.; Kamezawa, C.; Inoue, I.; Osaka, T.; Owada, S.; Yabashi, M. Feasibility study of interferometric phase-contrast X-ray imaging using the hard-X-ray free-electron laser of the SPring-8 Angstrom Compact Free-Electron Laser. J. Synchrotron Radiat. 2020, 27, 1358–1361. [Google Scholar] [CrossRef]




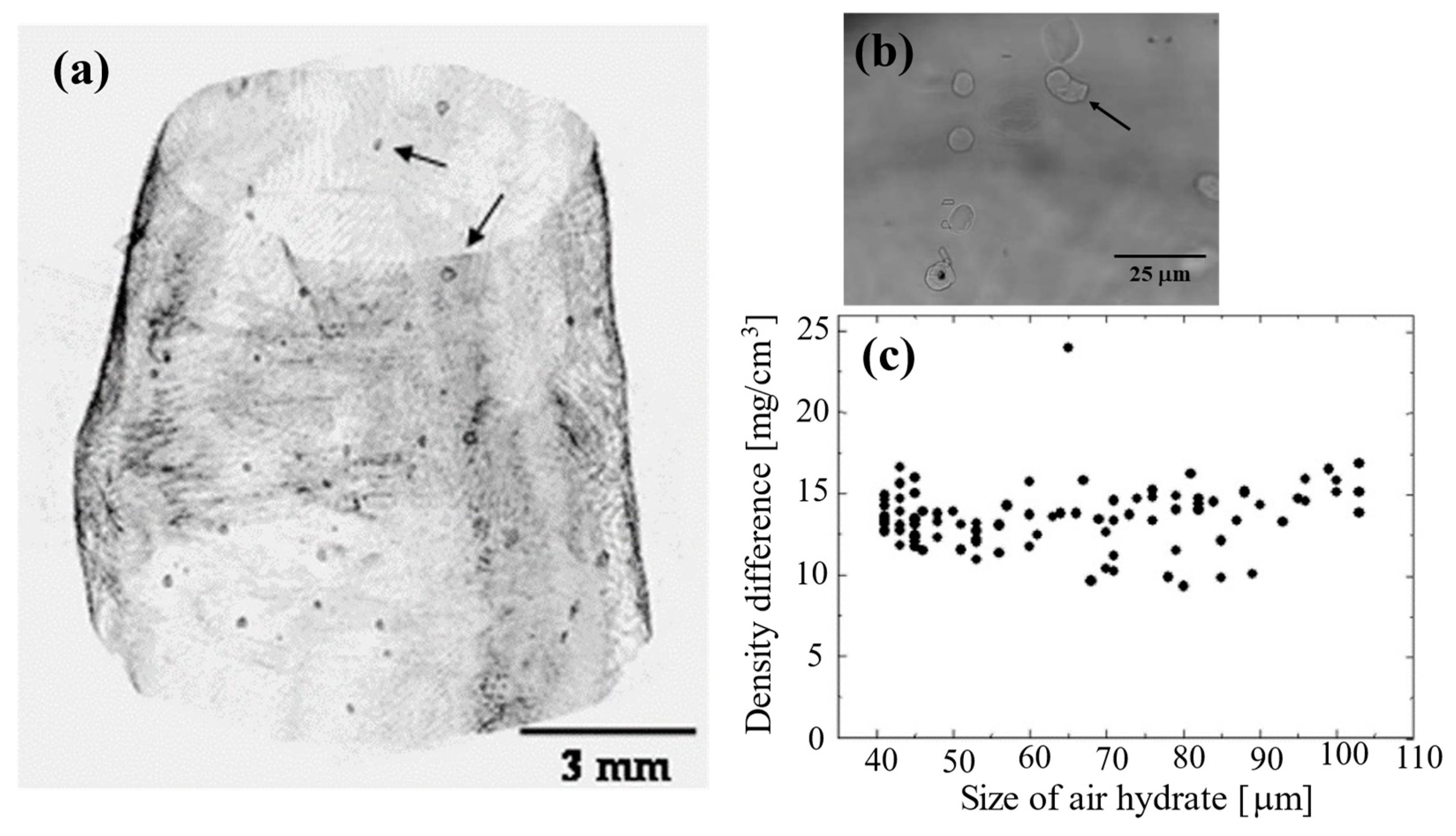
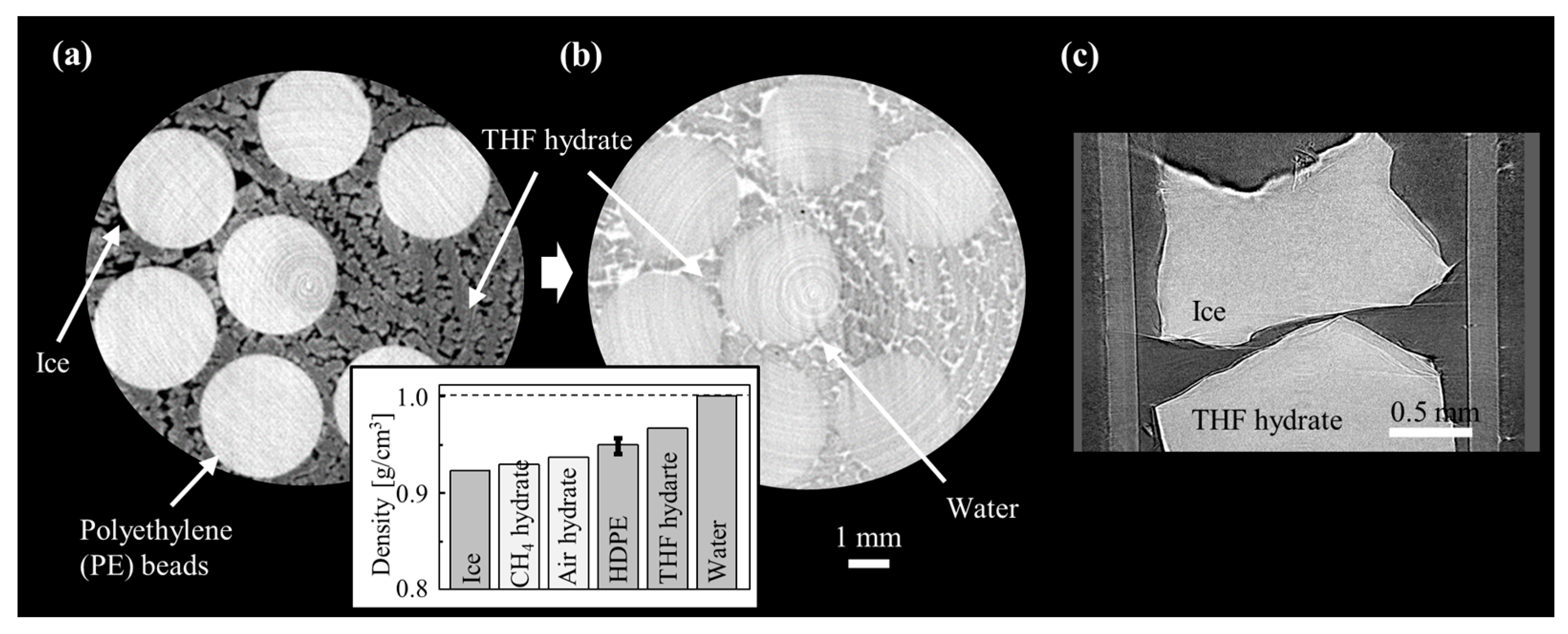

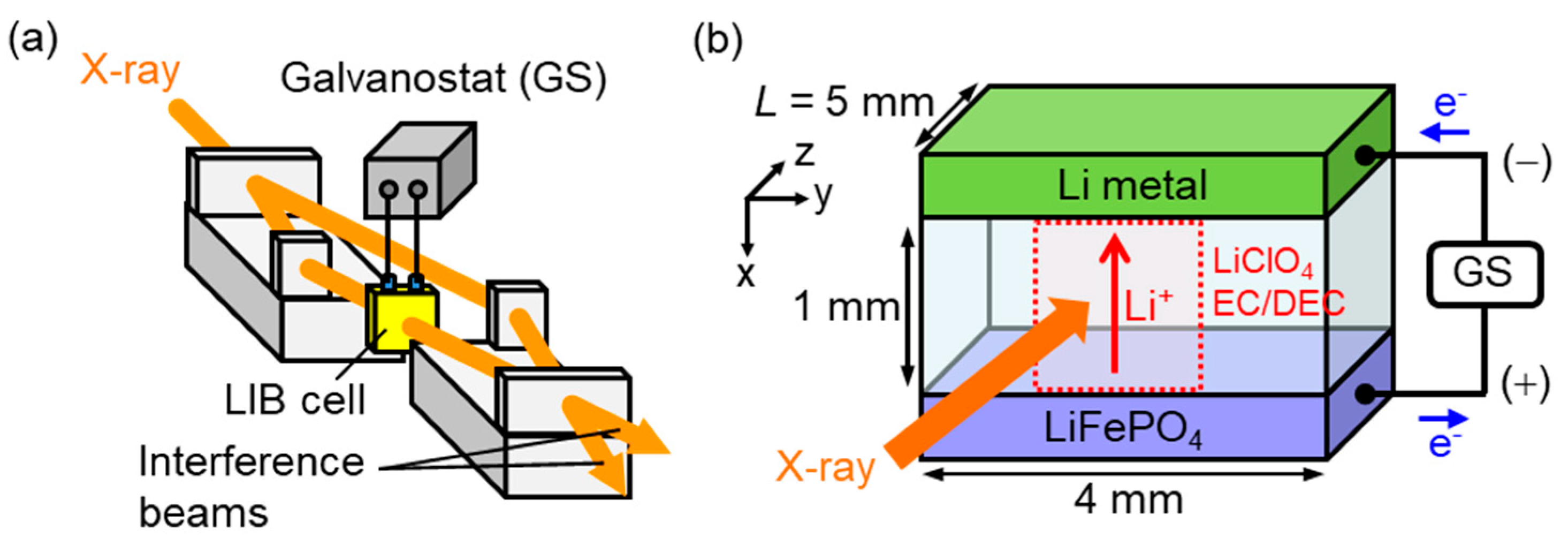


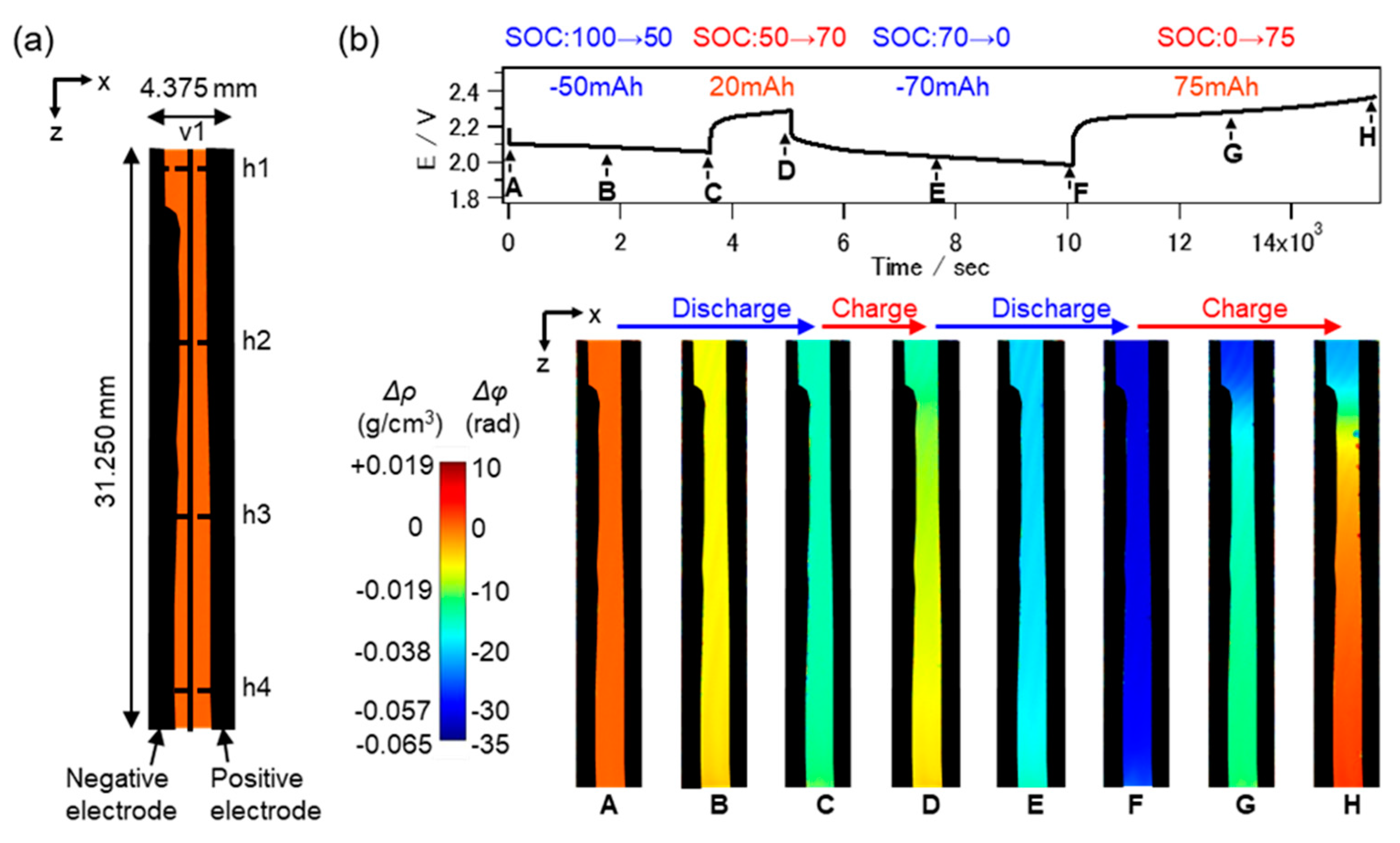

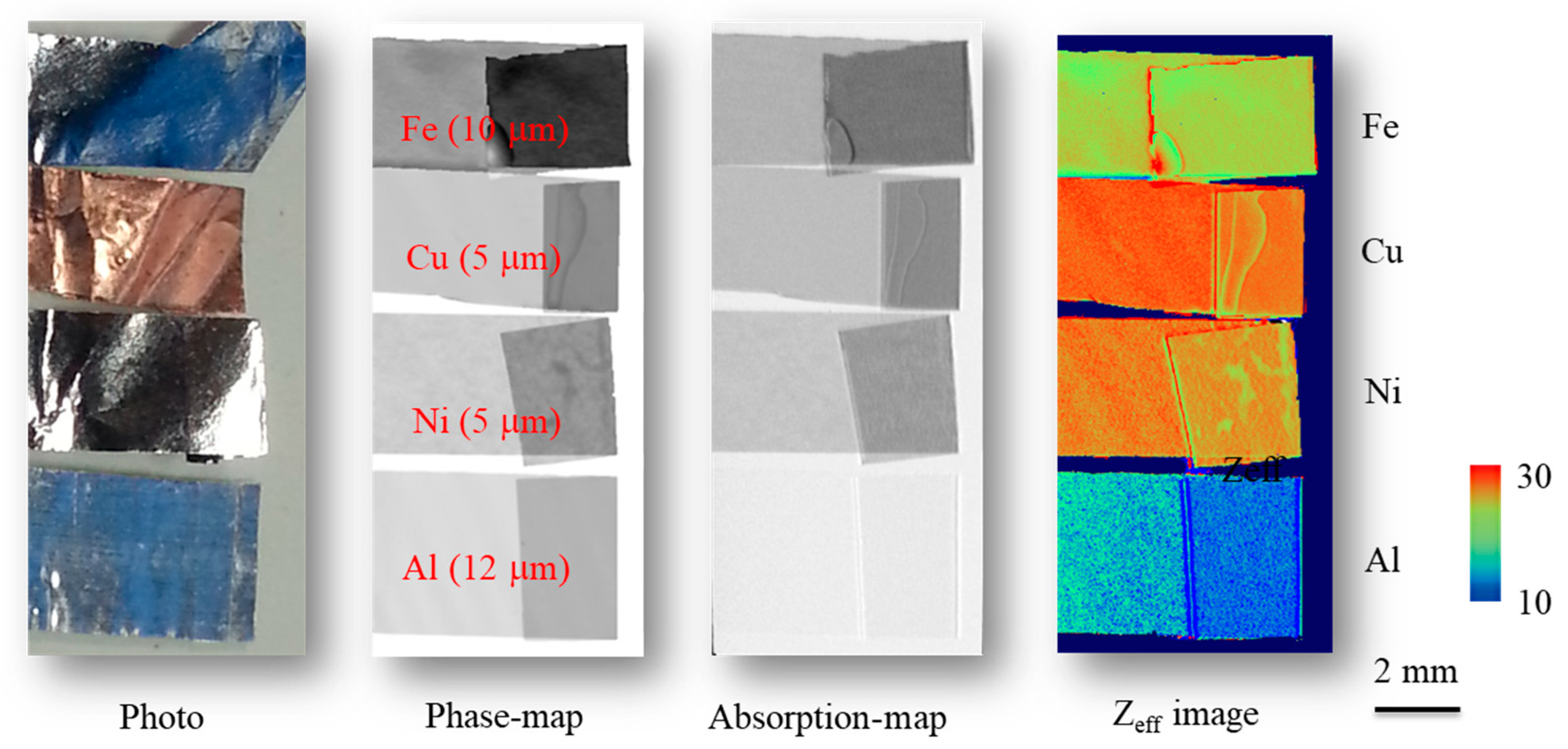
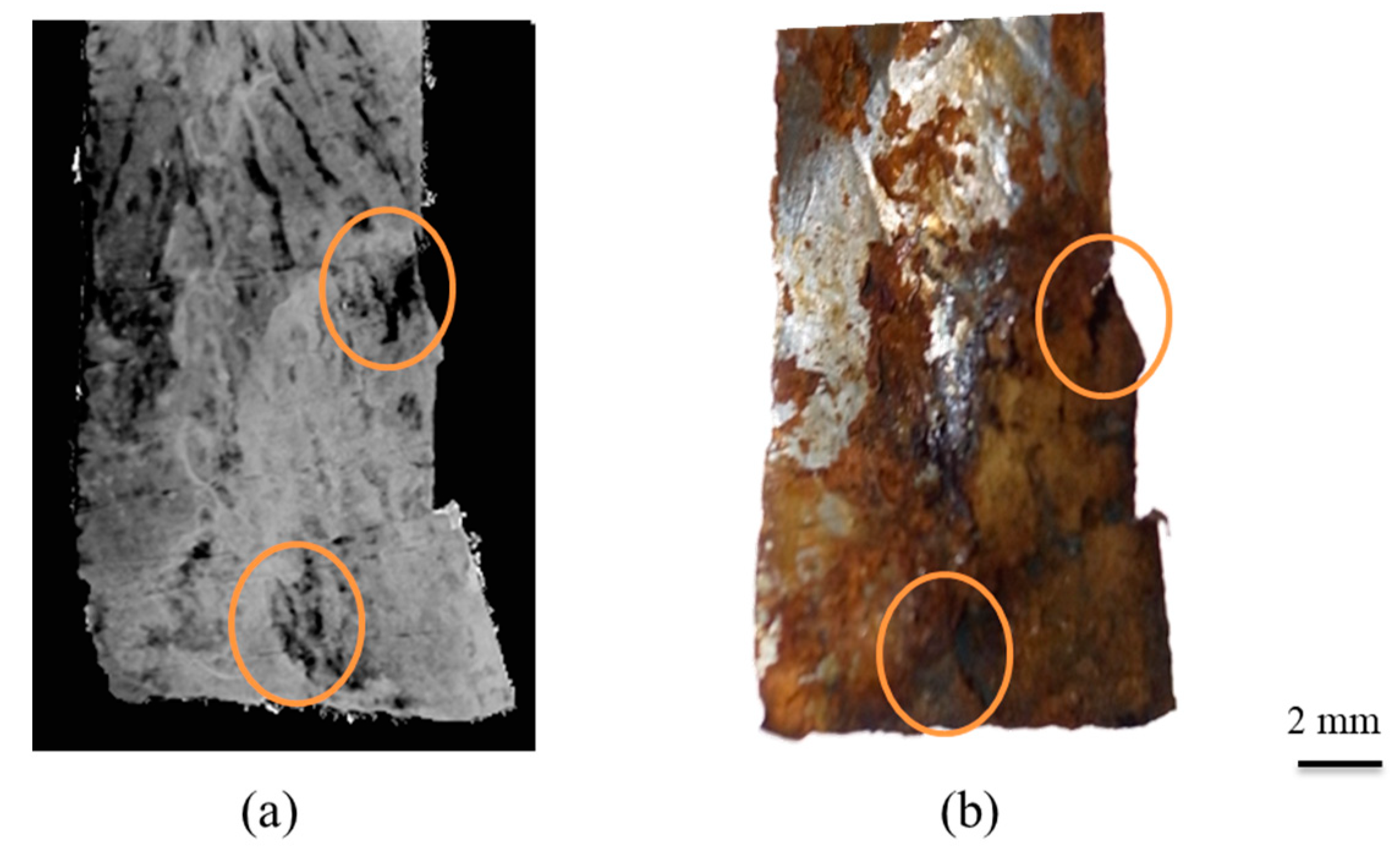
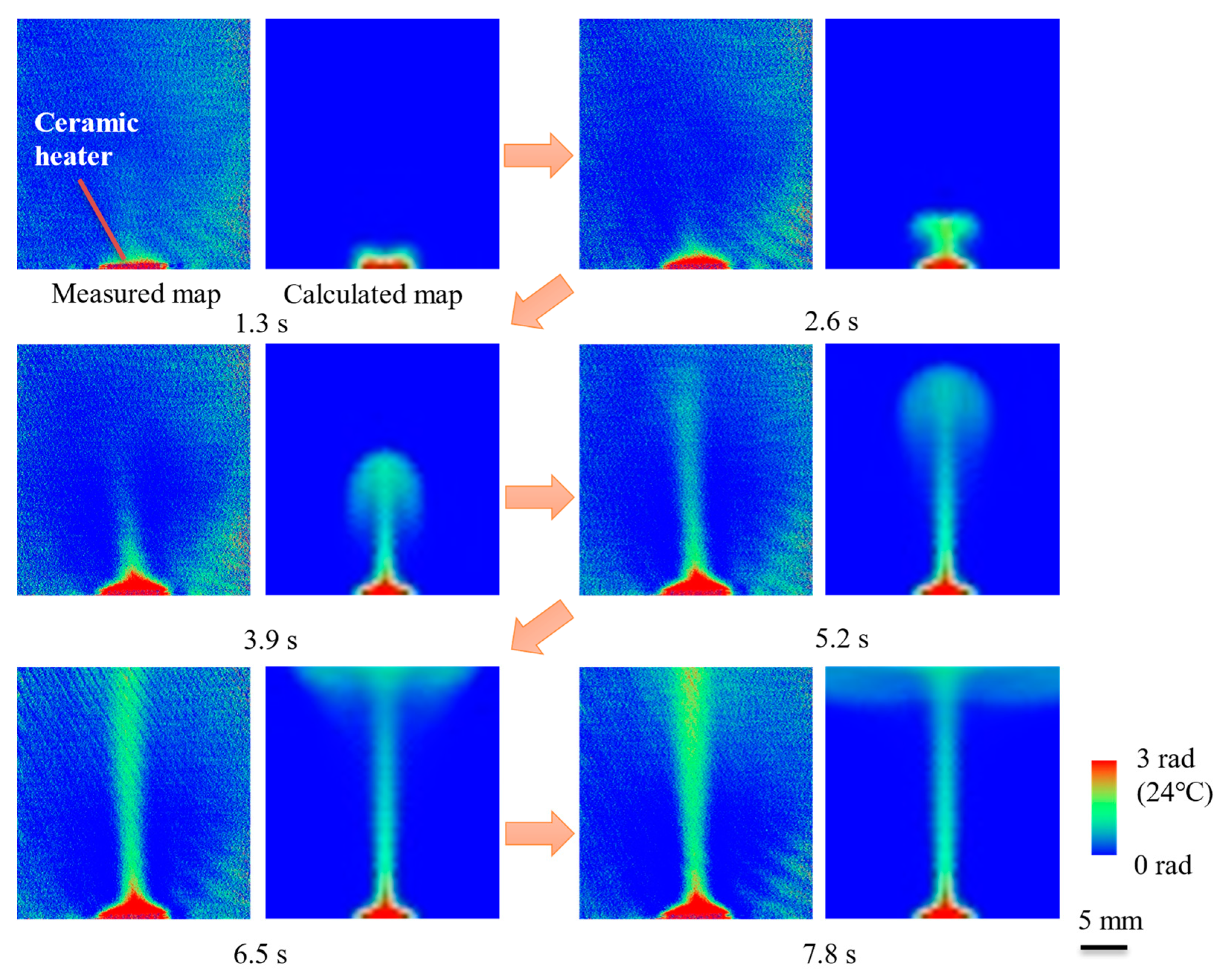
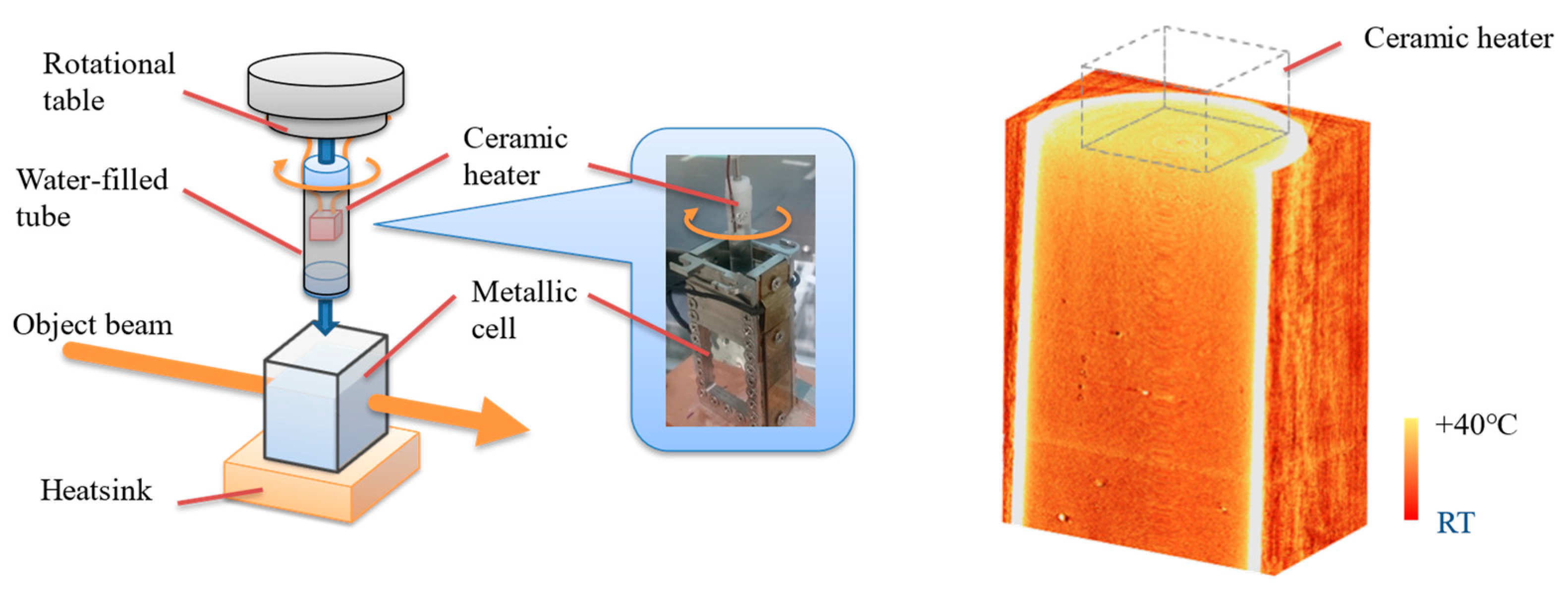
| Method | Detecting Physical Value | Density Resolution | Dynamic Range of Density | Spatial Resolution | Typical Measurement Time for CT |
|---|---|---|---|---|---|
| Crystal-based X-ray interferometry (CXI) | cos (p) | High | Narrow | ~30 μm | ~3 h |
| Diffraction-enhanced imaging (DEI) | Middle | Wide | ~10 μm | <30 min | |
| Grating-based X-ray interferometry (GXI) | Low | Middle | ~10 μm | <1 min for white synchrotron radiation 2 h for mono. synchrotron radiation | |
| Propagation-based imaging (PI) | Low | Wide | ~3 μm | ~1 h |
| No | Field of View | Imaging Capability | R&D Target |
|---|---|---|---|
| 1 | 25 × 15 mm | Interference pattern | Configuration and driving system of positional tables for prad rotational accuracy [33] |
| 2 | 30 × 30 mm | Phase map | Feedback system to suppress drift rotation and sample positioner [34] |
| 3 | 40 × 60 mm | Phase-contrast CT | Image feedback system and sample manipulation for CT [29] |
| X-ray Energy | 15–35 keV | |
| Field of view | 50 × 35 mm at 17.8 keV 24 × 35 mm at 35 keV | |
| CT density resolution | <0.5 mg/cm3 at 17.8 keV | |
| Typical CT measurement time | >2 h | |
| X-ray imager | Type | Fiber-coupled |
| Pixel size | 6.5 μm | |
| Pixel number | 2560 × 2160 | |
| Frame rate | 50 fps for full image | |
| CXI | 4.74-T MRI | |
|---|---|---|
| Volumetric resolution | (0.018)3 mm3 | (0.075)3 mm3 |
| Acquisition time | 2 h | 1.8 h |
| SNR * in nonnecrotic cancer lesions | 48.4 | 12.1 |
| CXI | 7-T MRI | |
|---|---|---|
| Volumetric resolution | (0.013)3 mm3 | (0.036)3 mm3 |
| Acquisition time | 2 h | 69 h |
| CXI | Absorption-Contrast CT | |
|---|---|---|
| Photon energy [keV] | 35 | 10 |
| Volumetric resolution | (0.013)3 mm3 | (0.0013)3 mm3 |
| Acquisition time | 0.5 h | 0.3 h |
| Identification of water and ice | Possible | Impossible |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yoneyama, A.; Takamatsu, D.; Lwin, T.-T.; Yamada, S.; Takakuwa, T.; Hyodo, K.; Hirano, K.; Takeya, S. Crystal-Based X-ray Interferometry and Its Application to Phase-Contrast X-ray Imaging, Zeff Imaging, and X-ray Thermography. Appl. Sci. 2023, 13, 5424. https://doi.org/10.3390/app13095424
Yoneyama A, Takamatsu D, Lwin T-T, Yamada S, Takakuwa T, Hyodo K, Hirano K, Takeya S. Crystal-Based X-ray Interferometry and Its Application to Phase-Contrast X-ray Imaging, Zeff Imaging, and X-ray Thermography. Applied Sciences. 2023; 13(9):5424. https://doi.org/10.3390/app13095424
Chicago/Turabian StyleYoneyama, Akio, Daiko Takamatsu, Thet-Thet Lwin, Shigehito Yamada, Tetsuya Takakuwa, Kazuyuki Hyodo, Keiichi Hirano, and Satoshi Takeya. 2023. "Crystal-Based X-ray Interferometry and Its Application to Phase-Contrast X-ray Imaging, Zeff Imaging, and X-ray Thermography" Applied Sciences 13, no. 9: 5424. https://doi.org/10.3390/app13095424
APA StyleYoneyama, A., Takamatsu, D., Lwin, T.-T., Yamada, S., Takakuwa, T., Hyodo, K., Hirano, K., & Takeya, S. (2023). Crystal-Based X-ray Interferometry and Its Application to Phase-Contrast X-ray Imaging, Zeff Imaging, and X-ray Thermography. Applied Sciences, 13(9), 5424. https://doi.org/10.3390/app13095424





