The Nonlinear Lateral Stability of a Four-Axle Freight Car with Y25 Bogies and Measures to Improve Its Faults
Abstract
:1. Introduction
1.1. The Literature Survey
1.2. The Fundamental Theoretical Considerations
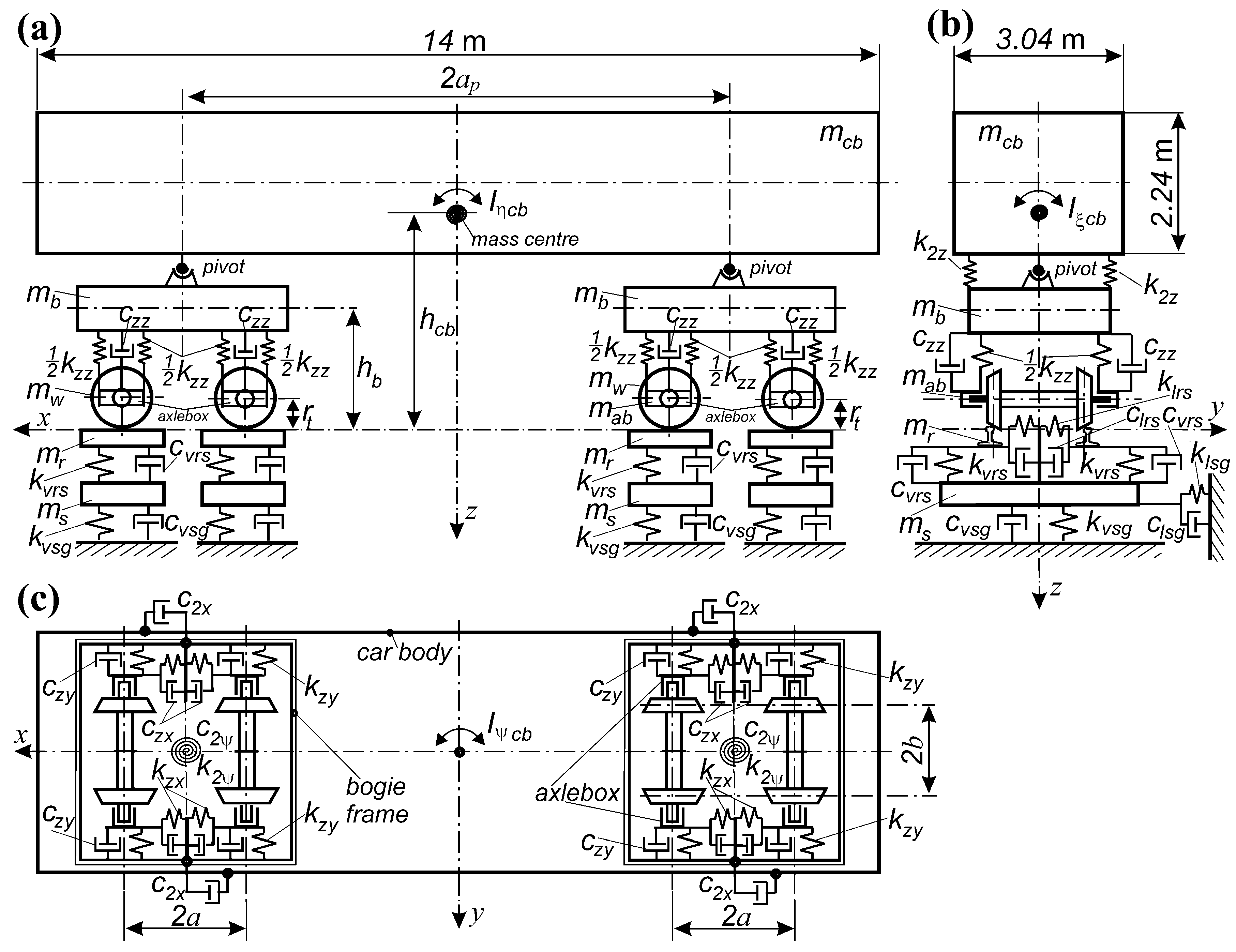
2. The Approach to Stability and the Corresponding Model
3. The Method of Research
4. Results of the Research
4.1. The Empty Car Analysis
4.2. Loaded Car Analysis
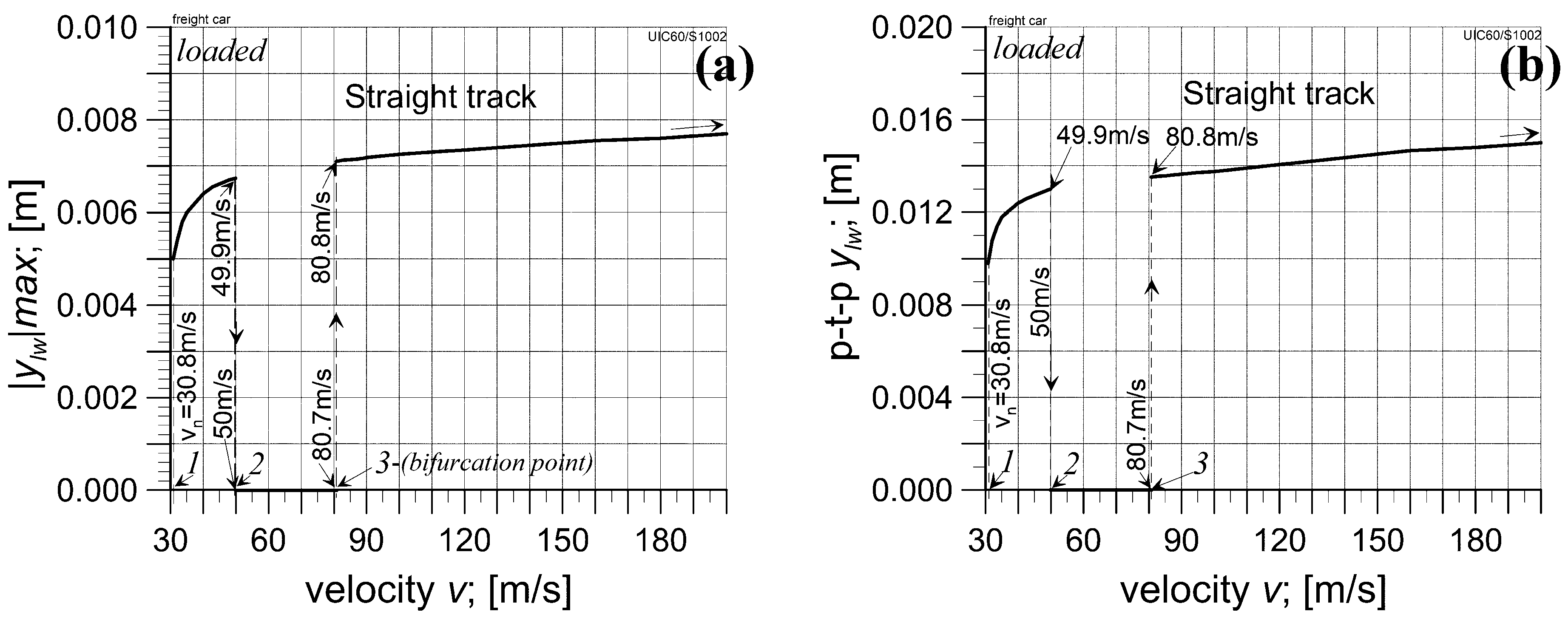
4.2.1. Analysis on a Straight Track
4.2.2. Analysis on Curved Tracks
4.3. Empty Car Analysis at Reduced kzy Values
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Notation | Description | Unit | Value | |
|---|---|---|---|---|
| Empty | Loaded | |||
| mcb | Vehicle body mass | kg | 11,000 | 72,000 |
| mb | Bogie frame mass | kg | 1600 | |
| mw | Wheelset mass | kg | 1400 | |
| mab | Axle box mass | kg | 100 | |
| mtb | Bogie’s total mass (mb + 2mw + 4mab) | kg | 4800 | |
| Iξcb | Body’s moment of inertia; longitudinal axis | kg·m2 | 17,300 | 90,055 |
| Iηcb | Body’s moment of inertia; lateral axis | kg·m2 | 188,500 | 1,210,606 |
| Iψcb | Body’s moment of inertia; vertical axis | kg·m2 | 188,140 | 1,231,450 |
| Iξb | Bogie frame’s moment of inertia; longitudinal axis | kg·m2 | 790 | |
| Iηb | Bogie frame’s moment of inertia; lateral axis | kg·m2 | 1000 | |
| Iψb | Bogie frame’s moment of inertia; vertical axis | kg·m2 | 1090 | |
| Iξw | Wheelset’s moment of inertia; longitudinal axis | kg·m2 | 747 | |
| Iηw | Wheelset’s moment of inertia; lateral axis | kg·m2 | 131 | |
| Iψw | Wheelset’s moment of inertia; vertical axis | kg·m2 | 747 | |
| kzz | Vertical stiffness of the primary suspension | kN/m | 1017 | 2280 |
| kzy | Lateral stiffness of the primary suspension | kN/m | 3890 | 5560 |
| kzx | Longitudinal stiffness of the primary suspension | kN/m | 12,000 | 12,000 |
| czz | Vertical damping of the primary suspension | kN·s/m | 7 | 123.3 |
| czy | Lateral damping of the primary suspension | kN·s/m | 42 | 138 |
| czx | Longitudinal damping of the primary suspension | kN·s/m | 100 | |
| k2z | Vertical stiffness of the bogie frame—car body side bearer | kN/m | 22,500 | |
| c2x | Longitudinal damping of the bogie frame—car body side bearer | kN·s/m | 6 | 10 |
| k2ψ | Torsional stiffness between the bogie frame and car body | kN·m/rad | 20 | |
| c2ψ | Torsional damping between the bogie frame and car body | kN·m·s/rad | 0.5 | |
| ap | Half of bogies’ pivot-t-pivot distance | m | 4.5 | |
| a | Semi-wheel base | m | 0.9 | |
| tc | Half the distance between the wheelset’s wheels’ rolling circles | m | 0.75 | |
| hb | Vertical distance between bogie frame centre of mass and track plane | m | 0.69 | |
| hcb | Vertical distance between car body centre of mass and track plane | m | 1.5 | 1.87 |
| rt | Wheel’s rolling radius | m | 0.46 | |
References
- Zboinski, K.; Golofit-Stawinska, M. Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies. Energies 2024, 17, 967. [Google Scholar] [CrossRef]
- Zboinski, K.; Golofit-Stawinska, M. Investigation into nonlinear phenomena for various railway vehicles in transition curves at velocities close to critical one. Nonlinear Dyn. 2019, 98, 1555–1601. [Google Scholar] [CrossRef]
- Csiba, J. Bogie type anniversary: The bogie type Y25 is over 50 years. In Proceedings of the 10th International Conference on Railway Bogies and Running Gears, Budapest, Hungary, 12–15 September 2016; pp. 253–262. [Google Scholar]
- Pagaimo, J.; Magalheas, H.; Costa, J.N.; Ambrosio, J. Derailment study of railway cargo vehicle using a response surface methodology. Veh. Syst. Dyn. 2022, 60, 309–334. [Google Scholar] [CrossRef]
- Zboiński, K.; Dusza, M. Self-exciting vibrations and Hopf’s bifurcation in non-linear stability analysis of rail vehicles in curved track. Eur. J. Mech. Part A Solids 2010, 29, 190–203. [Google Scholar] [CrossRef]
- Zboiński, K.; Dusza, M. Development of the method and analysis for non-linear lateral stability of railway vehicles in a curved track. Veh. Syst. Dyn. 2006, 44 (Suppl. S1), 147–157. [Google Scholar] [CrossRef]
- Zboiński, K.; Dusza, M. Bifurcation analysis of 4-axle rail vehicle models in a curved track. Nonlinear Dyn. 2017, 89, 863–885, ISSN 0924-090X. [Google Scholar] [CrossRef]
- Bustos, A.; Tomas-Rodriguez, M.; Rubio, H.; Castejon, C. On the nonlinear hunting stability of a high-speed train boogie. Nonlinear Dyn. 2023, 111, 2059–2078. [Google Scholar] [CrossRef]
- Xiao, F.; Hu, J.; Zhu, P.; Deng, C. A method of three-dimensional stability region and ideal roll angle to improve vehicle stability. Nonlinear Dyn. 2023, 111, 2353–2377. [Google Scholar] [CrossRef]
- Sun, J.; Meli, E.; Song, X.; Chi, M.; Jiao, W.; Jiang, Y. A novel measuring system for high-speed railway vehicles hunting monitoring able to predict wheelset motion and wheel/rail contact characteristics. Veh. Syst. Dyn. 2023, 61, 1621–1643. [Google Scholar] [CrossRef]
- Umemoto, J.; Yabuno, H. Parametric and self-excited oscillation produced in railway wheelset due to mass imbalance and large wheel tread angle. Nonlinear Dyn. 2023, 111, 4087–4106. [Google Scholar] [CrossRef]
- Seth, S.; Kudra, G.; Wasilewski, G.; Awrejcewicz, J. Study the bifurcations of a 2DoF mechanical impacting system. Nonlinear Dyn. 2024, 112, 1713–1728. [Google Scholar] [CrossRef]
- Margazoglou, G.; Magri, L. Stability analysis of chaotic systems from data. Nonlinear Dyn. 2023, 111, 8799–8819. [Google Scholar] [CrossRef] [PubMed]
- Guo, J.; Shi, H.; Luo, R.; Zeng, J. Bifurcation analysis of a railway wheelset with nonlinear wheel–rail contact. Nonlinear Dyn. 2021, 104, 989–1005. [Google Scholar] [CrossRef]
- Ge, P.; Wie, X.; Liu, J.; Cao, H. Bifurcation of a modified railway wheelset model with nonlinear equivalent conicity and wheel-rail force. Nonlinear Dyn. 2020, 102, 79–100. [Google Scholar] [CrossRef]
- Yang, C.; Huang, Y.; Li, F. Influence of Curve Geometric Parameters on Dynamic Interactions of Side-Frame Cross-Braced Bogie. In Proceedings ICRT 2021, Second International Conference on Rail Transportation; ASCE Library: Reston, VA, USA, 2022. [Google Scholar]
- Chernysheva, Y.; Gorskiy, A. Methods Proposed for Analysis of Vibrations of Railway Cars. In International Scientific Siberian Transport Forum TransSiberia—2021; TransSiberia 2021; Lecture Notes in Networks and Systems; Manakov, A., Edigarian, A., Eds.; Springer: Cham, Switzerland, 2022; Volume 402. [Google Scholar] [CrossRef]
- Skerman, D.; Colin Cole, C.; Spiryagin, M. Determining the critical speed for hunting of three-piece freight bogies: Practice versus simulation approaches. Veh. Syst. Dyn. 2022, 60, 3314–3335. [Google Scholar] [CrossRef]
- Pandey, M.; Bhattacharya, B. Effect of bolster suspension parameters of three-piece freight bogie on the lateral frame force. Int. J. Rail Transp. 2020, 8, 45–65. [Google Scholar] [CrossRef]
- Sun, J.; Chi, M.; Jin, X.; Liang, S.; Wang, J.; Li, W. Experimental and numerical study on carbody hunting of electric locomotive induced by low wheel-rail contact conicity. Veh. Syst. Dyn. 2021, 59, 203–223. [Google Scholar] [CrossRef]
- Shvets, A.O. Dynamic interaction of a freight car body and a three-piece bogie during axle load increase. Veh. Syst. Dyn. 2022, 60, 3291–3313. [Google Scholar] [CrossRef]
- Guo, J.; Zhang, G.; Shi, H.; Zeng, J. Small amplitude bogie hunting identification method for high-speed trains based on machine learning. Veh. Syst. Dyn. 2023, 62, 1253–1267. [Google Scholar] [CrossRef]
- He, S.; Jonsson, E.; Martins, J.R.R.A. Adjoint-based limit cycle oscillation instability sensitivity and suppression. Nonlinear Dyn. 2023, 111, 3191–3205. [Google Scholar] [CrossRef]
- Wang, X.; Lu, Z.; Wen, J.; Wie, J.; Wang, Z. Kinematics modelling and numerical investigation on the hunting oscillation of wheel-rail nonlinear geometric contact system. Nonlinear Dyn. 2022, 107, 2075–2097. [Google Scholar] [CrossRef]
- Knothe, K.; Böhm, F. History of Stability of Railway and Road Vehicles. Veh. Syst. Dyn. 1999, 31, 283–323. [Google Scholar] [CrossRef]
- Kass-Petersen, C.; True, H. A bifurcation analysis of nonlinear oscillations in railway vehicles. Veh. Syst. Dyn. 1984, 13, 655–665. [Google Scholar]
- True, H. On the theory of nonlinear dynamics and its applications in vehicle systems dynamics. Veh. Syst. Dyn. 1999, 31, 393–421. [Google Scholar] [CrossRef]
- Iwnicki, S. (Ed.) Handbook of Railway Vehicle Dynamics; CRC Press Inc.: Boca Raton, FL, USA, 2006. [Google Scholar]
- Shabana, A.A.; Zaazaa, K.E.; Sugiyama, H. Railroad Vehicle Dynamics: A Computational Approach; Taylor & Francis LLC: Abingdon, UK; CRC: Boca Raton, FL, USA, 2008. [Google Scholar]
- Piotrowski, J.; Pazdzierniak, P.; Adamczewski, T. Curving dynamics of freight wagon with one- and two-dimensional friction damping. In Proceedings of the 10th Mini Conference on Vehicle System Dynamics, Identification and Anomalies, Budapest, Hungary, 6–8 November 2006; Zobory, I., Ed.; pp. 215–222. [Google Scholar]
- Bruni, S.; Vinolas, J.; Berg, M.; Polach, O.; Stichel, S. Modeling of suspension components in a rail vehicle dynamics context. Veh. Syst. Dyn. 2011, 49, 1021–1072. [Google Scholar] [CrossRef]
- Evans, J.; Berg, M. Challenges in simulation of rail vehicle dynamics. Veh. Syst. Dyn. 2009, 47, 1023–1048. [Google Scholar] [CrossRef]
- Chudzikiewicz, A.; Drozdziel, J.; Kisilowski, J.; Zochowski, A. Modelowanie i Analiza Dynamiki Układu Mechanicznego Tor-Pojazd; Państwowe Wydawnictwo Naukowe (PWN): Warszawa, Poland, 1982; ISBN 83-01-04727-5. (In Polish) [Google Scholar]
- Shi, H.; Zeng, J.; Guo, J. Disturbance observer-based sliding mode control of active vertical suspension for high-speed rail vehicles. Veh. Syst. Dyn. 2024. [Google Scholar] [CrossRef]
- Kalker, J.J. A fast algorithm for the simplified theory of rolling contact. Veh. Syst. Dyn. 1982, 11, 1–13. [Google Scholar] [CrossRef]
- Piotrowski, J. Kalker’s algorithm Fastsim solves tangential contact problems with slip-dependent friction and friction anisotropy. Veh. Syst. Dyn. 2010, 48, 869–889. [Google Scholar] [CrossRef]
- Bogusz, W. Technical Stability; PWN: Warszawa, Poland, 1972. (In Polish) [Google Scholar]



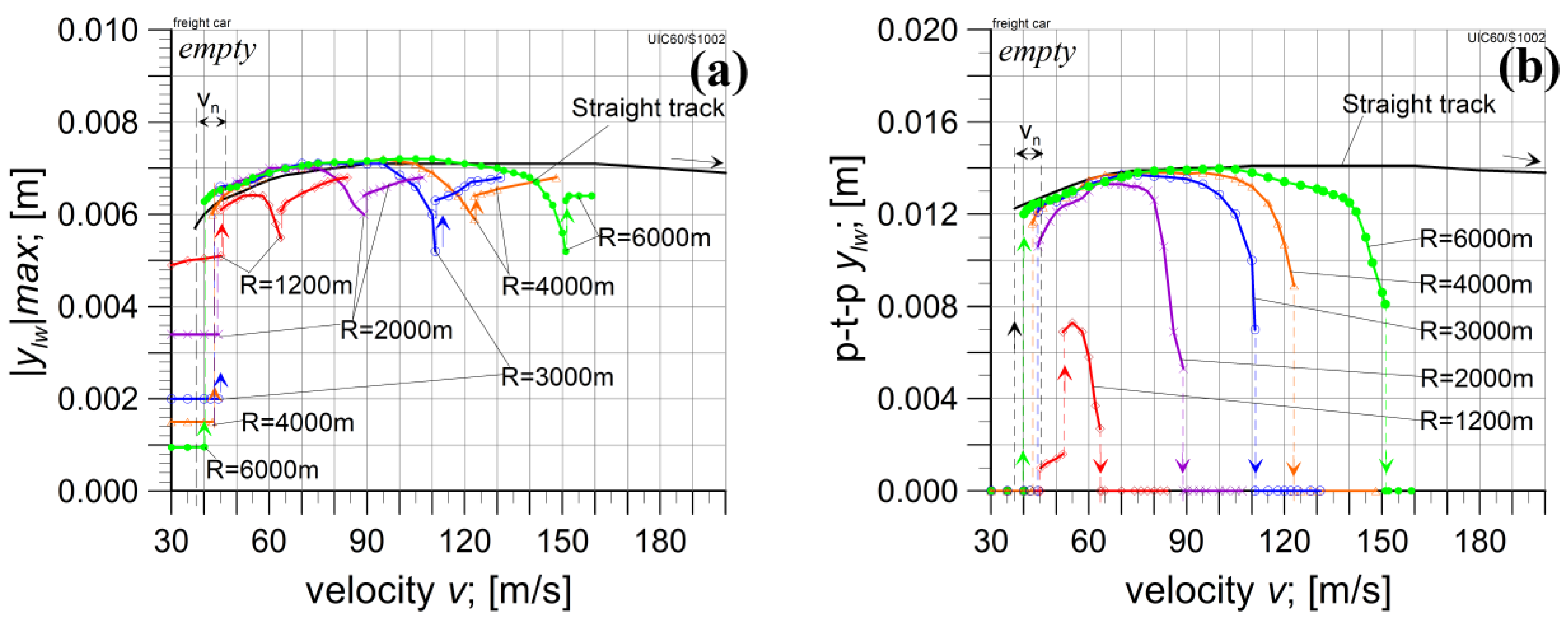
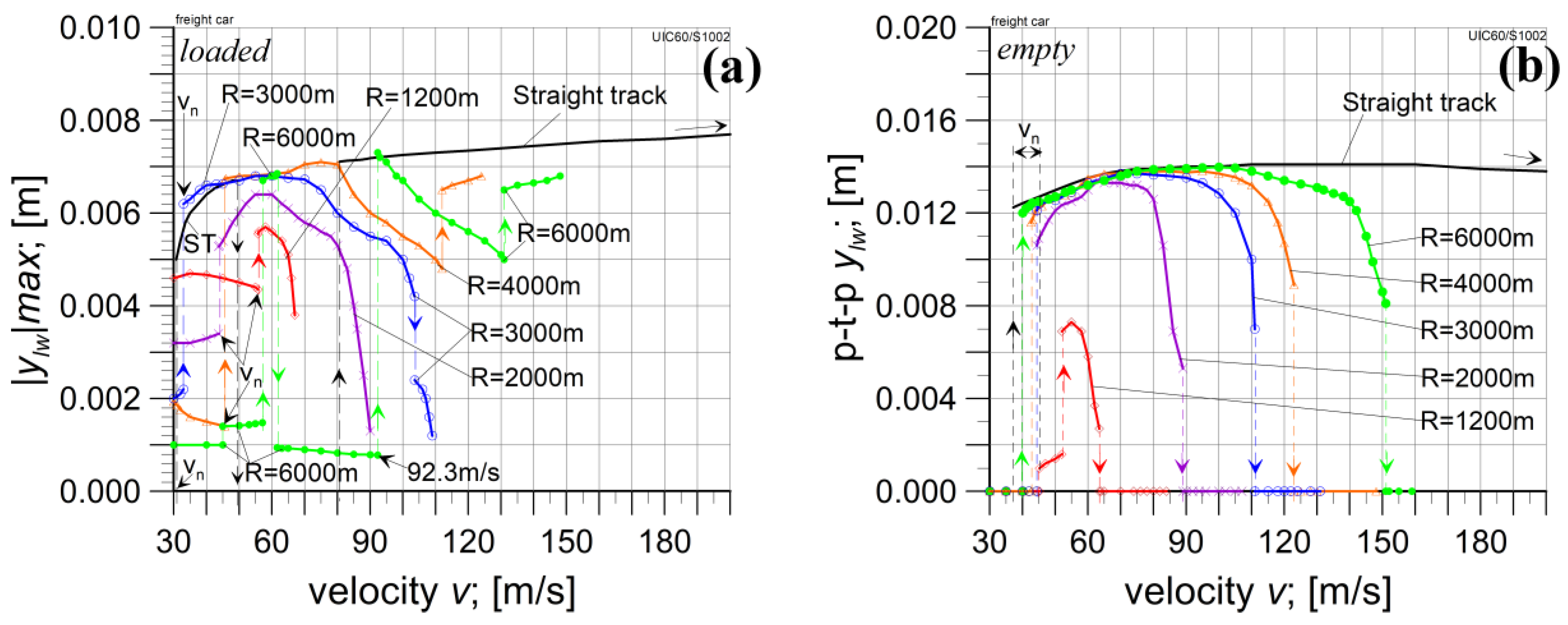
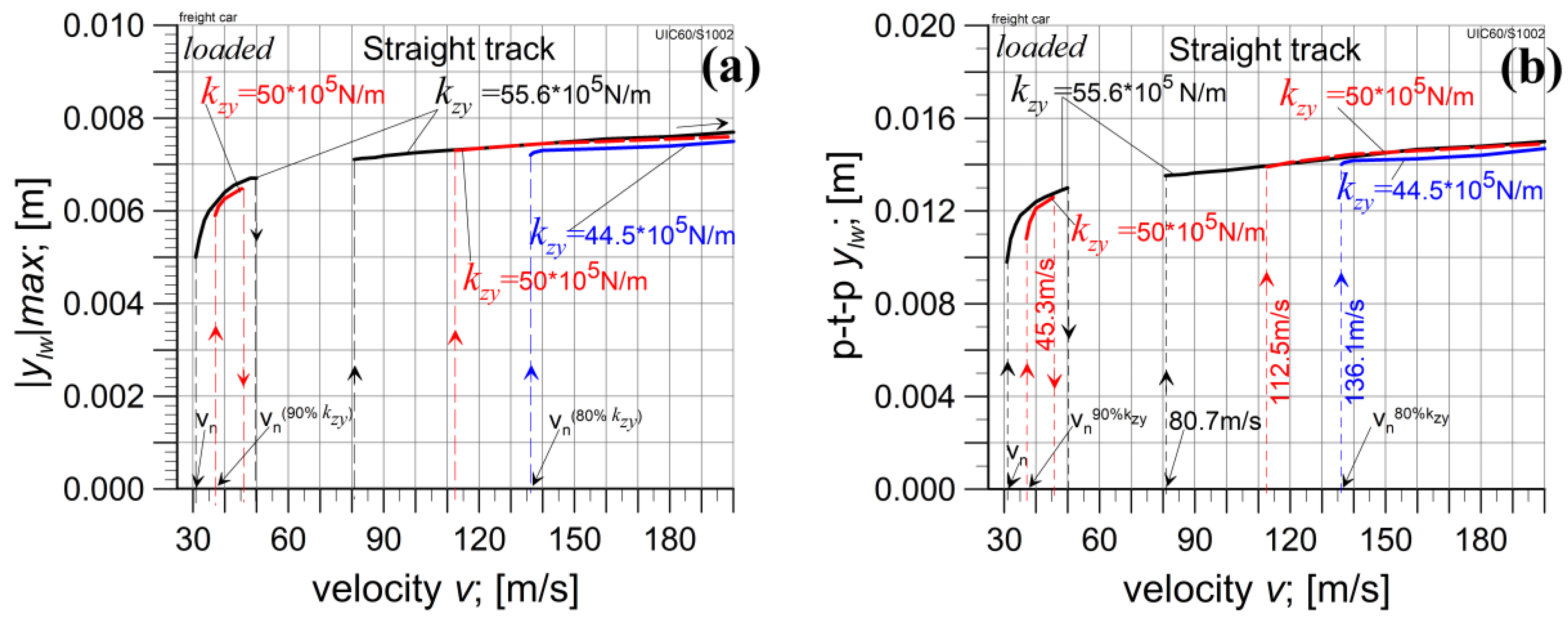
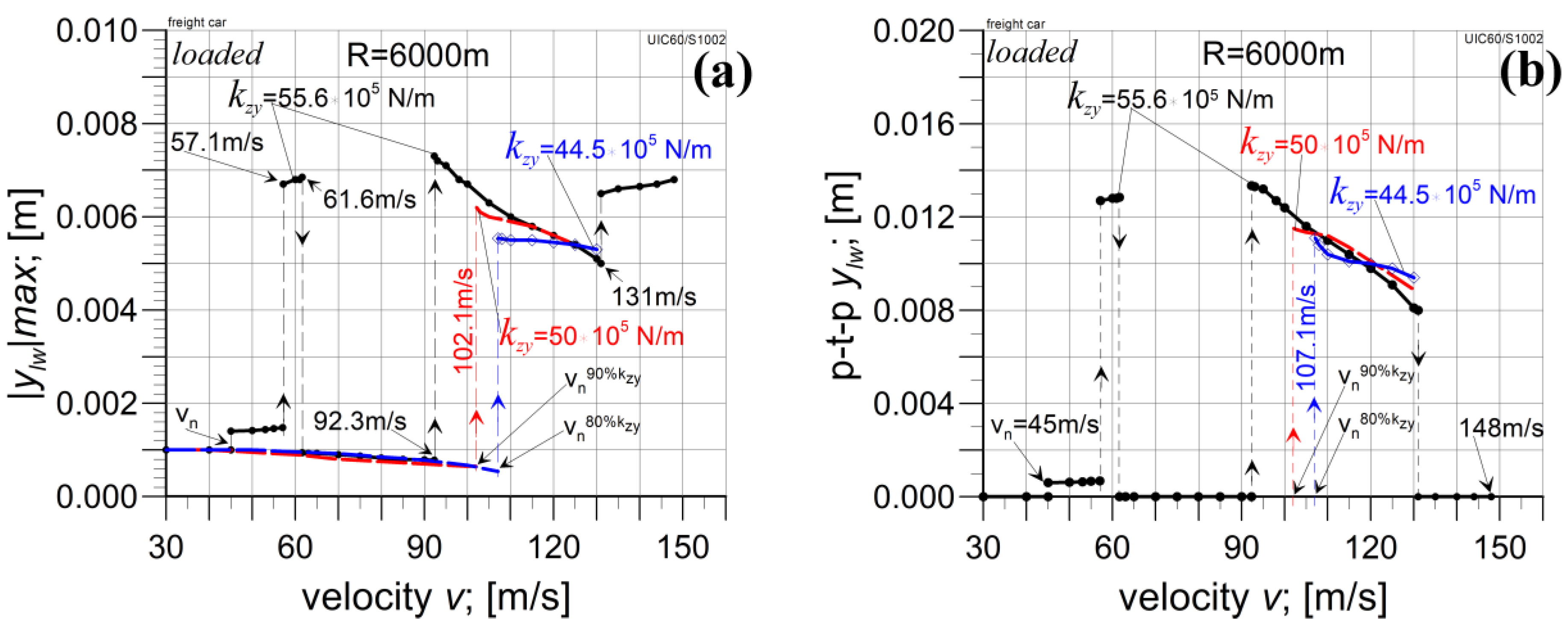
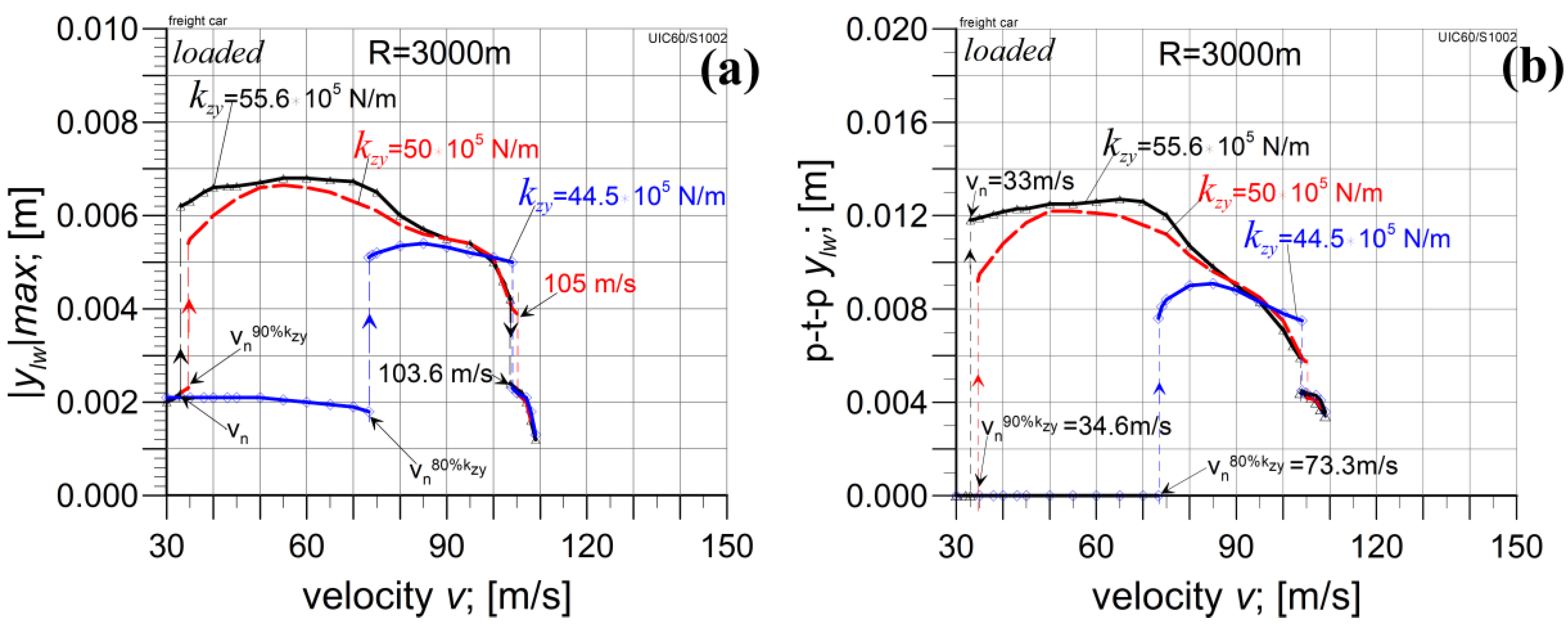
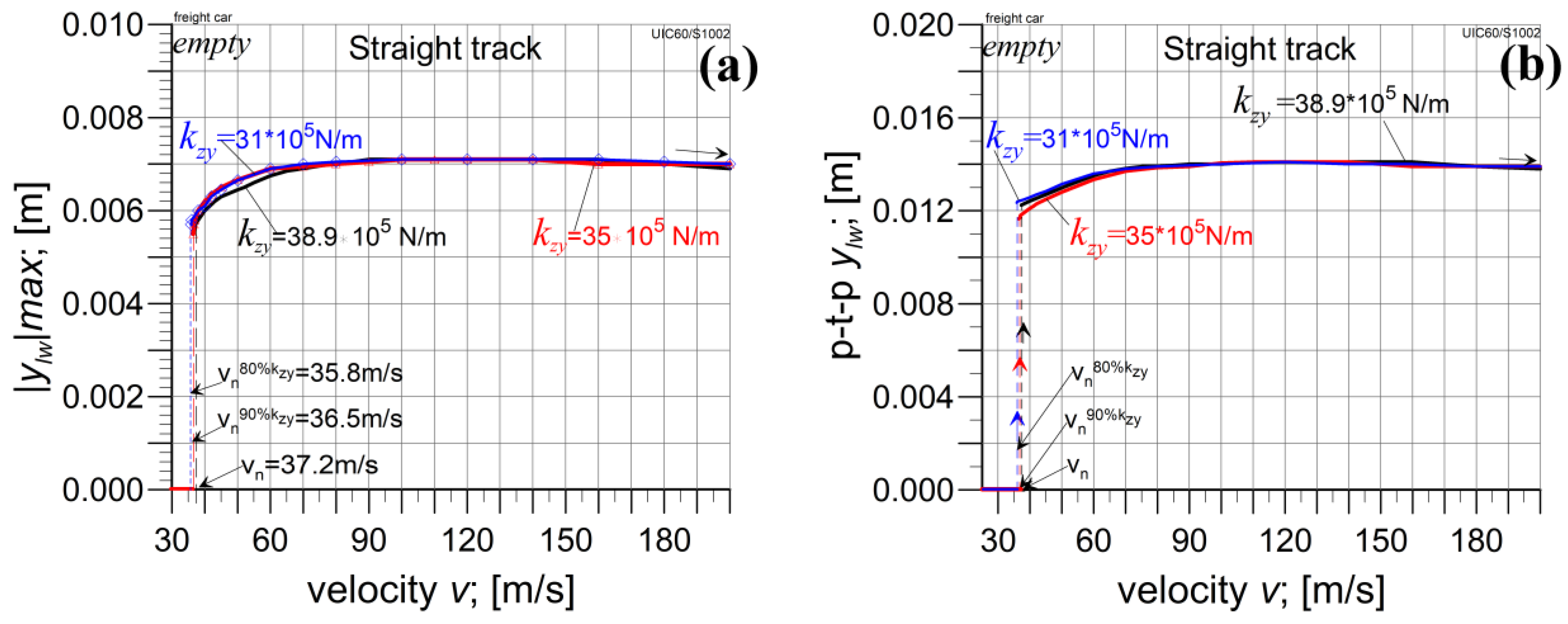


| CC radius; R in m | 1200 | 2000 | 3000 | 4000 | 6000 | ∞ |
| Superelevation; h in m | 0.150 | 0.130 | 0.110 | 0.077 | 0.051 | 0 |
| CC radius; R in m | 1200 | 2000 | 3000 | 4000 | 6000 | ∞ |
| Empty car; vn in m/s | 45.2 | 44.4 | 44.4 | 42.6 | 40.1 | 37.2 |
| Laden car; vn in m/s | 55.8 | 43.7 | 33 | 45.6 | 45 | 30.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dusza, M.; Golofit-Stawinska, M.; Zboinski, K. The Nonlinear Lateral Stability of a Four-Axle Freight Car with Y25 Bogies and Measures to Improve Its Faults. Appl. Sci. 2024, 14, 4545. https://doi.org/10.3390/app14114545
Dusza M, Golofit-Stawinska M, Zboinski K. The Nonlinear Lateral Stability of a Four-Axle Freight Car with Y25 Bogies and Measures to Improve Its Faults. Applied Sciences. 2024; 14(11):4545. https://doi.org/10.3390/app14114545
Chicago/Turabian StyleDusza, Miroslaw, Milena Golofit-Stawinska, and Krzysztof Zboinski. 2024. "The Nonlinear Lateral Stability of a Four-Axle Freight Car with Y25 Bogies and Measures to Improve Its Faults" Applied Sciences 14, no. 11: 4545. https://doi.org/10.3390/app14114545





