Analysis of Damage Process in a Pre-Notched Rock Specimen: The Synergy between Experimental Results and Simulations Using a Peridynamic Model
Abstract
:1. Introduction
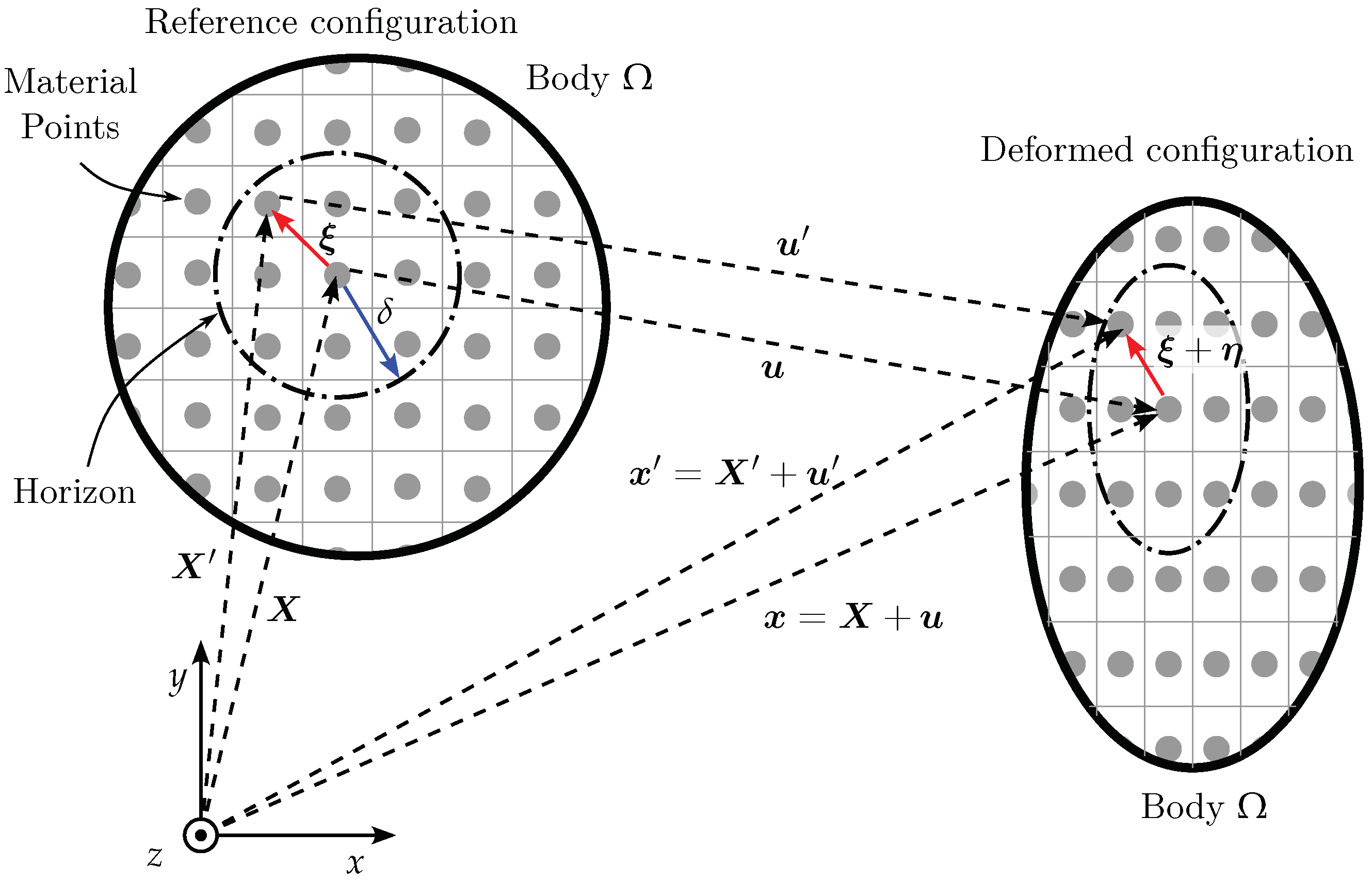
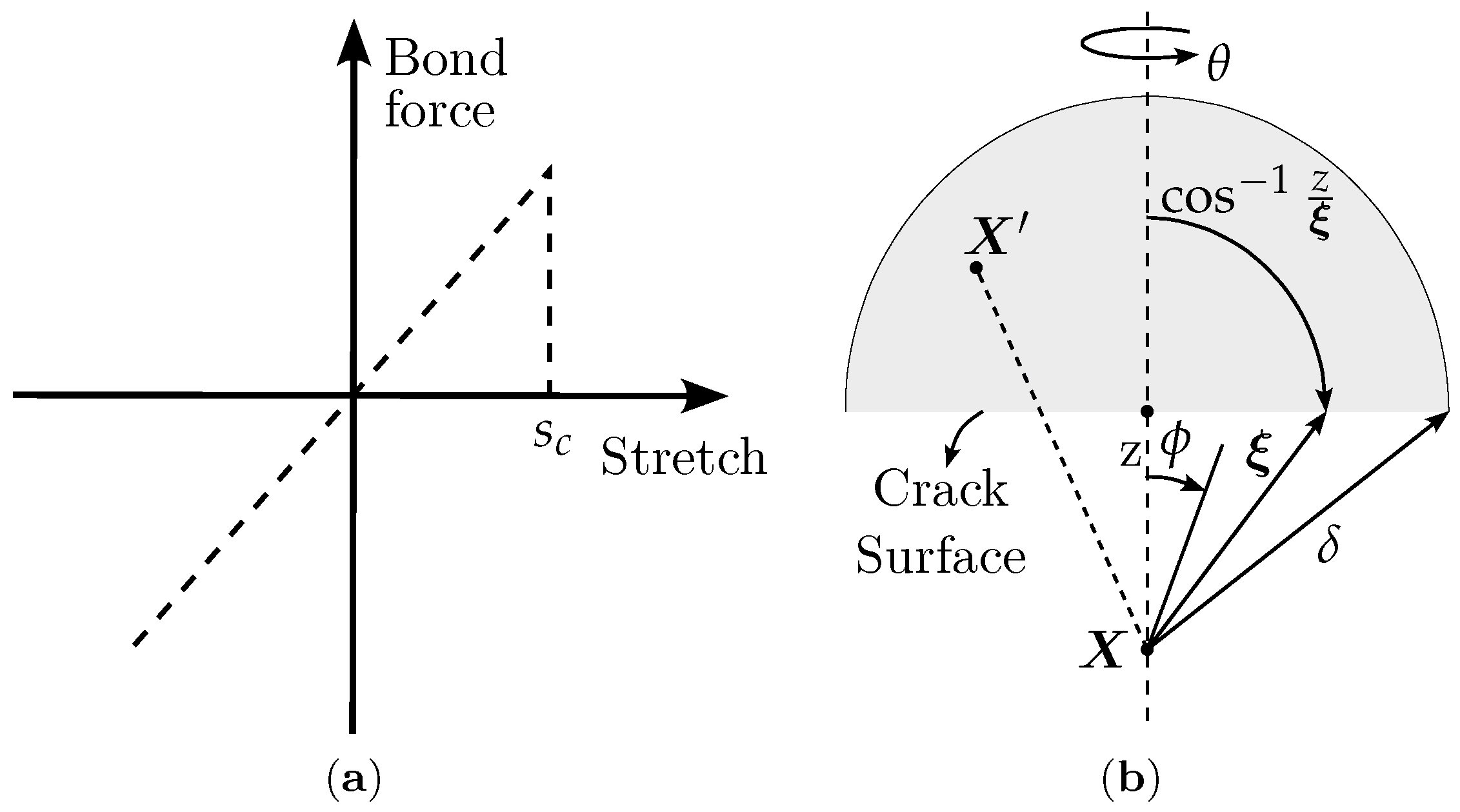
2. Bond-Based Peridynamic
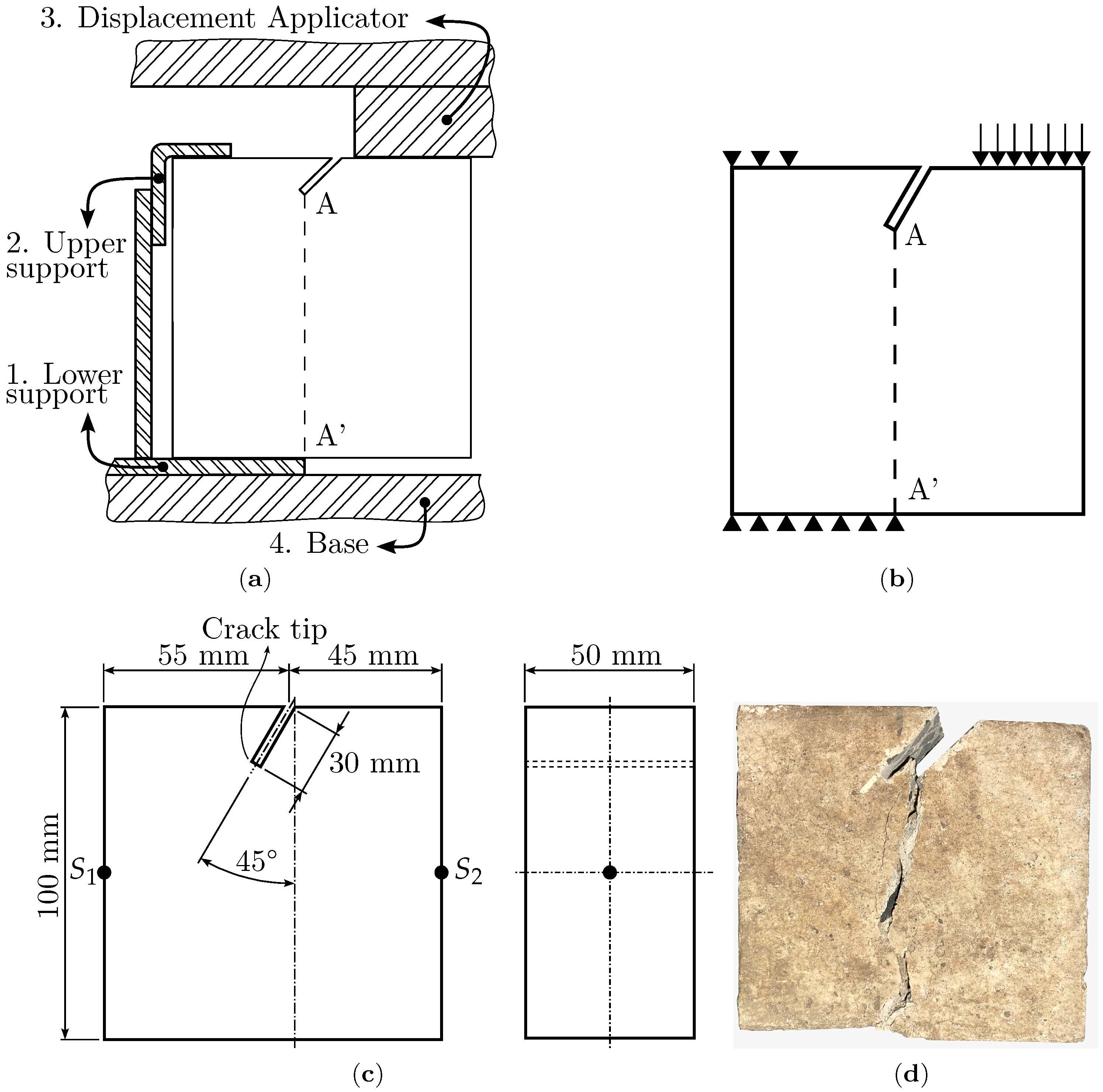
3. Experimental Tests
4. Numerical Analyses Using Peridynamic Model
- (i)
- In the peridynamic context, the characteristic length of the material indicates the interconnection length between neighboring nodes;
- (ii)
- A good relation between the horizon and the discretization level () for numerical reasons is considered by [9] to be . This relation is used in the present work;
- (iii)
- Toughness, , is considered a random field regarding the mean, variation coefficient, Weibull distribution, and the correlation length of a random space field. Details about the implementation of this random field are explained in [27];
- (iv)
- The research group developed proposals that allow for establishing a link between the independent material characteristic length and discretization. In [33], replacing the constitutive law for the bound with a bi-linear law is proposed, which allows for defining the material characteristic length independent of the discretization level. This possibility was not used in the simulation implemented in the present paper.
5. Results
5.1. Experimental Results
- (i)
- (ii)
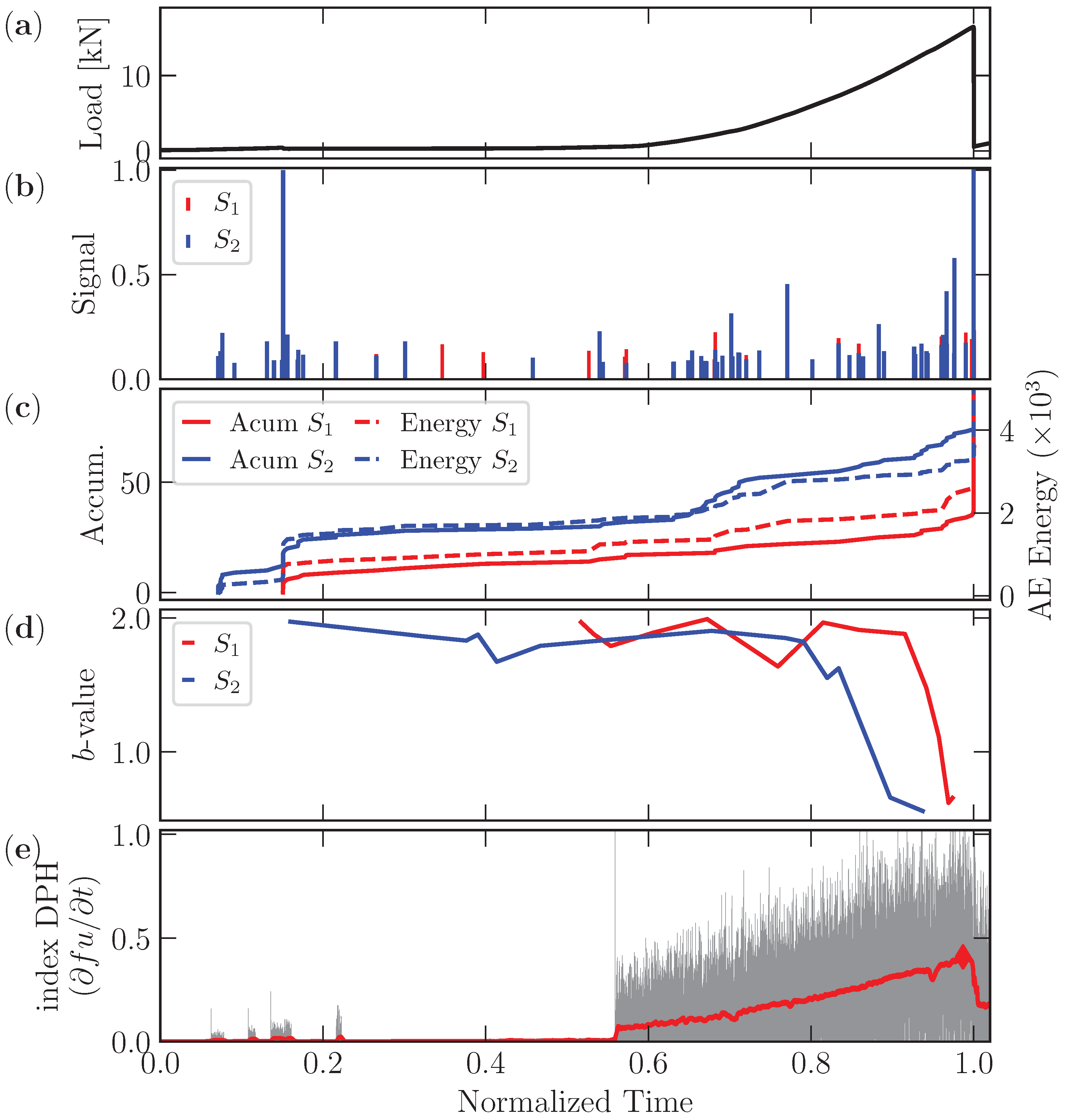
- The AE signals from sensors 1 and 2 are presented in Figure 7b. Note the high activity recorded at sensor 2 around the normalized time 0.15, which is due to the movement of the support, which is indicated by label 3 in Figure 5a. Furthermore, more AE emissions can be observed from sensor 2 (, right side) than from sensor 1 (, left side). This information is consistent with the final configuration presented in Figure 8c, where it is evident that the damage is concentrated on the right side of the specimen.
- (iii)
- (iv)
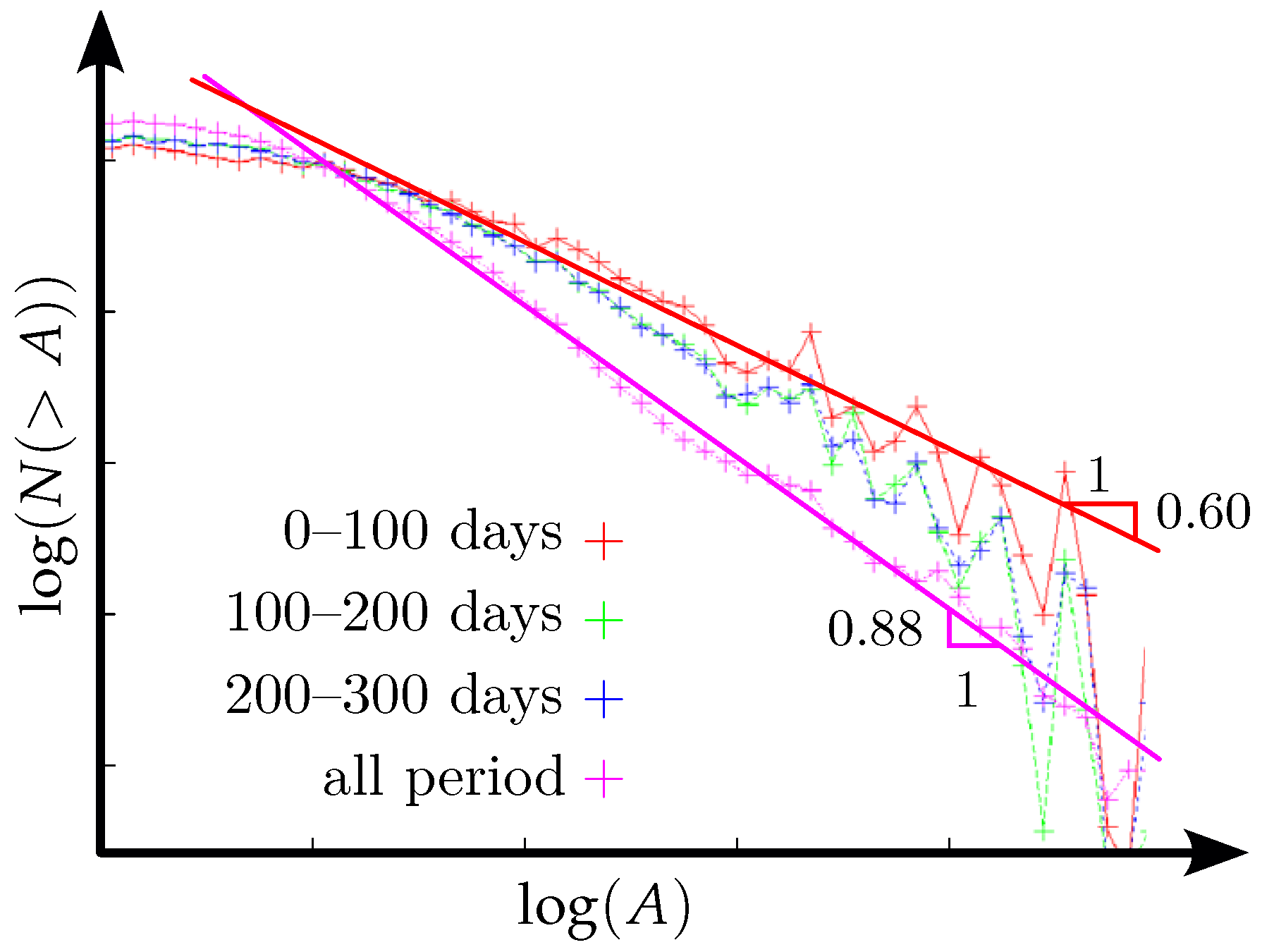
- The evolution of the b-value is presented in Figure 7d. Note that both sensors indicate a drop in the b-value only at the end of the test when a collapse is expected. At , the b-value does not change due to the movement of the supports previously mentioned.
- (v)
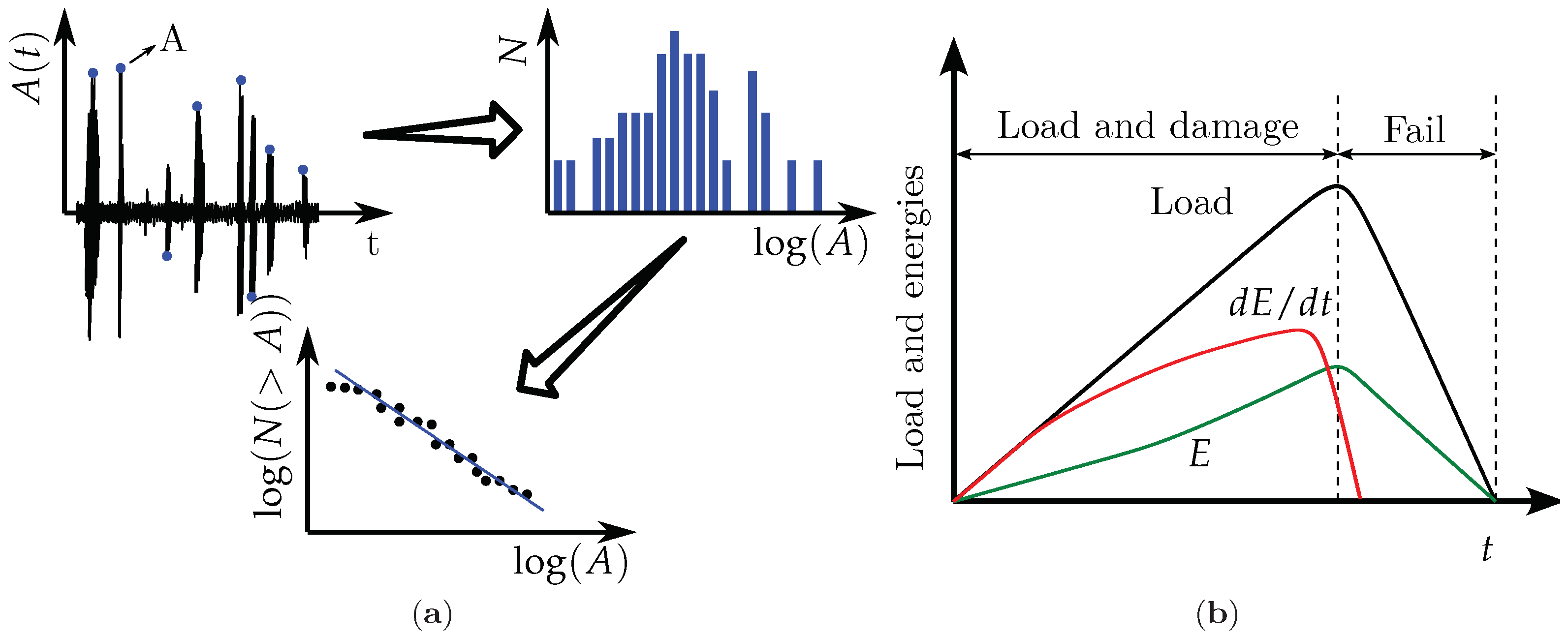
- The evolution of the DPH index is presented in dark gray in Figure 7e. The moving average carried out in a window of 175 values is indicated in red in the same figure, and a red diamond indicates the maximum value of the DPH index. This value, as discussed in [26], can be considered a complementary precursor to collapse when an acoustic emission test is carried out. Note that, in the experimental case, the reaction load of the support (indicated by label 3 in Figure 5a) times the prescribed displacement is considered a measure of elastic energy, and from this value the derivative is calculated.
- (vi)
- Considering the methodology adopted by [26] and that which was previously described for determining a measure of elastic energy in an experimental test, it is possible to see an abrupt increase in the DPH index at . This is further evidence that the support cannot fix the specimen correctly before .
- (i)
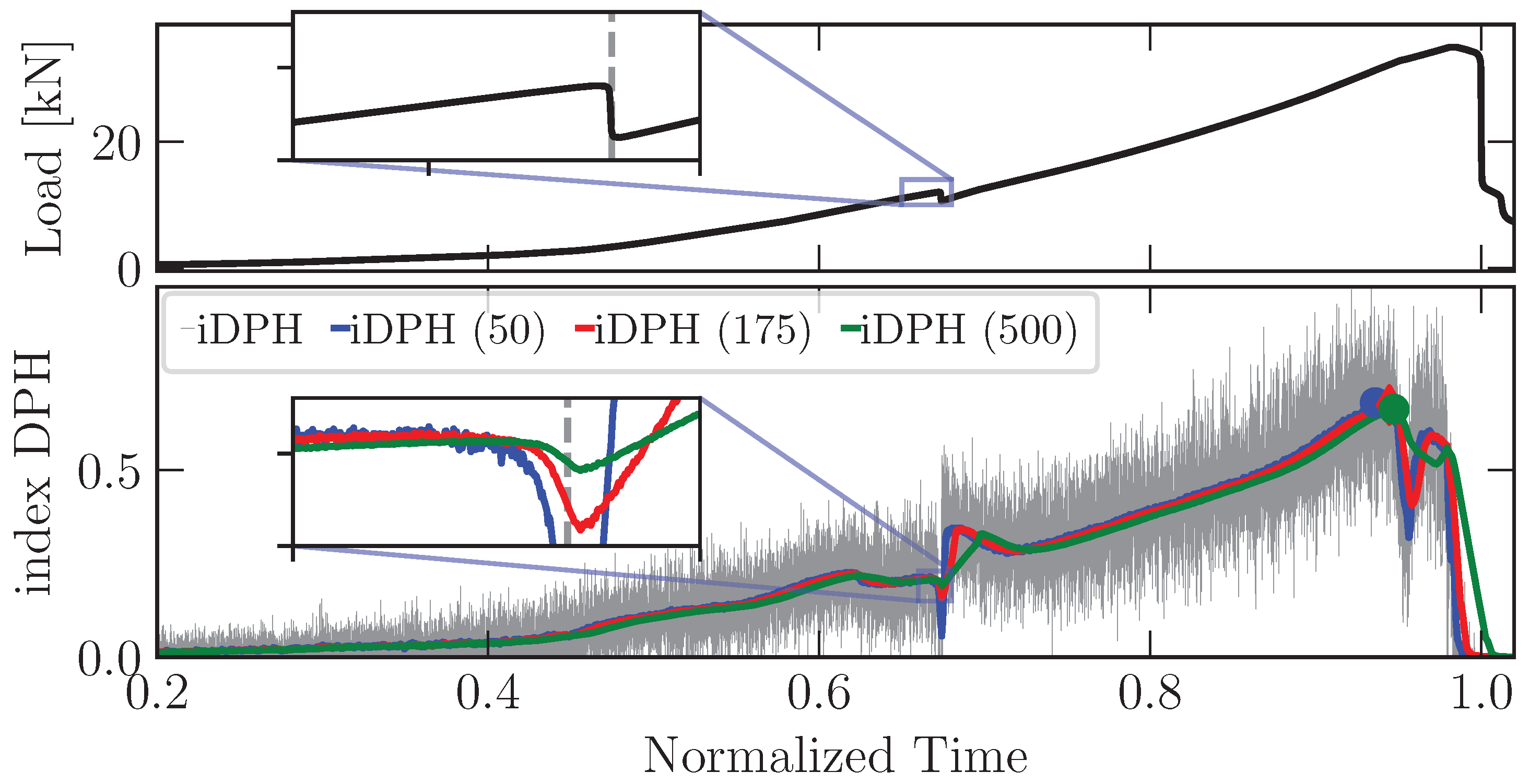
- The initial interval before the upper support actually solicited the specimen () was removed from the results presented for specimen 4 in Figure 8.
- (ii)
- The DPH index in all cases is presented in a dark gray color, and the moving average using 175 points is presented as a red line.
- (iii)
- Specimen 3 shows a final configuration different from specimens 2 and 4; this is also evidenced by the load and DPH index evolution. The local failure that appears in , when viewed in terms of DPH index evolution, shows that, before the local minimum appears, a smooth local maximum occurs, as evidenced by the detail presented in Figure 8b. This behavior is also investigated in Figure 9, where the sensibility of the number of data used to make the moving average is investigated.
- (iv)
- In all the tests, the maximum value of the DPH index appears close to the failure, and the local maximum also indicates some local failure during the test.
5.2. Simulations Results
- (i)
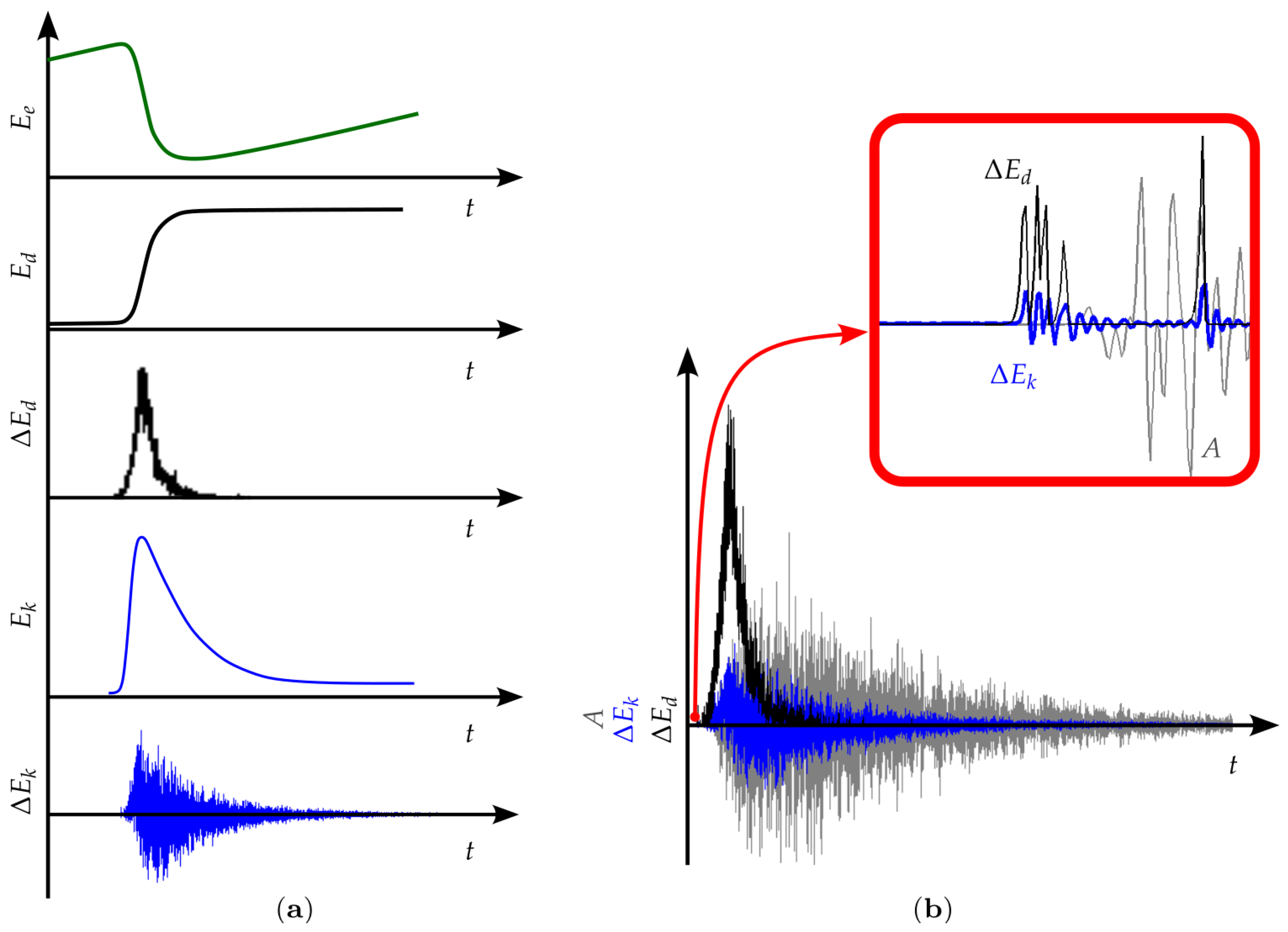
- Damage energy does not begin at zero because when a pre-notch is created (as shown in Figure 6c) the peridynamic model computes the damage energy of each bond deactivated to create this pre-notch.
- (ii)
- The abrupt drop in damage energy that is wasted when the final failure happens is called , and the value of energy dissipated smoothly up to reach the eminence of the final fracture is called . The inspection of these two values confirms that Config. 1 presents more brittle behavior than Config. 2, dissipating practically the same quantity of energy up to arriving at the final configuration ( value) but presenting a sharper decrease in terms of for Config. 1 ( ) than that of Config. 2 ( ).
- (iii)
- Reinforcing the observation (ii) that the increase in elastic energy is more accentuated in Config. 1 than in Config. 2, there is an indication that more elastic energy () is available to produce a brittle failure in Config. 1 ( ) than in Config. 2 ( ).
- (iv)
- The kinetic energy evaluation during the simulation shows a sensible increase after the specimens begin to show a smooth increase in the dissipated energy close to for both configurations. In addition, fluctuations increase at the end of the simulations when the final failure happens, and notice that in Config. 1 these fluctuations are higher than in Config. 2, which is in agreement with the fact that Config. 1 presents increased brittle behavior.
- (i)
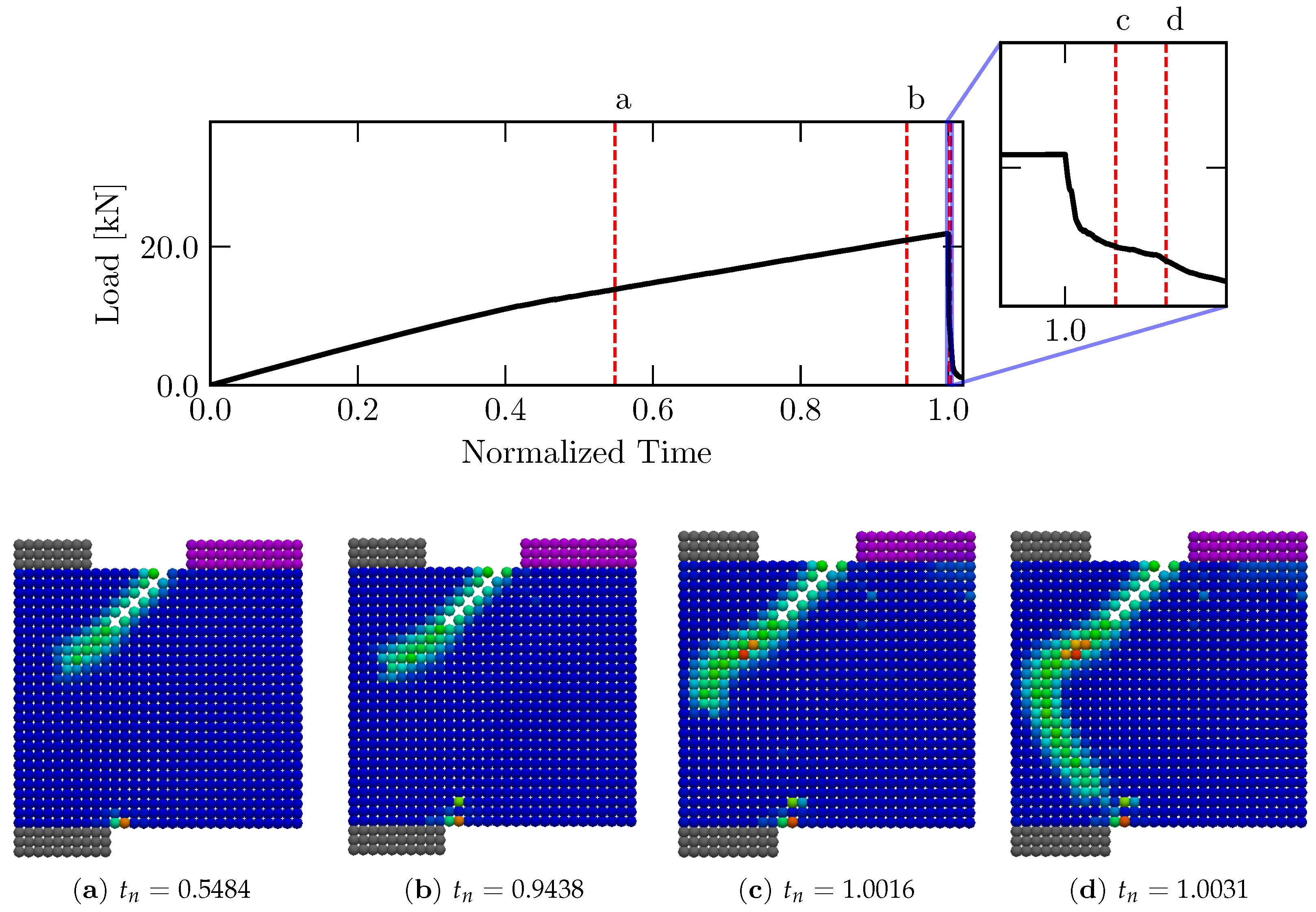
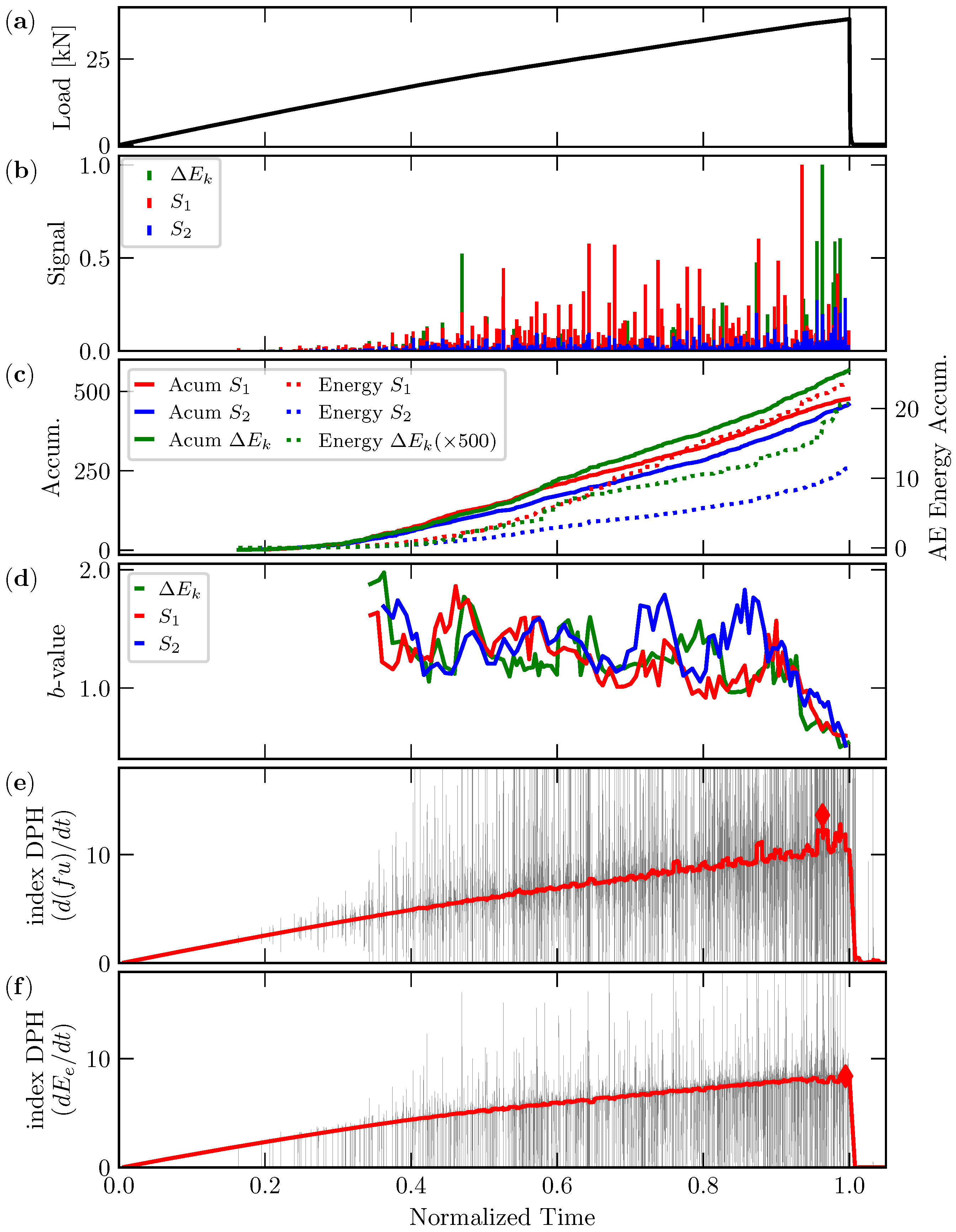
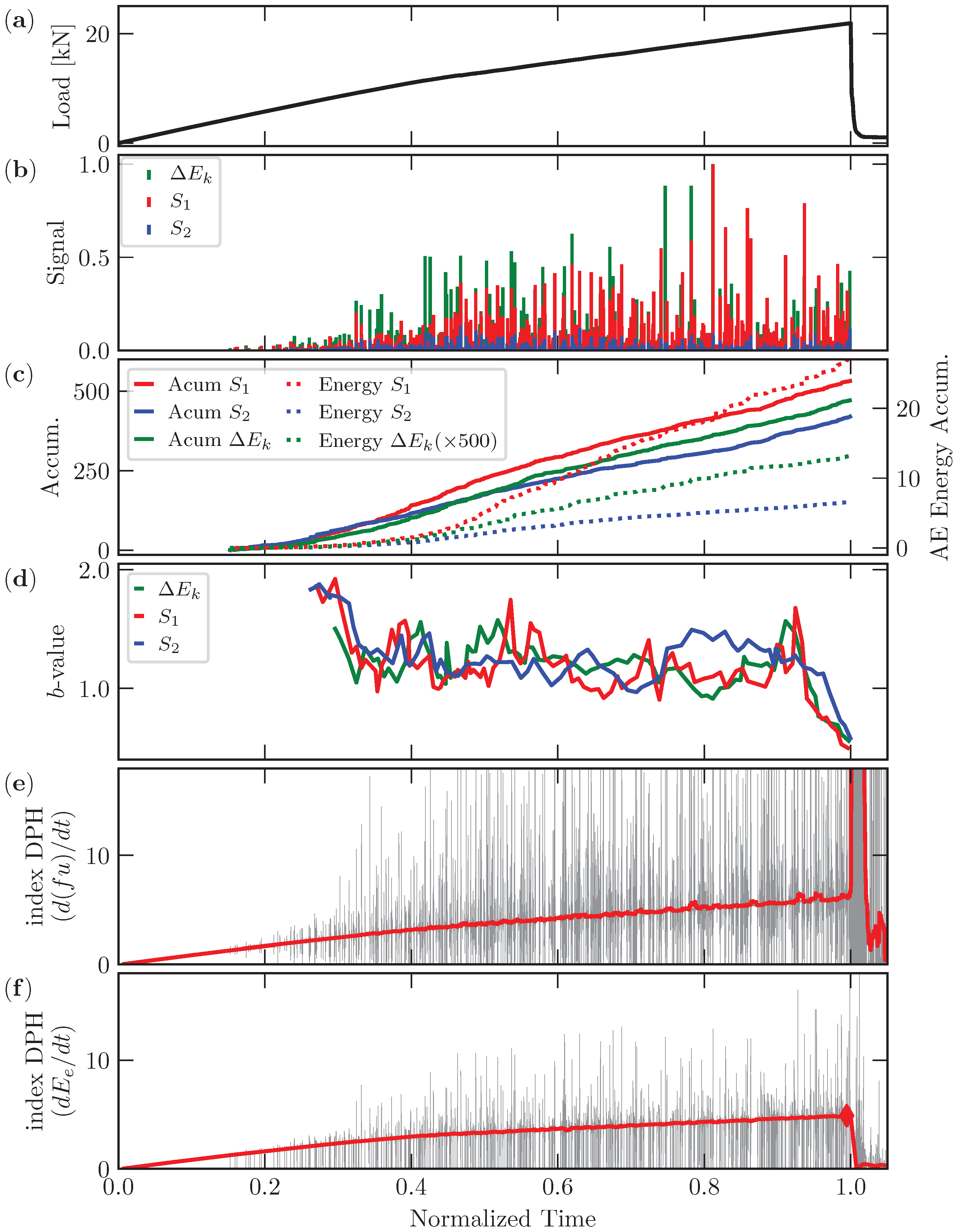
- For Config. 1, it is evidenced that, in Figure 15b, sensor 1 recorded more signal activity than sensor 2 until . After this time, the same activity in both sensors is observed. This fact is coherent with the evolution of the configurations presented in Figure 11a,b; until , high AE activity comes from the pressure head. This source is close to sensor 1. This tendency changes after , when the failure mechanism changes, as is depicted in Figure 11c,d. In the second mechanism, the source of AE activity is close to sensor 2.
- (ii)
- On the other hand, sensor 1 registers more AE activity during all of the simulations in Config. 2, as shown in Figure 16b, and, in this case, this fact is coherent with the configuration presented in Figure 12a–d, which show the crack tip as the main failure source of AE activity during all simulations.
- (iii)
- In Figure 15b and Figure 16b, it is interesting to compare the signal obtained from the two sensors and the equivalent information obtained from . In this comparison, it is possible to measure how the travel between the event source and the device modified the acoustic emission signal captured. The simulation of this situation might suggest placing the AE sensor in a better position in the experimental test. Particularly, in the present case, sensor 1 is better positioned than sensor 2. In general, it will be possible to determine if the combination of specimen geometry, boundary condition, and damage distribution can help identify the best position for the AE sensors.
- (iv)
- In Figure 15c and Figure 16c, the number of accumulated signals and the change in signal energy during the simulation are presented. In both configurations, the results in terms of the number of signals and energy indicate higher activity registered in sensor 1 until the final part of the simulation in Config. 1 and during all the simulations in Config. 2. The measure of the accumulated number of events and the accumulated energy computed from are coherent with the results obtained from the signal information obtained with the AE sensors.
- (v)
- (vi)
- The DPH index presented in Figure 15e,f shows a maximum value before arriving at the failure in both cases. Notice that obtaining the DPH index directly from the elastic energy or from a measure of the elastic energy (reaction support times the support displacement) indicates the same tendency.
6. Conclusions
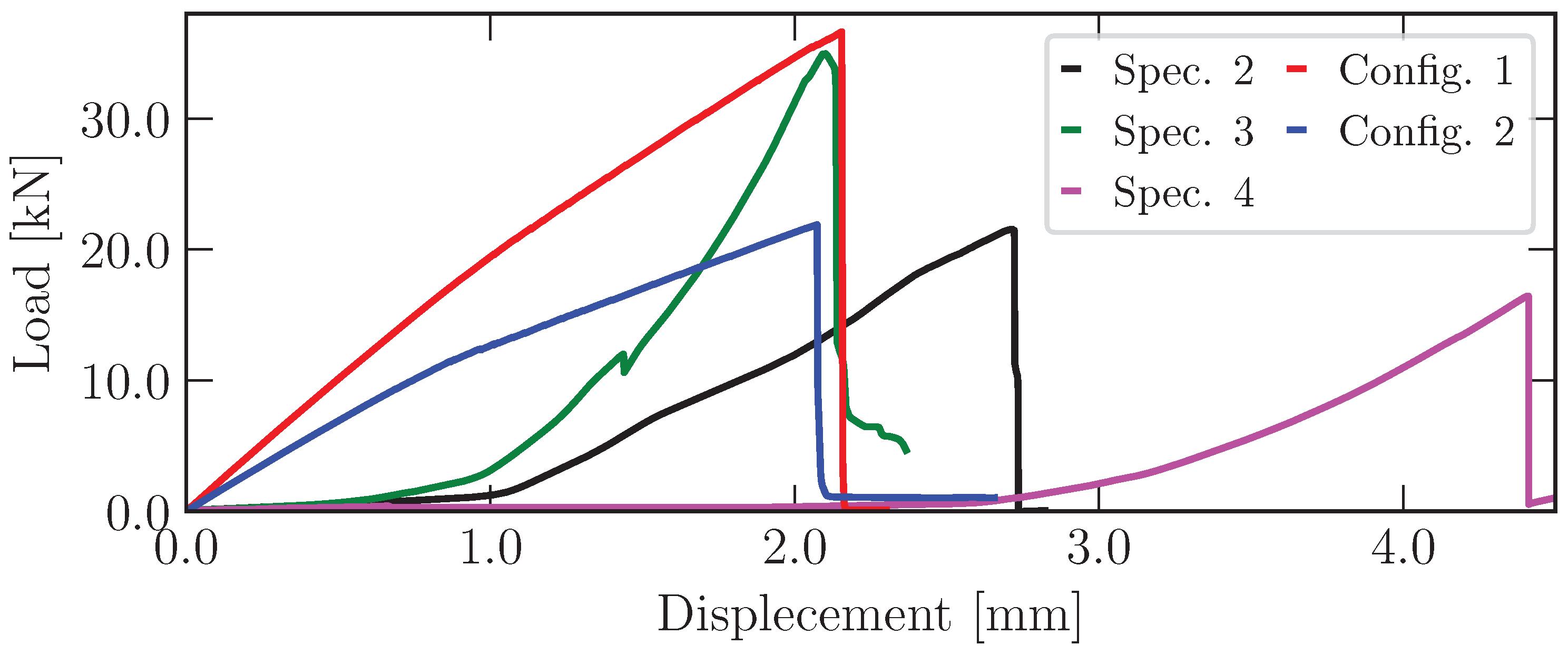
- The three tests carried out showed high dependency on the flexibility of the support, with the three tests presenting quite different displacements and final configurations.
- A strong dependence on boundary conditions was also seen in the simulation results using the peridynamic model.
- The acoustic emission information obtained in the tests presented coherency, and the results allowed for explanations regarding the damage process analyzed.
- The evaluation of the DPH index for all the specimens tested and the simulations demonstrated the potential of this index to indicate a maximum value close to a local or global collapse. In all the cases analyzed, the value of the DPH index presented a maximum before the collapse occurs. This parameter could provide complementary information in AE analysis.
- The simulation of the two configurations indicated the potential of the peridynamic model to represent an AE test and the possibility to obtain information that aids AE experimental testing.
- Specifically in the simulations, the possibility of comparing the signal measured with the device and an alternative measure called is interesting; this information could be used to perceive distortions in the waves that travel between the signal event source and the signal captured by the device. This analysis could be used to define the best position for the AE device in the experimental test.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AE | Acoustic emission |
| DPH | Dȩbski–Pradhan–Hansen |
| GR | Gutenberg–Richter |
References
- Kachanov, L.M. Introduction to Continuum Damage Mechanics. In Mechanics of Elastic Stability; Springer: Dordrecht, The Netherlands, 1986; Volume 10. [Google Scholar] [CrossRef]
- Park, T.; Ahmed, B.; Voyiadjis, G.Z. A review of continuum damage and plasticity in concrete: Part I—Theoretical framework. Int. J. Damage Mech. 2022, 31, 901–954. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Ahmed, B.; Park, T. A review of continuum damage and plasticity in concrete: Part II—Numerical framework. Int. J. Damage Mech. 2022, 31, 762–794. [Google Scholar] [CrossRef]
- Zhang, K.; Badeddline, H.; Yue, Z.; Hfaiedh, N.; Saanouni, K.; Liu, J. Failure prediction of magnesium alloys based on improved CDM model. Int. J. Solids Struct. 2021, 217–218, 155–177. [Google Scholar] [CrossRef]
- Krajcinovic, D. Damage Mechanics; North-Holland series in applied mathematic and mechanics, 41; Elsevier: Amsterdam, The Netherlands; New York, NY, USA, 1996; ISBN 978-0-444-82349-6. [Google Scholar]
- Mastilovic, S.; Rinaldi, A. Two-Dimensional Discrete Damage Models: Discrete Element Methods, Particle Models, and Fractal Theories. In Handbook Of Damage Mechanics; Springer: New York, NY, USA, 2013; pp. 1–27. [Google Scholar] [CrossRef]
- Jenabidehkordi, A. Computational methods for fracture in rock: A review and recent advances. Front. Struct. Civ. Eng. 2018, 13, 273–287. [Google Scholar] [CrossRef]
- Silling, S. Reformulation of elasticity theory for discontinuities and long-range forces. J. Mech. Phys. Solids 2000, 48, 175–209. [Google Scholar] [CrossRef]
- Silling, S.; Askari, E. A meshfree method based on the peridynamic model of solid mechanics. Comput. Struct. 2005, 83, 1526–1535. [Google Scholar] [CrossRef]
- Bobaru, F.; Foster, J.; Geubelle, P.; Silling, S. Handbook of Peridynamic Modeling; Taylor & Francis: Boca Raton, FL, USA, 2015. [Google Scholar]
- Huang, K. Statistical Mechanics, 2nd ed.; Wiley: New York, NY, USA, 1987. [Google Scholar]
- Biswas, S.; Ray, P.; Chakrabarti, B.K. Statistical Physics of Fracture, Breakdown, and Earthquake: Effects of Disorder and Heterogeneity; John Wiley & Sons: Weinheim, Germany, 2015. [Google Scholar]
- Kawamura, H.; Hatano, T.; Kato, N.; Biswas, S.; Chakrabarti, B.K. Statistical Physics of Fracture, Friction and Earthquake. Rev. Mod. Phys. 2012, 84, 839–884. [Google Scholar] [CrossRef]
- Rundle, J.B.; Turcotte, D.L.; Shcherbakov, R.; Klein, W.; Sammis, C. Statistical physics approach to understanding the multiscale dynamics of earthquake fault systems: Statistical physics of Earthquakes. Rev. Geophys. 2003, 41. [Google Scholar] [CrossRef]
- Hansen, A.; Hemmer, P.C.; Pradhan, S. The Fiber Bundle Model: Modeling Failure in Materials; Statistical Physics of Fracture and Breakdown; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2015. [Google Scholar]
- Varotsos, P. Spatio-temporal complexity aspects on the interrelation between seismic electric signals and seismicity. Pr. Athens Acad 2001, 76, 294. [Google Scholar]
- Wilson, K.G. Problems in Physics with many Scales of Length. Sci. Am. 1979, 241, 158–179. [Google Scholar] [CrossRef]
- Richter, C.F. Elementary Seismology; W. H. Freeman and Company: New York, NY, USA; Bailey Bros. & Swinfen Ltd.: London, UK, 1958; Volume 2. [Google Scholar]
- Sethna, J.; Dahmen, K.; Myers, C. Crackling noise. Nature 2001, 410, 242–250. [Google Scholar] [CrossRef] [PubMed]
- Grosse, C.; Ohtsu, M. (Eds.) Acoustic Emission Testing; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Carpinteri, A.; Lacidogna, G.; Puzzi, S. From criticality to final collapse: Evolution of the “b-value” from 1.5 to 1.0. Chaos Solitons Fractals 2009, 41, 843–853. [Google Scholar] [CrossRef]
- Aki, K. Scaling Law of Seismic Spectrum. J. Geophys. Res. 1967, 72, 1217–1231. [Google Scholar] [CrossRef]
- Carpinteri, A.; Lacidogna, G.; Pugno, N. Richter’s laws at the laboratory scale interpreted by acoustic emission. Mag. Concr. Res. 2006, 58, 619–625. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S. Natural Time Analysis: The New View of Time; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar] [CrossRef]
- Dȩbski, W.; Pradhan, S.; Hansen, A. Criterion for Imminent Failure During Loading—Discrete Element Method Analysis. Front. Phys. 2021, 9, 675309. [Google Scholar] [CrossRef]
- Rojo Tanzi, B.N.; Sobczyk, M.; Iturrioz, I.; Lacidogna, G. Damage Evolution in Quasi-Brittle Materials: Experimental Analysis by AE and Numerical Simulation. Appl. Sci. 2023, 13, 10947. [Google Scholar] [CrossRef]
- Puglia, V.B.; Kosteski, L.E.; Riera, J.D.; Iturrioz, I. Random field generation of the material properties in the lattice discrete element method. J. Strain Anal. Eng. Des. 2019, 54, 236–246. [Google Scholar] [CrossRef]
- Shimadzu Corporation. AGS-X Plus: Instruction Manual; Shimadzu: Kyoto, Japan, 2012. [Google Scholar]
- Madenci, E.; Oterkus, E. Peridynamic Theory and Its Applications; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Taylor, D. The theory of critical distances. Eng. Fract. Mech. 2008, 75, 1696–1705. [Google Scholar] [CrossRef]
- Bažant, Z.; Planas, J. Fracture and Size Effect in Concrete and Other Quasibrittle Materials; Routledge: London, UK, 2019; Volum 3. [Google Scholar] [CrossRef]
- Birck, G.; Iturrioz, I.; Lacidogna, G.; Carpinteri, A. Damage process in heterogeneous materials analyzed by a lattice model simulation. Eng. Fail. Anal. 2016, 70, 157–176. [Google Scholar] [CrossRef]
- Rossi Cabral, N.; Invaldi, M.; Barrios D’Ambra, R.; Iturrioz, I. An alternative bilinear peridynamic model to simulate the damage process in quasi-brittle materials. Eng. Fract. Mech. 2019, 216, 106494. [Google Scholar] [CrossRef]
- Iturrioz, I.; Lacidogna, G.; Carpinteri, A. Acoustic emission detection in concrete specimens: Experimental analysis and lattice model simulations. Int. J. Damage Mech. 2014, 23, 327–358. [Google Scholar] [CrossRef]
- Carpinteri, A.; Lacidogna, G.; Corrado, M.; Di Battista, E. Cracking and crackling in concrete-like materials: A dynamic energy balance. Eng. Fract. Mech. 2016, 155, 130–144. [Google Scholar] [CrossRef]
- Rojo Tanzi, B.N.; Birck, G.; Sobczyk, M.; Iturrioz, I.; Lacidogna, G. Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials. Appl. Sci. 2023, 13, 5119. [Google Scholar] [CrossRef]




| Properties | |
|---|---|
| Young modulus (E) | 17 |
| Fracture toughness () | 70/ |
| Horizon () | |
| Discretization () | |
| Poisson’s ratio () | 0.25 |
| Coefficient of variation for () | 50% |
| Failure stress 1 | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almeida, W.R.; Tanzi, B.N.R.; Birck, G.; Iturrioz, I.; Lacidogna, G. Analysis of Damage Process in a Pre-Notched Rock Specimen: The Synergy between Experimental Results and Simulations Using a Peridynamic Model. Appl. Sci. 2024, 14, 4721. https://doi.org/10.3390/app14114721
Almeida WR, Tanzi BNR, Birck G, Iturrioz I, Lacidogna G. Analysis of Damage Process in a Pre-Notched Rock Specimen: The Synergy between Experimental Results and Simulations Using a Peridynamic Model. Applied Sciences. 2024; 14(11):4721. https://doi.org/10.3390/app14114721
Chicago/Turabian StyleAlmeida, William Ramires, Boris Nahuel Rojo Tanzi, Gabriel Birck, Ignacio Iturrioz, and Giuseppe Lacidogna. 2024. "Analysis of Damage Process in a Pre-Notched Rock Specimen: The Synergy between Experimental Results and Simulations Using a Peridynamic Model" Applied Sciences 14, no. 11: 4721. https://doi.org/10.3390/app14114721
APA StyleAlmeida, W. R., Tanzi, B. N. R., Birck, G., Iturrioz, I., & Lacidogna, G. (2024). Analysis of Damage Process in a Pre-Notched Rock Specimen: The Synergy between Experimental Results and Simulations Using a Peridynamic Model. Applied Sciences, 14(11), 4721. https://doi.org/10.3390/app14114721







