Reliability Evaluation and Reliability-Based Sensitivity for Transposition System in Power Servo Tool Holder
Abstract
1. Introduction
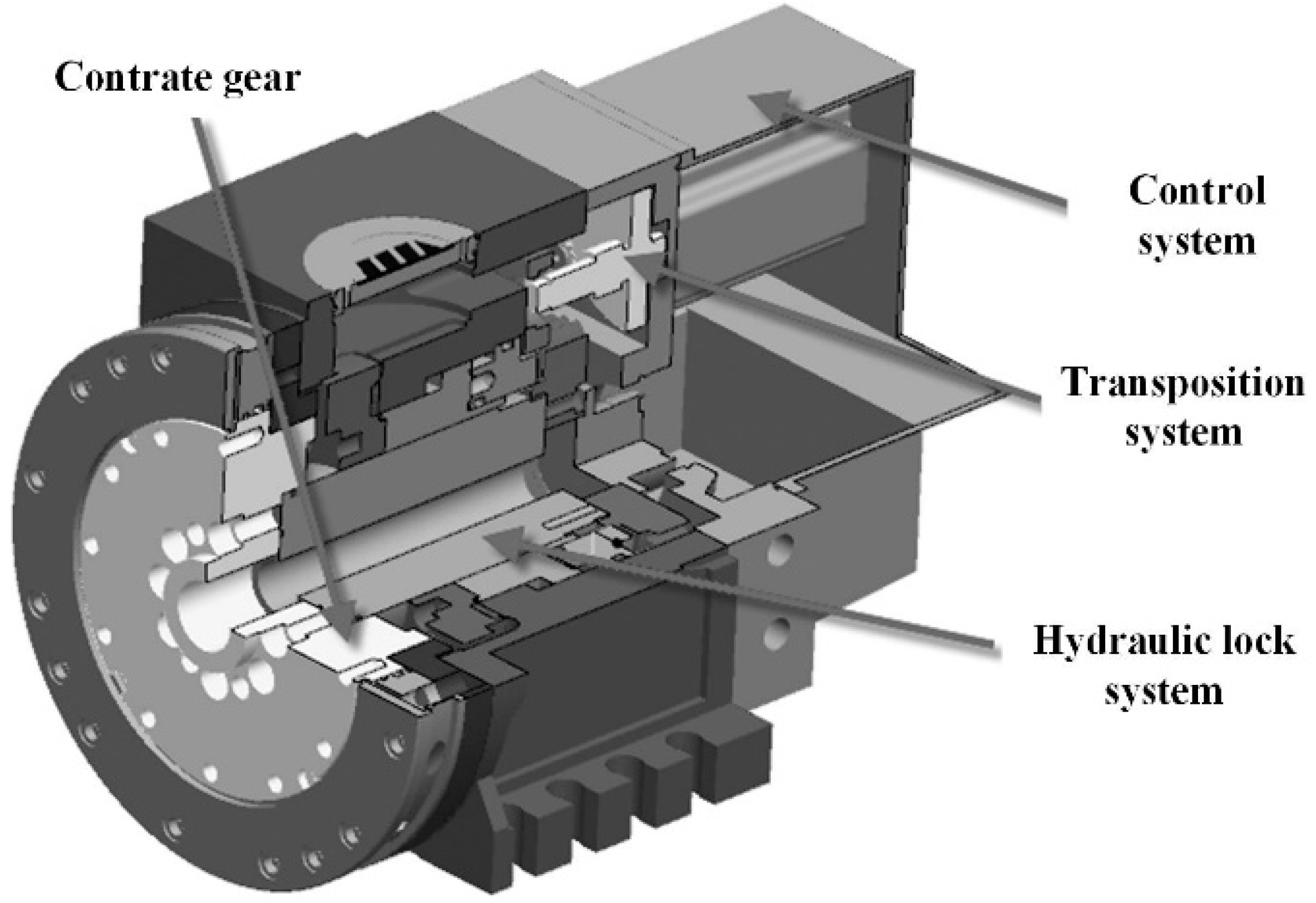
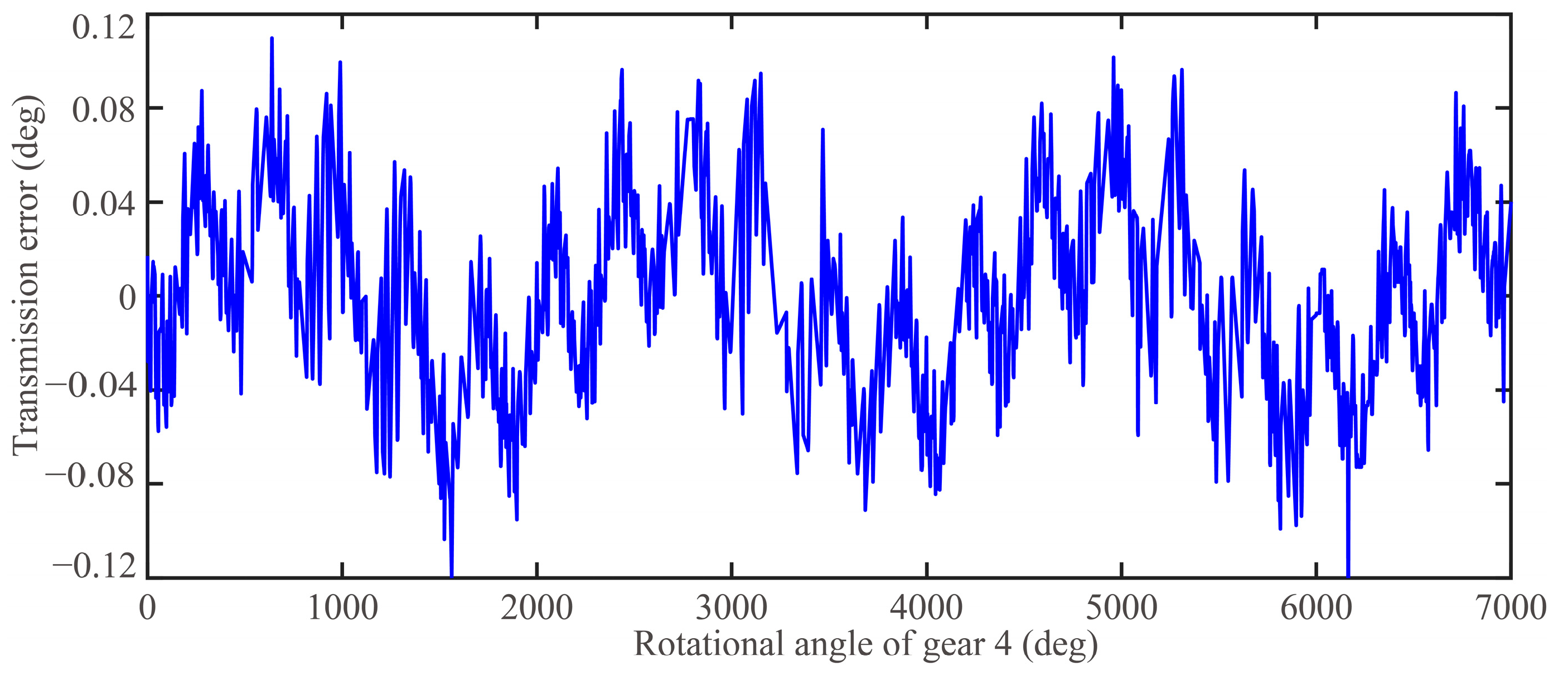
2. Reponses of Transposition System in Tool Holder
3. System Statistical Moments
4. Reliability Evaluation by Moments
5. Reliability-Based Sensitivity Analysis
6. Reliability Analysis for Transposition System
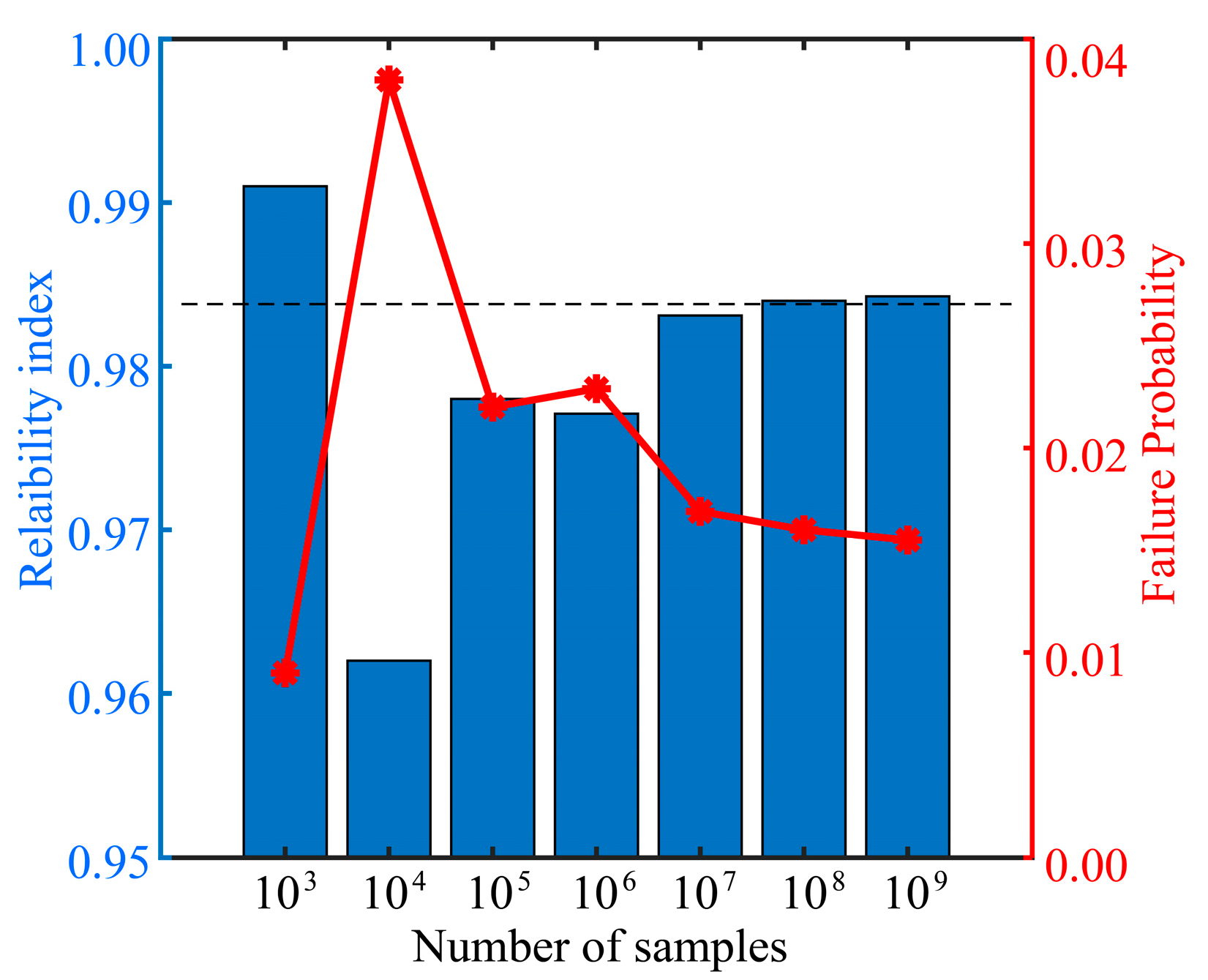
6.1. Reliability Evaluation
6.2. Reliability Optimization
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- An, Q.L.; Yang, J. A State-of-the-art Review on the Intelligent Tool Holders in Machining. Intell. Sustain. Manuf. 2024, 1, 10002. [Google Scholar] [CrossRef]
- Zhu, L.S.; Li, L.S. Research on Reliability for Servo Turret Based on Vibration Transmission Path System with Stiffness Degradation Model. Math. Probl. Eng. 2020, 2020, 3764546. [Google Scholar] [CrossRef]
- Wu, F.H.; Li, Y.X. The Design of Force Measuring Tool Holder System Based on Wireless Transmission. IEEE Access 2018, 6, 38556–38566. [Google Scholar] [CrossRef]
- Zhang, P.F.; Gao, D. A Novel Smart Toolholder with Embedded Force Sensors for Milling Operations. Mech. Syst. Signal Process 2022, 175, 109130. [Google Scholar] [CrossRef]
- Liu, J.F.; Luo, T.C. A Novel Fast Servo Tool Device with Double Piezoelectric Driving. Micromachines 2023, 14, 85. [Google Scholar] [CrossRef]
- Chen, Y.L.; Li, Z.W. Development of an Optimized Three-Axis Fast Tool Servo for Ultraprecision Cutting. IEEE/ASME Trans. Mech. 2022, 27, 3244–3254. [Google Scholar] [CrossRef]
- Huang, P.; Wu, X.Y. Deterioration of Form Accuracy Induced by Servo Dynamics Errors and Real-Time Compensation for Slow Tool Servo Diamond Turning of Complex-Shaped Optics. Int. J. Mach. Tools Manuf. 2020, 154, 103556. [Google Scholar] [CrossRef]
- Wang, L.P.; Zhu, B. Dynamic Performance Evaluation of Parallel Manipulators and Its Application in a 5-Axis Loading Device. Multibody Syst. Dyn. 2022, 56, 49–67. [Google Scholar] [CrossRef]
- Zhao, D.P.; Du, H.H. Development of a Novel Fast Tool Servo Using Topology Optimization. Int. J. Mech. Sci. 2023, 250, 108283. [Google Scholar] [CrossRef]
- Kriechenbauer, S.; Müller, P. Evolutionary Optimization of Deep-Drawing Processes on Servo Screw Presses with Freely Programmable Force and Motion Functions. Procedia CIRP 2021, 104, 1482–1487. [Google Scholar] [CrossRef]
- You, D.Z.; Pham, H. Reliability Analysis of the CNC System Based on Field Failure Data in Operating Environments. Qual. Reliab. Eng. Int. 2016, 32, 1955–1963. [Google Scholar] [CrossRef]
- Li, H.; Deng, Z.M. Reliability Analysis of the Main Drive System of a CNC Machine Tool Including Early Failures. Reliab. Eng. Syst. Saf. 2021, 215, 107846. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, Y.M. A Reliability Allocation Method of CNC Lathes Based on Copula Failure Correlation Model. Chin. J. Mech. Eng. 2018, 111, 31. [Google Scholar] [CrossRef]
- Yang, Z.; Zhu, Y.P. Comprehensive Reliability Allocation Method for CNC Lathes Based on Cubic Transformed Functions of Failure Mode and Effects Analysis. Chin. J. Mech. Eng. 2015, 28, 315–324. [Google Scholar] [CrossRef]
- Liu, Y.J.; Peng, H. Reliability Modeling and Evaluation Method of CNC Grinding Machine Tool. Appl. Sci. 2019, 9, 14. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Jia, Z.X. Reliability Prediction and FMEA of Loading and Unloading Truss Robot for CNC Punch. Appl. Sci. 2023, 13, 4951. [Google Scholar] [CrossRef]
- Rępalska, M.; Woźniak, A. Reliability of probes for CNC machine tools. Precis. Eng. 2024, 88, 767–776. [Google Scholar] [CrossRef]
- Duan, C.Q.; Deng, C. Reliability Assessment for CNC Equipment Based on Degradation Data. Int. J. Adv. Manuf. Technol. 2019, 100, 421–434. [Google Scholar] [CrossRef]
- Cheng, Q.; Cao, Y. A Health Management Technology Based on PHM for Diagnosis, Prediction of Machine Tool Servo System Failures. Appl. Sci. 2024, 14, 2656. [Google Scholar] [CrossRef]
- Zhang, Z.L.; Yang, Y.J. Machining Accuracy Reliability Evaluation of CNC Machine Tools Based on the Milling Stability Optimization. Int. J. Adv. Manuf. Technol. 2023, 124, 4057–4074. [Google Scholar] [CrossRef]
- Li, Y.F.; Zhang, Y.J. Accuracy Reliability Analysis of CNC Machine Tools Considering Manufacturing Errors Degrees. Proc. Inst. Mech. Eng. Part O J. Risk Reliab. 2024, 2383, 643–653. [Google Scholar] [CrossRef]
- Okwudire, C.E.; Lu, X. A Three-Tier Redundant Architecture for Safe and Reliable Cloud-Based CNC over Public Internet Networks. Robot. Comput. Manuf. 2020, 62, 101880. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, Y.M. Investigation on the Multifactor Reliability Allocation Method for CNC Lathes Based on Modified Criticality and Objective Information. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 1647–1656. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, H. Moment-Based Evaluation of Structural Reliability. Reliab. Eng. Syst. Saf. 2019, 181, 38–45. [Google Scholar] [CrossRef]
- Wu, J.H.; Tian, P.P. Reliability Analysis of Industrial Robot Positional Errors Based on Statistical Moment Similarity Metrics. Appl. Math. Model. 2024, 131, 1–21. [Google Scholar] [CrossRef]
- Xu, J.; Kong, F. An Efficient Method For Statistical Moments and Reliability Assessment of Structures. Struct. Multidiscip. Optim. 2018, 58, 2019–2035. [Google Scholar] [CrossRef]
- Ding, C.; Xu, J. An Improved Adaptive Bivariate Dimension-Reduction Method for Efficient Statistical Moment and Reliability Evaluations. Mech. Syst. Signal Process. 2021, 149, 107309. [Google Scholar] [CrossRef]
- Low, Y.M. A New Distribution for Fitting Four Moments and Its Applications to Reliability Analysis. Struct. Saf. 2013, 42, 12–25. [Google Scholar] [CrossRef]
- Zhang, T.X.; He, D. An Improved High-Order Statistical Moment Method for Structural Reliability Analysis with Insufficient Data. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 1050–1056. [Google Scholar] [CrossRef]
- Zhang, T.X. An Improved High-Moment Method for Reliability Analysis. Struct. Multidiscip. Optim. 2017, 56, 1225–1232. [Google Scholar] [CrossRef]
- Yılmaz, E.; Brian, J.G. Optimizing Resource Allocations to Improve System Reliability via the Propagation of Statistical Moments through Fault Trees. Reliab. Eng. Syst. Saf. 2023, 230, 108873. [Google Scholar] [CrossRef]
- Lu, H.; Cao, S. An Improved High Order Moment-Based Saddle-point Approximation Method for Reliability Analysis. Appl. Math. Model. 2020, 82, 836–847. [Google Scholar] [CrossRef]
- Zhou, D.; Pan, E.S. Dynamic Model-based Saddle-point Approximation for Reliability and Reliability-based Sensitivity Analysis. Reliab. Eng. Syst. Saf. 2020, 201, 106972. [Google Scholar] [CrossRef]
- Zhang, X.F.; Liu, J.K.; Yan, Y.; Pandey, M. An Effective Approach for Reliability-Based Sensitivity Analysis with the Principle of Maximum Entropy and Fractional Moments. Entropy 2019, 21, 649. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Y.L.; Xiao, Z.M.; Huan, L.R.; Fu, C. Simulation Analysis of Planetary Gears Train of Semi-Direct Drive Wind Turbine Based on ADAMS. Appl. Mech. Mater. 2015, 780–790, 311–315. [Google Scholar] [CrossRef]
- Lai, Q.; Yu, Q.; Dong, J. Dynamic Analysis of Rotary Tiller Gearbox Based on EDEM, ADAMS and ANSYS. J. Intell. Fuzzy Syst. 2019, 36, 1153–1160. [Google Scholar] [CrossRef]
- Zhou, D.; Pan, E.; Zhang, Y. Fractional polynomial function in stochastic response surface method for reliability analysis. J. Mech. Sci. Technol. 2021, 35, 121–131. [Google Scholar] [CrossRef]
- Zhou, D.; Zhang, X.; Zhang, Y. Reliability analysis on traction unit of shearer mechanism with response surface method. J. Mech. Sci. Technol. 2017, 31, 4679–4689. [Google Scholar] [CrossRef]
- Zhu, Z.F.; Du, X.P. Reliability Analysis with Monte Carlo Simulation and Dependent Kriging Predictions. ASME J. Mech. Des. 2016, 138, 121403. [Google Scholar] [CrossRef]
- Song, C.X.; Kawai, R. Monte Carlo and Variance Reduction Methods for Structural Reliability Analysis: A Comprehensive Review. Probab. Eng. Mech. 2023, 73, 103479. [Google Scholar] [CrossRef]
- Litvin, F.L.; Fuentes, A. Gear Geometry and Applied Theory; Cambridge University Press: New York, NY, USA, 2004; pp. 23–56. [Google Scholar]



| Parameter | Symbol | Gear 1 | Dual Gear 2 | Dual Gear 3 | Gear 4 | ||
|---|---|---|---|---|---|---|---|
| Modulus | m/mm | 1.5 | 1.5 | 1.75 | 1.75 | 3 | 3 |
| Number of teeth | Z | 18 | 54 | 13 | 52 | 9 | 54 |
| Modification coefficient | χ/mm | 0.4 | −0.4 | 0.3 | −0.3 | 0.33 | 0 |
| Tooth width | B/mm | 18 | 14 | 20 | 15 | 32 | 26.5 |
| X-coordinate | Xλ/mm (λ = 1,2,3,4) | 62.27 | 113.18 | 113.18 | 163.24 | 163.24 | 116 |
| Y-coordinate | Yλ/mm (λ = 1,2,3,4) | 198 | 216 | 216 | 189 | 189 | 106 |
| Transmission ratio | iξ (ξ = 12,23,34) | i12 = 3 | i23 = 4 | i34 = 6 | |||
| X1 | Y1 | X2 | Y2 | X3 | Y3 | X4 | Y4 | |
|---|---|---|---|---|---|---|---|---|
| 3rd Moment × 10−3 | 1.5 | 1.5 | 2.0 | 2.0 | 3.0 | 3.0 | 3.5 | 3.5 |
| 4th Moment × 10−5 | 6.0 | 6.0 | 6.0 | 6.0 | 8.0 | 8.0 | 10 | 10 |
| FOSM | AFOSM | Proposed Method | Monte Carlo | ||
|---|---|---|---|---|---|
| R | -- | 0.9674 | 0.9734 | 0.9838 | 0.9831 |
| ∂R/∂μX11 | X1 | −0.0981 | −0.1027 | −0.1079 | −0.1081 |
| ∂R/∂μX21 | Y1 | 0.0400 | 0.0314 | 0.0401 | 0.0399 |
| ∂R/∂μX31 | X2 | −0.2743 | −0.2160 | −0.2789 | −0.2599 |
| ∂R/∂μX41 | Y2 | 0.1300 | 0.1281 | 0.1214 | 0.1218 |
| ∂R/∂μX51 | X3 | 0.0227 | 0.0228 | 0.0250 | 0.0250 |
| ∂R/∂μX61 | Y3 | −0.1667 | −0.1677 | −0.1695 | −0.1699 |
| ∂R/∂μX71 | X4 | −0.0682 | −0.0695 | −0.0763 | −0.0787 |
| ∂R/∂μX81 | Y4 | 0.0097 | 0.0102 | 0.0115 | 0.0113 |
| Parameter | Before Optimization (mm) | After Optimization (mm) |
|---|---|---|
| X1 | 62.27 | 62.245 |
| Y1 | 198 | 198.024 |
| X2 | 113.18 | 113.137 |
| Y2 | 216 | 216.010 |
| X3 | 163.24 | 163.266 |
| Y3 | 189 | 188.969 |
| X4 | 116 | 115.979 |
| Y4 | 106 | 106.004 |
| R | 0.983795 | 0.999998 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, D.; Guo, Y.; Gu, G.; Man, Q.; Zhang, Y. Reliability Evaluation and Reliability-Based Sensitivity for Transposition System in Power Servo Tool Holder. Appl. Sci. 2024, 14, 7117. https://doi.org/10.3390/app14167117
Zhou D, Guo Y, Gu G, Man Q, Zhang Y. Reliability Evaluation and Reliability-Based Sensitivity for Transposition System in Power Servo Tool Holder. Applied Sciences. 2024; 14(16):7117. https://doi.org/10.3390/app14167117
Chicago/Turabian StyleZhou, Di, Yonglin Guo, Guojun Gu, Qixiang Man, and Yimin Zhang. 2024. "Reliability Evaluation and Reliability-Based Sensitivity for Transposition System in Power Servo Tool Holder" Applied Sciences 14, no. 16: 7117. https://doi.org/10.3390/app14167117
APA StyleZhou, D., Guo, Y., Gu, G., Man, Q., & Zhang, Y. (2024). Reliability Evaluation and Reliability-Based Sensitivity for Transposition System in Power Servo Tool Holder. Applied Sciences, 14(16), 7117. https://doi.org/10.3390/app14167117






