An Alternative Series Solution for Free Vibration Analysis of Asymmetric L-Shaped Membranes
Abstract
1. Introduction
2. Formulations
3. Results
3.1. Convergence for the Symmetric Case
3.2. Verification for the Symmetric Case
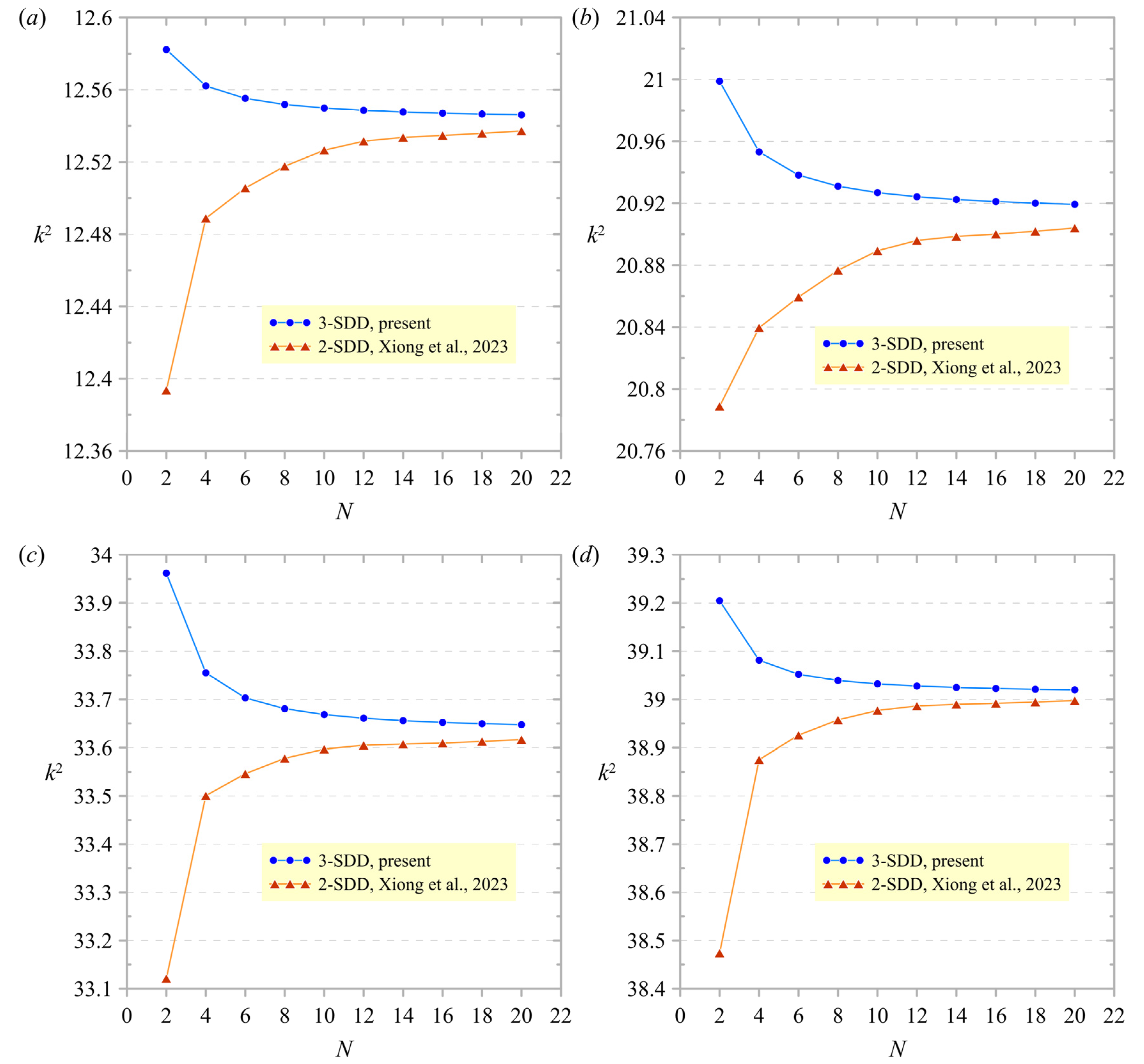
3.3. Convergence for the Asymmetric Case
3.4. Verification for the Asymmetric Case
4. Discussion
4.1. Convergence Behavior
4.2. Numerical Stability
4.3. Accuracy and Performance
4.4. Mode Shapes
4.5. Eigenvalue Extraction from Sub-Squares
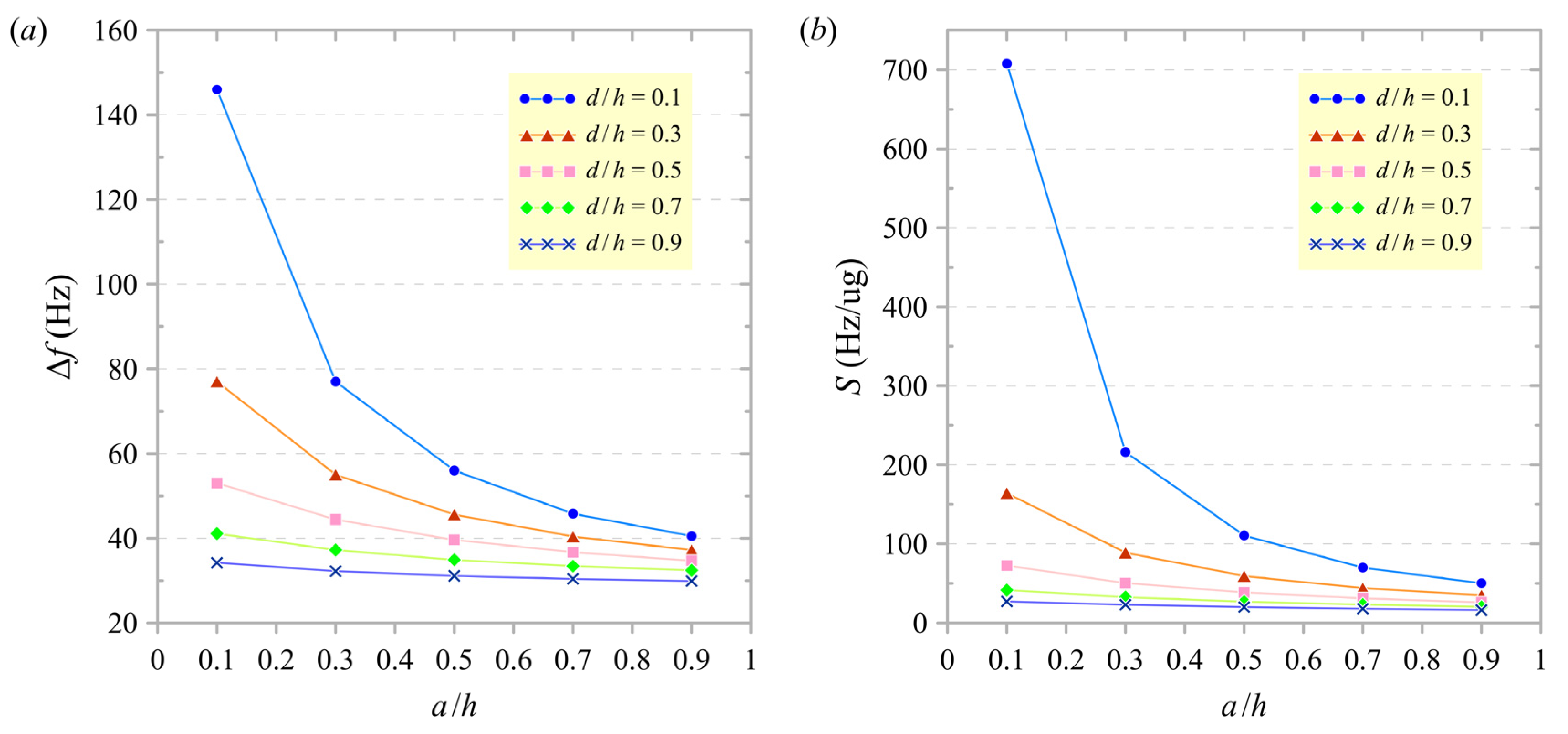
5. Potential Practical Applications
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tamadapu, G.; DasGupta, A. In-plane surface modes of an elastic toroidal membrane. Int. J. Eng. Sci. 2012, 60, 25–36. [Google Scholar] [CrossRef]
- Batra, R.C.; Porfiri, M.; Spinello, D. Analysis of electrostatic MEMS using meshless local Petrov-Galerkin (MLPG) method. Eng. Anal. Bound. Elem. 2006, 30, 949–962. [Google Scholar] [CrossRef]
- Wan, K.T.; Guo, S.; Dillard, D.A. A theoretical and numerical study of a thin clamped circular film under an external load in the presence of a tensile residual stress. Thin Solid Film. 2003, 425, 150–162. [Google Scholar] [CrossRef]
- Komaragiri, U.; Begley, M.R.; Simmonds, J.G. The mechanical response of freestanding circular elastic films under point and pressure loads. J. Appl. Mech. 2005, 72, 203–212. [Google Scholar] [CrossRef]
- Maner, K.C.; Begley, M.R.; Oliver, W.C. Nanomechanical testing and circular freestanding polymer films with sub-micron thickness. Acta Mater. 2004, 52, 5451–5460. [Google Scholar] [CrossRef]
- Espinosa, H.D.; Prorok, B.C.; Fischer, M. A methodology for determining mechanical properties of freestanding thin films and MEMS materials. J. Mech. Phys. Solids 2003, 51, 47–67. [Google Scholar] [CrossRef]
- Ruggiero, E.J.; Inman, D.J. Modeling and vibration control of an active membrane mirror. Smart. Mater. Struct. 2009, 18, 095027. [Google Scholar] [CrossRef]
- Zhang, J.; Cui, Y.; Li, Y.; Liu, Y. Free vibration and buckling of a stripped solar sail. J. Spacecr. Rocket. 2019, 56, 1267–1272. [Google Scholar] [CrossRef]
- Jenkins, C.H.M. Gossamer Spacecraft: Membrane and Inflatable Structures Technology for Space Applications; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2001. [Google Scholar]
- Kreyszig, E.; Kreyszig, H.; Norminton, E.J. Advanced Engineering Mathematics, 10th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Weaver, W. Vibration Problems in Engineering, 5th ed.; John Wiley & Sons: Hoboken, NJ, USA, 1990. [Google Scholar]
- Morse, P.M. Theoretical Acoustics; McGraw-Hill: New York, NY, USA, 1986. [Google Scholar]
- Heuer, R.; Irschik, H. A boundary element method for eigenvalue problems of polygonal membranes and plates. Acta Mech. 1987, 66, 9–20. [Google Scholar] [CrossRef]
- Kang, S.W.; Lee, J.M.; Kang, Y.J. Vibration analysis of arbitrarily shaped membranes using non-dimensional dynamic influence function. J. Sound Vib. 1999, 221, 117–132. [Google Scholar] [CrossRef]
- Karageorghis, A. The method of fundamental solutions for the calculation of the eigenvalues of the Helmholtz equation. Appl. Math. Lett. 2001, 14, 837–842. [Google Scholar] [CrossRef]
- Platte, R.B.; Driscoll, T.A. Computing eigenmodes of elliptic operators using radial basis functions. Comput. Math. Applic. 2004, 48, 561–576. [Google Scholar] [CrossRef]
- Betcke, T.; Trefethen, L.N. Reviving the method of particular solutions. SIAM Rev. 2005, 47, 469–491. [Google Scholar] [CrossRef]
- Buchanan, G.R.; Peddieson, J. A finite element in elliptic coordinates with application to membrane vibration. Thin-Walled Struct. 2005, 43, 1444–1454. [Google Scholar] [CrossRef]
- Houmat, A. Free vibration analysis of arbitrarily shaped membranes using the trigonometric p-version of the finite-element method. Thin-Walled Struct. 2006, 44, 943–951. [Google Scholar] [CrossRef]
- Houmat, A. A sector elliptic p-element applied to membrane vibrations. Thin-Walled Struct. 2009, 47, 172–177. [Google Scholar] [CrossRef]
- Amore, P.; Boyd, J.P.; Fernández, F.M.; Rösler, B. High order eigenvalues for the Helmholtz equation in complicated non-tensor domains through Richardson extrapolation of second order finite differences. J. Comput. Phys. 2016, 312, 252–271. [Google Scholar] [CrossRef]
- Alves, C.J.S.; Antunes, P.R.S. Determination of elastic resonance frequencies and eigenmodes using the method of fundamental solutions. Eng. Anal. Bound. Elem. 2019, 101, 330–342. [Google Scholar] [CrossRef]
- Ren, H.; Fan, W. An adaptive triangular element of absolute nodal coordinate formulation for thin plates and membranes. Thin-Walled Struct. 2023, 182, 110257. [Google Scholar] [CrossRef]
- Milsted, M.G.; Hutchinson, J.R. Use of trigonometric terms in the finite element method with application to vibrating membranes. J. Sound Vib. 1974, 32, 327–346. [Google Scholar] [CrossRef]
- Schiff, B. Finite element eigenvalues for the Laplacian over an L-shaped domain. J. Comput. Phys. 1988, 76, 233–242. [Google Scholar] [CrossRef]
- Amore, P. Solving the Helmholtz equation for membranes of arbitrary shape: Numerical results. J. Phys. A Math. Theor. 2008, 41, 265206. [Google Scholar] [CrossRef]
- Liu, X.; Oishi, S. Verified eigenvalue evaluation for the Laplacian over polygonal domains of arbitrary shape. SIAM J. Numer. Anal. 2013, 51, 1634–1654. [Google Scholar] [CrossRef]
- Fox, L.; Henrici, P.; Moler, C. Approximations and bounds for eigenvalues of elliptic operators. SIAM J. Numer. Anal. 1967, 4, 89–102. [Google Scholar] [CrossRef]
- Sideridis, A.B. A numerical solution of the membrane eigenvalue problem. Computing 1984, 32, 167–176. [Google Scholar] [CrossRef]
- Leung, A.Y.T.; Zhu, B.; Zheng, J.; Yang, H. A trapezoidal Fourier p-element for membrane vibrations. Thin-Walled Struct. 2003, 41, 479–491. [Google Scholar] [CrossRef]
- Still, G. Approximation theory methods for solving elliptic eigenvalue problems. Z. Angew. Math. Mech. 2003, 83, 468–478. [Google Scholar] [CrossRef]
- Wu, W.X.; Shu, C.; Wang, C.M. Vibration analysis of arbitrarily shaped membranes using local radial basis function-based differential quadrature method. J. Sound Vib. 2007, 306, 252–270. [Google Scholar] [CrossRef]
- Yuan, Q.; He, Z. Bounds to eigenvalues of the Laplacian on L-shaped domain by variational methods. J. Comput. Appl. Math. 2009, 233, 1803–1810. [Google Scholar] [CrossRef][Green Version]
- Jones, R.S. Computing ultra-precise eigenvalues of the Laplacian within polygons. Adv. Comput. Math. 2017, 43, 1325–1354. [Google Scholar] [CrossRef]
- Katsikadelis, J.T.; Sapountzakis, E.J. An approach to the vibration problem of homogeneous, non-homogeneous and composite membranes based on the boundary element method. Int. J. Numer. Meth. Eng. 1988, 26, 2439–2455. [Google Scholar] [CrossRef]
- Houmat, A. Hierarchical finite element analysis of the vibration of membranes. J. Sound Vib. 1997, 201, 465–472. [Google Scholar] [CrossRef]
- Fantuzzi, N.; Tornabene, F.; Viola, E. Generalized differential quadrature finite element method for vibration analysis of arbitrarily shaped membranes. Int. J. Mech. Sci. 2014, 79, 216–251. [Google Scholar] [CrossRef]
- Xiong, C.H.; He, L.G.; Chang, K.H.; Huang, C.W. Free vibration analysis of a tunable micro-fabrication device comprising asymmetric L-shaped membranes. Polymers 2023, 15, 2293. [Google Scholar] [CrossRef]
- Sandmaier, H.; Kuhl, K. A square-diaphragm piezoresistive pressure sensor with a rectangular central boss for low-pressure ranges. IEEE Trans. Electron Devices 1993, 40, 1754–1759. [Google Scholar] [CrossRef]
- Huang, X.; Zhang, D. A high sensitivity and high linearity pressure sensor based on a peninsula-structured diaphragm for low-pressure ranges. Sens. Actuators A Phys. 2014, 216, 176–189. [Google Scholar] [CrossRef]
- Kinnell, P.K.; King, J.; Lester, M.; Craddock, R. A hollow stiffening structure for low-pressure sensors. Sens. Actuators A Phys. 2010, 160, 35–41. [Google Scholar] [CrossRef]
- Achouch, S.; Bousseta, R.; Regragui, F.; Gharbi, M. Conception and realization of a novel design for a capacitive pressure sensor with high sensitivity and linear response. Results Eng. 2022, 15, 100535. [Google Scholar] [CrossRef]
- Kang, S.W.; Lee, J.M. Free vibration analysis of composite rectangular membranes with an oblique interface. J. Sound Vib. 2002, 251, 505–517. [Google Scholar] [CrossRef]
- Kang, S.W. Free vibration analysis of composite rectangular membranes with a bent interface. J. Sound Vib. 2004, 272, 39–53. [Google Scholar] [CrossRef]
- Gottlieb, H.P.W. On vibrations of L-shaped regions. J. Sound Vib. 2001, 246, 387–388. [Google Scholar] [CrossRef][Green Version]
- Morse, P.M.; Feshbach, H. Methods of Theoretical Physics; McGraw-Hill: New York, NY, USA, 1953; p. 1364. [Google Scholar]
- Kang, J.H.; Wei, B. Degenerate mode shapes for rectangular membranes and simply supported rectangular plates. J. Sound Vib. 2013, 332, 1633–1638. [Google Scholar] [CrossRef]
- Sanz-Jiménez, A.; Ruz, J.J.; Gil-Santos, E.; Malvar, O.; García-López, S.; Kosaka, P.M.; Cano, Á.; Calleja, M.; Tamayo, J. Square membrane resonators supporting degenerate modes of vibration for high-throughput mass spectrometry of single bacterial cells. ACS Sens. 2023, 8, 2060–2067. [Google Scholar] [CrossRef] [PubMed]
- Pang, W.; Zhao, H.; Kim, E.S.; Zhang, H.; Yu, H.; Hu, X. Piezoelectric microelectromechanical resonant sensors for chemical and biological detection. Lab Chip 2012, 12, 29–44. [Google Scholar] [CrossRef] [PubMed]
- Hajjam, A.; Wilson, J.C.; Pourkamali, S. Individual air-borne particle mass measurement using high-frequency micromechan-ical resonators. IEEE Sens. J. 2011, 11, 2883–2890. [Google Scholar] [CrossRef]
- Choi, J.S.; Park, W.T. MEMS particle sensor based on resonant frequency shifting. Micro Nano Syst. Lett. 2020, 8, 1–6. [Google Scholar]
- Nazemi, H.; Antony Balasingam, J.; Swaminathan, S.; Ambrose, K.; Nathani, M.U.; Ahmadi, T.; Babu Lopez, Y.; Emadi, A. Mass sensors based on capacitive and piezoelectric micromachined ultrasonic transducers—CMUT and PMUT. Sensors 2020, 20, 2010. [Google Scholar] [CrossRef]
- Park, S.J.; Lee, H.B.; Kim, G.W. Eardrum-inspired soft viscoelastic diaphragms for CNN-Based speech recognition with audio visualization images. Sci. Rep. 2023, 13, 6414. [Google Scholar] [CrossRef]



| Dimensionless Parameters | d/h | a/h | b/h |
|---|---|---|---|
| Symmetric Case | 1.00 | 1.00 | 1.00 |
| Asymmetric Case | 0.57 | 1.24 | 0.68 |
| N | ||||||
|---|---|---|---|---|---|---|
| 10 | 60 | 120 | 1000 | 1600 | 1800 | |
| 1 | 9.6558 | 9.6413 | 9.6403 | 9.6398 | 9.6397 | 9.6397 |
| 2 | 15.1978 | 15.1973 | 15.1973 | 15.1973 | 15.1973 | 15.1973 |
| 3 | 19.7392 | 19.7392 | 19.7392 | 19.7392 | 19.7392 | 19.7392 |
| 4 | 29.5217 | 29.5215 | 29.5215 | 29.5215 | 29.5215 | 29.5215 |
| 5 | 31.9521 | 31.9164 | 31.9142 | 31.9127 | 31.9127 | 31.9127 |
| 6 | 41.5043 | 41.4774 | 41.4757 | 41.4746 | 41.4745 | 41.4745 |
| 7 | 44.9511 | 44.9485 | 44.9485 | 44.9485 | 44.9485 | 44.9485 |
| 8 | 49.3480 | 49.3480 | 49.3480 | 49.3480 | 49.3480 | 49.3480 |
| 9 | 49.3480 | 49.3480 | 49.3480 | 49.3480 | 49.3480 | 49.3480 |
| 10 | 56.7430 | 56.7128 | 56.7109 | 56.7097 | 56.7096 | 56.7096 |
| Present | Yuan and He [33] | Still [31] | Leung et al. [30] * | Wu et al. [32] * | |
|---|---|---|---|---|---|
| 1 | 9.6397 | 9.6397 | 9.6397 | 9.6814 | 9.6870 |
| 2 | 15.1973 | 15.1973 | 5.1973 | 15.2022 | 15.1960 |
| 3 | 19.7392 | 19.7392 | 19.7474 | 19.7394 | |
| 4 | 29.5215 | 29.5215 | 29.5215 | 29.5316 | 29.5218 |
| 5 | 31.9127 | 31.9126 | 31.9126 | 32.0560 | 32.0277 |
| 6 | 41.4745 | 41.4745 | 41.4745 | 41.5612 | 41.5625 |
| 7 | 44.9485 | 44.9485 | 44.9485 | 44.9798 | 44.9423 |
| 8 | 49.3480 | 49.3480 | 49.3773 | 49.3492 | |
| 9 | 49.3480 | 49.3480 | 49.4195 | - | |
| 10 | 56.7096 | 56.7096 | 56.7096 | 56.8878 | - |
| N | ||||||
|---|---|---|---|---|---|---|
| 20 | 80 | 160 | 240 | 420 | 520 | |
| 1 | 12.5462 | 12.5439 | 12.5436 | 12.5435 | 12.5435 | 12.5435 |
| 2 | 20.9193 | 20.9149 | 20.9143 | 20.9142 | 20.9141 | 20.9141 |
| 3 | 33.6475 | 33.6362 | 33.6349 | 33.6346 | 33.6343 | 33.6343 |
| 4 | 39.0199 | 39.0129 | 39.0121 | 39.0119 | 39.0117 | 39.0117 |
| 5 | 44.0337 | 44.0335 | 44.0335 | 44.0335 | 44.0335 | 44.0335 |
| 6 | 51.4015 | 51.3983 | 51.3978 | 51.3977 | 51.3976 | 51.3976 |
| 7 | 57.7097 | 57.7091 | 57.7090 | 57.7089 | 57.7089 | 57.7089 |
| 8 | 63.7629 | 63.7609 | 63.7606 | 63.7606 | 63.7605 | 63.7605 |
| 9 | 77.7272 | 77.7215 | 77.7210 | 77.7208 | 77.7207 | 77.7207 |
| 10 | 84.7964 | 84.7879 | 84.7869 | 84.7867 | 84.7864 | 84.7864 |
| k2 | 3-SDD, Present | 2-SDD, [38] | Abaqus, [38] |
|---|---|---|---|
| 1 | 12.5435 | 12.5436 | 12.5436 |
| 2 | 20.9141 | 20.9142 | 20.9151 |
| 3 | 33.6343 | 33.6342 | 33.6342 |
| 4 | 39.0117 | 39.0113 | 39.0125 |
| 5 | 44.0335 | 44.0338 | 44.0325 |
| 6 | 51.3976 | 51.3974 | 51.3989 |
| 7 | 57.7089 | 57.7083 | 57.7099 |
| 8 | 63.7605 | 63.7602 | 63.7602 |
| 9 | 77.7207 | 77.7201 | 77.7219 |
| 10 | 84.7864 | 84.7854 | 84.7873 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, K.-H.; Kuo, W.-T. An Alternative Series Solution for Free Vibration Analysis of Asymmetric L-Shaped Membranes. Appl. Sci. 2024, 14, 7156. https://doi.org/10.3390/app14167156
Chang K-H, Kuo W-T. An Alternative Series Solution for Free Vibration Analysis of Asymmetric L-Shaped Membranes. Applied Sciences. 2024; 14(16):7156. https://doi.org/10.3390/app14167156
Chicago/Turabian StyleChang, Kao-Hao, and Wen-Ten Kuo. 2024. "An Alternative Series Solution for Free Vibration Analysis of Asymmetric L-Shaped Membranes" Applied Sciences 14, no. 16: 7156. https://doi.org/10.3390/app14167156
APA StyleChang, K.-H., & Kuo, W.-T. (2024). An Alternative Series Solution for Free Vibration Analysis of Asymmetric L-Shaped Membranes. Applied Sciences, 14(16), 7156. https://doi.org/10.3390/app14167156






