A Method to Estimate Dynamic Pore Water Pressure Growth of Saturated Sand-Gravel Materials
Abstract
:1. Introduction
2. Test Design
2.1. Test Apparatus and Sample Preparation
2.2. Test Conditions
3. Analysis of Dynamic Triaxial Test Results of Saturated Sand-Gravel Material
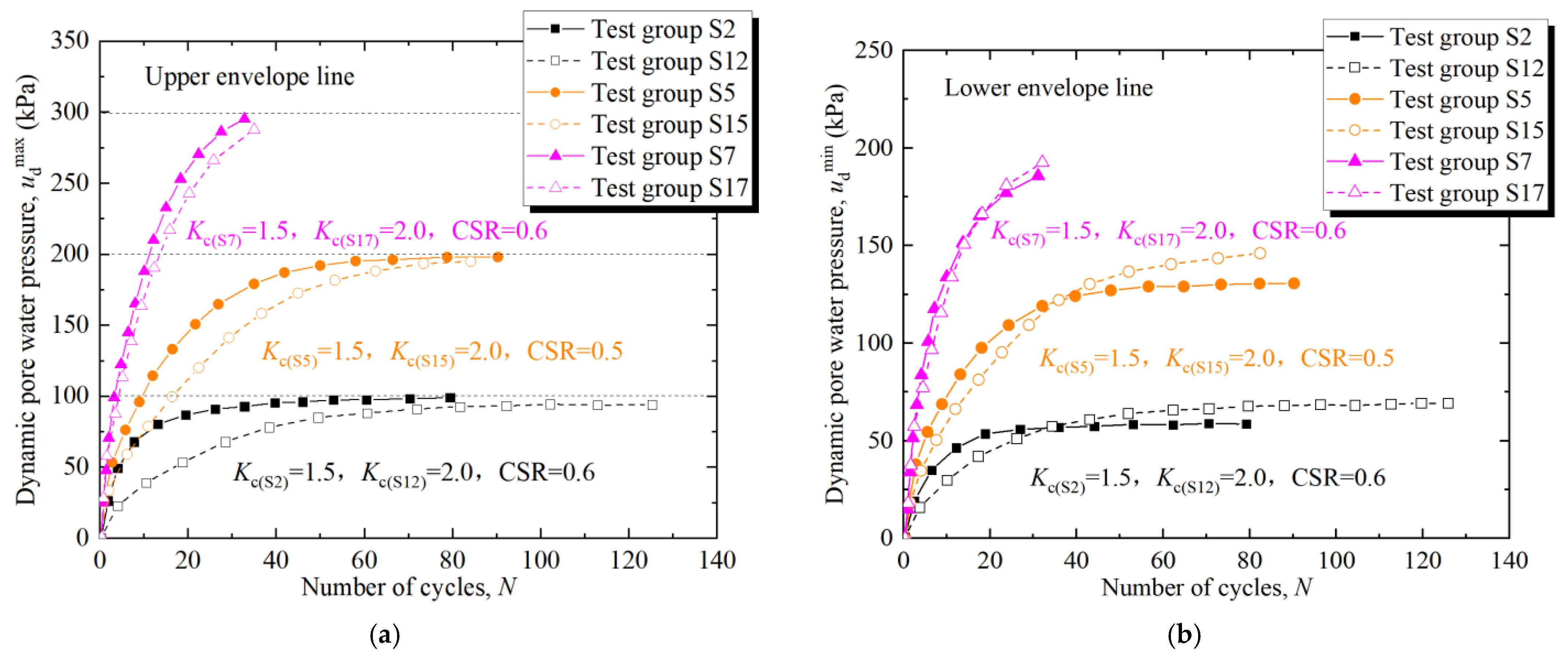
3.1. Dynamic Pore Water Pressure Curve
3.2. Relationship between the Strain and the Cyclic Numbers
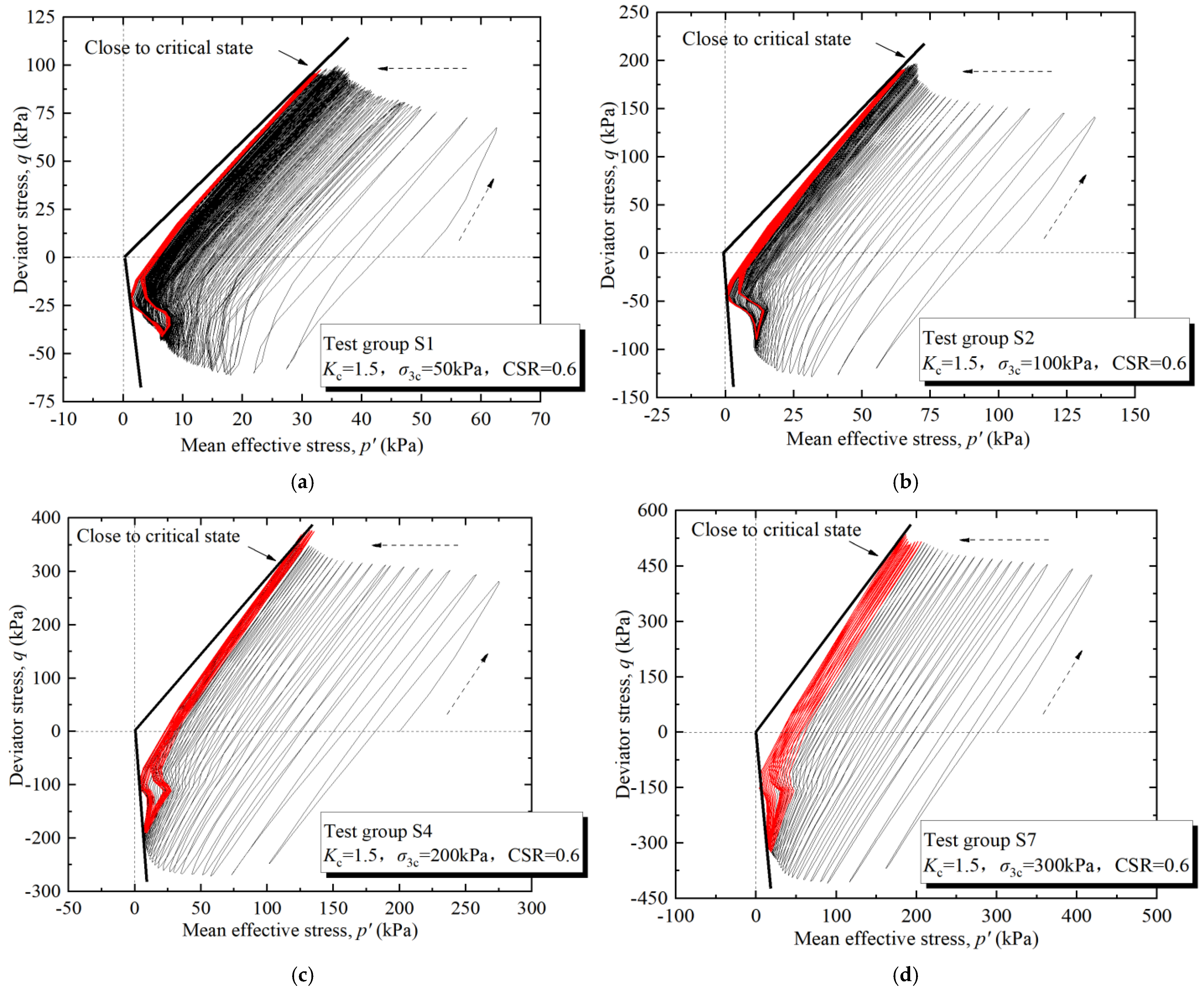
3.3. Effective Stress Path
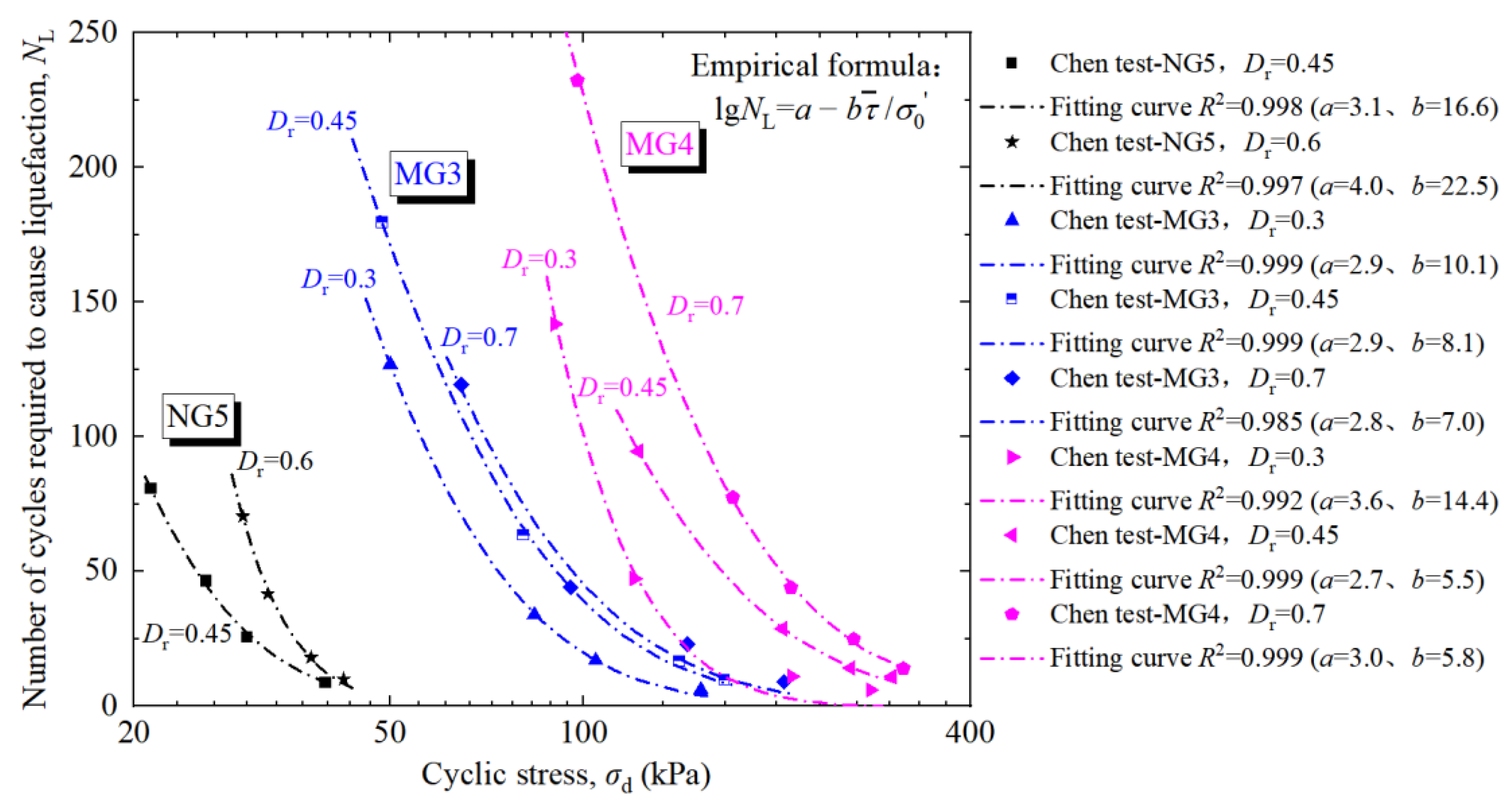
4. The Estimation Model of the Number of Cycles Required to Reach Liquefaction, NL
4.1. The Verification of NL
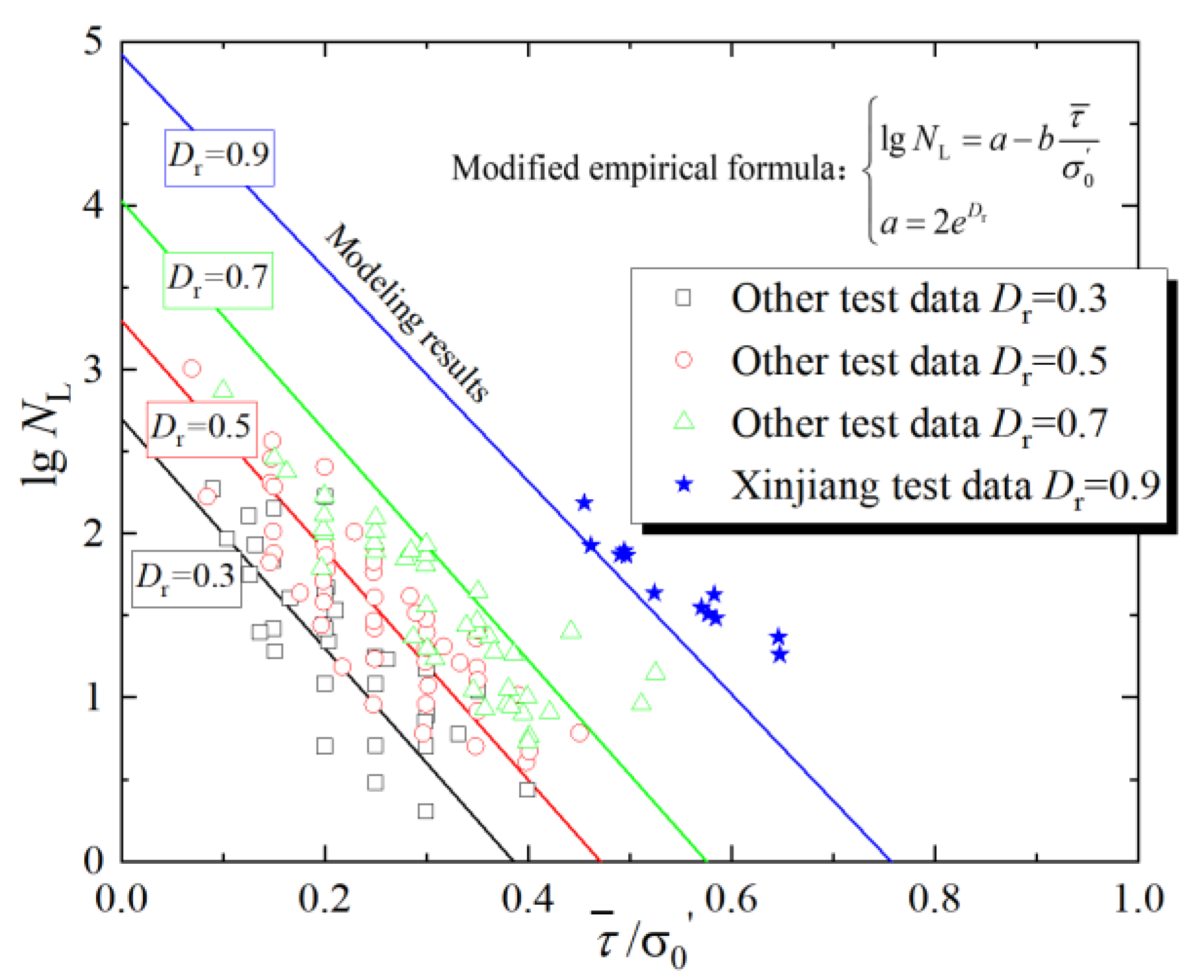
4.2. The Modification of NL
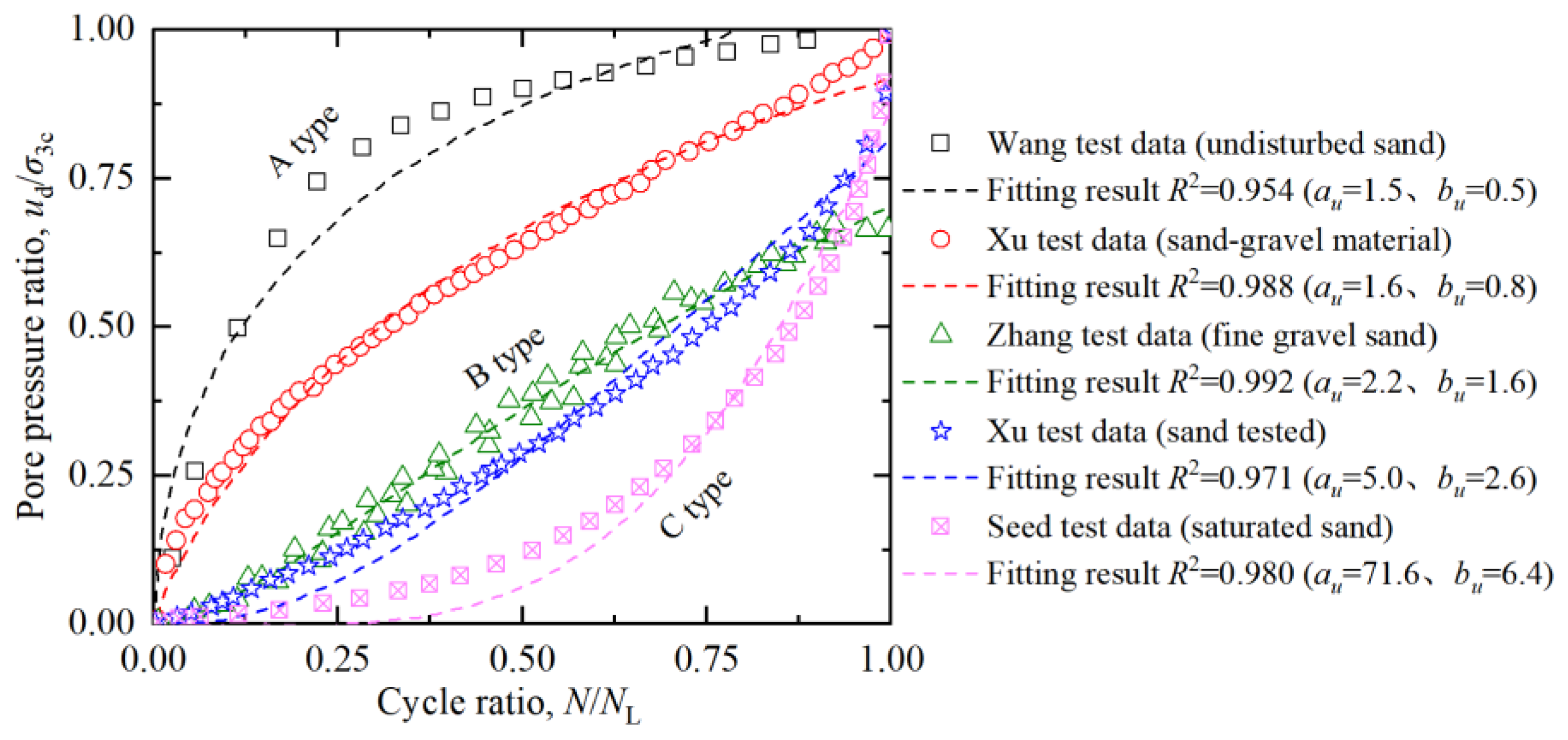
5. Estimation of the Dynamic Pore Water Pressure of the Saturated Sand-Gravel Material
6. Conclusions
- (1)
- Dislocation and slip occur between the grains of the saturated sand-gravel material under cyclic loading. This causes both reversible and irreversible two-part volume strains, causing an increase in the periodic fluctuation of the dynamic pore water pressure. The increase in the dynamic pore water pressure is more obvious and the amplitude is larger due to the large pores between the sand-gravel materials. The dense saturated sand-gravel material with its large consolidation stress ratio shows a small volume shrinkage and a large volume expansion, where dilatancy can inhibit the increase in the pore water pressure in the sand-gravel.
- (2)
- During cyclic loading, volume expansion or shrinkage occurs alternately in the saturated sand-gravel material. The mean effective stress of the sand-gravel material decreases, causing the dynamic shear modulus of the material to decrease and the dynamic strain of the material to increase under the same dynamic stress conditions.
- (3)
- Even if the relative density of the sand-gravel material reaches 90%, there is still the possibility of liquefaction under dynamic loading. Earth-rock dams that are built on sand-gravel overburdens and earth-rock dams filled with sand-gravel materials in earthquake-prone areas should use our proposed analysis and evaluation method to investigate the possibility of sand-gravel liquefaction. We used test data and considered the influence of relative density to modify the existing empirical formula and provide an evaluation method for the number of cycles required to reach liquefaction. We propose a pore water pressure growth model that is suitable for both sand-gravel and sandy materials. Comparisons and validations demonstrated that the proposed model accurately describes the three types of pore water pressure growth processes during liquefaction. The pore pressure growth model proposed in this paper can be used to analyze the liquefaction resistance of the dam foundations and dam structure of high earth-rock dams and high sand-gravel dams located on deep overburdens.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, C.S.; Yue, C.; Du, X.L.; Liang, K.; Wang, B. Experimental study on the influence of cyclic loading frequency on liquefaction characteristics of saturated sand. Géotechnique 2024, 25, 1–14. [Google Scholar] [CrossRef]
- Sahin, A.; Cetin, K.O. Gravelly Liquefaction Case Histories after 2008 Wenchuan-China Earthquake Mw = 7.9. Int. J. Geosyn. Ground Eng. 2023, 9, 42. [Google Scholar] [CrossRef]
- Zhou, Y.G.; Xia, P.; Ling, D.S.; Chen, Y.M. Liquefaction case studies of gravelly soils during the 2008 Wenchuan earthquake. Eng. Geol. 2020, 274, 105691. [Google Scholar] [CrossRef]
- Yuan, J.Y.; Wang, Y.L.; Zhan, B.L.; Yuan, X.M.; Wu, X.Y.; Ma, J.J. Comprehensive investigation and analysis of liquefaction damage caused by the Ms7. 4 Maduo earthquake in 2021 on the Tibetan Plateau, China. Soil Dyn. Earthq. Eng. 2022, 155, 107191. [Google Scholar] [CrossRef]
- Casagrand, A. Characteristics of cohesionless soils affecting the stability of slopes and earth fills. Bost. Soc. Civ. Eng. 1936, 23, 13–32. [Google Scholar]
- Seed, H.B.; Idriss, I.M. A Simplified procedure for evaluating soil liquefaction potential. Soil Mech. Found. Div. J. 1971, 97, 1249–1273. [Google Scholar] [CrossRef]
- Booker, J.R.; Rahman, M.S.; Seed, H.B. GADFLEA: A Computer Program for the Analysis of Pore Pressure Generation and Dissipation during Cyclic or Earthquake Loading; College of Engineering, University of California: Berkeley, CA, USA, 1976; pp. 24–76. [Google Scholar]
- Finn, W.L.; Lee, K.W.; Martin, G.R. An effective stress model for liquefaction. J. Geotech. Eng. Div. 1977, 103, 517–533. [Google Scholar] [CrossRef]
- Xu, Z.Y.; Shen, Z.J. 2-D dynamic analysis of effective stresses of seismic liquefaction. J. East China Tech. Univ. Water Resour. 1981, 2, 1–14. [Google Scholar] [CrossRef]
- Zhang, J.M.; Xie, D.Y. A practical algorithm for increasing pore water pressure of saturated sand vibrating. J. Hydraul. Eng. 1991, 8, 45–51. [Google Scholar] [CrossRef]
- Martin, G.R.; Finn, W.; Seed, H.B. Fundamentals of liquefaction under cyclic loading. J. Geotech. Eng. Div. 1975, 101, 423–438. [Google Scholar] [CrossRef]
- Xie, D.Y. Soil Dynamics; Higher Education Press: Beijing, China, 2011. [Google Scholar]
- Cao, Y.L.; He, G.N.; Lin, G. Energy analysis of the rise degree of vibrational pore water pressure in soil. J. Dalian Inst. Technol. 1987, 26, 83–89. [Google Scholar]
- Finn, W.; Bhatia, S.K. Prediction of seismic porewater pressures. In Proceedings of the 10th International Conference on Soil Mechanics and Foundation Engineering (Stockholm), Stockholm, Sweden, 15 June 1981. [Google Scholar]
- Ishihara, K. Soil Behavior in Earthquake Geotechnics; Oxford University Press: New York, NY, USA, 1996. [Google Scholar]
- Huang, C.X.; Cao, T.; Wang, L.; Zhang, L.; Chen, Y.S.; Wang, L. Experimental study on liquefaction influence factors of saturated silty soil using orthogonal design method. Bull. Eng. Geol. Environ. 2024, 83, 304. [Google Scholar] [CrossRef]
- Amini, P.F.; Yang, J. Energy-based liquefaction assessment of partially and fully saturated clayey sands. Eng. Geol. 2024, 331, 107434. [Google Scholar] [CrossRef]
- Cai, Y.B.; Zhang, Y.L.; Qi, Q.J.; Cheng, Y.; Shi, Y.B.; Sun, Z. Experimental study on strength and liquefaction characteristics of sand under dynamic loading. Sustainability 2023, 15, 10306. [Google Scholar] [CrossRef]
- Vargas, R.R.; Ueda, K.; Uemura, K. Influence of the relative density and K0 effects in the cyclic response of Ottawa F-65 sand–cyclic Torsional Hollow-Cylinder shear tests for LEAP-ASIA-2019. Soil Dyn. Earthq. Eng. 2020, 133, 106111. [Google Scholar] [CrossRef]
- Lakkimsetti, B.; Latha, G.M. Role of grain size and shape on undrained monotonic shear, liquefaction, and post-liquefaction behaviour of granular ensembles. Soil Dyn. Earthq. Eng. 2023, 173, 108086. [Google Scholar] [CrossRef]
- Haeri, S.M.; Nikoonejad, K. Liquefaction behavior of a well-graded gravelly soil under initial static shear stress in cyclic triaxial and simple shear conditions. Int. J. Geomech. 2023, 23, 4023053. [Google Scholar] [CrossRef]
- Wang, X.L.; Xu, B.; Su, H.; Pang, R. Elastoplastic constitutive model of gravelly soil and its validation through numerical analysis of the Kobe Port Island liquefaction. Comput. Geotech. 2024, 169, 106213. [Google Scholar] [CrossRef]
- Kim, J.; Athanasopoulos-Zekkos, A.; Cubrinovski, M. Monotonic and cyclic simple shear response of well-graded sandy gravel soils from Wellington, New Zealand. J. Geotech. Geoenviron. Eng. 2023, 149, 04023046. [Google Scholar] [CrossRef]
- Pokhrel, A.; Chiaro, G.; Kiyota, T.; Cubrinovski, M. Liquefaction characteristics of sand-gravel mixtures: Experimental observations and its assessment based on intergranular state concept. Soils Found. 2024, 64, 101444. [Google Scholar] [CrossRef]
- Yuan, X.M.; Wang, H.; Cao, Z.Z.; Xu, H.X. Interpretation to characteristics of gravelly soils sites that liquefied in Wenchuan earthquake. Chin. J. Geophys. 2017, 60, 2733–2743. [Google Scholar] [CrossRef]
- Xu, B.; Kong, X.J.; Zou, D.G.; Lou, S.L. Study of dynamic pore water pressure and axial strain in saturated sand-gravel composites. Rock Soil Mech. 2006, 27, 925–928. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, Y.F.; Zhang, L.K.; Liu, Z.J. Influence of confining pressure and vibration frequency on the liquefaction strength of the saturated gravel sand. J. Xinjiang Agric. Univ. 2015, 38, 68–71. [Google Scholar] [CrossRef]
- Shamoto, Y.; Zhang, J.M.; Tokimatsu, K. New methods for evaluating large residual post-liquefaction ground settlement and horizontal displacement. Soils Found. 1998, 38, 69–84. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.M. Reversible and irreversible dilatancy of sand. Chin. J. Geotech. Eng. 2000, 22, 12–17. [Google Scholar] [CrossRef]
- Xu, Z.Y.; Shen, Z.J. Dynamic analysis of effective stress for seismic strengthening of Yuecheng Reservoir earth dam. Earthq. Eng. Eng. Vib. 1983, 3, 89–109. [Google Scholar] [CrossRef]
- Gong, X.Y.; Wang, M.M.; Yang, S.G.; Hu, S.M.; Gou, C. Dynamic response analysis of an upstream tailings dam under earthquake. Nonferrous Met. (Min. Sect.) 2022, 74, 71–77. [Google Scholar] [CrossRef]
- Ai, X.Q.; Li, J. Seismic response analysis of underground pipelines under effective stress. J. Disaster Prev. Mitig. Eng. 2005, 25, 1–7. [Google Scholar] [CrossRef]
- Xu, Z.Y.; Zhou, J. Dynamic analysis of three-dimensional drainage effective stress of Auroville earth dam. J. Hydraul. Eng. 1991, 19–27+34. [Google Scholar] [CrossRef]
- Chen, G.X.; Wu, Q.; Sun, T.; Zhao, K.; Zhou, E.Q.; Xu, L.Y.; Zhou, Y.G. Cyclic behaviors of saturated sand-gravel mixtures under undrained cyclic triaxial loading. J. Earthq. Eng. 2021, 25, 756–789. [Google Scholar] [CrossRef]
- Liu, X.; Li, S.; Sun, L.Q. The study of dynamic properties of carbonate sand through a laboratory database. Bull. Eng. Geol. Environ. 2020, 79, 3843–3855. [Google Scholar] [CrossRef]
- Eseller, E.E.; Monkul, M.M.; Akin, Ö.; Yenigun, S. The coupled influence of relative density, CSR, plasticity and content of fines on cyclic liquefaction resistance of sands. J. Earthq. Eng. 2017, 23, 909–929. [Google Scholar] [CrossRef]
- Hoque, M.M.; Ansary, M.A.; Yasin, S.J.M. Effects of relative density and effective confining pressure on liquefaction resistance of sands. In Proceedings of the 19th International Conference on Soil Mechanics and Geotechnical Engineering, ICSMGE, Seoul, Republic of Korea, 17–22 September 2017. [Google Scholar]
- Zhang, B.; Muraleetharan, K.K.; Liu, C. Liquefaction of unsaturated sands. Int. J. Geomech. 2016, 16, 1–8. [Google Scholar] [CrossRef]
- Chen, G.X.; Sun, T.; Wang, B.H.; Li, X.J. Undrained cyclic failure mechanisms and resistance of saturated sand-gravel mixtures. Chin. J. Geotech. Eng. 2015, 37, 2140–2148. [Google Scholar] [CrossRef]
- Salem, M.; Elmamlouk, H.; Agaiby, S. Static and cyclic behavior of North Coast calcareous sand in Egypt. Soil Dyn. Earthq. Eng. 2013, 55, 83–91. [Google Scholar] [CrossRef]
- Xenaki, V.C.; Athanasopoulos, G.A. Dynamic properties and liquefaction resistance of two soil materials in an earthfill dam–Laboratory test results. Soil Dyn. Earthq. Eng. 2008, 28, 605–620. [Google Scholar] [CrossRef]
- Wang, B.H.; Liu, J.D.; Chen, G.X. Effects of relative density and structural properties on liquefaction resistance of Nanjing fine sand. J. Disaster Prev. Mitig. Eng. 2007, 27, 383–388+400. [Google Scholar] [CrossRef]
- Lin, P.S.; Chang, C.W. Damage investigation and liquefaction potential analysis of gravelly soil. J. Chin. Inst. Eng. 2002, 25, 543–554. [Google Scholar] [CrossRef]
- Chien, L.K.; Oh, Y.N.; Chang, C.H. Effects of fines content on liquefaction strength and dynamic settlement of reclaimed soil. Can. Geotech. J. 2002, 39, 254–265. [Google Scholar] [CrossRef]
- Evans, M.D.; Zhou, S. Liquefaction behavior of sand-gravel composites. J. Geotech. Eng. 1995, 121, 287–298. [Google Scholar] [CrossRef]
- Alba, P.D.; Seed, H.B.; Chan, C.K. Sand liquefaction in large-scale simple shear tests. J. Geotech. Eng. Div. 1976, 102, 909–927. [Google Scholar] [CrossRef]
- Seed, H.B.; Martin, P.P.; Lysmer, J. The Generation and Dissipation of Pore Water Pressures during Soil Liquefaction; University of California: Los Angeles, CA, USA, 1975. [Google Scholar]
- Wang, G.; Yin, H.; Zheng, H.H.; Yang, J. Comparative experimental study on liquefaction characteristics of carbonate and silica sands. J. Eng. Geol. 2021, 29, 69–76. [Google Scholar] [CrossRef]
- Seed, H.B.; Lysmer, J.; Martin, P.P. Pore-water pressure changes during soil liquefaction. J. Geotech. Eng. Div. 1976, 4, 323–346. [Google Scholar] [CrossRef]






| Pore Water Pressure Model | Equation | Explanation | Reference |
|---|---|---|---|
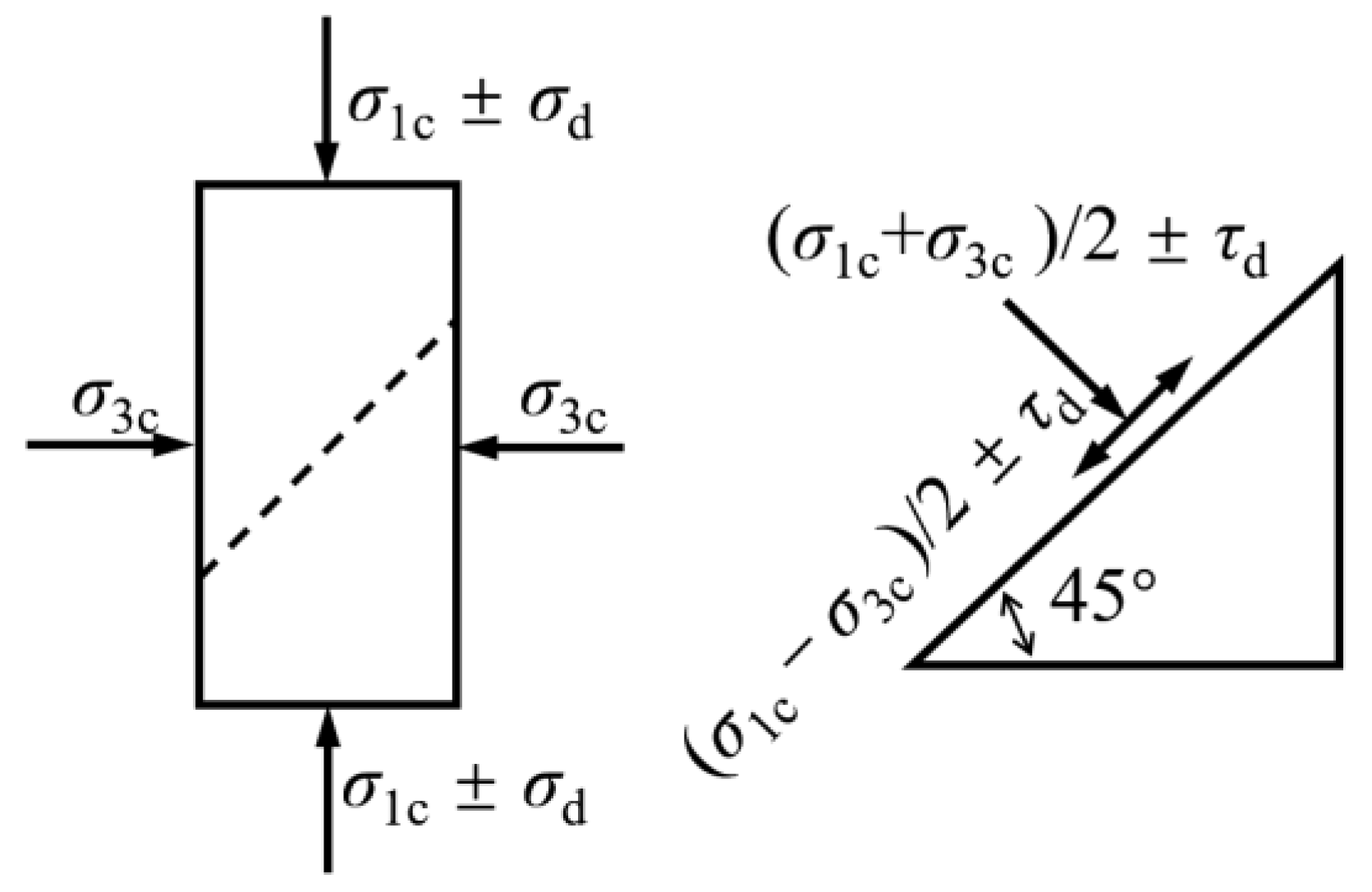
| Stress model | is the dynamic pore water pressure; is the initial confining pressure; is the number of cycles required to cause liquefaction; is the experimental constant related to the type of material. | Seed [6] | |
| Strain model | is the tangent modulus of the one-dimensional unloading curve at a point corresponding to the initial vertical effective stress; are test constants. | Martin et al. [11] | |
| Transient model | are increments of the pore water pressure during ; is the pore water pressure without dissipation at ; is the dissipation pore water pressure at . | Xie [12] | |
| Energy model | is the dissipated energy; and are test constants. | Cao et al. [13] | |
| Endochronic theory | K is the damage parameter; the variable includes the shear strain amplitude and number of cycles. A and B are test parameters. | Finn [14] | |
| Effective stress path model | Under loading conditions, additional plastic shear deformation occurs in the soil. The variation of pore water pressure is equal to the variation of the mean effective stress. | Ishihara [15] |
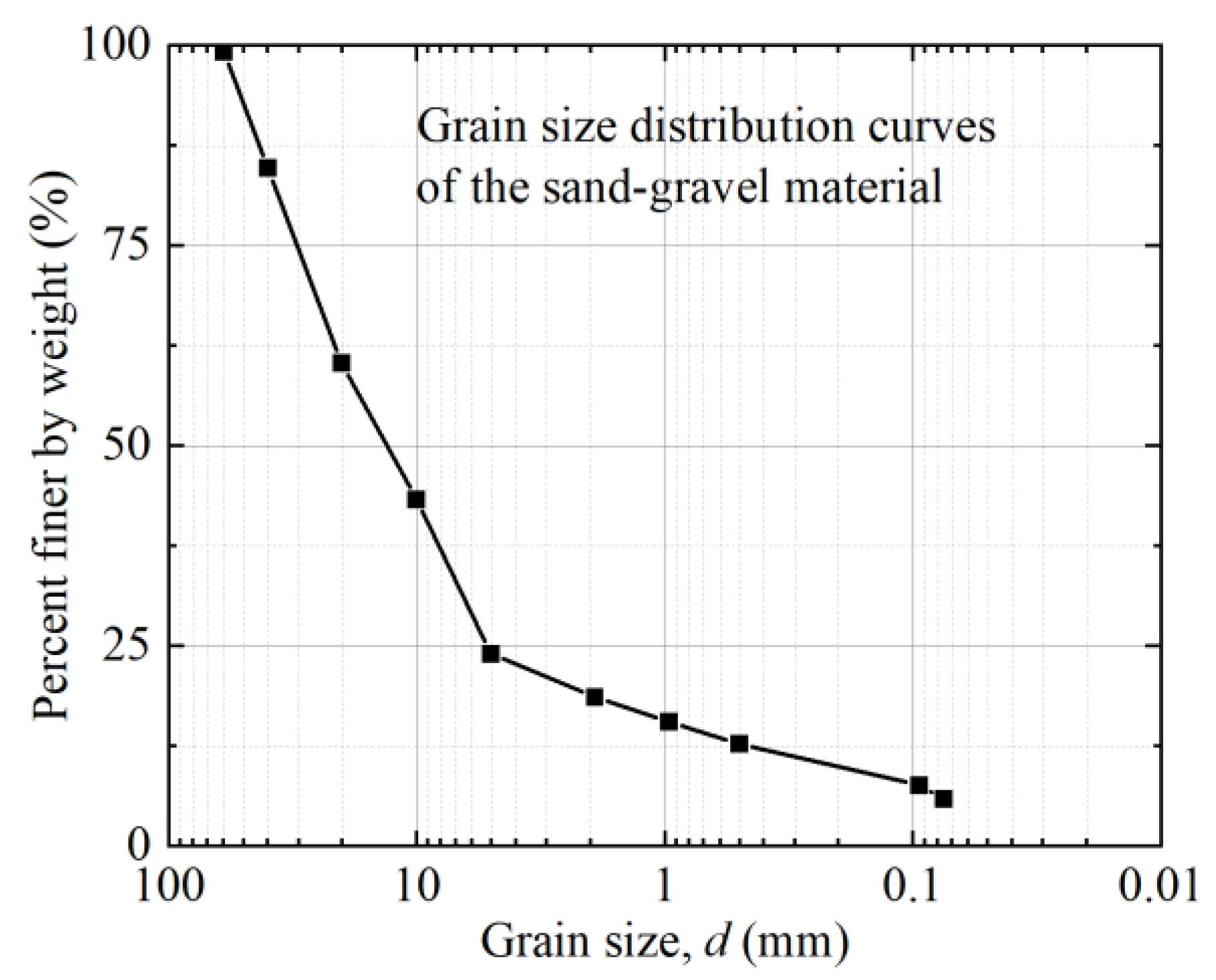
| Sand-Gravel Materials in Xinjiang | Mean Grain Size d50/mm | Specific Gravity Gs | Minimum Dry Density ρmin/g·cm−3 | Maximum Dry Density ρmax/g·cm−3 | Relative Density Dr | Dry Density ρd/g·cm−3 |
| 14 | 2.75 | 1.85 | 2.34 | 0.9 | 2.28 |
| Test Number | Kc | σ3c (kPa) | σd (kPa) | |
|---|---|---|---|---|
| S1 | 1.5 | 50 | 70.50 | 0.56 |
| S2 | 100 | 142.90 | 0.57 | |
| S3 | 121.25 | 0.49 | ||
| S4 | 200 | 291.45 | 0.58 | |
| S5 | 245.25 | 0.49 | ||
| S6 | 227.65 | 0.46 | ||
| S7 | 300 | 438.45 | 0.58 | |
| S8 | 393.15 | 0.52 | ||
| S9 | 346.30 | 0.46 | ||
| S10 | 2.0 | 50 | 95.10 | 0.63 |
| S11 | 100 | 190.95 | 0.64 | |
| S12 | 175.75 | 0.59 | ||
| S13 | 200 | 387.65 | 0.65 | |
| S14 | 342.25 | 0.57 | ||
| S15 | 296.75 | 0.49 | ||
| S16 | 300 | 582.80 | 0.65 | |
| S17 | 519.35 | 0.58 | ||
| S18 | 447.15 | 0.50 |
| Test Number | Kc | σ3c (kPa) | ud (kPa) | NL |
|---|---|---|---|---|
| S1 | 1.5 | 50 | 49.93 | 174.4 |
| S2 | 100 | 99.95 | 65.4 | |
| S3 | 100.01 | 147.5 | ||
| S4 | 200 | 197.1 | 42.35 | |
| S5 | 198.54 | 74.3 | ||
| S6 | 199.79 | 153.3 | ||
| S7 | 300 | 295.87 | 30.37 | |
| S8 | 298.07 | 43.35 | ||
| S9 | 299.94 | 84.3 | ||
| S10 | 2.0 | 50 | 49.24 | 145.3 |
| S11 | 100 | 95.3 | 44.3 | |
| S12 | 95.06 | 105.25 | ||
| S13 | 200 | 187.77 | 23.3 | |
| S14 | 193.42 | 35.25 | ||
| S15 | 195.93 | 78.25 | ||
| S16 | 300 | 280.62 | 18.25 | |
| S17 | 282.71 | 32.2 | ||
| S18 | 281.03 | 73.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, J.; Fu, Z.; Chen, S.; Shi, B. A Method to Estimate Dynamic Pore Water Pressure Growth of Saturated Sand-Gravel Materials. Appl. Sci. 2024, 14, 7909. https://doi.org/10.3390/app14177909
Chen J, Fu Z, Chen S, Shi B. A Method to Estimate Dynamic Pore Water Pressure Growth of Saturated Sand-Gravel Materials. Applied Sciences. 2024; 14(17):7909. https://doi.org/10.3390/app14177909
Chicago/Turabian StyleChen, Jinyi, Zhongzhi Fu, Shengshui Chen, and Beixiao Shi. 2024. "A Method to Estimate Dynamic Pore Water Pressure Growth of Saturated Sand-Gravel Materials" Applied Sciences 14, no. 17: 7909. https://doi.org/10.3390/app14177909
APA StyleChen, J., Fu, Z., Chen, S., & Shi, B. (2024). A Method to Estimate Dynamic Pore Water Pressure Growth of Saturated Sand-Gravel Materials. Applied Sciences, 14(17), 7909. https://doi.org/10.3390/app14177909





