Design of a Steady-State Adjustment Method and Sensitivity Analysis for an ORC System with Plate Heat Exchangers
Abstract
:1. Introduction
1.1. ORC System
1.2. Variable Heat Source Temperature of an ORC System
1.3. Evaporators in an ORC
2. Mathematical Model
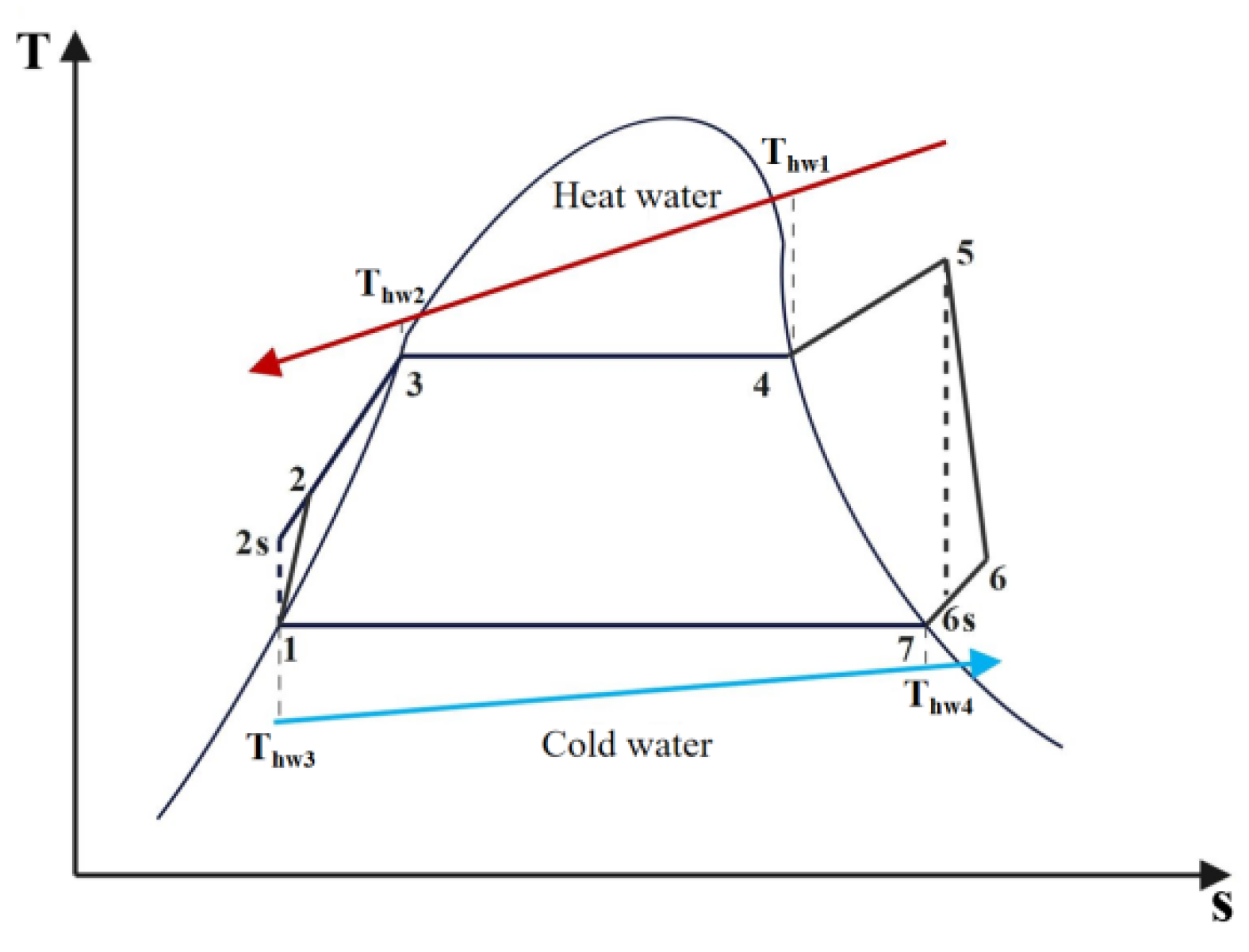
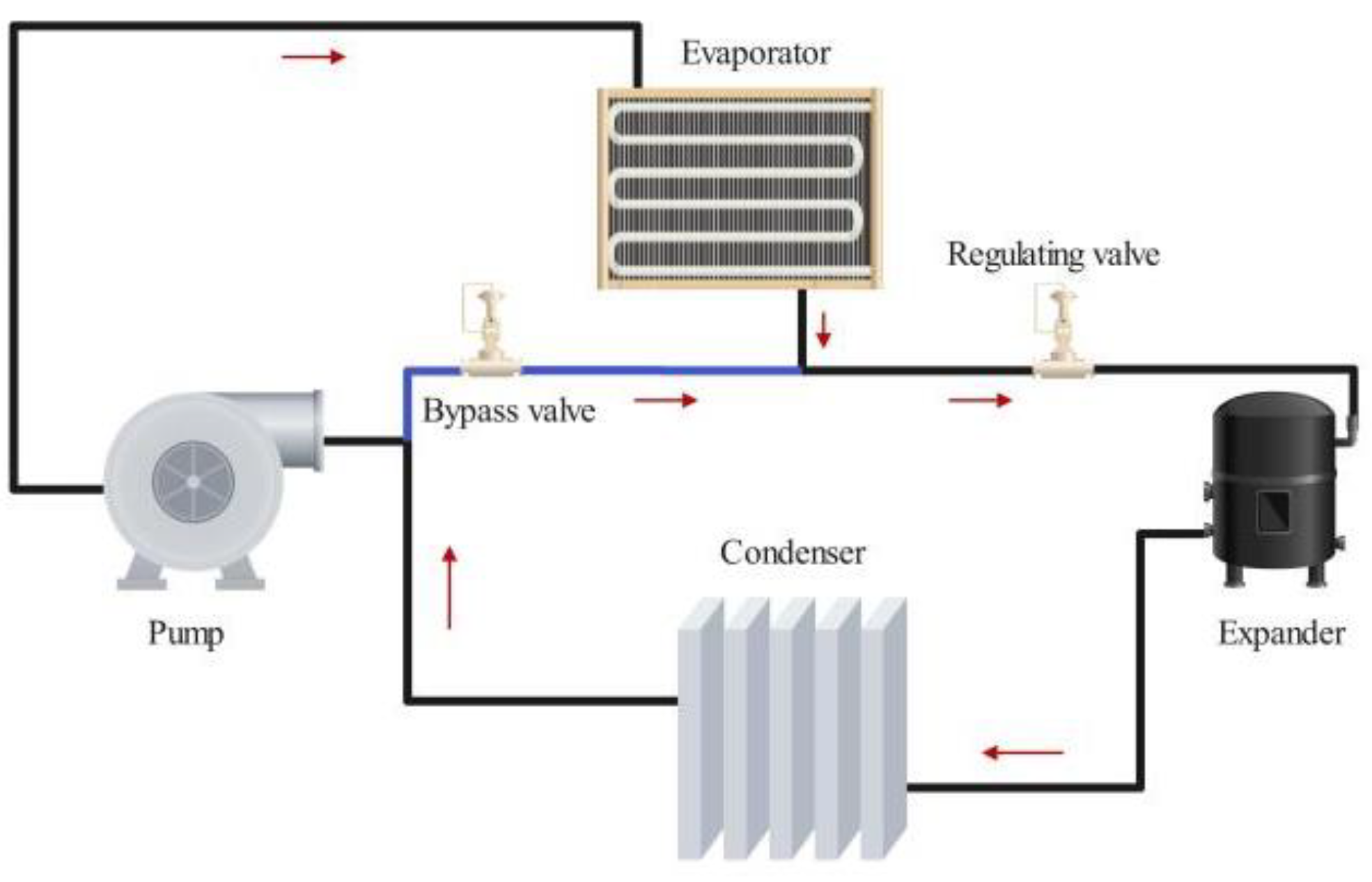
2.1. ORC System Description
2.2. Working Fluids
2.3. Operating Conditions
2.4. Model Assumptions and Construction
- (1)
- Assuming that there is equal heat exchange between the working medium side and the water side, the heat loss is not taken into account.
- (2)
- The flow pressure drop in the condenser is ignored, that is, the momentum equation is not considered.
- (3)
- The steady flow satisfies the mass equation (continuity equation), only considering the energy equation.
- (4)
- The mass flow rate at each position in the heat exchanger remains unchanged.
- (1)
- Under the initial design values in Table 2, calculate and obtain the initial value of the evaporation pressure when the expander output power is maximum, which was 827.46 kPa.
- (2)
- Calculate the pressure drop and actual heat exchange area of the heat exchanger, then select the appropriate type from the 12 brazed plate heat exchangers provided by the manufacturer [48].
- (3)
- Establish the range of changing working conditions through the self-regulation of the thermal parameters like the heat transfer coefficient and mass flow rate, etc., based on the structural and thermal parameters of the chosen heat exchanger type. The altering rules of the parameters under various working conditions were therefore obtained.
2.5. Mathematical Model
2.5.1. Heat Exchanger
- (1)
- Water volume flow
- (2)
- Number of flow channels and heat exchange area
- (3)
- Working fluid pressure drop and phase area ratio
- (4)
- Heat transfer coefficient of water
- (5)
- Convection heat transfer coefficient on the working fluid side
- (6)
- Boiling heat transfer coefficient on the working fluid side
- (7)
- Total working fluid side heat transfer coefficient
- (8)
- Total heat transfer coefficient of the evaporator
- (9)
- Logarithmic mean temperature difference
- (10)
- Actual heat exchange area
2.5.2. Expander
2.5.3. Pump
2.5.4. System
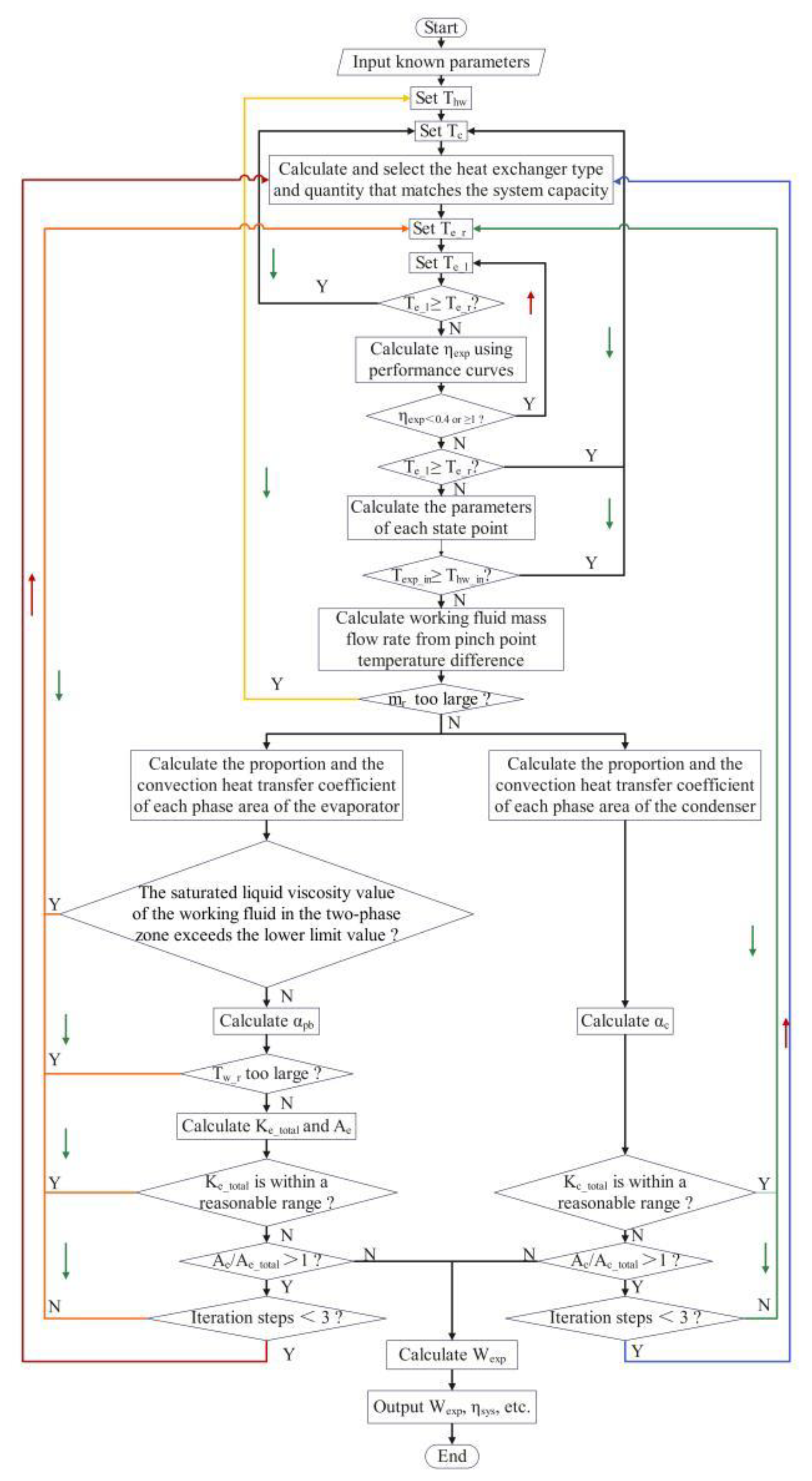
3. Algorithmic Logic Construction
3.1. System Sensitivity Analysis
- (1)
- Define the target parameters.
- (2)
- Determine the input parameters.
- (3)
- Preliminarily give the range of variable evaporation pressures:
- (1)
- Lower the limit of the interval. In order to ensure that the temperature difference or pressure difference in the system drives heat conversion, the lower limit setting value of the evaporation pressure cannot be lower than or equal to the condensation pressure value.
- (2)
- Since the mass flow rate is iteratively obtained by setting the pinch point temperature difference value, and taking into account the critical parameters of the working fluid, there are initially two judgment conditions for the upper limit value:
- (4)
- Ascertain the quantity and content of judgment conditions based on the parameters that may cause errors and require debugging under the aforementioned fundamental presumptions. Apart from modifying the variable interval, the set input parameter values are subject to the following constraints:
- (5)
- Establish calculating models for each component.
- (6)
- Determine the output parameters and curves of the system.
- (7)
- Perform program debugging.
3.2. System Steady-State Adjustment
4. Results and Discussion
4.1. Variable Working Condition Parameters and Interval Ranges
4.2. Variable Evaporation Pressure
4.3. Variable Condensation Temperature
4.4. Steady-State Regulation
5. Conclusions
- A system model for the application scenario of a 100–200 kW ORC system with BPHEs was designed.
- A sensitivity analysis of the system was carried out based on the performance changes in the heat exchangers.
- To make sure the system could continue to produce a steady power output even when the heat source’s temperature fluctuated, an adjustment strategy and modeling computation were proposed.
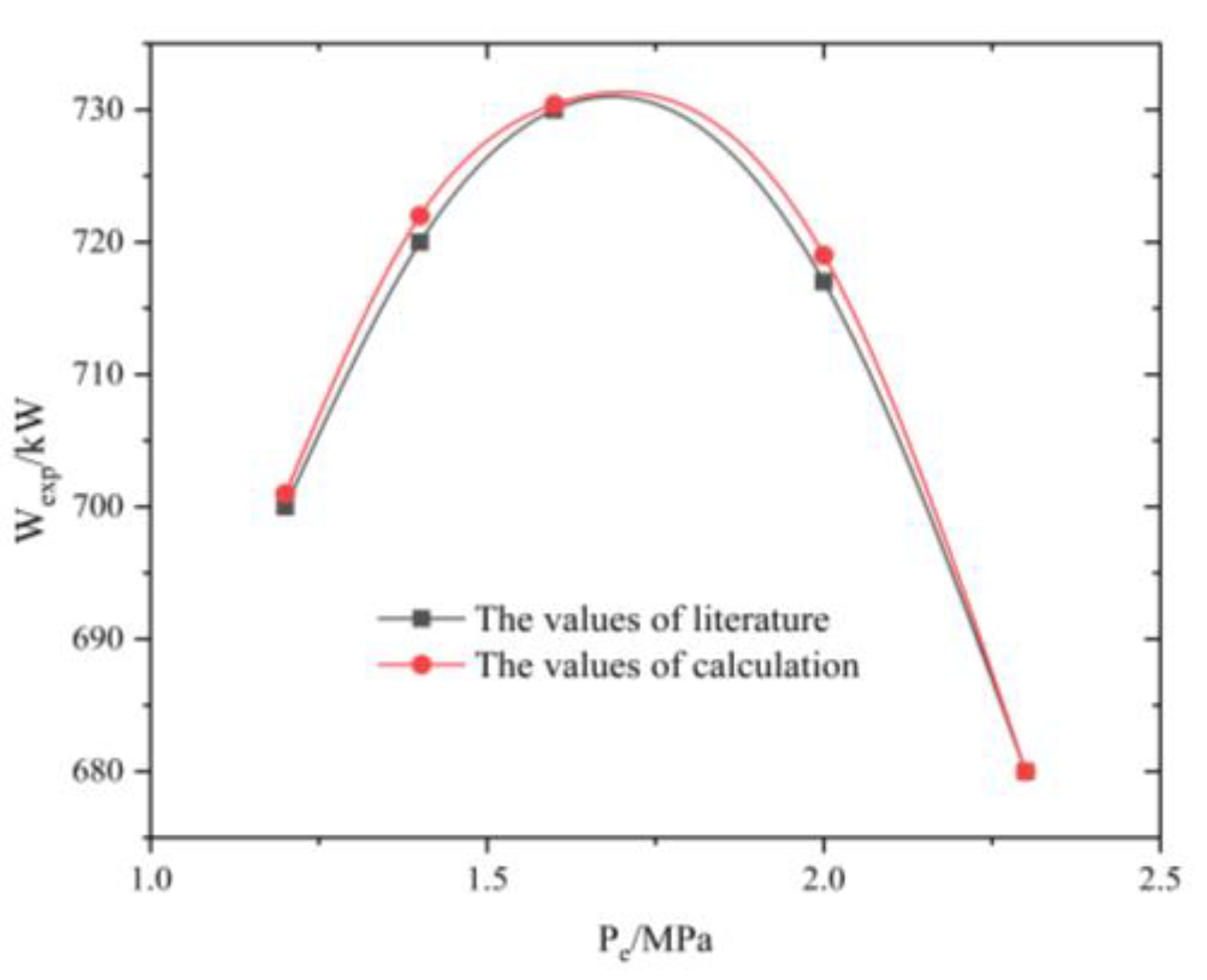
- The model results were compared and verified with the results of the R245fa-ORC experimental test papers, which provided a foundation for the design and practical application of the ORC system.
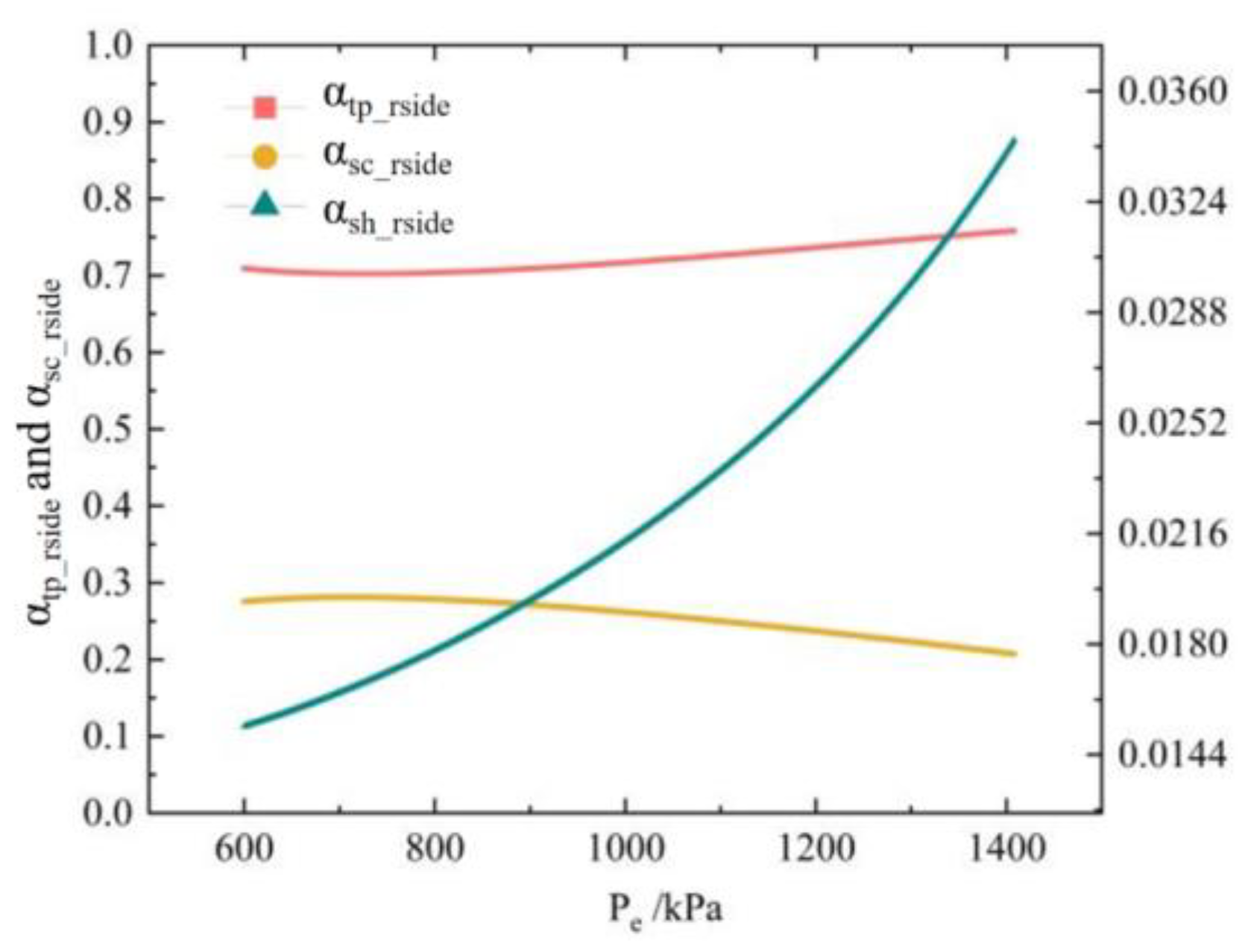
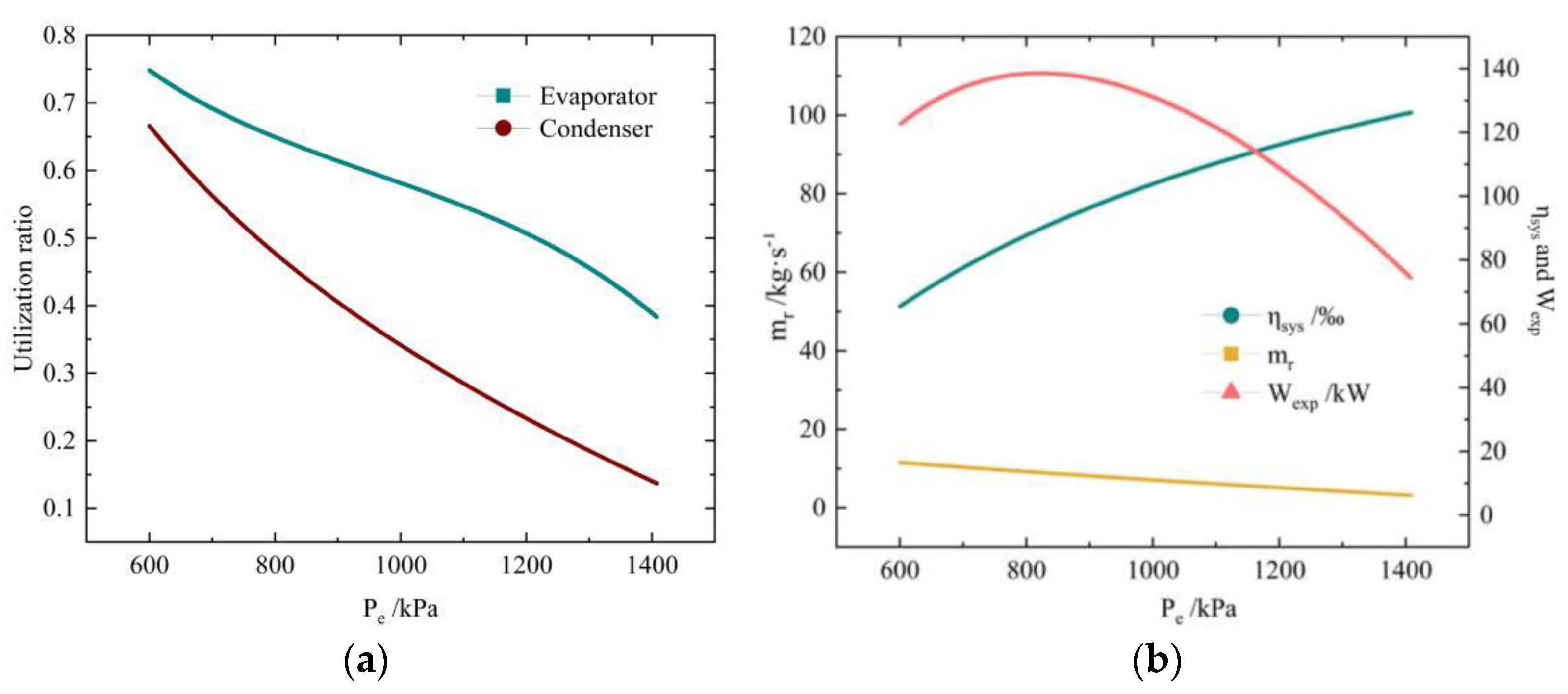
- When the heat source temperature was 125 °C and the condenser temperature was 45 °C, as the evaporation pressure increased from 600 to 1410 kPa, the two-phase zone in the evaporator accounted for the largest proportion, increasing from 70.22% to 75.83%. The supercooled zone followed, decreasing from 27.56% to 20.72%. The superheated zone, which accounted for the smallest proportion, increased from 1.53% to 3.44%. The decrease in the mass flow rate and heat flux made the heat transfer coefficient decrease from 46.32 kW/m2 to 14.04 kW/m2. The evaporator utilization ratio decreased from 74.85% to 38.32% and the condenser utilization ratio from 66.61% to 13.69%. The output power had a maximum value of 153.11 kW. When the output power reached its maximum, the system efficiency was 7.78% and the mass flow rate was 9.13 kg/s. The system efficiency increased from 5.74 to 11.04%.
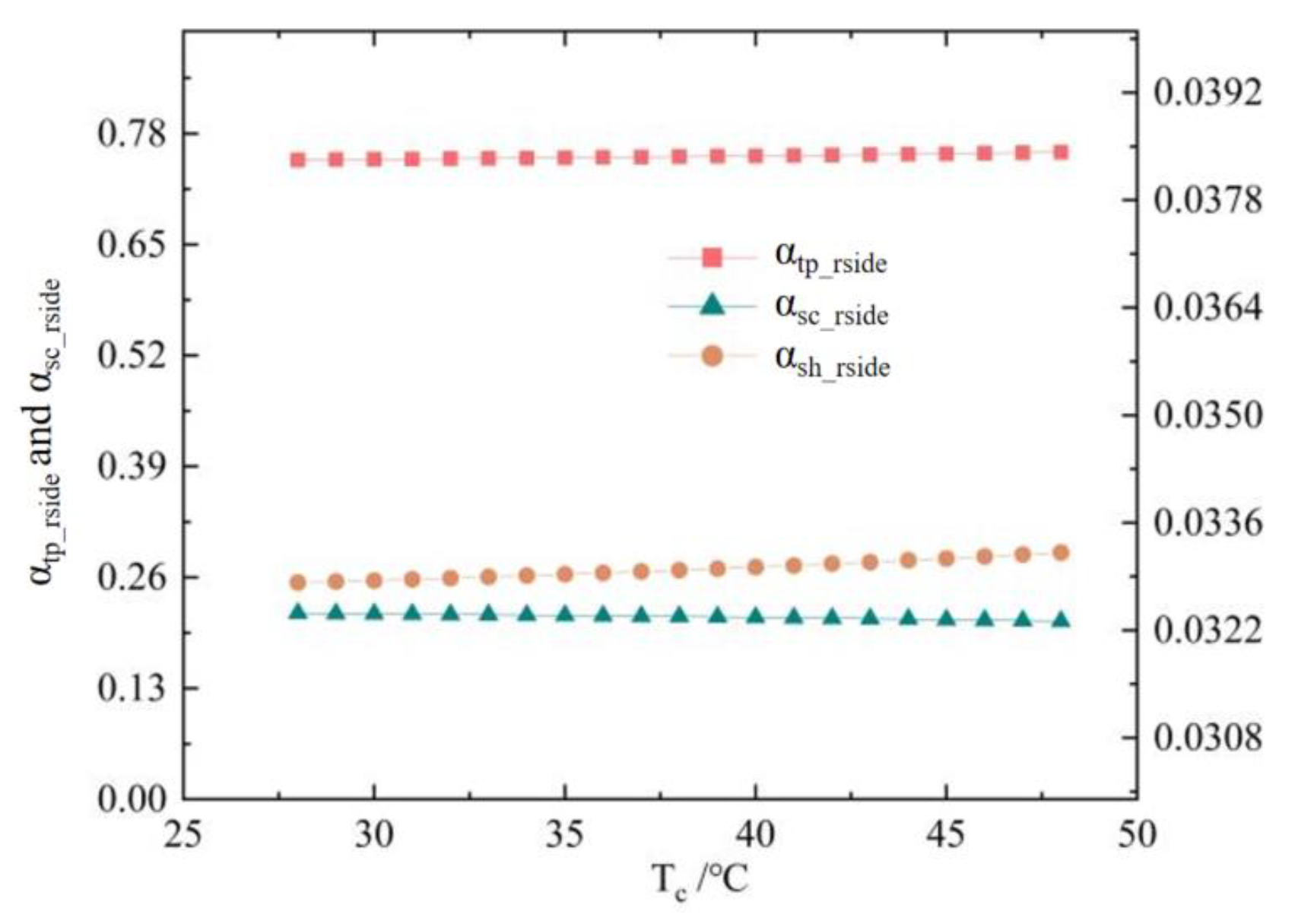
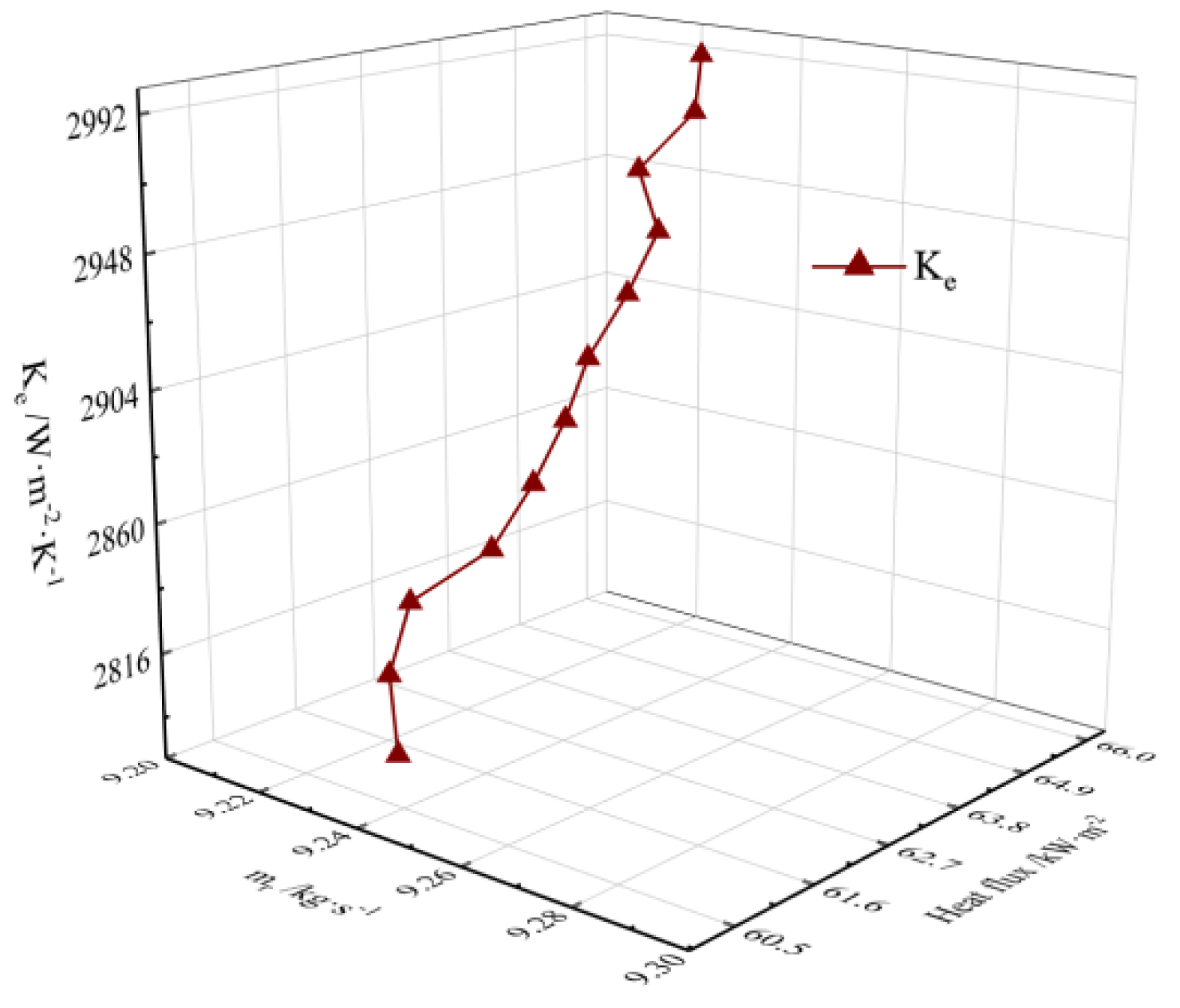
- When the heat source temperature was 125 °C and evaporation temperature was 104 °C, the increase in the condensation temperature from 28 to 48 °C made the mass flow rate have a tiny increase of 0.02% and an average value of 3.36 kg/s. The two-phase zone and superheated zone slightly increased from 74.91% to 75.81% and from 3.28% to 3.32%, respectively. The ratio of the supercooled zone decreased from 21.80% to 20.87%. The heat flux increased from 52.83 kW/m2 to 63.94 kW/m2, while the heat transfer coefficient increased from 1721.31 W/(m2·K) to 2374.77 W/(m2·K). The evaporator utilization ratio dropped from 70.56% to 51.91%, and the condenser utilization ratio dropped from 95.94% to 45.71%. The system efficiency dropped from 13.64% to 10.43%, and the expander output power dropped from 116.95 kW to 80.60 kW.
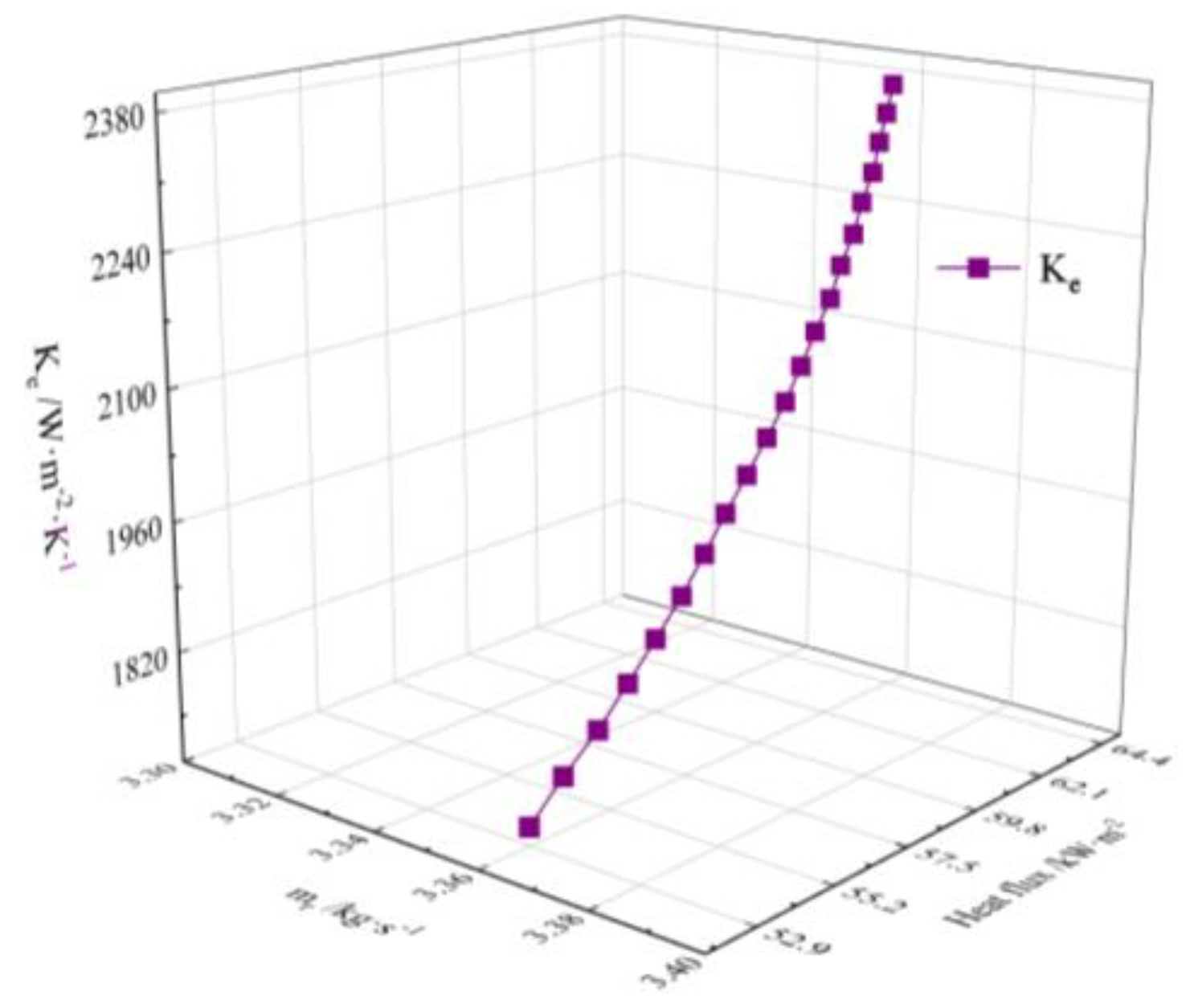
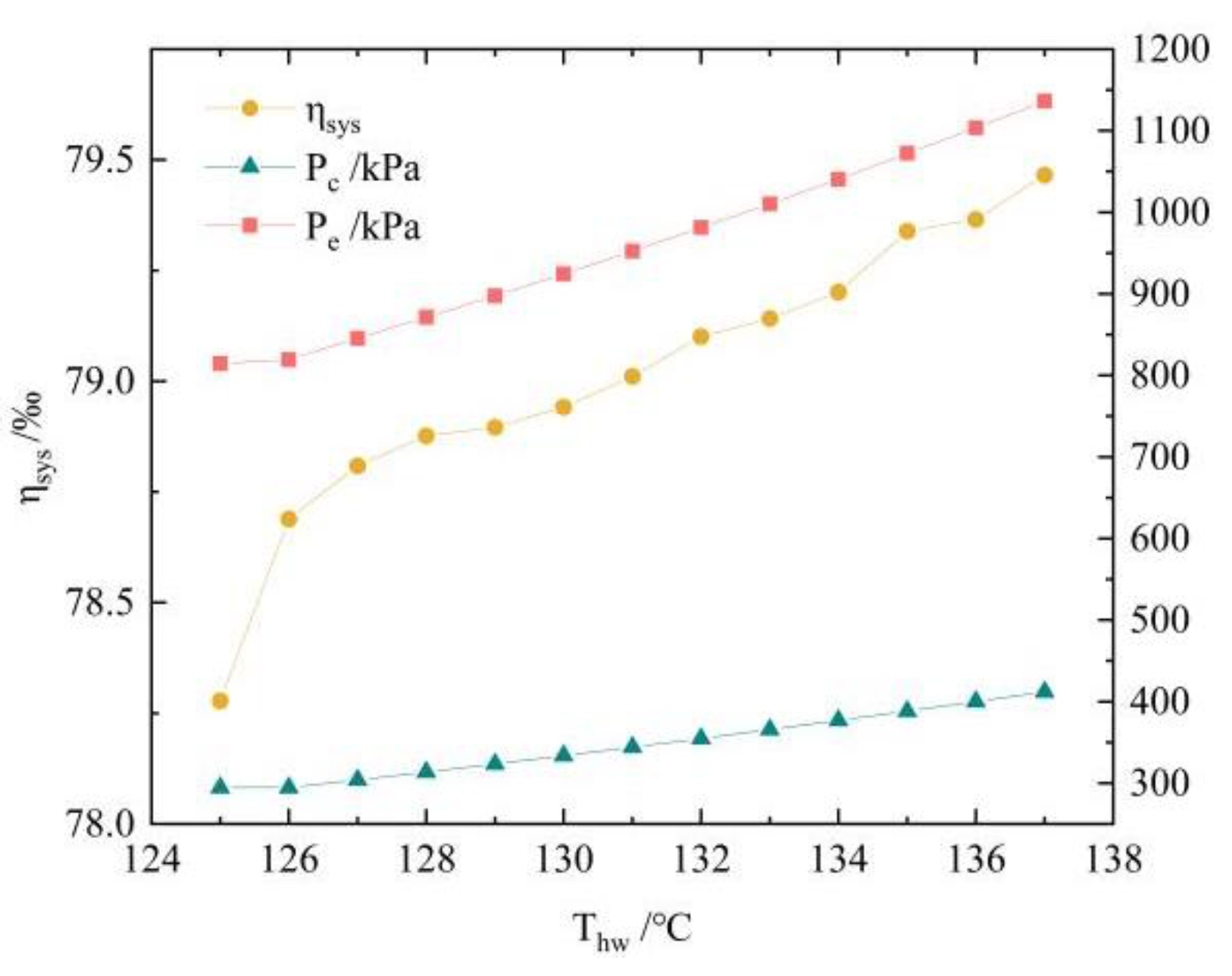
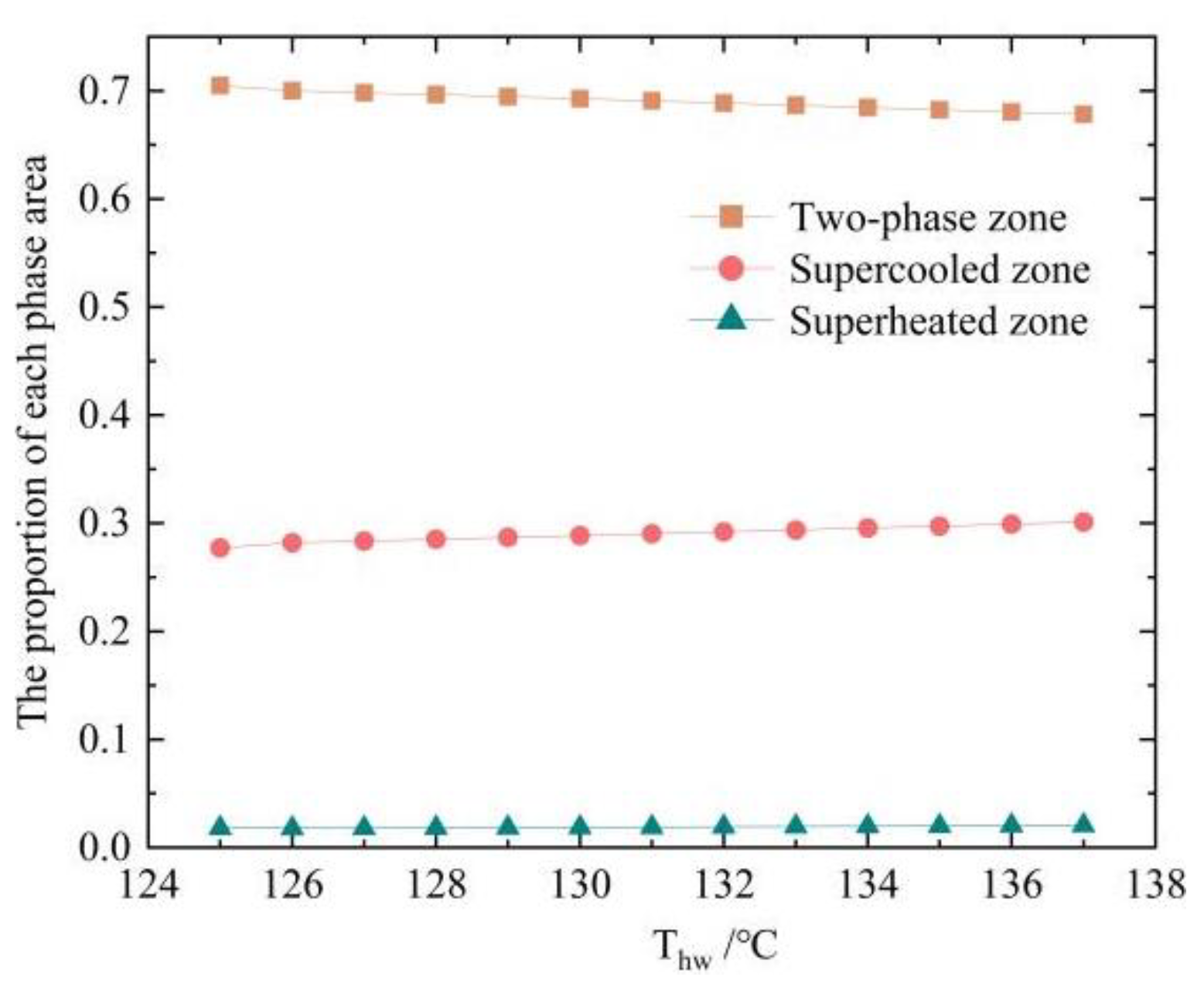
- When the heat source temperature increased, fluctuations in power generation were produced. The was in order to balance out these fluctuations and simulate the law that the mass flow rate was controlled by the working fluid pump and remained basically unchanged during actual stable operation. The evaporation pressure increased from 814.46 kPa to 1136.46 kPa, while the condensation temperature increased from 294.46 kPa to 412.01 kPa. System efficiency increased from 7.83% to 7.95%. At this time, in the BPE, the two-phase zone proportion decreased from 69.65% to 67.82%, the supercooled zone proportion increased from 28.70% to 30.12%, and the superheated zone proportion increased from 1.86% to 2.06%. The heat transfer coefficient increased from 2776.07 W/(m2·K) to 2992.74 W/(m2·K) as the heat flux increased.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature and Abbreviations
| A | area | μ | dynamic viscosity |
| C | constant | λ | flow resistance coefficient |
| c | ratio | η | efficiency |
| D | rotor diameter | α | heat transfer coefficient |
| d | equivalent diameter | Φ | liquid-phase friction coefficient |
| F | correction factor | Subscripts | |
| f | friction coefficient | e | evaporator |
| G | condensation volume | exp | expander |
| H | pump head | g | gas |
| h | specific enthalpy | hw | hot water |
| K | heat transfer coefficient | in | inlet |
| L | phase length | l | liquid |
| l | specific heat | le | liquid in evaporator |
| m | mass flow rate | log | logarithm |
| n | number of flow channels or rotation speed | m | mean |
| P | pressure | max | maximum |
| Pr | Prandtl number | min | minimum |
| Q/q | heat exchange amount | out | outlet |
| Re | Reynolds number | pb | pool boiling |
| R | thermal resistance | pump | pump |
| s | single-channel cross-sectional area | r | refrigerant |
| T/t | temperature | sc | supercooled |
| u | flow rate | sh | superheated |
| v | specific volume | tp | two-phase |
| W | power | w | wall |
| X | Martinelli parameters | Acronyms | |
| x | dryness | BPHE | brazed plate heat exchanger |
| Greek letters | BPE | brazed plate evaporator | |
| ρ | density | BFPE | brazed nickel foam plate evaporator |
| υ | kinematic viscosity | PHE | plate heat exchanger |
| ψ | liquid-phase friction coefficient | ORC | organic Rankine cycle |
| δ | plate thickness | ORC-R | ORC with regulated heat source |
References
- Thurairaja, K.; Wijewardane, A.; Jayasekara, S.; Ranasinghe, C. Working Fluid Selection and Performance Evaluation of ORC. Energy Procedia 2017, 156, 244–248. [Google Scholar] [CrossRef]
- Mao, L.F.; Peng, H.; Zhao, J.W.; Hu, B.S. Generator Selection and Design of Grid-connected for ORC Power System. Energy Conserv. Technol. 2017, 35, 71–74. [Google Scholar]
- Tang, H. Research on Twin-Screw Expander Characteristics and Organic Rankine Cycle System Performance. Ph.D. Thesis, Xi’an Jiaotong University, Xi’an, China, 2016. [Google Scholar]
- Xing, C.D.; Peng, X.; Guo, R.L.; Zhang, H.G.; Yang, F.B.; Yu, M.Z.; Yang, A.R.; Wang, Y. Machine learning-based multi-objective optimization and thermodynamic evaluation of organic Rankine cycle (ORC) system for vehicle engine under road condition. Appl. Therm. Eng. 2023, 231, 120904. [Google Scholar] [CrossRef]
- Li, T.L.; Wang, Q.L.; Zhu, J.L.; Hu, J.L.; Fu, W.C. Thermodynamic optimization of organic Rankine cycle using two-stage evaporation. Renew. Energy 2015, 75, 654–664. [Google Scholar] [CrossRef]
- Mago, P.J.; Srinivasan, K.K.; Chamra, L.M.; Somayaji, C. An examination of exergy destruction in organic Rankine cycles. Int. J. Energ. Res. 2008, 32, 26–38. [Google Scholar] [CrossRef]
- Tchanche, B.F.; Lambrinos, G.; Frangoudakis, A.; Papadakis, G. Exergy analysis of micro-organic Rankine power cycles for a small scale solar driven reverse osmosis desalination system. Appl. Energy 2010, 87, 306–1295. [Google Scholar] [CrossRef]
- Yamamoto, T.; Furuhata, T.; Arai, N.; Mori, K. Design and testing of the Organic Rankine Cycle. Energy 2001, 26, 239–251. [Google Scholar] [CrossRef]
- Hou, S.Y.; Zhou, Y.D.; Yu, L.J.; Zhang, F.Y.; Cao, S.; Wu, Y.D. Optimization of a novel cogeneration system including a gas turbine, a supercritical CO2 recompression cycle, a steam power cycle and an organic Rankine cycle. Energy Convers. Manag. 2018, 172, 457–471. [Google Scholar] [CrossRef]
- Wang, W.; Deng, S.; Zhao, D.P.; Zhao, L.; Lin, S.; Chen, M.C. Application of machine learning into organic Rankine cycle for prediction and optimization of thermal and exergy efficiency. Energy Convers. Manag. 2020, 210, 112700. [Google Scholar] [CrossRef]
- Wei, D.; Lu, X.; Lu, Z.; Gu, J. Dynamic modeling and simulation of an organic Rankine cycle (ORC) system for waste heat recovery. Appl. Therm. Energy 2008, 28, 1216–1224. [Google Scholar] [CrossRef]
- Feng, X. Theoretical Research on Low-Temperature Waste Heat Power Generation System in Organic Rankine Cycle. Master’s Thesis, Shanghai Jiaotong University, Shanghai, China, 2011. [Google Scholar]
- Zhar, R.; Allouhi, A.; Jamil, A.; Lahrech, K. A comparative study and sensitivity analysis of different ORC configurations for waste heat recovery. Case Stud. Therm. Eng. 2021, 28, 101608. [Google Scholar] [CrossRef]
- Fan, G.L.; Gao, Y.J.; Ayed, H.; Marzouki, R.; Aryanfar, Y.; Jarad, F.; Guo, P.X. Energy and exergy and economic (3E) analysis of a two-stage organic Rankine cycle for single flash geothermal power plant exhaust exergy recovery. Case Stud. Therm. Eng. 2021, 28, 101554. [Google Scholar] [CrossRef]
- Wang, J.L.; Zhao, L.; Wang, X.D. A comparative study of pure and zeotropic mixtures in low-temperature solar Rankine cycle. Appl. Therm. Energy 2010, 87, 66–73. [Google Scholar] [CrossRef]
- Hung, T.C.; Shai, T.Y.; Wang, S.K. A review of organic Rankine cycles (ORCs) for the recovery of low-grade waste heat. Energy 1997, 22, 7–661. [Google Scholar] [CrossRef]
- Pedro, J.M.; Louay, M.C.; Kalyan, S.; Chandramohan, S. An examination of regenerative organic Rankine cycles using dry fluids. Appl. Therm. Energy 2008, 28, 998–1007. [Google Scholar]
- Chacartegui, R.; Sanchez, D.; Munoz, J.M.; Sanchez, T. Alternative ORC bottoming cycles for combined cycle power plants. Appl. Energy 2009, 86, 662–670. [Google Scholar] [CrossRef]
- Rayegan, R.; Tao, Y.X. A procedure to select working fluids for solar Organic Rankine Cycles (ORCs). Renew. Energy 2011, 36, 659–670. [Google Scholar] [CrossRef]
- Yu, W.; Ban, X.; Liu, C.; Li, Q.B.; Xin, L.Y.; Huang, Z.Y.; Wang, S.K. Experimental and Theoretical Study on the Thermal Stability and Pyrolysis Mechanism of Octamethyltrisiloxane (MDM) with Lubricating Oil. J. Anal. Appl. Pyrolysis 2024, 179, 106521. [Google Scholar] [CrossRef]
- Liu, Y.; Jing, Y.; Liu, C. Heat Capacity of Liquid Octamethyltrisiloxane (MDM) and Hexamethyldisiloxane (MM): Measurement and Molecular Dynamics Study. J. Mol. Liq. 2024, 404, 124937. [Google Scholar] [CrossRef]
- Yu, W.; Liu, C.; Tan, L.; Li, Q.B.; Xin, L.Y.; Wang, S.K. Thermal Stability and Thermal Decomposition Mechanism of Octamethyltrisiloxane (MDM): Combined Experiment, ReaxFF-MD and DFT Study. Energy 2023, 284, 129289. [Google Scholar] [CrossRef]
- Yang, L.; Gong, M.; Guo, H.; Dong, X.Q.; Shen, J.; Wu, J.F. Effects of Critical and Boiling Temperatures on System Performance and Fluid Selection Indicator for Low Temperature Organic Rankine Cycles. Energy 2016, 109, 830–844. [Google Scholar] [CrossRef]
- Zhai, H.; An, Q.; Shi, L. Analysis of the Quantitative Correlation Between the Heat Source Temperature and the Critical Temperature of the Optimal Pure Working Fluid for Subcritical Organic Rankine Cycles. Appl. Therm. Eng. 2016, 99, 383–391. [Google Scholar] [CrossRef]
- Ye, Z.; Yang, J.; Shi, J.; Chen, J. Thermo-economic and Environmental Analysis of Various Low-GWP Refrigerants in Organic Rankine Cycle System. Energy 2020, 199, 117344. [Google Scholar] [CrossRef]
- Özcan, Z.; Ekici, Ö. A Novel Working Fluid Selection and Waste Heat Recovery by An Exergoeconomic Approach for a Geothermally Sourced ORC System. Geothermics 2021, 95, 102151. [Google Scholar] [CrossRef]
- Sylvain, Q.L.; Lemort, V.; Lebrun, J. Experimental study and modeling of an Organic Rankine Cycle using scroll expander. Appl. Energy 2010, 87, 1260–1268. [Google Scholar]
- Kane, H.E. Intégration et Optimisation Thermoéconomique and Environomique de Centrales Thermiques Solaires Hybrides. Ph.D. Thesis, Laboratoire d’ Energétique Industrielle, Ecole Polytechnique Fédérale de Lausanne, Lausanne, Switzerland, 2002. [Google Scholar]
- Qi, F.L. Experimental Research and Simulation of Organic Rankine Cycle; North China Electric Power University: Beijing, China, 2017. [Google Scholar]
- Zhang, Y.; Wu, Y.; Xia, G.; Ma, C.; Ji, W.; Liu, S.; Yang, K.; Yang, F. Development and experimental study on organic Rankine cycle system with single-screw expander for waste heat recovery from exhaust of diesel engine. Energy 2014, 77, 499–508. [Google Scholar] [CrossRef]
- Ni, J.X. Analysis to the Dynamic Performance with Variable Conditions and Simulation to Organic Rankine Cycle. Master’s Thesis, Tianjin University, Tianjin, China, 2017. [Google Scholar]
- Tchanche, B.F.; Lambrinos, G.; Frangoudakis, A.; Papadakis, G. Low-grade heat conversion into power using organic Rankine cycles-A review of various applications. Renew. Sustain. Energy Rev. 2011, 15, 3963–3979. [Google Scholar] [CrossRef]
- Manente, G.; Toffolo, A.; Lazzaretto, A.; Paci, M. An Organic Rankine Cycle off-design model for the search of the optimal control strategy. Energy 2013, 58, 97–106. [Google Scholar] [CrossRef]
- Calise, F.; Capuozzo, C.; Carotenuto, A.; Vanoli, L. Thermoeconomic analysis and off-design performance of an organic Rankine cycle powered by medium-temperature heat sources. Sol. Energy 2014, 103, 595–609. [Google Scholar] [CrossRef]
- Calise, F.; D’Accadia, M.D.; Macaluso, A.; Piacentino, A.; Vanoli, L. Exergetic and exergoeconomic analysis of a novel hybrid solar-geothermal polygeneration system producing energy and water. Energy Convers. Manag. 2016, 115, 200–220. [Google Scholar] [CrossRef]
- Shi, R.; He, T.; Peng, J.; Zhang, Y.; Zhuge, W. System design and control for waste heat recovery of automotive engines based on Organic Rankine Cycle. Energy 2016, 102, 276–286. [Google Scholar] [CrossRef]
- Yang, X.L.; Huang, S.R.F.F.; Dai, W.Z. Analysis of thermal performance of heat source-regulated organic Rankine cycle power generation system. J. Eng. Therm. Energy Power 2017, 32, 34–40. [Google Scholar]
- Bull, J.; Buick, J.M.; Radulovic, J. Heat exchanger sizing for organic Rankine cycle. Energies 2020, 13, 3615. [Google Scholar] [CrossRef]
- Xu, J.; Luo, X.; Chen, Y.; Mo, S. Multi-criteria design optimization and screening of heat exchangers for a subcritical ORC. Energy Procedia 2015, 75, 1639–1645. [Google Scholar] [CrossRef]
- Walraven, D.; Laenen, B.; D’haeseleer, W. Comparison of shell-and-tube with plate heat exchangers for the use in low-temperature organic Rankine cycles. Energy Convers. Manag. 2014, 87, 227–237. [Google Scholar] [CrossRef]
- Chatzopoulou, M.A.; Lecompte, S.; Markides, N.C. Off-design optimisation of organic Rankine cycle (ORC) engines with different heat exchangers and volumetric expanders in waste heat recovery applications. Appl. Energy 2019, 253, 113442. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, C.; Wang, S.K.; Xu, X.X.; Li, Q.B. Thermo-economic comparison of subcritical organic Rankine cycle based on different heat exchanger configurations. Energy 2017, 123, 728–741. [Google Scholar] [CrossRef]
- Hu, K.Y.; Zhu, J.L.; Li, T.L.; Zhang, W. R245fa Evaporation Heat Transfer and Pressure Drop in a Brazed Plate Heat Exchanger for Organic Rankine Cycle (ORC). In Proceedings of the World Geothermal Congress, Melbourne, Australia, 16–24 April 2015. [Google Scholar]
- Longo, G.A.; Gasparella, A. Refrigerant R134a vaporisation heat transfer and pressure drop inside a small brazed plate heat exchanger. Int. J. Refrig. 2007, 30, 821–830. [Google Scholar] [CrossRef]
- Tari, Z.G. Flow boiling heat transfer characteristics of R245fa refrigerant in a plate heat exchanger. J. Phys. Conf. Ser. 2020, 1599, 012007. [Google Scholar] [CrossRef]
- Kim, D.Y.; Kim, K.C. Thermal performance of brazed metalfoam-plate heat exchanger as an evaporator for organic Rankine cycle. Energy Procedia 2017, 129, 451–458. [Google Scholar] [CrossRef]
- Muhammad, I.; Muhammad, U.; Yang, Y.; Park, B.S. Flow boiling of R245fa in the brazed plate heat exchanger: Thermal and hydraulic performance assessment. Int. J. Heat Mass Transf. 2017, 110, 657–670. [Google Scholar]
- Cheng, B.H.; Li, X.R.; Yao, R.Y. Plate Heat Exchanger and Heat Exchange Device Technical Application Manual; China Architecture & Building Press: Beijing, China, 2005. [Google Scholar]
- Zhang, H.G.; Yang, Y.X.; Meng, F.X.; Zhao, R.; Tian, Y.M.; Liu, Y. Running performance of working fluid pump for organic Rankine cycle system. CIESC J. 2017, 68, 3573–3579. [Google Scholar]
- Pei, G.; Wang, D.Y.; Li, J.; Li, Y.Z.; Ji, J. Experimental study on organic Rankine cycle combined heat and power system. CIESC J. 2013, 64, 1994–2000. [Google Scholar]
- Hu, B.; Ma, W.B. Thermodynamic analysis of low-temperature geothermal refrigeration system based on organic Rankine cycle. Adv. New Renew. Energy 2014, 2, 122–128. [Google Scholar]
- Shao, Y.J.; Jin, B.S.; Zhong, W.Q.; Hao, L. Modeling and performance analysis of micro organic Rankine cycle thermoelectric system. J. Southeast Univ. 2013, 43, 798–802. [Google Scholar]
- Muhammad, I.; Muhammad, U.M.; Sik, P.B.; Yang, Y.M. Comparative assessment of Organic Rankine Cycle integration for low temperature geothermal heat source applications. Energy 2016, 102, 473–490. [Google Scholar]
- Li, Y.R.; Wang, X.Q.; Li, C.C.; Wu, S.Y.; Liu, C. Performance analysis of a coupled transcritical and subcritical organic Rankine cycle. J. Eng. Thermophys. 2015, 36, 176–1181. [Google Scholar]
- Roy, J.P.; Mishra, M.K.; Misra, A. Performance analysis of an organic Rankine cycle with superheating under different heat source temperature conditions. Appl. Energy 2011, 88, 2995–3004. [Google Scholar] [CrossRef]
- Wei, D.H.; Lu, X.S.; Lu, Z.; Gu, J.M. Performance analysis and optimization of organic Rankine cycle (ORC) for waste heat recovery. Energy Convers. Manag. 2007, 48, 1113–1119. [Google Scholar] [CrossRef]
- Weng, Y.W. Low Grade Heat Energy Conversion Processes and Their Utilizations; Shanghai Jiaotong University Press: Shanghai, China, 2014. [Google Scholar]
- Twomey, B.; Jacobs, P.A.; Gurgenci, H. Dynamic performance estimation of small-scale solar cogeneration with an organic Rankine cycle using a scroll expander. Appl. Therm. Eng. 2013, 51, 1307–1316. [Google Scholar] [CrossRef]
- Zhang, J.; Zhou, Y.; Wang, R.; Xu, J.L.; Fang, F. Modeling and constrained multivariable predictive control for ORC (Organic Rankine Cycle) based waste heat energy conversion systems. Energy 2014, 66, 128–138. [Google Scholar] [CrossRef]
- Lin, Q.Y.; Wang, D.; Zhao, Y.Y. Impact analysis of key design parameters on ORC system performance. Turbine Technol. 2023, 65, 352–370. [Google Scholar]




| Name | M | Tcrit/°C | Pcrit/MPa | ODP-GWP | Tbp/°C | Ttp/°C | Safety a |
|---|---|---|---|---|---|---|---|
| R245fa | 134 | 154.01 | 3.651 | 0-790 | 15.14 | −102.1 | B1 |
| R114 | 171 | 145.68 | 3.257 | 0.85-4 | 3.59 | −92.5 | A1 |
| R123 | 153 | 183.68 | 3.662 | 0.02-77 | 27.82 | −107.15 | B1 |
| R601a | 72 | 187.20 | 3.378 | 0-20 | 27.83 | −160.5 | A3 |
| R601 | 72 | 196.55 | 3.370 | 0-20 | 36.06 | −129.68 | A3 |
| R141b | 117 | 204.35 | 4.212 | 0.086-0.15 | 32.05 | −103.47 | A1 |
| R113 | 187 | 214.06 | 3.392 | 0.9-1.55 | 47.59 | −36.22 | A1 |
| Parameter | Value | Unit |
|---|---|---|
| vcw | 0.2 | m/s |
| Tcw | 20 | °C |
| Pcw | 1000 | kPa |
| Phw | 1000 | kPa |
| mhw | 10 | kg/s |
| Tair | 25 | °C |
| Tsh | 5 | K |
| Tsc | 0 | K |
| ΔTp | 10 | K |
| nexp | 1500 | r/min |
| Dexp | 0.3556 | m |
| ηpump | 0.7 | – |
| Thw_initial | 125 | °C |
| Tc_initial | 45 | °C |
| Parameter | Value | Unit |
|---|---|---|
| Single board channel volume | 0.86 | L |
| Maximum water flow rate | 203 | m3/h |
| Plate thickness | 0.0004 | m |
| Board spacing | 0.0023 | m |
| Effective heat exchange area of a single plate | 0.45 | m2 |
| Single channel cross-sectional area | 0.0014 | m2 |
| Process length | 0.63 | m |
| Plate width | 0.42 | m |
| Number of installed boards in the evaporator | 41 | – |
| Number of installed boards in the condenser | 80 | – |
| (a) | ||
| Parameter | Value | Unit |
| Thw_in | 125 | °C |
| Tc | 45 | °C |
| Interval ranges of Pe | 600–1410 | kPa |
| (b) | ||
| Parameter | Value | Unit |
| Thw_in | 125 | °C |
| Te | 104 | °C |
| Interval ranges of Tc | 28–48 | °C |
| (c) | ||
| Parameter | Value | Unit |
| Wexp | 153.11 | kW |
| mr | 9.09 | kg/s |
| Interval ranges of Thw_in | 125–137 | °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, L.; Wang, X.; He, Z.; Xing, Z. Design of a Steady-State Adjustment Method and Sensitivity Analysis for an ORC System with Plate Heat Exchangers. Appl. Sci. 2024, 14, 8728. https://doi.org/10.3390/app14198728
Ji L, Wang X, He Z, Xing Z. Design of a Steady-State Adjustment Method and Sensitivity Analysis for an ORC System with Plate Heat Exchangers. Applied Sciences. 2024; 14(19):8728. https://doi.org/10.3390/app14198728
Chicago/Turabian StyleJi, Lantian, Xiao Wang, Zhilong He, and Ziwen Xing. 2024. "Design of a Steady-State Adjustment Method and Sensitivity Analysis for an ORC System with Plate Heat Exchangers" Applied Sciences 14, no. 19: 8728. https://doi.org/10.3390/app14198728
APA StyleJi, L., Wang, X., He, Z., & Xing, Z. (2024). Design of a Steady-State Adjustment Method and Sensitivity Analysis for an ORC System with Plate Heat Exchangers. Applied Sciences, 14(19), 8728. https://doi.org/10.3390/app14198728







