The One-Dimensional Flow Pressure Loss Correction Model Based on the Particle Flow through Concrete Bend
Abstract
:1. Introduction
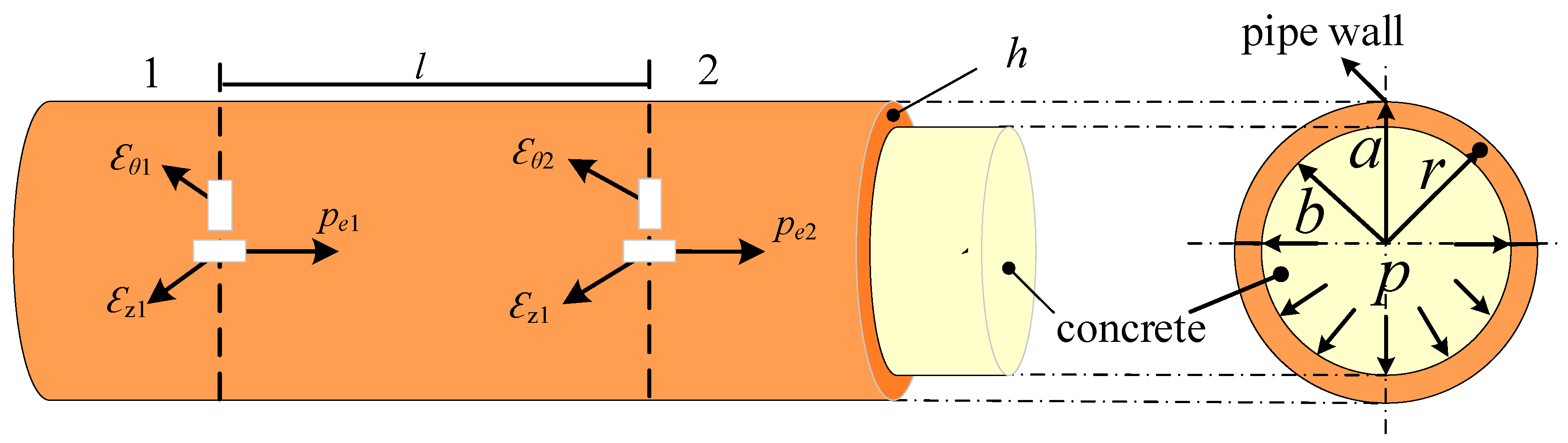
2. Concrete Pumping Pressure Loss Engineering Calculation
2.1. Experimental Measurements
2.2. Empirical Formula
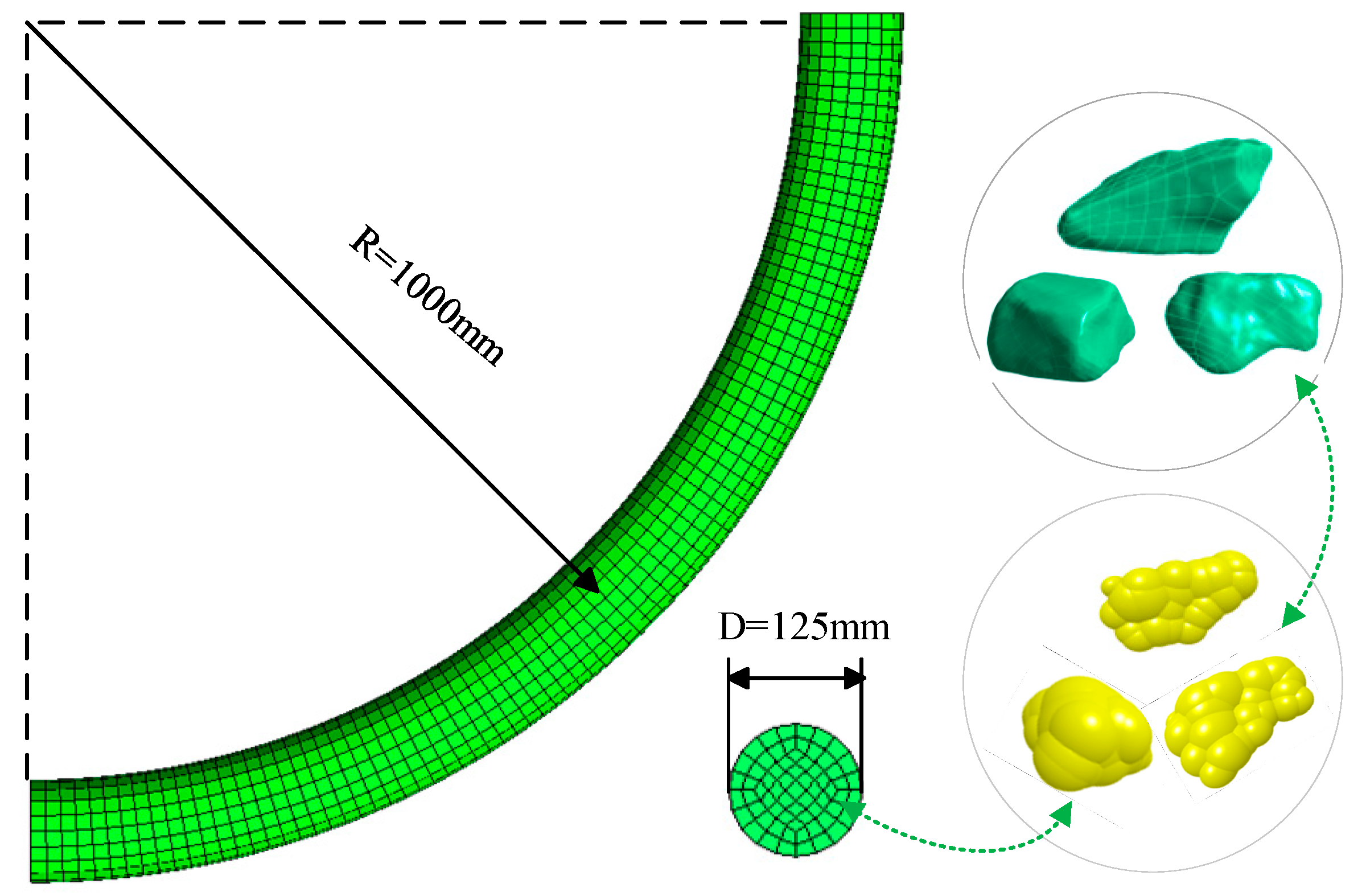
3. Concrete Pumping Particle Flow Simulation
3.1. The Principle of Particle Flow Simulation Calculation
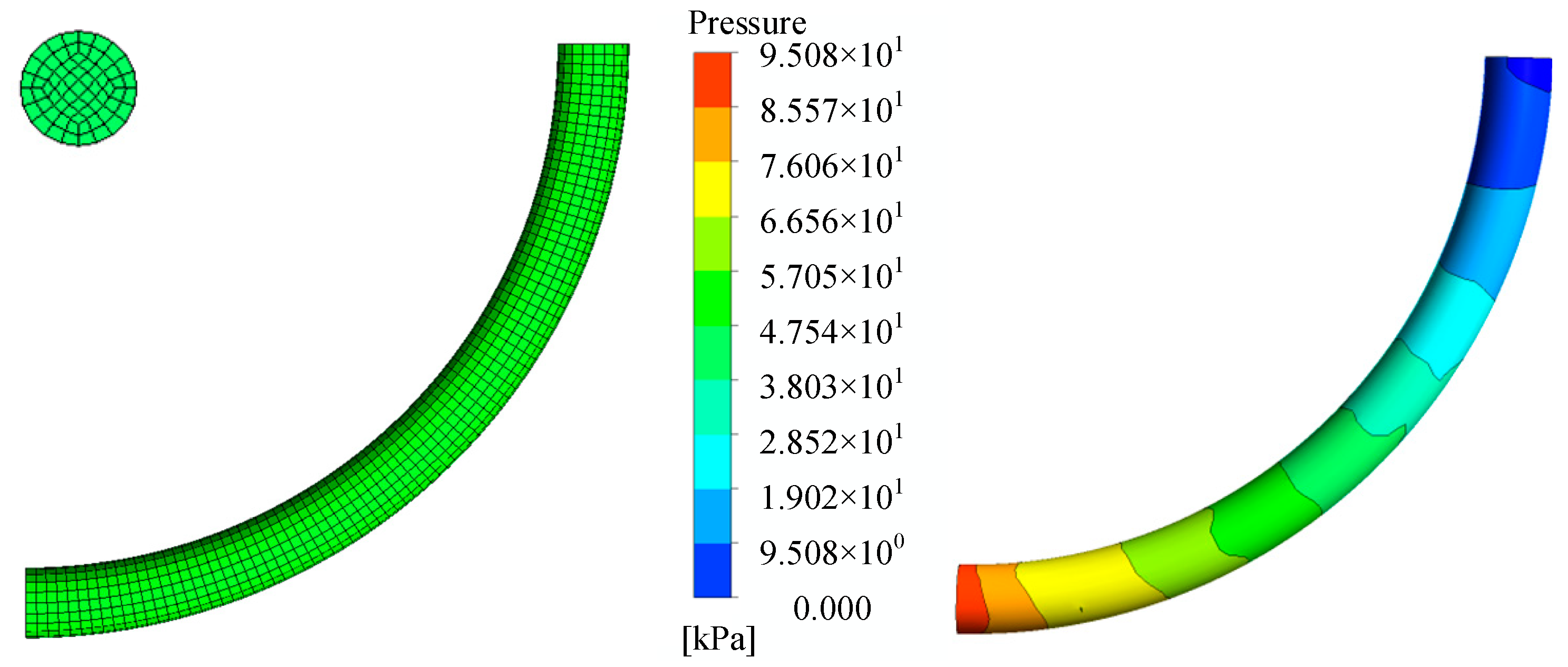
3.2. Particle Flow Numerical Simulation Verification
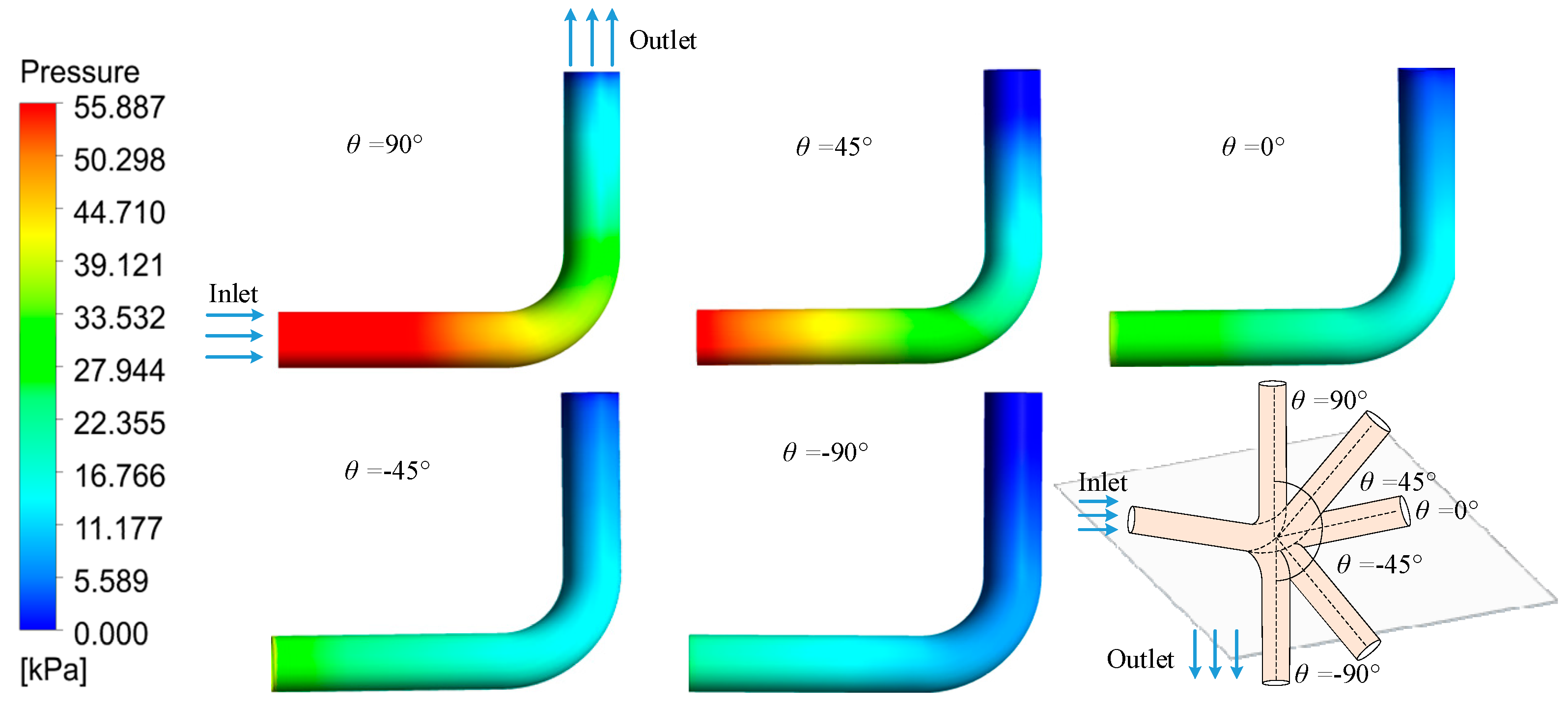
4. Pressure Loss Correction Model for Bend Section
4.1. One-Dimensional Flow Model for Horizontal Straight Pipe Section
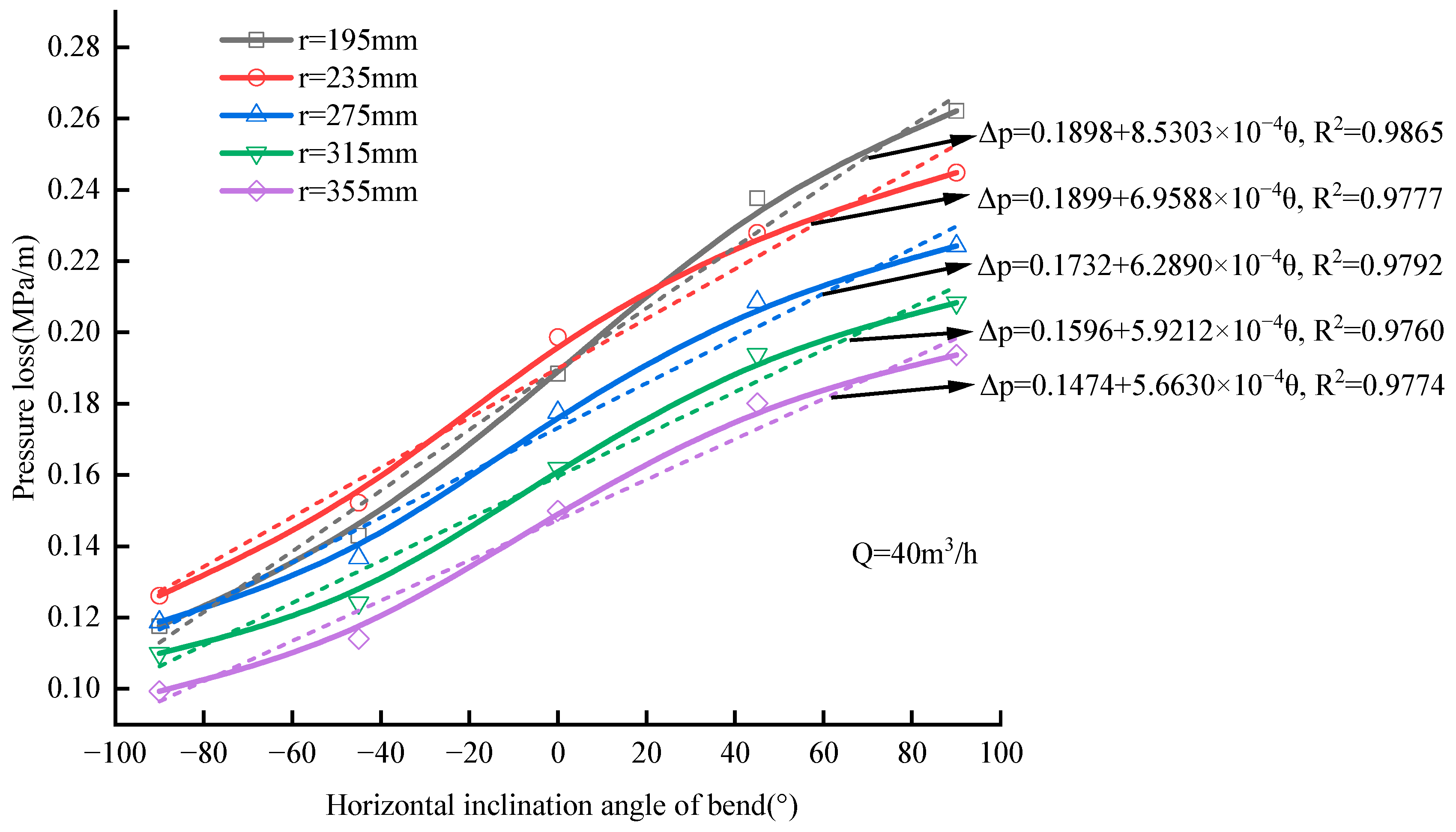
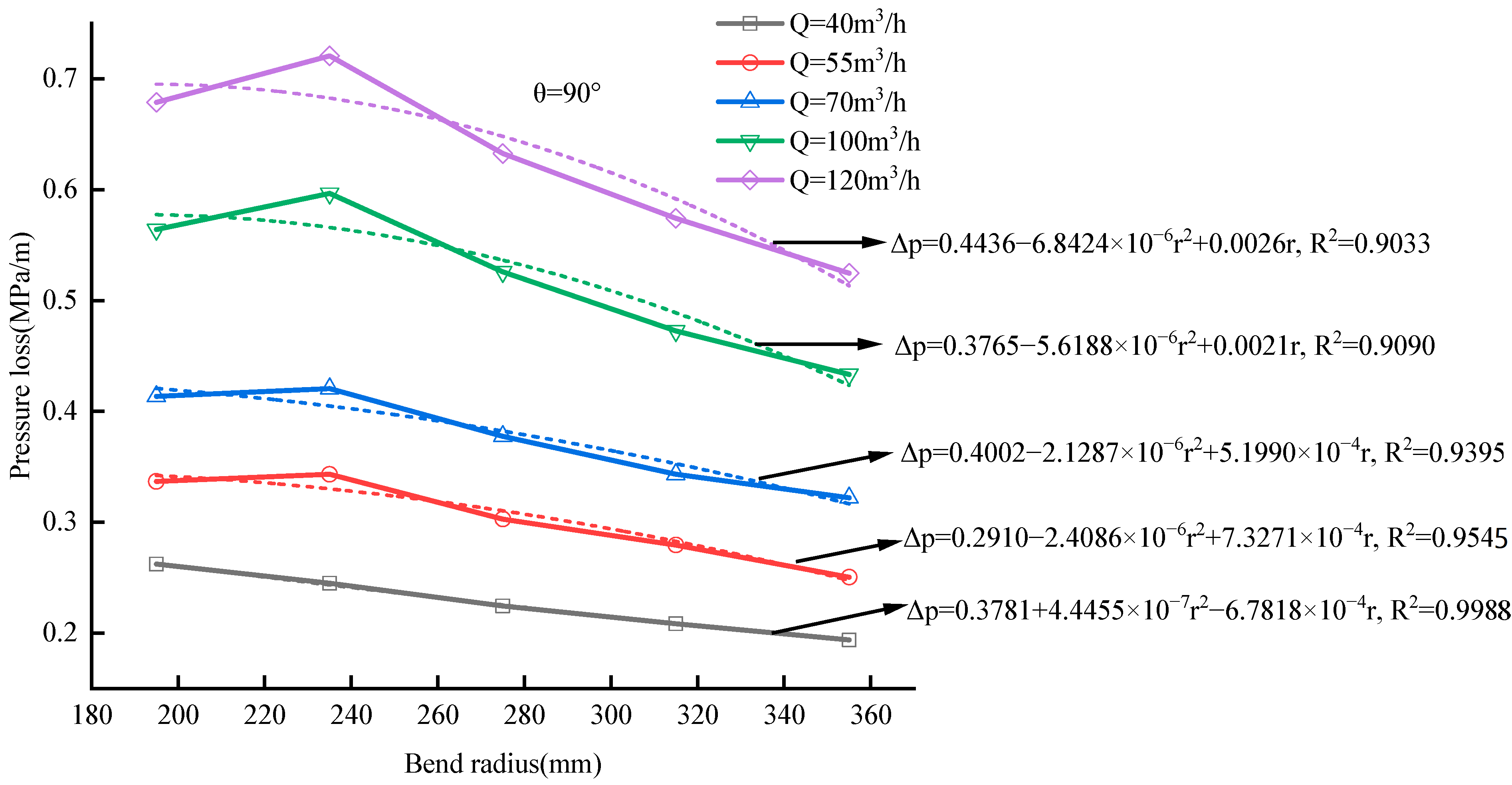
4.2. Sensitivity Analysis of Parameters in Bend Section
4.3. Correction Model
4.4. Verification of Pressure Loss in Bend Section
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, F.; Shen, W.; Yuan, Q.; Hu, X.; Li, Z.; Shi, C. An overview on the effect of pumping on concrete properties. Cem. Concr. Compos. 2022, 129, 104501. [Google Scholar] [CrossRef]
- Secrieru, E.; Mohamed, W.; Fataei, S.; Mechtcherine, V. Assessment and prediction of concrete flow and pumping pressure in pipeline. Cem. Concr. Compos. 2020, 107, 103495. [Google Scholar] [CrossRef]
- Lee, J.S.; Kim, E.S.; Jang, K.P.; Park, C.K.; Kwon, S.H. Prediction of concrete pumping based on correlation between slump and rheological properties. Adv. Concr. Constr. 2022, 13, 395–410. [Google Scholar]
- Jo, S.D.; Park, C.K.; Jeong, J.H.; Lee, S.H.; Kwon, S.H. A computational approach to estimating a lubricating layer in concrete pumping. Comput. Mater. Contin. 2012, 27, 189–210. [Google Scholar]
- Ingber, M.S.; Graham, A.L.; Mondy, L.A.; Fang, Z. An improved constitutive model for concentrated suspensions accounting for shear-induced particle migration rate dependence on particle radius. Int. J. Multiph. Flow 2009, 35, 270–276. [Google Scholar] [CrossRef]
- Choi, M.; Roussel, N.; Kim, Y.; Kim, J. Lubrication layer properties during concrete pumping. Cem. Concr. Res. 2013, 45, 69–78. [Google Scholar] [CrossRef]
- Choi, M.S.; Kim, Y.J.; Jang, K.P.; Kwon, S.H. Effect of the coarse aggregate size on pipe flow of pumped concrete. Constr. Build. Mater. 2014, 66, 723–730. [Google Scholar] [CrossRef]
- Kwon, S.H.; Park, C.K.; Jeong, J.H.; Jo, S.D.; Lee, S.H. Prediction of concrete pumping: Part II-Analytical prediction and experimental verification. ACI Mater. J. 2013, 110, 657. [Google Scholar]
- Kaplan, D.; de Larrard, F.; Sedran, T. Design of concrete pumping circuit. ACI Mater. J. 2005, 102, 110. [Google Scholar]
- Zhao, X.; Gao, G.; Wan, M.; Dai, J. One-Dimensional Modeling of the Pressure Loss in Concrete Pumping and Experimental Verification. Appl. Sci. 2024, 14, 3101. [Google Scholar] [CrossRef]
- H, Y.; C, C.; J, M. Engineering Fluid Mechanics; Southwest Jiaotong University Press: Chengdu, China, 2018. [Google Scholar]
- Beccati, N.; Ferrari, C. Predicting the capacity of an off-road self-loading drum mixer with different concrete consistencies through three-dimensional fluid dynamics analysis. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 379. [Google Scholar] [CrossRef]
- Nerella, V.N.; Mechtcherine, V. Virtual Sliding Pipe Rheometer for estimating pumpability of concrete. Constr. Build. Mater. 2018, 170, 366–377. [Google Scholar] [CrossRef]
- Wei, Z.Y.; An, X.P.; Shi, C.J.; Wu, B.; Yuan, Q. Pressure Loss Prediction of Fresh Concrete Pumping Based on CFD Simulation. Mater. Rep. 2019, 33, 3738–3743. [Google Scholar]
- Hao, J.; Jin, C.; Li, Y.; Wang, Z.; Liu, J.; Li, H. Simulation of Motion Behavior of Concrete in Pump Pipe by DEM. Adv. Civ. Eng. 2021, 2021, 3750589. [Google Scholar] [CrossRef]
- Cao, G.; Zhang, H.; Tan, Y.; Wang, J.; Deng, R.; Xiao, X.; Wu, B. Study on the Effect of Coarse Aggregate Volume Fraction on the Flow Behavior of Fresh Concrete via DEM. Procedia Eng. 2015, 102, 1820–1826. [Google Scholar] [CrossRef]
- Jiang, S.; Chen, X.; Cao, G.; Tan, Y.; Xiao, X.; Zhou, Y.; Wu, Y. Optimization of fresh concrete pumping pressure loss with CFD-DEM approach. Constr. Build. Mater. 2021, 276, 122204. [Google Scholar] [CrossRef]
- Xuan, Z.; Yonggang, Y.; Xinwei, Z. Research on Transient Multiphase Flow Field Characteristics Based on Fluid-structure Interaction. China Mech. Eng. 2023, 59, 406–417. [Google Scholar]
- De Schryver, R.; El Cheikh, K.; Yardimci, M.Y.; Lesage, K.; De Schutter, G. Fresh Concrete Pumping Arrest Investigation for Thixotropy by a CFD Modelling Approach; Cham, F., Ed.; Springer International Publishing: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Liao, Y.; Cheng, K.; Sun, W.; Zhao, Y. Study on pumping wear characteristics of concrete pipeline based on CFD-DEM coupling. Sci. Rep. 2023, 13, 16119. [Google Scholar] [CrossRef]
- Jiang, S.; Zhang, W.; Chen, X.; Cao, G.; Tan, Y.; Xiao, X.; Tong, Z. CFD-DEM simulation research on optimization of spatial attitude of concrete pumping boom based on evaluation of minimum pressure loss. Powder Technol. 2022, 403, 117365. [Google Scholar] [CrossRef]
- Morinaga, M. Pumpability of concrete and pumping pressure in pipelines. Fresh Concr. Important Prop. Their Meas. 1973, 7, 1–39. [Google Scholar]
- JGJ/T 10; Technical Specification for Construction of Concrete Pumping. Ministry of Housing and Urban-Rural Development of the People’s Republic of China: Beijing, China, 2011.
- Zhang, Z.; Mao, M.; Pan, Y.; Zhang, M.; Ma, S.; Cheng, Z.; Wu, Z. Experimental study for joint leakage process of tunnel lining and particle flow numerical simulation. Eng. Fail. Anal. 2022, 138, 106348. [Google Scholar] [CrossRef]
- Di Felice, R. The voidage function for fluid-particle interaction systems. Int. J. Multiph. Flow 1994, 20, 153–159. [Google Scholar] [CrossRef]
- Wen, S.; Lumin, Z.; Hanyang, H. Test and Discrete Element Simulation Study on Concrete Pumping Pressure Loss in Vertical Elbow. J. Wuhan Univ. Technol. 2021, 43, 48–55. [Google Scholar]


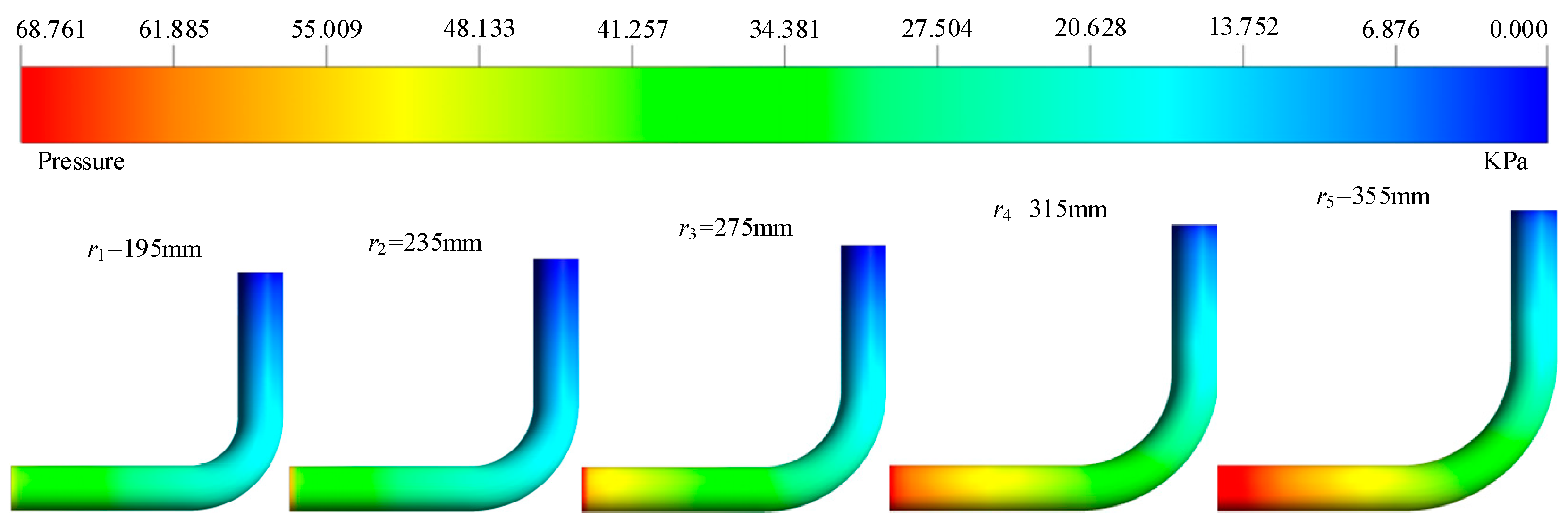
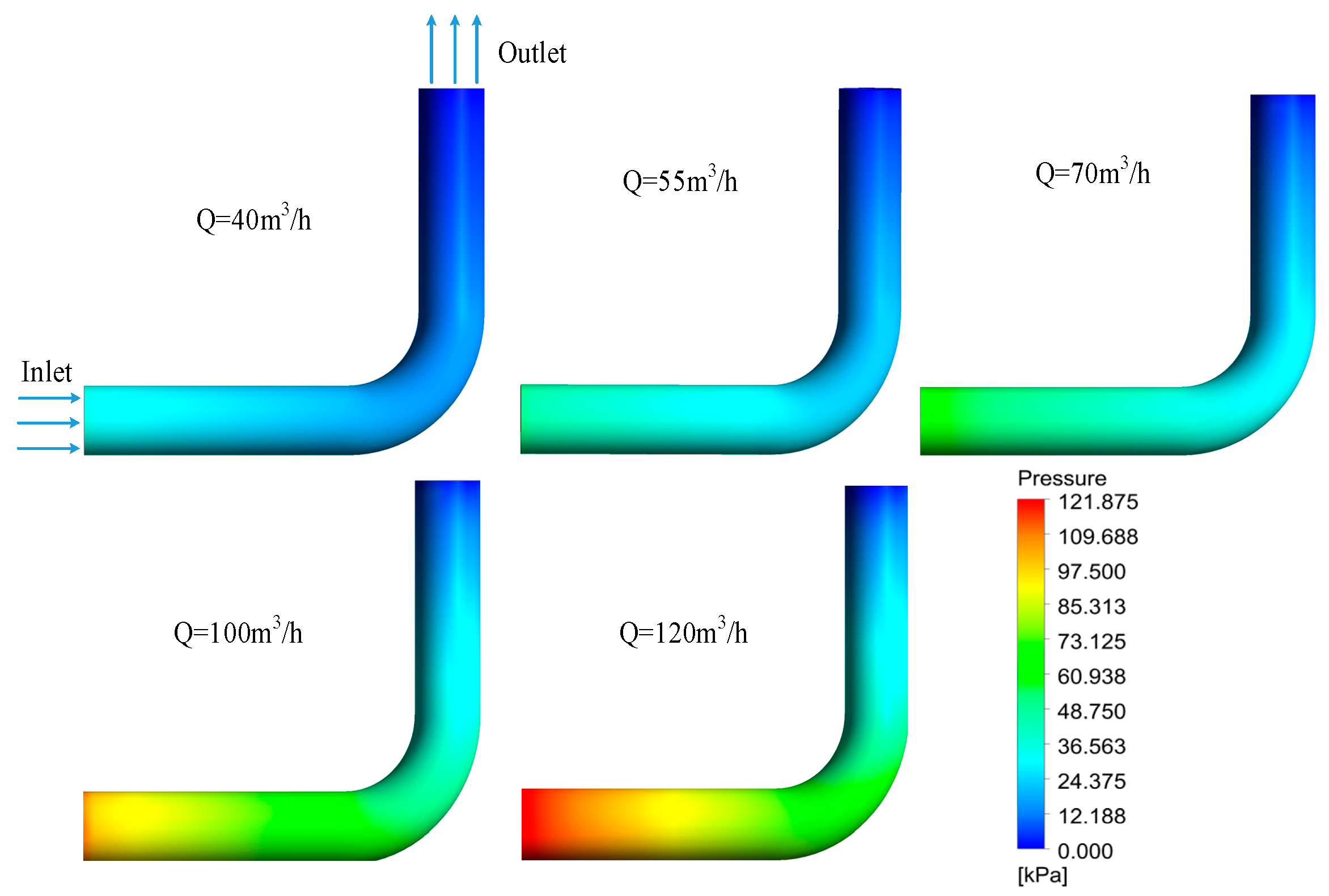

| Bend Type | Type of Conversion | Pipe Diameter/Curvature Radius (mm) | Conversion Length (m) |
|---|---|---|---|
| Bent Pipes | everyone | 500 | 12β/90 |
| Bent Pipes | everyone | 1000 | 9β/90 |
| Parameter | Value |
|---|---|
| Particle Poisson’s ratio | 0.35 |
| Pipe Poisson’s ratio | 0.30 |
| Particle–particle static friction coefficient | 0.30 |
| Particle–particle rolling friction coefficient | 0.02 |
| Particle–wall static friction coefficient | 0.20 |
| Particle–wall rolling friction coefficient | 0.03 |
| Particle–wall coefficient of restitution | 0.60 |
| Particle–particle coefficient of restitution | 0.70 |
| Average Flow Velocity | Experimental Data Value | Simulated Calculation of Pressure Loss Value | Relative Error |
|---|---|---|---|
| 0.63 m/s | 90.1 KPa/m | 83.6 KPa/m | −6.59% |
| 0.89 m/s | 96.8 KPa/m | 89.6 KPa/m | −7.43% |
| 1.13 m/s | 102.3 KPa/m | 93.8 KPa/m | −8.28% |
| Horizontal Inclination Angle | Pumping Flow Rate (m3/h) | Radii of Curvature (mm) | ||||
|---|---|---|---|---|---|---|
| 195 | 235 | 275 | 315 | 355 | ||
| 0° | 40 | 0.1884 | 0.1987 | 0.1776 | 0.1619 | 0.1499 |
| 55 | 0.2632 | 0.2763 | 0.2408 | 0.2259 | 0.2075 | |
| 70 | 0.3305 | 0.3616 | 0.3202 | 0.2874 | 0.2638 | |
| 100 | 0.4895 | 0.5287 | 0.4673 | 0.4268 | 0.3912 | |
| 120 | 0.6079 | 0.6555 | 0.5757 | 0.5158 | 0.4750 | |
| 45° | 40 | 0.2376 | 0.2279 | 0.2086 | 0.1938 | 0.1801 |
| 55 | 0.3098 | 0.3238 | 0.2876 | 0.2649 | 0.2420 | |
| 70 | 0.3872 | 0.4058 | 0.3552 | 0.3226 | 0.3037 | |
| 100 | 0.5425 | 0.5749 | 0.5124 | 0.4613 | 0.4232 | |
| 120 | 0.6572 | 0.7031 | 0.6181 | 0.5555 | 0.5121 | |
| 90° | 40 | 0.2622 | 0.2448 | 0.2243 | 0.2083 | 0.1937 |
| 55 | 0.3368 | 0.3432 | 0.3028 | 0.2794 | 0.2503 | |
| 70 | 0.4135 | 0.4206 | 0.3775 | 0.3432 | 0.3221 | |
| 100 | 0.5641 | 0.5966 | 0.5258 | 0.4726 | 0.4334 | |
| 120 | 0.6789 | 0.7208 | 0.6327 | 0.5742 | 0.5247 | |
| −45° | 40 | 0.1430 | 0.1522 | 0.1368 | 0.1241 | 0.1140 |
| 55 | 0.2205 | 0.2373 | 0.2109 | 0.1959 | 0.1783 | |
| 70 | 0.2920 | 0.3220 | 0.2838 | 0.2557 | 0.2456 | |
| 100 | 0.4465 | 0.4902 | 0.4322 | 0.3892 | 0.3589 | |
| 120 | 0.5627 | 0.6216 | 0.5411 | 0.4850 | 0.4423 | |
| −90° | 40 | 0.1176 | 0.1261 | 0.1187 | 0.1099 | 0.0993 |
| 55 | 0.2023 | 0.2176 | 0.1913 | 0.1733 | 0.1574 | |
| 70 | 0.2810 | 0.3048 | 0.2667 | 0.2427 | 0.2157 | |
| 100 | 0.4331 | 0.4778 | 0.4155 | 0.3734 | 0.3414 | |
| 120 | 0.5388 | 0.5978 | 0.5249 | 0.4721 | 0.4324 | |
| Pumping Flow Rate (m/s) | Morinage Empirical Formula + the Converted Horizontal Length Method | One-Dimensional Flow Model + the Converted Horizontal Length Method | One-Dimensional Flow Correction Model | Experimental Measurement Values [26] |
|---|---|---|---|---|
| 0.63 | 0.0505 | 0.2016 | 0.0995 | 0.0901 |
| 0.77 | 0.0581 | 0.1926 | 0.1042 | 0.0942 |
| 0.89 | 0.0647 | 0.1971 | 0.1150 | 0.0968 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, G.; Wei, L.; Zhao, X.; Wan, M.; Li, H. The One-Dimensional Flow Pressure Loss Correction Model Based on the Particle Flow through Concrete Bend. Appl. Sci. 2024, 14, 8824. https://doi.org/10.3390/app14198824
Gao G, Wei L, Zhao X, Wan M, Li H. The One-Dimensional Flow Pressure Loss Correction Model Based on the Particle Flow through Concrete Bend. Applied Sciences. 2024; 14(19):8824. https://doi.org/10.3390/app14198824
Chicago/Turabian StyleGao, Guoqiang, Lixiang Wei, Xuan Zhao, Minshun Wan, and Hongzhou Li. 2024. "The One-Dimensional Flow Pressure Loss Correction Model Based on the Particle Flow through Concrete Bend" Applied Sciences 14, no. 19: 8824. https://doi.org/10.3390/app14198824
APA StyleGao, G., Wei, L., Zhao, X., Wan, M., & Li, H. (2024). The One-Dimensional Flow Pressure Loss Correction Model Based on the Particle Flow through Concrete Bend. Applied Sciences, 14(19), 8824. https://doi.org/10.3390/app14198824






