Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer Augmentation for Flight Dynamics
Abstract
:1. Introduction
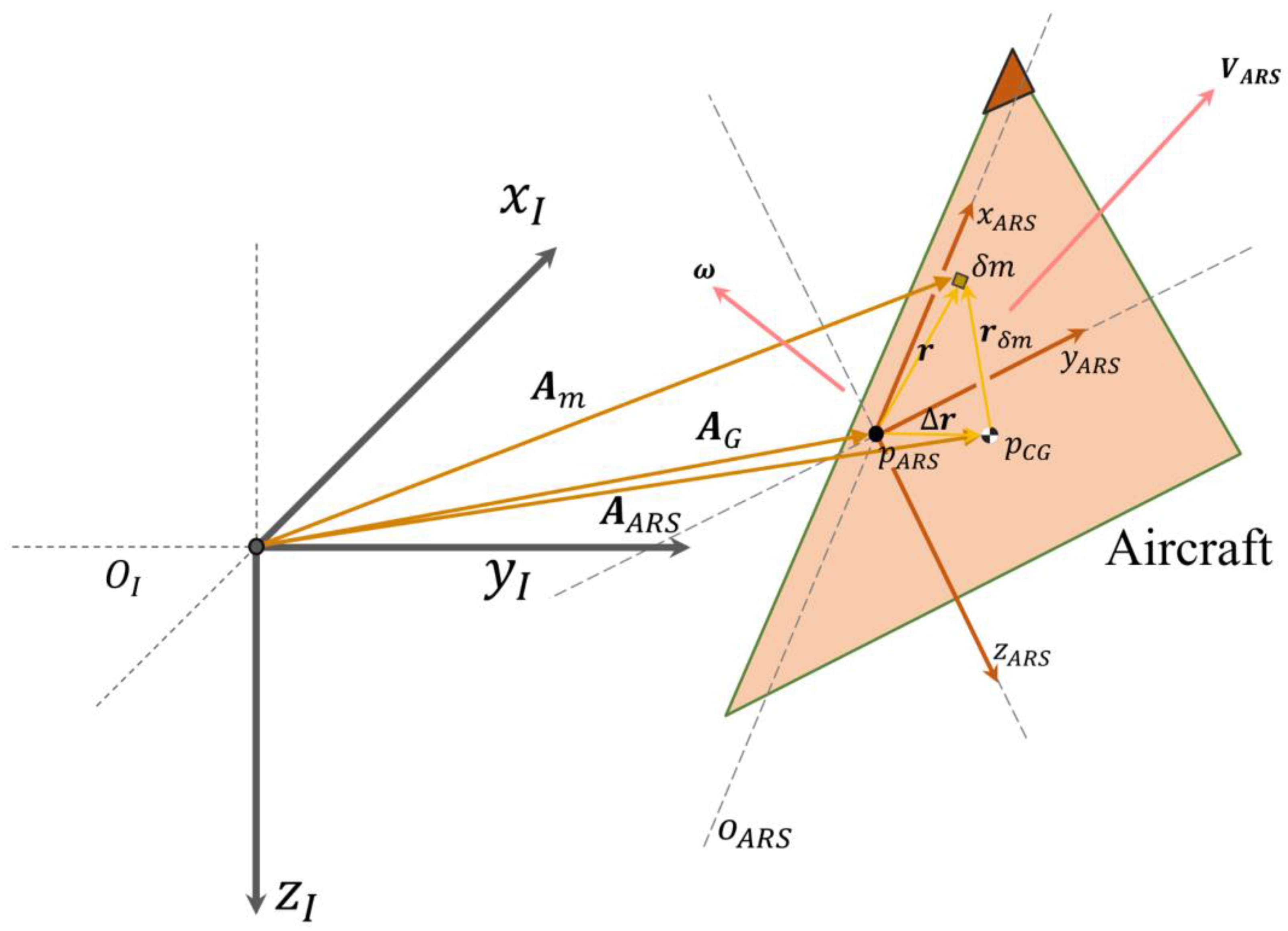
2. Problem Statement
3. Equation of Motion
4. Proposed Control Method
4.1. Incremental Dynamics
4.2. Nonlinear Disturbance Observer
4.3. Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer
4.4. Control Objective
5. Stability Analysis
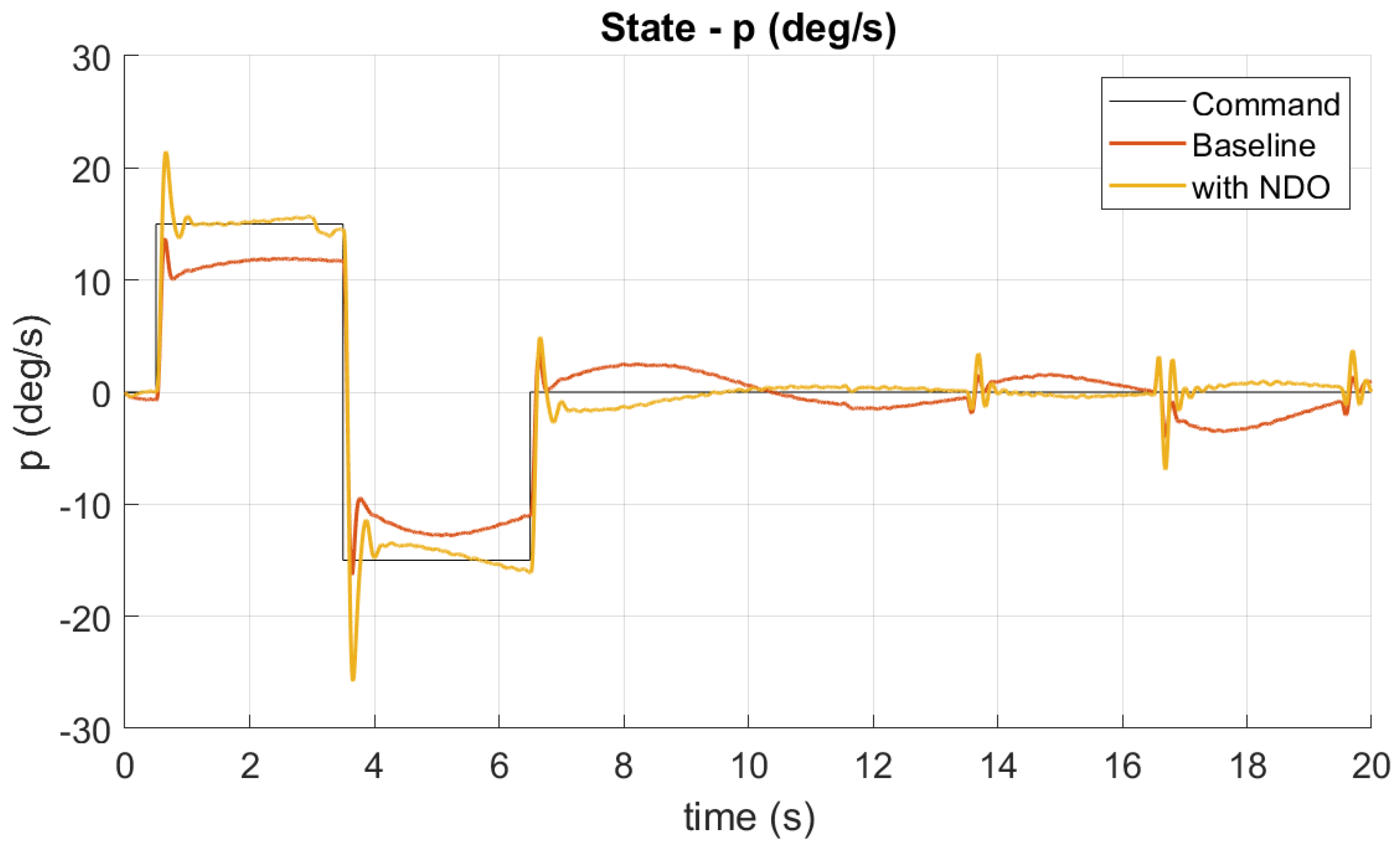
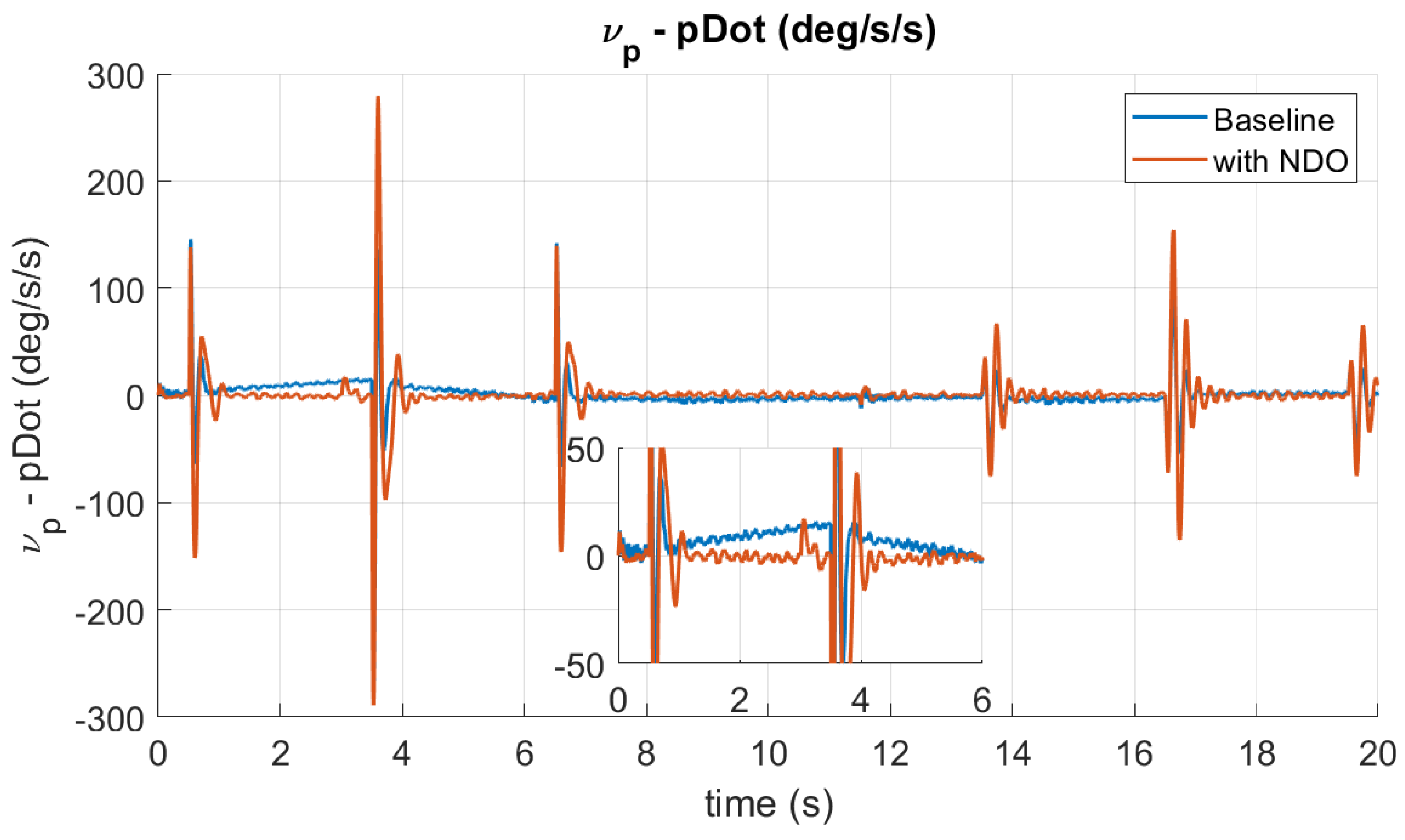
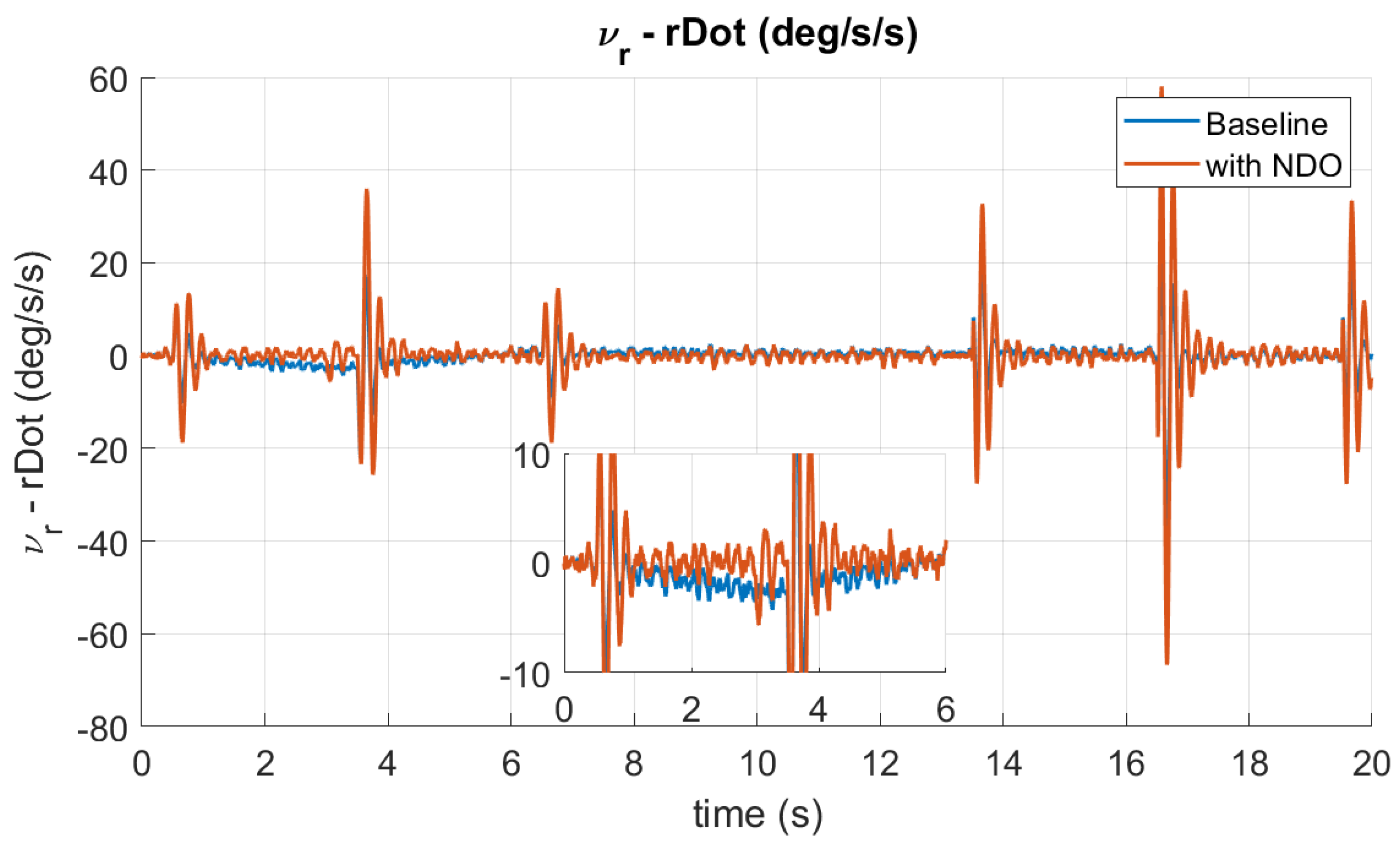
6. Simulation Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Nelson, R.C. Flight Stability and Automatic Control; WCB, McGraw Hill: New York, NJ, USA, 1998; Volume 2. [Google Scholar]
- Chrif, L.; Kadda, Z.M. Aircraft control system using LQG and LQR controller with optimal estimation-Kalman filter design. Proc. Eng. 2014, 80, 245–257. [Google Scholar] [CrossRef]
- Gadewadikar, J.; Lewis, F. Aircraft flight controller tracking design using h-infinity static output-feedback. Trans. Inst. Meas. Control 2006, 28, 429–440. [Google Scholar] [CrossRef]
- Balas, G.J. Flight control law design: An industry perspective. Eur. J. Control 2003, 9, 207–226. [Google Scholar] [CrossRef]
- Prasad, B.; Pradeep, S. Automatic landing system design using feedback linearization method. In Proceedings of the AIAA Infotech@Aerospace 2007 Conference and Exhibit, Rohnert Park, CA, USA, 7–10 May 2007; p. 2773. [Google Scholar]
- Härkegård, O. Backstepping and Control Allocation with Applications to Flight Control. Ph.D. Thesis, Linköpings Universitet, Linköping, Sweden, 2003. [Google Scholar]
- Slotine, J.J.E. Applied Nonlinear Control; Prentice Hall: Upper Saddle River, NJ, USA, 1991; Volume 199. [Google Scholar]
- Lu, Q.; Sun, Y.; Mei, S. Nonlinear Control Systems and Power System Dynamics; Kluwer Academic Publishers: Norwell, MA, USA, 2001; Volume 10. [Google Scholar]
- Lee, C.H.; Jun, B.E.; Lee, J.I. Connections between linear and nonlinear missile autopilots via three-loop topology. J. Guid. Control Dyn. 2016, 39, 1426–1432. [Google Scholar] [CrossRef]
- Balas, G.; Hodgkinson, J. Control design methods for good flying qualities. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference, Chicago, IL, USA, 10–13 August 2009; p. 6319. [Google Scholar]
- van’t Veld, R. Incremental Nonlinear Dynamic Inversion Flight Control: Stability and Robustness Analysis and Improvements. Master’s Thesis, TU Delft, Delft, The Netherlands, 2016. [Google Scholar]
- Smith, P. A simplified approach to nonlinear dynamic inversion based flight control. In Proceedings of the 23rd Atmospheric Flight Mechanics Conference, Boston, MA, USA, 10–12 August 1998; p. 4461. [Google Scholar]
- Bacon, B.J.; Ostroff, A.J.; Joshi, S.M. Reconfigurable NDI controller using inertial sensor failure detection & isolation. IEEE Trans. Aerosp. Electron. Syst. 2001, 37, 1373–1383. [Google Scholar]
- Ronald, V.; Kampen, E.J.V.; Chu, Q.P. Stability and robustness analysis and improvements for incremental nonlinear dynamic inversion control. In Proceedings of the 2018 AIAA Guidance, Navigation, and Control Conference, Kissimmee, FL, USA, 8–12 January 2018; p. 1127. [Google Scholar]
- Smeur, E.J.; Chu, Q.; Croon, G.C. Adaptive incremental nonlinear dynamic inversion for attitude control of micro air vehicles. J. Guid. Control Dyn. 2016, 39, 450–461. [Google Scholar] [CrossRef]
- Steffensen, R.; Steinert, A.; Holzapfel, F. Incremental control as an enhanced and robust implementation of gain scheduled controllers avoiding hidden coupling terms. Aerosp. Sci. Technol. 2023, 141, 108500. [Google Scholar] [CrossRef]
- Shtessel, Y.; Edwards, C.; Fridman, L.; Levant, A. Sliding Mode Control and Observation; Springer: New York, NY, USA, 2014; Volume 10. [Google Scholar]
- Lee, C.H.; Kim, T.H.; Tahk, M.J. Agile missile autopilot design using nonlinear backstepping control with time-delay adaptation. Trans. Jpn. Soc. Aeronaut. Space Sci. 2014, 57, 9–20. [Google Scholar]
- Ignatyev, D.; Shin, H.S.; Tsourdos, A. Gaussian process adaptive incremental backstepping flight control. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 2032. [Google Scholar]
- Chen, W.H.; Ostroff, A.J.; Joshi, S.M. A nonlinear disturbance observer for robotic manipulators. IEEE Trans. Ind. Electron. 2000, 47, 932–938. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, Y.; Zhu, W.; Fu, C.; Lu, K. A comprehensive study on seismic dynamic responses of stochastic structures using sparse grid-based polynomial chaos expansion. Eng. Struct. 2024, 306, 117753. [Google Scholar] [CrossRef]
- Zhao, H.; Fu, C.; Zhang, Y.; Zhu, W.; Lu, K.; Francis, E.M. Dimensional decomposition-aided metamodels for uncertainty quantification and optimization in engineering: A review. Comput. Methods Appl. Mech. Eng. 2024, 428, 117098. [Google Scholar] [CrossRef]
- Bacon, B.; Gregory, I. General equations of motion for a damaged asymmetric aircraft. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference and Exhibit, Hilton Head, SC, USA, 20–23 August 2007; p. 6306. [Google Scholar]
- Kim, L.; Lee, J.D.; Zewege, N.S.; Bang, H. Gaussian process-based state derivative estimator with temporal input in incremental flight control design. Aerosp. Sci. Technol. 2024, 148, 109070. [Google Scholar] [CrossRef]
- Nguyen, N.; Krishnakumar, K.; Kaneshige, J.; Nespeca, P. Flight dynamics and hybrid adaptive control of damaged aircraft. J. Guid. Control Dyn. 2008, 31, 751–764. [Google Scholar] [CrossRef]
- Yan, B.; Li, Y.; Dai, P.; Liu, S. Aerodynamic analysis, dynamic modeling, and control of a morphing aircraft. J. Aerosp. Eng. 2019, 32, 04019058. [Google Scholar] [CrossRef]
- Khalil, H.K. Nonlinear Systems, 3rd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2002. [Google Scholar]
- Ouellette, J.; Raghavan, B.; Patil, M.; Kapania, R. Flight dynamics and structural load distribution for a damaged aircraft. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference, Chicago, IL, USA, 10–13 August 2009; p. 6153. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, L.; Kim, J.I. Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer Augmentation for Flight Dynamics. Appl. Sci. 2024, 14, 10615. https://doi.org/10.3390/app142210615
Kim L, Kim JI. Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer Augmentation for Flight Dynamics. Applied Sciences. 2024; 14(22):10615. https://doi.org/10.3390/app142210615
Chicago/Turabian StyleKim, Lamsu, and Jeong I. Kim. 2024. "Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer Augmentation for Flight Dynamics" Applied Sciences 14, no. 22: 10615. https://doi.org/10.3390/app142210615
APA StyleKim, L., & Kim, J. I. (2024). Incremental Nonlinear Dynamics Inversion Control with Nonlinear Disturbance Observer Augmentation for Flight Dynamics. Applied Sciences, 14(22), 10615. https://doi.org/10.3390/app142210615




