Study on Strength of Slip Soil–Tuff Contact Surfaces in Tuff Landslide Based on Model Test
Abstract
1. Introduction
2. Materials and Methods
2.1. Instruments
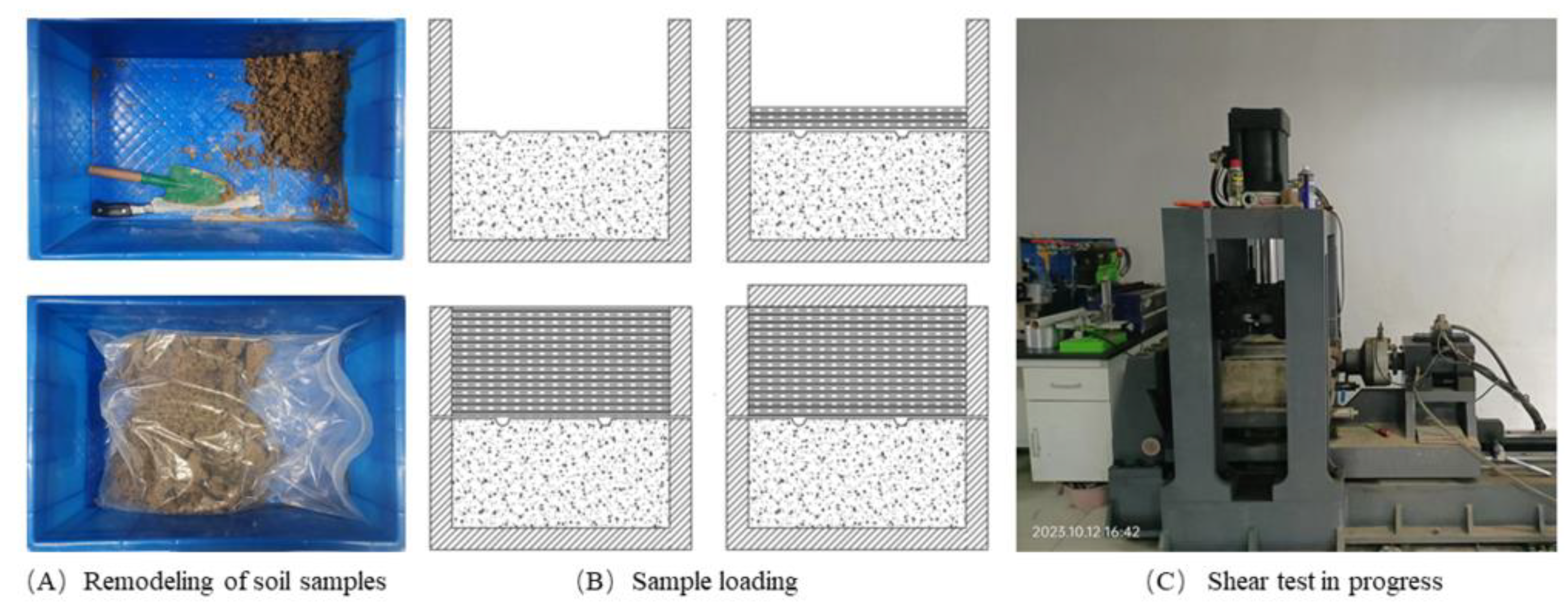
2.2. Preparation of Materials and Samples
2.3. Experimental Process
- (1)
- The mass of slip soil required for the test was calculated based on the volume of soil required for the test (volume of the upper shear box plus the volume of the grooves on the surface of the concrete block) and the slip soil was dried, pulverized, sieved (2 cm), and remolded according to the parameters in Table 1. Deionized water was used throughout the remolding process.
- (2)
- The shear box and its guide rails were cleaned, and a layer of Vaseline jelly was applied to the inside; the securing pins were inserted into the upper and lower shear boxes. Markers were marked on the inside wall of the upper shear box with chalk at 20 mm intervals from the bottom to the top.
- (3)
- The prepared concrete block was placed into the lower shear box, and the grooves on the concrete block were kept perpendicular to the shear direction; we ensured that the concrete block was in tight contact with all four walls of the shear box and that the upper surface was flush with the upper edge of the shear box.
- (4)
- The mass of soil required to fill the grooves by the volume of the grooves on the concrete block’s surface was calculated. This portion of the soil sample from the remolded slip soil sample using a weighing scale was removed, and the remaining soil sample was divided into five equal parts by mass.
- (5)
- One of the five equal parts of the soil sample from Step 4 was taken and mixed with the soil sample that filled the grooves. One more soil sample and four soil samples of uniformly less weight were obtained.
- (6)
- The larger mass of the soil sample was filled in the shear box first; its surface was gently swept and compacted evenly with a lightweight rubber mallet. We ensured that it was 20 mm thick according to the markers marked in Step 1.
- (7)
- The remaining four soil samples were sequentially loaded into the shear box; we swept their surfaces each time and compacted them evenly with a lightweight rubber mallet, thus ensuring that the thickness of each layer was 20 mm.
- (8)
- After all soil samples were loaded, the surface of the soil samples was planned to ensure uniformity. Finally, the pins were removed, the soil surface was covered with a rigid cover plate, the box was pushed into the test system, and a vertical load of 50 kPa was applied for 5 min.
- (9)
- We applied vertical stress and horizontal force to the shear test and collected the data using sensors and computers in real time.
3. Analysis of Shear Test Results
3.1. Calculation of Contact Interface Friction (R)
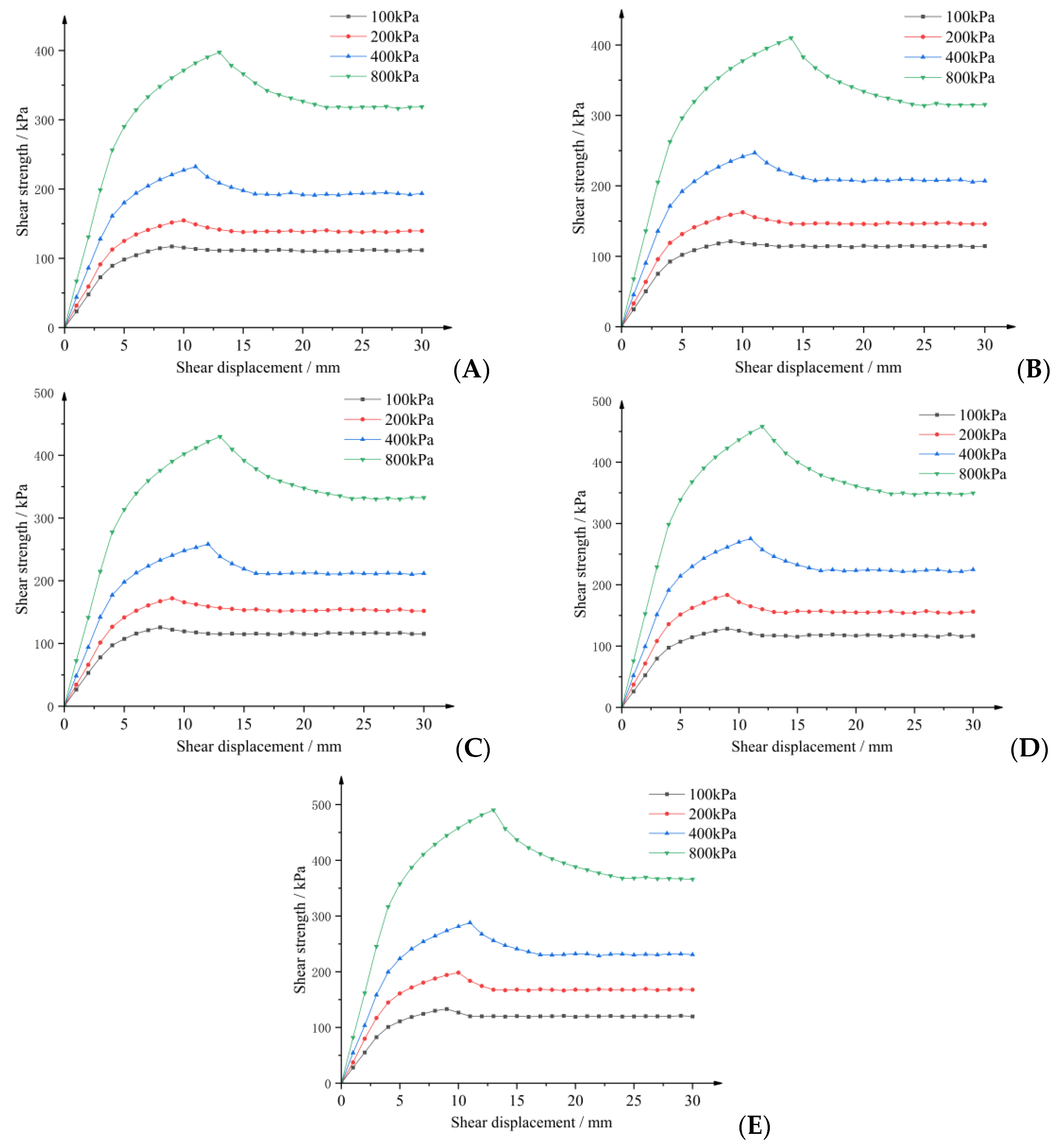
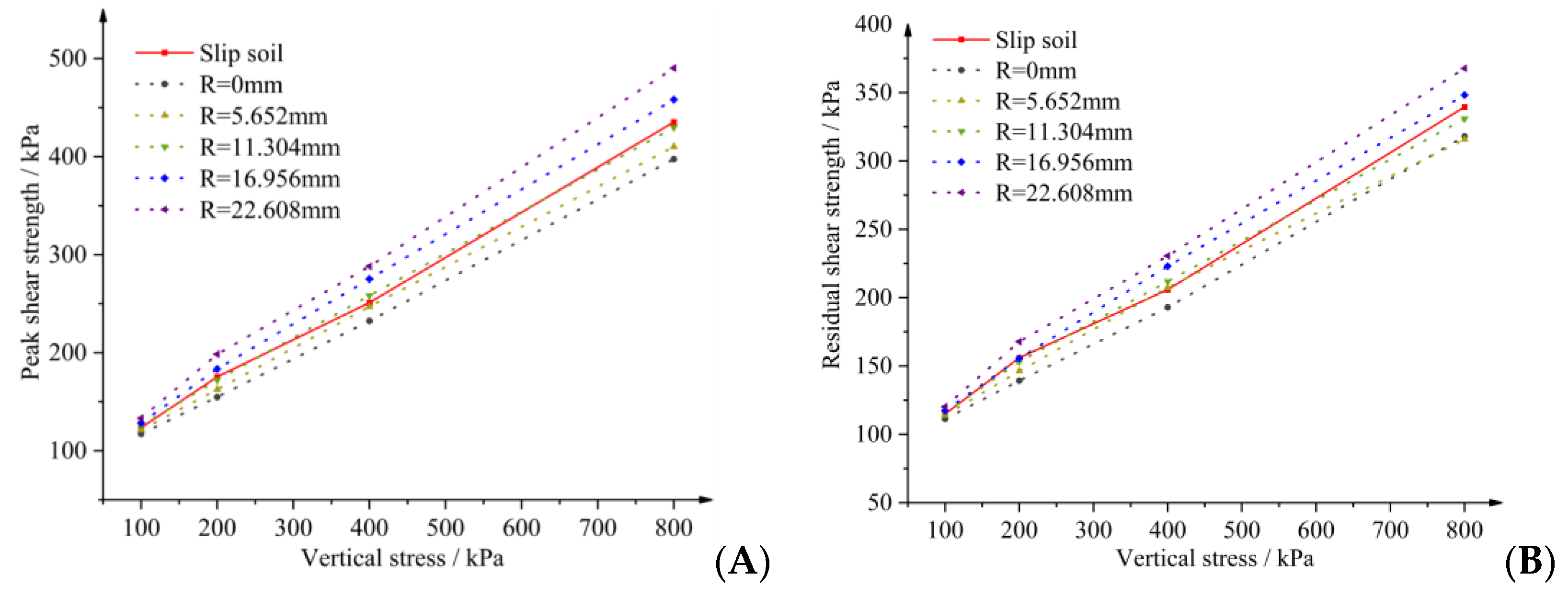
3.2. Results of Slip Soils
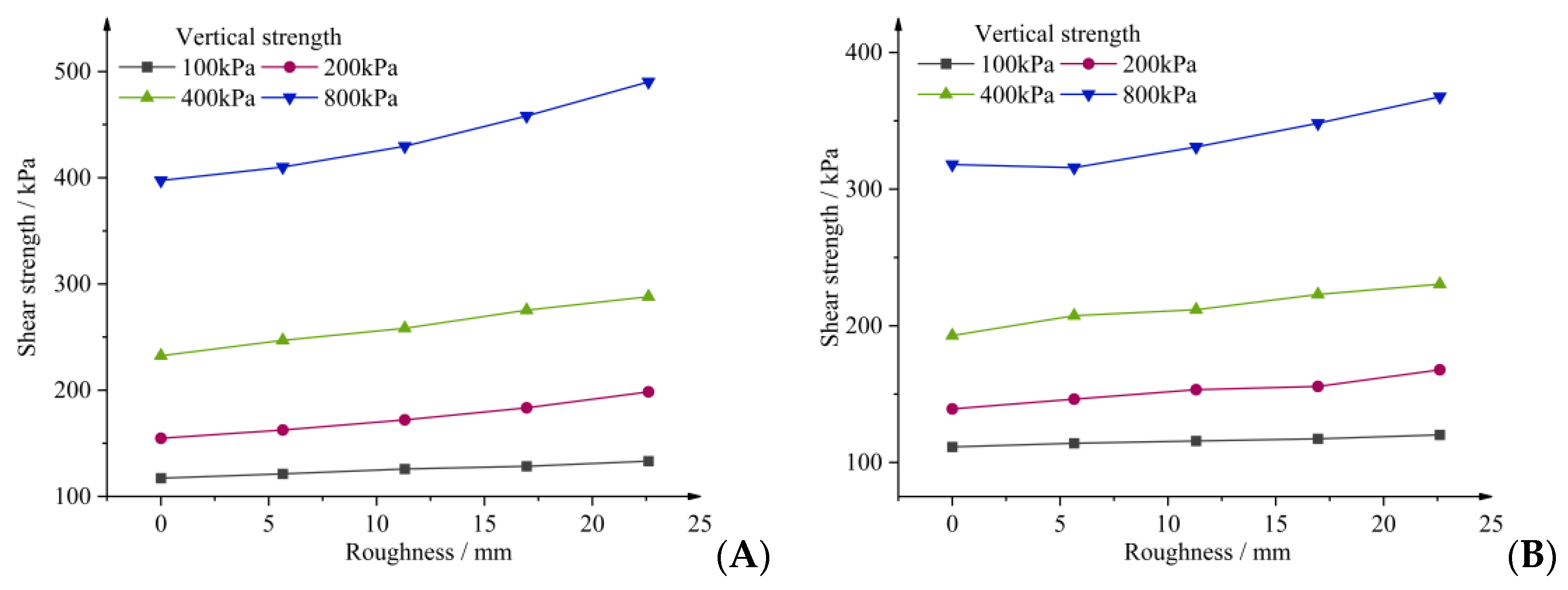
3.3. Effect of Vertical Stress on Different Roughness
3.4. Effect of Roughness on Shear Strength
- (1)
- When the roughness of the contact surface was low, the contact surface’s peak shear strength and residual shear strength were lower than that of the slip soil itself.
- (2)
- When the roughness of the contact surface reached R3 (11.304 mm), the shear strengths of the contact surface fell near the strength of the soil itself at different vertical stresses, which can be regarded as basically equal to the strength of the slip soil.
- (3)
- When the roughness of the contact surface was more significant than 11.304 mm, the shear strength of the contact surface was higher than the strength of the soil itself.
3.5. Shear Strength of Slip Soil-Rigid Contact Surfaces Considering Roughness
4. Conclusions
- (1)
- At low vertical stress, the stress–displacement curves show a folded line shape at all roughness levels. With the increase in vertical stress, the stress–displacement curves of all roughness levels are gradually transformed into a hyperbolic shape.
- (2)
- The roughness of the contact surface of the slip soil and tuff determines the slip soil’s failure mode and shear strength. In nature, shear failure of low roughness contact surfaces is a sliding failure in the partial contact region. Shear failure of a contact surface with high roughness is an elastic–plastic failure within the slip soil. When the roughness is low, the shear strength of the contact surface is less than the shear strength of the slip soil itself; when the roughness is high, the shear strength of the contact surface is higher than the shear strength of the slip soil itself.
- (3)
- Based on Moore–Cullen’s law, a formula for calculating the shear strength of the contact surface of the slip soil as a function of roughness was found. The formula has a correlation coefficient of R2 = 0.982, which is compatible with the test data and can be used as a reference for evaluating the shear strength of the interface between slip soil and rigid objects.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yin, Q.; Wu, J.; Zhu, C.; He, M.; Meng, Q.; Jing, H. Shear mechanical responses of sandstone exposed to high temperature under constant normal stiffness boundary conditions. Geomech. Geophys. Geo-Energy Geo-Resour. 2021, 7, 35. [Google Scholar] [CrossRef]
- Boukpeti, N.; White, D.J. Interface shear box tests for assessing axial pipe–soil resistance. Géotechnique 2016, 67, 18–30. [Google Scholar] [CrossRef]
- Hu, L.; Pu, J. Testing and modeling of soil-structure interface. J. Geotech. Geoenviron. Eng. 2004, 130, 851–860. [Google Scholar] [CrossRef]
- Yazdani, S.; Helwany, S.; Olgun, G. Influence of temperature on soil–pile interface shear strength. Geomech. Energy Environ. 2019, 18, 69–78. [Google Scholar] [CrossRef]
- Fleming, I.R.; Sharma, J.S.; Jogi, M.B. Shear strength of geomembrane–soil interface under unsaturated conditions. Geotext. Geomembr. 2006, 24, 274–284. [Google Scholar] [CrossRef]
- Martinez, A.; Frost, J.D. The influence of surface roughness form on the strength of sand–structure interfaces. Géotechnique Lett. 2017, 7, 104–111. [Google Scholar] [CrossRef]
- Martinez, A.; Stutz, H.H. Rate effects on the interface shear behaviour of normally and overconsolidated clay. Géotechnique 2019, 69, 801–815. [Google Scholar] [CrossRef]
- Xu, Q.; Wang, W.; Li, L. Failure mechanism of gently inclined shallow landslides along the soil-bedrock interface on ring shear tests. Bull. Eng. Geol. Environ. 2021, 80, 3733–3746. [Google Scholar] [CrossRef]
- Senthilkumar, V.; Chandrasekaran, S.S.; Maji, V.B. Rainfall-induced landslides: Case study of the Marappalam landslide, Nilgiris District, Tamil Nadu, India. Int. J. Geomech. 2018, 18, 05018006. [Google Scholar] [CrossRef]
- Wang, L.; Jiang, H.; Yang, Z. Development of discontinuous deformation analysis with displacement-dependent interface shear strength. Comput. Geotech. 2013, 47, 91–101. [Google Scholar] [CrossRef]
- Potyondy, J.G. Skin friction between various soils and construction materials. Géotechnique 1961, 11, 339–353. [Google Scholar] [CrossRef]
- Jewell, R.A.A. Direct shear tests on sand. Geotechnique 1989, 39, 309–322. [Google Scholar] [CrossRef]
- Yoshimi, Y.; Kishida, T. A ring torsion apparatus for evaluating friction between soil and metal surfaces. Geotech Test. J. 1981, 4, 8. [Google Scholar] [CrossRef]
- Kou, H.L.; Diao, W.Z.; Zhang, W.C.; Zheng, J.B.; Wu, C. Experimental study of interface shearing between calcareous sand and steel plate considering surface roughness and particle size. Appl. Ocean. Res. 2021, 107, 102490. [Google Scholar] [CrossRef]
- Clough, G.W.; Duncan, J.M. Finite element analyses of retaining wall behavior. J. Soil Mech. Found. Eng. 1971, 97, 1657–1673. [Google Scholar] [CrossRef]
- Uesugi, M.; Kishida, H. Frictional resistance at yield between dry sand and mild steel. Soils Found. 1986, 26, 139–149. [Google Scholar] [CrossRef]
- Huck, P.J.L.T. Dynamic Response of Soil/Concrete Interfaces at High Pressure; Air Force Weapons Laboratory: Washington, DC, USA, 1974. [Google Scholar]
- Tiwari, B.; Ajmera, B.; Kaya, G. Shear Strength Reduction at Soil Structure Interface. In GeoFlorida 2010: Advances in Analysis, Modeling & Design; ASCE: Washington, DC, USA, 2010. [Google Scholar]
- Liu, F.; Wang, P.; Wang, J.; Cai, Y. Cyclic and post-cyclic shear behavior of sand-geogrid interface under different shear rates. Chin. J. Rock Mech. Eng. 2016, 35, 387–395. [Google Scholar]
- Kulatilake, P.; Um, J.; Panda, B.; Nghiem, N. Development of new peak shear strength criterion for anisotropic rock joints. J. Eng. Mech. ASCE 1999, 125, 1010–1017. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, C.; Wang, Y.; Lin, H. Shear-related roughness classification and strength model of natural rock joint based on fuzzy comprehensive evaluation. Int. J. Rock Mech. Min. Sci. 2020, 137, 104550. [Google Scholar] [CrossRef]
- Fathipour-Azar, H. Data-driven estimation of joint roughness coefficient. J. Rock Mech. Geotech. Eng. 2021, 13, 10. [Google Scholar] [CrossRef]
- Shi, X.; Zhang, J.S.; Liu, P. Experimental research on shearing properties of the interface between red clay and concrete. J. Cent. South Univ. (Sci. Technol.) 2015, 46, 1826–1831. [Google Scholar]
- Hu, J.; Cui, H.; Yang, X. Experimental research on shearing properties of interface between silty clay and recycled concrete. Sci. Technol. Eng. 2019, 19, 231–235. [Google Scholar]
- Wan, X.S.; Zhou, H.; Lu, J.G. Experimental study on shear characteristics of saline frozen soil-concrete interface. J. Eng. Geol. 2023, 277. [Google Scholar]
- Xu, F.; Chen, L.; Zhao, C.Y. Experimental study on the interface shear behavior of clay and cement soil. Adv. Eng. Sci. 2021, 53, 110–117. [Google Scholar]
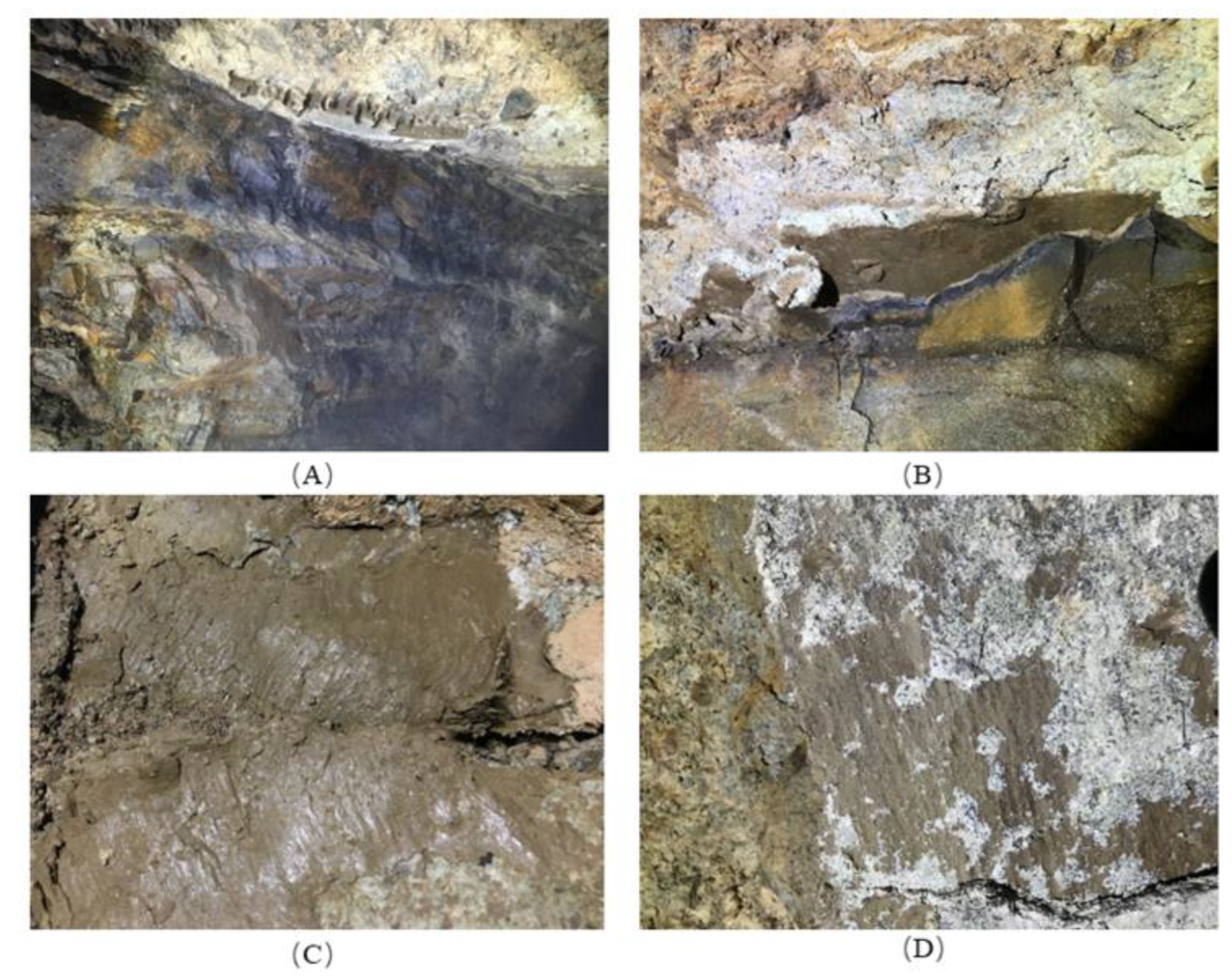
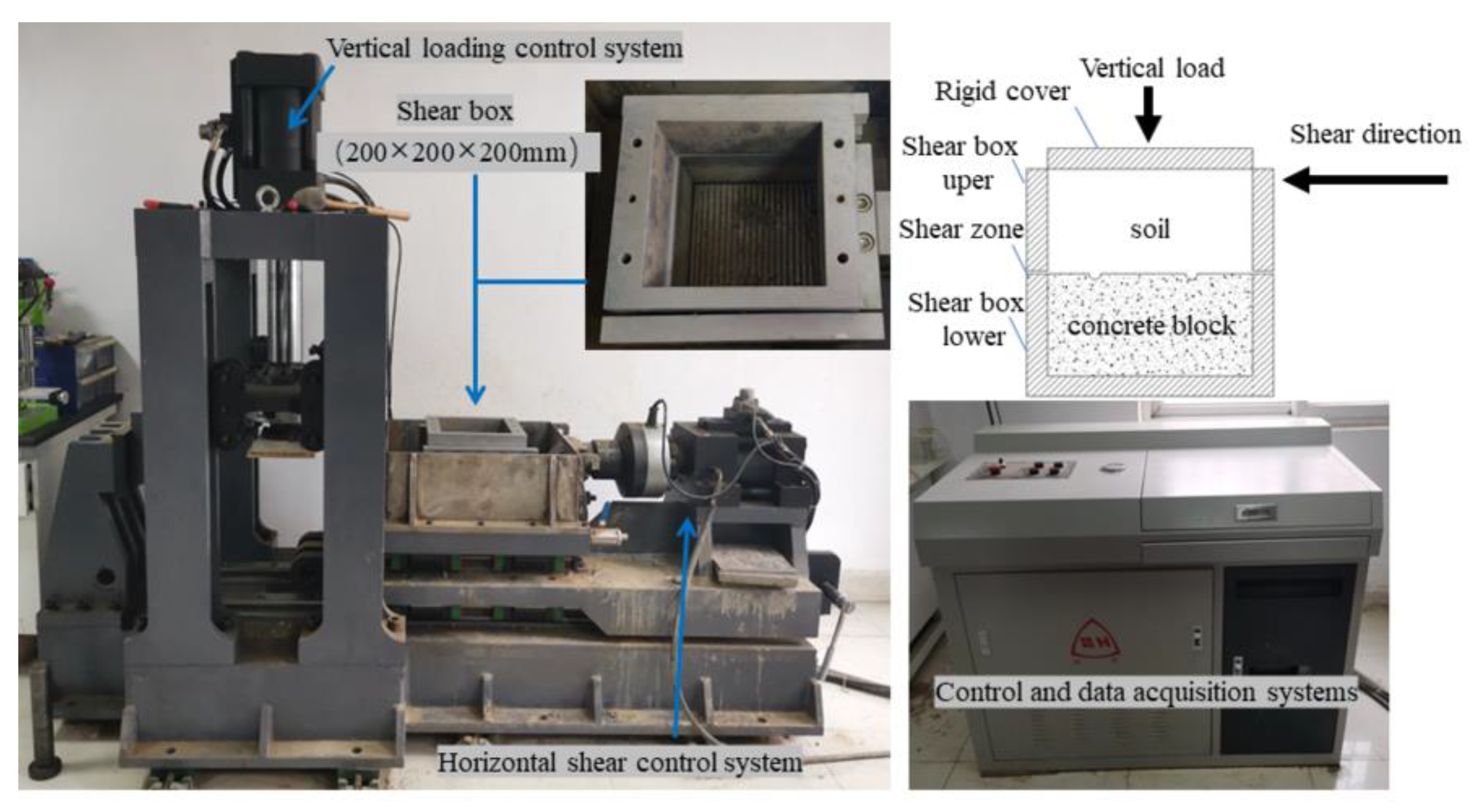





| Density | Dry Density | Natural Moisture Content | Plastic Limit | Liquid Limit | Plasticity |
|---|---|---|---|---|---|
| g/cm3 | g/cm3 | % | % | % | % |
| 2.01 | 1.70 | 17 | 16.4 | 37.6 | 21.2 |
| Sample Number | Contact Surface Roughness | ||
|---|---|---|---|
| n | Vi/mm3 | R/mm | |
| Ⅰ | 0 | 1130.4 | R1 = 0 |
| Ⅱ | 2 | 1130.4 | R2 = 5.652 |
| Ⅲ | 4 | 1130.4 | R3 = 11.304 |
| Ⅳ | 6 | 1130.4 | R4 = 16.956 |
| Ⅴ | 8 | 1130.4 | R5 = 22.608 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, M.; Zhang, J.; Xu, G. Study on Strength of Slip Soil–Tuff Contact Surfaces in Tuff Landslide Based on Model Test. Appl. Sci. 2024, 14, 1687. https://doi.org/10.3390/app14051687
Xue M, Zhang J, Xu G. Study on Strength of Slip Soil–Tuff Contact Surfaces in Tuff Landslide Based on Model Test. Applied Sciences. 2024; 14(5):1687. https://doi.org/10.3390/app14051687
Chicago/Turabian StyleXue, Mengqi, Junyu Zhang, and Guangli Xu. 2024. "Study on Strength of Slip Soil–Tuff Contact Surfaces in Tuff Landslide Based on Model Test" Applied Sciences 14, no. 5: 1687. https://doi.org/10.3390/app14051687
APA StyleXue, M., Zhang, J., & Xu, G. (2024). Study on Strength of Slip Soil–Tuff Contact Surfaces in Tuff Landslide Based on Model Test. Applied Sciences, 14(5), 1687. https://doi.org/10.3390/app14051687






