Influencing Factors of Shear Instability Characteristics of Rock Joints: Experimental and Theoretical Study
Abstract
:1. Introduction
2. Laboratory Tests and Results
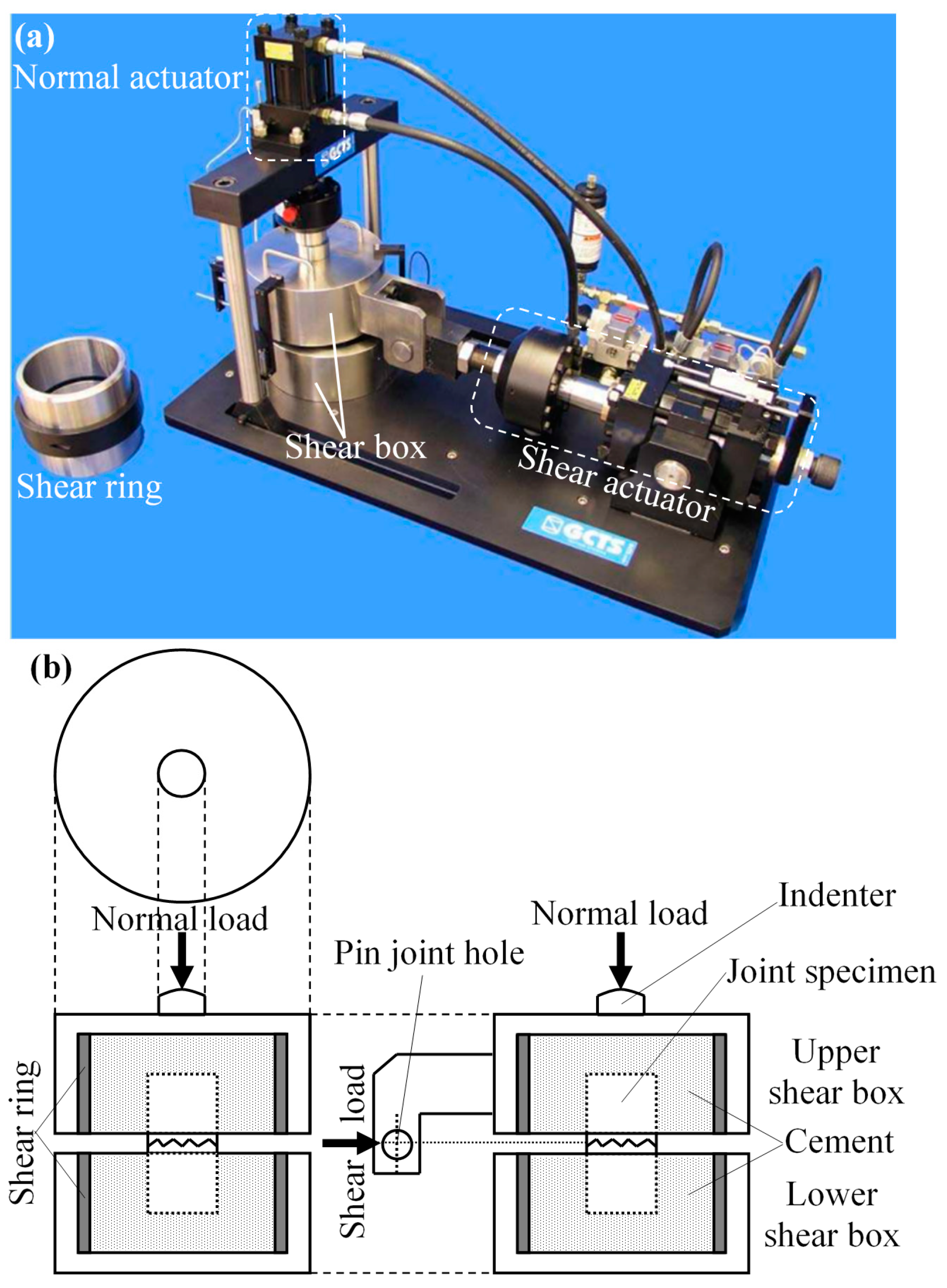
2.1. Experimental System
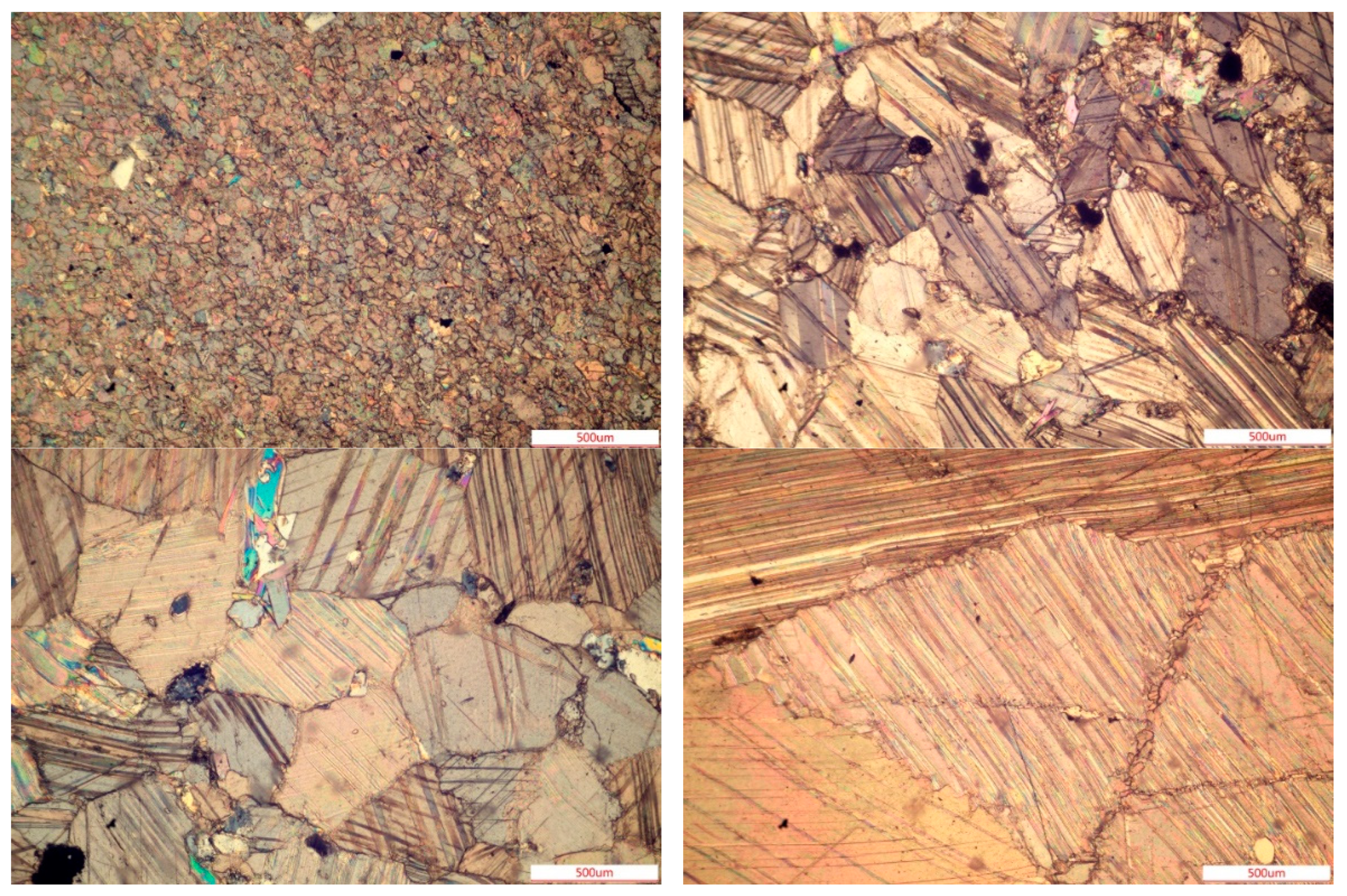
2.2. Sample Preparation
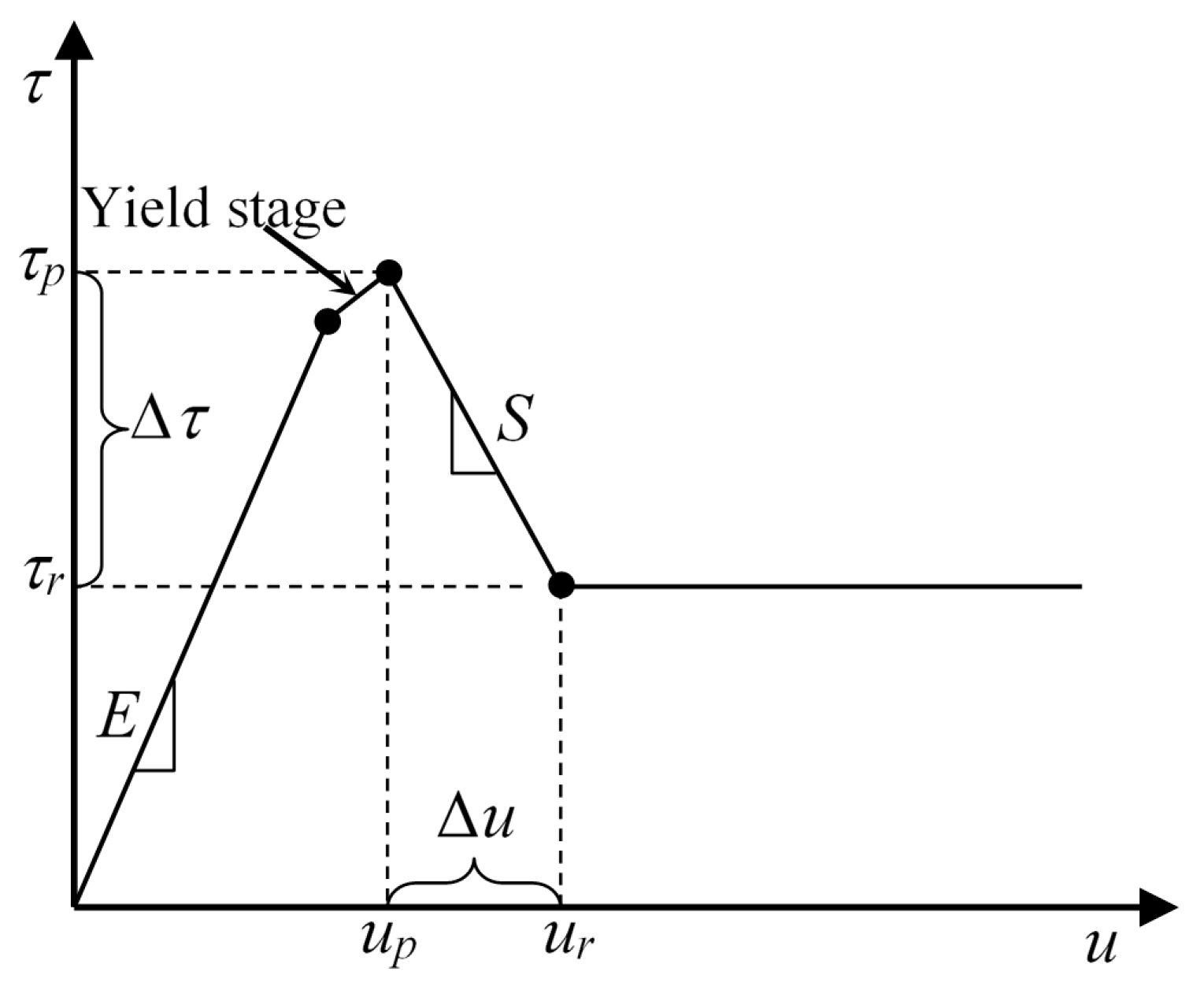
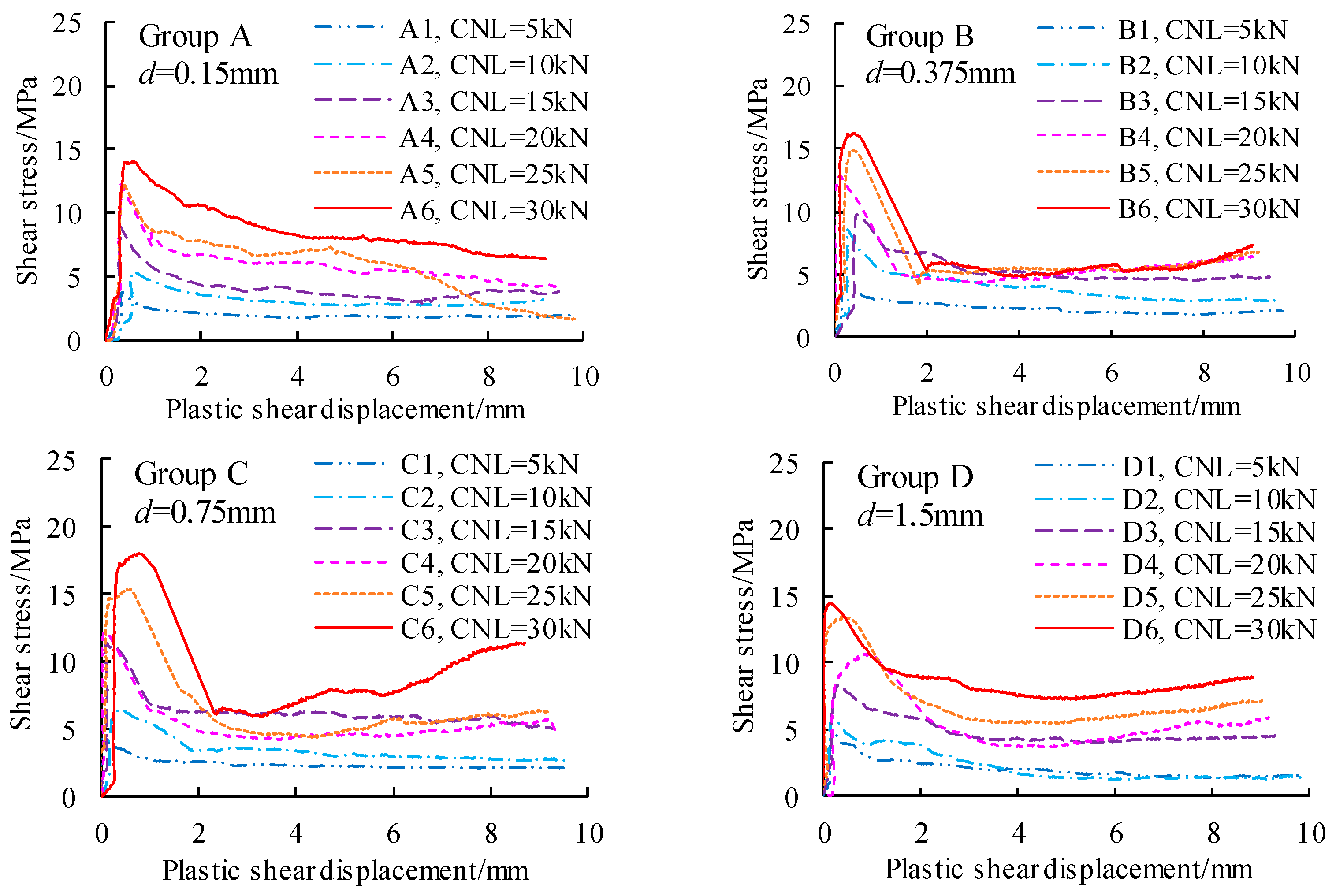
2.3. Experimental Scheme and Results
3. Analysis of Experimental Results
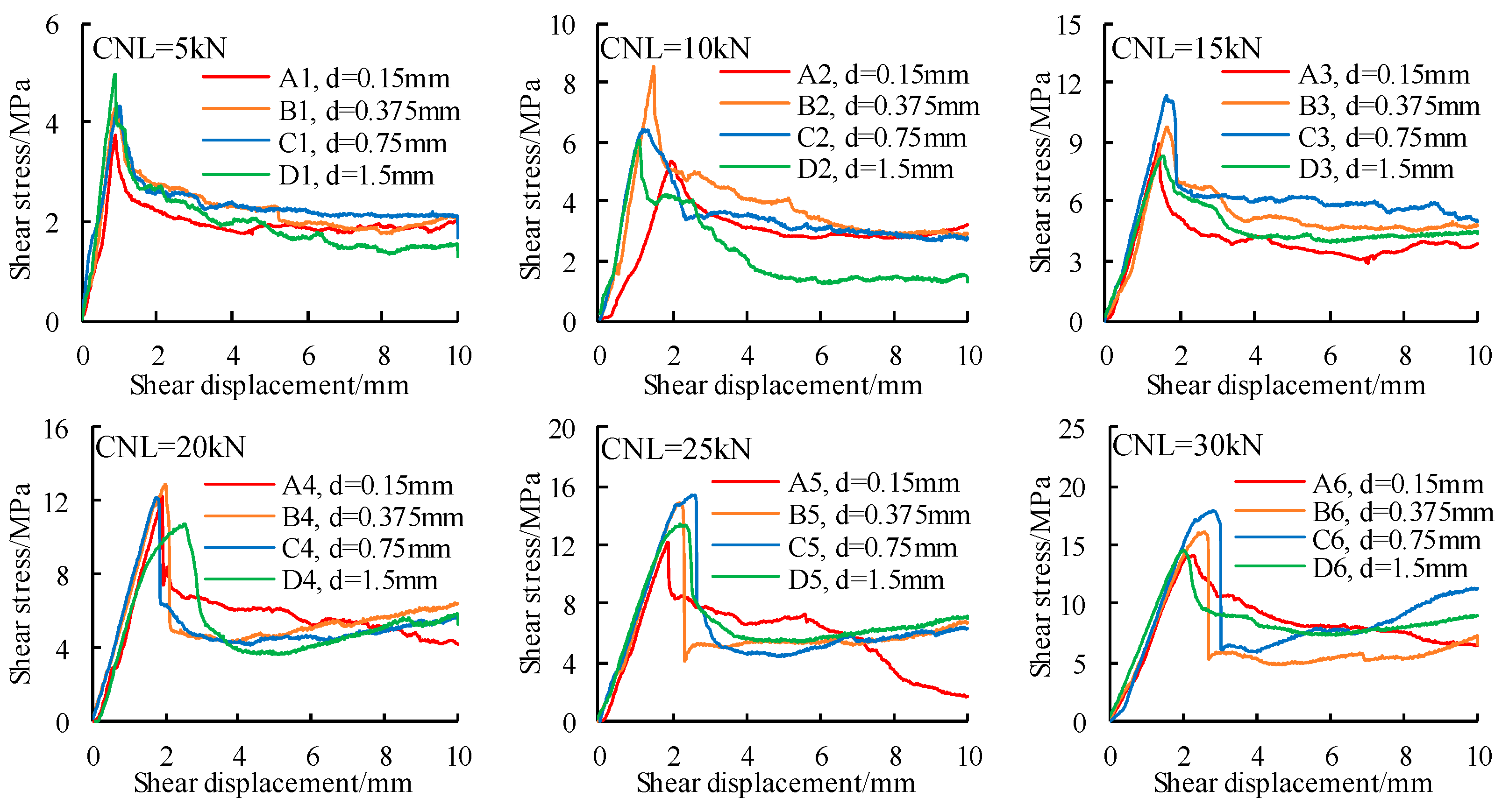
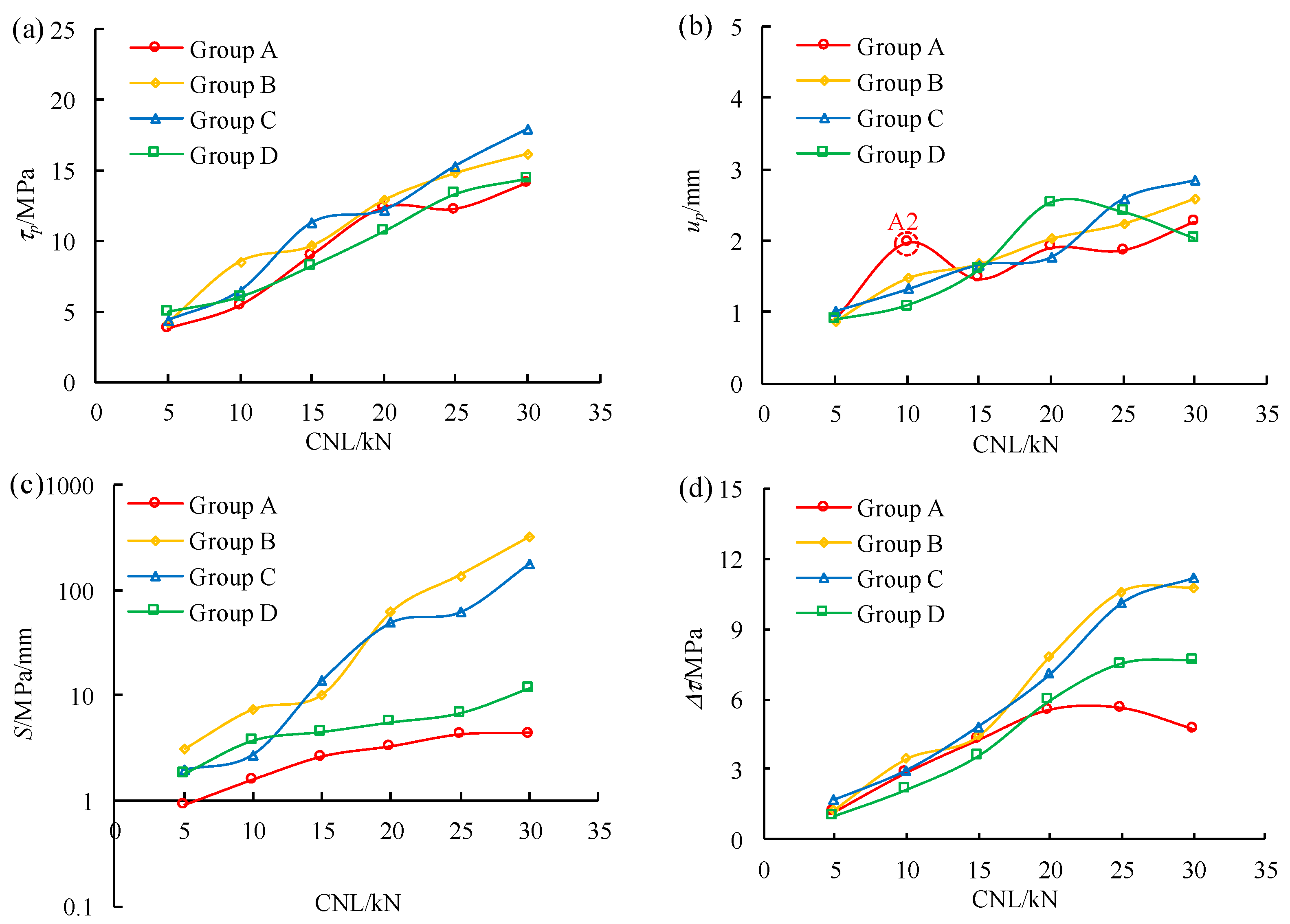
3.1. Influencing Factor of Grain Size (d)
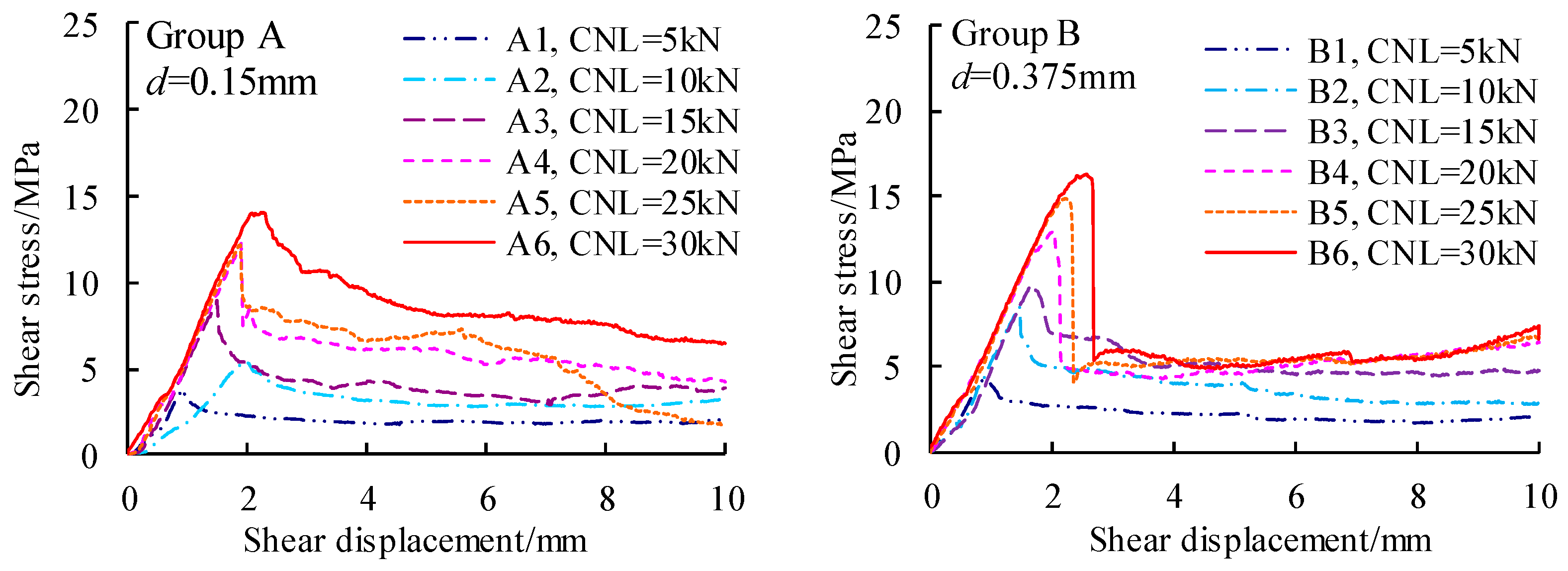
3.2. Influencing Factor of Normal Load (CNL)
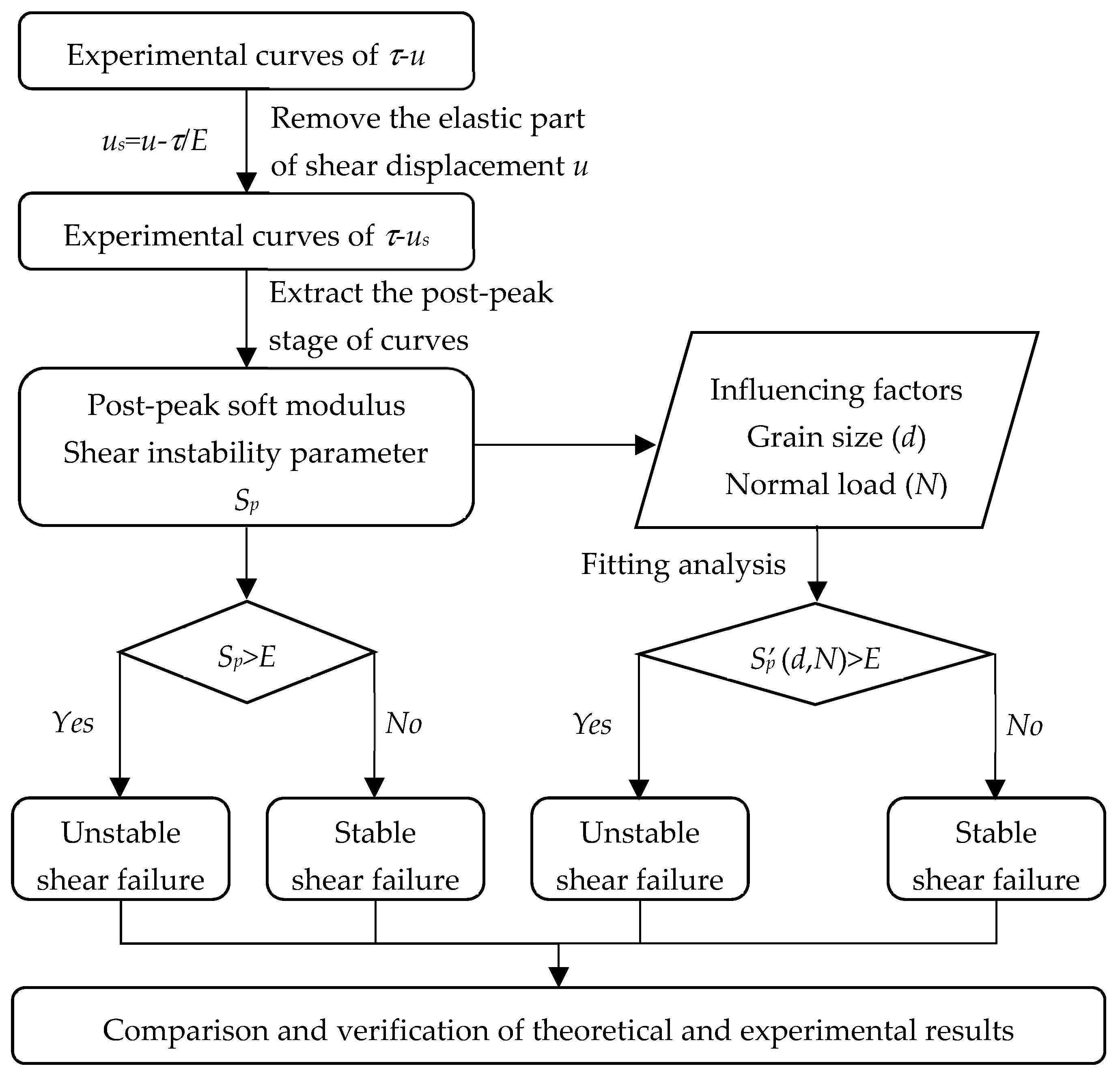
4. Shear Instability Analysis
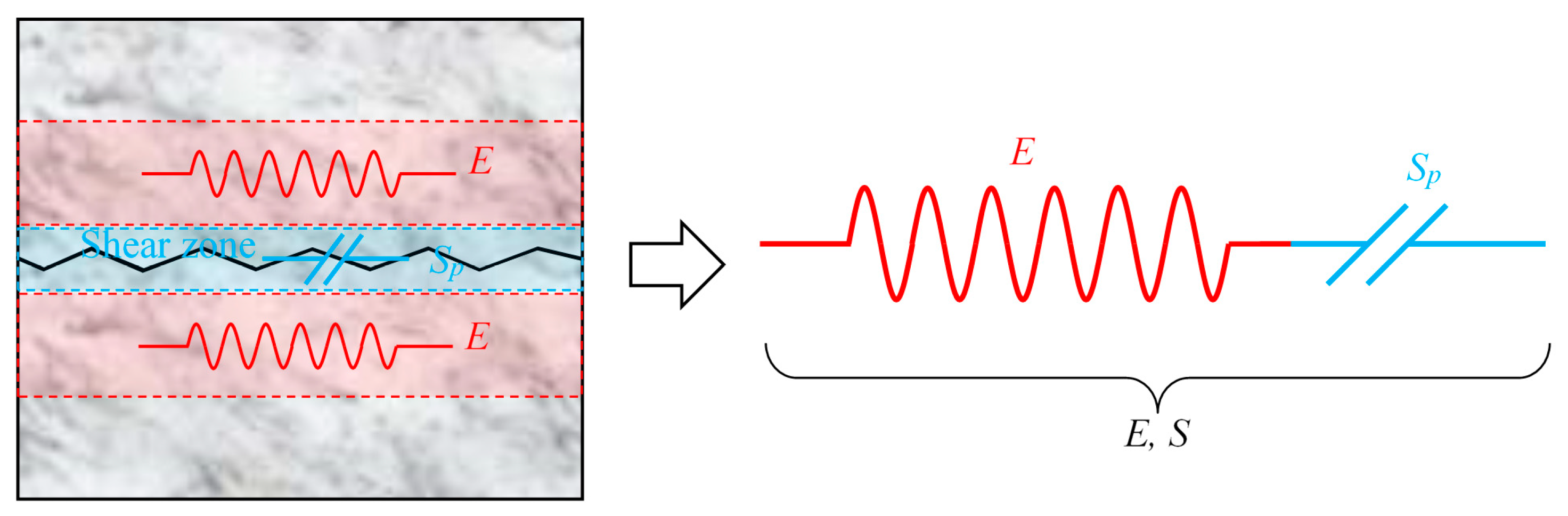
4.1. Shear Instability Mechanism
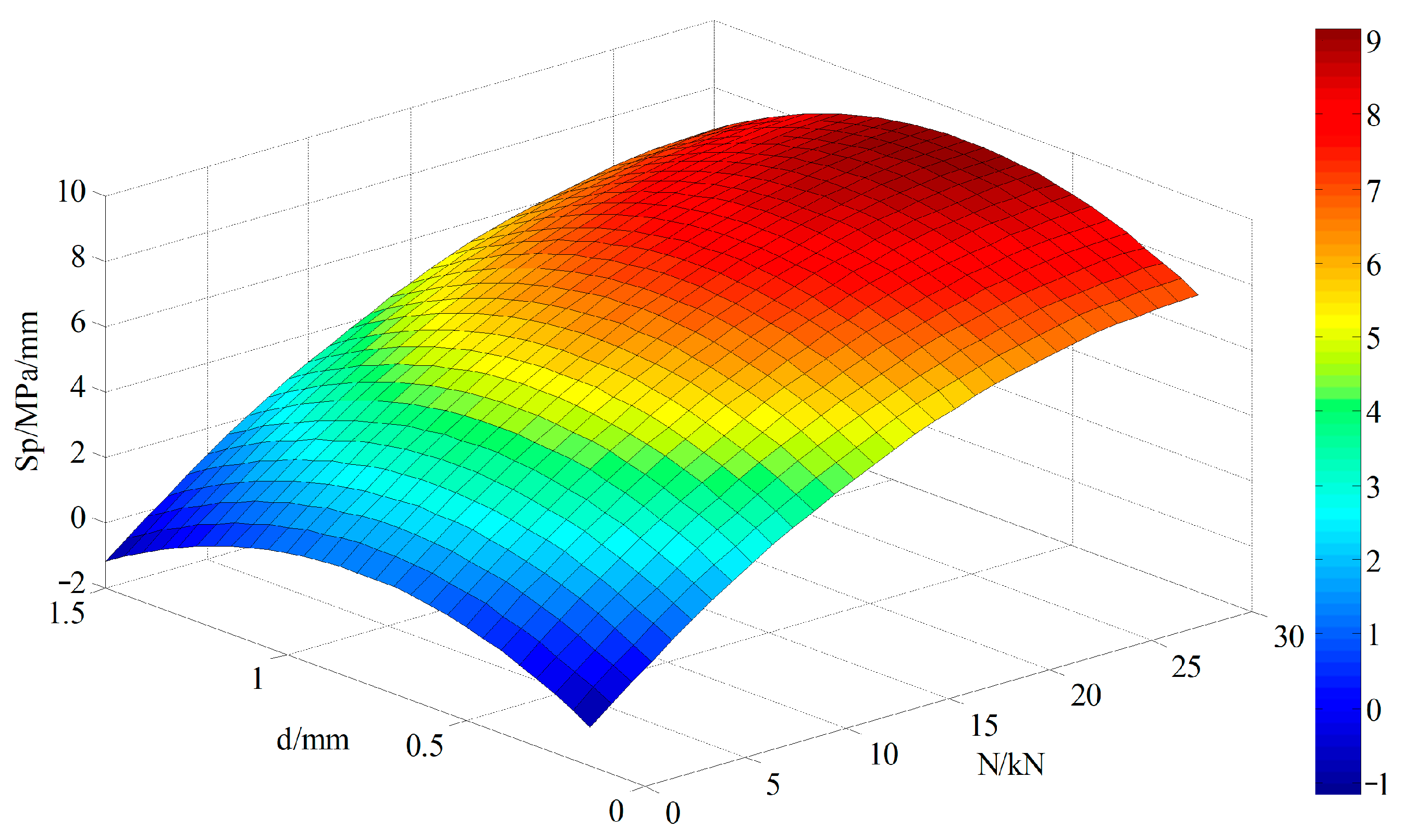
4.2. Shear Instability Model and Criterion
5. Conclusions
- The grain size (d) and normal load (CNL) have significant effects on the shear mechanical properties of rock joints, such as the peak shear strength (τp), peak shear displacement (up), post-peak modulus (S), and stress drop (Δτ). On the one hand, the τp, up, S, and Δτ of rock joints generally increase first and then decrease with the increase in grain size (d). On the other hand, the τp, up, S, and Δτ of rock joints generally increase with the increase in normal load (CNL).
- Marble specimens with a smaller grain size have a compact structure, fewer inherent cracks, and a higher proportion of dolomite with large Mohs hardness, which improves τp and up. Marble specimens with a larger grain size have a loose structure, fewer grain boundaries, and a higher proportion of calcite with lower Mhos hardness, resulting in decreases in shear stress dispersion, τp, and up. The mineral composition and microstructure have certain influences on the shear mechanical properties of rock joints.
- The established mechanical model of the shear instability of rock joints and the proposed shear instability parameter of the post-peak soften modulus () can be used to predict the possibility of the shear instability of marble joints. The shear instability behavior of marble joints is verified by comparing the empirical result () with the experimental result (Sp), that is, the rock joint specimens B4, B5, B6, C4, C5, and C6 are determined as shear instability.
- The proposed shear instability parameter (Sp) can describe the weakening behavior of the local damage shear zone, while S describes the average weakening behavior of the whole shear zone. The shear instability analysis proves that Sp is more suitable than S to describe the shear instability behavior of rock joints.
- This paper only studied the shear instability characteristics and influencing factors of marble joints (metamorphic rock), and the research on other types of rock is still insufficient. In future research, the shear instability characteristics and influencing factors of sandstone (sedimentary rock) and granite (igneous rock) joints will be studied to improve our understanding of the shear instability mechanism of different types of rock joints.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Meng, F.; Zhou, H.; Wang, Z.; Zhang, C.; Li, S.; Zhang, L.; Kong, L. Characteristics of Asperity Damage and Its Influence on the Shear Behavior of Granite Joints. Rock Mech. Rock Eng. 2017, 51, 429–449. [Google Scholar] [CrossRef]
- Li, B.; Gong, X.; Wang, G.; Qiao, J. Benchmark experiment on shear behavior of ice-filled planar rock joints using a novel direct shear testing apparatus. Int. J. Rock Mech. Min. Sci. 2024, 178, 105757. [Google Scholar] [CrossRef]
- Sarfarazi, V.; Karimi Javid, H.; Asgari, K. Study of rock pillar failure consisting of non-persistent joint using experimental test and fracture analysis code in two dimensions. J. Min. Environ. 2021, 12, 163–179. [Google Scholar] [CrossRef]
- Dong, H.; Zhu, W.; Niu, L.; Hou, C.; Liu, X. Numerical and theoretical study of load transfer behavior during cascading pillar failure. J. Rock Mech. Geotech. Eng. 2024, 16, 3014–3033. [Google Scholar] [CrossRef]
- Gu, Q.; Zhang, Q.; Dai, W.; Ye, S.; Li, T. Measurement of 3D joint surface morphology and estimation for shear mechanical behavior: A theoretical model and experimental investigation. Measurement 2024, 237, 115202. [Google Scholar] [CrossRef]
- Lindqvist, J.E.; Åkesson, U.; Malaga, K. Microstructure and functional properties of rock materials. Mater. Charact. 2007, 58, 1183–1188. [Google Scholar] [CrossRef]
- Zhang, X.; Jiang, Q.; Kulatilake, P.H.S.W.; Xiong, F.; Yao, C.; Tang, Z. Influence of Asperity Morphology on Failure Characteristics and Shear Strength Properties of Rock Joints under Direct Shear Tests. Int. J. Geomech. 2019, 19, 04018196. [Google Scholar] [CrossRef]
- Meng, F.; Wong, L.N.Y.; Zhou, H.; Wang, Z. Comparative study on dynamic shear behavior and failure mechanism of two types of granite joint. Eng. Geol. 2018, 245, 356–369. [Google Scholar] [CrossRef]
- Kabeya, K.K.; Legge, T.F.H. Relationship between grain size and some surface roughness parameters of rock joints. Int. J. Rock Mech. Min. Sci. 1997, 34, 146.e1–146.e15. [Google Scholar] [CrossRef]
- Zhang, T.; Yu, L.; Peng, Y.; Ju, M.; Yin, Q.; Wei, J.; Jia, S. Influence of grain size and basic element size on rock mechanical characteristics: Insights from grain-based numerical analysis. Bull. Eng. Geol. Environ. 2022, 81, 347. [Google Scholar] [CrossRef]
- Peng, J.; Wong, L.N.Y.; Teh, C.I. Influence of grain size on strength of polymineralic crystalline rock: New insights from DEM grain-based modeling. J. Rock Mech. Geotech. Eng. 2021, 13, 755–766. [Google Scholar] [CrossRef]
- Wang, C.; Elsworth, D.; Fang, Y.; Zhang, F. Influence of fracture roughness on shear strength, slip stability and permeability: A mechanistic analysis by three-dimensional digital rock modeling. J. Rock Mech. Geotech. Eng. 2020, 12, 720–731. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, L.; Asce, F.; Wang, W.; Liu, Q.; Tang, L.; Cheng, G. Experimental Study on Shear Behavior and a Revised Shear Strength Model for Infilled Rock Joints. Int. J. Geomech. 2020, 20, 04020141. [Google Scholar] [CrossRef]
- Shrivastava, A.K.; Rao, K.S. Physical Modeling of Shear Behavior of Infilled Rock Joints Under CNL and CNS Boundary Conditions. Rock Mech. Rock Eng. 2018, 51, 101–118. [Google Scholar] [CrossRef]
- Wang, W.; Yao, Q.; Tang, C.; Li, X.; Chong, Z.; Xu, Q. Experimental study on the shear characteristics and weakening mechanism of water-bearing rock joints. Bull. Eng. Geol. Environ. 2021, 80, 7653–7668. [Google Scholar] [CrossRef]
- Fan, H.; Liu, H.; Li, L.; Wang, X.; Tu, W.; Gao, J.; Yang, G. Weakening mechanism of shear strength of jointed rock mass considering the filling characteristics. Bull. Eng. Geol. Environ. 2024, 83, 224. [Google Scholar] [CrossRef]
- Meng, F.; Zhou, H.; Li, S.; Zhang, C.; Wang, Z.; Kong, L.; Zhang, L. Shear behaviour and acoustic emission characteristics of different joints under various stress levels. Rock Mech. Rock Eng. 2016, 49, 4919–4928. [Google Scholar] [CrossRef]
- Jia, B.-X.; Gao, Z.-X.; Han, X.-H.; Jin, J.-X.; Zhang, J.-J. Experimental analysis of the stick-slip characteristics of faults at different loading rates. PLoS ONE 2020, 15, e0231464. [Google Scholar] [CrossRef]
- Scuderi, M.M.; Collettini, C.; Viti, C.; Tinti, E.; Marone, C. Evolution of shear fabric in granular fault gouge from stable sliding to stick slip and implications for fault slip mode. Geology 2017, 45, 731–734. [Google Scholar] [CrossRef]
- Yuan, W.; Li, J.C.; Zou, C.J.; Zhao, J. A New Apparatus for Testing Shear-Slip Properties of Rock Joint Subjected to Dynamic Disturbance. Exp. Mech. 2024, 64, 745–759. [Google Scholar] [CrossRef]
- Gong, H.; Wang, G.; Luo, Y.; Liu, T.; Li, X.; Liu, X. Shear failure and mechanical behaviors of granite with discontinuous joints under dynamic disturbance: Laboratory tests and numerical simulation. Arch. Civ. Mech. Eng. 2023, 23, 171. [Google Scholar] [CrossRef]
- Kilgore, B.D.; McGarr, A.; Beeler, N.M.; Lockner, D.A. Earthquake Source Properties From Instrumented Laboratory Stick-Slip. In Fault Zone Dynamic Processes; American Geophysical Union: Washington, DC, USA, 2017; pp. 151–169. [Google Scholar]
- Gu, R.; Ozbay, U. Numerical investigation of unstable rock failure in underground mining condition. Comput. Geotech. 2015, 63, 171–182. [Google Scholar] [CrossRef]
- Zhang, B.; Zhang, S.; Shen, B.; Li, Y.; Song, S.; Han, X. Conducting Research to Identify Key Features and Critical Nodes in the Coalescence and Instability of Pre-Fabricated Jointed Rock. Appl. Sci. 2024, 14, 7905. [Google Scholar] [CrossRef]
- Tuğrul, A.; Zarif, I.H. Correlation of mineralogical and textural characteristics with engineering properties of selected granitic rocks from Turkey. Eng. Geol. 1999, 51, 303–317. [Google Scholar] [CrossRef]
- Dong, H.; Guo, B.; Li, Y.; Si, K.; Wang, L. Empirical formula of shear strength of rock fractures based on 3D morphology parameters. Geotech. Geol. Eng. 2017, 35, 1169–1183. [Google Scholar] [CrossRef]
- Dong, H.; Zhu, W.; Hou, C.; Liu, X. Load Transfer Behavior During Cascading Pillar Failure: An Experimental Study. Rock Mech. Rock Eng. 2022, 55, 1445–1460. [Google Scholar] [CrossRef]
- Dong, H.; Liu, X.; Zhu, W. Experimental and Theoretical Study of Shear Instability of Rock Joints in the Direct Shear Test. Int. J. Geomech. 2021, 21, 04021004. [Google Scholar] [CrossRef]





| Group | Grain Size Classification | Average Grain Size (mm) | Dol (%) | Cal (%) | Ep (%) | Sum (%) |
|---|---|---|---|---|---|---|
| A | Powder-grained | 0.15 | 72 | 26 | 2 | 100 |
| B | Fine-grained | 0.375 | 65 | 33 | 2 | 100 |
| C | Medium-grained | 0.75 | 35 | 64 | 1 | 100 |
| D | Coarse-grained | 1.5 | 26 | 74 | 0 | 100 |
| Group | UCS/MPa | Es/GPa | c/MPa | φ/° | ν | σt/MPa | Vp/m/s | JRC |
|---|---|---|---|---|---|---|---|---|
| A | 141.68 | 83.87 | 50.90 | 25.28 | 0.377 | 17.45 | 5854.56 | 9.07 |
| B | 70.93 | 47.45 | 25.79 | 25.28 | 0.316 | 17.30 | 5235.98 | 15.11 |
| C | 65.17 | 53.12 | 28.96 | 23.61 | 0.293 | 12.91 | 5270.34 | 15.66 |
| D | 48.38 | 41.88 | 15.04 | 30.02 | 0.119 | 10.30 | 5414.74 | 18.27 |
| CNL (kN) | 5 | 10 | 15 | 20 | 25 | 30 | |
|---|---|---|---|---|---|---|---|
| Grain Size (mm) | |||||||
| Group A: powder-grained 0.15 | A1 | A2 | A3 | A4 | A5 | A6 | |
| Group B: fine-grained 0.375 | B1 | B2 | B3 | B4 | B5 | B6 | |
| Group C: medium-grained 0.75 | C1 | C2 | C3 | C4 | C5 | C6 | |
| Group D: coarse-grained 1.5 | D1 | D2 | D3 | D4 | D5 | D6 | |
| No. | CNL/kN | d/mm | Sp/MPa/mm | /MPa/mm | E/MPa/mm | Experimental | Theoretical | Shear Instability Analysis |
|---|---|---|---|---|---|---|---|---|
| A1 | 5 | 0.15 | 1.11 | 1.79 | 6.9 | E > Sp | E > | No |
| B1 | 5 | 0.375 | 2.31 | 3.00 | 6.63 | E > Sp | E > | No |
| C1 | 5 | 0.75 | 1.7 | 3.88 | 4.86 | E > Sp | E > | No |
| D1 | 5 | 1.5 | 1.1 | 1.41 | 6.76 | E > Sp | E > | No |
| A2 | 10 | 0.15 | 1.43 | 3.73 | 3.91 | E > Sp | E > | No |
| B2 | 10 | 0.375 | 4.61 | 4.94 | 7.36 | E > Sp | E > | No |
| C2 | 10 | 0.75 | 3.06 | 5.82 | 7.07 | E > Sp | E > | No |
| D2 | 10 | 1.5 | 1.2 | 3.36 | 6.63 | E > Sp | E > | No |
| A3 | 15 | 0.15 | 3.24 | 5.25 | 7.32 | E > Sp | E > | No |
| B3 | 15 | 0.375 | 4.41 | 6.46 | 7.82 | E > Sp | E > | No |
| C3 | 15 | 0.75 | 5.6 | 7.34 | 7.56 | E > Sp | E > | No |
| D3 | 15 | 1.5 | 2.82 | 4.88 | 6.04 | E > Sp | E > | No |
| A4 | 20 | 0.15 | 5.46 | 6.35 | 7.79 | E > Sp | E > | No |
| B4 | 20 | 0.375 | 7.3 | 7.55 | 7.27 | E < Sp | E < | Yes |
| C4 | 20 | 0.75 | 7.55 | 8.44 | 7.47 | E < Sp | E < | Yes |
| D4 | 20 | 1.5 | 4.34 | 5.97 | 6.61 | E > Sp | E > | No |
| A5 | 25 | 0.15 | 6.28 | 7.02 | 7.36 | E > Sp | E > | No |
| B5 | 25 | 0.375 | 8.01 | 8.23 | 7.94 | E < Sp | E < | Yes |
| C5 | 25 | 0.75 | 7.65 | 9.11 | 7.5 | E < Sp | E < | Yes |
| D5 | 25 | 1.5 | 6.36 | 6.64 | 7.1 | E > Sp | E > | No |
| A6 | 30 | 0.15 | 3.3 | 7.27 | 7.61 | E > Sp | E > | No |
| B6 | 30 | 0.375 | 7.84 | 8.47 | 7.64 | E < Sp | E < | Yes |
| C6 | 30 | 0.75 | 8.7 | 9.36 | 7.63 | E < Sp | E < | Yes |
| D6 | 30 | 1.5 | 5.29 | 6.89 | 7.65 | E > Sp | E > | No |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, H.; Guo, B.; Ma, Q.; Si, K.; Wang, H. Influencing Factors of Shear Instability Characteristics of Rock Joints: Experimental and Theoretical Study. Appl. Sci. 2025, 15, 561. https://doi.org/10.3390/app15020561
Dong H, Guo B, Ma Q, Si K, Wang H. Influencing Factors of Shear Instability Characteristics of Rock Joints: Experimental and Theoretical Study. Applied Sciences. 2025; 15(2):561. https://doi.org/10.3390/app15020561
Chicago/Turabian StyleDong, Hangyu, Baohua Guo, Qing Ma, Kai Si, and Hongjian Wang. 2025. "Influencing Factors of Shear Instability Characteristics of Rock Joints: Experimental and Theoretical Study" Applied Sciences 15, no. 2: 561. https://doi.org/10.3390/app15020561
APA StyleDong, H., Guo, B., Ma, Q., Si, K., & Wang, H. (2025). Influencing Factors of Shear Instability Characteristics of Rock Joints: Experimental and Theoretical Study. Applied Sciences, 15(2), 561. https://doi.org/10.3390/app15020561






