Case Study on Response Characteristic of Surroundings Induced by a Covered Semi-Top-Down Excavation with Synchronous Construction of the Superstructure and Substructure

Featured Application

The purpose of this article is to explore the mechanical response characteristics and influencing factors of the structure inside the pit and the surrounding environment during the construction process.

Abstract

Relying on a foundation pit project leveraging the covered semi-top-down method with synchronous construction of the superstructure and substructure in Beijing, the whole process of construction was simulated by using ABAQUS finite-element software. The impact of the whole construction on the surrounding ground, the adjacent building, and the retaining structure were studied, and the influence of the existing building, the strength of diaphragm wall, and the construction process were carried out. As shown from the results, the foundation pit and the existing building are in a safe state during the whole construction process. The ground settlement shows an obvious groove shape. The deformation of the diaphragm wall has obvious spatial effects, which changes from “single peak” to “double peaks”. The maximum horizontal displacement of strata behind the diaphragm wall occurs at a depth of 22.5 m, which is 1.4–2.0 times the top horizontal displacement. The presence of existing buildings reduced the ground settlement between the buildings and the excavation surface. The construction process has little impact on the settlement of adjacent existing buildings, which can be adjusted appropriately.

1. Introduction

Excavation and construction of underground projects will inevitably cause disturbance to the surrounding ground, which in turn may have a series of adverse effects on adjacent buildings, and it has become a hotspot of concern in the engineering community. To date, many authors have already conducted vast amounts of studies on the issues. A database of 92 case histories with extensive excavation widths in sites with soft soil in China was collected and analyzed by Xiao et al.; they found that foundation pits have obvious spatial effects, and the lateral wall deflection, ground movement, and basal heave stability are closely related with the excavation width, but the type of retaining structure has little relevance to the excavation width of the pit [1]. Finno and Bryson investigated the effects of a three-story school supported by shallow foundations subjected to excavation of an adjacent 12.2 m deep soft clay foundation pit and summarized the inter-relationship between pit deformation and school structural damage [2]. Zheng et al. presented a simple assessment technique using a multivariate adaptive regression splines (MARSs) model, which could map the nonlinear interactions between the influencing factors and the maximum horizontal deformation of tunnels [3]. Korff et al. developed an analytical model to determine the pile response to lateral displacements, by relating the axial pile deformation to the vertical soil displacement resulting from the deep excavation [4]. Huang et al. presented a finite-element parametric study of tunnel behavior caused by nearby deep excavation and investigated the effects of several parameters [5]. Palmstrom and Stille found that the main features determining the behavior of the ground surrounding an excavation are the ground conditions (e.g., site stratum distribution, stress, and hydrogeological conditions) and the project-related features (e.g., size and shape of the opening and excavation method) [6], which means that the ground deformation is controlled by many factors such as the geological conditions of site, the construction environment, and the construction scheme and process. Therefore, using reasonable excavation methods can reduce the impact on the surroundings.

The top-down construction method, originated from the Toronto subway project in Canada in 1951, is one of the safest and most feasible methods in urban environments [7] which has gradually been widely employed in the construction of underground projects [8,9,10,11]. Many scholars have conducted in-depth research on the issues caused by top-down excavation. For example, Ou et al. studied the characteristics of movements of the diaphragm wall and the soil caused by excavation when using the top-down construction method [12]. Kung compared the excavation-induced wall deflection caused by the top-down method and the bottom-up method in Taipei silty clay, investigated the key factors affecting wall deflection, and found that the difference in the wall deflection induced by the creep effect between the two construction methods is quite insignificant [13]. Fateh et al. assessed the structural behavior of three casting stages of strut ring slab using finite-element simulation [14]. Wang et al. investigated the differential uplift and settlement between the inner column and diaphragm wall in top-down excavation [15]. Gui et al. examined the measured excavation responses when the pit was excavated by the top-down method, including the deflections of the diaphragm wall, vertical column movement, slab axial force, building settlement caused by ground deformation, and the influence of pit excavation on the adjacent subway tunnel [16]. Tan and Wang investigated the behaviors of the pit under top-down construction in Shanghai soft clay [17]. Jamsawang et al. studied the effectiveness of deep cement mixing walls created using the top-down construction technique for a deep excavation, and found that the top-down construction method is more effective than the bottom-up construction method in terms of the limitations of lateral wall movement, the bending moment in a deep cement mixing wall, and the thickness of a deep cement mixing wall for various depths because of a larger system stiffness [18]. Wang et al. simulated the construction process of the Zhongshan hospital project in Shanghai and found that the time effect of foundation pit deformation under top-down construction was particularly significant [19]. Chen et al. found that the top-down excavation can effectively minimize the ground and building settlement, while the excavation may induce uneven displacement of the interior supporting columns [20].

Although the top-down method has many merits, it is difficult to remove the soils below cast ground floors due to the restricted head space, and soil removal cannot start until the poured substructure components have cured, which means that the top-down method requires longer time to complete an excavation than the bottom-up method [21]. Meanwhile, due to an excavation under the slab having low efficiency and creating a difficult environment for work, the cost of the top-down method is higher than the bottom-up method. In response to some complex construction conditions and special technical and economic requirements, the covered semi-top-down method, which combines the traditional cover-cut method and the semi-top-down method, has gradually become a concern for the engineering community [22]. Huang et al. found that the semi-top-down method has a good effect on controlling the surrounding deformation [23]. Tan et al. investigated the performance characteristics of semi-top-down (STD) excavation for a subway station in Shanghai soft ground and found that spatial corner stiffening behavior was not apparent for this STD excavation because of the combined result of segmented construction procedure and narrow pit width, which is different from bottom-up and top-down excavations [24]. Compared with the top-down method, the covered semi-top-down method achieves flexible adjustment of the construction sequence of the structure inside the pit, which can save construction space, reduce construction costs, and improve efficiency. However, the mechanical characteristics of the surroundings under covered semi-top-down excavation with synchronous construction of the superstructure and substructure is still unclear, resulting in the need for an in-depth study.

To improve our understanding of the mechanical response characteristics of the structure inside the pit and the surrounding environment during the construction process of the STD method, this article reports on a project in Beijing, involving a systematic study of the synchronous construction of superstructures and underground structures under a covered semi-top-down construction method. The construction process of the project was simulated using the software ABAQUS^® 6.14, the mechanical response of the surroundings during the whole construction process were analyzed, and the interaction between the construction and the surroundings was explored. This paper is arranged as follows: the research status in this field is described in the introduction section; then, an overview of the engineering projects on which this study relies was introduced; afterwards, the numerical methods used in this study were described in detail; furthermore, after verifying the rationality of the model in this article, the numerical calculation results were discussed in detail, and the influence of some construction factors was also discussed. The results can provide reference for the subsequent construction and similar projects.

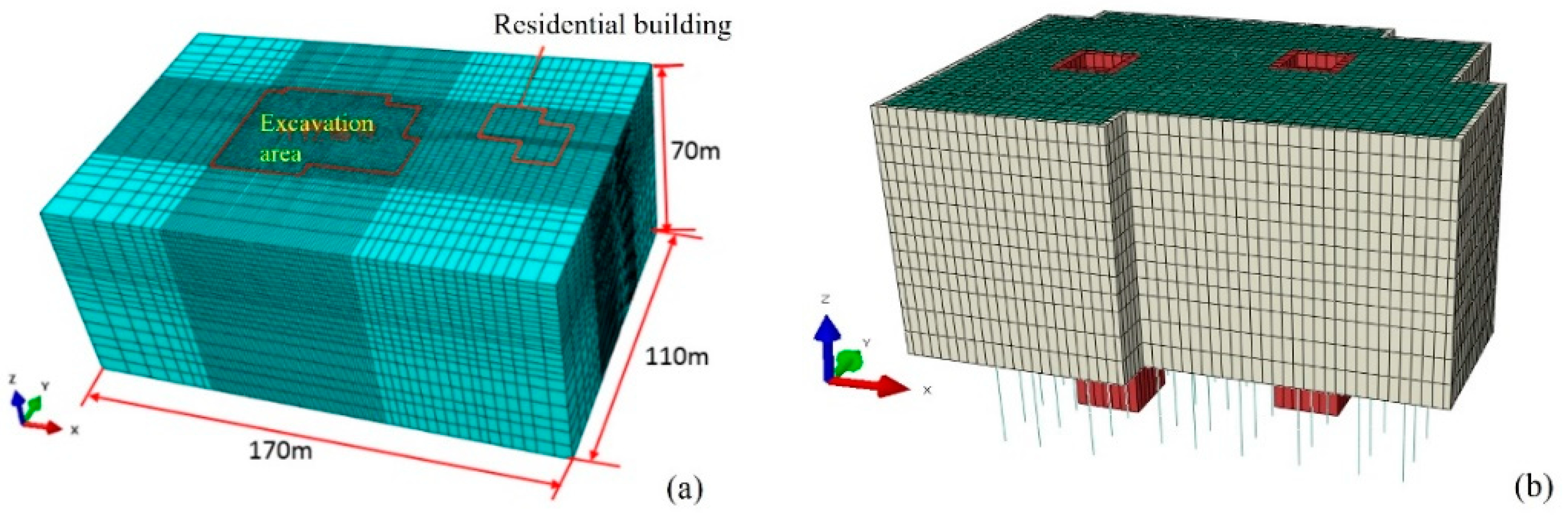
2. Project Overview

The project under study is for a shantytown renovation in Beijing; the surrounding environment is complex, with some existing buildings on the east side of the area and a subway station on the west side. The plots are planned to be 5 floors underground and 23 floors above ground, adopting the method of synchronous construction of the superstructure and substructure under a semi-top-down construction method. In this project, the foundation pit is 62.3 m east–west and 45.7 m north–south, and the excavation depth is set to be 25.5 m. The diaphragm walls with a depth of 36.5 m were used as the foundation pit retaining structures, and the vertical supports adopted integrated piles and columns. Figure 1 presents the relationship between the foundation pit and the existing buildings; the distance between them is 23.4 m and 32.1 m, respectively. Meanwhile, the monitoring points used to validate the numerical model in this article are also marked in Figure 1, where DB-1 and DB-2 are the two monitoring points of ground settlement, and JC-1 and JC-2 are used to monitor the building subsidence. For the convenience of analyzing the calculation results, the side walls of the pit are numbered clockwise according to I to VII. Correspondingly, the monitoring sections set perpendicular to them in the middle of each wall are numbered as I–I, II–II, III–III, IV–IV, V–V, VI–VI, and VII–VII.

According to the engineering geological survey results, the site strata from top to bottom are artificial fill, sandy silt–clay silt, medium sand, silty clay, pebble layer, silty clay layer, pebble layer, and silty clay layer.

Table 1. Mechanical properties of soil layers.

Soil	Thickness H (m)	Unit Weight γ (kN/m3)	Compression Modulus Es (MPa)	Poisson Ratio ν	Cohesive Force c (kPa)	Internal Friction Angle ϕ (°)
Artificial fill	2	17.9	3.7	0.30	5.0	10.0
Silt	7	19.1	8.5	0.30	31.6	14.3
Medium sand	13	20.7	40.0	0.24	0	40.0
Silty clay	2	19.9	15.7	0.30	31.2	14.7
Pebble	7	22.0	65.0	0.20	0	42.0
Silty clay	10	20.1	31.7	0.30	14.5	26.0
Pebble	17	22.0	75.0	0.20	0	42.0
Silty clay	12	19.8	35.4	0.30	15.4	26.7

lists the property parameters of the strata. There are two major groundwaters in the site area, namely phreatic water in the middle sand layer and interstitial water in the pebble layer. The groundwaters do not corrode the concrete structures or the reinforcing steels in the reinforced concrete, and the interstitial water is located about 3 m below the bottom of the foundation pit and has little effect on the project.

In the project, a method of synchronous construction of the superstructure and substructure was adopted, that is, the superstructure was constructed by the traditional bottom-up method, and the substructure was constructed simultaneously using a covered semi-top-down construction method, as shown in Figure 2. The construction processes are detailed as follows.

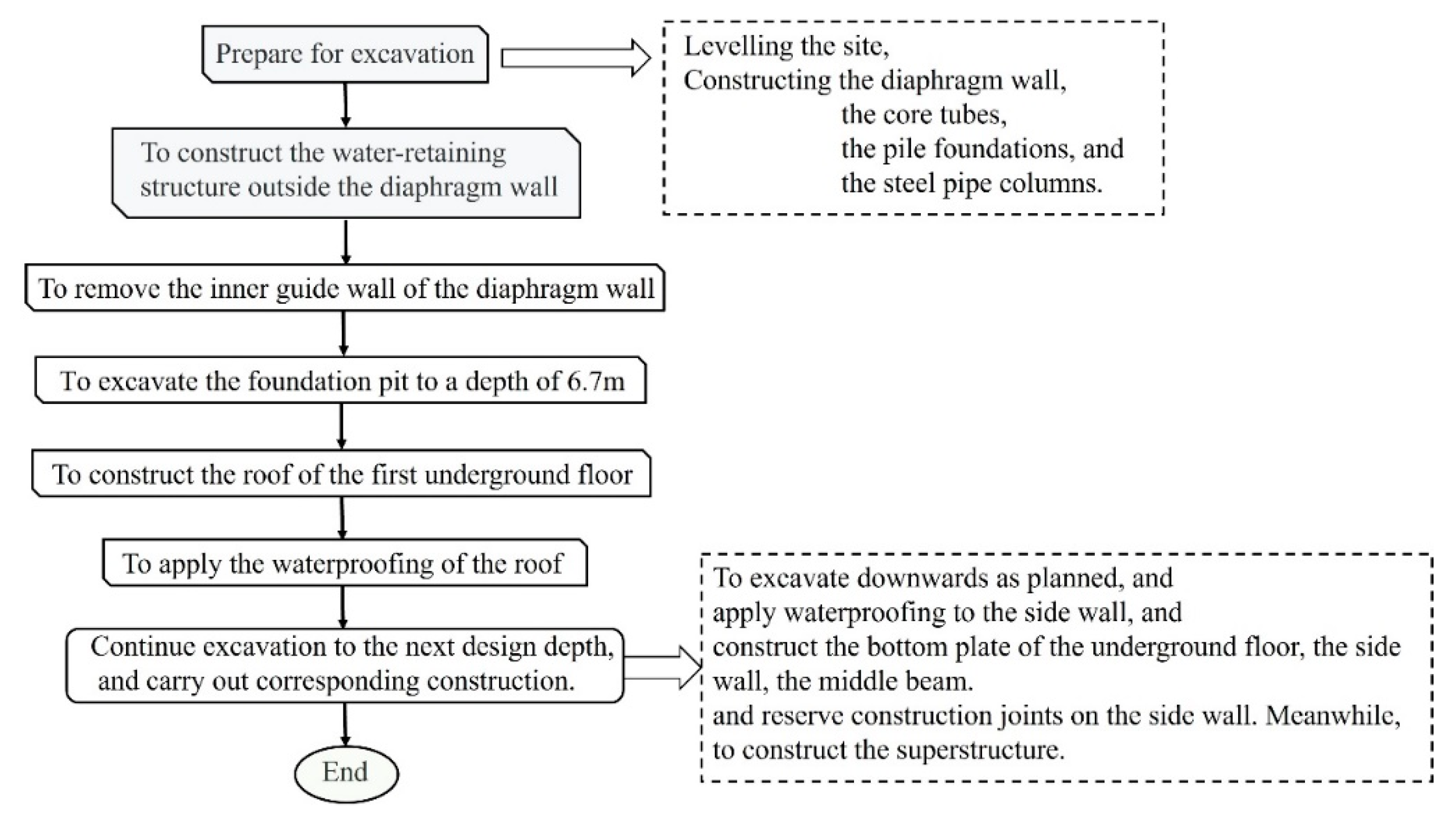
(1)

Prepare for excavation, including leveling the site, constructing the diaphragm wall, the core tubes, the pile foundations, and the steel pipe columns inside the pit;

(2)

To construct the water-retaining structure outside the diaphragm wall, remove the inner guide wall of the diaphragm wall, then excavate 6.7 m downwards and construct the roof of the first underground floor;

(3)

After the strength of the roof of the first underground floor reaches 80% of the design strength, the waterproofing of the roof will be applied. Then, continue to excavate 2.3 m downwards, apply waterproofing to the side wall, and construct the roof of the second underground floor, the side wall of the first underground floor, and the middle beam, following reserve construction joints on the side wall. Meanwhile, the construction of the superstructure will reach the 5th floor;

(4)

After the strength of the roof of the second underground floor reaches 75% of the design strength, continue to excavate 4.1 m downwards, apply waterproofing for the side wall of the second underground floor, and construct the roof of the third underground floor, the side wall of the second underground floor, and the middle beam, following reserve construction joints on the side wall. At the same time, the construction of the superstructure reaches the 8th floor;

(5)

After the strength of the roof of the third underground floor reaches 75% of the design strength, continue to excavate 3.6 m downward, apply waterproofing for the side wall of the third underground floor, and construct the roof of the fourth underground floor, the side wall of the third underground floor, and the middle beam, following reserve construction joints on the side wall. Meanwhile, construct the superstructure to the 10th floor;

(6)

After the strength of the roof of the fourth underground floor reaches 75% of the design strength, continue to excavate 4.0 m downward, apply waterproofing for the side wall of the fourth underground floor, and construct the roof of the fifth underground floor, the side wall of the fourth underground floor, and the middle beam, following reserve construction joints on the side wall. Meanwhile, construct the superstructure to the 15th floor;

(7)

After the strength of the roof of the fifth underground floor reaches 70% of the design strength, continue to excavate down to the design elevation of the bottom slab; manual excavation will be adopted when 200–300 mm away from the bottom of the pit. Construct the bottom slab, apply waterproofing for the side wall of the fifth underground floor, and construct the side wall of the fifth underground floor and the sill, following reserve construction joints on the side wall. After the completion of the substructure construction, continue to complete the construction of the superstructure from the 16th to the 23rd floors.

3. Methodology

The ABAQUS software was used to simulate the whole construction process. The shape of the foundation pit in this project is complex and irregular, so the size of numerical model was determined based on the maximum size of the foundation pit in each direction, which was 62.3 m in the east–west direction, 45.7 m in the north–south direction, and 25.5 m in the excavation depth. For eliminating the effects of the model boundaries on the calculation results, the model calculative height (in the z-direction), length (in the x-direction), and width (in the y-direction) were set to be 70.0 m, 170.0 m, and 110.0 m, respectively, and the boundary conditions of the model were set as follows: the top surface was free, while the bottom surface was fixed by constraining all the degrees of freedom of the nodes on it. The nodes on the side surface were allowed to have vertical displacement, but their normal displacements were frozen.

The finite-element model for the excavation area and surrounding site is shown in Figure 3a,b which present the structure of the excavation area.

In the finite-element model, the soil, the diaphragm wall, and the core tubes were modeled using the solid elements. The 2D plate elements were used to simulate the behavior of the slabs and the side walls. The piles and columns were simulated with the 1D beam elements. In this study, the Mohr–Coulomb model was chosen to describe the stress–strain relationship of the soils. The modulus of deformation used was calculated from Equation (1) using the parameters in Table 1 according to the literature [25].

$$E_0=\beta \times E_s$$

(1)

where β = 1 − 2υK₀ = (1 + υ)(1 − 2υ)(1 − υ), E₀ is the modulus of deformation, E_s is the compression modulus, and υ is the Poisson ratio.

In the practical engineering, the materials for the diaphragm wall, core tubes, slabs, side walls, piles are all reinforced concrete, and the columns are made of steel tube concrete. Considering that the possibility of plastic deformation of structural components under normal excavation construction is very small, and for the convenience of calculation, material equivalence was performed on the diaphragm wall, core tubes, slabs, side walls, piles, and columns in this study, all of which were assumed to be elastic components. According to the engineering design scheme, the material parameters of the structure components during the excavation process are shown in

Table 2. Material parameters of structure components.

Type	Thickness or Diameter t or d (m)	Elastic Modulus E (MPa)	Poisson Ratio ν	Unit Weight γ (kN/m3)
Piles	1.50	32,500	0.2	23.4
Columns	0.80	45,000	0.2	24.5
The diaphragm wall	0.80	30,000	0.3	23.4
Core tubes	0.80	37,000	0.2	24.0
The roof of the 1st underground floor	0.50	34,500	0.2	23.7
The roof of the 2nd to the 4th underground floor	0.25	32,500	0.2	23.5
The roof of the 5th underground floor	0.60	32,500	0.2	23.5
The bottom slab of the 5th underground floor	1.50	32,500	0.2	23.5
The side wall	0.70	37,000	0.2	24.0

.

Due to the lack of relevant information on the surrounding buildings, this study only analyzes the influence of loads of the adjacent existing buildings, by applying the weight of existing buildings to the corresponding ground surface in the form of loads. The loads of the existing buildings and that of the new superstructure constructed were all taken as 20 kPa per story. Since the project construction area was far from the subway tunnel, the effect of the subway tunnel was ignored in this study, while the effect of groundwater was also not considered.

Given that it is difficult to achieve ground stress equilibrium when existing buildings are simulated in the form of loads. In this study, ground stress equilibrium was performed only for the initial geological condition, and then the influence of existing buildings was eliminated by phase subtraction to achieve the zeroing of the initial displacement field of the model before the construction of the foundation pit. According to the actual construction situation, the numerical simulation process is listed in

Table 3. Numerical simulation of synchronous construction processes.

Process	Clarification of the Status
1	Initial ground stress equilibrium
2	Apply the existing buildings loads
3	Construct the diaphragm wall, the core tubes, the piles, and the steel pipe columns
4	Excavate 6.7 m downwards and construct the roof of the 1st underground floor
5	Excavate 2.3 m downwards, construct the bottom slab and the side wall of the 1st underground floor, and construct the superstructure to the 5th floor
6	Excavate 4.1 m downwards, construct the bottom slab and the side wall of the 2nd underground floor, and construct the superstructure to the 8th floor
7	Excavate 3.6 m downwards, construct the bottom slab and the side wall of the 3rd underground floor, and construct the superstructure to the 10th floor
8	Excavate 4.0 m downwards, construct the bottom slab and the side wall of the 4th underground floor, and construct the superstructure to the 15th floor
9	Excavate 4.8 m downwards and construct the bottom slab and the side wall of the 5th underground floor
10	Construct the superstructure from the 16th to the 23rd floor

. The construction process was realized by deactivating and reactivating elements in ABAQUS software.

4. Results

4.1. Validation of the Numerical Model

In order to simplify the work, the correctness of the numerical model is verified only by the measured data from the start of construction to the completion of the excavation of the 2nd underground floor.

Figure 4 shows the measured ground settlements and the numerical simulation results at the locations of the monitoring points DB-1 and DB-2. It can be seen that the measured data are consistent with the simulation results. In addition, Figure 5 gives the settlement history curves of existing buildings, indicating that the simulation results also match the measured data for building settlement. From Figure 4 and Figure 5, it can be concluded that the numerical model is reasonable, and the numerical model will be used to analyze the whole construction process.

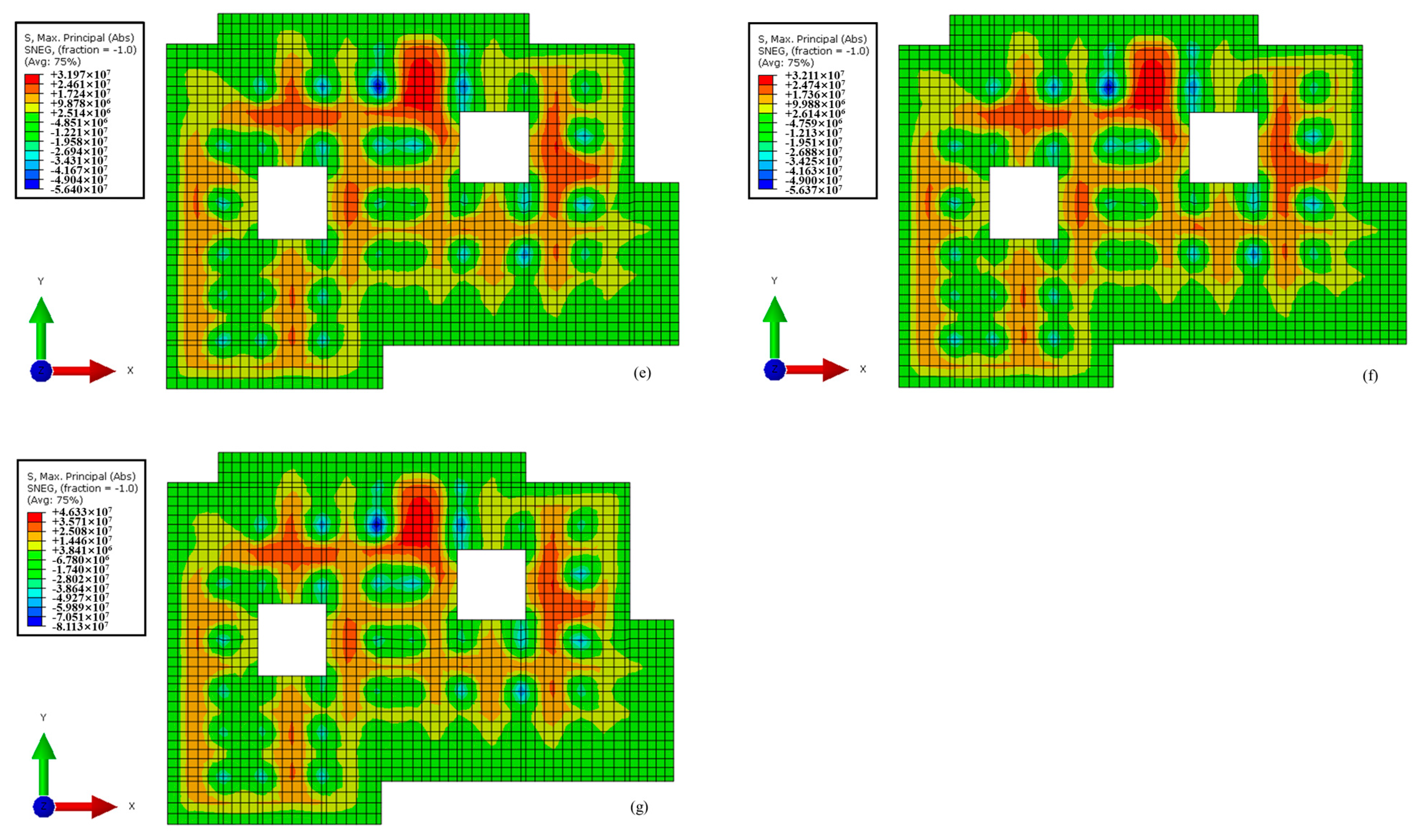
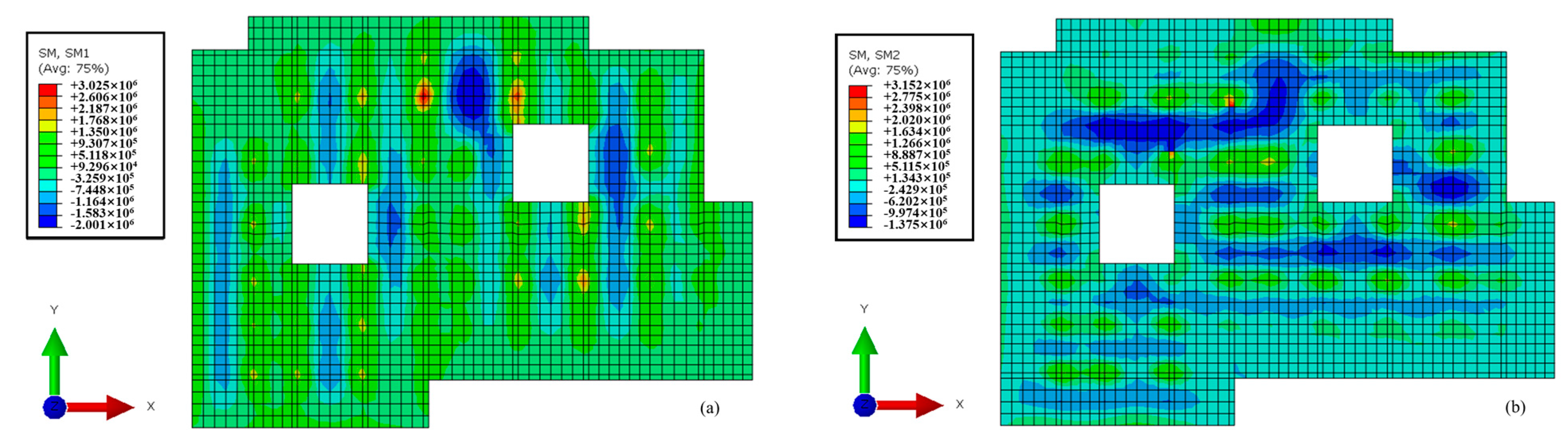
4.2. Structural Response Inside the Pit

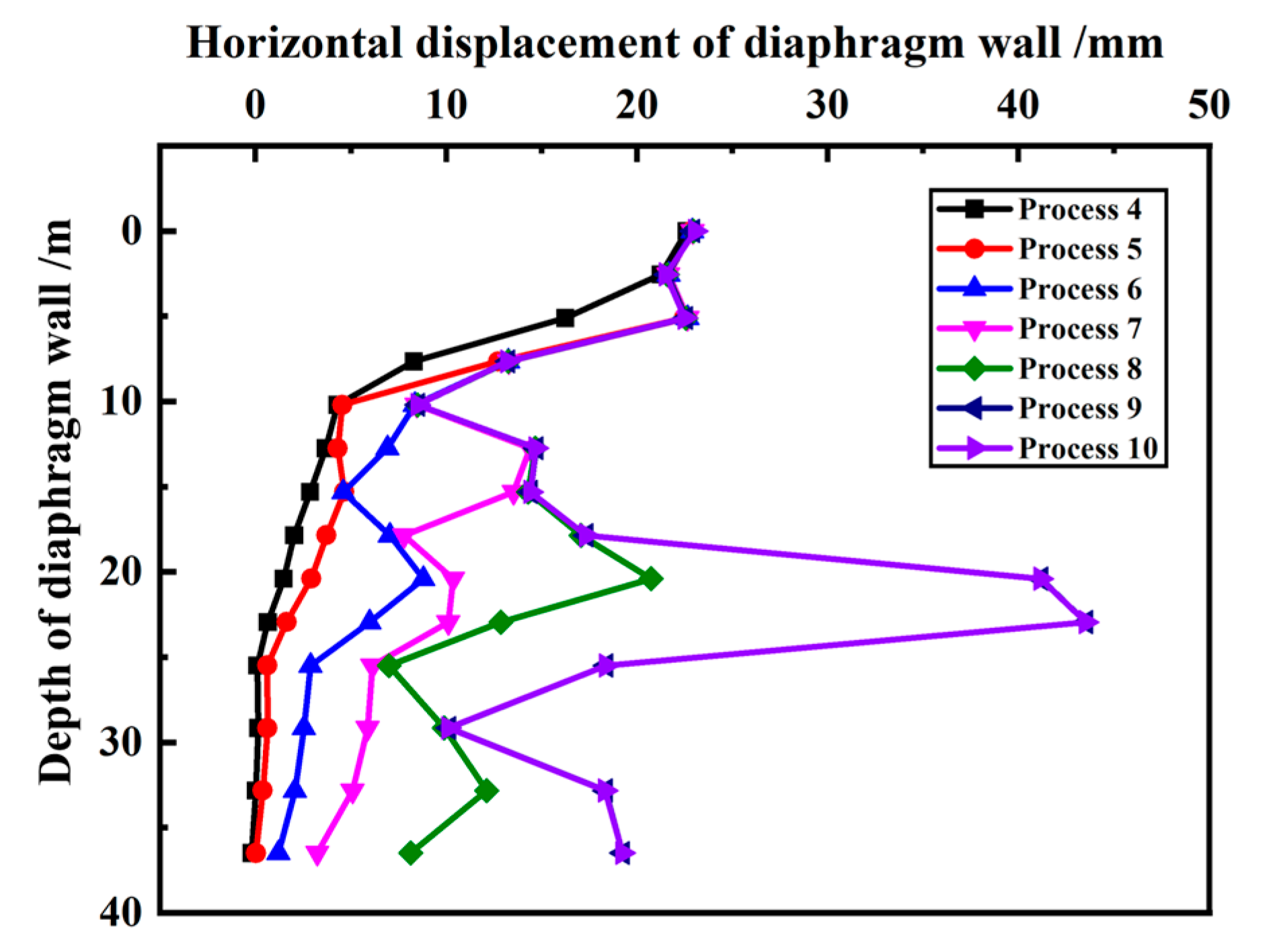
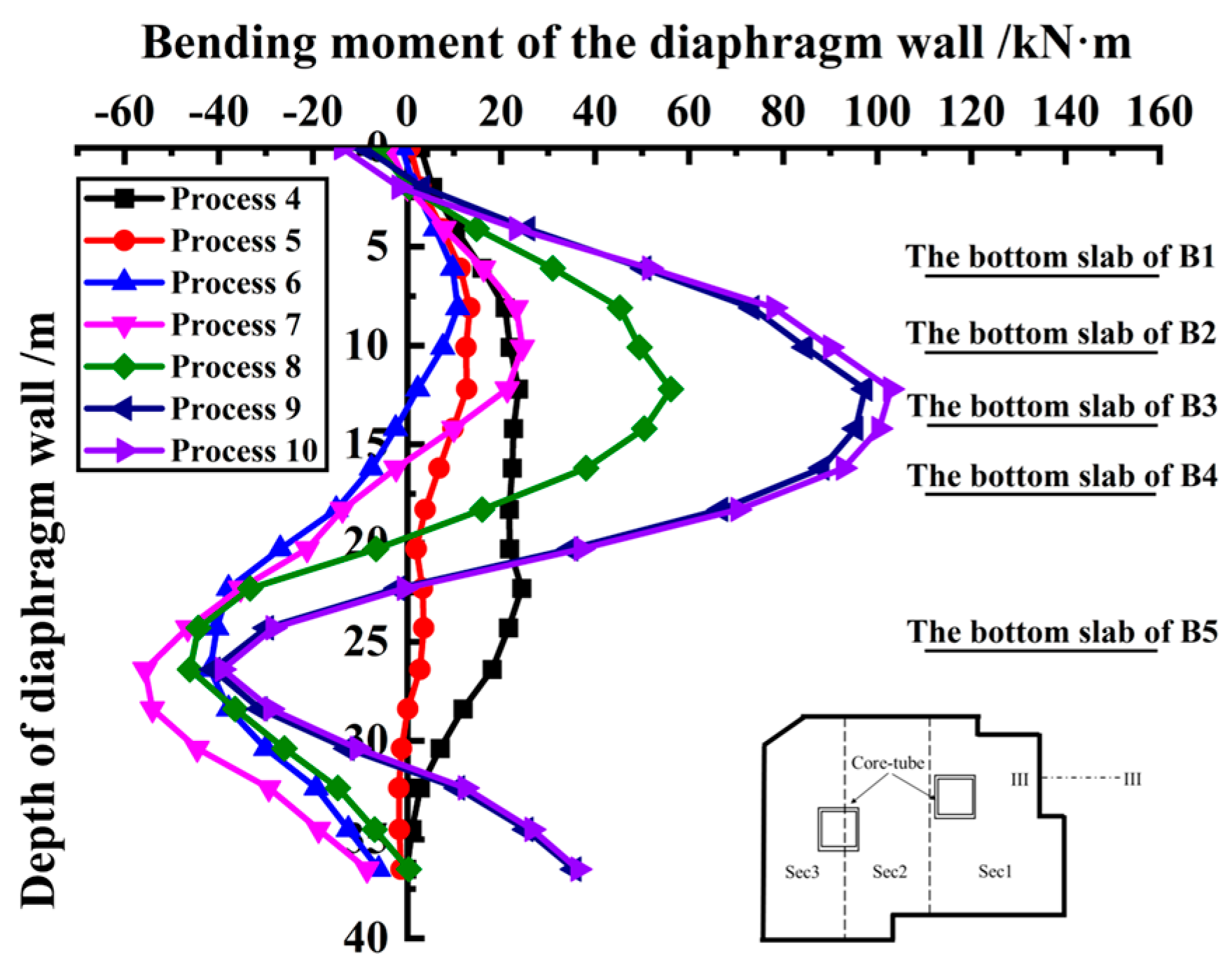
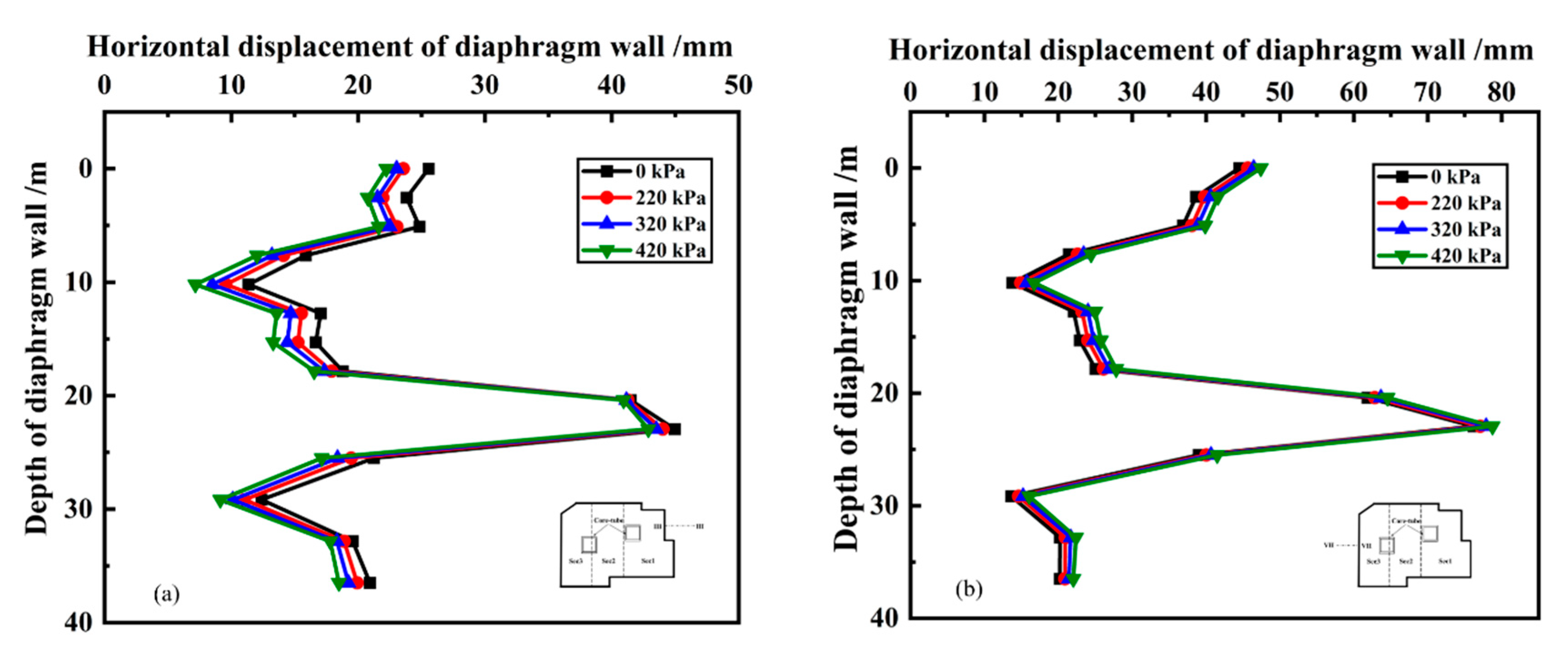
4.2.1. Diaphragm Wall

Figure 6 depicts the horizontal displacement contour maps of the diaphragm wall after construction. As shown in Figure 6, the diaphragm walls show a deformation pointing into the pit, and the deformation distribution has obvious spatial effects. The displacement corresponding to the long side is greater than that corresponding to the short side, and the sudden change in shape of the local position of the foundation pit causes a reduction in the maximum horizontal deformation of the retaining structure. However, since the roof of the first underground floor is built after the excavation of the first underground floor is completed, the top of the diaphragm wall shows a large deformation, which is different from the small deformation of the top of the diaphragm wall adopting the covered top-down method [17,26].

Figure 7 shows the horizontal displacement distribution of the diaphragm wall at the monitoring section III–III for different construction processes. It can be found that the top deformation of diaphragm wall is larger than others at the end of process 4, which is different from the deformation trend of the conventional covered top-down method [17,26]. This may be owing to the fact that the construction of the pit is equivalent to a cantilever-supported excavation during process 4, resulting in a large deformation at the top of diaphragm wall, whereas when the subsequent processes are carried out, the top of the diaphragm wall is completely constrained, and the horizontal displacement is no longer increasing following the completion of the roof of the first underground floor. During process 5, the position where the maximum deformation occurs shifts to 6.0–7.0 m below the ground level. In the subsequent construction, the floor slab plays a great supporting role, and the deformation value at this portion remains almost unchanged. But the position of maximum deformation gradually moves downwards as construction continues, the horizontal deformation distribution along the depth changes from “single peak” to “double peaks”, and the peak value of displacement of the lower part appears at a depth of 22.5 m below the ground (about 88% of the excavation depth of the pit). With the subsequent excavation, the position of the maximum horizontal displacement of the diaphragm wall does not change, but its displacement value is increasing. In process 10, only the superstructure was constructed, which has little impact on the horizontal displacement of the diaphragm wall (as shown in Figure 7, the curve of process 10 almost completely overlaps with that of process 9), and the maximum horizontal displacement still appears at a depth of 22.5 m after the whole construction. Therefore, more attention should be paid to the deformation of this position of 22.5 m below the ground (about 88% of the excavation depth of pit) during construction to avoid damage to the diaphragm wall, and if necessary, additional support can be adopted to reduce the deformation of this location. Additionally, as the design depth of the diaphragm wall is 36.5 m, the bottom of the diaphragm wall is closer to the bottom slab of the 5th underground floor (only 11.0 m). With the progress of construction, the constraint of stratum on the bottom of the diaphragm wall gradually decreases, resulting in a larger deformation at the bottom of the diaphragm wall.

Figure 8 summarizes the final monitoring horizontal displacement curves of the diaphragm wall at different monitoring sections after construction, from which it can be seen that the horizontal deformation of the diaphragm wall points to the foundation pit, and the horizontal displacement distribution presents a “double peak” shape. With the increase in the horizontal extension length of the diaphragm wall, the horizontal displacement increases, but the deformation shape of wall is similar, and the maximum horizontal displacement occurs 22.5 m below the ground. The horizontal displacement of the top and that of the bottom are significantly affected by the horizontal extension length of the diaphragm wall. When the extension length is short, the horizontal displacement of the top is almost the same as that of the bottom. When the extension length is large, the horizontal displacement of the top is greater than that of the bottom.

Figure 9 presents the variation in the bending moment of the diaphragm wall at the monitoring section III–III for different construction processes, in which a positive value indicates the deformation of the wall towards the pit due to the compression of the soil mass, and a negative value indicates the deformation of the wall towards the outside of the pit. From Figure 9, it can be concluded that the bending moment at the top of diaphragm wall changes very little (within 20 kN·m) throughout the construction process. The construction processes 4 and 5 cause little change in the bending moment of diaphragm wall, but from process 6 onwards, a large negative bending moment occurs at about 26.4 m at the bottom. It might be due to the deformation of the diaphragm wall pointing to the foundation pit caused by excavation, while the pebble layer at 26.4 m below the ground has a certain inhibitory effect on the deformation of the diaphragm wall, resulting in a large negative bending moment. With the subsequent construction, the maximum positive bending moment of the diaphragm wall gradually increases, and its position gradually moves down, finally stabilizing near the middle of the excavation pit. The maximum positive bending moment of the diaphragm wall reaches 102 kN·m when the construction is finished. The maximum negative bending moment tends to increase then decrease following the construction, which is caused by the floor construction and the roof support, but the position of the maximum negative bending moment does not change with the construction. As shown in Figure 9, process 10, a process of constructing the superstructure above the pit, only leads to an increase of about 5.7% in the maximum positive bending moment of diaphragm wall and has no effect on the maximum negative bending moment. The effect of process 10 on the horizontal deformation of the diaphragm wall can be almost negligible.

4.2.2. Core Tubes

The core tube is used to set up the elevator, stairs, ventilation shafts, and cable shafts. The underground part of the core tubes is completed before excavation of the foundation pit. Figure 10 shows the horizontal displacement contour maps of the twin core tubes in the X- and Y-directions when the construction is finished, from which it can be seen that in the X-direction, the deformation of the core tubes mainly concentrates at the depth of the pit bottom. The deformation directions of the twin core tubes are different, with one displacement of about 10.0 mm outward and the other displacement of about 6.2 mm inward. In the Y-direction, the deformation mainly concentrates at the bottom corners of the core tubes, with a maximum outward displacement of about 8.9 mm and a maximum inward displacement of about 7.9 mm. This difference may be explained by the complexity of the construction and the combined effect of the vertical support by piles and columns and the horizontal support by floor slabs. Overall, although the direction and value of deformation are different, the deformation of the twin core tubes is very small, with a maximum deformation of only 10.0 mm.

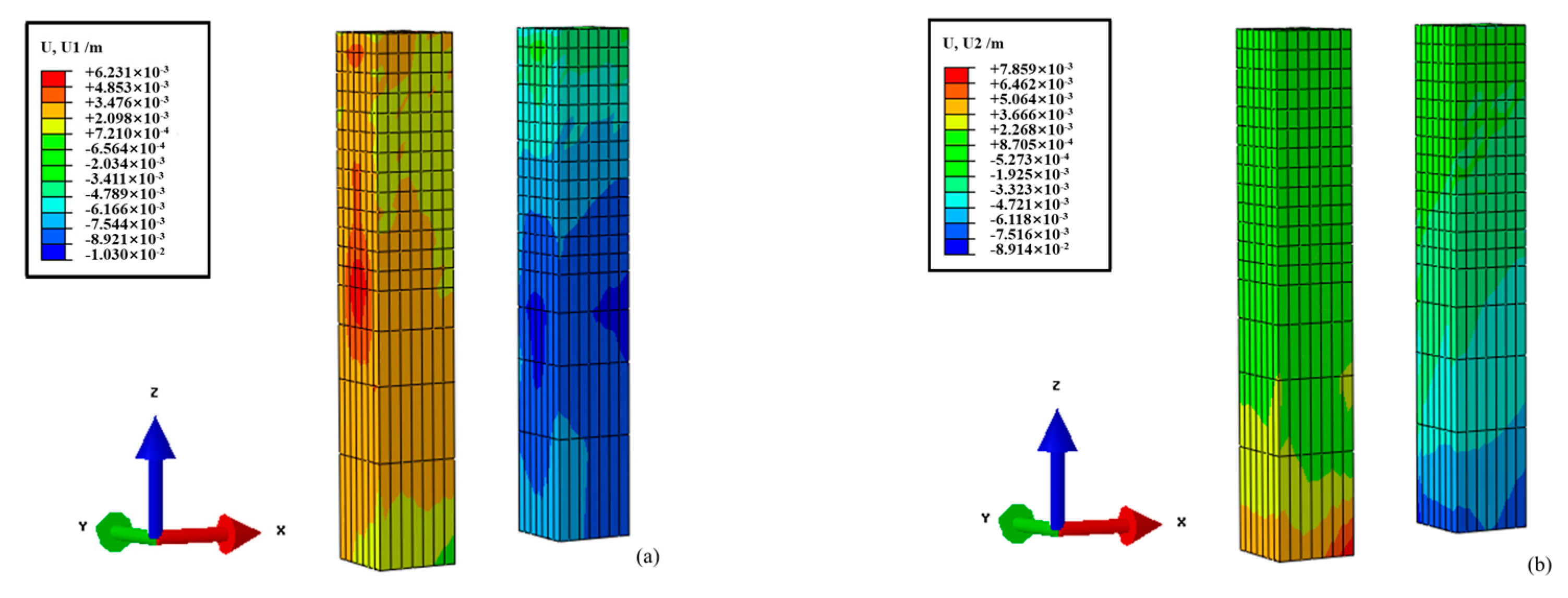
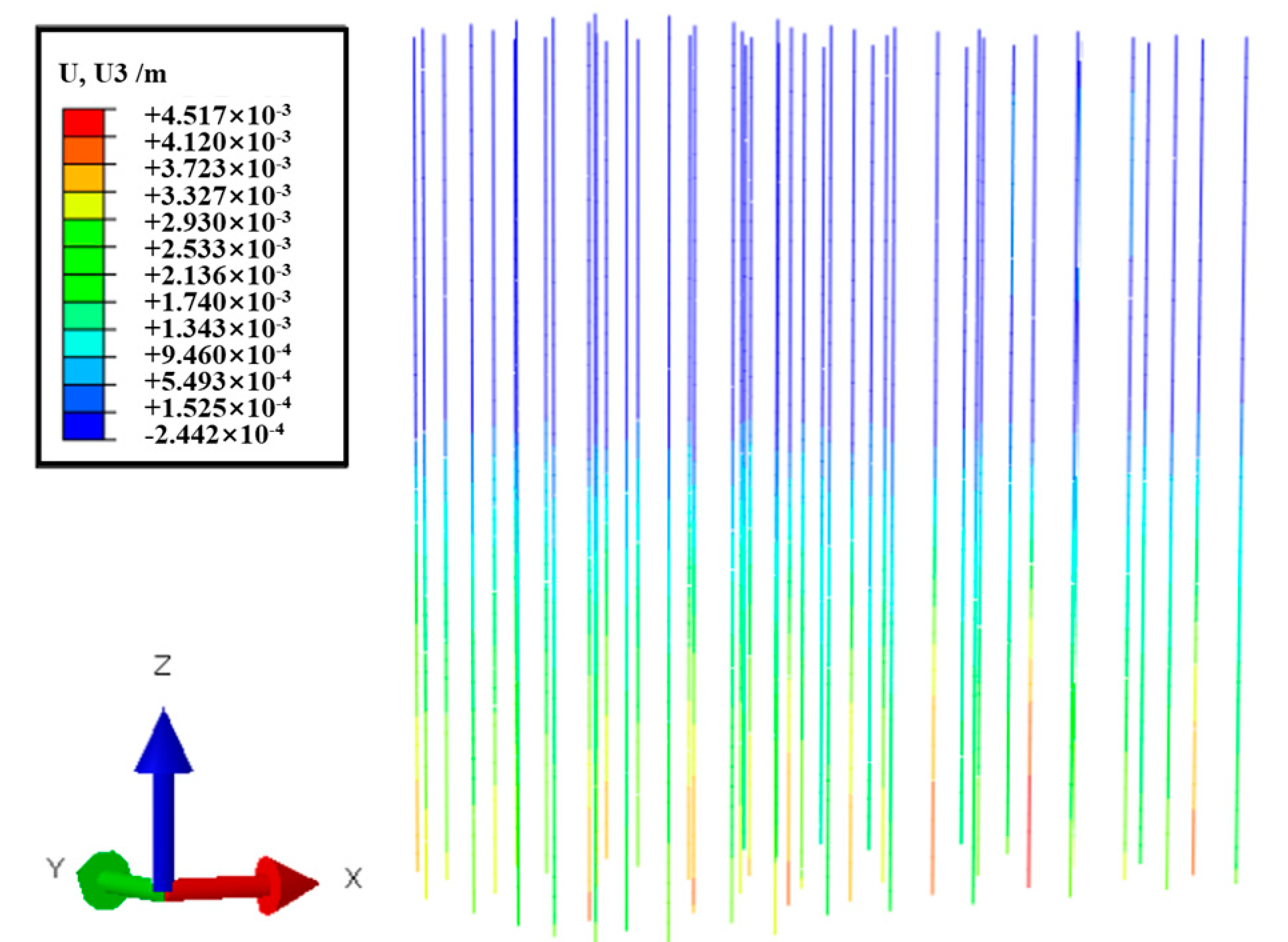
4.2.3. Integrated Piles and Columns

Integrated piles and columns are used as the foundation and internal structural components of buildings under construction and are also constructed before excavation of the pit. Figure 11 gives the vertical displacement contour map of the integrated piles and columns in the pit. It is found that the deformation of columns presents mainly settlement deformation, while the pile has a certain degree of bulge deformation, but the column itself undergoes a certain degree of compression. As we know, the unloading during excavation causes the floating of the integrated piles and columns, but the weight of the superstructure and substructure leads to the settlement of the integrated piles and columns, with the combination of the two effects resulting in the sinking of the columns and the floating of the support piles. However, the maximum floating is only 3.3 mm, and the maximum sinking is only 4.5 mm, with neither of them reaching half of the control value (10.0 mm). Therefore, the integrated piles and columns used as vertical supports in the pit are in a safe condition during the whole construction process.

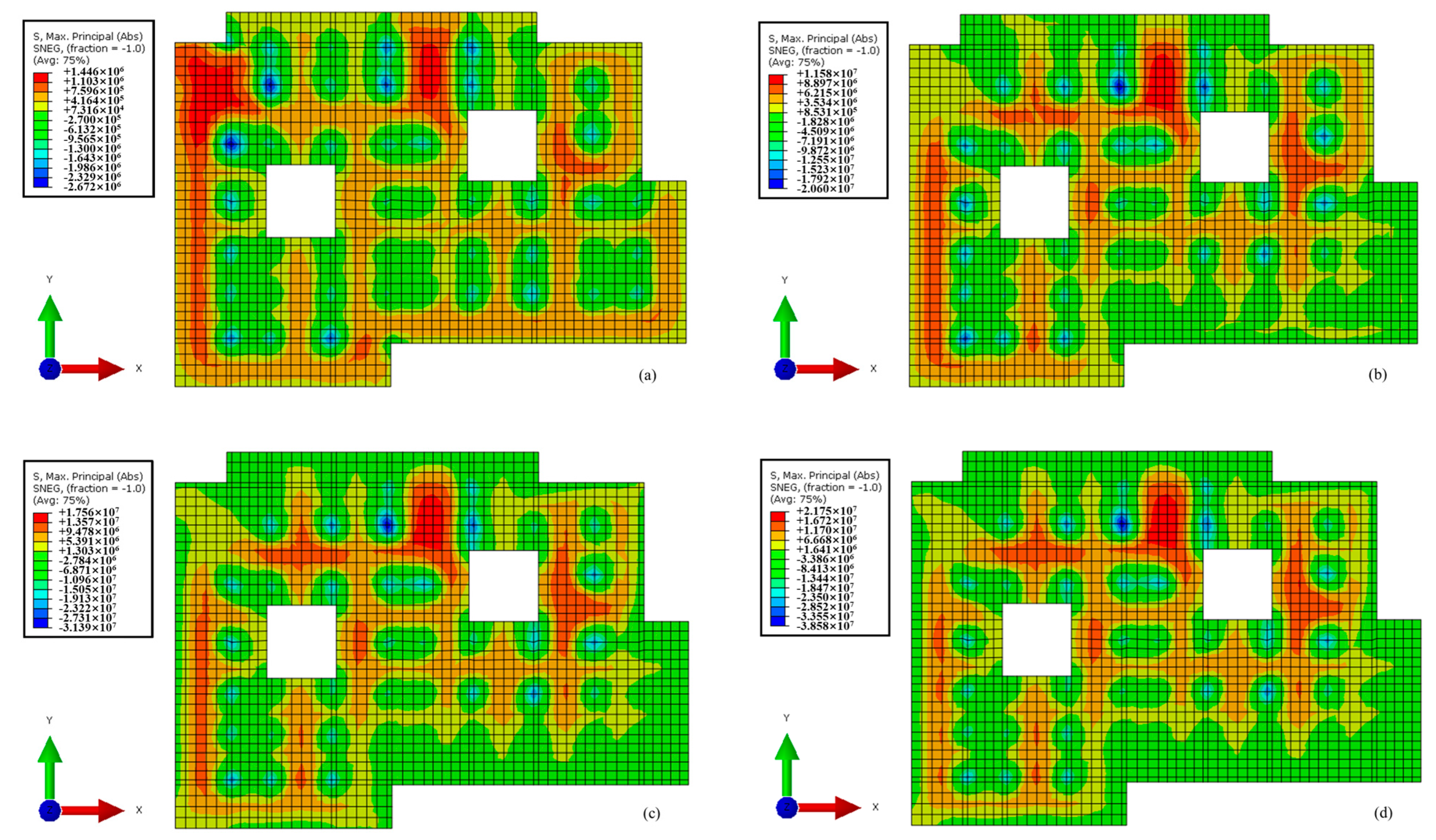
4.2.4. The Roof of the First Underground Floor

Figure 12 presents the stress contour maps of the roof of the first underground floor at the end of each construction process, where positive values represent tensile stress and negative values represent compressive stress. From Figure 12, it can be seen that as the construction progresses, the tensile stress distribution of the top roof of the first underground floor only slightly changes, while the compressive stress distribution changes significantly between the 4th and 7th processes, but the change is very small after the 7th process, with most floors in a compressed state. Tensile stress takes on a cross-distribution pattern and mainly distributes between columns and columns or columns and side walls. Meanwhile, the compressive stress mainly appears at the intersection between the roof of the first underground floor and the columns. The tensile stress and the compressive stress increase with the construction process, but the maximum tensile stress and the maximum compressive stress suddenly increase by about 10 times between process 4 and process 5 due to the gravity load of the superstructure during synchronous construction. From process 5 to process 10, the maximum tensile stress and the compressive stress both increased by about 4 times. As the construction progresses, the ratio of maximum compressive stress to maximum tensile stress gradually decreases but remains around 1.8 times. At the end of construction, the maximum tensile stress reaches 46 MPa, and the maximum compressive stress reaches 81 MPa, which both occur on the north side of the pit. These results highlight that it is necessary to strengthen the supervision of the roof of the first underground floor on the north side of the pit during the construction to avoid its cracking and damage affecting the progress of the project.

Figure 13 shows the bending moment contour maps of the roof of the first underground floor in the X- and Y-direction at the end of construction, from which it can be found that in the X-direction, the maximum positive bending moment is 3.0 × 10⁶ N·m, and the maximum negative bending moment is 2.0 × 10⁶ N·m. Namely, the maximum positive bending moment is about 1.5 times the maximum negative bending moment. In addition, the positive bending moment is mainly distributed at the intersection between the roof of the first underground floor and the columns, and the negative bending moment mainly takes on a parallel distribution to the Y-axis. In the Y-direction, the maximum positive bending moment is 3.2 × 10⁶ N·m, and the maximum negative bending moment is 1.4 × 10⁶ N·m, which means the maximum positive bending moment is about 2.3 times the maximum negative bending moment. The positive bending moment is mainly distributed at the intersection between the roof of the first underground floor and columns and around them, while the negative bending moment is mainly distributed parallel to the X-axis. Therefore, it is particularly important to pay special attention to the parts with significant changes in the bending moment, especially the north side of the roof of the first underground floor and the intersection between the roof of the first underground floor and the columns, to avoid sudden changes in the stress direction that may cause cracking and other damages.

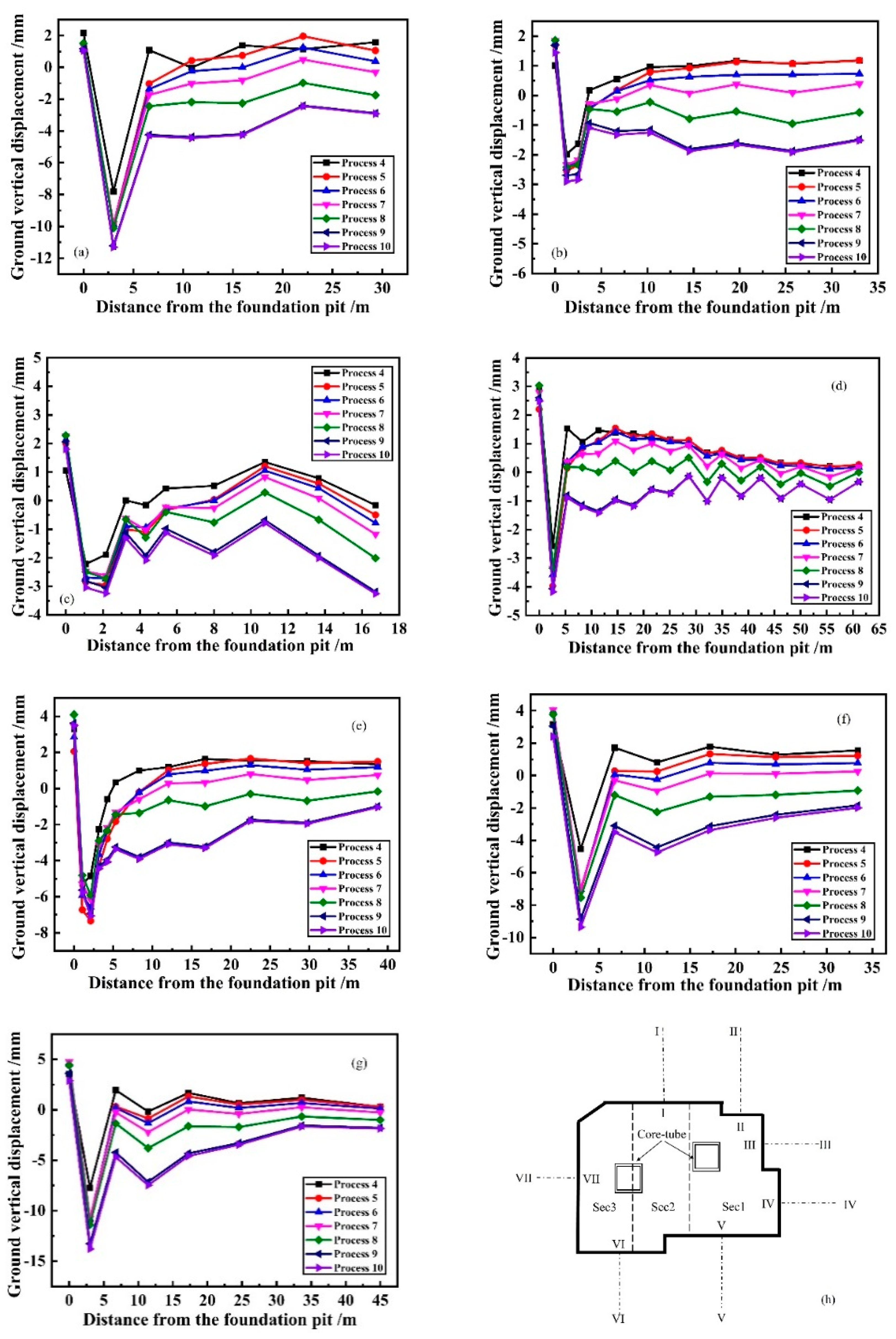
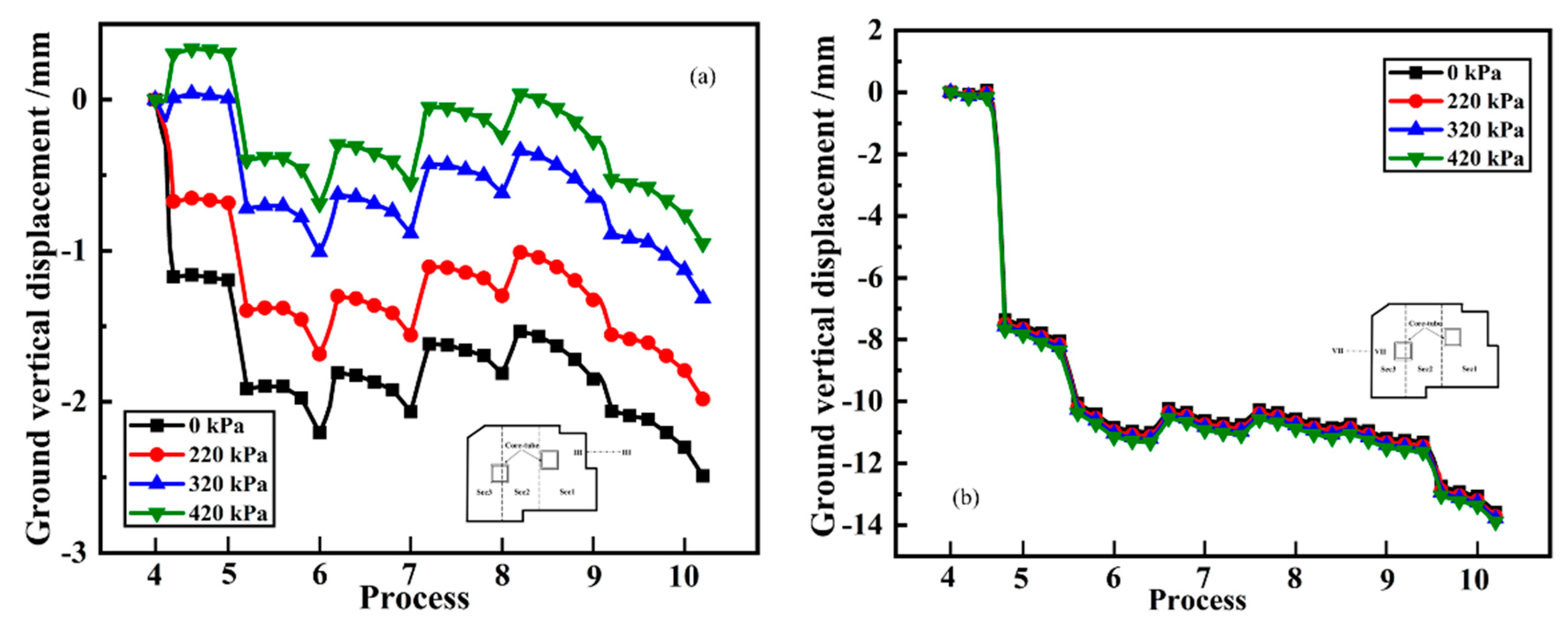
4.3. Site Response

Figure 14 shows the surrounding ground settlement after the excavation of 6.7 m. It is obvious that the ground settlement along the foundation pit wall is distributed as a gradual decrease from the middle to the sides with a bulge at the corner of the foundation pit, indicating a significant spatial effect. Meanwhile, the ground settlement on the east side of the foundation pit is smaller than the other three areas, which means that the presence of adjacent existing buildings on the east side has a certain impact on ground settlement. Thus, the pit wall was divided into seven different segments and numbered clockwise according to I to VII; then, seven monitoring sections perpendicular to wall were set in the middle of segments, numbered as I–I, II–II, III–III, IV–IV, V–V, VI–VI, and VII–VII, as shown in Figure 1.

Figure 15 presents the ground settlement troughs of seven monitoring sections during the construction process. As the construction progresses, the settlement continues to increase. From Figure 15, it can be seen that there is uplift deformation near the pit wall, the ground settlement curve shows a clear groove shape, and the settlement reaches its maximum at a distance of about 2.0–3.0 m from the pit wall. Due to the existing residential buildings on the east of the pit, the settlements of monitoring section III–III and section IV–IV increase as the distance from the pit wall increases after exceeding 11.0 m. That of the other monitoring sections are different, manifested as the ground settlements being basically the same, with a slight decrease after the distance from the pit wall exceeding 10.0 m. The construction of process 10 has a relatively minimal impact on the ground settlement, and all the construction processes have no impact on the position of the maximum deformation value of the ground settlement trough. After the completion of construction, the maximum settlement value reaches 13.8 mm, and the maximum uplift value reaches 4.8 mm, both of which are far less than the design control value (30.0 mm), meeting the safety requirements. From Figure 15, the maximum settlement for all sections are approximately between 0.11‰ H and 0.54‰ H (H is the excavation depth of the pit), and the influence range of excavation is between around 0.88 H and 1.37 H, which indicates that the spatial position of the diaphragm wall, the wall length, and the spatial position of existing buildings have a significant impact on the magnitude and the shape of the settlement trough but have little impact on the location of the maximum settlement in the settlement trough. The distribution of the settlement trough indicates that the significant impact range of excavation of the pit in this project is approximately within 10.0 m around the pit. Therefore, it is necessary to strengthen the monitoring of the structures and traffic routes within 10.0 m around the pit to avoid the adverse impact of construction during excavation.

Figure 16 shows the horizontal displacement of strata behind the wall at different monitoring sections after the completion of construction. It is observed that the strata behind the wall have a horizontal displacement towards the pit, and the deformation characteristics are quite similar to that of the diaphragm wall, also showing the shape of “double peaks”, and the deformation values are basically the same, indicating that the deformation of the pit was in a stable state. With the increase in the extension length of the diaphragm wall, the horizontal displacement of strata behind the wall gradually increases, but the maximum displacement always appears around 22.5 m below the ground. The maximum horizontal displacement is about 1.4–2.0 times that of the ground.

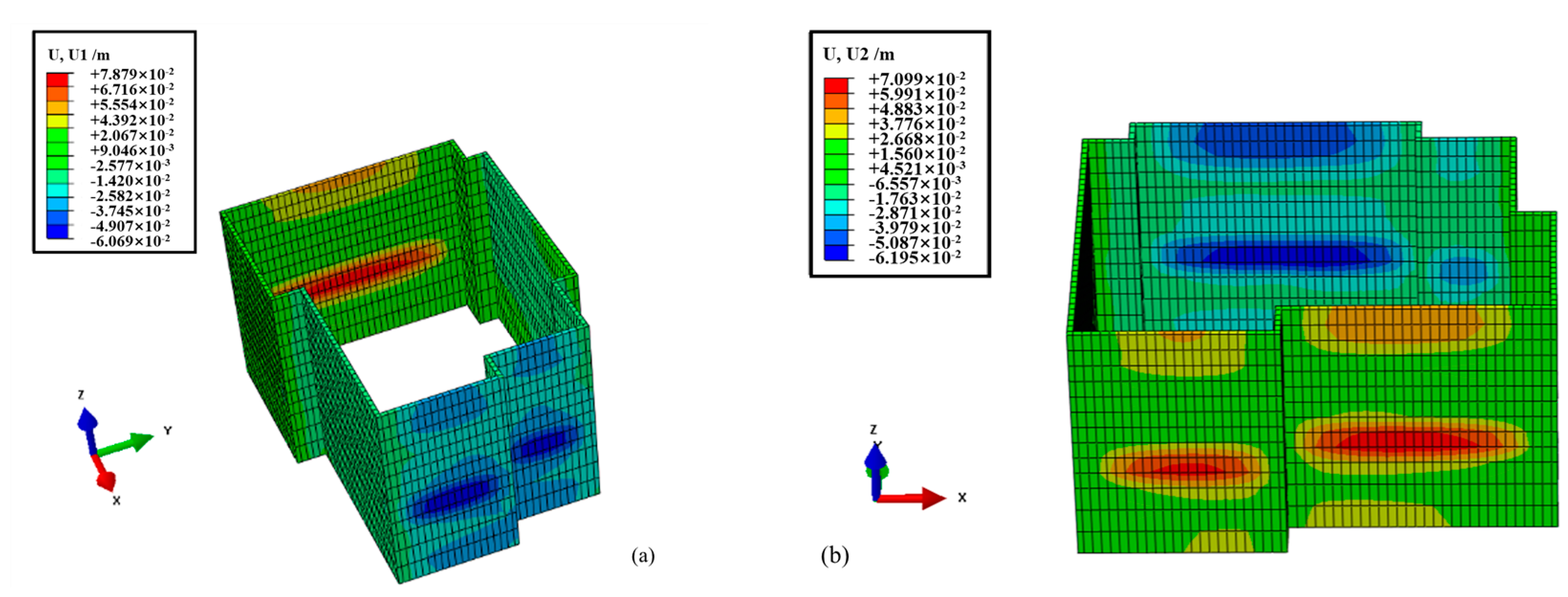
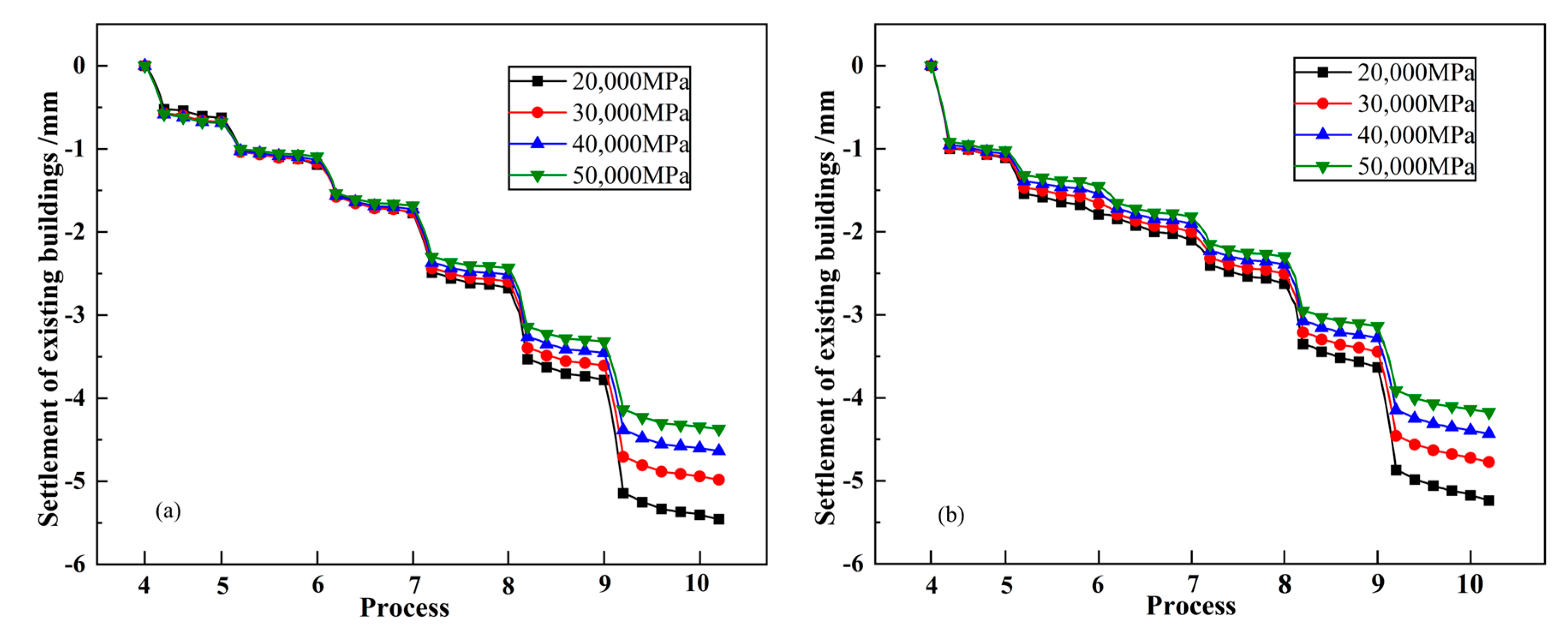
4.4. Settlement of Existing Buildings

Figure 17 provides the history curves of the settlement of existing buildings during the whole construction process. As can be seen, the deformation trend of the two points is similar. Both of them gradually increase as construction proceeds and present an obvious spatial effect: the settlement rate is fast and the settlement amount is large in the excavation of Sec1, while the settlement rate decreases and the settlement amount is small in the excavation of Sec2 and Sec3. At the beginning of excavation, the settlement of JC-2 is larger than that of JC-1, but as excavation proceeds, the settlement of JC-1 gradually exceeds that of JC-2. The final settlement of JC-1 and JC-2 are 5.0 mm and 4.8 mm, respectively, which are both much less than the design control value (15.0 mm), satisfying the safety requirements.

5. Discussions

5.1. Construction Sequence

The response of existing buildings induced by excavation is a very important issue,

Table 4. The final settlement of existing buildings under different construction sequences.

The Cooperative Construction Sequence	Settlement of Existing Buildings (mm)
	JC-1	JC-2
Actual construction sequence	4.981	4.772
Excavate after the construction of the roof of the first underground floor and the superstructure	4.523	4.573
Construct the superstructure after all the substructures are completed	5.029	4.780
Construct the superstructure of the 16th to the 23rd floors during the excavation of the last layer of pit	4.992	4.783
Construct the superstructure during excavation of the 2nd layer of pit	5.005	4.777
Construct the superstructure during excavation of the 3rd layer of pit	5.089	4.795

lists the final settlement of existing buildings under different construction sequences. The data indicate that the impact of the construction sequence on the settlement of existing buildings is so small that it is almost negligible; the construction sequence can be adjusted appropriately to meet the requirements of the construction period under the condition of ensuring safety in the actual project.

5.2. Existing Buildings

Figure 18 shows the horizontal displacements of the diaphragm wall of section III–III and section VII–VII under four loads of adjacent buildings. It can be found that the impact of existing buildings on the horizontal displacement of the diaphragm wall is related to the relative position between the existing buildings and the diaphragm wall. For the diaphragm wall close to the existing buildings, the horizontal displacement gradually decreases with the increase in the weight of existing buildings. Whereas, for the diaphragm wall far away from the existing buildings, the horizontal displacement gradually increases with the increase in the weight of existing buildings. The impact of the existing buildings on the horizontal displacement of the diaphragm wall is mainly concentrated in the upper and lower portions of the wall and has little impact on the middle portion.

Figure 19 shows the settlement history curves of the ground monitoring points behind the pit wall on the monitoring section of III–III and VII–VII under different buildings loads. It is found that the existing buildings have a significant impact on the ground settlement between them and the excavation pit, while the impact on the site on the other side of the pit is very small. As the building load increases, the ground settlement between the building and the excavation pit decreases, and the final settlement values under the four building load conditions are 2.5 mm, 2.0 mm, 1.3 mm, and 1.0 mm, respectively. However, when the load of existing buildings is 420 kPa, there will be a certain degree of heave at the monitoring point during the initial construction stage; as the construction progresses, the heave gradually transforms into settlement deformation, and the overall shape of the ground settlement curve remains unchanged. Comparing the existing building loads of 0 kPa and 320 kPa, it is found that ground settlement decreased by approximately 47.1% due to the existence of buildings.

5.3. Strength of the Diaphragm Wall

The diaphragm wall is a reinforced concrete wall made of steel cages inserted into concrete. In this section, we explore the influence of the diaphragm wall strength on its deformation and the settlement of existing buildings using changes in the elastic modulus.

Figure 20 shows the horizontal displacements of the diaphragm wall at section V–V and section VII–VII when the wall adopts different strength concrete. From Figure 20, it can be seen that the strength of diaphragm wall only affects the horizontal displacement above the excavation depth and has no effect on the horizontal displacement below the excavation depth. As the strength of diaphragm wall increases, the horizontal displacement above the excavation depth gradually decreases, but this effect mainly occurs at a depth of 22.5 m. Compared with the strength of 20,000 MPa, the horizontal displacement at 22.5 m depth of section V–V decreases by 12.4%, 20.5%, and 26.5%, in turn, while that of section VII–VII decreases by 12.3%, 20.2%, and 25.9%, in turn. It could be concluded that the change in the strength of the diaphragm wall has similar influence on the maximum horizontal displacement of diaphragm walls, and the influence degree gradually weakens.

Figure 21 shows the settlement history curves of existing buildings under different diaphragm wall strengths. As shown in Figure 21, the settlement of existing buildings decreases with the increase in the diaphragm wall strengths. The change in strength of the diaphragm wall will not affect the shape of the settlement history curve. Compared with the strength of 20,000 MPa, the final settlement of JC-1 decreases by 8.7%, 15.1%, and 19.9%, in turn, while that of JC-2 decreases by 8.8%, 15.3%, and 20.3%, in turn. The variation in settlement values is very close, which may be related to the fact that the two monitoring points are at the same distance from the excavation surface of the pit.

6. Conclusions

Based on the Abaqus finite-element software platform, a 3D numerical simulation was conducted on a cooperative construction of the superstructure and substructure under a covered semi-top-down method, analyzing the mechanical characteristics of the foundation pit itself and the surrounding environments and exploring the main influencing factors.

(1)

During the whole process of cooperative construction of the superstructure and substructure under the covered semi-top-down method, the horizontal displacement of the diaphragm wall and of soil behind the wall along the depth direction gradually changes from a “single peak” to a “double peak” distribution, and the deformation value is related to the horizontal extension length and location of the wall;

(2)

The final deformations of the surrounding ground and of the existing buildings are settlements as the construction progress. The ground settlement shows an obvious groove shape, but it would be affected by the existing buildings and the corner effect of the pit. The significant impact range of excavation is approximately within 10.0 m around the pit. It is necessary to strengthen the monitoring of the structures and traffic routes within 10.0 m around the pit to avoid the adverse impact of construction during excavation;

(3)

The existing buildings have a greater impact on the deformation of the ground and the diaphragm wall between the building and the excavation, with a certain restraining effect;

(4)

The strength of the diaphragm wall has an obvious impact on its deformation and the settlement of existing buildings. As the strength increase, the horizontal displacement of the diaphragm wall and the vertical displacement of existing buildings both tend to decrease, but the degree of decrease is gradually diminished;

(5)

The impact of the construction sequence on the settlement of existing buildings can be negligible; the construction sequence can be adjusted appropriately to meet the requirements of the construction period under the condition of ensuring safety;

(6)

In this article, we only conducted research on specific engineering; comparative studies on different construction methods in complex site conditions need to be conducted in the future. Meanwhile, refined models also need to be gradually incorporated into engineering to obtain better results.