Laboratory Study on Rockburst Control by Step Method in Deep Tunnel
Abstract
1. Introduction
2. Experimental Methods
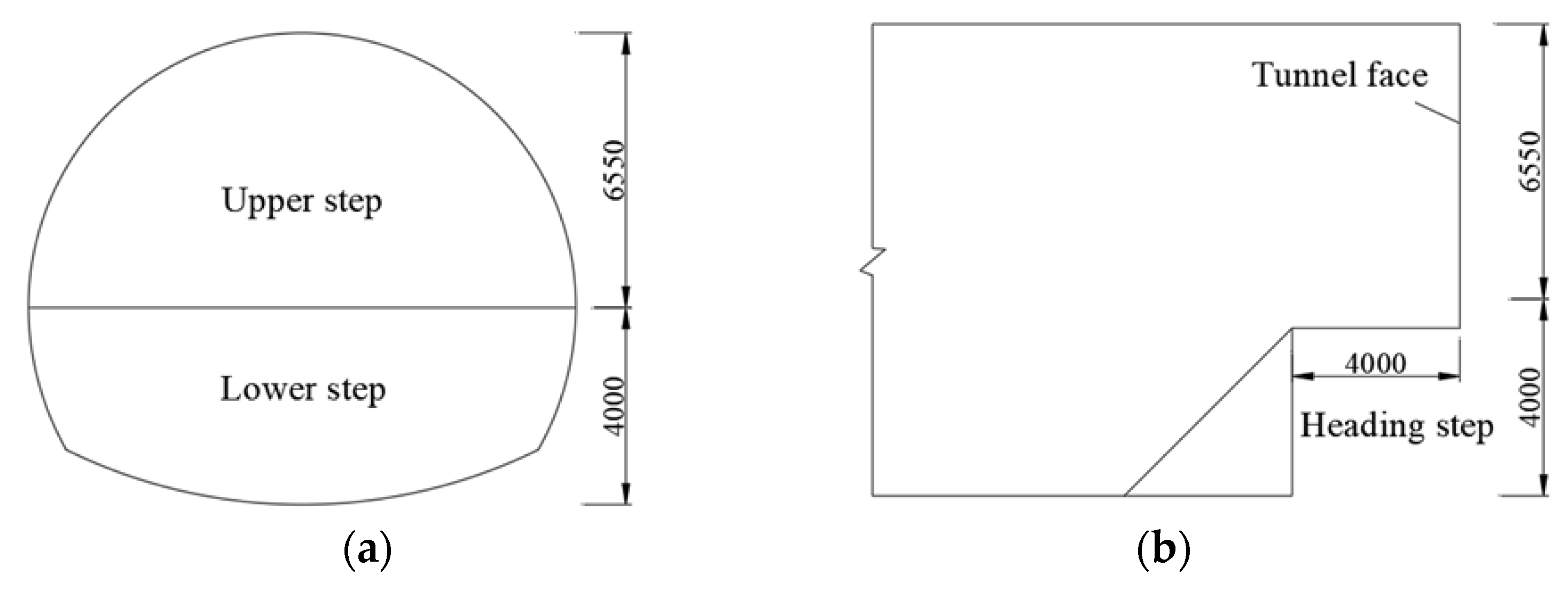
2.1. Engineering Background
2.2. Design of Rockburst Control
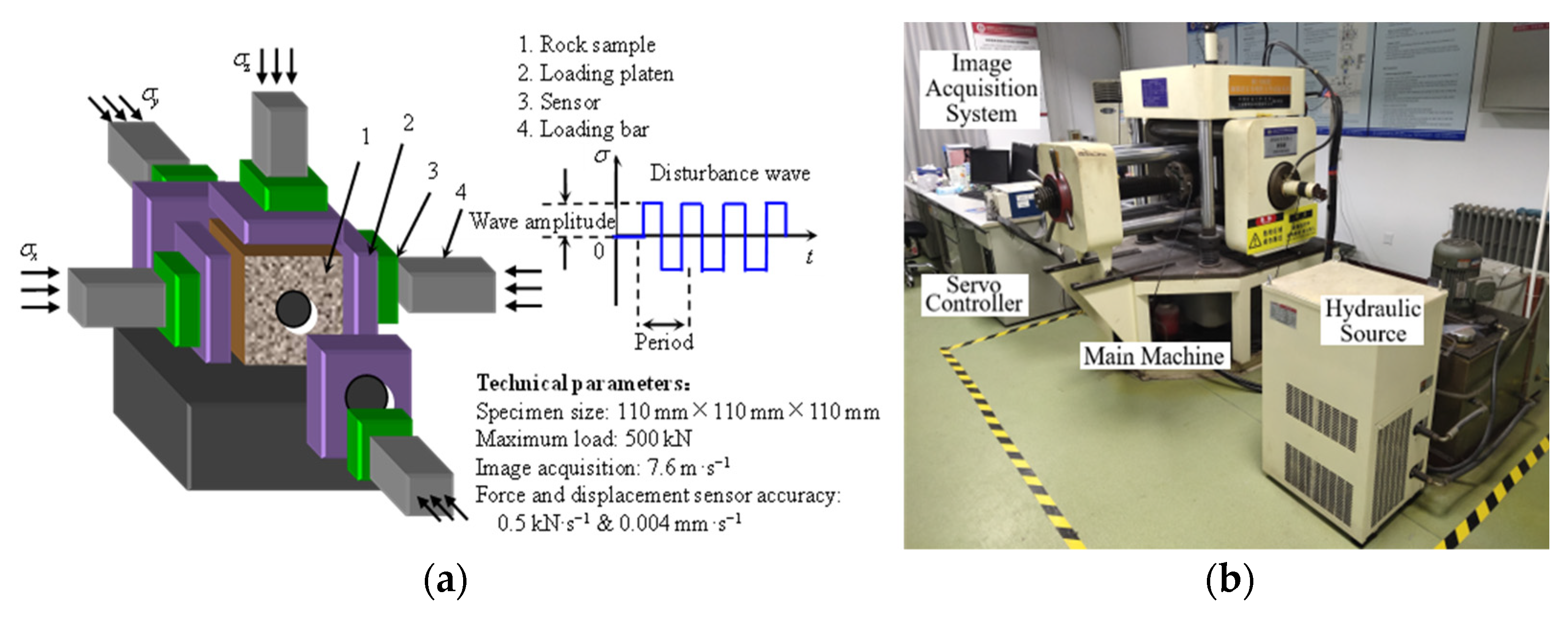
2.3. Testing Equipment
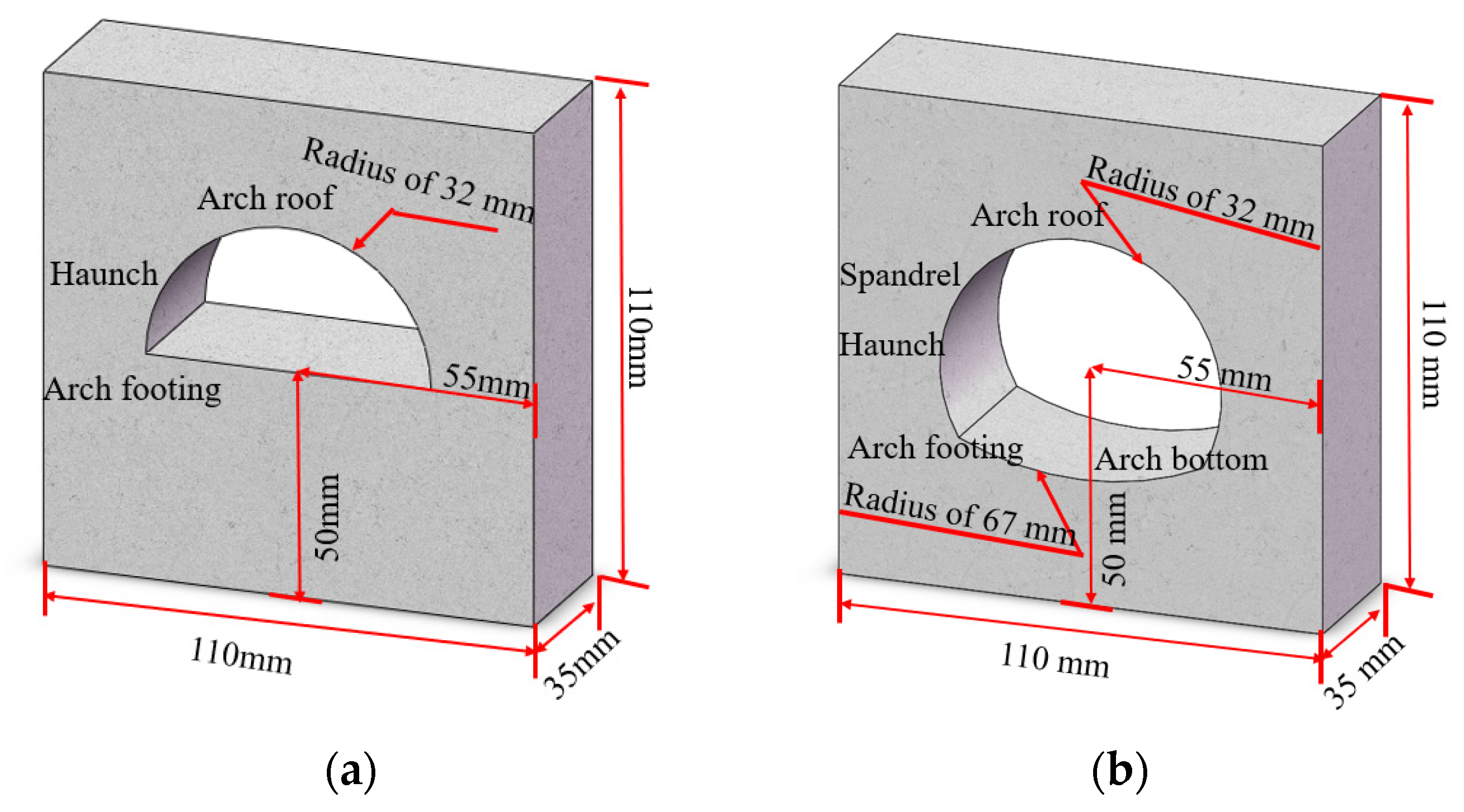
2.4. Rock Description and Specimen Preparation
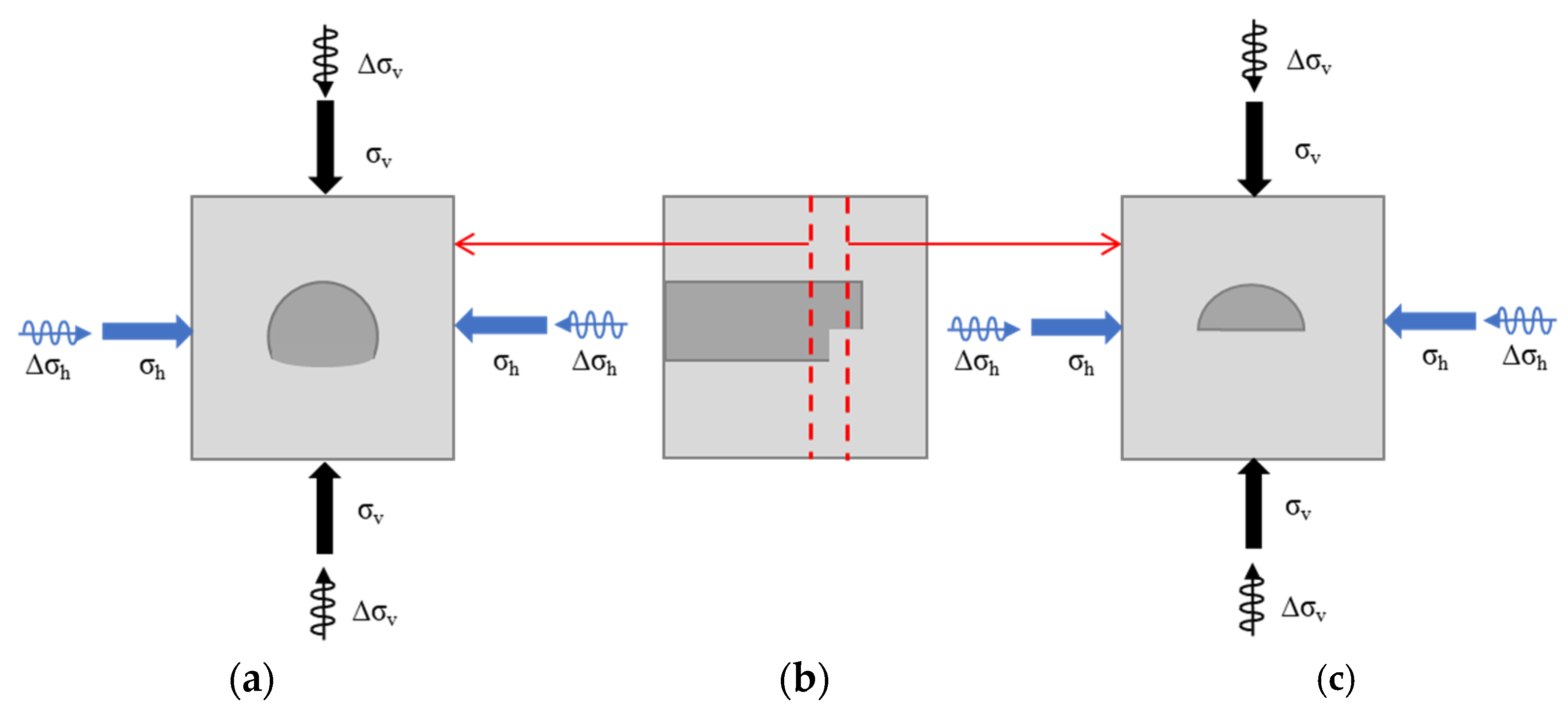
2.5. Experimental Scheme
3. Testing Results Analysis
3.1. Analysis of Experimental Results of Upper Step Specimen
3.1.1. Space–Time Evolution Process of Surrounding Rock Deformation and Fracture
3.1.2. Characteristics of Acoustic Emission Energy
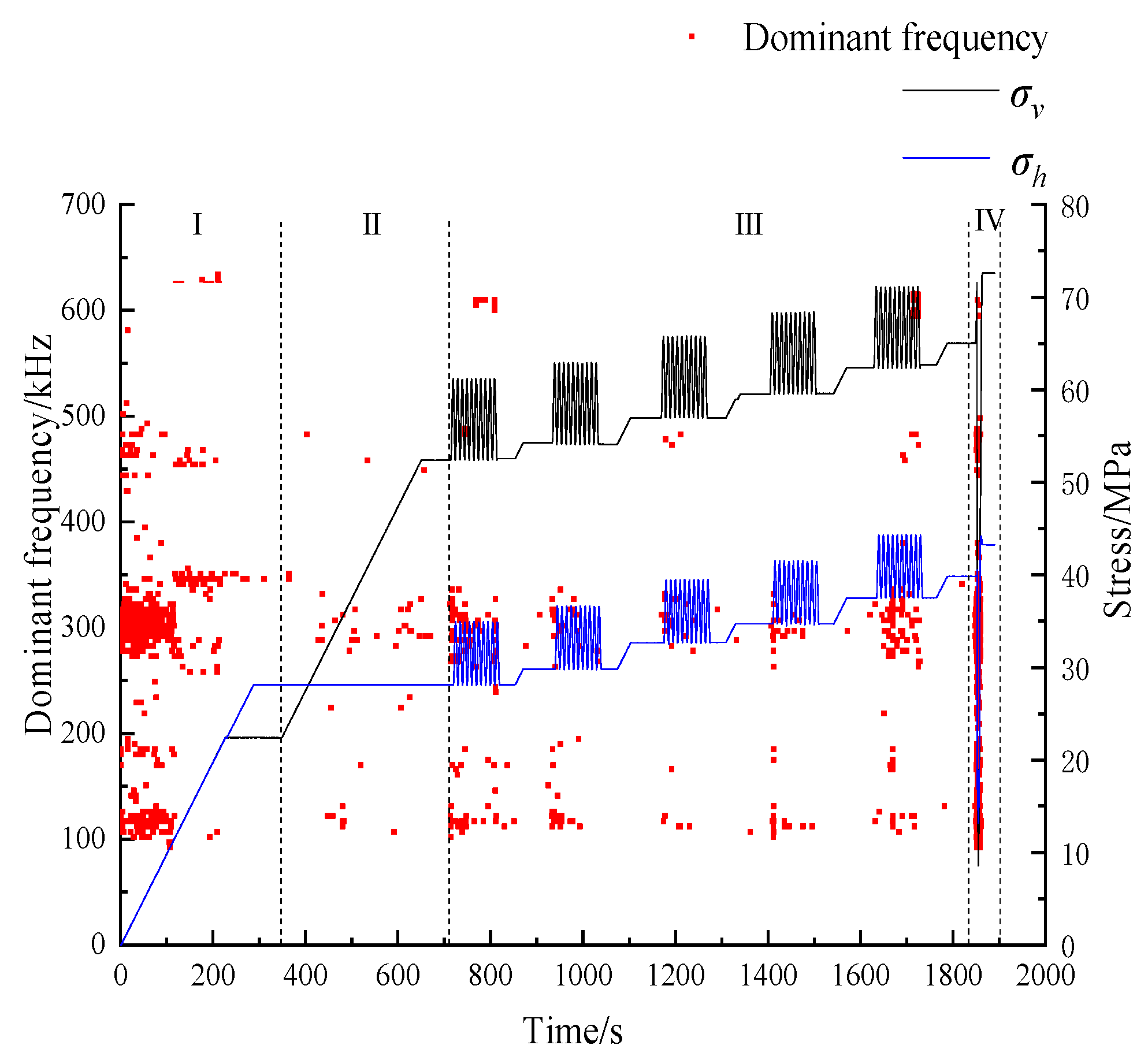
3.1.3. Analysis of Characteristics of Two-Dimensional Spectrum
3.2. Analysis of Experimental Results of Lower Step Specimen
3.2.1. Space–Time Evolution Process of Deformation and Fracture of Surrounding Rock
3.2.2. Characteristics of Acoustic Emission Energy
3.2.3. Analysis of Characteristics of Two-Dimensional Spectrum
4. Discussion and Conclusions
4.1. Discussion
4.2. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, G.K.; Xue, Y.G.; Qu, C.Q.; Qiu, D.H.; Wang, P.; Liu, Q.S. Intelligent prediction of rockburst in tunnels based on back propagation neural network integrated beetle antennae search algorithm. Environ. Sci. Pollut. Res. 2023, 30, 33960–33973. [Google Scholar]
- He, X.Q.; Zhou, C.; Song, D.Z.; Li, Z.L.; Cao, A.Y.; He, S.Q.; Khan, M. Mechanism and monitoring and early warning technology for rockburst in coal mines. Int. J. Miner. Metall. Mater. 2021, 28, 1097–1111. [Google Scholar]
- Niu, W.J.; Feng, X.T.; Feng, G.L.; Xiao, Y.X.; Yao, Z.B.; Zhang, W.; Hu, L. Selection and characterization of microseismic information about rock mass failure for rockburst warning in a deep tunnel. Eng. Fail. Anal. 2022, 131, 105910. [Google Scholar] [CrossRef]
- Sun, L.J.; Hu, N.Y.; Ye, Y.C.; Tan, W.K.; Wu, M.L.; Wang, X.H.; Huang, Z.Y. Ensemble stacking rockburst prediction model based on Yeo-Johnson, K-means SMOTE, and optimal rockburst feature dimension determination. Sci. Rep. 2022, 12, 15352. [Google Scholar] [CrossRef]
- Wang, G.F.; Gong, S.Y.; Dou, L.M.; Cai, W.; Yuan, X.Y.; Fan, C.J. Rockburst mechanism and control in coal seam with both syncline and hard strata. Saf. Sci. 2019, 115, 320–328. [Google Scholar]
- Konicek, P.; Waclawik, P. Stress changes and seismicity monitoring of hard coal longwall mining in high rockburst risk areas. Tunn. Undergr. Space Technol. 2018, 81, 237–251. [Google Scholar] [CrossRef]
- Vennes, I.; Mitri, H.; Chinnasane, D.R.; Yao, M.K. Large-scale destress blasting for seismicity control in hard rock mines: A case study. Int. J. Min. Sci. Technol. 2020, 30, 141–149. [Google Scholar]
- Vennes, I.; Mitri, H.; Chinnasane, D.R.; Yao, M.K. Effect of Stress Anisotropy on the Efficiency of Large-Scale Destress Blasting. Rock Mech. Rock Eng. 2021, 54, 31–46. [Google Scholar] [CrossRef]
- Gao, H.; Zhao, W.S.; Chen, W.Z.; Xie, P.Y.; Zhong, K.; Qin, C.K. Continuous three-dimensional stress monitoring in roof of coal mines for investigating the rockburst control effect with hydraulic fracturing. Environ. Earth Sci. 2022, 81, 433. [Google Scholar]
- Feng, X.T.; Liu, J.P.; Chen, B.R.; Xiao, Y.X.; Feng, G.L.; Zhang, F.P. Monitoring, Warning, and Control of Rockburst in Deep Metal Mines. Engineering 2014, 3, 538–545. [Google Scholar]
- Kaiser, P.; Mccreath, D.; Tannant, D. Canadian Rockburst Support Handbook; CAMIRO Mining Division: Sudbury, ON, Canada, 1996. [Google Scholar]
- Li, C.C. Principles and methods of rock support for rockburst control. J. Rock Mech. Geotech. Eng. 2021, 13, 46–59. [Google Scholar]
- Wang, Q.; Wu, W.R.; Wang, Y.T.; He, M.C.; Xue, H.J.; Wei, H.Y. Evolution and Control Mechanism of Rockburst in Rock Anchored by New Energy-Absorbing Material CREA material bolt. Rock Mech. Rock Eng. 2023, 56, 4569–4582. [Google Scholar]
- He, M.C.; Li, C.; Gong, W.L.; Wang, J.; Tao, Z.G. NPR bolt/cable support principle and large deformation control technology. J. Rock Mech. Eng. 2016, 35, 1513–1529. [Google Scholar]
- Luo, S.; Gong, F.Q. Evaluation of energy storage and release potentials of highly stressed rock pillar from rockburst control perspectives. Int. J. Rock Mech. Min. Sci. 2023, 163, 105324. [Google Scholar]
- Tang, J.L.; Li, T.B.; Zeng, P.; Han, Y.X.; Zhou, X.H. Study on rockburst flexible protective net and its dynamic characteristics. Chin. J. Rock Mech. Eng. 2019, 38, 794–797. [Google Scholar]
- Lu, X.F.; Pan, Y.S. The Oretical Analysis and Experimental Research on Rockburst Prevention Mechanism of Rigid-Flexible-Rigid Supporting Structure. Chin. J. Rock Mech. Eng. 2012, 31, 52–59. [Google Scholar]
- Wu, A.X.; Sun, Y.Z.; Sen, G.; Shen, H.M.; Yang, B.H. Characteristics of rockburst and its mining technology in mines. J. Cent. South Univ. Technol. 2002, 9, 255–259. [Google Scholar]
- Zhou, C.; Zhu, S.T.; Li, J.J.; Jiang, F.X.; Gao, Y.T.; Qu, X.C.; Ji, M.W.; Wei, Q.D. Basic Research on Rockburst Control Technology for Deep Well Filling of Municipal Solid Waste. Lithosphere 2022, 2022, 7262715. [Google Scholar]
- Liu, Y.; Lu, C.P.; Zhang, H. Mutation effect of acoustic and electromagnetic emissions of hard rock impact failure. Int. J. Distrib. Sens. Netw. 2019, 15, 1550147718824473. [Google Scholar]
- Su, G.S.; Li, P.F.; Xu, H.J.; Liu, X.J. A two-step method for predicting rockburst using sound signals. Acta Geotech. 2024, 19, 273–303. [Google Scholar]
- Zhang, Z.; Wang, E.; Zhang, H.; Bai, Z.; Zhang, Y.; Chen, X. Research on nonlinear variation of elastic wave velocity dispersion characteristic in limestone dynamic fracture process. Fractals 2023, 31, 2350008. [Google Scholar]
- Sun, X.M.; Xu, H.C.; Zheng, L.G.; He, M.C.; Gong, W.L. An experimental investigation on acoustic emission characteristics of sandstone rockburst with different moisture contents. Sci. China Technol. Sci. 2016, 59, 1549–1558. [Google Scholar]
- Hu, X.C.; Su, G.S.; Chen, G.Y.; Mei, S.M.; Feng, X.T.; Mei, G.X.; Huang, X.H. Experiment on Rockburst Process of Borehole and Its Acoustic Emission Characteristics. Rock Mech. Rock Eng. 2019, 52, 783–802. [Google Scholar]
- He, M.C.; Miao, J.L.; Feng, J.L. Rock burst process of limestone and its acoustic emission characteristics under true-triaxial unloading conditions. Int. J. Rock Mech. Min. Sci. 2009, 47, 286–298. [Google Scholar]
- Zhai, S.B.; Su, G.S.; Yin, S.D.; Yan, S.Z.; Wang, Z.F.; Yan, L.B. Fracture evolution during rockburst under true-triaxial loading using acoustic emission monitoring. Bull. Eng. Geol. Environ. 2020, 79, 4957–4974. [Google Scholar]


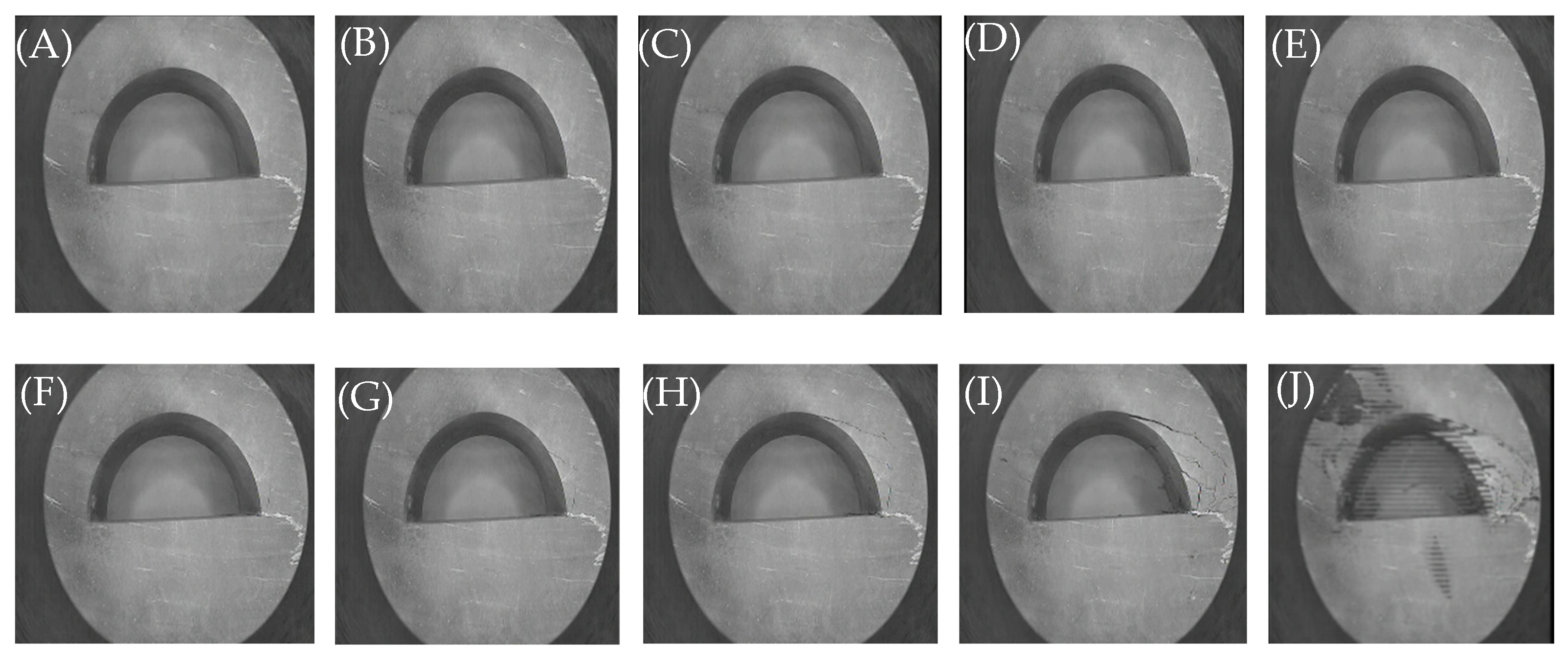


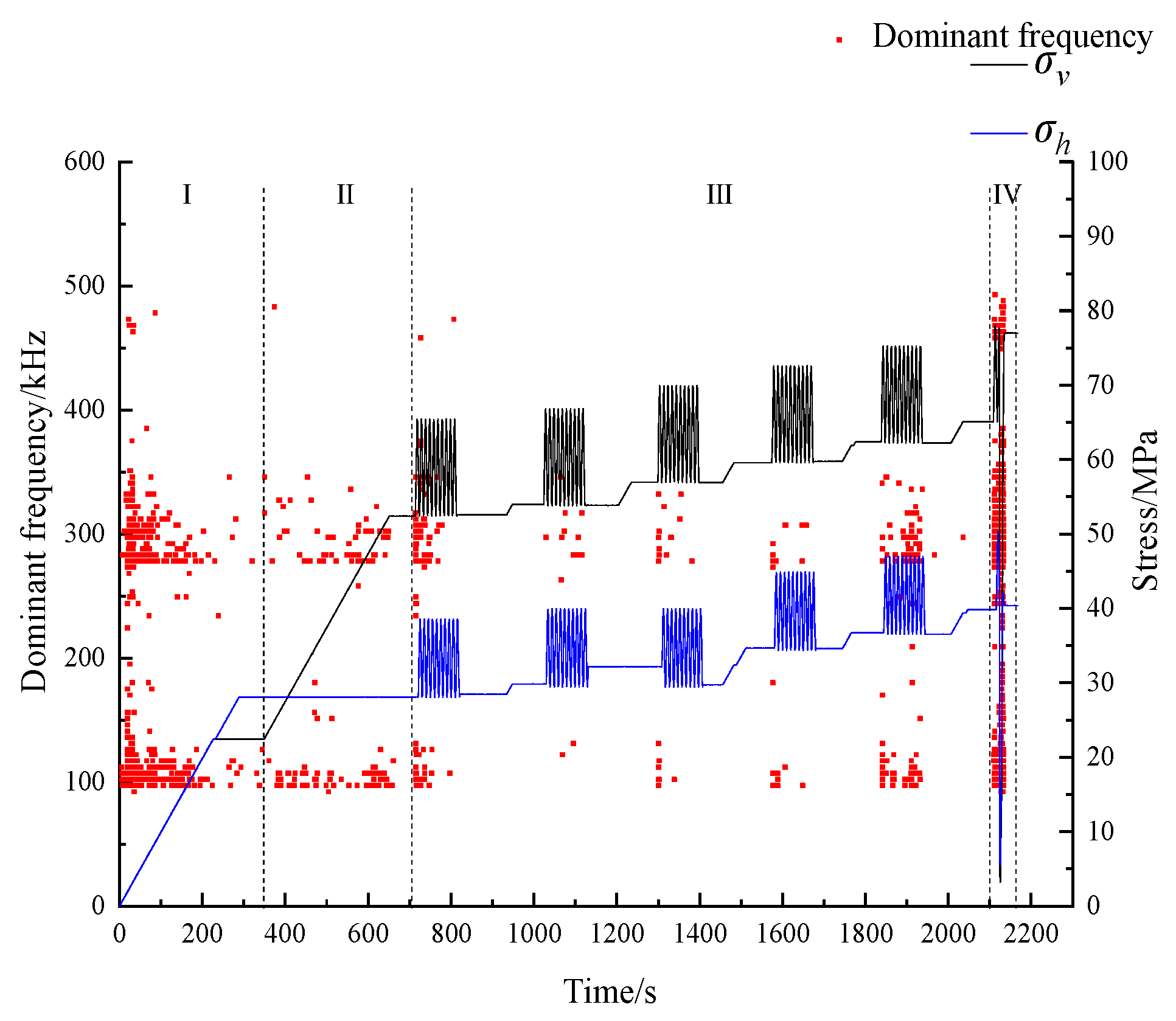

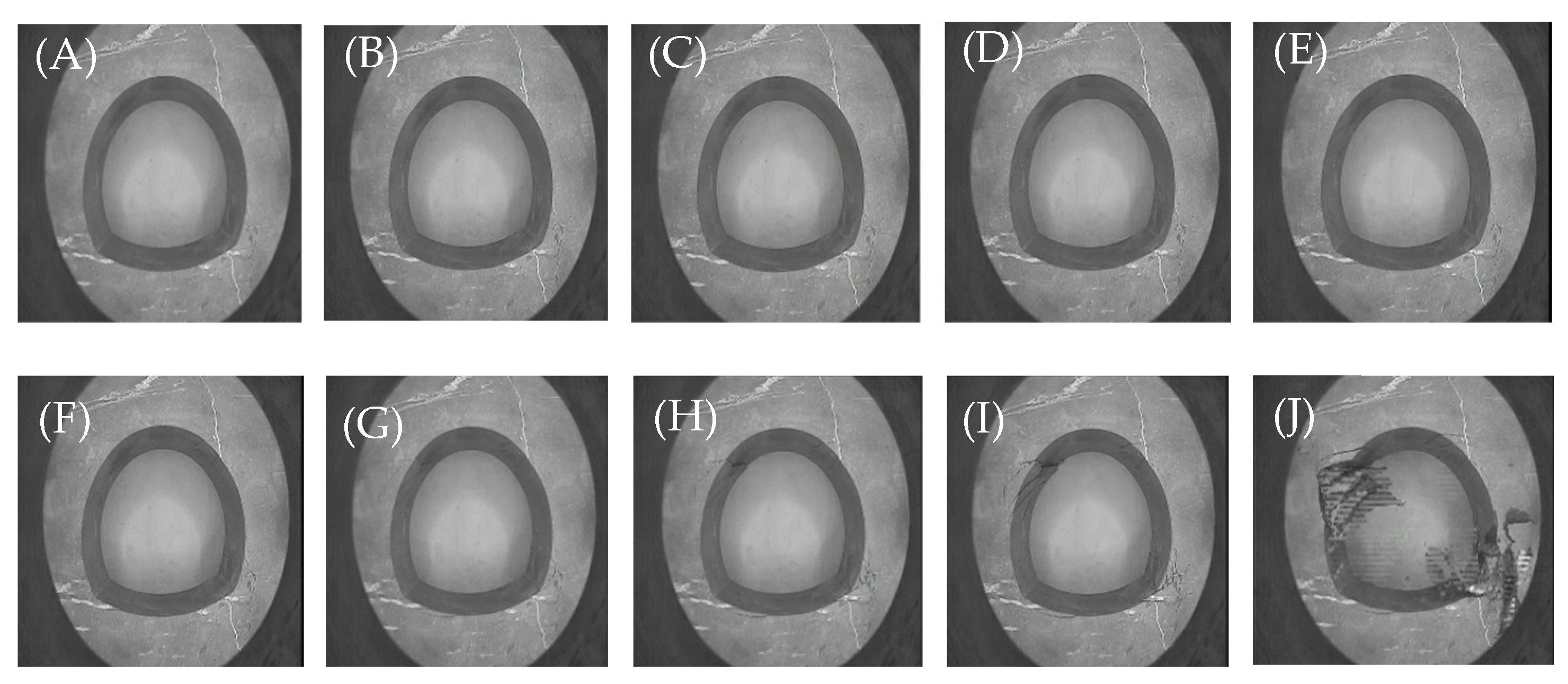

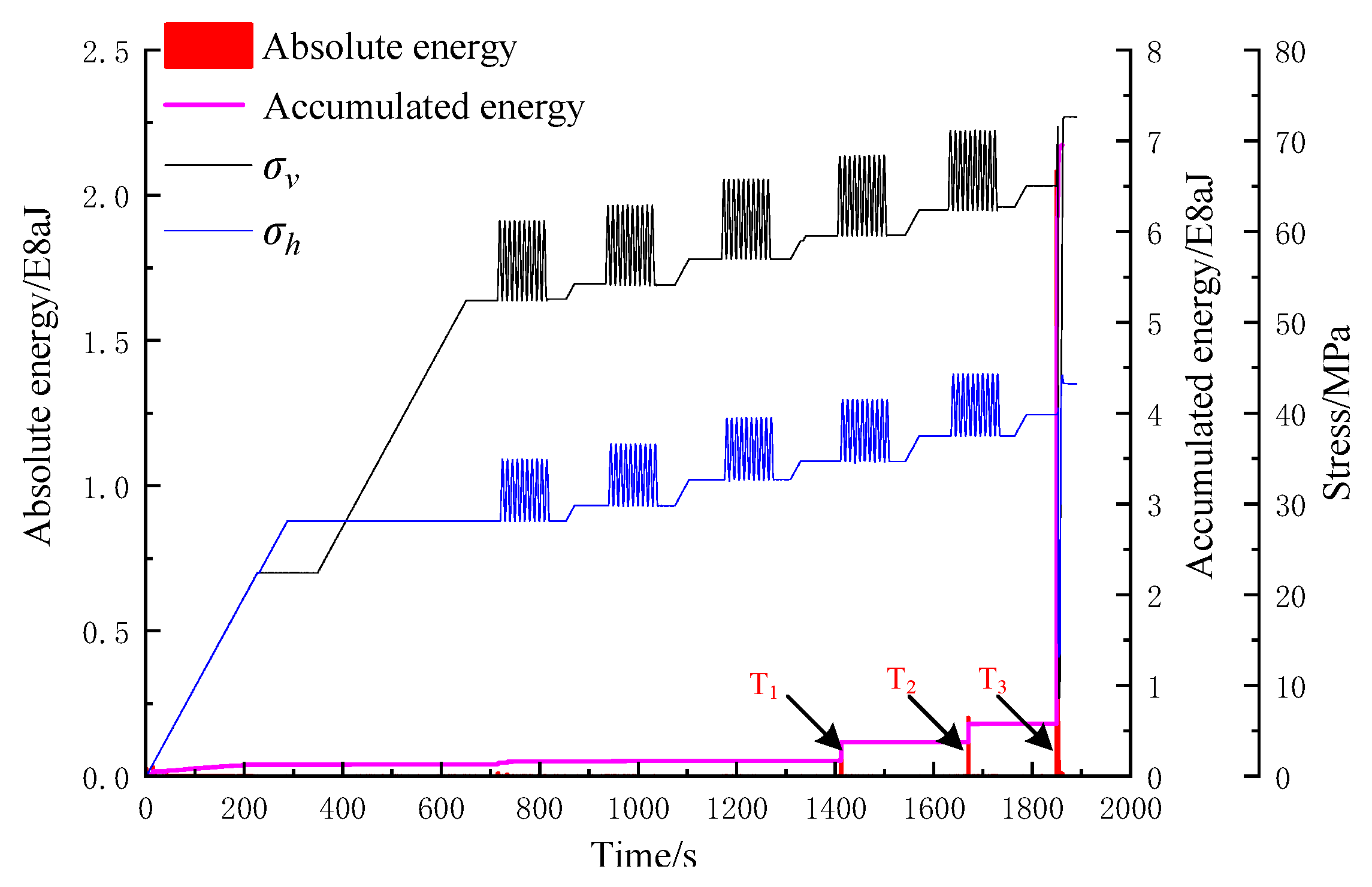
| Stages | Signal Proportion (%) | |||
|---|---|---|---|---|
| Low Frequency (100~200 kHz) | Intermediate Frequency (250~350 kHz) | High Frequency (450~600 kHz) | Ultra High Frequency (600~700 kHz) | |
| I | 51.70 | 37.93 | 0.97 | 0.00 |
| II | 31.01 | 46.51 | 0.78 | 0.00 |
| III | 32.79 | 56.72 | 0.66 | 0.00 |
| IV | 18.54 | 69.59 | 2.65 | 0.00 |
| Stages | Signal Proportion (%) | |||
|---|---|---|---|---|
| Low Frequency (100~200 kHz) | Intermediate Frequency (250~350 kHz) | High Frequency (450~600 kHz) | Ultra High Frequency (600~700 kHz) | |
| I | 19.33 | 67.54 | 9.43 | 0.00 |
| II | 18.60 | 65.12 | 4.65 | 0.00 |
| III | 33.65 | 56.09 | 4.53 | 5.97 |
| IV | 41.38 | 54.17 | 1.43 | 0.07 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, C.; Sun, X.; Liu, D.; Yang, J. Laboratory Study on Rockburst Control by Step Method in Deep Tunnel. Appl. Sci. 2025, 15, 3853. https://doi.org/10.3390/app15073853
Ren C, Sun X, Liu D, Yang J. Laboratory Study on Rockburst Control by Step Method in Deep Tunnel. Applied Sciences. 2025; 15(7):3853. https://doi.org/10.3390/app15073853
Chicago/Turabian StyleRen, Chao, Xiaoming Sun, Dongqiao Liu, and Jinkun Yang. 2025. "Laboratory Study on Rockburst Control by Step Method in Deep Tunnel" Applied Sciences 15, no. 7: 3853. https://doi.org/10.3390/app15073853
APA StyleRen, C., Sun, X., Liu, D., & Yang, J. (2025). Laboratory Study on Rockburst Control by Step Method in Deep Tunnel. Applied Sciences, 15(7), 3853. https://doi.org/10.3390/app15073853






