Exploring Chemical Catalytic Mechanisms for Enhancing Bonding Energy in Direct Silicon Dioxide Wafer Bonding
Abstract
:Featured Application
Abstract
1. Introduction
2. Materials and Methods
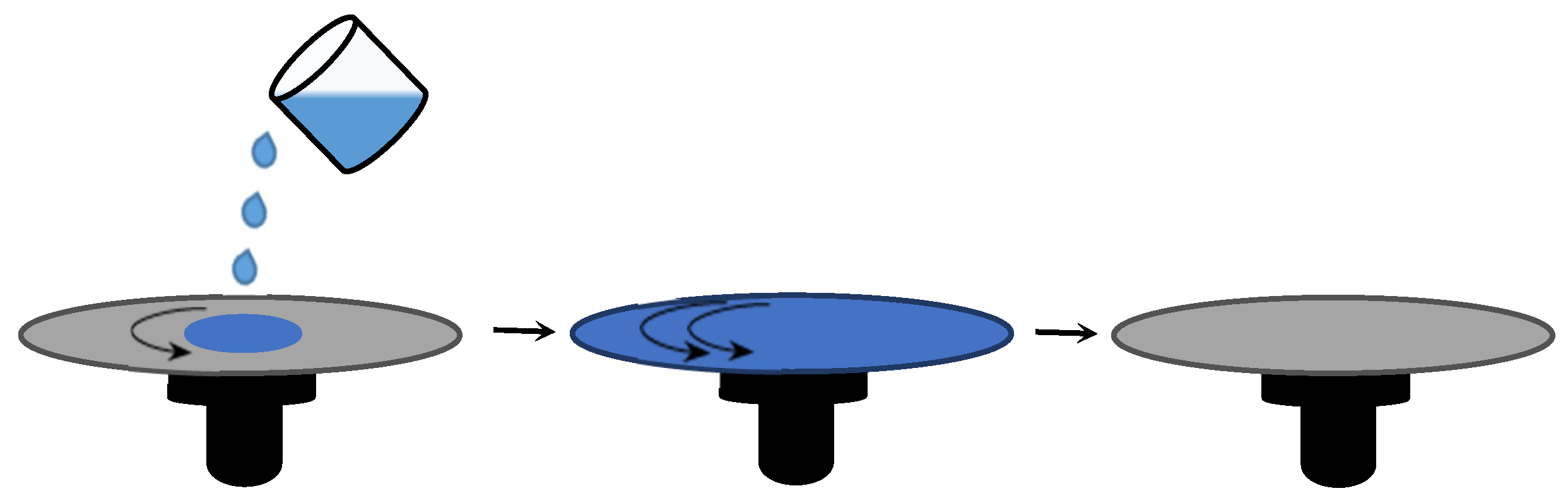
2.1. Adsorption of Chemical Species and Wafer Bonding
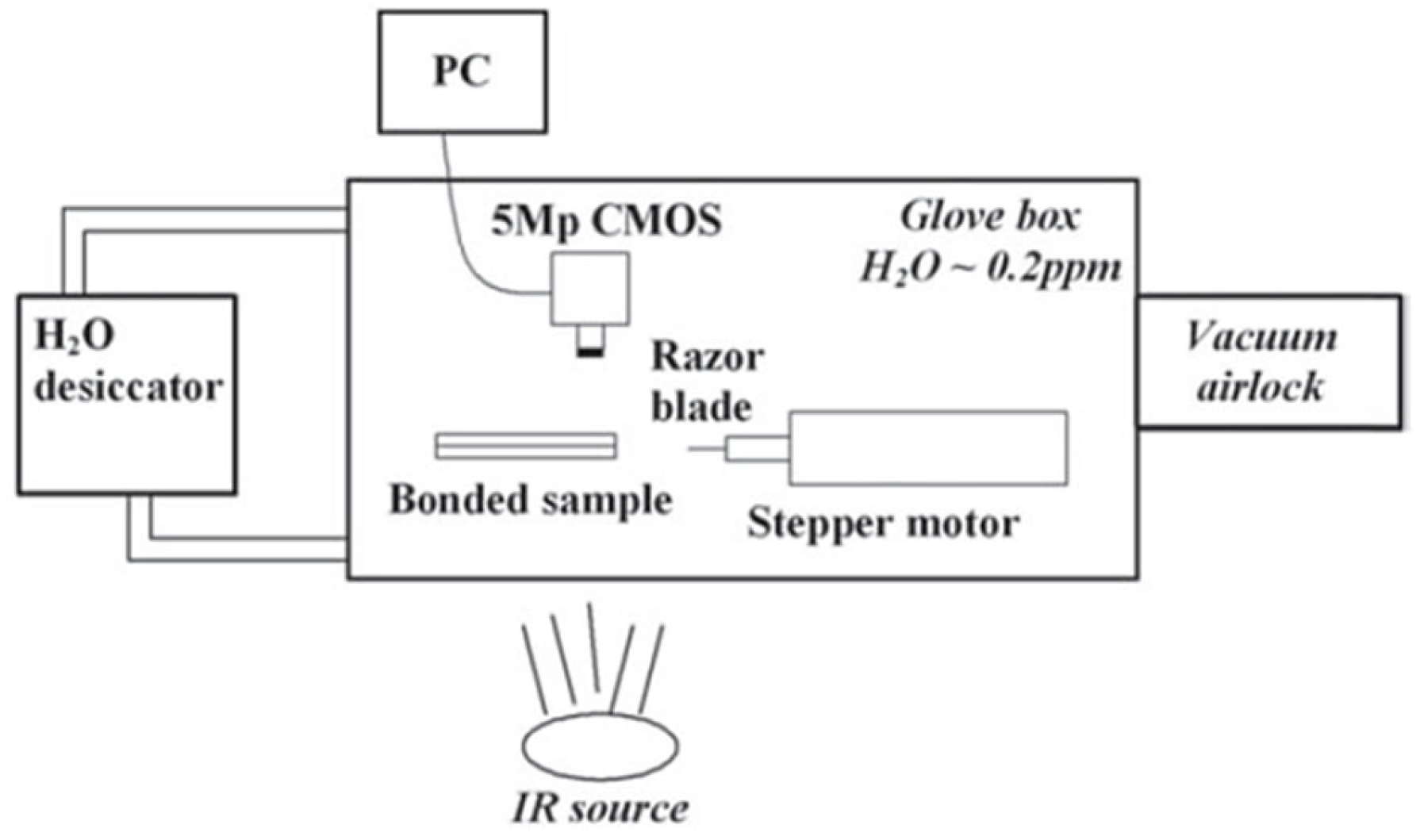
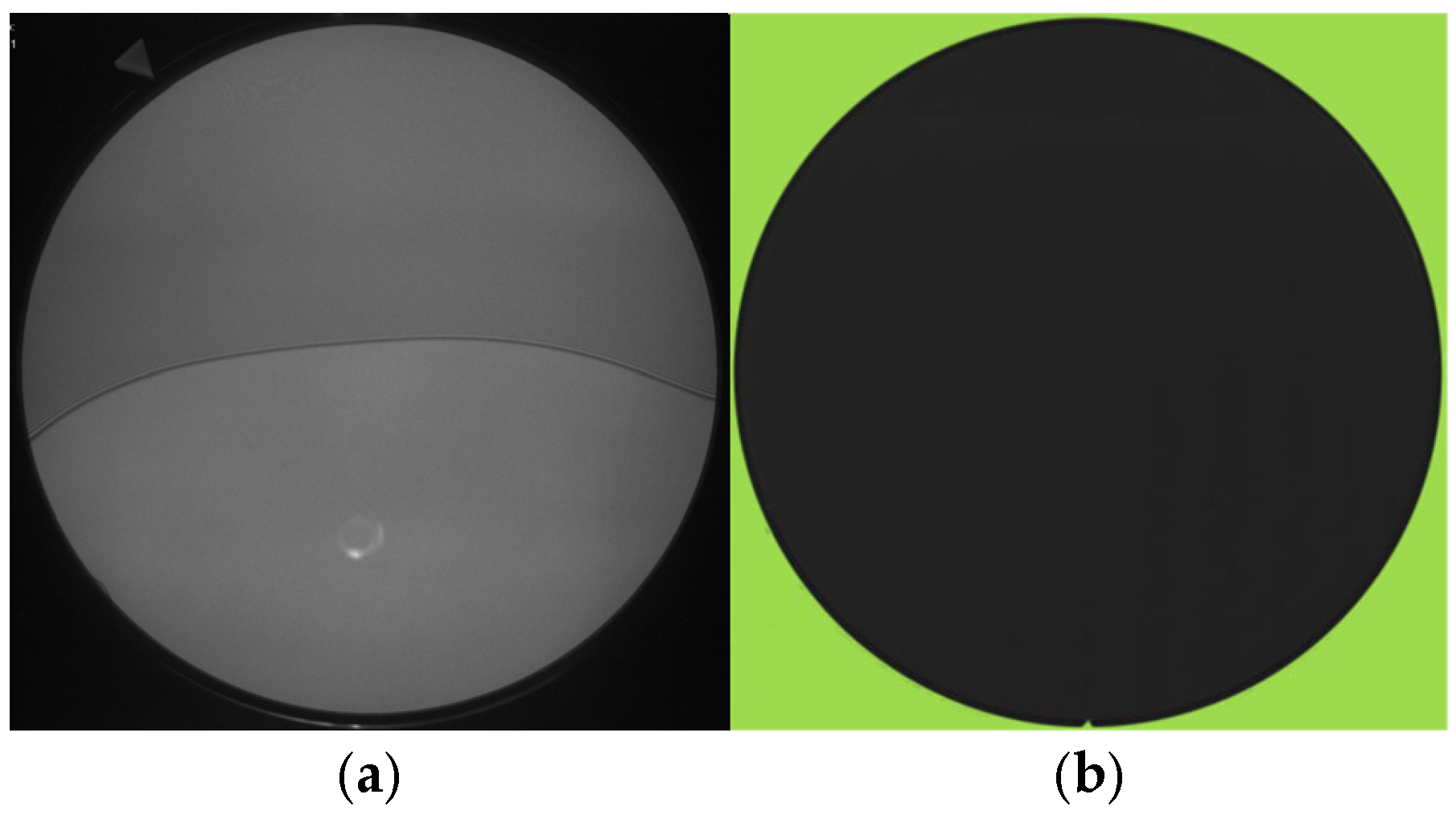
2.2. Bonding Energy Measurement
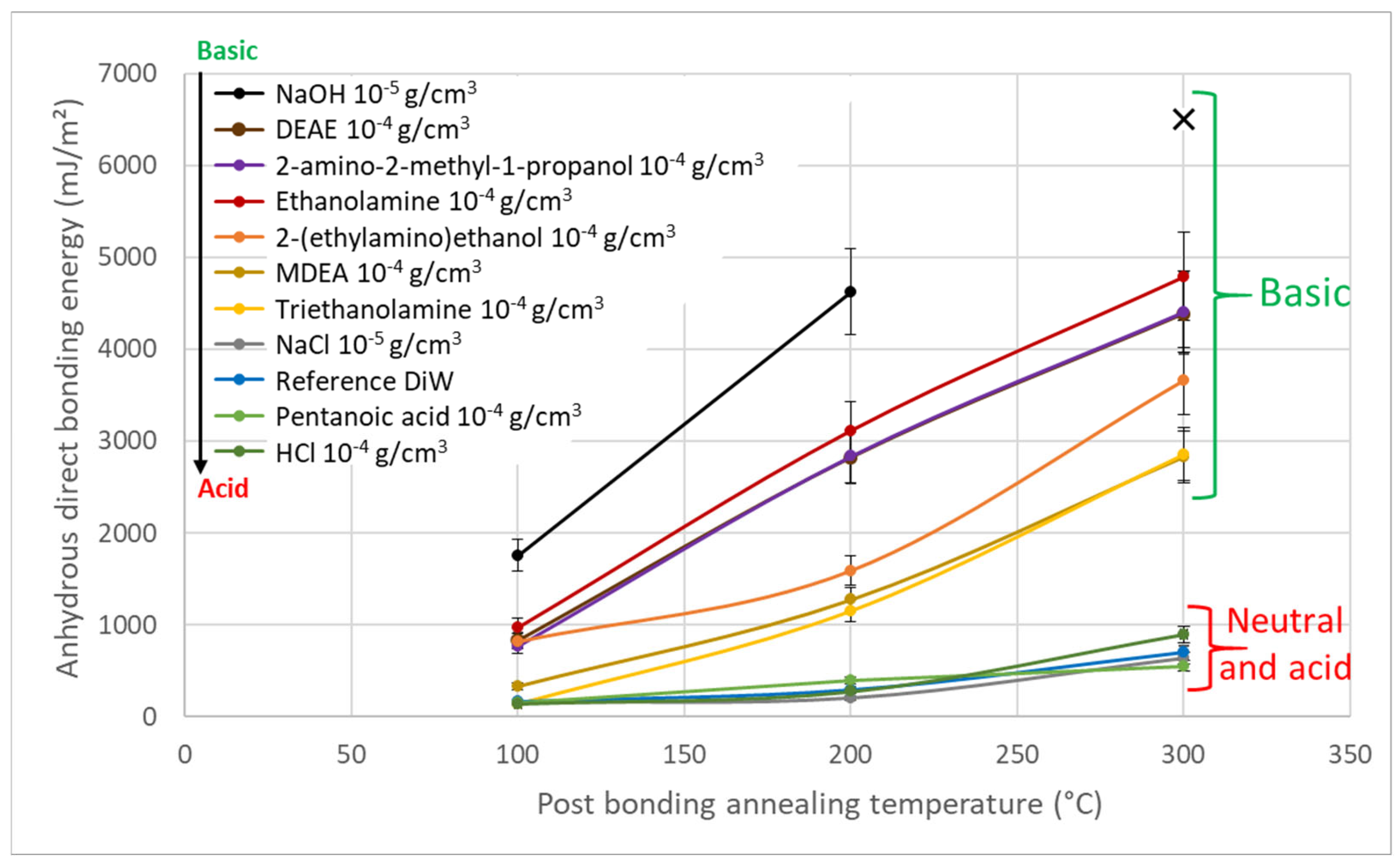
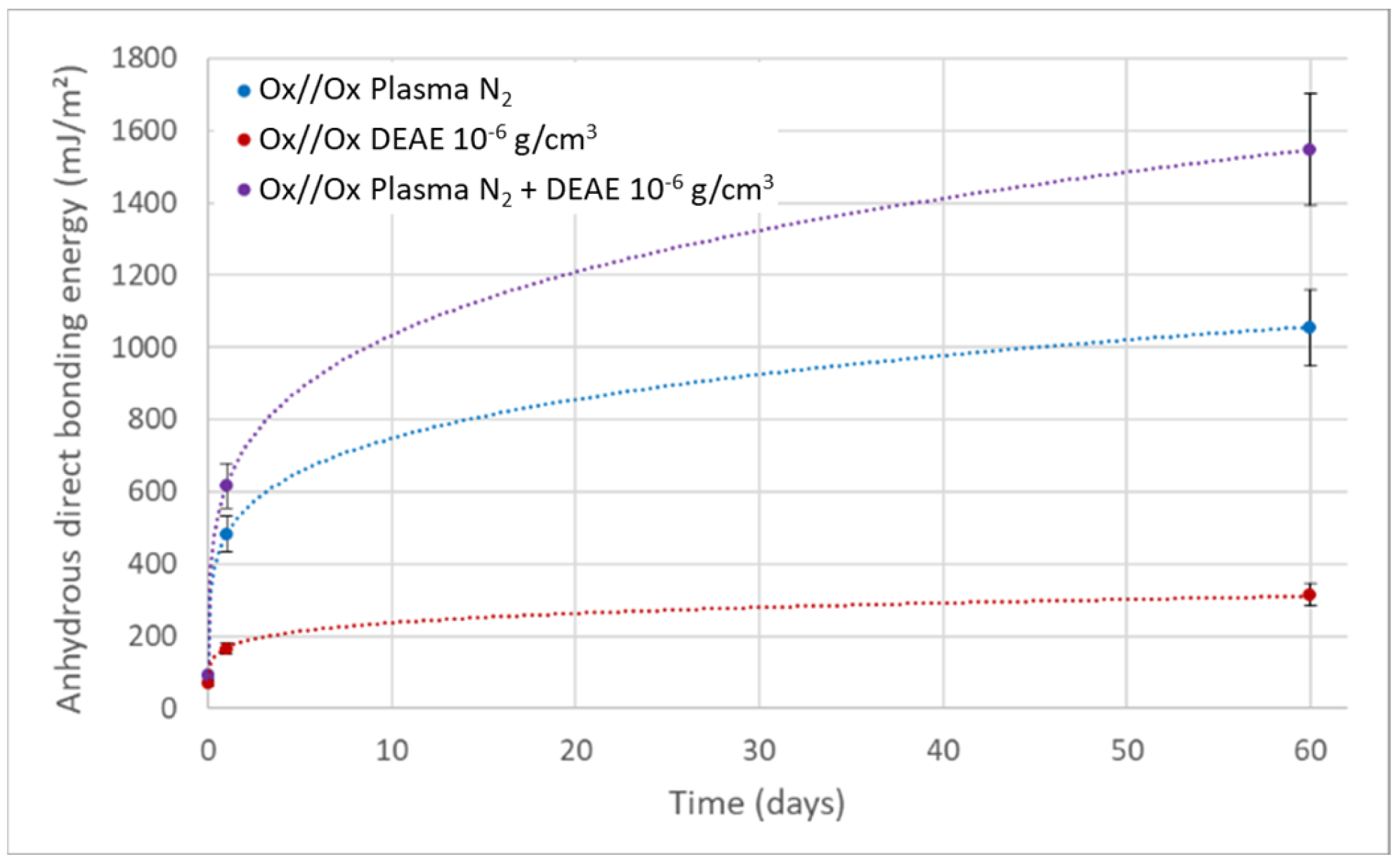
3. Results
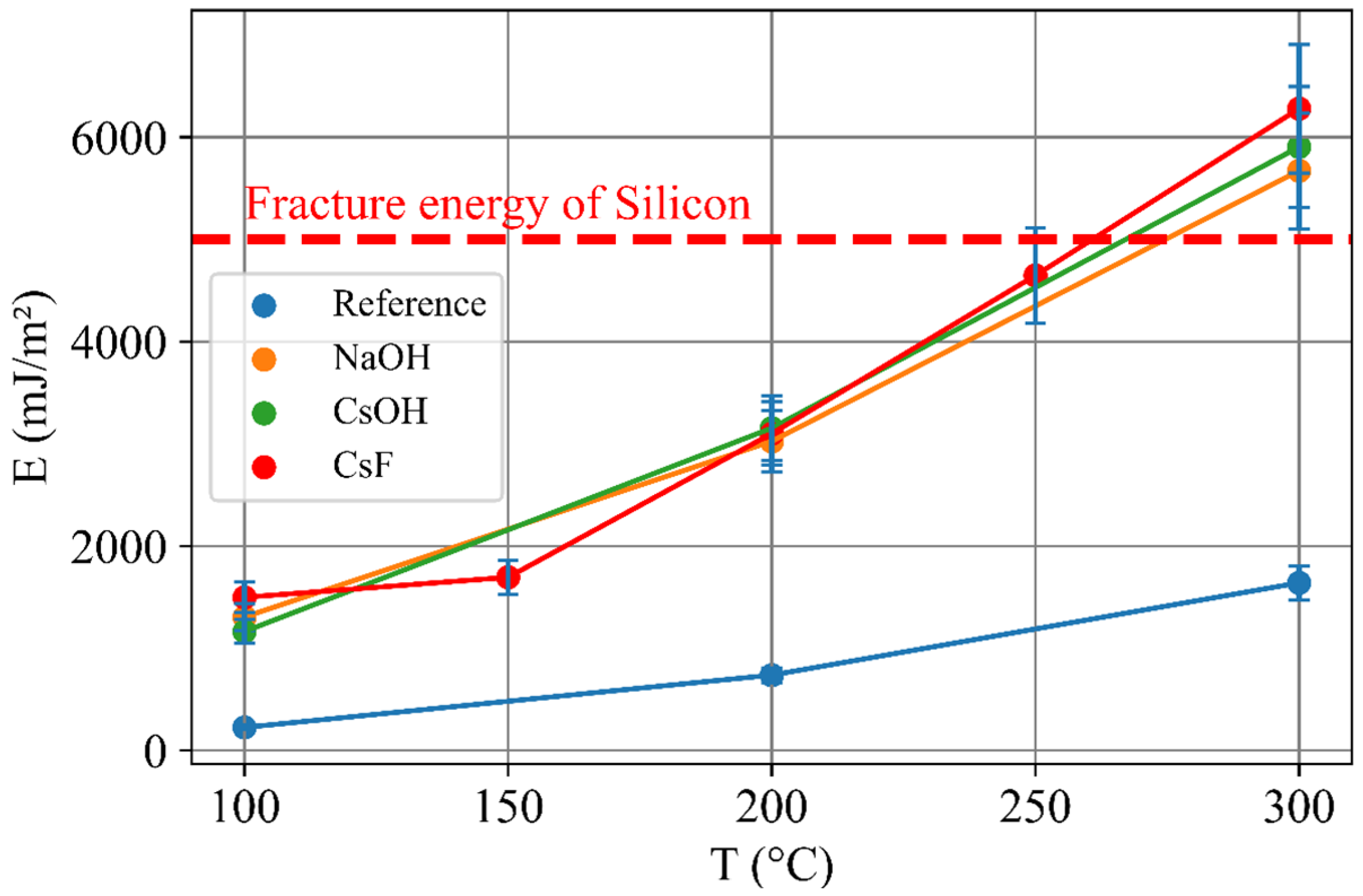
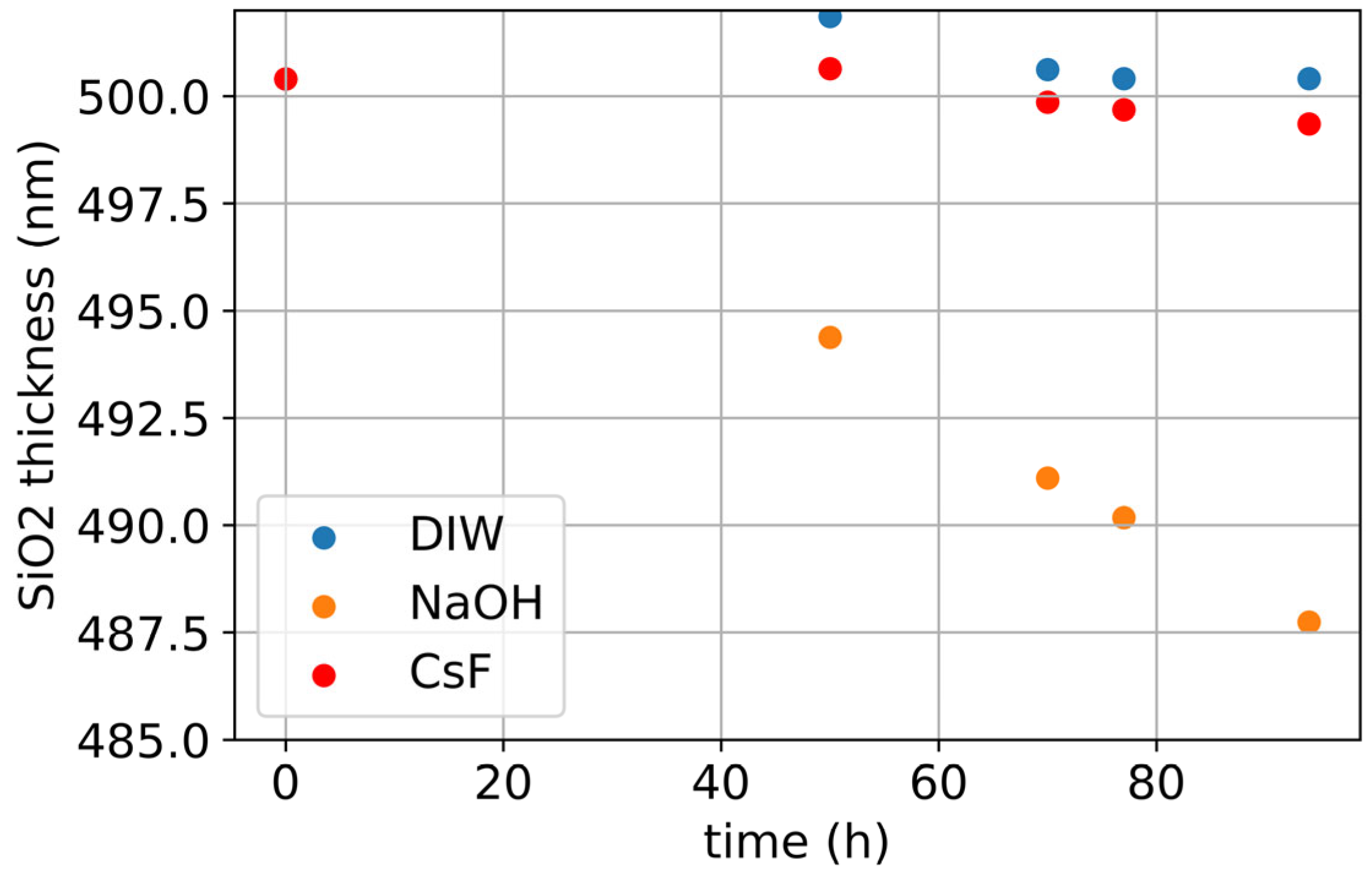
4. Discussion
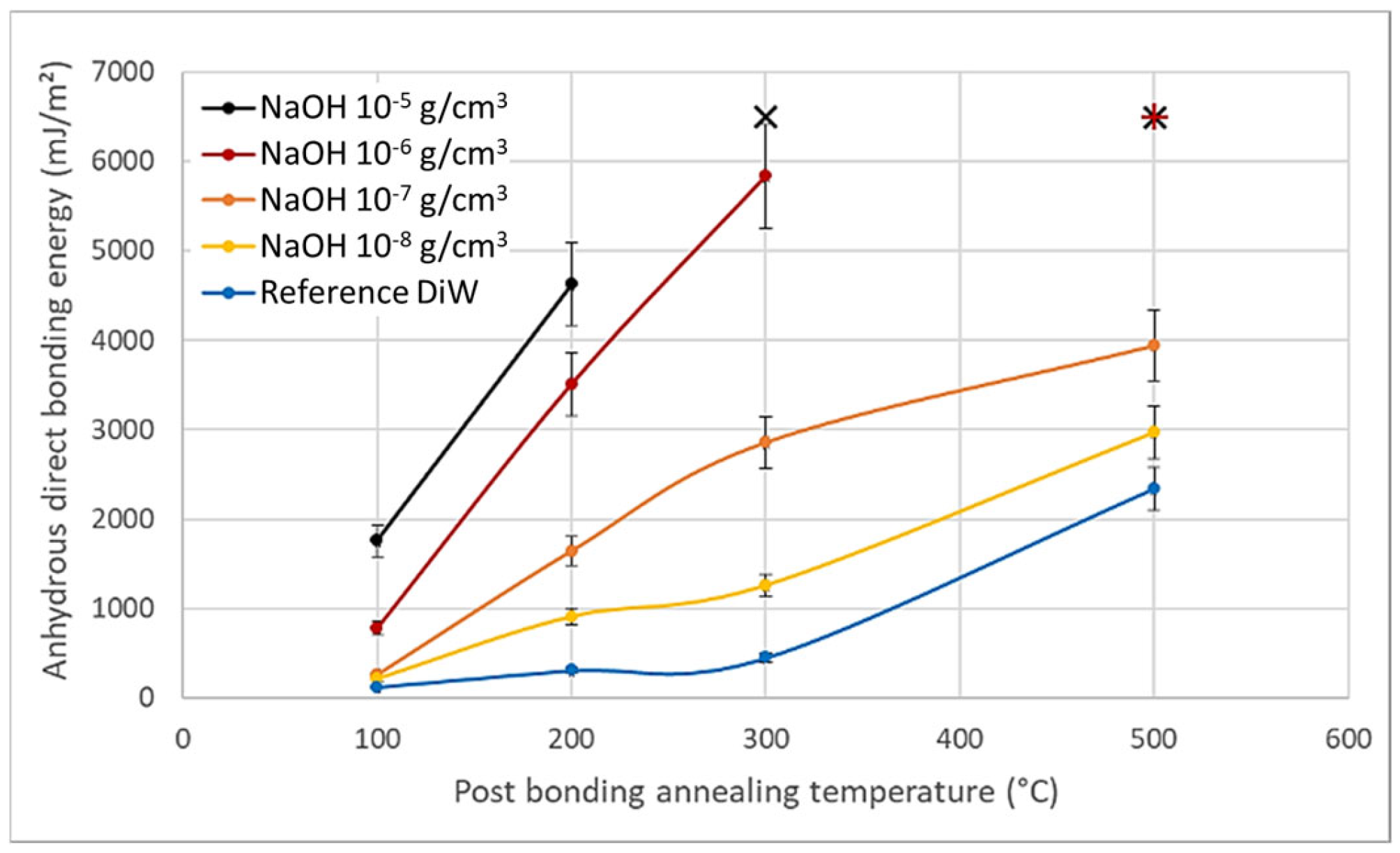
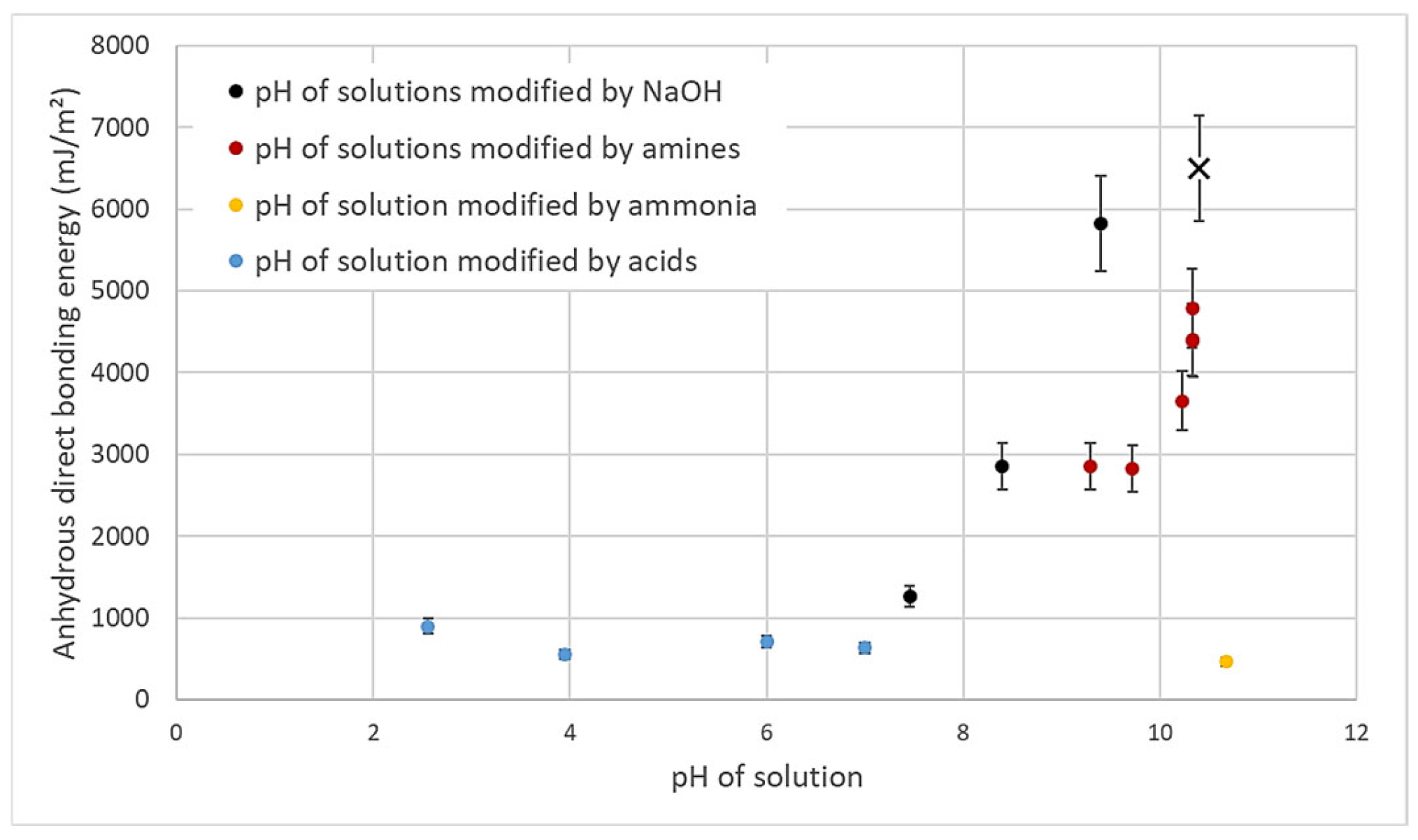
- According to the model of Rieutord et al., direct bonding relies heavily on the contact of asperities [50]. For hydrophilic bonding, at room temperature, wafer adhesion primarily results from capillary bridges, hydrogen bonds and van der Waals forces [6]. Even at room temperature, occasional covalent bonds may also form at contact points (asperity summits), accounting for the observed increase in bonding energy under specific circumstances at room temperature [39]. OH− ions are known to speed up the dynamics of siloxane bond formation by condensation of silanols, as observed e.g., in silica gel formation processes [49]. In silanol condensation, OH− play the role of nucleophilic agent. Increasing the pH will then increase the silanol condensation kinetics.It is indeed widely recognized that OH− ions can initiate nucleophilic attacks on surface Si atoms, resulting in the formation of siloxane bonds [51], as shown in Equations (2) and (3).In presence of SiO−, the formation of siloxanes bonds is favored (Equation (6)).As obvious from the equilibrium between Equations (5) and (6), OH− ions directly catalyze the condensation reaction of silanols into covalent siloxane bonds, resulting in a bonding energy increase.
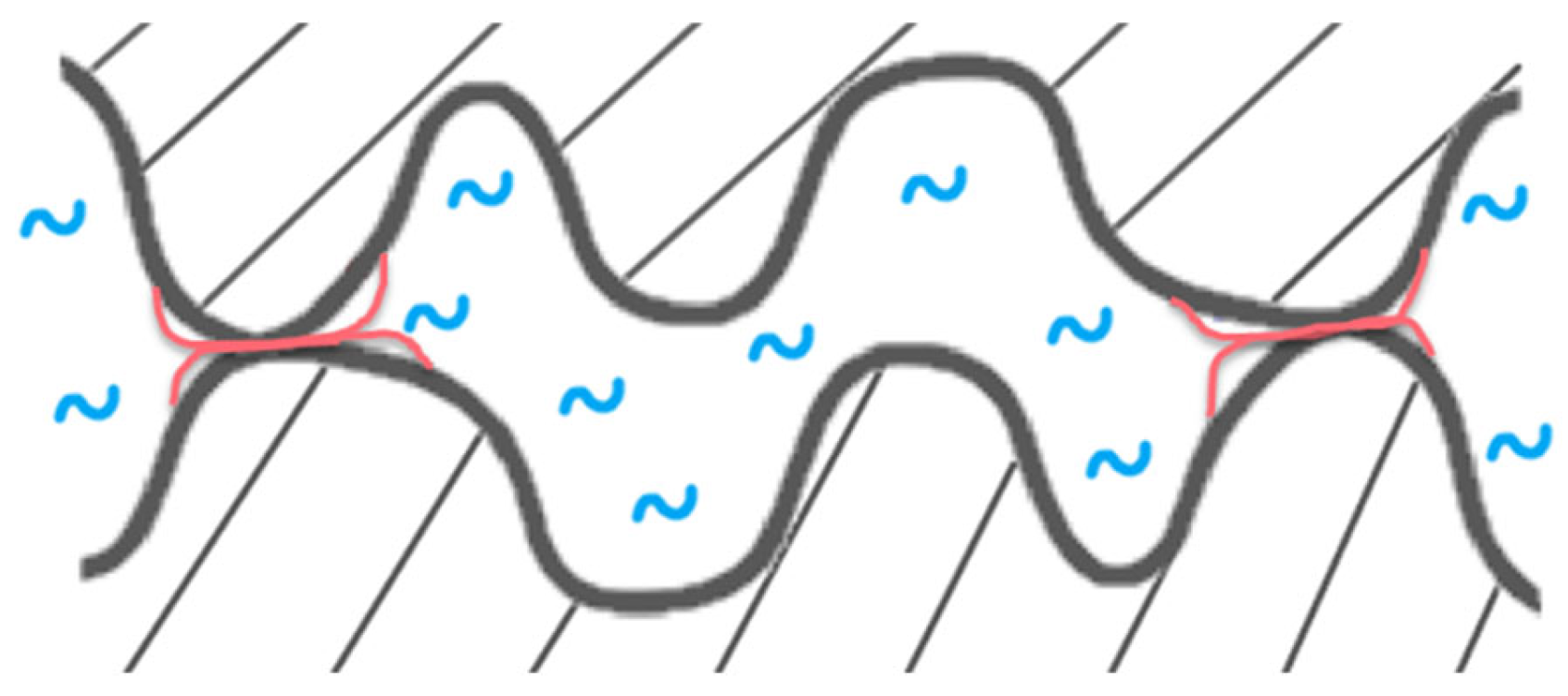
- Based on the mechanism proposed by Fournel et al. [39], during annealing, water molecules trapped at the bonding interface hydrolyze silicon dioxide asperities. As the annealing progresses, water molecules infiltrate the oxide asperities volume and break certain silanol bonds. Consequently, asperities become more viscoplastic and are flattened, enabling the formation of new interfacial covalent bonds and thereby increasing the bonding energy (see Figure 11).Extensive research has been conducted on the exacerbation of silica and quartz hydrolysis under alkaline conditions [52,53]. Hydrolysis even intensifies with higher temperatures. The incorporation of basic molecules, which change the pH of interfacial water, increases hydrolysis mechanism described above and explains the bonding energy enhancement. This influence becomes even more pronounced during post-bonding anneals. Notably, the impact of alkaline ions on silica hydrolysis has also been extensively explored [54,55]. In addition to pH influence, Na+ ions could also enhance the silica hydrolysis. However, as shown by Callagon La Plante et al. [56], the influence of a high pH (≥10) predominates over the type of cation influence. The cation influence might then be neglected for the moment.
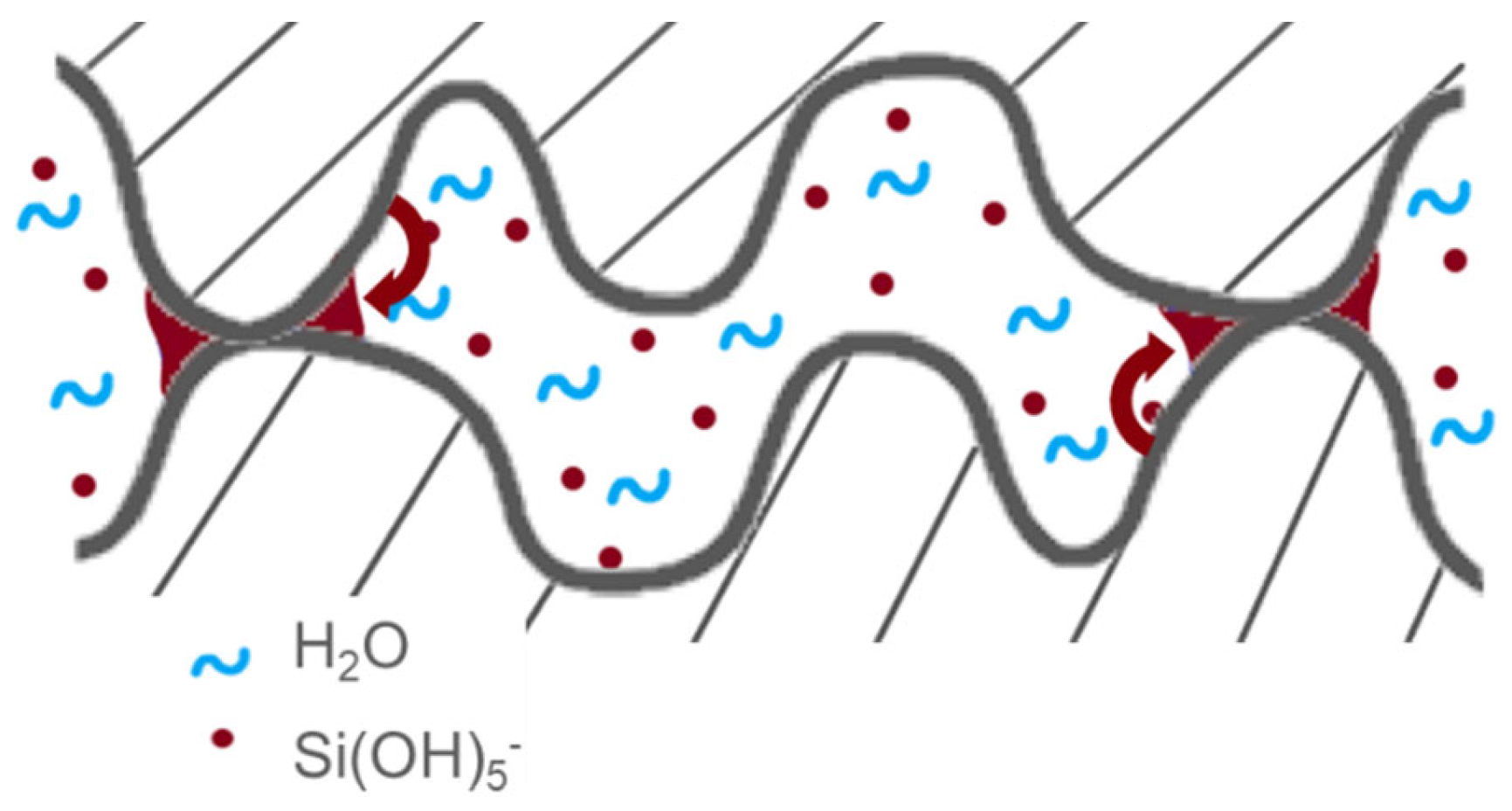
- Another mechanism could be proposed suggesting that bonding energy enhancement can be attributed to silica dissolution and immediate condensation on the bonded asperity surface. It is well-established that OH− ions attack and dissolve silica surface, as described in Equation (4) [57]:A portion of the silica is dissolved increasing species in the interfacial water. These silicate ions subsequently form (Equation (5)):Subsequently, can change into siloxane chains, as described in Equation (6):Given that the bonding interface is a confined system (interface width ≈ 1 nm), the silicate compound could condense elsewhere at the interface [58]. In our context, it is conceivable that condensation is more efficient around contact points, potentially due to an increased number of binding sites, thereby resulting in higher bonding energies (see Figure 12). These contact points experience compression (approximately 100 bars [50]), which could explain the localization of condensation to mitigate elastic energy. Enlarging the contact point will reduce the compression pressure inside the asperities induced by van der Waals. This will reduce the interfacial elastic mechanical energy.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Barth, P.W. Silicon Fusion Bonding for Fabrication of Sensors, Actuators and Microstructures. Sens. Actuators Phys. 1990, A, 919–926. [Google Scholar]
- Haisma, J.; Hattu, N.; Pulles, J.T.C.M.; Steding, E.; Vervest, J.C.G. Direct Bonding and Beyond. Appl. Opt. 2007, 46, 6793. [Google Scholar] [CrossRef]
- Plößl, A.; Kräuter, G. Wafer Direct Bonding: Tailoring Adhesion between Brittle Materials. Mater. Sci. Eng. R Rep. 1999, 25, 1–88. [Google Scholar]
- Tong, Q.-Y.; Gösele, U. Semiconductor Wafer Bonding: Science and Technology; The Electrochemical Society Series; John Wiley: New York, NY, USA, 1999; ISBN 978-0-471-57481-1. [Google Scholar]
- Bruel, M. Silicon on Insulator Material Technology. Electron. Lett. 1995, 31, 1201–1202. [Google Scholar] [CrossRef]
- Radisson, D. Direct Bonding of Patterned Surfaces. Ph.D. Thesis, Université de Grenoble, Grenoble, France, 2014. Available online: https://tel.archives-ouvertes.fr/tel-01362940/document (accessed on 24 March 2025).
- Grierson, D.; Turner, K.T. Characterization of Hysteresis of Surface Energy in Room-Temperature Direct Bonding Processes. ECS Trans. 2010, 33, 573–580. [Google Scholar] [CrossRef]
- Persson, B.N.J.; Mate, C.M. Role of Humidity and Surface Roughness on Direct Wafer Bonding. Eur. Phys. J. B 2024, 97, 46. [Google Scholar] [CrossRef]
- Turner, K.T.; Spearing, S.M. Modeling of Direct Wafer Bonding: Effect of Wafer Bow and Etch Patterns. J. Appl. Phys. 2002, 92, 7658–7666. [Google Scholar] [CrossRef]
- Ip, N.; Nejadsadeghi, N.; Fonseca, C.; Kohama, N.; Motoda, K. Multi-Physics Simulation of Wafer-to-Wafer Bonding Dynamics. In Proceedings of the 2022 IEEE 72nd Electronic Components and Technology Conference (ECTC), San Diego, CA, USA, 31 May–3 June 2022; pp. 502–506. [Google Scholar]
- Zhao, R.R.; Turner, K.T.; Bassani, J.L. Exploiting Interface Patterning for Adhesion Control. J. Mech. Phys. Solids 2022, 160, 104740. [Google Scholar] [CrossRef]
- Majidi, C.; Adams, G.G. A Simplified Formulation of Adhesion Problems with Elastic Plates. Proc. R. Soc. Math. Phys. Eng. Sci. 2009, 465, 2217–2230. [Google Scholar] [CrossRef]
- Maszara, W.P.; Goetz, G.; Caviglia, A.; McKitterick, J.B. Bonding of Silicon Wafers for Silicon-on-insulator. J. Appl. Phys. 1988, 64, 4943–4950. [Google Scholar] [CrossRef]
- Vallin, Ö.; Jonsson, K.; Lindberg, U. Adhesion Quantification Methods for Wafer Bonding. Mater. Sci. Eng. R Rep. 2005, 50, 109–165. [Google Scholar] [CrossRef]
- Gillis, P.P.; Gilman, J.J. Double-Cantilever Cleavage Mode of Crack Propagation. J. Appl. Phys. 1964, 35, 647–658. [Google Scholar]
- El-Zein, M.S.; Reijsnider, K.L. Evaluation of GIC in a DCB Specimen Using an Anisotropic Solution. J. Compos. Technol. Res. 1988, 10, 151–155. [Google Scholar]
- Masteika, V.; Rogers, T.; Santilli, R.; Fraser, S.; Aioubi, M.Y.A. Automatic Maszara Testing Jig. In Proceedings of the 2017 5th International Workshop on Low Temperature Bonding for 3D Integration (LTB-3D), Tokyo, Japan, 16–18 May 2017; p. 62. [Google Scholar]
- Ko, C.-T.; Chen, K.-N. Low Temperature Bonding Technology for 3D Integration. Microelectron. Reliab. 2012, 52, 302–311. [Google Scholar] [CrossRef]
- Kang, Q.; Wang, C.; Zhou, S.; Li, G.; Lu, T.; Tian, Y.; He, P. Low-Temperature Co-Hydroxylated Cu/SiO2 Hybrid Bonding Strategy for a Memory-Centric Chip Architecture. ACS Appl. Mater. Interfaces 2021, 13, 38866–38876. [Google Scholar] [CrossRef]
- Schmidt, R.; Schwarz, C.; Tamer, E.; Dieter, S. Optimization of the Copper Microstructure to Improve Copper-to-Copper Direct Bonding. IMAPSource Proc. 2023, 2022, 000280–000286. [Google Scholar] [CrossRef]
- Chiu, W.-L.; Lee, O.-H.; Kuo, T.-Y.; Lo, J.Y.-J.; Shih, C.-L.; Chiu, H.-Y.; Chang, H.-H. Low Temperature and Fine Pitch Nanocrystalline Cu/SiCN Wafer-to-Wafer Hybrid Bonding. In Proceedings of the 2023 IEEE 73rd Electronic Components and Technology Conference (ECTC), Orlando, FL, USA, 30 May–2 June 2023; pp. 1105–1109. [Google Scholar]
- Zhou, W.; Kwon, M.; Chiu, Y.; Guo, H.; Bhushan, B.; Street, B.; Parekh, K.; Singh, A. Critical Challenges with Copper Hybrid Bonding for Chip-to-Wafer Memory Stacking. In Proceedings of the 2023 IEEE 73rd Electronic Components and Technology Conference (ECTC), Orlando, FL, USA, 30 May–2 June 2023; pp. 336–341. [Google Scholar]
- Bayu-Aji, L.B.; Pirouz, P. Brittle-to-Ductile Transition Temperature in InP. Phys. Status Solidi A 2009, 207, 1190–1195. [Google Scholar] [CrossRef]
- Lee, Y.; Yang, I.; Tan, H.H.; Jagadish, C.; Karuturi, S.K. Monocrystalline InP Thin Films with Tunable Surface Morphology and Energy Band Gap. ACS Appl. Mater. Interfaces 2020, 12, 36380–36388. [Google Scholar] [CrossRef]
- Suni, T.; Henttinen, K.; Suni, I.; Makinen, J. Effects of Plasma Activation on Hydrophilic Bonding of Si and SiO2. J. Electrochem. Soc. 2002, 149, G348–G351. [Google Scholar]
- Cocheteau, N.; Maurel-Pantel, A.; Lebon, F.; Mazerolle, F.; Rosu, I.; Ait-Zaid, S.; De Larclause, I.S. Influence of Roughness on Mechanical Strength of Direct Bonded Silica and Zerodur® Glasses. Int. J. Adhes. Adhes. 2016, 68, 87–94. [Google Scholar] [CrossRef]
- Hahn, S.H.; Kim, W.; Shin, D.; Lee, Y.; Kim, S.; Choi, W.; Lim, K.; Moon, B.; Rhee, M. Contamination-Free Cu/SiCN Hybrid Bonding Process Development for Sub- Μm Pitch Devices with Enhanced Bonding Characteristics. In Proceedings of the 2023 IEEE 73rd Electronic Components and Technology Conference (ECTC), Orlando, FL, USA, 30 May–2 June 2023; pp. 1390–1396. [Google Scholar]
- Larrey, V.; Arribehaute, A.; Caulfield, B.; Acosta Alba, P.; Morales, C.; Noël, P.; Opprecht, M.; Fournel, F.; Landru, D.; Rieutord, F. Nanosecond Laser Irradiation for Interface Bonding Characterization. ECS Trans. 2023, 112, 39–49. [Google Scholar] [CrossRef]
- Calvez, A.; Fournel, F.; Larrey, V.; Eleouet, G.; Morales, C.; Rieutord, F. Impact of Amino-Alcohol Organic Molecule on Various Silicon Oxide Bondings. ECS J. Solid State Sci. Technol. 2021, 10, 064005. [Google Scholar] [CrossRef]
- Birckigt, P.; Rothhardt, C.; Zeitner, U.D.; Risse, S. Water Stress Corrosion in a Directly Bonded Borofloat Glass Interface. ECS J. Solid State Sci. Technol. 2024, 13, 123005. [Google Scholar] [CrossRef]
- Tabata, T.; Sanchez, L.; Larrey, V.; Fournel, F.; Moriceau, H. SiO2-SiO2 Die-to-Wafer Direct Bonding Interface Weakening. Microelectron. Reliab. 2020, 107, 113589. [Google Scholar] [CrossRef]
- Qi, X.; Zhou, S.; Ma, Y.; Suga, T.; Wang, C. Boosting Non-Oxide Interfacial Co/SiO2 Hybrid Bonding by Selective Surface Activation. Int. J. Extreme Manuf. 2025, 7, 035101. [Google Scholar] [CrossRef]
- Fournel, F.; Continni, L.; Morales, C.; Da Fonseca, J.; Moriceau, H.; Rieutord, F.; Barthelemy, A.; Radu, I. Measurement of Bonding Energy in an Anhydrous Nitrogen Atmosphere and Its Application to Silicon Direct Bonding Technology. J. Appl. Phys. 2012, 111, 104907. [Google Scholar] [CrossRef]
- Wiederhorn, S.M.; Freiman, S.W.; Fuller Jr, E.R.; Simmons, C.J. Effects of Water and Other Dielectrics on Crack Growth. J. Mater. Sci. 1982, 17, 3460–3478. [Google Scholar] [CrossRef]
- Ciccotti, M. Stress-Corrosion Mechanisms in Silicate Glasses. J. Phys. Appl. Phys. 2009, 42, 214006. [Google Scholar] [CrossRef]
- Martini, T.; Steinkirchner, J.; Gösele, U. The Crack Opening Method in Silicon Wafer Bonding How Useful Is It? J. Electrochem. Soc. 1997, 144, 354–357. [Google Scholar] [CrossRef]
- Bertholet, Y.; Iker, F.; Raskin, J.P.; Pardoen, T. Steady-State Measurement of Wafer Bonding Cracking Resistance. Sens. Actuators Phys. 2004, 110, 157–163. [Google Scholar] [CrossRef]
- Iwata, T.; Fuse, J.; Yoshihara, Y.; Kondo, Y.; Sano, M.; Inoue, F. Water Stress Corrosion at Wafer Bonding Interface during Bond Strength Evaluation. Mater. Sci. Semicond. Process. 2024, 184, 108820. [Google Scholar] [CrossRef]
- Fournel, F.; Martin-Cocher, C.; Radisson, D.; Larrey, V.; Beche, E.; Morales, C.; Delean, P.A.; Rieutord, F.; Moriceau, H. Water Stress Corrosion in Bonded Structures. ECS J. Solid State Sci. Technol. 2015, 4, P124–P130. [Google Scholar] [CrossRef]
- Fujino, M.; Takahashi, K.; Kikuchi, K.; Miyata, N.; Yoshida, T.; Miyazaki, T. Interfacial Analysis of Plasma Fusion Bonded Interfaces under Various Bonding Conditions by Neutron Reflectometry. ECS Trans. 2020, 98, 11–16. [Google Scholar] [CrossRef]
- Huang, R.; Lan, T.; Li, C.; Wang, Z. Plasma-Activated Direct Bonding at Room Temperature to Achieve the Integration of Single-Crystalline GaAs and Si Substrate. Results Phys. 2021, 31, 105070. [Google Scholar] [CrossRef]
- Takeuchi, K.; Suga, T. Quantification of Wafer Bond Strength under Controlled Atmospheres. Jpn. J. Appl. Phys. 2022, 61, SF1010. [Google Scholar] [CrossRef]
- Du, Y.; Liu, L.; Zhu, B.; Jiang, H.; Zhang, X.; Wang, C. Low-Temperature Fusion Bonding of Aluminosilicate Glass via Intermediate Water. Ceram. Int. 2024, 50, 10252–10258. [Google Scholar] [CrossRef]
- Tedjini, M. Gestion de L’eau à L’interface de Collage Silicium/Silicium. Ph.D. Thesis, Université de Grenoble, Grenoble, France, 2017. [Google Scholar]
- Behrens, S.H.; Grier, D.G. The Charge of Glass and Silica Surfaces. J. Chem. Phys. 2001, 115, 6716–6721. [Google Scholar] [CrossRef]
- Castex, A.; Broekaart, M.; Rieutord, F.; Landry, K.; Lagahe-Blanchard, C. Mechanism of Edge Bonding Void Formation in Hydrophilic Direct Wafer Bonding. ECS Solid State Lett. 2013, 2, P47–P50. [Google Scholar] [CrossRef]
- Iacovo, S.; Beyne, E.; Horiguchi, N. Bonding for Advanced 3D Logic Devices. In Proceedings of the 2024 8th International Workshop on Low Temperature Bonding for 3D Integration (LTB-3D), Nara, Japan, 30 October–1 November 2024; p. 1. [Google Scholar]
- Lin, W.; Shi, L.; Yao, Y.; Madan, A.; Pinto, T.; Zavolas, N.; Murphy, R.; Skordas, S.; Iyer, S. Low-Temperature Oxide Wafer Bonding for 3-D Integration: Chemistry of Bulk Oxide Matters. IEEE Trans. Semicond. Manuf. 2014, 27, 426–430. [Google Scholar] [CrossRef]
- Iler, R.K. The Chemistry of Silica: Solubility, Polymerization, Colloid and Surface Properties, and Biochemistry; John Wiley and Sons: Hoboken, NJ, USA, 1979. [Google Scholar]
- Rieutord, F.; Moriceau, H.; Beneyton, R.; Capello, L.; Morales, C.; Charvet, A.-M. Rough Surface Adhesion Mechanisms for Wafer Bonding. ECS Trans. 2006, 3, 205–215. [Google Scholar] [CrossRef]
- Brinker, C.J. Hydrolysis and Condensation of Silicates: Effects on Structure. J. Non-Cryst. Solids 1988, 100, 31–50. [Google Scholar] [CrossRef]
- House, W.A.; Hickinbotham, L.A. Dissolution Kinetics of Silica between 5 and 35 °C. Application of a titrimetric method. J. Chem. Soc. Faraday Trans. 1992, 88, 2021–2026. [Google Scholar]
- Crundwell, F.K. On the Mechanism of the Dissolution of Quartz and Silica in Aqueous Solutions. ACS Omega 2017, 2, 1116–1127. [Google Scholar] [CrossRef]
- Dove, P.M.; Nix, C.J. The Influence of the Alkaline Earth Cations, Magnesium, Calcium, and Barium on the Dissolution Kinetics of Quartz. Geochim. Cosmochim. Acta 1997, 61, 3329–3340. [Google Scholar] [CrossRef]
- Rimstidt, J.D.; Zhang, Y.; Zhu, C. Rate Equations for Sodium Catalyzed Amorphous Silica Dissolution. Geochim. Cosmochim. Acta 2016, 195, 120–125. [Google Scholar] [CrossRef]
- La Plante, E.C.; Oey, T.; Hsiao, Y.-H.; Perry, L.; Bullard, J.W.; Sant, G. Enhancing Silicate Dissolution Kinetics in Hyperalkaline Environments. J. Phys. Chem. C 2019, 123, 3687–3695. [Google Scholar] [CrossRef]
- Elliffe, E.J.; Bogenstahl, J.; Deshpande, A.; Hough, J.; Killow, C.; Reid, S.; Robertson, D.; Rowan, S.; Ward, H.; Cagnoli, G. Hydroxide-Catalysis Bonding for Stable Optical Systems for Space. Class. Quantum Gravity 2005, 22, S257–S267. [Google Scholar] [CrossRef]
- Gouze, B.; Cambedouzou, J.; Parrès-Maynadié, S.; Rébiscoul, D. How Hexagonal Mesoporous Silica Evolves in Water on Short and Long Term: Role of Pore Size and Silica Wall Porosity. Microporous Mesoporous Mater. 2014, 183, 168–176. [Google Scholar] [CrossRef]
- Noel, P.; Larrey, V.; Tardif, S.; Rieutord, F.; Landru, D.; Fournel, F. Hydroxide and Fluoride Catalyzed Bonding Interface Closure for Low-Temperature Wafer Bonding. Jpn. J. Appl. Phys. 2025, 64, 03SP06. [Google Scholar] [CrossRef]
- Rauch, N.; Andersen, E.; Vicente-Gabás, I.G.; Duchoslav, J.; Minenkov, A.; Gasiorowski, J.; Flötgen, C.; Hingerl, K.; Groiss, H. A Model for Spectroscopic Ellipsometry Analysis of Plasma-Activated Si Surfaces for Direct Wafer Bonding. Appl. Phys. Lett. 2022, 121, 081603. [Google Scholar] [CrossRef]
- Lomonaco, Q.; Abadie, K.; Hartmann, J.-M.; Morales, C.; Noël, P.; Marion, T.; Lecouvey, C.; Papon, A.-M.; Fournel, F. Soft Surface Activated Bonding of Hydrophobic Silicon Substrates. ECS Trans. 2023, 112, 139–145. [Google Scholar] [CrossRef]


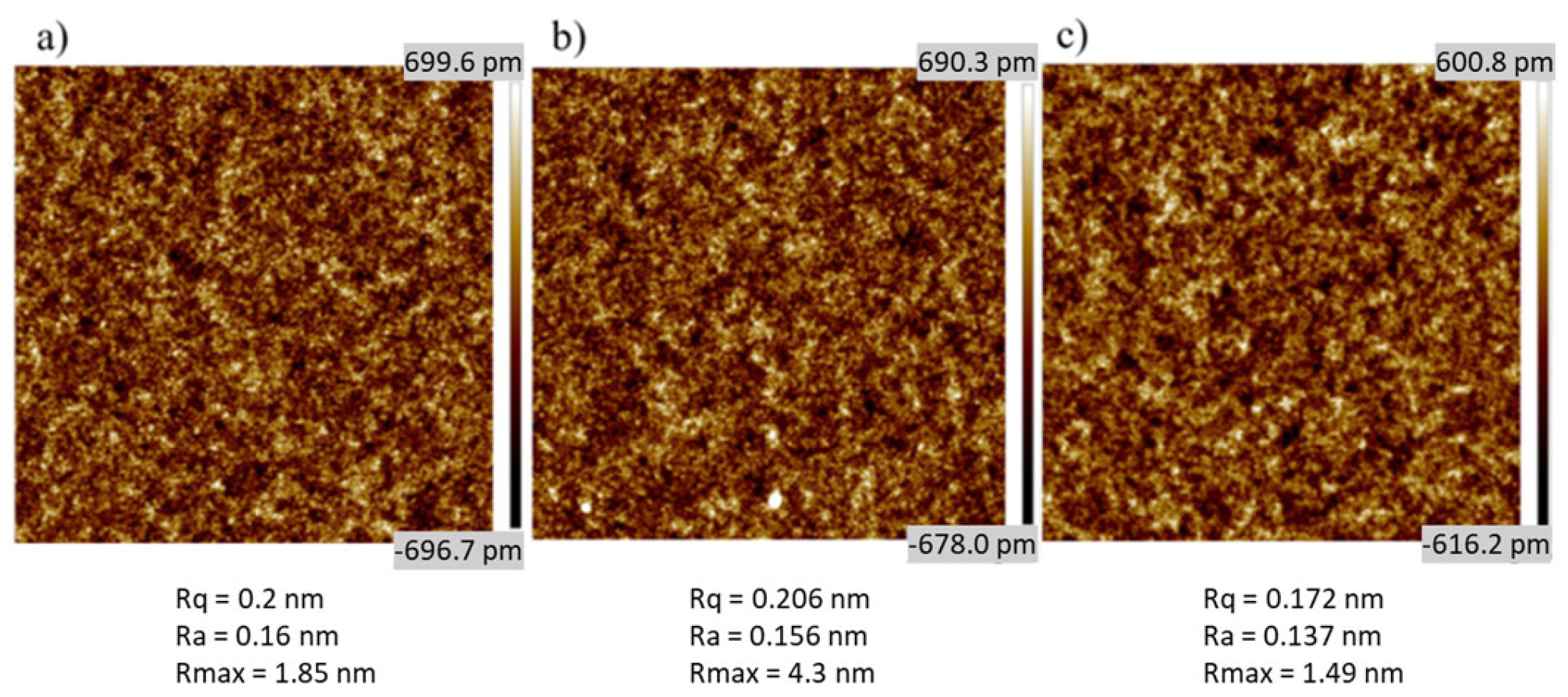
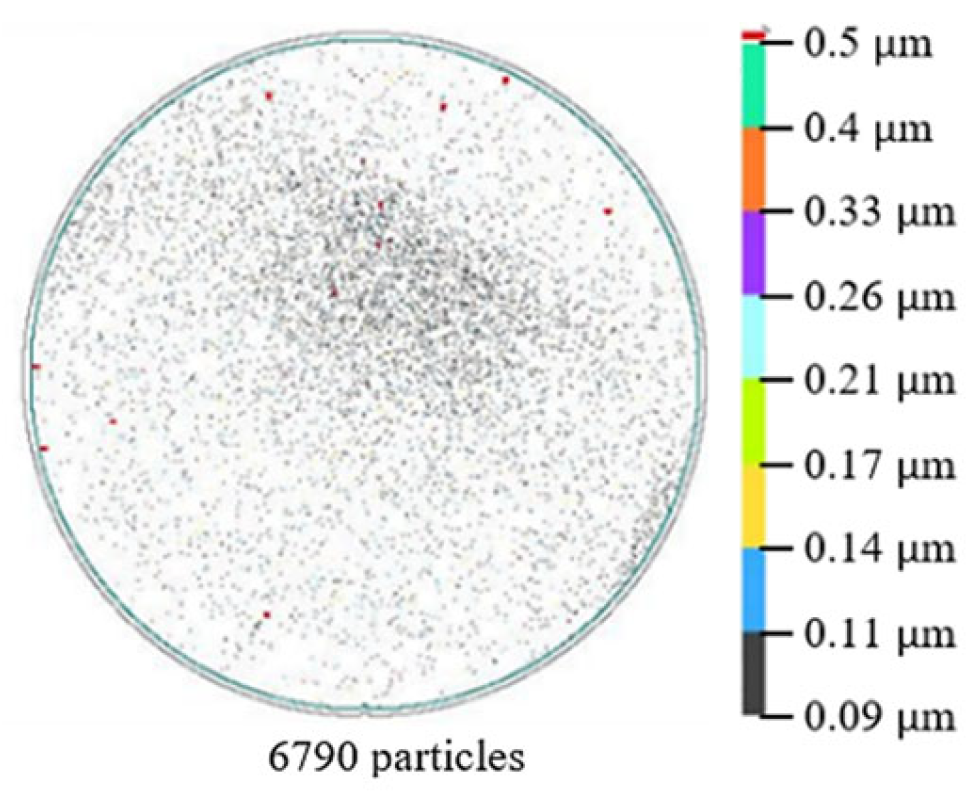
| Molecule Name | Pvap (Pa) | pKa | Formula | Molecular Weight | Supplier Merck |
|---|---|---|---|---|---|
| Hydrochloric acid | 0 | HCl | 36 | 1.09057 | |
| Pentanoic acid | 23 | 4.84 |  | 102 | 8.00821 |
| Deionized water (DiW) | 2340 | 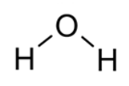 | 18 | ||
| Sodium chloride | <1 | NaCl | 58 | 59222C | |
| Triethanolamine | 1 | 7.76 | 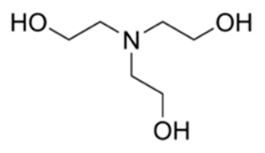 | 149 | 90279 |
| N-Methyldiethanolamine (MDEA) | <1 | 8.52 |  | 119 | 471828 |
| Ammoniac | 860 × 103 | 9.23 | 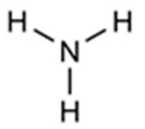 | 17 | 09859 |
| 2-ethylamino-ethanol | <1 | 9.47 |  | 89 | 471461 |
| Ethanolamine | 50 | 9.5 |  | 61 | 15014 |
| 2-amino-2-methyl-1-propanol | <1 | 9.7 | 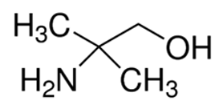 | 89 | 08578 |
| N,N-Diethylethanolamine (DEAE) | 190 | 9.87 | 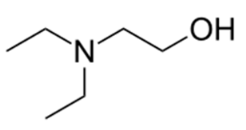 | 117 | 471321 |
| Sodium hydroxide | 0 | NaOH | 40 | 1.09137 |
| NaOH Solution Concentration | Calculated pH |
|---|---|
| 10−5 g/cm3 | 10.4 |
| 10−6 g/cm3 | 9.4 |
| 10−7 g/cm3 | 8.4 |
| 10−8 g/cm3 | 7.4 |
| Dispense Solution | Na+ Concentration on Wafer Surface (at/cm2) |
|---|---|
| H2O | <2 × 1011 |
| NaOH 10−8 g/cm3 | <2 × 1011 |
| NaOH 10−7 g/cm3 | 2.8 × 1011 |
| NaOH 10−6 g/cm3 | 1.8 × 1012 |
| NaOH 10−5 g/cm3 | 1.2 × 1013 |
| NaOH 10−4 g/cm3 | 8.4 × 1013 |
| Dispense Solution | DEAE Concentration on Wafer Surface (molecule/cm2) |
|---|---|
| H2O | ND |
| DEAE 10−6 g/cm3 | ND |
| DEAE 10−5 g/cm3 | 9.74 × 1012 |
| DEAE 10−4 g/cm3 | 8.14 × 1013 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Calvez, A.; Larrey, V.; Noël, P.; Rieutord, F.; Fournel, F. Exploring Chemical Catalytic Mechanisms for Enhancing Bonding Energy in Direct Silicon Dioxide Wafer Bonding. Appl. Sci. 2025, 15, 3883. https://doi.org/10.3390/app15073883
Calvez A, Larrey V, Noël P, Rieutord F, Fournel F. Exploring Chemical Catalytic Mechanisms for Enhancing Bonding Energy in Direct Silicon Dioxide Wafer Bonding. Applied Sciences. 2025; 15(7):3883. https://doi.org/10.3390/app15073883
Chicago/Turabian StyleCalvez, Aziliz, Vincent Larrey, Paul Noël, François Rieutord, and Frank Fournel. 2025. "Exploring Chemical Catalytic Mechanisms for Enhancing Bonding Energy in Direct Silicon Dioxide Wafer Bonding" Applied Sciences 15, no. 7: 3883. https://doi.org/10.3390/app15073883
APA StyleCalvez, A., Larrey, V., Noël, P., Rieutord, F., & Fournel, F. (2025). Exploring Chemical Catalytic Mechanisms for Enhancing Bonding Energy in Direct Silicon Dioxide Wafer Bonding. Applied Sciences, 15(7), 3883. https://doi.org/10.3390/app15073883







