Evaluation of Hydrogen Bubble Growth on a Platinum Microelectrode Under Varying Electrical Potential
Abstract
:Featured Application
Abstract
1. Introduction
Bubble Growth Dynamics
2. Materials and Methods
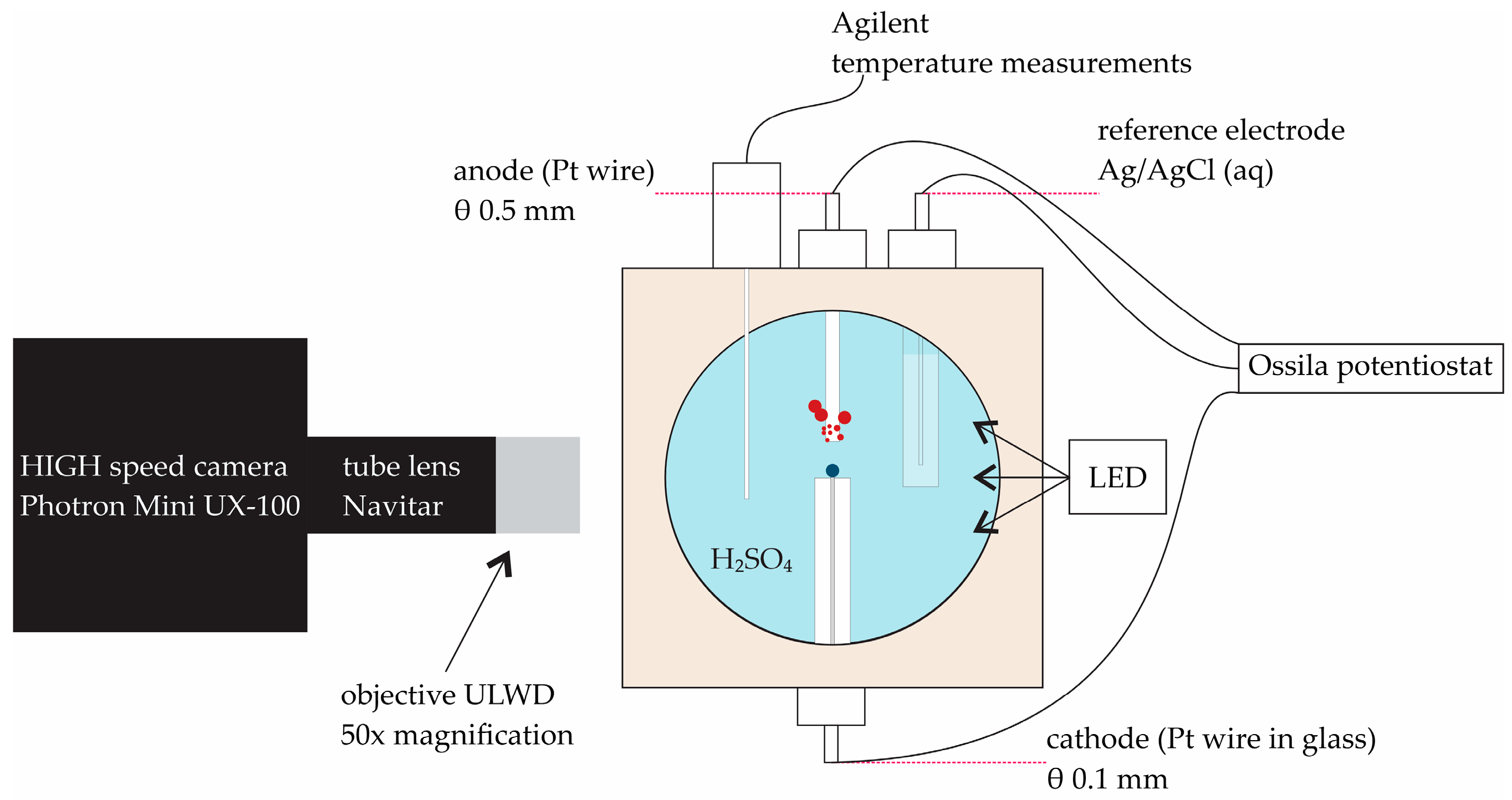
2.1. Experimental Setup
2.2. Measurement Protocol
2.3. Data Reduction
3. Results and Discussion
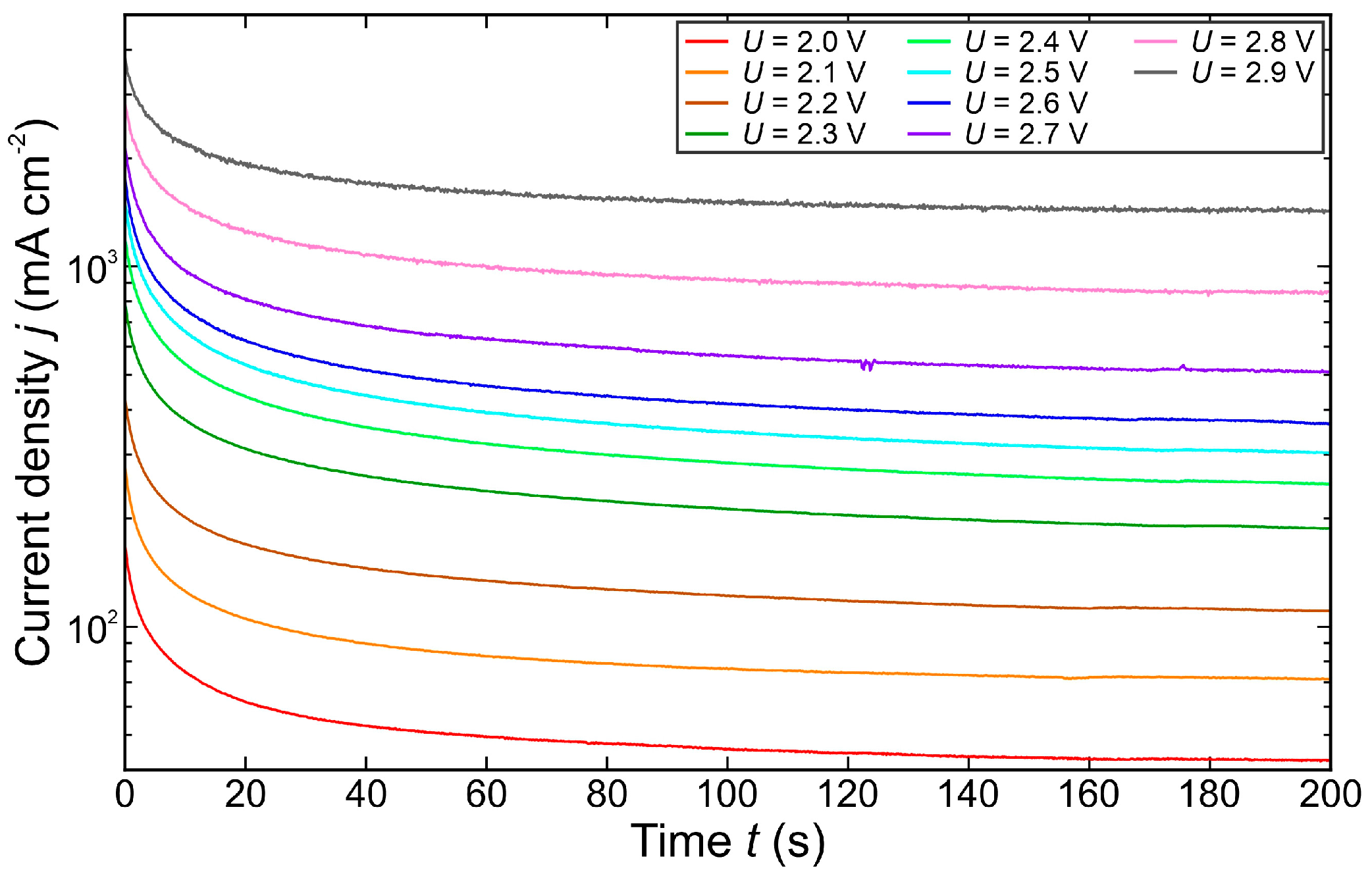
3.1. Temporal Dynamics of the Electrical Current Density
3.2. Bubble Departure Diameters and Frequencies
3.3. Bubble Growth and Departure Regimes
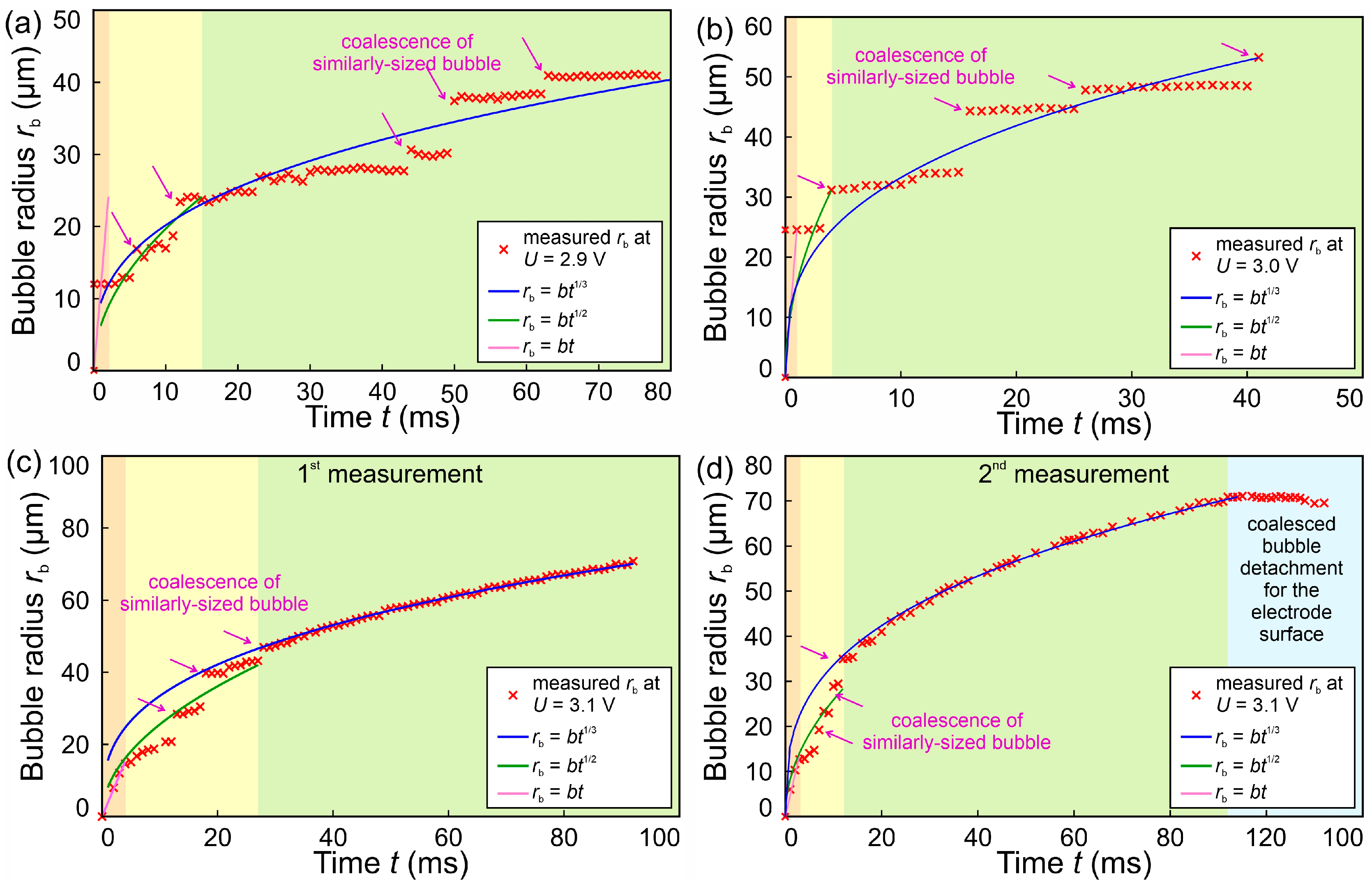
3.4. Bubble Growth Rates
4. Conclusions
- (1)
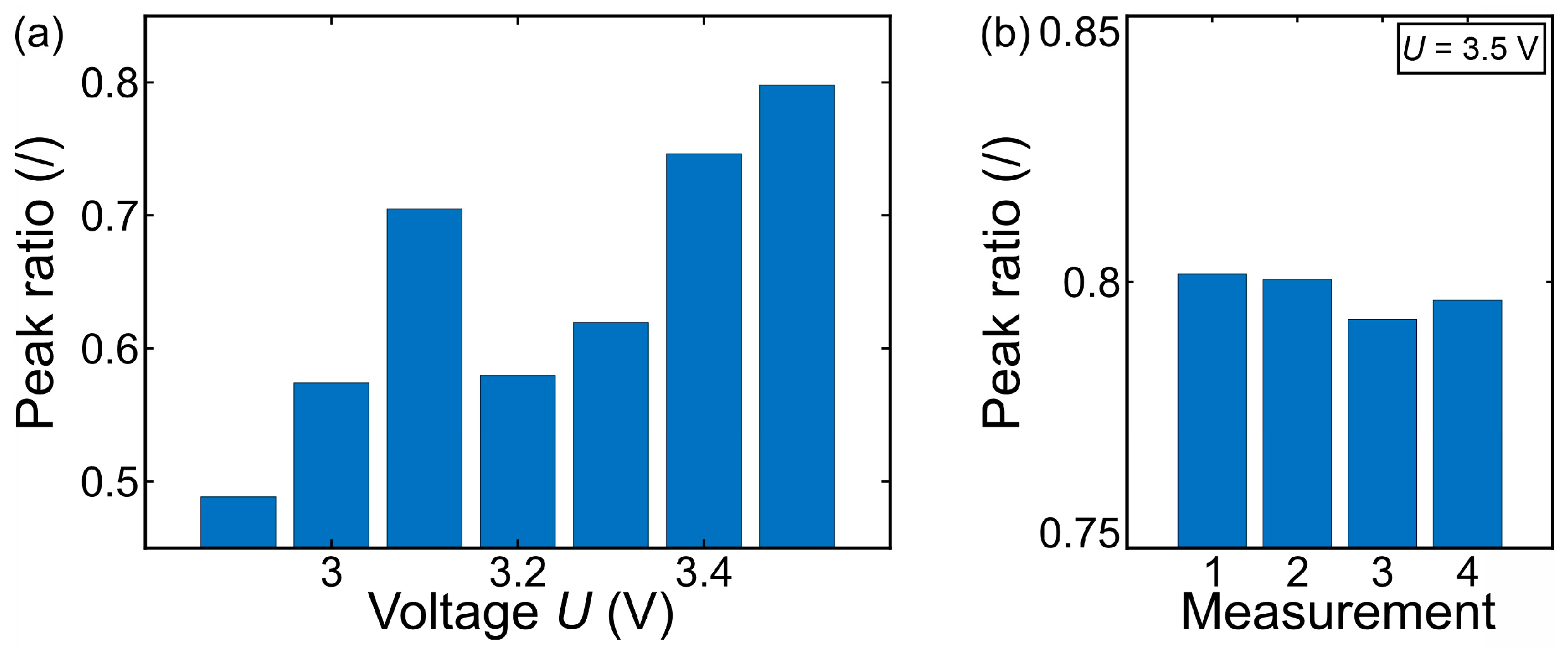
- In the range of 2.5–2.8 V, bubble coalescence was minimal, with microbubbles individually detaching from the electrode surface. As the potential was increased, electrical current density fluctuations became more pronounced. Initially, the departure frequency rose, but at very high potentials, the departure frequency decreased due to the formation of very large bubbles. In general, higher potentials lead to the detachment of a single coalesced bubble.
- (2)
- Below 3.2 V, the departure frequency was difficult to quantify due to moderate coalescence and the departure of individual bubbles. Potentiostatic measurements at these applied potentials showed that fluctuations in electrical current density were less pronounced compared to the measurements at 3.2 V and above due to limited bubble coalescence. The observed trends in bubble diameter increase over time aligned well with theoretical predictions.
- (3)
- As observed by [53,57], bubble coalescence becomes significant at higher potentials. Increased potential enhances gas production rates, leading to the formation of larger bubbles. This observation aligns well with our findings that, at very high potentials, departure frequency decreases due to larger bubble formation. Notably, our study provides a novel insight by identifying a distinct potential range (2.5–2.8 V) where microbubbles detach individually with minimal coalescence—a behavior less emphasized in previous works.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vidas, L.; Castro, R. Recent Developments on Hydrogen Production Technologies: State-of-the-Art Review with a Focus on Green-Electrolysis. Appl. Sci. 2021, 11, 11363. [Google Scholar] [CrossRef]
- Nnabuife, S.G.; Quainoo, K.A.; Hamzat, A.K.; Darko, C.K.; Agyemang, C.K. Innovative Strategies for Combining Solar and Wind Energy with Green Hydrogen Systems. Appl. Sci. 2024, 14, 9771. [Google Scholar] [CrossRef]
- Brauns, J.; Turek, T. Alkaline Water Electrolysis Powered by Renewable Energy: A Review. Processes 2020, 8, 248. [Google Scholar] [CrossRef]
- AlZohbi, G. An Analysis of the Conceptual and Functional Factors Affecting the Effectiveness of Proton-Exchange Membrane Water Electrolysis. ChemEngineering 2024, 8, 116. [Google Scholar] [CrossRef]
- Dragan, M. Closing the Loop: Solid Oxide Fuel and Electrolysis Cells Materials for a Net-Zero Economy. Materials 2024, 17, 6113. [Google Scholar] [CrossRef]
- Yang, X.; Karnbach, F.; Uhlemann, M.; Odenbach, S.; Eckert, K. Dynamics of Single Hydrogen Bubbles at a Platinum Microelectrode. Langmuir 2015, 31, 8184–8193. [Google Scholar] [CrossRef]
- Zhan, S.; Yuan, R.; Wang, X.; Zhang, W.; Yu, K.; Wang, Z.; Wang, J. Dynamics of Growth and Detachment of Single Hydrogen Bubble on Horizontal and Vertical Microelectrode Surfaces. Phys. Fluids 2023, 3, 032111. [Google Scholar] [CrossRef]
- Sepahi, F.; Verzicco, R.; Lohse, D.; Krug, D. Mass Transport at Gas-Evolving Electrodes. J. Fluid Mech. 2024, 983, A19. [Google Scholar] [CrossRef]
- Zhang, Z.; Gu, C.; Wang, K.; Yu, H.; Qiu, J.; Wang, S.; Wang, L.; Yan, D. Bubbles Management for Enhanced Catalytic Water Splitting Performance. Catalysts 2024, 14, 254. [Google Scholar] [CrossRef]
- Zhao, P.; Gong, S.; Zhang, C.; Chen, S.; Cheng, P. Roles of Wettability and Wickability on Enhanced Hydrogen Evolution Reactions. ACS Appl. Mater. Interfaces 2024, 16, 27898–27907. [Google Scholar] [CrossRef]
- Gutić, S.J.; Dobrota, A.S.; Fako, E.; Skorodumova, N.V.; López, N.; Pašti, I.A. Hydrogen Evolution Reaction-from Single Crystal to Single Atom Catalysts. Catalysts 2020, 10, 290. [Google Scholar] [CrossRef]
- Kuczyński, M.; Mikołajczyk, T.; Pierożyński, B.; Karczewski, J. Optimizing NiFe-Modified Graphite for Enhanced Catalytic Performance in Alkaline Water Electrolysis: Influence of Substrate Geometry and Catalyst Loading. Molecules 2024, 29, 4755. [Google Scholar] [CrossRef] [PubMed]
- Raeymaekers, J.; Arhar, K.; Može, M.; Colinet, P.; Golobič, I.; Steelant, J.; Vetrano, M.R. Impact of Electrode Surface Texturing on Hydrogen Bubble Dynamics during Proton Exchange Membrane Water Electrolysis. SSRN 2024. [Google Scholar] [CrossRef]
- Sota, K.; Mondal, S.; Ando, K.; Uchimoto, Y.; Nakajima, T. Nanosecond Laser Texturing of Ni Electrodes as a High-Speed and Cost-Effective Technique for Efficient Hydrogen Evolution Reaction. Int. J. Hydrogen Energy 2024, 93, 1218–1226. [Google Scholar] [CrossRef]
- Alushi, A.; Al-Musawi, A.; Kim, K.; Lee, C.Y.; Wagner, K.; Swiegers, G.F. Hydrogen Generation by Nickel Electrodes Coated with Linear Patterns of PTFE. J. Compos. Sci. 2024, 8, 368. [Google Scholar] [CrossRef]
- Cai, Z.; Li, Z.; Zhang, Y.; Wei, C.; Tian, H.; Hao, M.; Wei, X.; Yang, Z. High Repetition Rate Ultrafast Laser-Structured Nickel Electrocatalyst for Efficient Hydrogen Evolution Reaction. Adv. Photonics Nexus 2023, 2, 056009. [Google Scholar] [CrossRef]
- Ränke, F.; Baumann, R.; Voisiat, B.; Soldera, M.; Lasagni, A.F. Nano/Microstructuring of Nickel Electrodes by Combining Direct Laser Interference Patterning and Polygon Scanner Processing for Efficient Hydrogen Production. Adv. Eng. Mater. 2024, 26, 2301583. [Google Scholar] [CrossRef]
- Sangtam, B.T.; Park, H. Review on Bubble Dynamics in Proton Exchange Membrane Water Electrolysis: Towards Optimal Green Hydrogen Yield. Micromachines 2023, 14, 2234. [Google Scholar] [CrossRef]
- An, L.; Tian, Y.; Zhao, H. Research on Water Flow Control Strategy for PEM Electrolyzer Considering the Anode Bubble Effect. Energies 2025, 18, 273. [Google Scholar] [CrossRef]
- Panchenko, O.; Giesenberg, L.; Borgardt, E.; Zwaygardt, W.; Kardjilov, N.; Markötter, H.; Arlt, T.; Manke, I.; Müller, M.; Stolten, D.; et al. Influence of Stoichiometry on the Two-Phase Flow Behavior of Proton Exchange Membrane Electrolyzers. Energies 2019, 12, 350. [Google Scholar] [CrossRef]
- Zerrougui, I.; Li, Z.; Hissel, D. Investigating Bubble Impacts on PEM Electrolysis Performance through Enhanced Multiphysics Modeling. Int. J. Hydrogen Energy 2025, 98, 626–638. [Google Scholar] [CrossRef]
- Yuan, S.; Zhao, C.; Cai, X.; An, L.; Shen, S.; Yan, X.; Zhang, J. Bubble Evolution and Transport in PEM Water Electrolysis: Mechanism, Impact, and Management. Prog. Energy Combust. Sci. 2023, 96, 101075. [Google Scholar] [CrossRef]
- Park, H. Resemblance and Difference in Hydrodynamic Aspects Between Critical Current Density in Water Electrolysis and Critical Heat Flux in Boiling System. Korean J. Chem. Eng. 2025. [Google Scholar] [CrossRef]
- Park, S.; Lohse, D.; Krug, D.; Koper, M.T.M. Electrolyte Design for the Manipulation of Gas Bubble Detachment during Hydrogen Evolution Reaction. Electrochim. Acta 2024, 485, 144084. [Google Scholar] [CrossRef]
- Guo, C.; Lu, X.; Zhu, Y.; Ma, L.; Jing, D. Insight into the Electrolyte Concentration Impact on Single Hydrogen Bubble Evolution Dynamics at a Microelectrode during Electrochemical Water Splitting. Phys. Fluids 2024, 36, 073339. [Google Scholar] [CrossRef]
- Lu, X.; Yadav, D.; Wang, J.; Jing, L.; Zhu, Y.; Ma, L.; Jing, D. Hydrogen Bubble Evolution and Gas Transport Mechanism on a Microelectrode Determined by Cathodic Potential and Temperature. Phys. Fluids 2024, 36, 073302. [Google Scholar] [CrossRef]
- Duan, Z.; Xu, Q.; She, Y.; Nie, T.; Luo, X.; Wang, M. In Situ Observation and Electrical Signal Analysis of Different Bubble Detachment Modes on Microelectrode Surface. J. Electrochem. 2024, 171, 076501. [Google Scholar] [CrossRef]
- Hossain, S.S.; Bashkatov, A.; Yang, X.; Mutschke, G.; Eckert, K. Force Balance of Hydrogen Bubbles Growing and Oscillating on a Microelectrode. Phys. Rev. E 2022, 106, 035105. [Google Scholar] [CrossRef]
- Bashkatov, A.; Hossain, S.S.; Mutschke, G.; Yang, X.; Rox, H.; Weidinger, I.M.; Eckert, K. On the Growth Regimes of Hydrogen Bubbles at Microelectrodes. Phys. Chem. Chem. Phys. 2022, 24, 26738–26752. [Google Scholar] [CrossRef]
- Bashkatov, A.; Babich, A.; Hossain, S.S.; Yang, X.; Mutschke, G.; Eckert, K. H 2 Bubble Motion Reversals during Water Electrolysis. J. Fluid Mech. 2023, 958, A43. [Google Scholar] [CrossRef]
- Angulo, A.; van der Linde, P.; Gardeniers, H.; Modestino, M.; Fernández Rivas, D. Influence of Bubbles on the Energy Conversion Efficiency of Electrochemical Reactors. Joule 2020, 4, 555–579. [Google Scholar] [CrossRef]
- Zhao, X.; Ren, H.; Luo, L. Gas Bubbles in Electrochemical Gas Evolution Reactions. Langmuir 2019, 35, 5392–5408. [Google Scholar] [CrossRef] [PubMed]
- Brandon, N.P.; Kelsall, G.H. Growth Kinetics of Bubbles Electrogenerated at Microelectrodes. J. Appl. Electrochem. 1985, 15, 475–484. [Google Scholar] [CrossRef]
- Lord, R., VIII. On the Pressure Developed in a Liquid during the Collapse of a Spherical Cavity. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1917, 34, 94–98. [Google Scholar] [CrossRef]
- Sakuma, G.; Fukunaka, Y.; Matsushima, H. Nucleation and Growth of Electrolytic Gas Bubbles under Microgravity. Int. J. Hydrogen Energy 2014, 39, 7638–7645. [Google Scholar] [CrossRef]
- Fernández, D.; Maurer, P.; Martine, M.; Coey, J.M.D.; Möbius, M.E. Bubble Formation at a Gas-Evolving Microelectrode. Langmuir 2014, 30, 13065–13074. [Google Scholar] [CrossRef]
- Scriven, L.E. On the Dynamics of Phase Growth. Chem. Eng. Sci. 1959, 10, 1–13. [Google Scholar] [CrossRef]
- Westerheide, D.E.; Westwater, J.W. Isothermal Growth of Hydrogen Bubbles during Electrolysis. AIChE J. 1961, 7, 357–362. [Google Scholar] [CrossRef]
- Glas, J.P.; Westwater, J.W. Measurements of the Growth of Electrolytic Bubbles. Int. J. Heat Mass Transf. 1964, 7, 1427–1443. [Google Scholar] [CrossRef]
- Higuera, F.J. A Model of the Growth of Hydrogen Bubbles in the Electrolysis of Water. J. Fluid Mech. 2021, 927, A33. [Google Scholar] [CrossRef]
- Darby, R.; Haquet, M.S. The Dynamics of Electrolytic Hydrogen Bubble Evolution. Chem. Eng. Sci. 1973, 28, 1129–1138. [Google Scholar] [CrossRef]
- Verhaart, H.F.A.; de Jonge, R.M.; van Stralen, S.J.D. Growth Rate of a Gas Bubble during Electrolysis in Supersaturated Liquid. Int. J. Heat Mass Transf. 1980, 23, 293–299. [Google Scholar] [CrossRef]
- Bashkatov, A.; Hossain, S.S.; Yang, X.; Mutschke, G.; Eckert, K. Oscillating Hydrogen Bubbles at Pt Microelectrodes. Phys. Rev. Lett. 2019, 123, 214503. [Google Scholar] [CrossRef] [PubMed]
- Darband, G.B.; Aliofkhazraei, M.; Shanmugam, S. Recent Advances in Methods and Technologies for Enhancing Bubble Detachment during Electrochemical Water Splitting. Renew. Sustain. Energy Rev. 2019, 114, 109300. [Google Scholar] [CrossRef]
- Fritz, W. Maximum Volume of Vapor Bubbles. Phys. Zeitschr. 1935, 36, 379–384. [Google Scholar]
- Duhar, G.; Colin, C. Dynamics of Bubble Growth and Detachment in a Viscous Shear Flow. Phys. Fluids 2006, 18, 077101. [Google Scholar] [CrossRef]
- Vogt, H.; Balzer, R.J. The Bubble Coverage of Gas-Evolving Electrodes in Stagnant Electrolytes. Electrochim. Acta 2005, 50, 2073–2079. [Google Scholar] [CrossRef]
- Lu, Z.; Zhang, L.; Iwata, R.; Wang, E.N.; Grossman, J.C. Transport-Based Modeling of Bubble Nucleation on Gas Evolving Electrodes. Langmuir 2020, 36, 15112–15118. [Google Scholar] [CrossRef]
- Zhao, P.; Zhang, C.; Gong, S. Size Ranges of Effective Nucleation Cavities on Gas-Evolving Surfaces. Langmuir 2023, 39, 16101–16110. [Google Scholar] [CrossRef]
- Nouri-Khorasani, A.; Tabu Ojong, E.; Smolinka, T.; Wilkinson, D.P. Model of Oxygen Bubbles and Performance Impact in the Porous Transport Layer of PEM Water Electrolysis Cells. Int. J. Hydrogen Energy 2017, 42, 28665–28680. [Google Scholar] [CrossRef]
- Iwata, R.; Zhang, L.; Wilke, K.L.; Gong, S.; He, M.; Gallant, B.M.; Wang, E.N. Bubble Growth and Departure Modes on Wettable/Non-Wettable Porous Foams in Alkaline Water Splitting. Joule 2021, 5, 887–900. [Google Scholar] [CrossRef]
- Iwata, R.; Zhang, L.; Lu, Z.; Gong, S.; Du, J.; Wang, E.N. How Coalescing Bubbles Depart from a Wall. Langmuir 2022, 38, 4371–4377. [Google Scholar] [CrossRef] [PubMed]
- Bashkatov, A.; Park, S.; Demirkır, Ç.; Wood, J.A.; Koper, M.T.M.; Lohse, D.; Krug, D. Performance Enhancement of Electrocatalytic Hydrogen Evolution through Coalescence-Induced Bubble Dynamics. J. Am. Chem. Soc. 2024, 146, 10177–10186. [Google Scholar] [CrossRef]
- German, S.R.; Edwards, M.A.; Ren, H.; White, H.S. Critical Nuclei Size, Rate, and Activation Energy of H2 Gas Nucleation. J. Am. Chem. Soc. 2018, 140, 4047–4053. [Google Scholar] [CrossRef]
- Luo, L.; White, H.S. Electrogeneration of Single Nanobubbles at Sub-50-Nm-Radius Platinum Nanodisk Electrodes. Langmuir 2013, 29, 11169–11175. [Google Scholar] [CrossRef] [PubMed]
- Chen, Q.; Luo, L.; Faraji, H.; Feldberg, S.W.; White, H.S. Electrochemical Measurements of Single H2 Nanobubble Nucleation and Stability at Pt Nanoelectrodes. J. Phys. Chem. Lett. 2014, 5, 3539–3544. [Google Scholar] [CrossRef]
- Rox, H.; Bashkatov, A.; Yang, X.; Loos, S.; Mutschke, G.; Gerbeth, G.; Eckert, K. Bubble Size Distribution and Electrode Coverage at Porous Nickel Electrodes in a Novel 3-Electrode Flow-through Cell. Int. J. Hydrogen Energy 2023, 48, 2892–2905. [Google Scholar] [CrossRef]

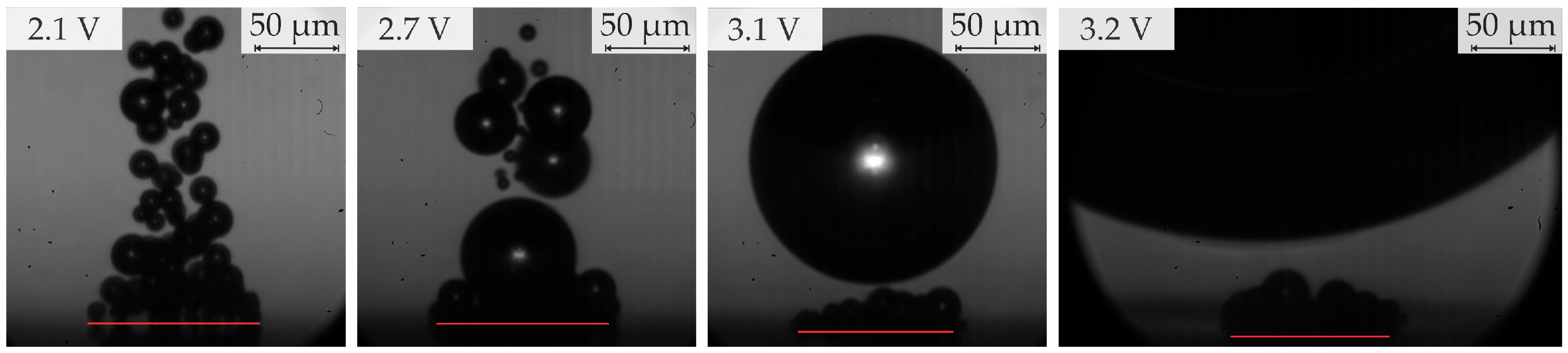
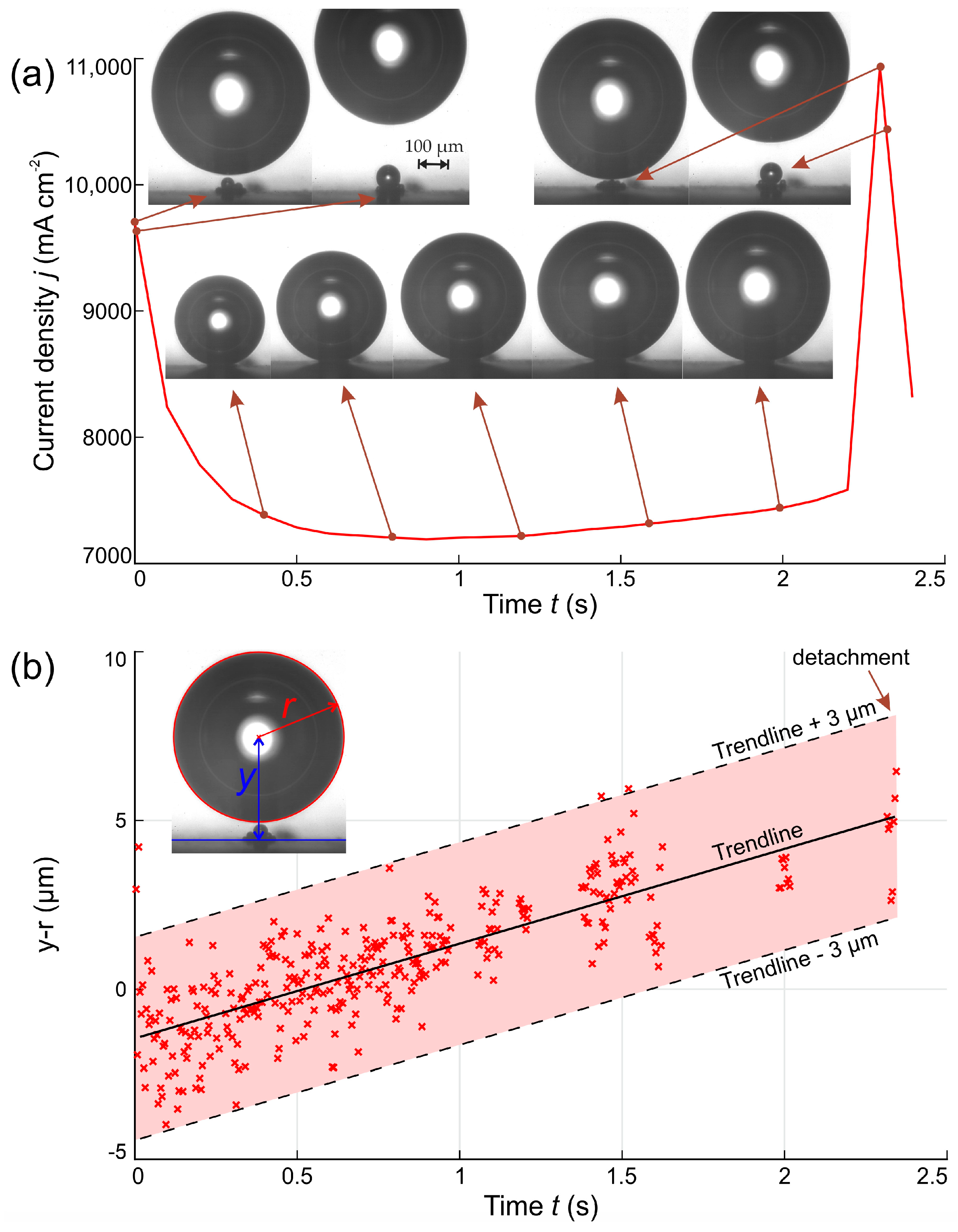
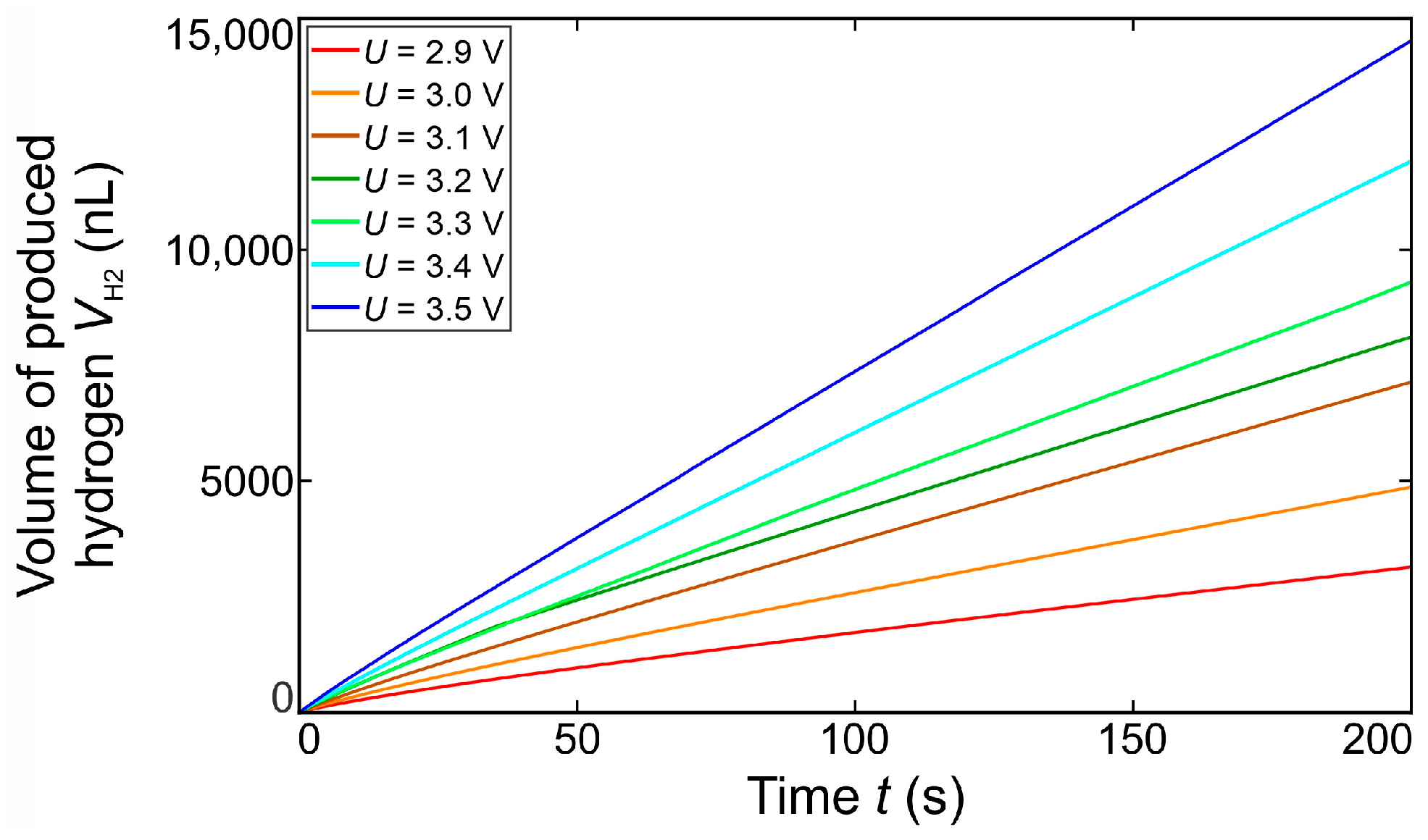

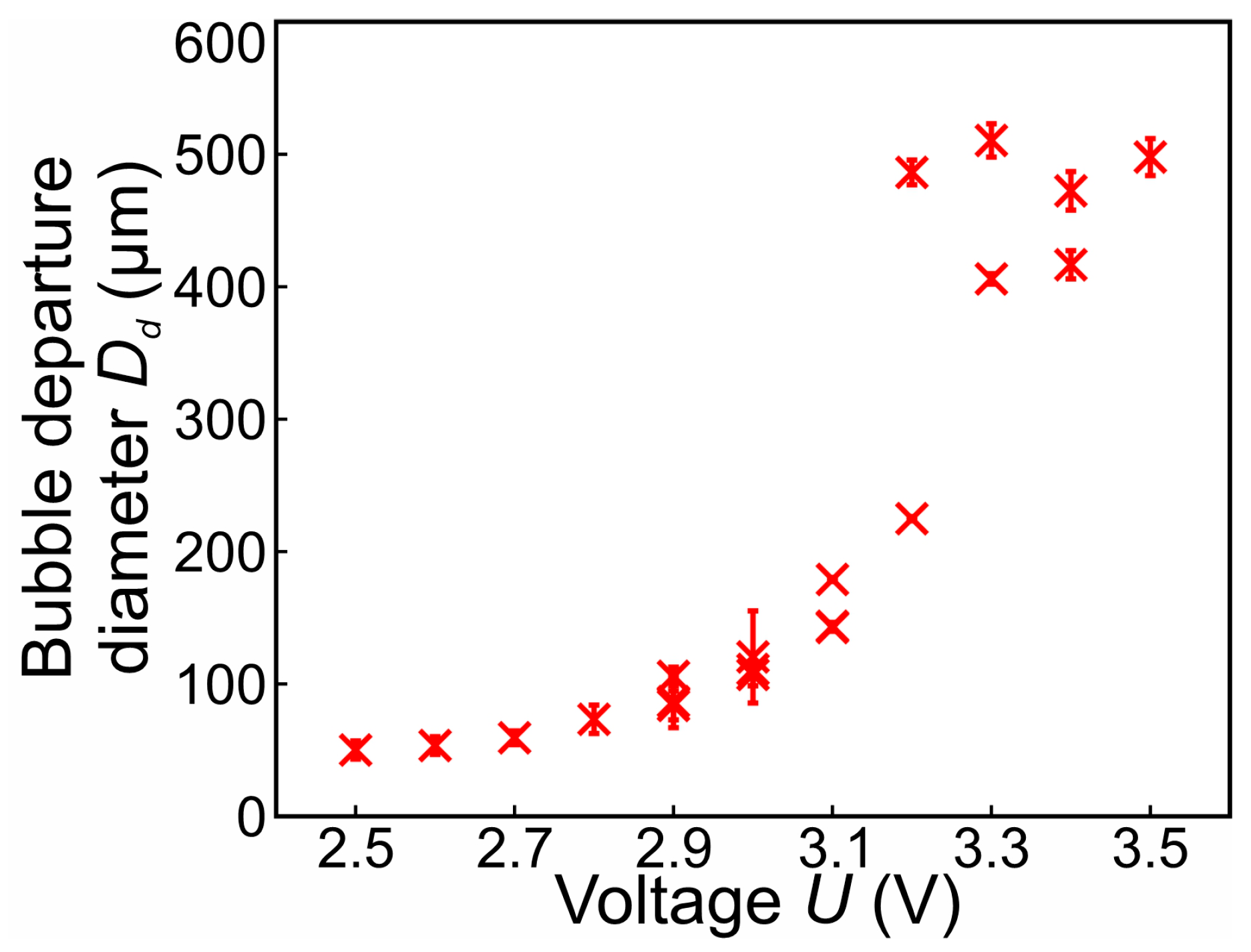
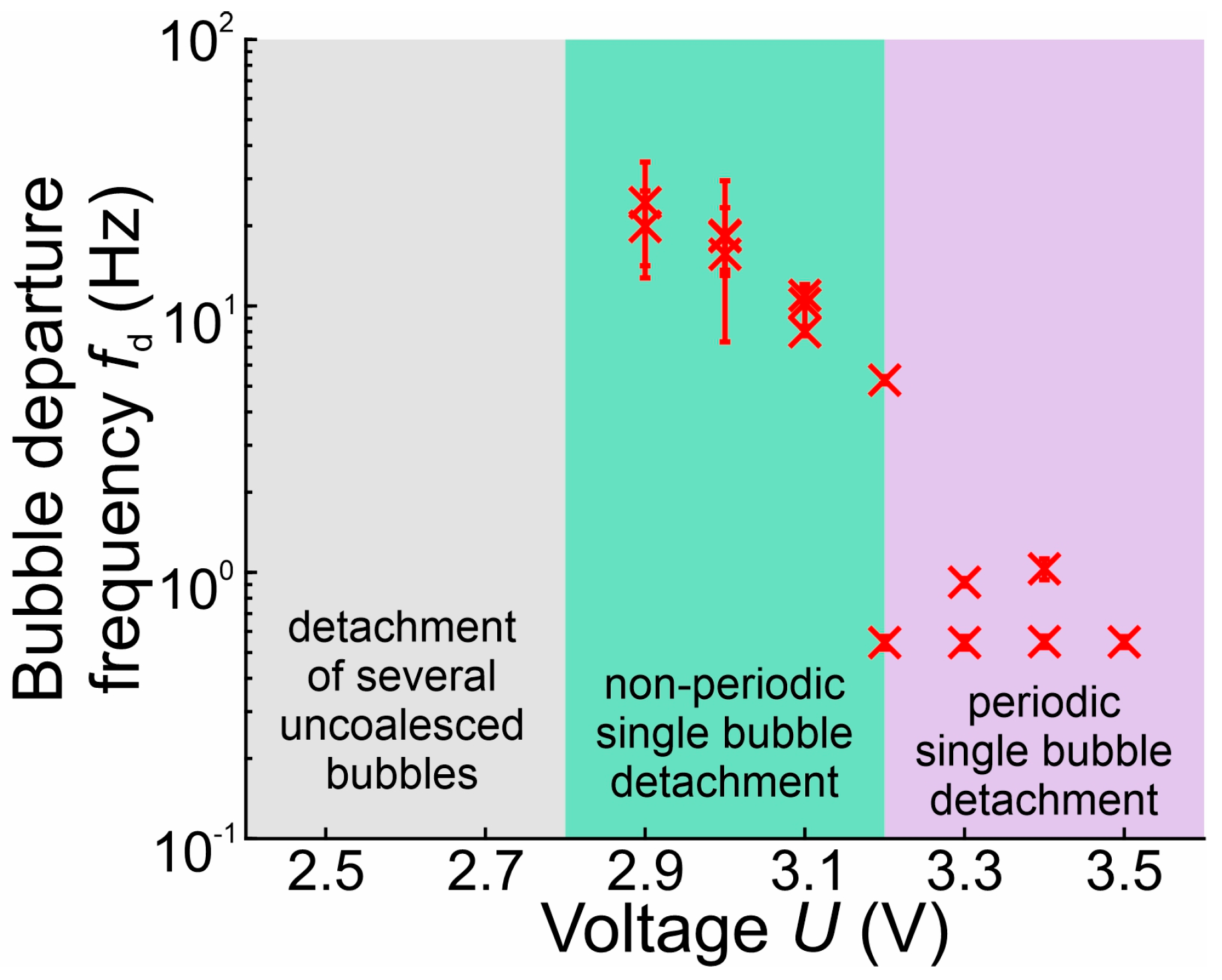
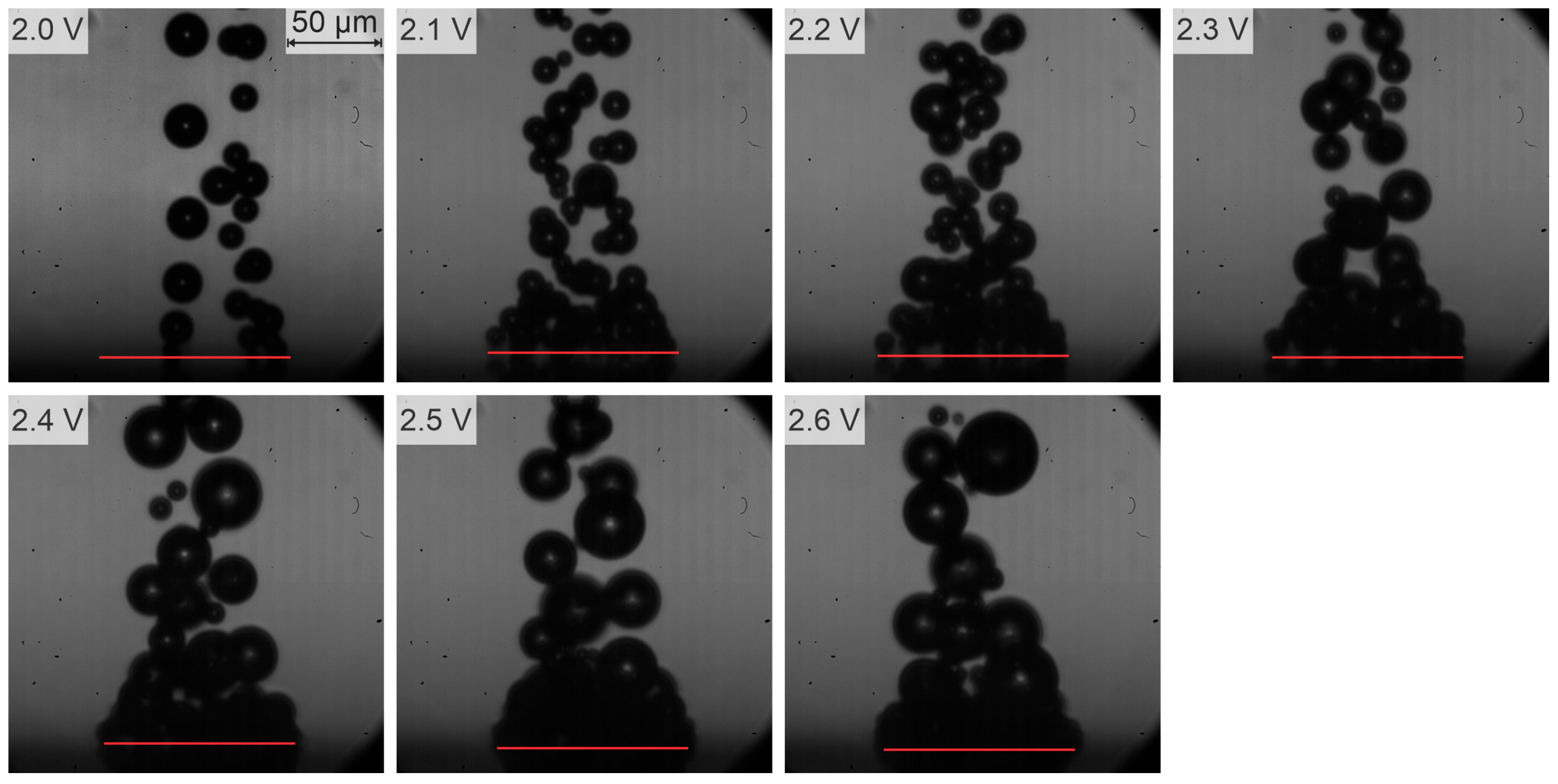


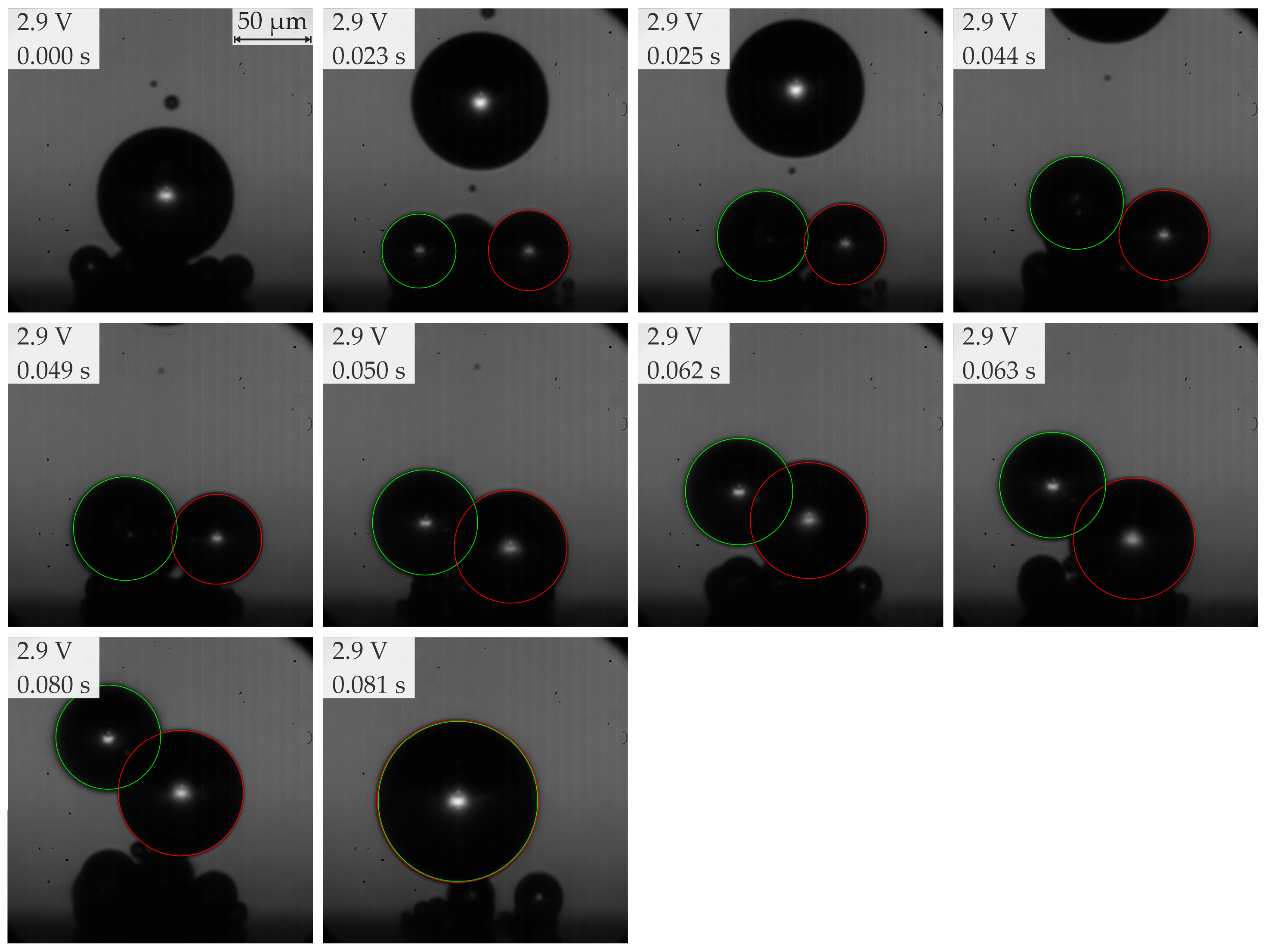
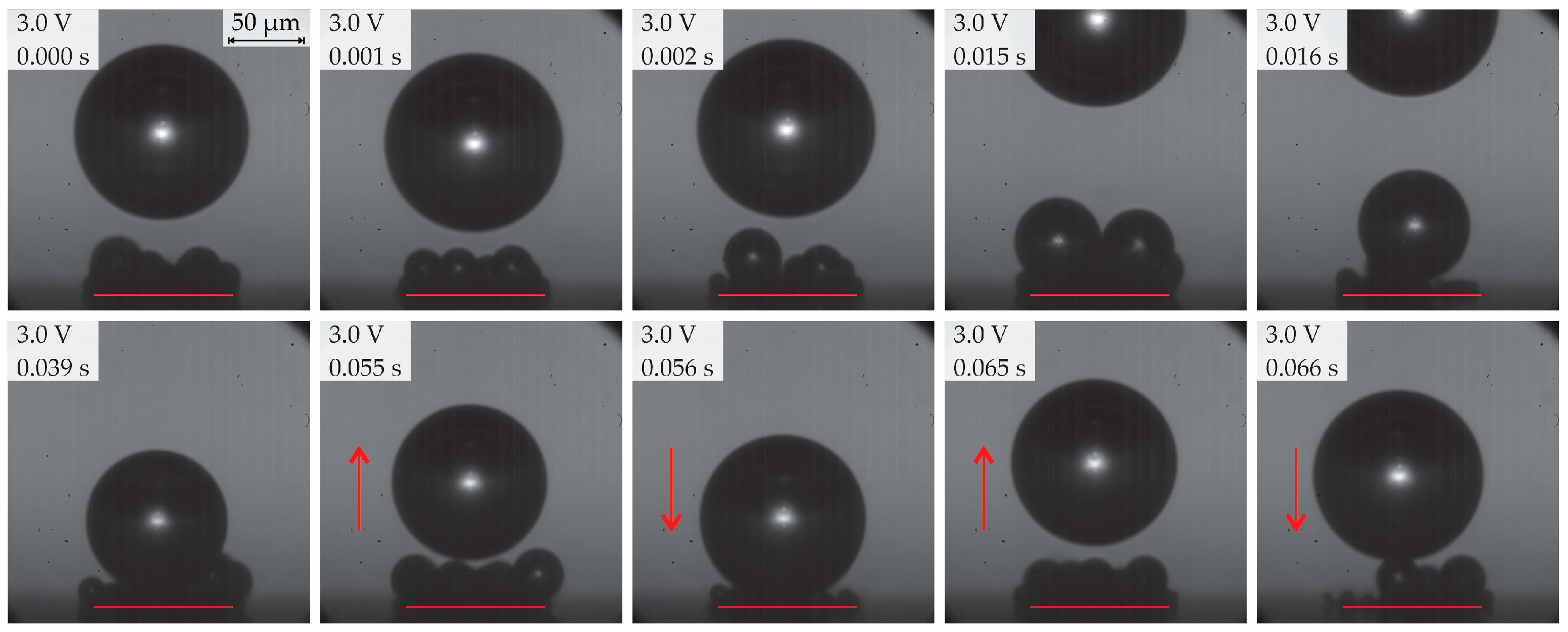
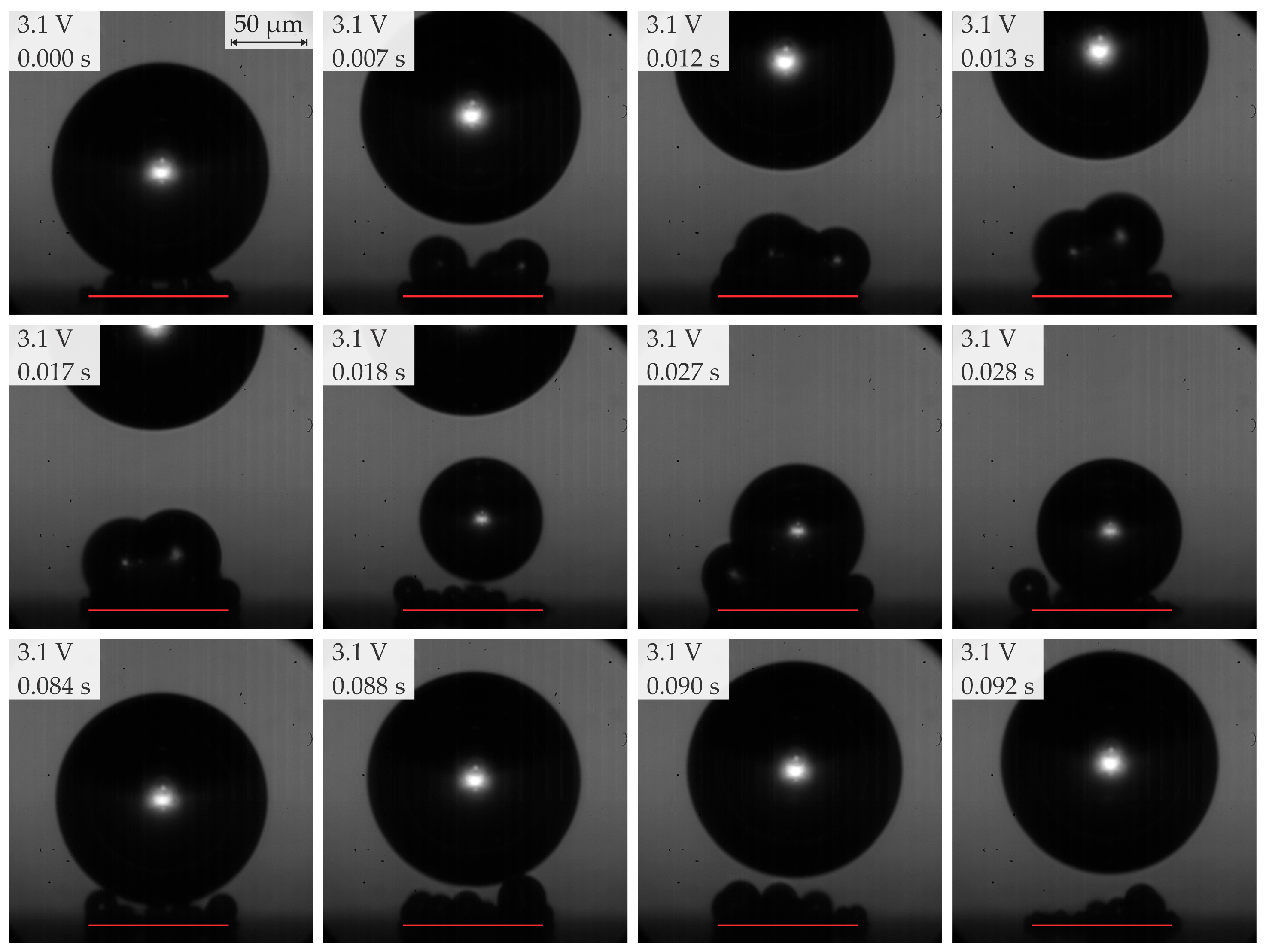
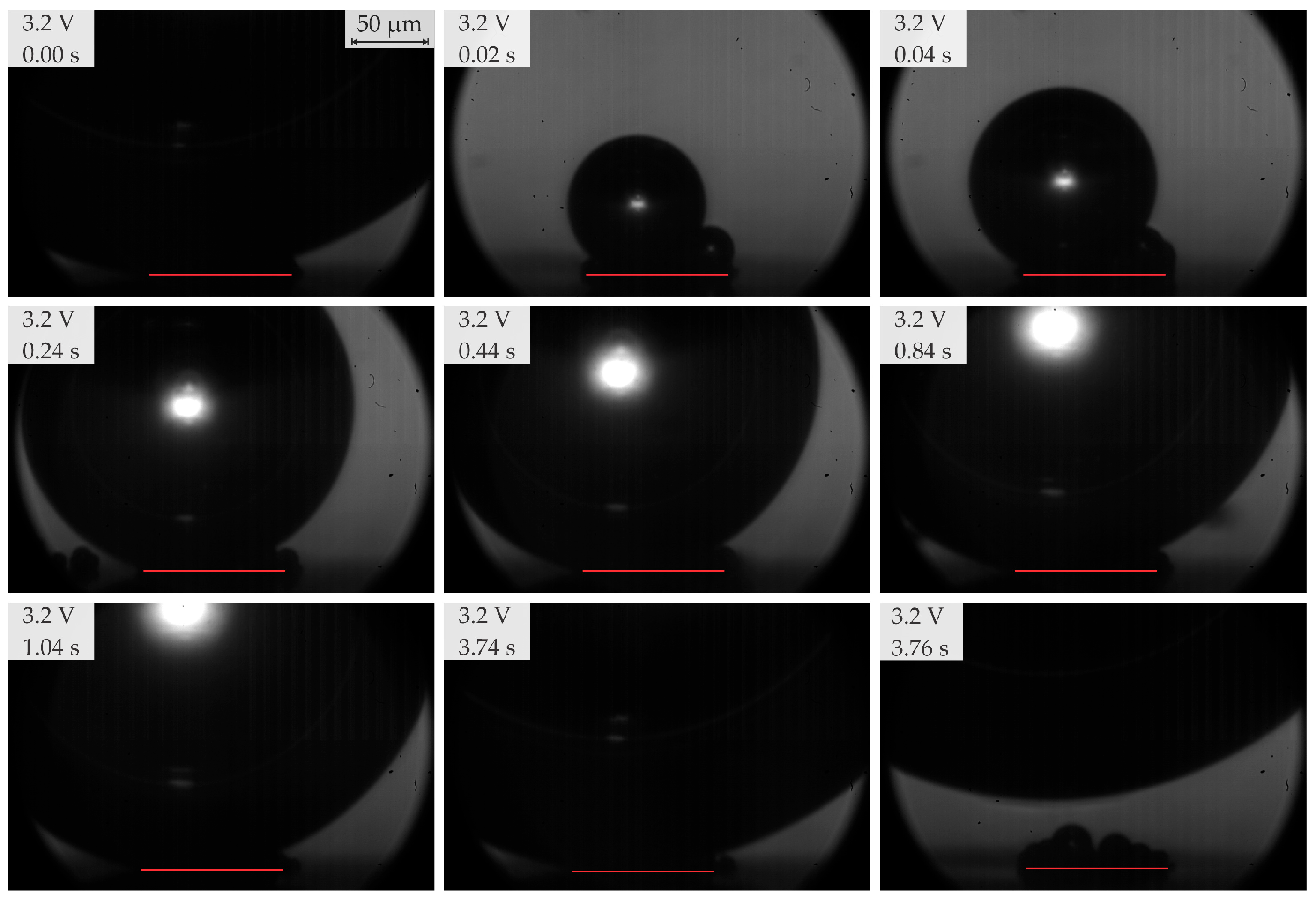

| Region | Applied Potential (V) | Bubble Behavior | Diameter at Detachment (μm) | Departure Frequency (Hz) |
|---|---|---|---|---|
| 1 | ≥2.0 <2.6 | No coalescence; departure of individual bubbles | ≤50 | N/A |
| 2a | ≥2.6 <2.8 (subregion a) | Partial coalescence: multiple coalesced bubbles depart simultaneously | 50–60 | N/A |
| 2b | ≥2.8 <3.2 (subregion b) | Predominantly single coalesced bubbles | 70–200 | 8–25 |
| 3 | ≥3.2 <3.5 | Departure of single coalesced bubbles | ≥450 | 0.5–5.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arhar, K.; Može, M.; Zupančič, M.; Golobič, I. Evaluation of Hydrogen Bubble Growth on a Platinum Microelectrode Under Varying Electrical Potential. Appl. Sci. 2025, 15, 4107. https://doi.org/10.3390/app15084107
Arhar K, Može M, Zupančič M, Golobič I. Evaluation of Hydrogen Bubble Growth on a Platinum Microelectrode Under Varying Electrical Potential. Applied Sciences. 2025; 15(8):4107. https://doi.org/10.3390/app15084107
Chicago/Turabian StyleArhar, Klara, Matic Može, Matevž Zupančič, and Iztok Golobič. 2025. "Evaluation of Hydrogen Bubble Growth on a Platinum Microelectrode Under Varying Electrical Potential" Applied Sciences 15, no. 8: 4107. https://doi.org/10.3390/app15084107
APA StyleArhar, K., Može, M., Zupančič, M., & Golobič, I. (2025). Evaluation of Hydrogen Bubble Growth on a Platinum Microelectrode Under Varying Electrical Potential. Applied Sciences, 15(8), 4107. https://doi.org/10.3390/app15084107









