Prediction of Experimental Rainfall-Eroded Soil Area Based on S-Shaped Growth Curve Model Framework
Abstract
:1. Introduction
2. Physical Experiments and Results
2.1. Physical Models, Monitoring Devices, and Experimental Procedures


2.2. Soil Erosion Area Calculation

2.3. Soil Erosion Area



2.4. Hydrological Characteristics





3. Soil Erosion Area Prediction Model
3.1. S-Shaped Growth Curve
3.2. S-Shaped Growth Curve Fitting
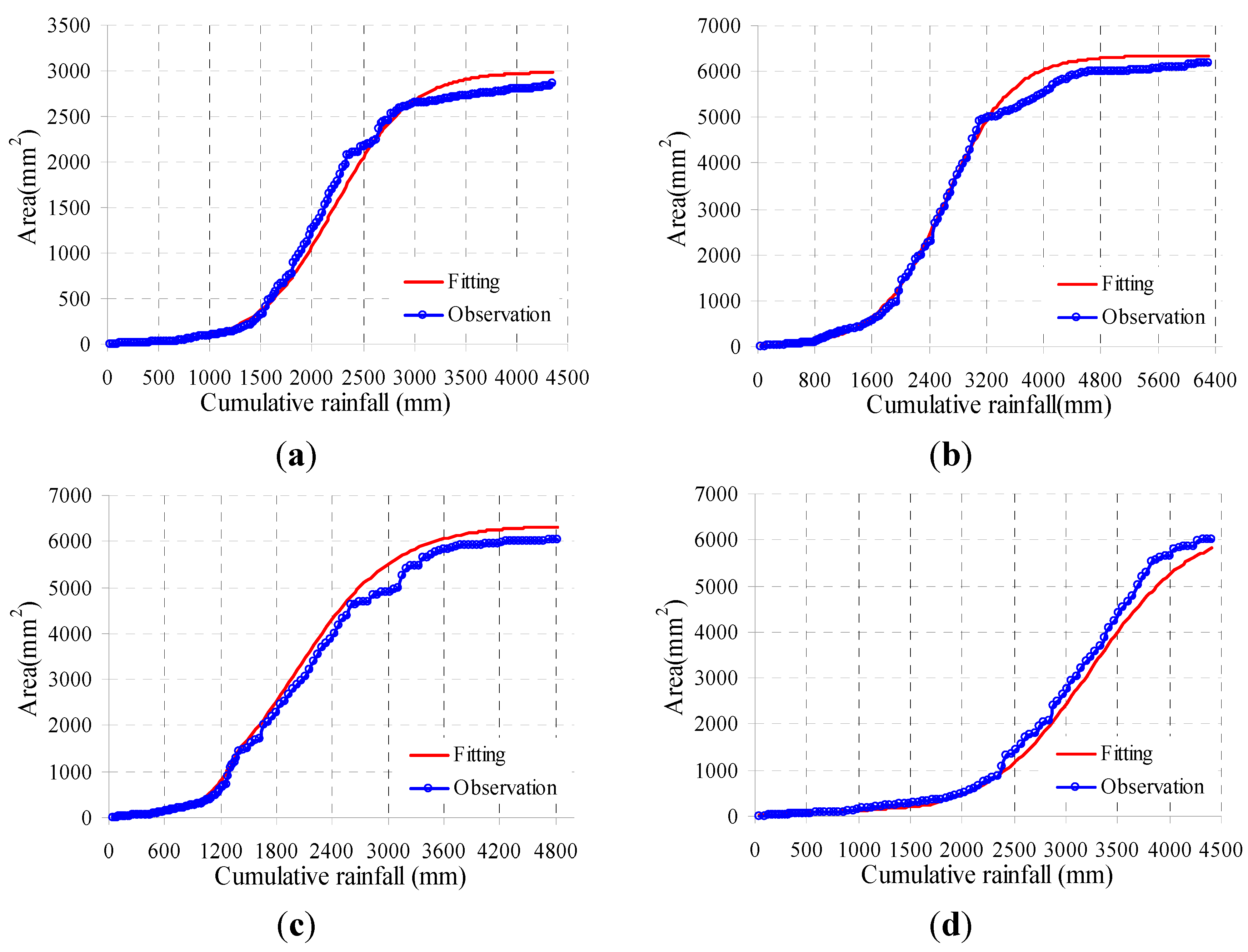

| Name | k | a | b |
|---|---|---|---|
| Soil Sample 1-25 mm/h | 3000 | 1.088 × e4 | 10.4 |
| Soil Sample 1-45 mm/h | 6350 | 4.75 × e5 | 15.2 |
| Soil Sample 1-65 mm/h | 9250 | 4.215 × e5 | 1.176 |
| Soil Sample 2-45 mm/h | 6350 | 4.4 × e5 | 20.6 |
| Soil Sample 3-45 mm/h | 6350 | 4.267 × e5 | 16.9 |
3.3. Development of Prediction Model


4. Discussion
4.1. Dimensionless Quantities of Soil Erosion Area

4.2. Comparison Between S-Curve Based Model and Time Series Analysis–Moving Average Model


4.3. Limitation and Suggestion of Experiments
5. Conclusions
- (1)
- The area of soil erosion obeys a trend of an S-growth curve under continuous rainfall.
- (2)
- A higher rainfall intensity can produce a greater area of soil erosion than lower rainfall intensity for the same material.
- (3)
- Under the same rainfall intensity, coarse material (stronger material) has a time lag against the soil erosion, but finally a similar area of soil erosion compared to fine material.
- (4)
- The S-curve model coupled with incremental parameter learning can effectively predict the soil erosion with just the rainfall information as input.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Wischmeier, W.H.; Smith, D.D. Predicting Rainfall Erosion Losses; USDA Agricultural Handbook, No. 537; U.S. Department of Agriculture: Washington, DC, USA, 1978. [Google Scholar]
- Renard, K.G.; Foster, G.R.; Weesies, G.A.; McCool, D.K.; Yoder, D.C. Predicting Soil Erosion by Water: A Guide to Conservation Planning with the Revised Universal Soil Loss Equation RUSLE; Agriculture Handbook, No. 703; U.S. Department of Agriculture: Washington, DC, USA, 1997; p. 404. [Google Scholar]
- Flanagan, D.C.; Nearing, M.A. USDA Water Erosion Prediction Project: Hillslope Profile and Watershed Model Documentation; NSERL report, No. 10; USDA-ARS National Soil Erosion Research Laboratory: West Lafayette, IN, USA, 1995. [Google Scholar]
- Morgan, R.P.C.; Quinton, J.N.; Smith, R.E.; Govers, G.; Poesen, J.W.A.; Auerswald, K.; Chisci, G.; Torri, D.; Styczen, M.E. The European soil erosion model EUROSEM: A dynamic approach for predicting sediment transport from fields and small catchments. Earth Surf. Process. Landf. 1993, 23, 527–544. [Google Scholar] [CrossRef]
- Smith, R.E.; Goodrich, D.C.; Quinton, J.N. Dynamic, distributed simulation of watershed erosion: the KINEROS2 and EUROSEM models. J. Soil Water Conserv. 1995, 50, 517–520. [Google Scholar]
- Soil Erosion: Application of Physically Based Models; Schmidt, J. (Ed.) Springer Science & Business Media: Berlin, Germany, 2013.
- Nearing, M.A. Capabilities and limitations of erosion models and data. In Proceedings of the 13th International Soil Conservation Organization Conference, Brisbane, Australia, 4–8 July 2004.
- Pieri, L.; Bittelli, M.; Wu, J.Q.; Dun, S.; Flanagan, D.C.; Pisa, P.R.; Ventura, F.; Salvatorelli, F. Using the Water Erosion Prediction Project (WEPP) model to simulate field observed runoff and erosion in the Apennines mountain range, Italy. J. Hydrol. 2007, 336, 84–97. [Google Scholar] [CrossRef]
- Cerdà, A. Effects of rock fragment cover on soil infiltration, interrill runoff and erosion. Eur. J. Soil Sci. 2001, 52, 59–68. [Google Scholar] [CrossRef]
- Ruiz-Sinoga, J.D.; García-Marín, R.; Martínez-Murillo, J.F.; Gabarrón-Galeote, M.A. Precipitation dynamics in southern Spain: trends and cycles. Int. J. Climatol. 2010, 31, 2281–2289. [Google Scholar] [CrossRef]
- Ruiz-Sinoga, J.D.; Martínez-Murillo, J.F.; Gabarrón-Galeote, M.A.; García-Marín, R. Effects of exposure, scrub position, and soil surface components on the hydrological response in small plots in southern Spain. Ecohydrology 2010, 3, 402–412. [Google Scholar] [CrossRef]
- Gaskin, G.J.; Miller, J.D. Measurement of soil water content using a simplified impedance measuring technique. J. Agric. Eng. Res. 1996, 63, 153–159. [Google Scholar] [CrossRef]
- Singh, P.; Kanwar, R.S.; Thompson, M.L. Measurement and characterization of macropores by using AUTOCAD and automatic image analysis. J. Environ. Qual. 1991, 20, 289–294. [Google Scholar] [CrossRef]
- Romkens, M.J.M.; Prasad, S.N.; Gerits, J.J.P. Soil erosion modes of sealing soils: a phenomenological study. Soil Technol. 1997, 11, 31–41. [Google Scholar] [CrossRef]
- Le Bissonnais, Y.; Bruand, A.; Jamagne, M. Laboratory experimental study of soil crusting: relation between aggregate breakdown mechanisms and crust structure. Catena 1989, 16, 377–392. [Google Scholar] [CrossRef]
- Andrea, R.; Helming, K.; Diestel, H. Effect of antecedent soil water content and rainfall regime on microrelief changes. Soil Technol. 1997, 10, 69–81. [Google Scholar]
- Backus, D.; Kehoe, P.J.; Kydland, F.E. Dynamics of the Trade Balance and the Terms of Trade: The S-curve; National Bureau of Economic Research: Cambridge, MA, USA, 1992. [Google Scholar]
- Bejan, A.; Lorente, S. The constructal law origin of the logistics S curve. J. Appl. Phys. 2011, 110, 024901. [Google Scholar] [CrossRef]
- Sun, W.; Won, S.H.; Ju, Y. In situ plasma activated low temperature chemistry and the S-curve transition in DME/oxygen/helium mixture. Combust. Flame 2014, 161, 2054–2063. [Google Scholar] [CrossRef]
- De Massis, A.; Chirico, F.; Kotlar, J.; Naldi, L. The temporal evolution of proactiveness in family firms: The horizontal S-curve hypothesis. Fam. Bus. Rev. 2013. [Google Scholar] [CrossRef] [Green Version]
- Van Rompaey, A.; Krasa, J.; Dostal, T.; Govers, G. Modelling sediment supply to rivers and reservoirs in Eastern Europe during and after collectivisation period. Hydrobiologia 2003, 494, 169–176. [Google Scholar] [CrossRef]
- USDA–Water Environment Prediction Project: Hillslope Profile and Watershed Model Documentation; Flanagan, D.C.; Nearing, M.A. (Eds.) US Department of Agriculture–Agricultural Research Service, National Soil Erosion Research Laboratory: West Lafayette, IN, USA, 1995.
- Bathurst, J.C.; Wicks, J.M.; O’Connell, P.E. The SHE/SHESED basin scale water flow and sediment transport modelling system. In Computer Models of Watershed Hydrology; Singh, V.P., Ed.; Water Resource Publication: Highlands Ranch, CO, USA, 1995; pp. 563–594. [Google Scholar]
- Hamilton, J.D. Time Series Analysis; Princeton University Press: Princeton, NJ, USA, 1994; Volume 2. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nie, W.; Huang, R.-Q.; Zhang, Q.-G.; Xian, W.; Xu, F.-L.; Chen, L. Prediction of Experimental Rainfall-Eroded Soil Area Based on S-Shaped Growth Curve Model Framework. Appl. Sci. 2015, 5, 157-173. https://doi.org/10.3390/app5030157
Nie W, Huang R-Q, Zhang Q-G, Xian W, Xu F-L, Chen L. Prediction of Experimental Rainfall-Eroded Soil Area Based on S-Shaped Growth Curve Model Framework. Applied Sciences. 2015; 5(3):157-173. https://doi.org/10.3390/app5030157
Chicago/Turabian StyleNie, Wen, Run-Qiu Huang, Qian-Gui Zhang, Wei Xian, Feng-Lin Xu, and Lin Chen. 2015. "Prediction of Experimental Rainfall-Eroded Soil Area Based on S-Shaped Growth Curve Model Framework" Applied Sciences 5, no. 3: 157-173. https://doi.org/10.3390/app5030157
APA StyleNie, W., Huang, R.-Q., Zhang, Q.-G., Xian, W., Xu, F.-L., & Chen, L. (2015). Prediction of Experimental Rainfall-Eroded Soil Area Based on S-Shaped Growth Curve Model Framework. Applied Sciences, 5(3), 157-173. https://doi.org/10.3390/app5030157







