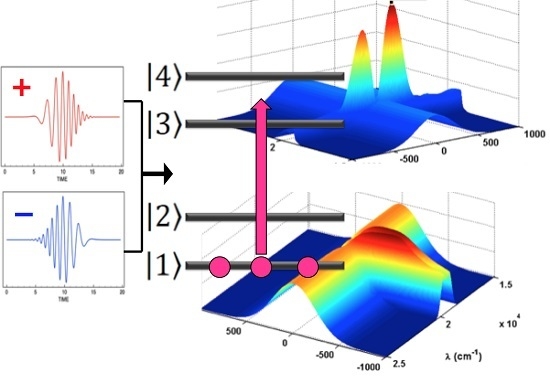
Quantum Control of Population Transfer and Vibrational States via Chirped Pulses in Four Level Density Matrix Equations
Abstract
:1. Introduction
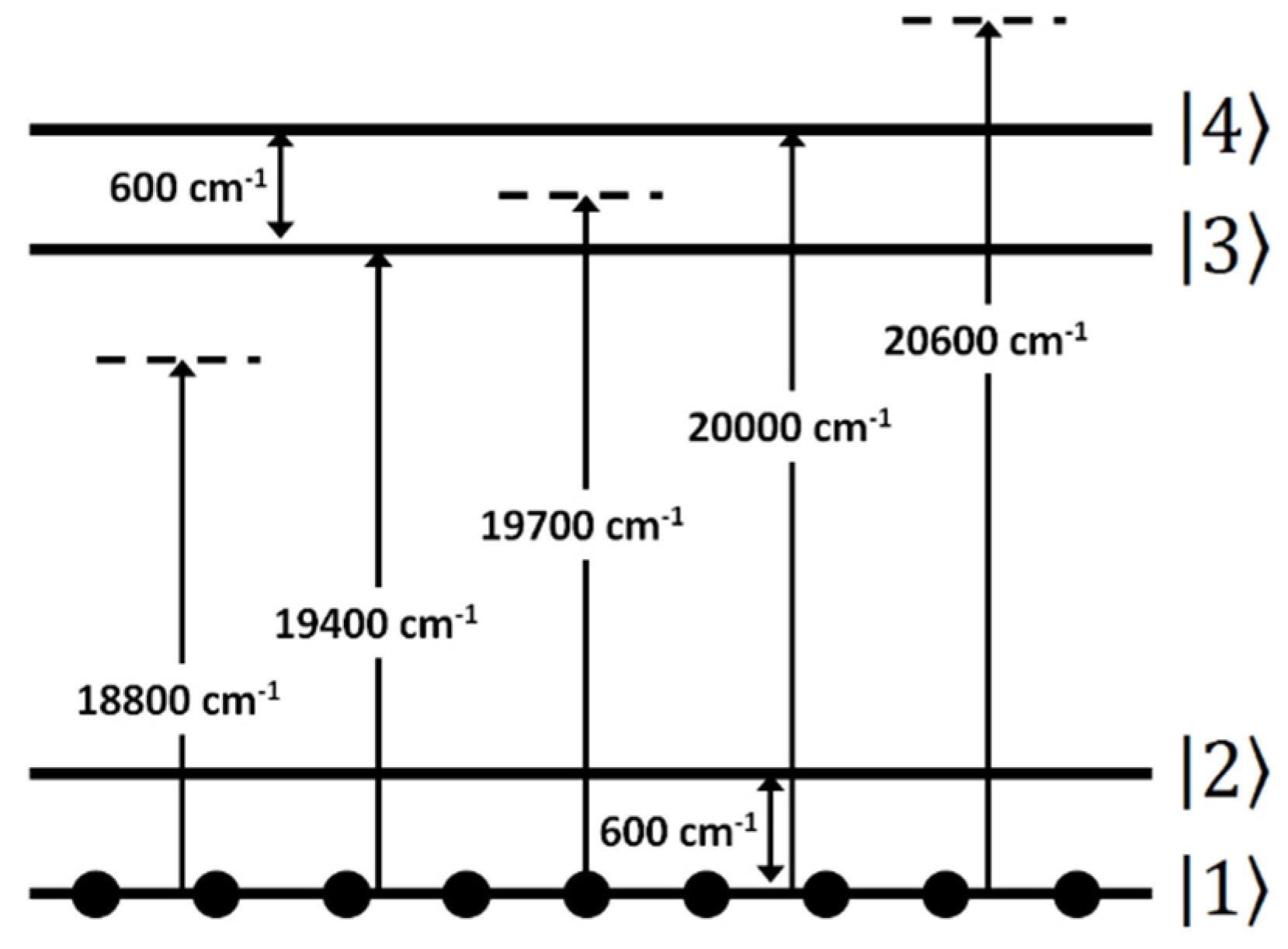
2. Density Matrix Simulations
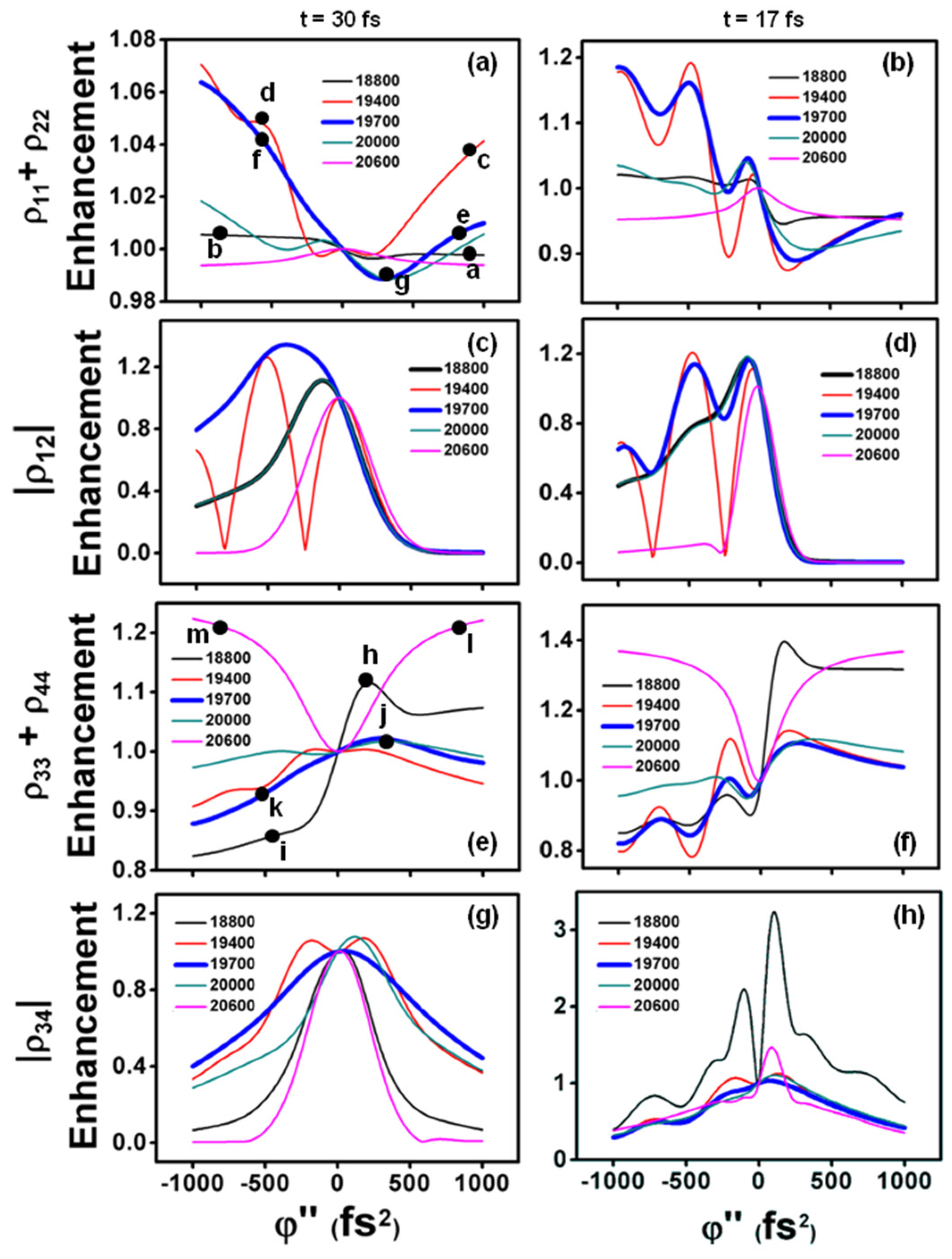
3. Main Results Obtained from the Simulations
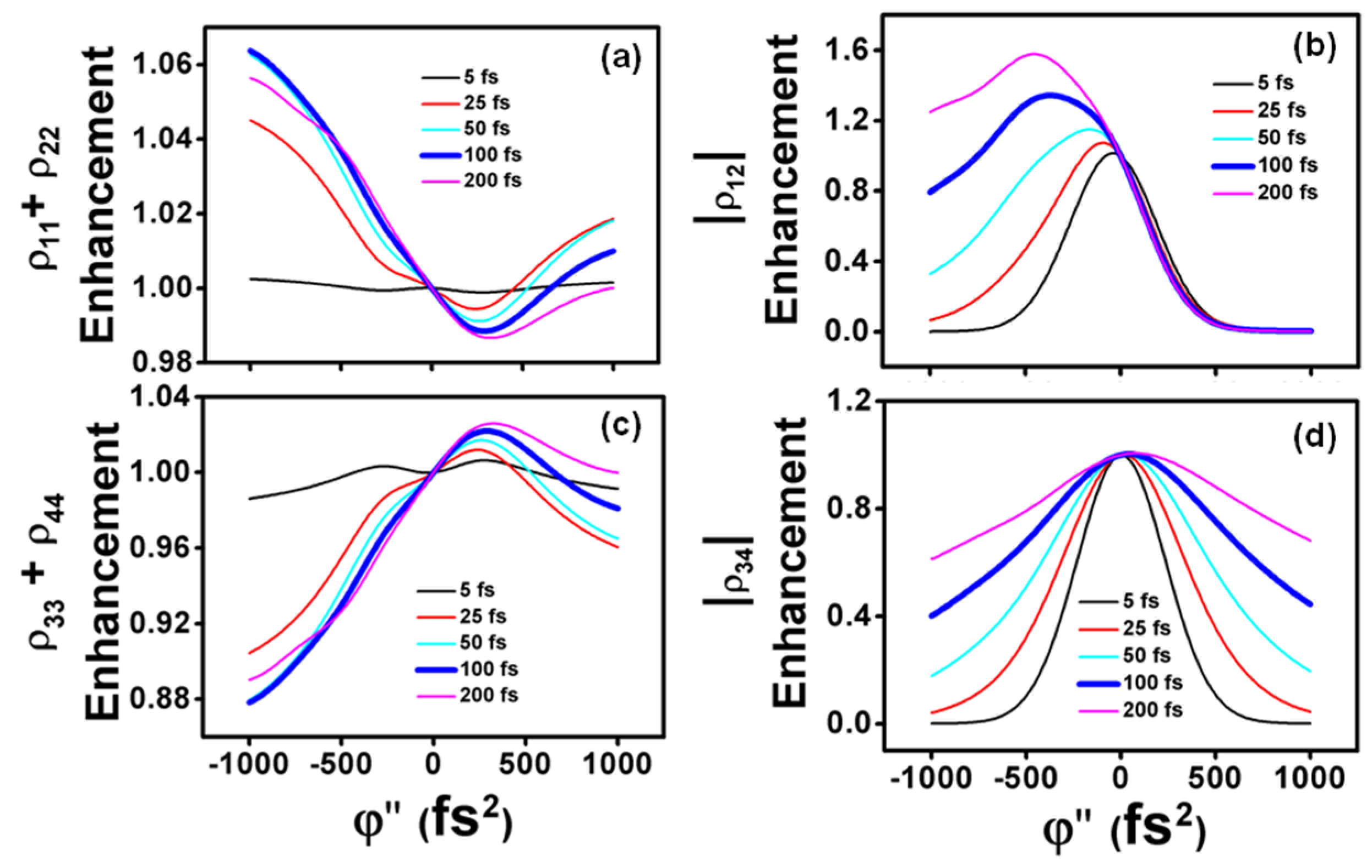
4. Influence of the Duration of the Laser Pulse
5. Influence of the Laser Peak Intensity
6. Influence of the Electronic Dephasing
7. Discussion and Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Brixner, T.; Pfeifer, T.; Gerber, G.; Wollenhaupt, M.; Baumert, T. 2004 Progress in Lasers: Femtosecond Laser Spectroscopy; Kluwer: Dordecht, The Netherlands, 2004; Volume 9, pp. 229–271. [Google Scholar]
- Kohler, B.; Yakovlev, V.V.; Che, J.; Krause, J.L.; Messina, M.; Wilson, K.R.; Schwentner, N.; Whitnell, R.M.; Yan, Y. Quantum control of wave packet evolution with tailored femtosecond pulses. Phys. Rev. Lett. 1995. [Google Scholar] [CrossRef] [PubMed]
- Baumert, T.; Grosser, M.; Thalweiser, R.; Gerber, G. Femtosecond time-resolved molecular multiphoton ionization: The Na2 system. Phys. Rev. Lett. 1991, 67. [Google Scholar] [CrossRef] [PubMed]
- Vogt, G.; Nuernberger, P.; Brixner, T.; Gerber, G. Femtosecond pump-shaped-dump quantum control of retinal isomerization in bacteriorhodopsin. Chem. Phys. Lett. 2006, 433, 211–215. [Google Scholar] [CrossRef]
- Baumert, T.; Helbing, J.; Gerber, G. Advances in Chemical Physics-Photochemistry; Wiley: New York, NY, USA, 1997; pp. 47–77. [Google Scholar]
- Tannor, D.; Kazakov, V.; Orlov, V. Time Dependent Quantum Molecular Dynamics; Plenum: New York, NY, USA, 1992; pp. 347–360. [Google Scholar]
- Meshulach, D.; Silberberg, Y. Coherent quantum control of manipulation transitions by shaped ultrashort optical pulses. Phys. Rev. A 1999, 60. [Google Scholar] [CrossRef]
- Meshulach, D.; Silberberg, Y. Coherent quantum control of two-photon transitions by femtosecond laser pulse. Nature 1998, 396, 239–242. [Google Scholar]
- Hildner, R.; Brinks, D.; Van Hulst, N.F. Femtosecond coherence and quantum control of single molecules at room temperature. Nat. Phys. 2011, 7, 172–177. [Google Scholar] [CrossRef]
- Krüger, M.; Schenk, M.; Hommelhoff, P. Attosecond control of electrons emitted from a nanoscale metal tip. Nature 2011, 475, 78–81. [Google Scholar] [CrossRef] [PubMed]
- Herek, J.L.; Wohlleben, W.; Cogdell, R.J.; Zeidler, D.; Motzkus, M. Quantum control of energy flow in light harvesting. Nature 2002, 417, 533–535. [Google Scholar] [CrossRef] [PubMed]
- Prokhorenko, V.I.; Nagy, A.M.; Waschuk, S.A.; Brown, L.S.; Birge, R.R.; Miller, R.J.D. Coherent control of retinal isomerization in Bacteriorhodopsin. Science 2006, 313, 1257–1261. [Google Scholar] [CrossRef] [PubMed]
- Feurer, T.; Vaughan, J.C.; Nelson, K.A. Spatiotemporal coherent control of lattice vibrational waves. Science 2003, 299, 374–377. [Google Scholar] [CrossRef] [PubMed]
- Lüker, S.; Gawarecki, K.; Reiter, D.E.; Grodecka-Grad, A.; Axt, V.M.; Machnikowski, P.; Kuhn, T. Influence of acoustic phonons on the optical control of quantum dots driven by adiabatic passage. Phys. Rev. B 2012, 85. [Google Scholar] [CrossRef]
- Debnath, A.; Meier, C.; Chatel, B.; Amand, T. Chirped laser excitation of quantum dot excitons coupled to a phonon bath. Phys. Rev. B 2012, 86. [Google Scholar] [CrossRef]
- Renaud, N.; Grozema, F.C. Cooperative biexciton generation and destructive interference in coupled quantum dots uing adiabatic rapid passage. Phys. Rev. B 2014, 90. [Google Scholar] [CrossRef]
- Kuroda, D.G.; Singh, C.P.; Peng, Z.; Kleiman, V.D. Mapping excited-state dynamics by coherent control of a dendrimer’s photoemission efficiency. Science 2009, 326, 263–267. [Google Scholar] [CrossRef] [PubMed]
- Zewail, A.H. Femtochemistry: Ultrafast Dynamics of the Chemical Bond; World Sci.: New York, NJ, USA, 1994. [Google Scholar]
- Brumer, P.; Shapiro, M. Coherent Control of Molecular Dynamics; Springer: Heidelberg, Germany, 1999; pp. 191–213. [Google Scholar]
- Nuernberger, P.; Vogt, G.; Brixner, T.; Gerber, G. Femtosecond quantum control of molecular dynamics in the condensed phase. Phys. Chem. Chem. Phys. 2007, 9, 2470–2497. [Google Scholar] [CrossRef] [PubMed]
- Levine, R.D.; Jortner, J. Mode Selective Chemistry. In Proceedings of the 24th Jerusalem Symp. On Quantum Chemistry and Biochemistry, Jerusalem, Israel, 20–23 May 1991; pp. 535–571.
- Picón, A.; Biegert, J.; Jaron-Becker, A.; Becker, A. Coherent control of the vibrational state population in a nonpolar molecule. Phys. Rev. A 2011, 83. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Yang, X.; Yan, X. Selective and efficient control of population transfer in the presence of an equally populated initial doublet. J. Opt. Soc. Am. B 2012, 29, 1551–1556. [Google Scholar] [CrossRef]
- Kumar, P.; Sarma, A.K. Ultrafast and selective coherent population transfer in four-level atoms by a single nonlinearly chirped femtosecond pulse. Phys. Rev. A 2013, 88. [Google Scholar] [CrossRef]
- Zhang, Z.; Tian, J.; Du, J. Selective population transfer and creation of an arbitrary superposition between quantum states in a Λ-type four-level system by a single linearly chirped pulse. Laser Phys. Lett. 2016, 13. [Google Scholar] [CrossRef]
- Weiner, A.M.; Leaird, D.E.; Wiederrecht, G.P.; Nelson, K.A. Femtosecond pulse sequences used for optical manipulation of molecular motion. Science 1990, 247, 1317–1319. [Google Scholar] [CrossRef] [PubMed]
- Hauer, J.; Skenderovic, H.; Kompa, K.L.; Motzkus, M. Enhancement of Raman modes by coherent control in β-carotene. Chem. Phys. Lett. 2006, 421, 523–528. [Google Scholar] [CrossRef]
- Buckup, T.; Hauer, J.; Motzkus, M. On the paradigm of coherent control: The phase-dependent light-matter interaction in the shaping window. New J. Phys. 2009, 11. [Google Scholar] [CrossRef]
- Hauer, J.; Buckup, T.; Motzkus, M. Quantum control spectroscopy of vibrational modes: Comparison of control scenarios for ground and excited states in β-carotene. Chem. Phys. 2008, 350, 220–229. [Google Scholar] [CrossRef]
- Wohllenben, W.; Buckup, T.; Herek, J.L.; Motzkus, M. Coherent control for spectroscopy and manipulation of biological dynamics. ChemPhysChem 2005, 6, 850–857. [Google Scholar] [CrossRef] [PubMed]
- Hauer, J.; Buckup, T.; Skenderovic, H.; Kompa, K.L.; Motzkus, M. Enhancement of Raman modes in complex molecules by coherent control. In Ultrafast Phenomena, X.V.; Springer: Berlin, Germany, 2007; p. 303. [Google Scholar]
- Hornung, T.; Skenderovic, H.; Motzkus, M. Observation of all-trans-β-carotene wavepacket motion on the electronic ground and excited state using degenerate four-wave mixing (DFWM) and pump-DFWM. Chem. Phys. Lett. 2005, 402, 283–288. [Google Scholar] [CrossRef]
- Kiefer, W.; Materny, A.; Schmitt, M. Femtosecond time-resolved spectroscopy of elementary molecular dynamics. Naturwissenschaften 2002, 89, 250–258. [Google Scholar] [CrossRef] [PubMed]
- Siebert, T.; Maksimenka, R.; Materny, A.; Engel, V.; Kiefer, W.; Schmitt, M. The role of specific normal modes during non-Born-Oppenheimer dynamics: The S1-S0 internal conversion of β-caroteneinterrogated on a femtosecond time-scale with coherent anti-Stokes Raman scattering. J. Ram. Spect. 2002, 33, 844–854. [Google Scholar] [CrossRef]
- Bitter, M.; Shapiro, E.A.; Milner, V. Enhancing strong-field-induced molecular vibration with femtosecond pulse shaping. Phys. Rev. A 2012, 86. [Google Scholar] [CrossRef]
- Buckup, T.; Hauer, J.; Voll, J.; Vivie-Riedle, R.; Motzkus, M. A general control mechanism of energy flow in the excited state of polyenic biochromophores. Faraday Discuss. 2011, 153, 213–225. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Amitay, Z.; Uberna, R.; Leone, S.R. Time-shifting the dynamics of Li2 multistate rovibrational wavepackets by state selective coherent phase control. In Proceedings of the US-Japan Workshop, Honolulu, HI, USA, 12–15 December 1999; Gordon, R.J., Fujimura, Y., Eds.; World Scientific: Singapore, Singapore, 2000; Volume 14, pp. 3–14. [Google Scholar]
- Yang, X.; Zhang, Z.; Yan, X.; Li, C. Enhanced selectivity and efficiency of coherent population transfer via train of pulse pairs. Phys. Rev. A 2010, 82. [Google Scholar] [CrossRef]
- Andrianov, I.V.; Paramonov, G.K. Selective excitation of the vibrational-rotational states and dissociation of diatomic molecules by picoseconds infrared laser pulses: Modeling for HF in the ground electronic state. Phys. Rev. A 1999, 59. [Google Scholar] [CrossRef]
- Korolkov, M.V.; Paramonov, G.K. Vibrationally state-selective electronic excitation of diatomic molecules by ultrashort laser pulses. Phys. Rev. A 1998, 57. [Google Scholar] [CrossRef]
- Savolainen, J.; Fanciulli, R.; Dijkhuizen, N.; Moore, A.L.; Hauer, J.; Buckup, T.; Motzkus, M.; Herek, J.L. Controlling the efficiency of an artificial light-harvesting complex. Proc. Natl. Acad. Sci. USA 2008, 105, 7641–7646. [Google Scholar] [CrossRef] [PubMed]
- Savolainen, J.; Buckup, T.; Hauer, J.; Jafarpour, A.; Serrat, C.; Motzkus, M.; Herek, J.L. Carotenoid deactivation in an artificial light-harvesting complex via a vibrationally hot ground state. Chem. Phys. 2009, 357, 181–187. [Google Scholar] [CrossRef]
- Buckup, T.; Lebold, T.; Weigel, A.; Wohlleben, W.; Motzkus, M. Singlet versus triplet dynamics of β-carotene studied by quantum control spectroscopy. J. Photochem. Photobiol. A 2006, 180, 314–321. [Google Scholar] [CrossRef]
- Buckup, T.; Hauer, J.; Möhring, J.; Motzkus, M. Multidimensional spectroscopy of β-carotene: Vibrational cooling in the excited state. Arch. Biochem. Biophys. 2009, 483, 219–223. [Google Scholar] [CrossRef] [PubMed]
- Buckup, T.; Lebold, T.; Weigel, A.; Wohlleben, W.; Motzkus, M. Multiphoton Quantum-control spectroscopy of β-carotene. In Ultrafast Phenomena, X.V.; Springer: Berlin, Germany, 2007; p. 483. [Google Scholar]
- Buckup, T.; Savolainen, J.; Wohlleben, W.; Herek, J.L.; Hashimoto, H.; Correia, R.R.B.; Motzkus, M. Pump-probe and pump-deplete-probe spectroscopies on carotenoids with N = 9–15 conjugated bonds. J. Chem. Phys. 2006, 125. [Google Scholar] [CrossRef] [PubMed]
- Buckup, T.; Wohlleben, W.; Heinz, B.; Savolainen, J.; Herek, J.L.; Hashimoto, H.; Cogdell, R.J.; Motzkus, M. Energy flow in carotenoids, studied with pump-deplete-probe, multiphoton- and coherent control spectroscopy. In Ultrafast Phenomena XIV; Springer: Berlin, Germany, 2005; p. 368. [Google Scholar]
- Consani, C.; Ruetzel, S.; Nuernberger, P.; Brixner, T. Quantum control spectroscopy of competing reaction pathways in a molecular switch. J. Phys. Chem. A 2014, 118, 11364–11372. [Google Scholar] [CrossRef] [PubMed]
- Wohlleben, W.; Buckup, T.; Hashimoto, H.; Cogdell, R.J.; Herek, J.L.; Motzkus, M. Pump-deplete-probe spectroscopy and the puzzle of carotenoid dark states. J. Phys. Chem. B 2004, 108, 3320–3325. [Google Scholar] [CrossRef]
- Kraack, J.P.; Motzkus, M.; Buckup, T. Selective nonlinear response preparation using femtosecond spectrally resolved four-wave-mixing. J. Chem. Phys. 2011, 135. [Google Scholar] [CrossRef]
- Nuernberger, P.; Ruetzel, S.; Brixner, T. Multidimensional electronic spectroscopy of photochemical reactions. Angew. Chem. Int. Ed. Engl. 2015, 54, 11368–11385. [Google Scholar] [CrossRef] [PubMed]
- Ruetzel, S.; Diekmann, M.; Nuernberger, P.; Walter, C.; Engels, B.; Brixner, T. Multidimensional spectroscopy of photoreactivity. Proc. Natl. Acad. Sci. USA 2014, 111, 4764–4769. [Google Scholar] [CrossRef] [PubMed]
- Meshulach, D.; Yelin, D.; Silberberg, Y. Adaptive real-time femtosecond pulse shaping. J. Opt. Soc. Am. B 1998, 15, 1615–1619. [Google Scholar] [CrossRef]
- Yelin, D.; Meshulach, D.; Silberberg, Y. Adaptive femtosecond pulse compression. Opt. Lett. 1997, 22, 1793–1795. [Google Scholar] [CrossRef] [PubMed]
- Feist, A.; Echternkamp, K.E.; Schauss, J.; Yalunin, S.V.; Schafer, S.; Ropers, C. Quantum coherent optical phase modulation in an ultrafast transmission electron microscope. Nature 2015, 521, 200–203. [Google Scholar] [CrossRef] [PubMed]
- Yan, Y.J.; Mukamel, S. Electronic dephasing, vibrational relaxation, and solvent friction in molecular nonlinear optical line shapes. J. Chem. Phys. 1988, 89. [Google Scholar] [CrossRef]
- Joo, T.; Albrecht, A.C. Electronic dephasing studies of molecules in solution at room temperature by femtosecond degenerate four wave mixing. Chem. Phys. 1993, 176, 233–247. [Google Scholar] [CrossRef]
- Nibbering, E.T.J.; Duppen, K.; Wiersma, D.A. Optical dephasing in solution: A line shape and resonance light scattering study of azulene in isopentane and cyclohexane. J. Chem. Phys. 1990, 93. [Google Scholar] [CrossRef]
- Wand, A.; Kallush, S.; Shoshanim, O.; Bismuth, O.; Kosloff, R.; Ruhman, S. Chirp effects on impulsive vibrational spectroscopy: A multimode perspective. Phys. Chem. Chem. Phys. 2010, 12, 2149–2163. [Google Scholar] [CrossRef] [PubMed]
- Malkmus, S.; Dürr, R.; Sobotta, C.; Pulvermacher, H.; Zinth, W.; Braun, M. Chirp dependence of wave packet motion in Oxazine 1. J. Phys. Chem. A 2005, 109, 10488–10492. [Google Scholar] [CrossRef] [PubMed]
- Ruhman, S.; Kosloff, R. Application of chirped ultrashort pulses for generating large-amplitude ground-state vibrational coherence: A computer simulation. J. Opt. Soc. Am. B 1990, 7, 1748–1752. [Google Scholar] [CrossRef]
- Bardeen, C.J.; Wang, Q.; Shank, C.V. Selective excitation of vibrational wave packet motion using chirped pulses. Phys. Rev. Lett. 1995, 75. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Bardeen, C.J.; Wilson, K.R. Molecular π-pulse for total inversion of electronic state population. Phys. Rev. Lett. 1998, 80. [Google Scholar] [CrossRef]
- Konar, A.; Lozovoy, V.V.; Dantus, M. Solvent environment revealed by positively chirped pulses. J. Phys. Chem. Lett. 2014, 5, 924–928. [Google Scholar] [CrossRef] [PubMed]
- Serrat, C.; Corbera, M.; Afa, J. Trichromatic π-Pulse for Ultrafast Total Inversion of a Four-Level Ladder System. Appl. Sci. 2015, 5, 1484–1493. [Google Scholar] [CrossRef] [Green Version]
- Diels, J.C.; Rudolph, W. Ultrashort Laser Pulse Phenomena, 2nd ed.; Academic Press: San Diego, CA, USA, 2006. [Google Scholar]
- Möhring, J.; Buckup, T.; Motzkus, M. A Quantum control spectroscopy approach by direct UV femtosecond pulse shaping. IEEE J. Sel. Top. Quant. Electron. 2012, 18, 449–459. [Google Scholar] [CrossRef]
- Florean, A.C.; Cardoza, D.; White, J.L.; Lanyi, J.K.; Sension, R.J.; Bucksbaum, P.H. Control of retinal isomerization in bacteriorhodopsin in the high-intensity regime. Proc. Natl. Acad. Sci. USA 2009, 106, 10896–10900. [Google Scholar] [CrossRef] [PubMed]
- Carroll, E.C.; Florean, A.C.; Bucksbaum, P.H.; Spears, K.G.; Sension, R.J. Phase control of the competition between electronic transitions in a solvated laser dye. Chem. Phys. 2008, 350, 75–86. [Google Scholar] [CrossRef]
- Buckup, T.; Hauer, J.; Serrat, C.; Motzkus, M. Control of excited-state population and vibrational coherence with shaped-resonant and near-resonant excitation. J. Phys. B At. Mol. Opt. Phys. 2008, 41. [Google Scholar] [CrossRef]




| Parameters | Value | |
|---|---|---|
| Transitional dipole coupling coefficient (μij) | ||
| Peak amplitude of field, E | ||
| Vibrational Frequency | ||
| ( and ) | 600 cm−1 | |
| Wave numbers of the different transitions. | ||
| → | 19,400 cm−1 | |
| → | 20,000 cm−1 | |
| → | 18,800 cm−1 | |
| Spectral chirp φʺ | −1000 fs2 to 1000 fs2 | |
| Purely dephasing rates () | ||
| Decay rates of the transitions, i − j () | ||
| Duration of the Gaussian transform-limited (TL) pulses | 30 fs, 17 fs | |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Afa, I.J.; Serrat, C. Quantum Control of Population Transfer and Vibrational States via Chirped Pulses in Four Level Density Matrix Equations. Appl. Sci. 2016, 6, 351. https://doi.org/10.3390/app6110351
Afa IJ, Serrat C. Quantum Control of Population Transfer and Vibrational States via Chirped Pulses in Four Level Density Matrix Equations. Applied Sciences. 2016; 6(11):351. https://doi.org/10.3390/app6110351
Chicago/Turabian StyleAfa, Iduabo John, and Carles Serrat. 2016. "Quantum Control of Population Transfer and Vibrational States via Chirped Pulses in Four Level Density Matrix Equations" Applied Sciences 6, no. 11: 351. https://doi.org/10.3390/app6110351
APA StyleAfa, I. J., & Serrat, C. (2016). Quantum Control of Population Transfer and Vibrational States via Chirped Pulses in Four Level Density Matrix Equations. Applied Sciences, 6(11), 351. https://doi.org/10.3390/app6110351