Determination of Permeability and Inertial Coefficients of Sintered Metal Porous Media Using an Isothermal Chamber
Abstract
:1. Introduction
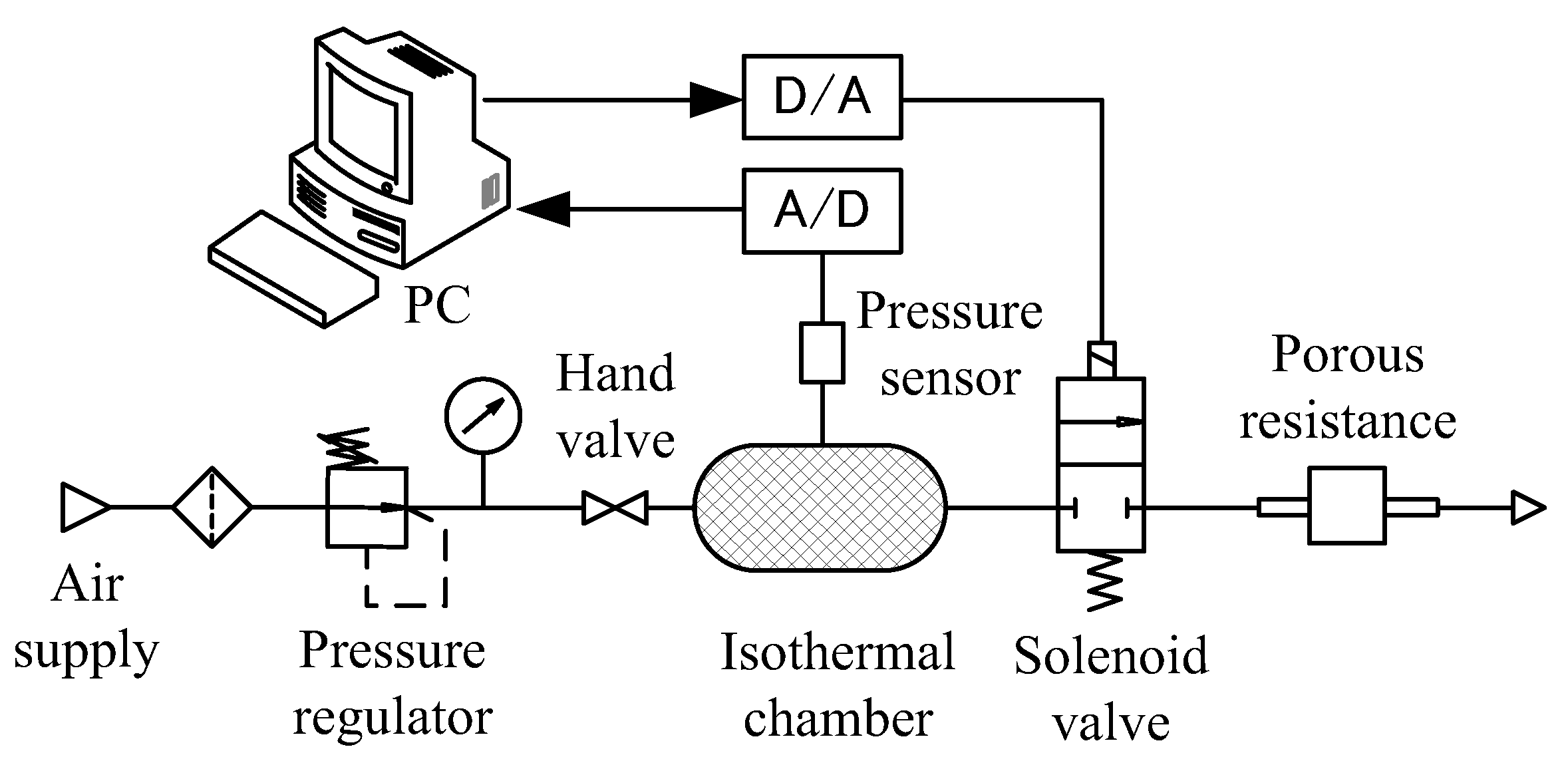
2. Materials and Methods
3. Results
3.1. Test Medium
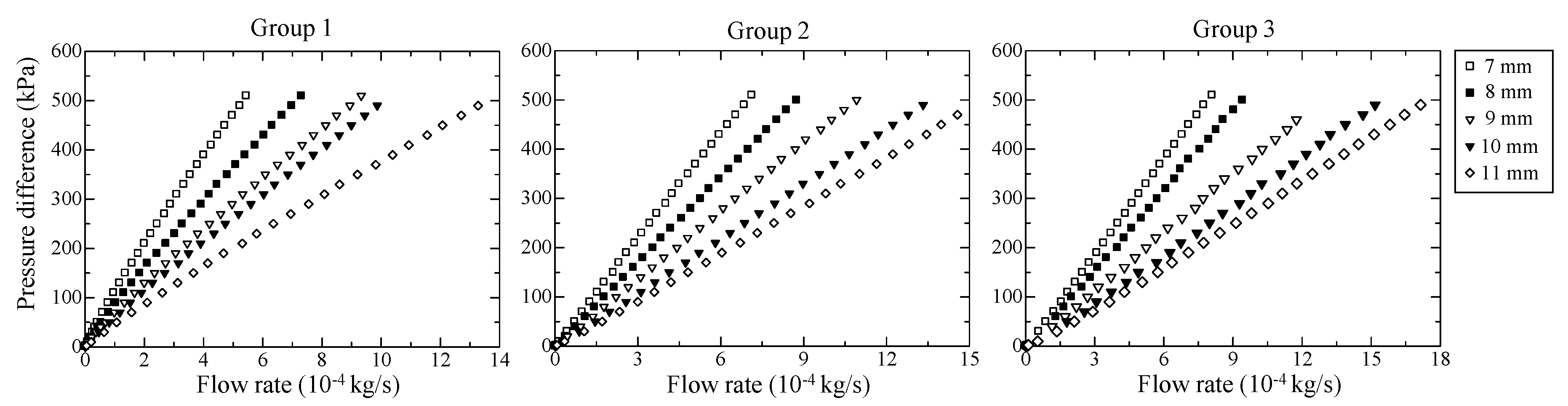
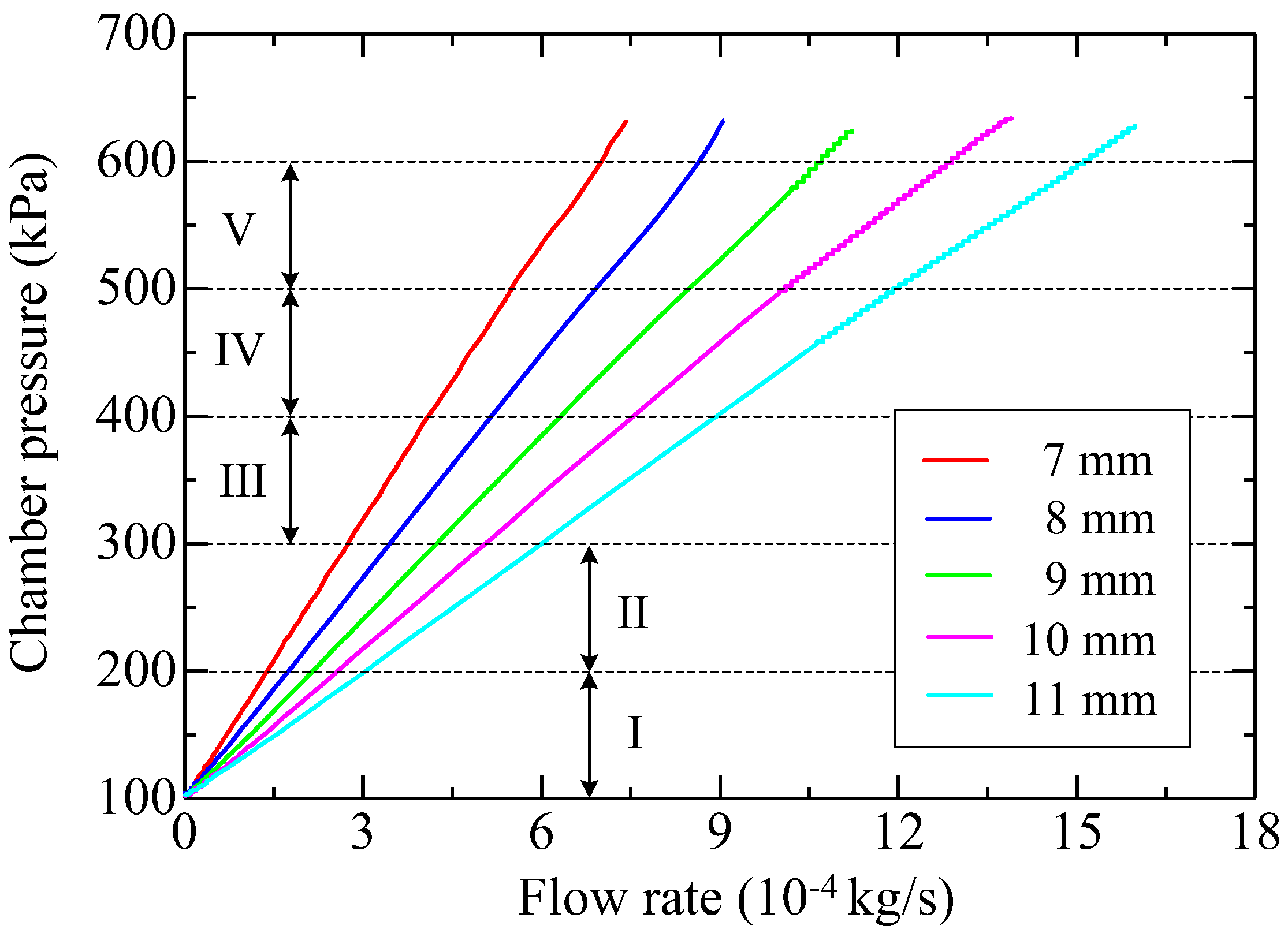
3.2. Steady-State Method
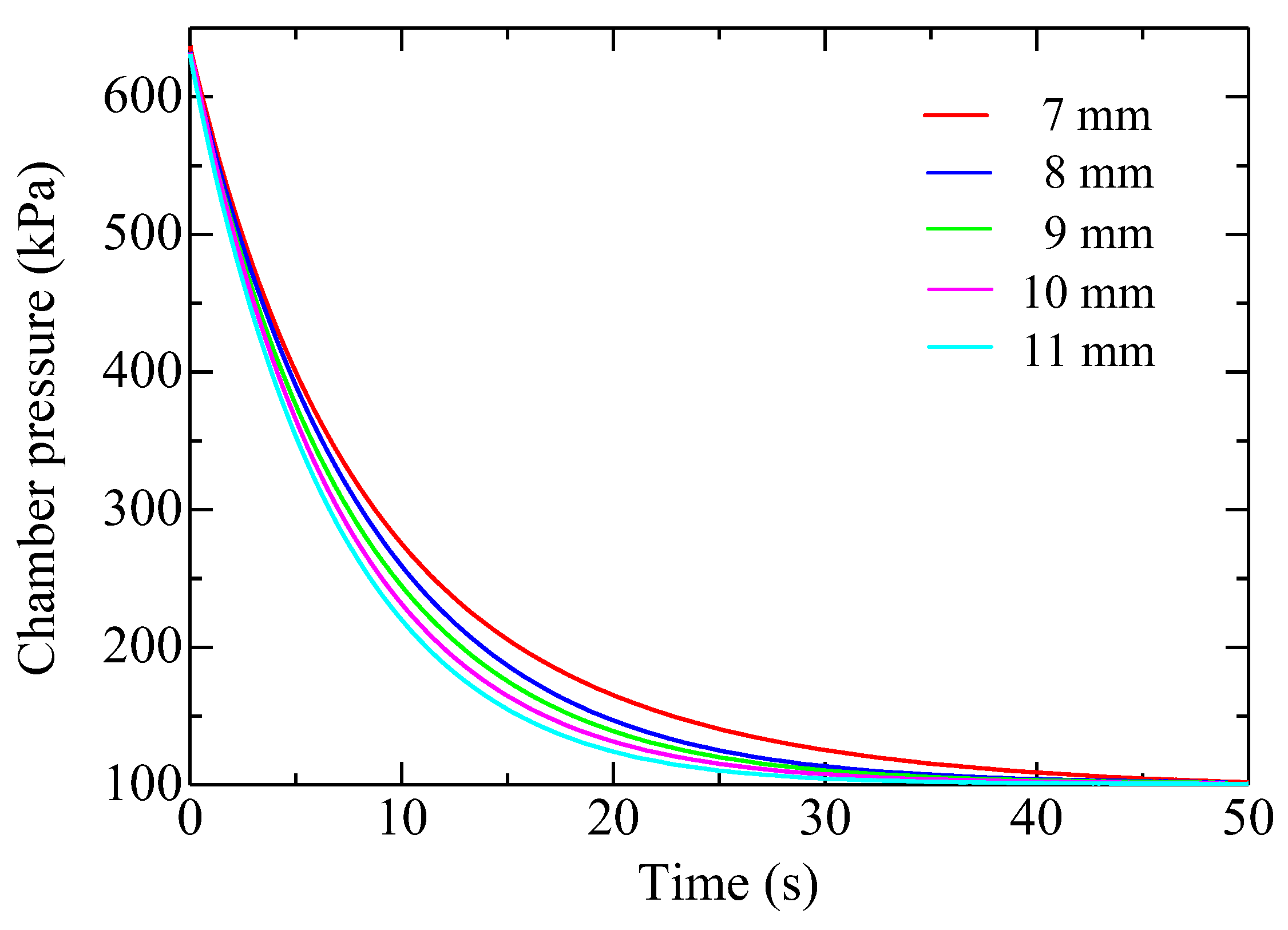
3.3. Discharge Method with an Isothermal Chamber
4. Discussion
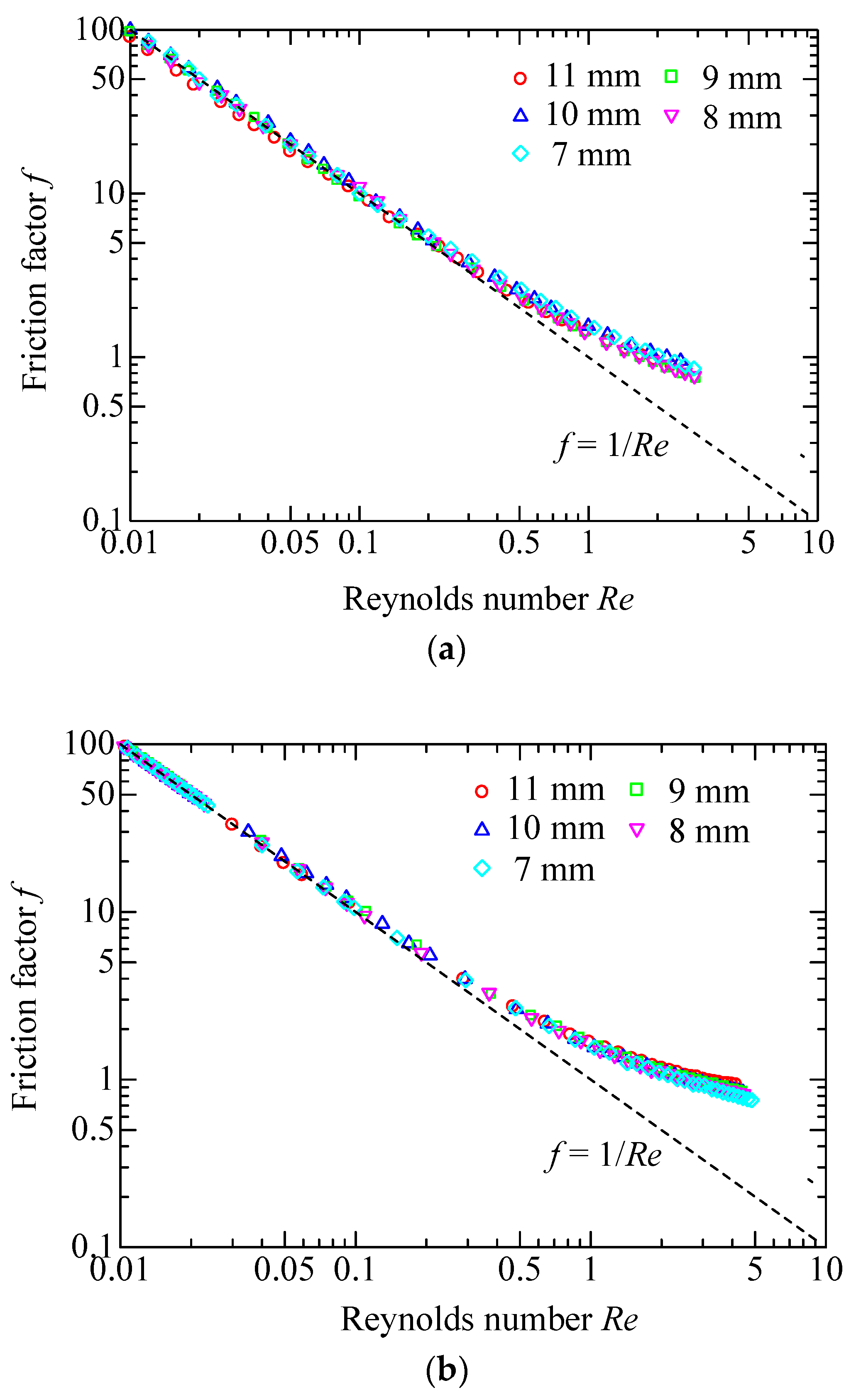
4.1. Results of the Steady-State Method
4.2. Results of the Discharge Method
- (1)
- Initial value K0 = 1 × 10−12 and β0 = 1 are given for the Forchheimer flow rate equation, Equation (10): .
- (2)
- The square residual error Spre is calculated by: . Here, The Gi values are obtained by differentiating the measured pressure in the chamber.
- (3)
- The elements of the first derivative , , and the residuals are calculated for i =1~n.
- (4)
- Solve the following two linear equations to obtain ΔK and Δβ.
- (5)
- Update K and β by K = K0 + ΔK and β = β0 + Δβ.
- (6)
- Calculate S = , if this value satisfies the convergence condition, i.e., |S − Spre| < ε, the determination process is ended. If it does not satisfy the condition, the aforementioned process is repeated with K0 and β0 replaced by K and β.
4.3. Comparison of the Steady-State Method and the Discharge Method
5. Conclusions
- (1)
- The test samples are mainly tight porous media, which have a permeability on the order of 10−12 m2 and are generally applied in air-bearing systems.
- (2)
- An isothermal condition for the chamber is necessary during the discharge process. Otherwise, the calculated flow rate would exhibit large error. This means that a reasonable discharge speed is required.
- (3)
- The theoretical model is somewhat realistic since the inner structure of the actual porous media is not uniform. Therefore, the determined coefficients might not reflect the true properties of the media.
- (4)
- Only the permeability and inertial coefficients are discussed. The influences of other parameters such as porosity φ, cross-sectional area A, and length of the medium L are not included.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| A | cross-sectional area (m2) |
| G | mass flow rate (kg/s) |
| G(cal)i | calculated flow rate (kg/s) |
| G(exp)i | steady-state flow rate (kg/s) |
| K | permeability (m2) |
| L | length of the test medium (m) |
| m | mass of the medium (kg) |
| P | pressure (Pa) |
| P1 | upstream pressure (Pa) |
| P2 | downstream pressure (Pa) |
| Pa | atmospheric pressure (Pa) |
| Pc | pressure in the chamber (Pa) |
| R | gas constant |
| t | time (s) |
| T | temperature (K) |
| V | total volume (m3) |
| Vc | volume of the chamber (m3) |
| β | inertial coefficient |
| γ | metal density (8.03 × 103 kg/m3) |
| Θ | room temperature (K) |
| μ | air viscosity (Pa⋅s) |
| v | velocity (m/s) |
| ρ | density (kg/m3) |
| φ | porosity |
Appendix A
References
- Liao, Y.; Li, X.; Zhong, W. Experimental study of pressure drop-flow rate characteristics of heated tight porous materials. J. Fluids Eng. 2016, 138, 071102. [Google Scholar] [CrossRef]
- Liao, Y.; Li, X.; Zhong, W. Study of pressure drop-flow rate and flow resistance characteristics of heated porous materials under local thermal non-equilibrium conditions. Int. J. Heat Mass Tran. 2016, 102, 528–543. [Google Scholar] [CrossRef]
- Lage, J.L.; Antohe, B.V.; Nield, D.A. Two Types of Nonlinear Pressure-Drop versus Flow-Rate Relation Observed for Saturated Porous Media. J. Fluids Eng. 1997, 119, 700–706. [Google Scholar] [CrossRef]
- Andrade, J.S.; Costa, U.M.S.; Almeida, M.P.; Makse, H.A.; Stanley, H.E. Inertial effects on fluid flow through disordered porous media. Phys. Rev. Lett. 1999, 82, 5249–5252. [Google Scholar] [CrossRef]
- Boomsma, K.; Poulikakos, D. The effects of compression and pore size variations on the liquid flow characteristics in metal foams. J. Fluids Eng. 2002, 124, 263–272. [Google Scholar] [CrossRef]
- Medraj, M.; Baril, E.; Loya, V.; Lefebvre, L.P. The effect of microstructure on the permeability of metallic foams. J. Mater. Sci. 2007, 42, 4372–4383. [Google Scholar] [CrossRef] [Green Version]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 3rd ed.; Springer: New York, NY, USA, 2006. [Google Scholar]
- Nihad, D.; Özer, B.; Mustafa, Ö. Experimental flow in various porous media and reconciliation of Forchheimer and Ergun relatons. Exp. Therm. Fluid Sci. 2014, 57, 425–433. [Google Scholar]
- Ergun, S. Fluid flow through packed columns. Chem. Eng. Prog. 1952, 48, 89–94. [Google Scholar]
- Macdonald, I.F.; EI-Sayed, M.S.; Mow, K.; Dullien, F.A.L. Flow through porous media-the Ergun Equation Revisited. Ind. Eng. Chem. Fundam. 1979, 18, 199–208. [Google Scholar] [CrossRef]
- Beavers, G.S.; Sparrow, E.M. Non-Darcy flow through fibrous porous media. J. Appl. Mech. 1969, 36, 711–714. [Google Scholar] [CrossRef]
- Beavers, G.S.; Sparrow, E.M.; Rodenz, D.E.J. Influence of bed size on the flow characteristics and porosity of randomly packed beds of spheres. Appl. Mech. 1973, 40, 655–660. [Google Scholar] [CrossRef]
- Montillet, A.; Akkari, E.; Comiti, J. About a correlating equation for predicting pressure drops through packed beds of spheres in a large range of Reynolds numbers. Chem. Eng. Process 2007, 46, 329–333. [Google Scholar] [CrossRef]
- Antohe, B.V.; Lage, J.L.; Price, D.C.; Weber, R.M. Experimental determination of permeability and inertia coefficients of mechanically compressed aluminum porous matrices. J. Fluids Eng. 1997, 119, 404–412. [Google Scholar] [CrossRef]
- Dukhan, N.; Minjeur, C.A., II. A two-permeability approach for assessing flow properties in metal foam. J. Porous Mat. 2011, 18, 417–424. [Google Scholar] [CrossRef]
- Liu, J.F.; Wu, W.T.; Chiu, W.C.; Hsieh, W.H. Measurement and correlation of friction characteristic of flow through foam matrixes. Exp. Therm. Fluid Sci. 2006, 30, 329–336. [Google Scholar] [CrossRef]
- Dukhan, N.; Patel, P. Equivalent particle diameter and length scale for pressure drop in porous metals. Exp. Therm. Fluid Sci. 2008, 32, 1059–1067. [Google Scholar] [CrossRef]
- Dietrich, B.; Schabel, W.; Kind, M.; Martin, H. Pressure drop measurements of ceramic sponges—Determining the hydraulic diameter. Chem. Eng. Sci. 2009, 64, 3633–3640. [Google Scholar] [CrossRef]
- Kim, T.; Lu, T.J. Pressure drop through anisotropic porous mediumlike cylinder bundles in turbulent flow regime. J. Fluids Eng. 2008, 130, 104501. [Google Scholar] [CrossRef]
- Jin, L.W.; Kai, C.L. Pressure drop and friction factor of steady and oscillating flows in open-cell porous media. Transp. Porous Med. 2008, 72, 37–52. [Google Scholar] [CrossRef]
- Mancin, S.; Zilio, C.; Cavallini, A.; Rossetto, L. Pressure drop during air flow in aluminum foams. Int. J. Heat Mass Tran. 2010, 53, 3121–3130. [Google Scholar] [CrossRef]
- Belforte, G.; Raparelli, T.; Viktorov, V.; Trivella, A. Metal woven wire cloth feeding system for gas bearings. Tribol. Int. 2009, 42, 600–608. [Google Scholar] [CrossRef]
- Belforte, G.; Raparelli, T.; Viktorov, V.; Trivella, A. Permeability and inertial coefficients of porous media for air bearing feeding systems. J. Tribol-T. Asme 2007, 129, 705–711. [Google Scholar] [CrossRef]
- Rodrigo, N.; Zilda, C.S.; Benedito, M.P. Modified Reynolds Equation for Aerostatic Porous Radial Bearings with Quadratic Forchheimer Pressure-Flow Assumption. J. Tribol-T. Asme 2008, 130, 031701. [Google Scholar]
- Amano, K.; Yoshimoto, S.; Miyatake, M.; Hirayama, T. Basic investigation of noncontact transportation system for large TFT-LCD glass sheet used in CCD inspection section. Precis. Eng. 2011, 35, 58–64. [Google Scholar] [CrossRef]
- Oiwa, N.; Masuda, M.; Hirayama, T.; Matsuoka, T.; Yabe, H. Deformation and flying height orbit of glass sheets on aerostatic porous bearing guides. Tribol. Int. 2012, 48, 2–7. [Google Scholar] [CrossRef]
- Miyatake, M.; Akahori, H.; Yoshimoto, S. Deformation of large liquid crystal display glass sheets across a gap between noncontact transportation devices. Precis. Eng. 2016, 46, 360–368. [Google Scholar] [CrossRef]
- Zhong, W.; Li, X.; Liu, F.H.; Tao, G.; Lu, B.; Kagawa, T. Measurement and Correlation of Pressure Drop Characteristics for Air Flow through Sintered Metal Porous Media. Transp. Porous. Med. 2014, 101, 53–67. [Google Scholar] [CrossRef]
- Zhong, W.; Xu, K.; Li, X.; Kagawa, T. Study on the basic characteristics of a noncontact air conveyor for large glass sheets. Adv. Mech. Eng. 2017, 9, 1–13. [Google Scholar] [CrossRef]
- Zhong, W.; Li, X.; Tao, G.L.; Kagawa, T. Measurement and Determination of Friction Characteristic of Air Flow through Porous Media. Metals 2015, 5, 336–349. [Google Scholar] [CrossRef] [Green Version]
- Zhong, W.; Xu, K.; Li, X.; Liao, T.; Tao, G.; Kagawa, K. Determination of pressure drop for air flow through sintered metal porous media using a modified Ergun equation. Adv. Powder Technol. 2016, 27, 1134–1140. [Google Scholar] [CrossRef]
- Kawashima, K.; Ishii, Y.; Funaki, T.; Kagawa, T. Determination of flow rate characteristics of pneumatic solenoid valves using an isothermal chamber. J. Fluid. Eng-T. Asme 2004, 126, 273–279. [Google Scholar] [CrossRef]
- Dukhan, N.; Ali, M. Strong wall and transverse size effects on pressure drop of flow through open-cell metal foam. Int. J. Therm. Sci. 2012, 57, 85–91. [Google Scholar] [CrossRef]
- Eisfeld, B.; Schnitzlein, K. The influence of confining walls on the pressure drop in packed beds. Chem. Eng. Sci. 2001, 56, 4321–4329. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Pour, M.S.; Jonsson, L.T.I.; Jönsson, P. Effect of fluid bypassing on the experimentally obtained Darcy and non-Darcy permeability parameters of ceramic foam filters. Metall. Mater. Trans. 2016, 48, 197–207. [Google Scholar] [CrossRef]
- Dybbs, A.; Edwards, R.V. A new look at porous media fluid mechanics—Darcy to Turbulent. In Fundamentals of Transport Phenomena in Porous Media; Bear, J., Corapcioglu, M.Y., Eds.; Springer Netherlands: Berlin, Germany, 1984; Volume 82, pp. 199–256. [Google Scholar]




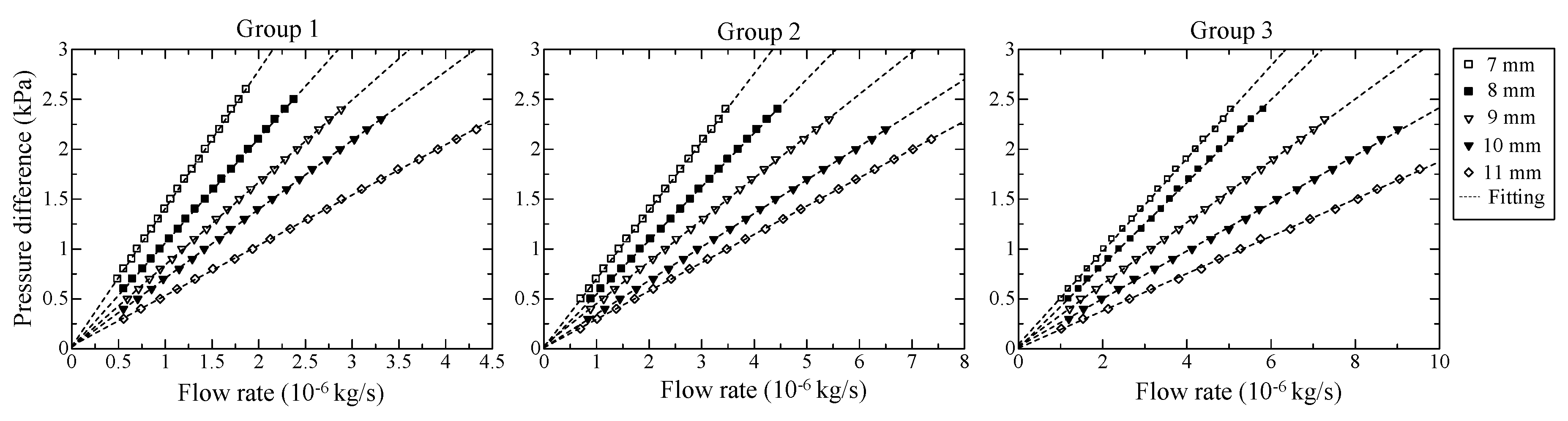



| Model | Authors | Material | Working Fluid |
|---|---|---|---|
| Forchheimer or Forchheimer-type equation | Liao et al., (2016) [1,2] | Sintered metal porous | Air |
| Lage et al., (1997) [3] | Aluminum porous media | Air | |
| Andrade et al., (1999) [4] | Assumed disorder porous media | Newtonian, Incompressible fluid | |
| Boomsma et al., (2002) [5] | Open-cell aluminum foams | Water | |
| Medraj et al., (2007) [6] | metallic foams | Air | |
| Beavers & Sparrow, (1969) [11] | Metallic fibers | Liquid | |
| Beavers et al., (1973) [12] | Packed beds of spheres | Liquid | |
| Antoheet al, (1997) [14] | Compressed aluminum porous matrices | Poly-alpha-olefin fluid | |
| Air | |||
| Dukhan & Minjeur, (2011) [15] | open-cell aluminum foam | Air | |
| Jin & Kai, (2008) [20] | Open-cell metal foams | Air | |
| Mancin et al., (2010) [21] | aluminum open-cell foam | Air | |
| Rodrigo et al., (2008) [24] | Bronze sintered porous bearing | Air | |
| Zhong et al., (2014) [28] | Sintered metal porous media | Air | |
| Dukhan & Ali, (2012) [33] | open-cell aluminum foam | Air | |
| Ergun or Ergun-type equation | Nihad et al., (2014) [8] | Packed spheres porous media | Water |
| aluminum foam | |||
| Liuet al, (2006) [16] | foam matrixes | Air | |
| Dukhan & Patel, (2008) [17] | Metal foam | Air | |
| Dietrich et al., (2009) [18] | Ceramic sponges | Air | |
| Zhong et al., (2016) [31] | Sintered metal porous media | Air | |
| A correlating equation | Montillet et al., (2007) [13] | packed beds of spheres | Liquid |
| A force balance flow model | Kim & Lu, (2008) [19] | Porous Medium-like Cylinder | Air |
| Bundles | |||
| Darcy Law | Amano et al., (2011) [25] | Porous bearing pad | Air |
| Oiwa et al., (2012) [26] | Porous bearing pad | Air |
| Porous Media | Estimated Particle Diameter (μm) | Length (mm) | Diameter (mm) | Porosity |
|---|---|---|---|---|
| Group 1 | 40–50 μm | 3 | 7, 8, 9, 10, 11 | 0.395, 0.392, 0.388, 0.389, 0.397 |
| Group 2 | 55–65 μm | 3 | 7, 8, 9, 10, 11 | 0.418, 0.419, 0.418, 0.418, 0.416 |
| Group 3 | 80–90 μm | 3 | 7, 8, 9, 10, 11 | 0.420, 0.412, 0.413, 0.420, 0.425 |
| Porous Medium | Group 1 | Group 2 | Group 3 | |||
|---|---|---|---|---|---|---|
| K (10−12 m2) | β | K (10−12 m2) | β | K (10−12 m2) | β | |
| 7 mm | 2.15 | 0.512 | 3.73 | 0.522 | 5.02 | 0.608 |
| 8 mm | 2.02 | 0.515 | 3.64 | 0.585 | 4.99 | 0.617 |
| 9 mm | 2.02 | 0.486 | 3.65 | 0.613 | 4.86 | 0.588 |
| 10 mm | 2.048 | 0.467 | 3.68 | 0.578 | 5.05 | 0.598 |
| 11 mm | 2.095 | 0.491 | 3.55 | 0.623 | 4.93 | 0.615 |
| Porous Medium | Group 1 | Group 2 | Group 3 | |||
|---|---|---|---|---|---|---|
| K (10−12 m2) | β | K (10−12 m2) | β | K (10−12 m2) | β | |
| 7 mm | 1.96 | 0.411 | 3.71 | 0.562 | 5.03 | 0.468 |
| 8 mm | 1.97 | 0.467 | 3.68 | 0.641 | 4.91 | 0.537 |
| 9 mm | 2.12 | 0.509 | 3.63 | 0.658 | 4.98 | 0.582 |
| 10 mm | 1.98 | 0.467 | 3.66 | 0.621 | 4.95 | 0.562 |
| 11 mm | 2.08 | 0.574 | 3.54 | 0.739 | 5.01 | 0.621 |
| Porous Medium | E (Group 1) | E (Group 2) | E (Group 3) | |||
|---|---|---|---|---|---|---|
| Steady-State Method | Discharge Method | Steady-State Method | Discharge Method | Steady-State Method | Discharge Method | |
| 7 mm | 0.97% | 2.16% | 0.88% | 2.39% | 1.03% | 2.55% |
| 8 mm | 0.59% | 0.80% | 1.09% | 1.64% | 1.21% | 1.78% |
| 9 mm | 1.94% | 1.18% | 0.51% | 2.34% | 0.77% | 1.28% |
| 10 mm | 1.60% | 2.26% | 0.93% | 1.91% | 0.62% | 1.25% |
| 11 mm | 2.08% | 2.74% | 1.02% | 2.31% | 0.58% | 0.76% |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, W.; Ji, X.; Li, C.; Fang, J.; Liu, F. Determination of Permeability and Inertial Coefficients of Sintered Metal Porous Media Using an Isothermal Chamber. Appl. Sci. 2018, 8, 1670. https://doi.org/10.3390/app8091670
Zhong W, Ji X, Li C, Fang J, Liu F. Determination of Permeability and Inertial Coefficients of Sintered Metal Porous Media Using an Isothermal Chamber. Applied Sciences. 2018; 8(9):1670. https://doi.org/10.3390/app8091670
Chicago/Turabian StyleZhong, Wei, Xiang Ji, Chong Li, Jiwen Fang, and Fanghua Liu. 2018. "Determination of Permeability and Inertial Coefficients of Sintered Metal Porous Media Using an Isothermal Chamber" Applied Sciences 8, no. 9: 1670. https://doi.org/10.3390/app8091670
APA StyleZhong, W., Ji, X., Li, C., Fang, J., & Liu, F. (2018). Determination of Permeability and Inertial Coefficients of Sintered Metal Porous Media Using an Isothermal Chamber. Applied Sciences, 8(9), 1670. https://doi.org/10.3390/app8091670





