Development of an Autonomous Underwater Helicopter with High Maneuverability
Abstract
1. Introduction
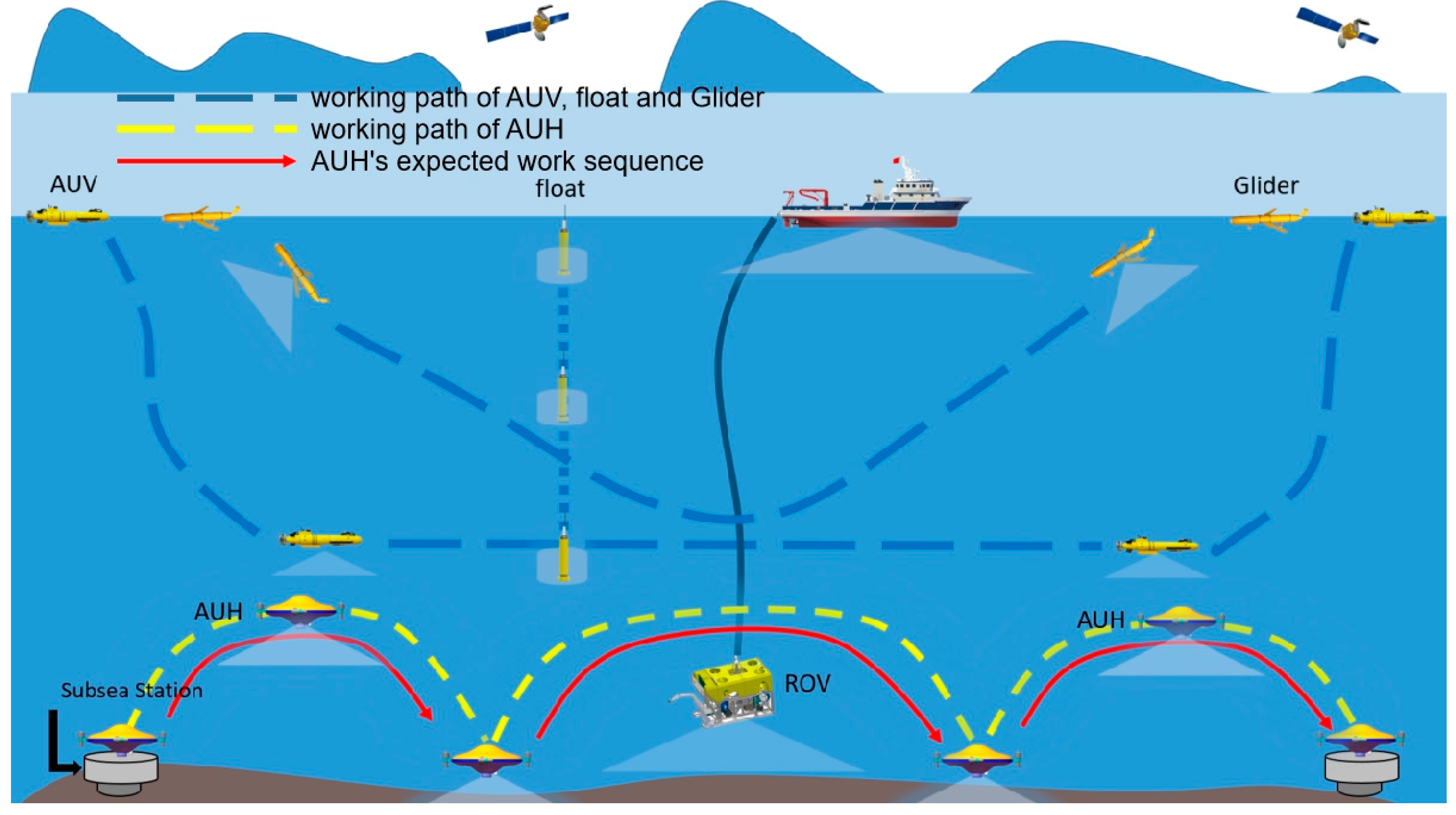
2. The Working Pattern of AUH
3. Dynamic Analysis of the AUH
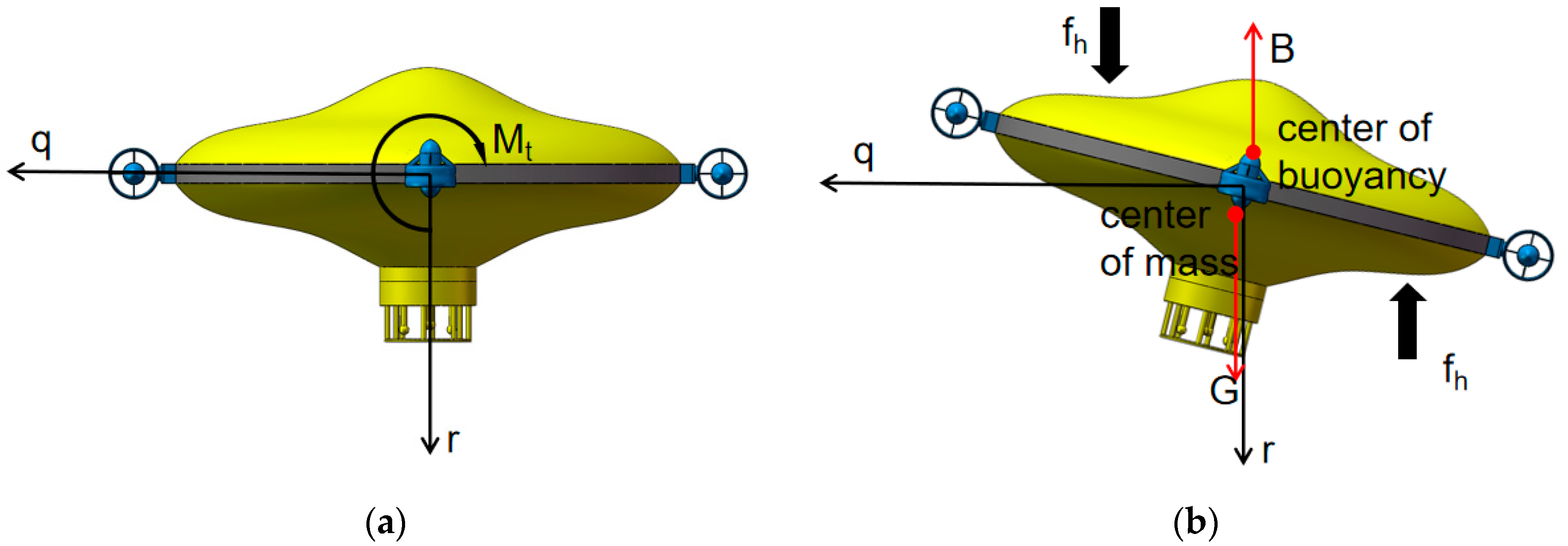
3.1. Dynamic Model of the AUH
3.2. Analysis of Motion in the Horizontal Plane
3.3. Analysis of Motion in the Vertical Direction
4. Mechatronics Design of AUH Prototype
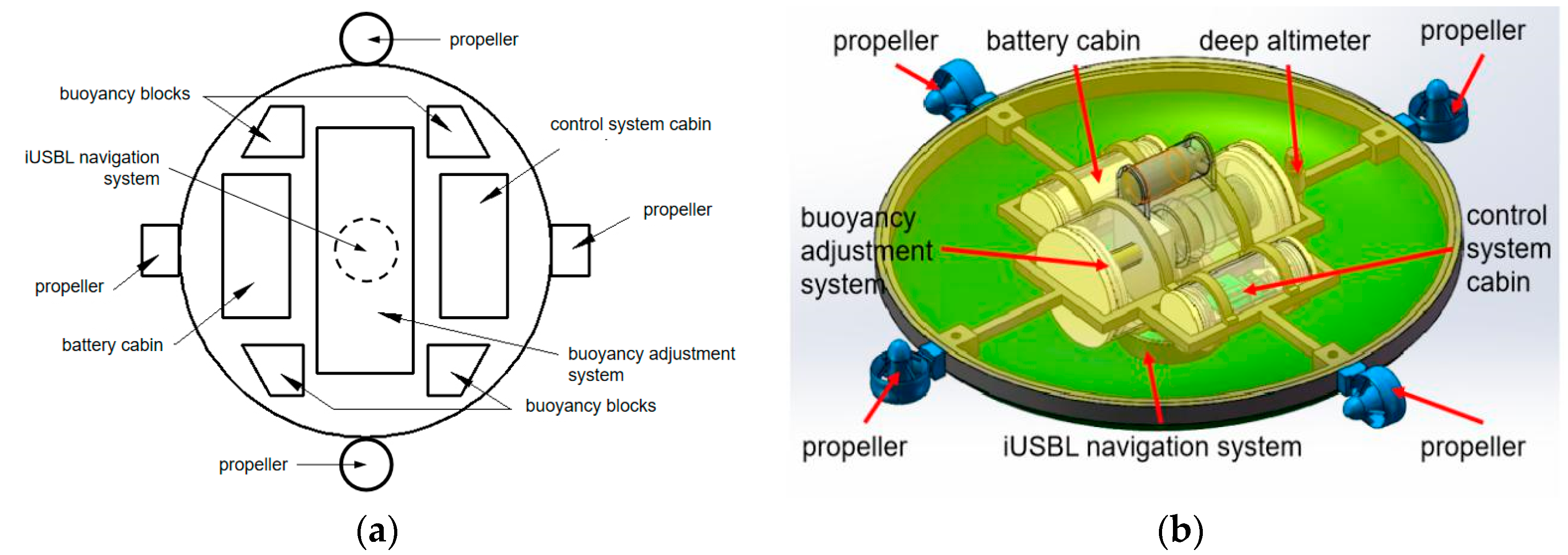
4.1. General Layout
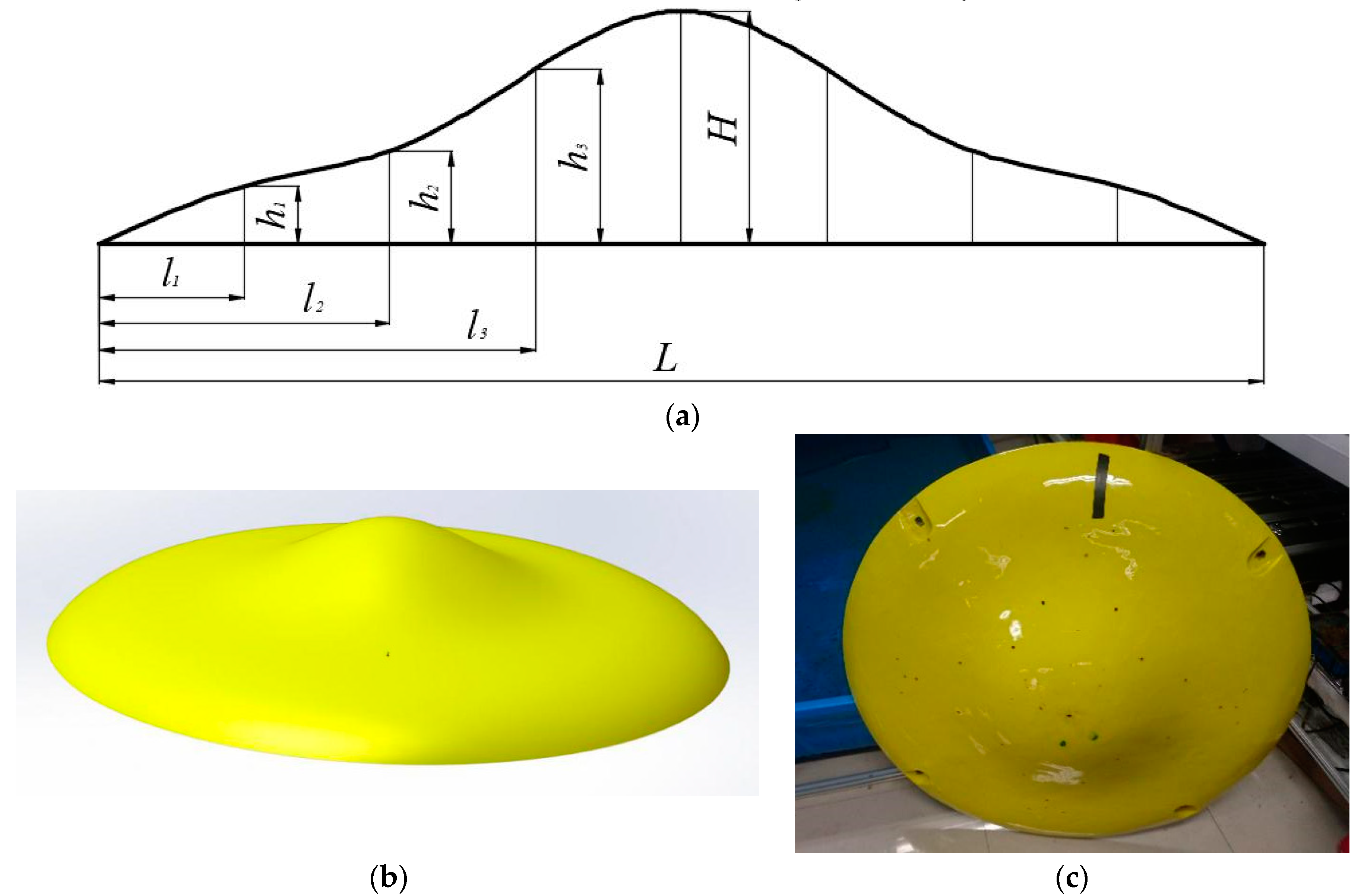
4.2. Disc-Shaped Hull
4.3. Propulsion Module
4.4. Positioning and Navigation System
4.5. Control System
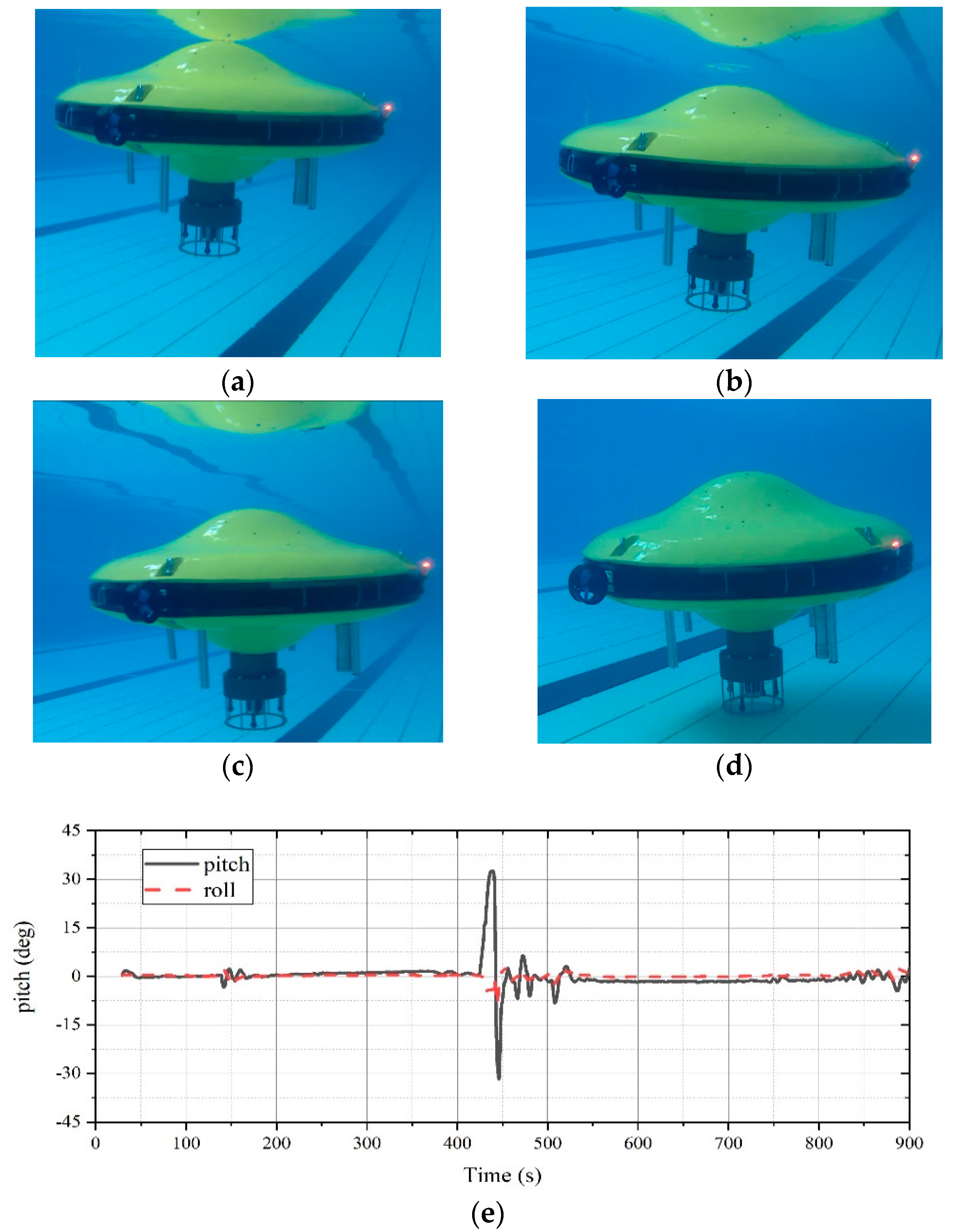
5. Experiments and Results
6. Conclusions
- (1)
- Because the special disc shape has the characteristics of horizontal in-plane isotropy, large vertical resistance, and small horizontal resistance, there is no need for over-actuation to achieve stable motion with high maneuverability within acceptable limits. It is feasible to increase endurance by reducing the number of propellers.
- (2)
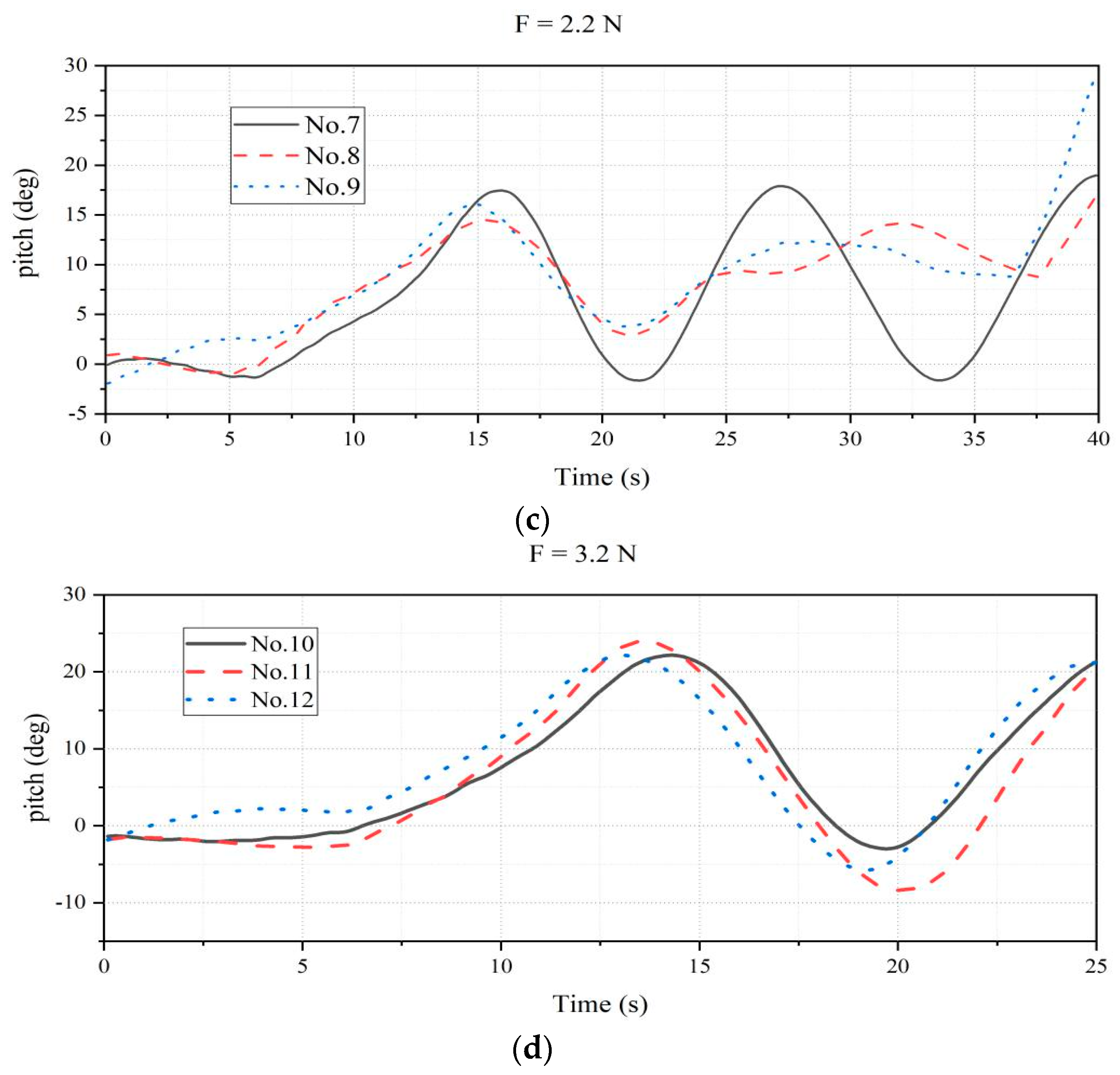
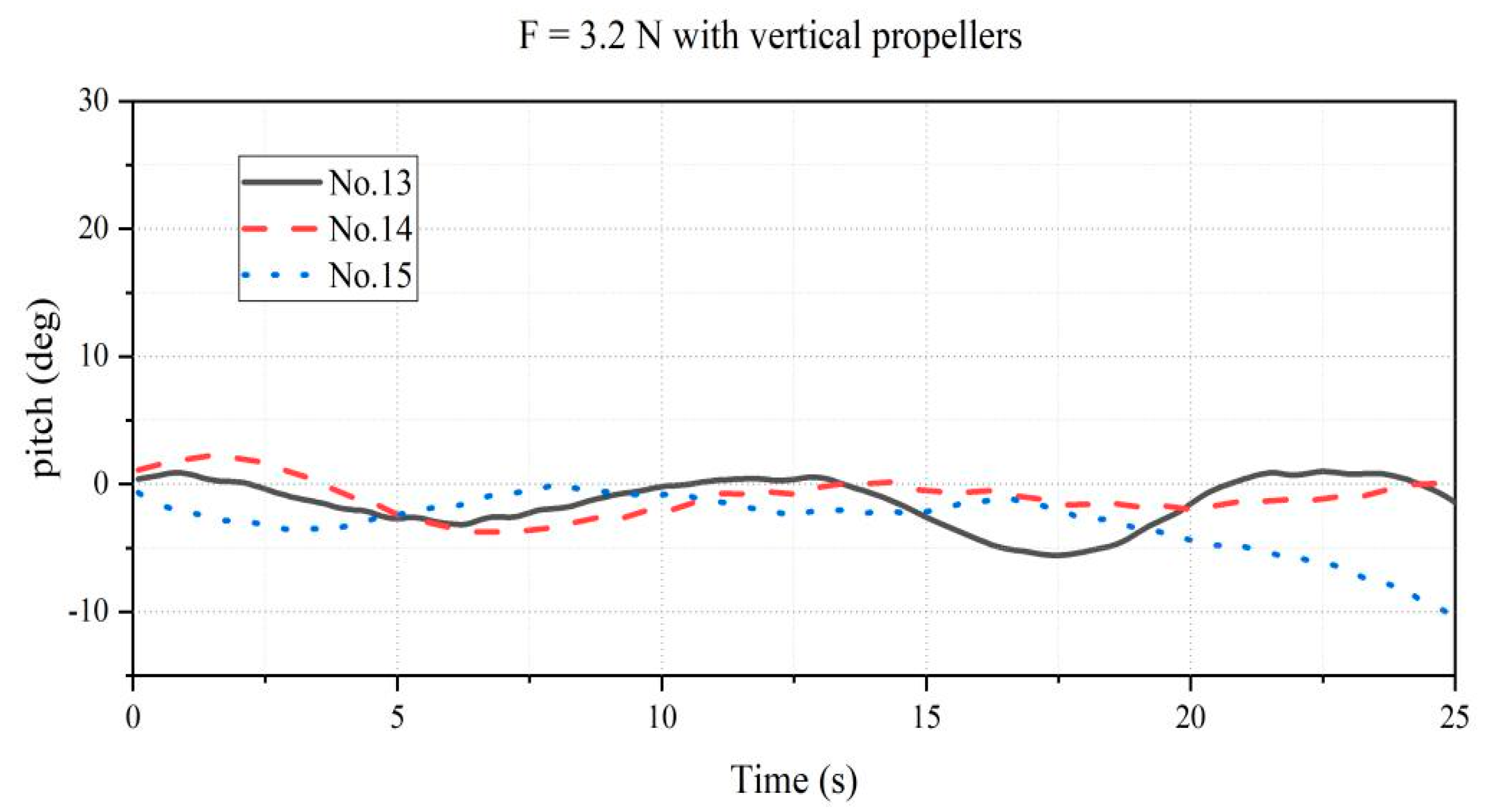
- At least four propellers are necessary for maneuverability of the AUH. The pitch vibration in the horizontal linear motion experiment is consistent with the prediction of dynamic analysis. To eliminate the harmful resistance and angle of attack caused by this vibration, a set of propellers that can provide an active recovery moment is required. Together with at least two propellers needed to complete the plane motion, four propellers in two groups are necessary for AUH to complete the stable motion of high maneuverability.
- (3)
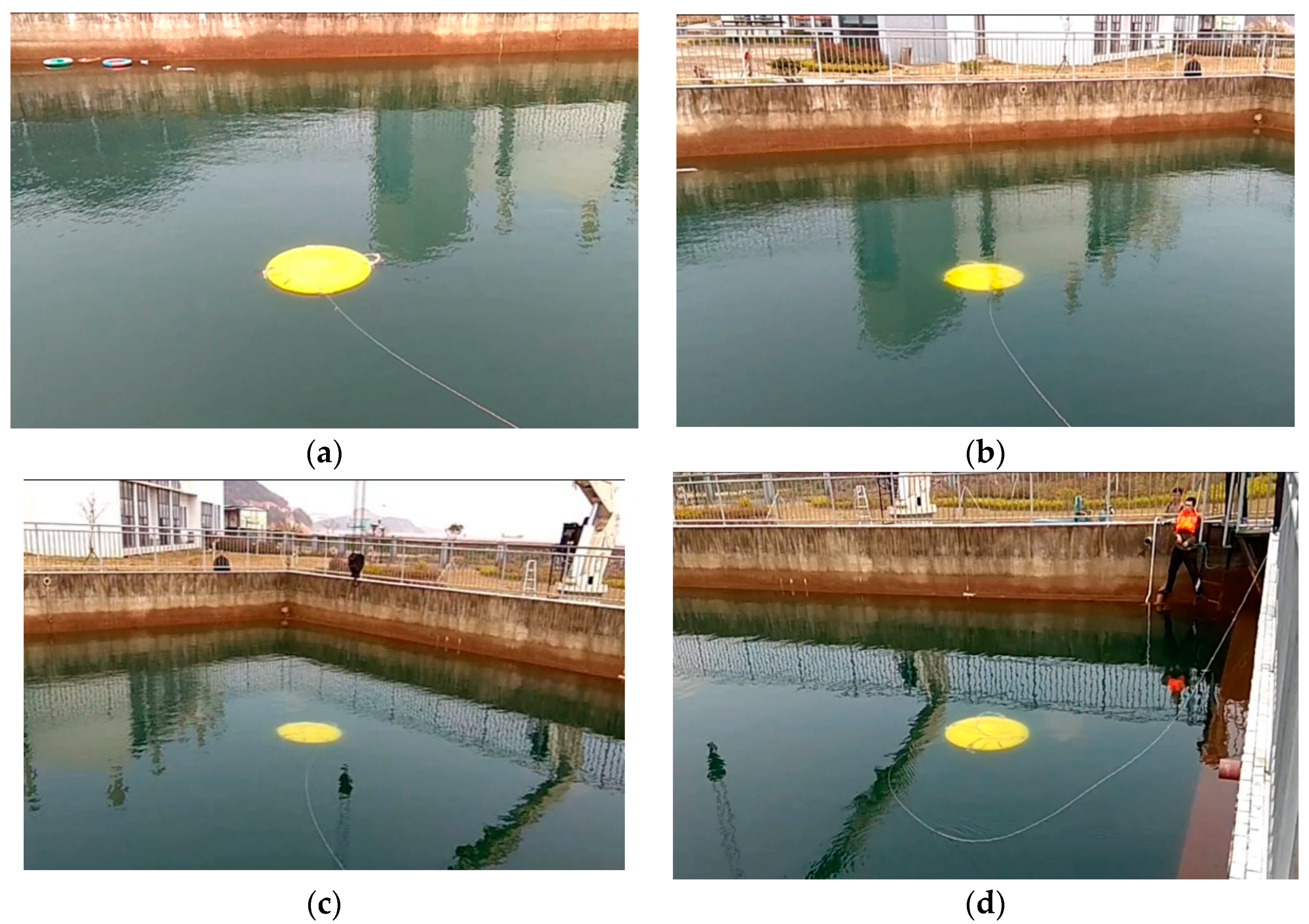
- The prototype of the AUH has the potential for high maneuverability. It has the potential to cruise in a small area flexibly, land on the seabed and take off. It is indicated that the AUH may be a solution for increasingly complex undersea engineering tasks, especially near-seabed operations.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wynn, R.B.; Huvenne, V.A.; Le Bas, T.P.; Murton, B.J.; Connelly, D.P.; Bett, B.J.; Ruhl, H.A.; Morris, K.J.; Peakall, J.; Parsons, D.R.; et al. Autonomous Underwater Vehicles (AUVs): Their past, present and future contributions to the advancement of marine geoscience. Mar. Geol. 2014, 352, 451–468. [Google Scholar] [CrossRef]
- Gafurov, S.A.; Klochkov, E.V. Autonomous Unmanned Underwater Vehicles Development Tendencies. Procedia Eng. 2015, 106, 141–148. [Google Scholar] [CrossRef]
- Ji, D.; Chen, C.; Chen, Y. Autonomous underwater helicopters. Sea Technol. 2018, 59, 25–27. [Google Scholar]
- Li, M.; Liu, S.; Wang, B. Review and Analysis on the Development of Ocean Environment Observation Technologies Based on Mooring Platforms. J. Ocean Technol. 2015, 34, 36–42. [Google Scholar]
- Phillips, A.B.; Furlong, M.; Turnock, S.R. The Use of Computational Fluid Dynamics to Determine the Dynamic Stability of an Autonomous Underwater Vehicle. Available online: https://www.researchgate.net/publication/242079237_The_use_of_Computational_Fluid_Dynamics_to_Determine_the_Dynamic_Stability_of_an_Autonomous_Underwater_Vehicle?ev=auth_pub (accessed on 6 December 2017).
- Zhang, C. The Design and Motion Control of Round Dish-shaped Underwater Vehicle. Master’s Thesis, Harbin Engineering University, Harbin, Heilongjiang, China, 2013. [Google Scholar]
- Jiang, Y. Computational Fluid Dynamics Study of Hydrodynamic and Motion Stability of an Autonomous Underwater Helicopter. Master Thesis, Zhejiang University, Zhoushan, Zhejiang, China, 2018. [Google Scholar]
- Zhang, F. Research of Disk type Underwater Glider’s Structural Stability and Strength. Master’s Thesis, Dalian Maritime University, Dalian, Shandong, China, 2017. [Google Scholar]
- Zhao, B. Study of modeling a simulation of disc-underwater glider dynamic stability. Ship Sci. Technol. 2016, 7, 82–86. [Google Scholar]
- Singh, Y.; Bhattacharyya, S.K.; Idichandy, V.G. CFD approach to modelling, hydrodynamic analysis and motion characteristics of a laboratory underwater glider with experimental results. J. Ocean Eng. Sci. 2017, 2, 90–119. [Google Scholar] [CrossRef]
- Bustos, C.; Sanchez, J.; Garcia, L.G.; Orozco, J.P.; Piedra, S. CFD Modeling of the Hydrodynamics of the CIDESI Underwater Glider. In Proceedings of the OCEANS 2018 MTS/IEEE, Charleston, SC, USA, 22–25 October 2018. [Google Scholar]
- Niewiadomska, K.; Jones, C.; Webb, D. Design of a mobile and bot-tom resting autonomous underwater gliding vehicle. In Proceedings of the 13th International Symposium on Unmanned Untethered Submersible Technology, Durham, NH, USA, 24–27 August 2003; Autonomous Undersea Systems Institute: Old Concord Turnpike Lee, NH, USA, 2003. [Google Scholar]
- Nakamura, M.; Ito, Y.; Koterayama, W.; Inada, M.; Noda, J.; Marubayashi, K.; Kanehara, H.; Aoshima, T.; Yoshikawa, Y.; Oda, H. Development of Disk Type Underwater Glider for Virtual Mooring-Part 3, Construction of Prototype Vehicle and Field Experiments. J. Jpn. Soc. Nav. Archit. Ocean Eng. 2013, 18, 157–166. [Google Scholar]
- Nakamura, M.; Hyodo, T.; Koterayama, W. “LUNA” test bed vehicle for virtual mooring. In Proceedings of the Seventeenth International Offshore and Polar Engineering Conference, Fukuoka, Japan, 1–6 July 2007; International Society of Offshore and Polar Engineers: Cupertino, CA, USA, 2007. [Google Scholar]
- Yu, P.; Wang, T.; Zhou, H.; Shen, C. Dynamic modeling and three-dimensional motion simulation of a disk type underwater glider. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 318–328. [Google Scholar] [CrossRef]
- Nakamura, M.; Koterayama, W.; Inada, M.; Marubayashi, K.; Hyodo, T.; Yoshimura, H.; Morii, Y. Disk-type underwater glider for virtual mooring and field experiment. Int. J. Offshore Polar Eng. 2009, 19, 66–70. [Google Scholar]
- Pyo, J. Development of hovering type AUV “cyclops” and its performance evaluation using image mosaicing. Ocean Eng. 2015, 109, 517–530. [Google Scholar] [CrossRef]
- Wu, J. Structure Design and Analysis of a Small Disk-shape Autonomous Underwater Helicopter. Master’s Thesis, Zhejiang University, Zhoushan, Zhejiang, China, 2019. [Google Scholar]
- Ma, Q. Structure and Control System Research of Teaching ROV. Master’s Thesis, Zhejiang University, Hangzhou, Zhejiang, China, 2016. [Google Scholar]
- Chen, C.W.; Jiang, Y.; Huang, H.C.; Ji, D.X.; Sun, G.Q.; Yu, Z.; Chen, Y. Computational fluid dynamics study of the motion stability of an autonomous underwater helicopter. Ocean Eng. 2017, 143, 227–239. [Google Scholar] [CrossRef]
- Rypkema, N.R.; Fischell, E.M.; Schmidt, H. One-way travel-time inverted ultra-short baseline localization for low-cost autonomous underwater vehicles. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; pp. 4920–4926. [Google Scholar] [CrossRef]








| 12 V | 16 V | |
|---|---|---|
| Max thrust (Forward) | 34.8 N | 50.0 N |
| Max thrust (Backward) | 29.4 N | 40.2 N |
| Min thrust | 0.098 N | |
| speed | ||
| Max current | 25 Amps | |
| Max power | 350 Watt | |
| Point | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Fx | No. | Time between Two Points (s) | Average Velocity (m/s) | ||||||||
| 0.7 N | 1 | 16.0 | 11.8 | 10.2 | 10.0 | 9.9 | 9.8 | 10.3 | 11.6 | 10.7 | 0.09 |
| 2 | 17.5 | 11.7 | 10.6 | 9.5 | 10.3 | 9.5 | 9.3 | 10.3 | 10.4 | 0.10 | |
| 3 | 20.4 | 12.3 | 10.5 | 9.0 | 8.3 | 9.3 | 8.4 | 9.3 | 10.4 | 0.10 | |
| 1.3 N | 4 | 13.5 | 8.2 | 7.6 | 7.2 | 7.9 | 6.7 | 7.0 | 7.2 | 6.3 | 0.14 |
| 5 | 15.8 | 10.2 | 7.9 | 8.1 | 7.6 | 7.1 | 7.0 | 6.3 | 6.0 | 0.13 | |
| 6 | 14.6 | 9.2 | 7.3 | 7.2 | 6.5 | 7.0 | 6.3 | 5.9 | 6.4 | 0.14 | |
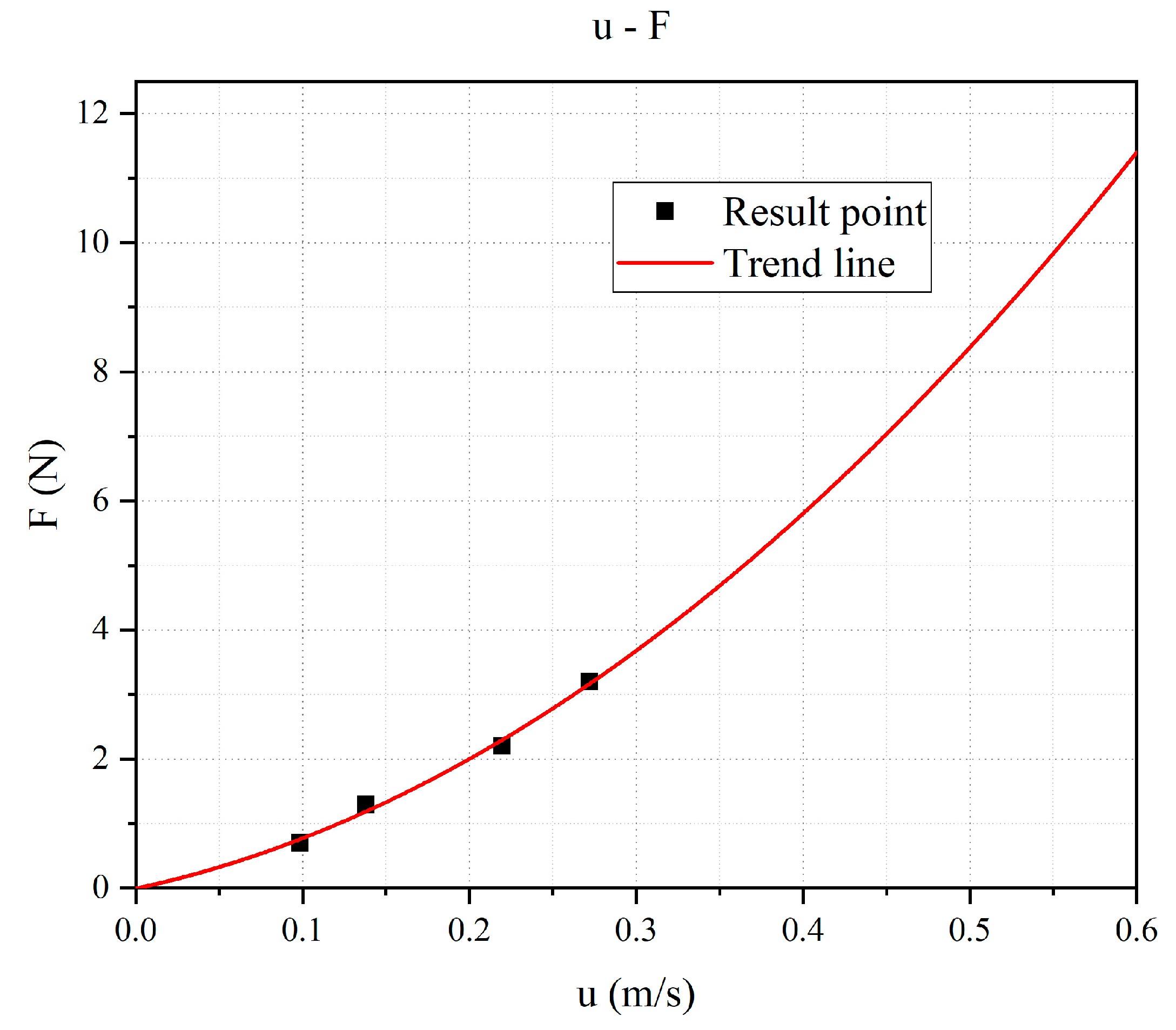
| 2.2 N | 7 | 7.1 | 5.5 | 4.4 | 4.6 | 3.9 | 4.3 | 4.7 | 4.4 | 4.1 | 0.22 |
| 8 | 8.4 | 5.6 | 4.3 | 4.1 | 5.2 | 4.2 | 4.3 | 4.3 | 4.1 | 0.22 | |
| 9 | 8.0 | 5.4 | 4.8 | 4.6 | 4.2 | 4.9 | 4.4 | 4.7 | 4.3 | 0.21 | |
| 3.2 N | 10 | 6.7 | 4.3 | 4.2 | 3.2 | 3.8 | 3.5 | / 1 | / | / | 0.26 |
| 11 | 6.2 | 4.2 | 3.5 | 3.3 | 3.3 | 3.2 | / | / | / | 0.29 | |
| 12 | 6.9 | 4.0 | 3.6 | 3.4 | 4.0 | 3.7 | / | / | / | 0.27 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Liu, X.; Huang, H.; Chen, Y. Development of an Autonomous Underwater Helicopter with High Maneuverability. Appl. Sci. 2019, 9, 4072. https://doi.org/10.3390/app9194072
Wang Z, Liu X, Huang H, Chen Y. Development of an Autonomous Underwater Helicopter with High Maneuverability. Applied Sciences. 2019; 9(19):4072. https://doi.org/10.3390/app9194072
Chicago/Turabian StyleWang, Zhikun, Xun Liu, Haocai Huang, and Ying Chen. 2019. "Development of an Autonomous Underwater Helicopter with High Maneuverability" Applied Sciences 9, no. 19: 4072. https://doi.org/10.3390/app9194072
APA StyleWang, Z., Liu, X., Huang, H., & Chen, Y. (2019). Development of an Autonomous Underwater Helicopter with High Maneuverability. Applied Sciences, 9(19), 4072. https://doi.org/10.3390/app9194072






