Adsorption of Ni2+ and Cd2+ from Water by Calcium Alginate/Spent Coffee Grounds Composite Beads
Abstract
:1. Introduction
2. Materials and Methods
2.1. Spent Coffee Grounds (SCGs)
2.2. Preparation of the Calcium Alginate Beads and the Calcium Alginate/Spent Coffee Grounds Composite Beads
2.3. Adsorption Experiments and Kinetic Experiments
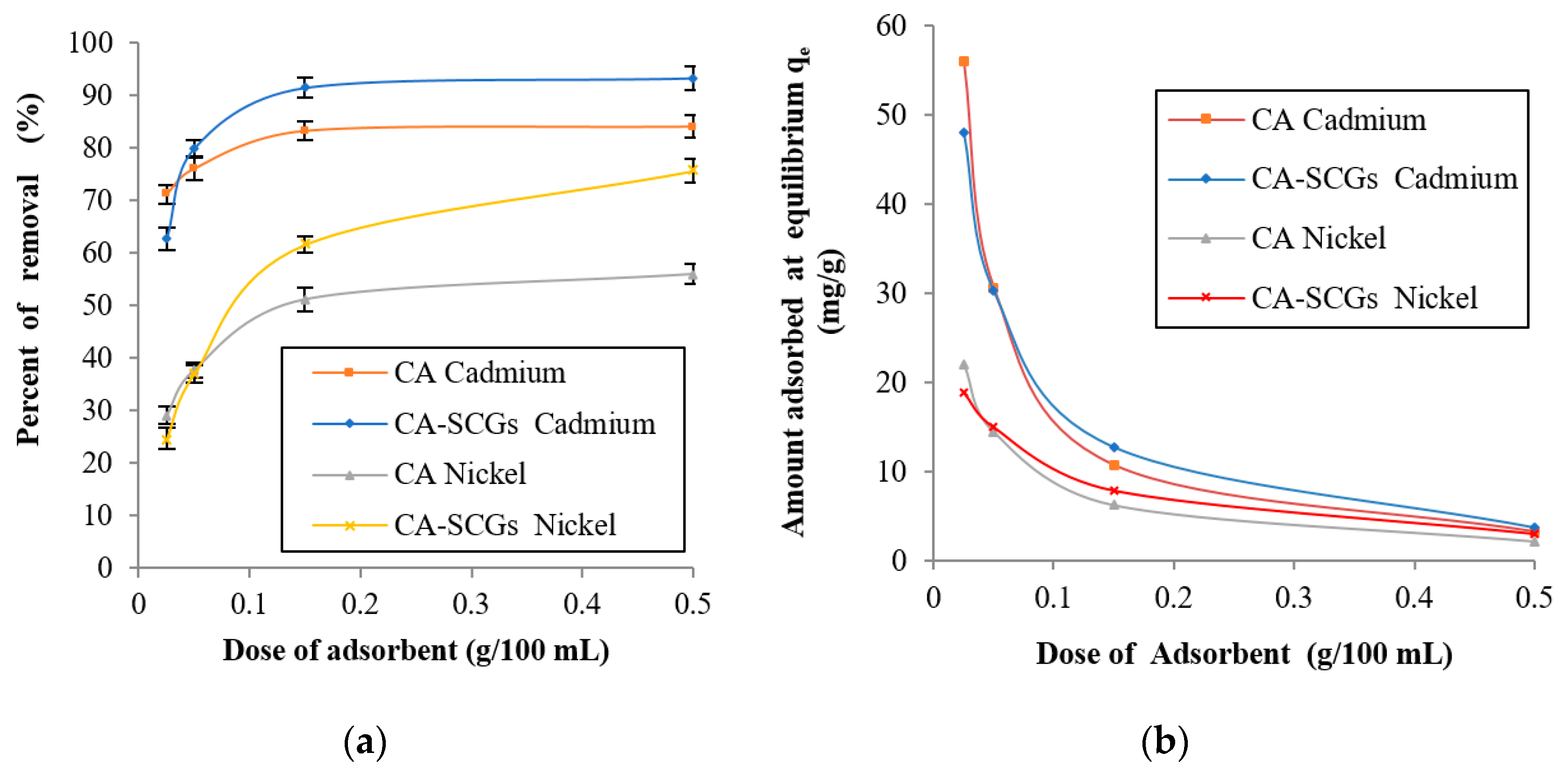
2.4. Adsorbent Dose Effect (Beads)
2.5. Adsorption/Desorption Studies
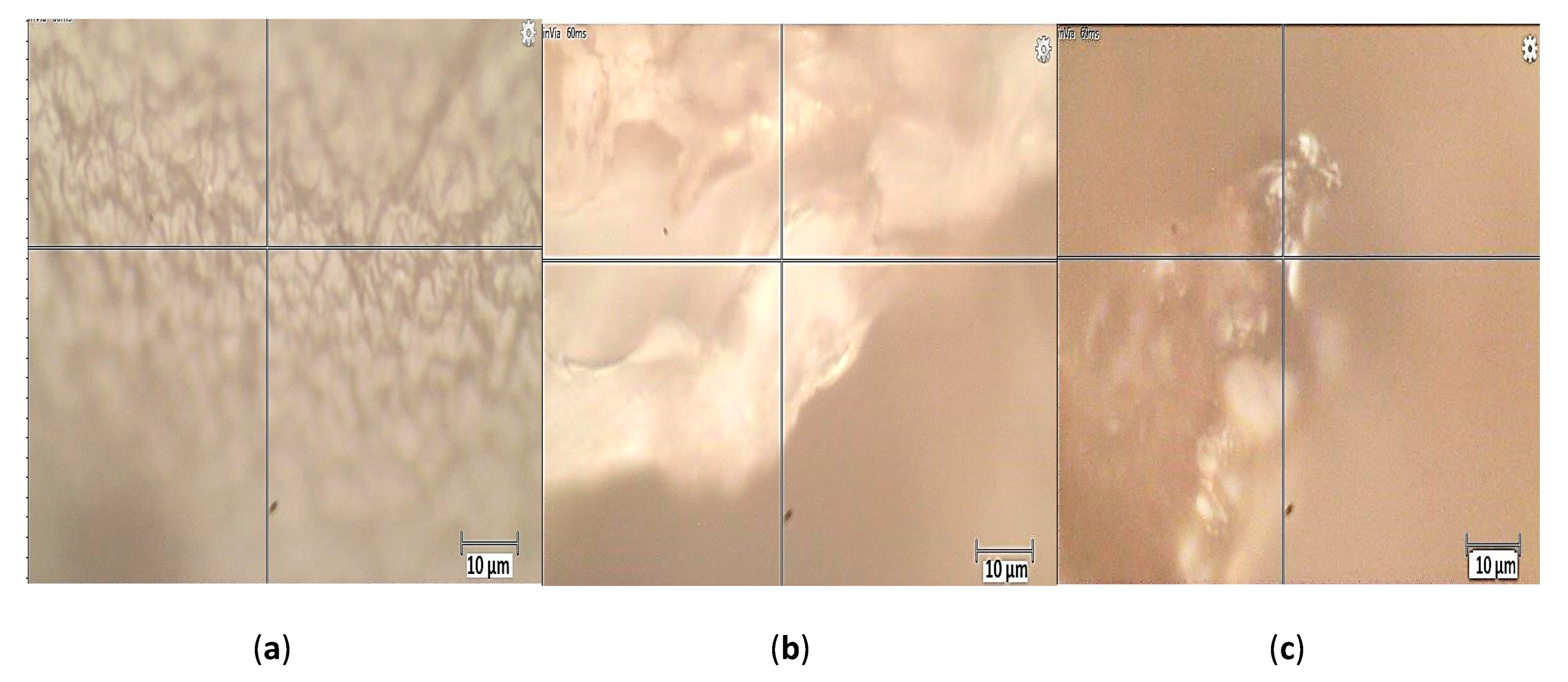
2.6. Characterization
3. Results and Discussion
3.1. Point of Zero Charge (pHpzc)
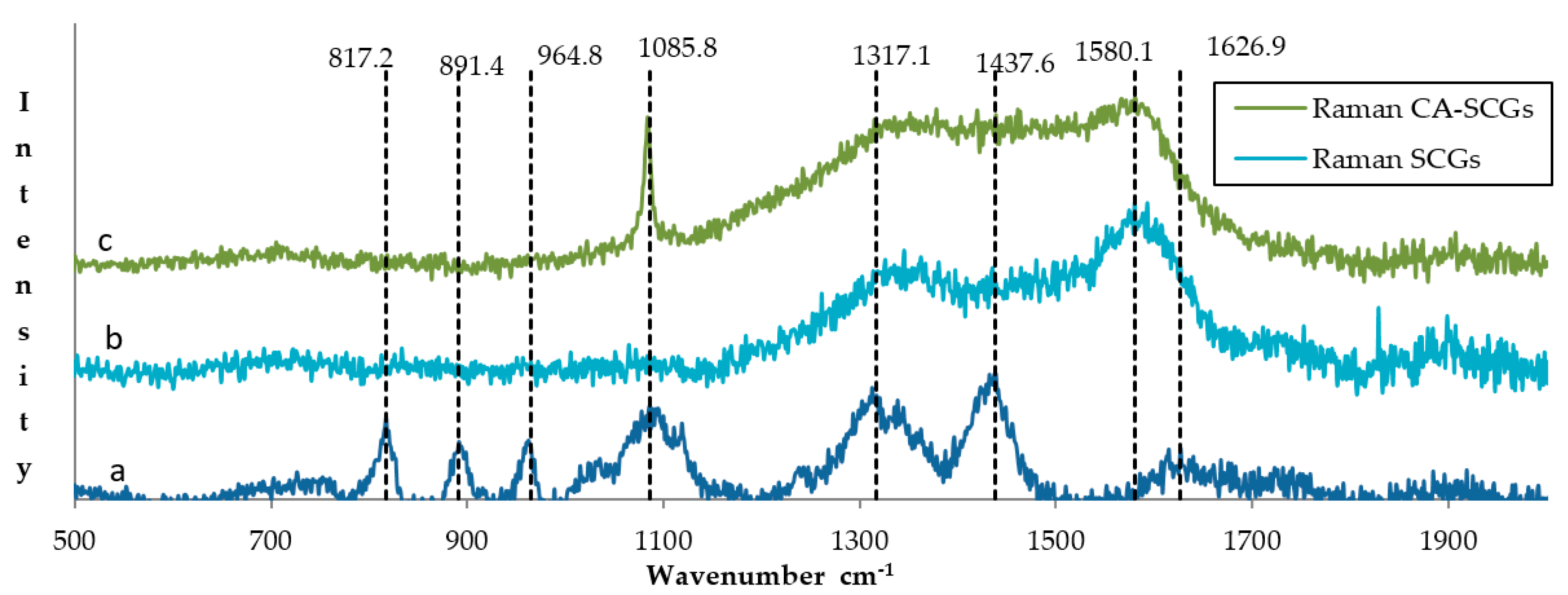
3.2. Characterization of CA Beads, CA–SCGs Beads, and SCGs by Raman Spectroscopy
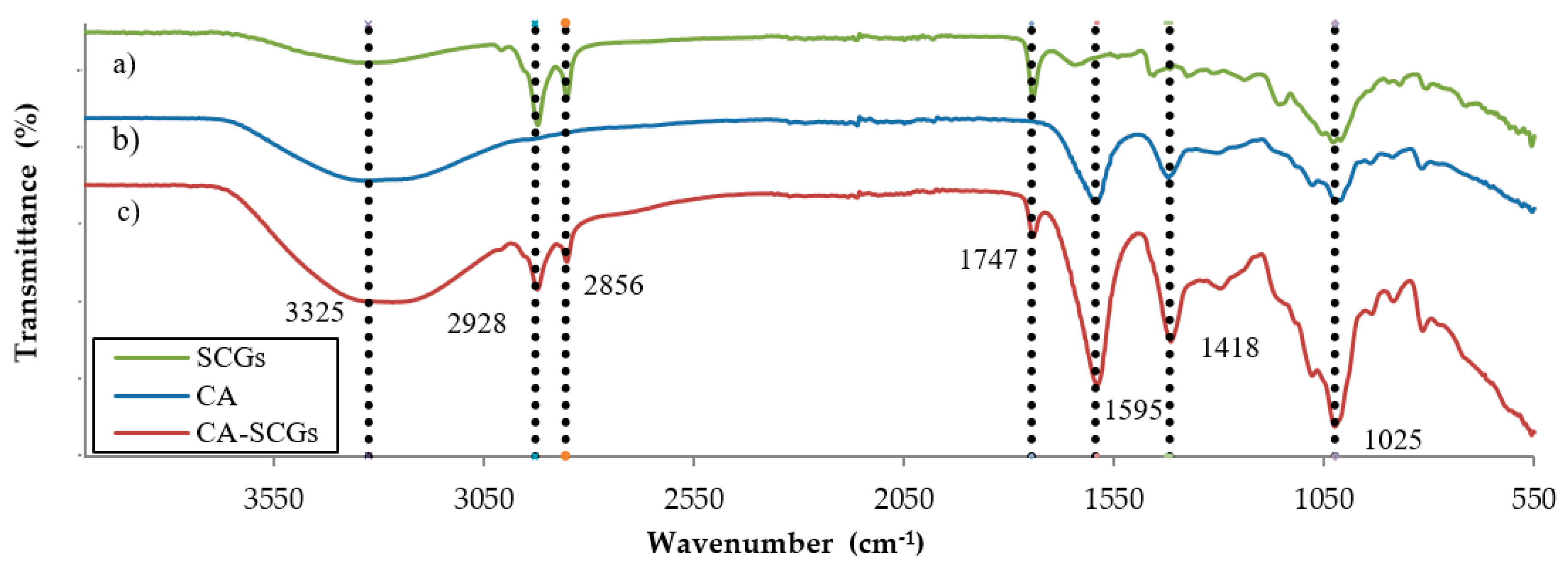
3.3. Characterization of CA Beads, CA–SCGs Beads, and SCGs by Fourier-Transform Infrared Spectroscopy (FT–IR)
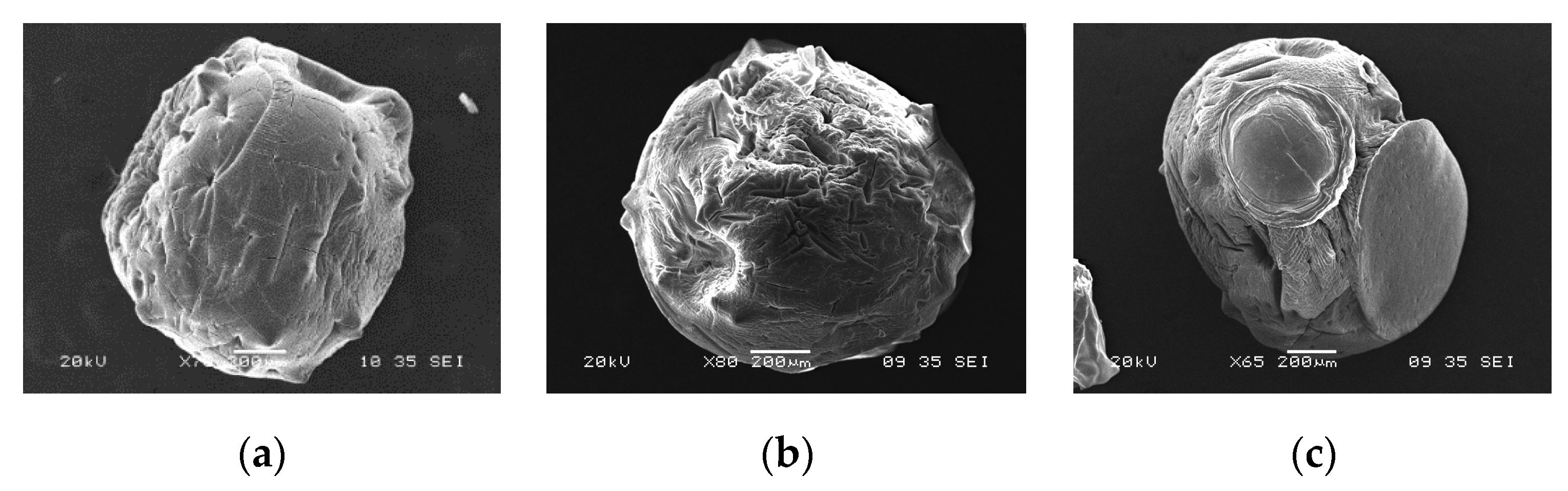
3.4. Characterization of CA Beads and CA–SCGs Beads by SEM–EDX Spectra
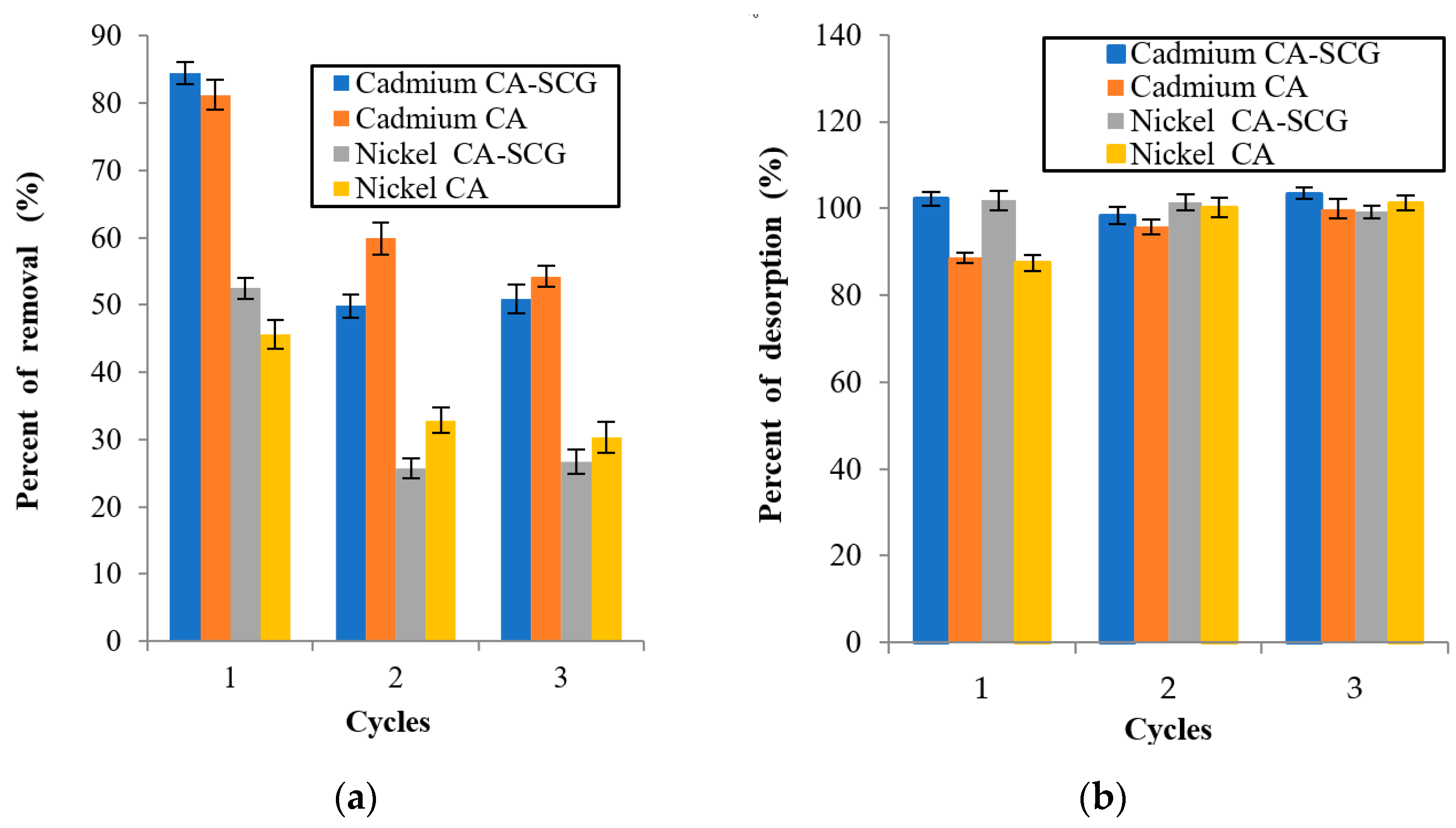
3.5. Adsorbent Dose Effect (Beads)
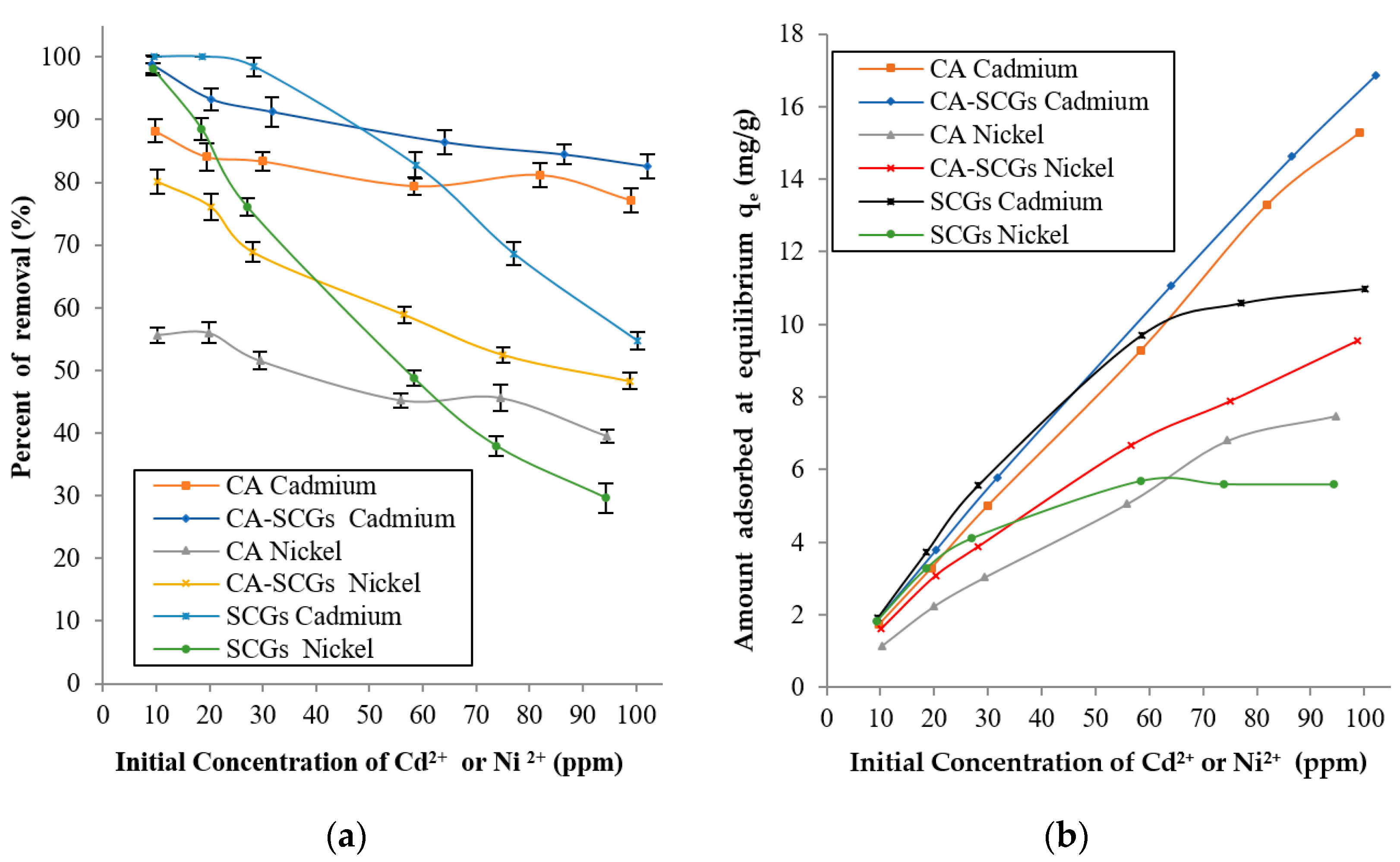
3.6. Adsorption Experiments
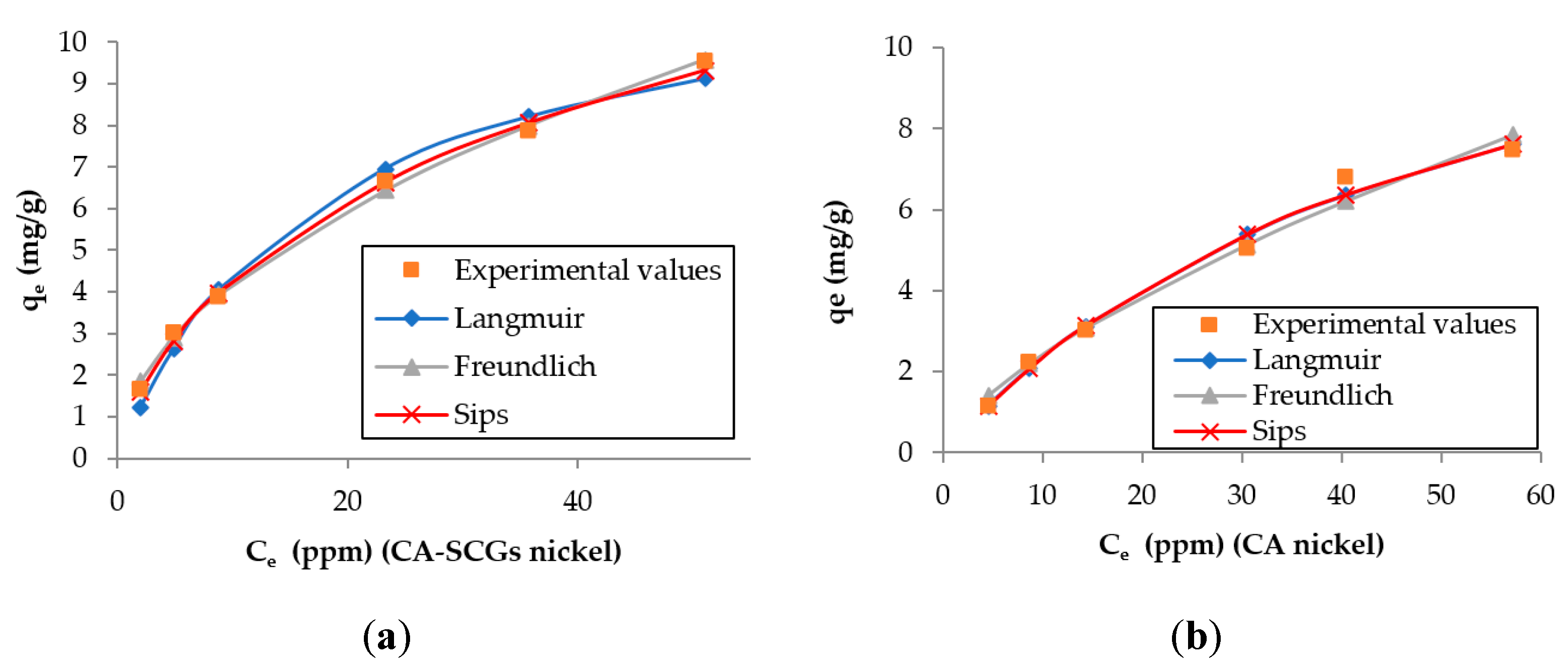
3.7. Analysis of Adsorption Isotherms
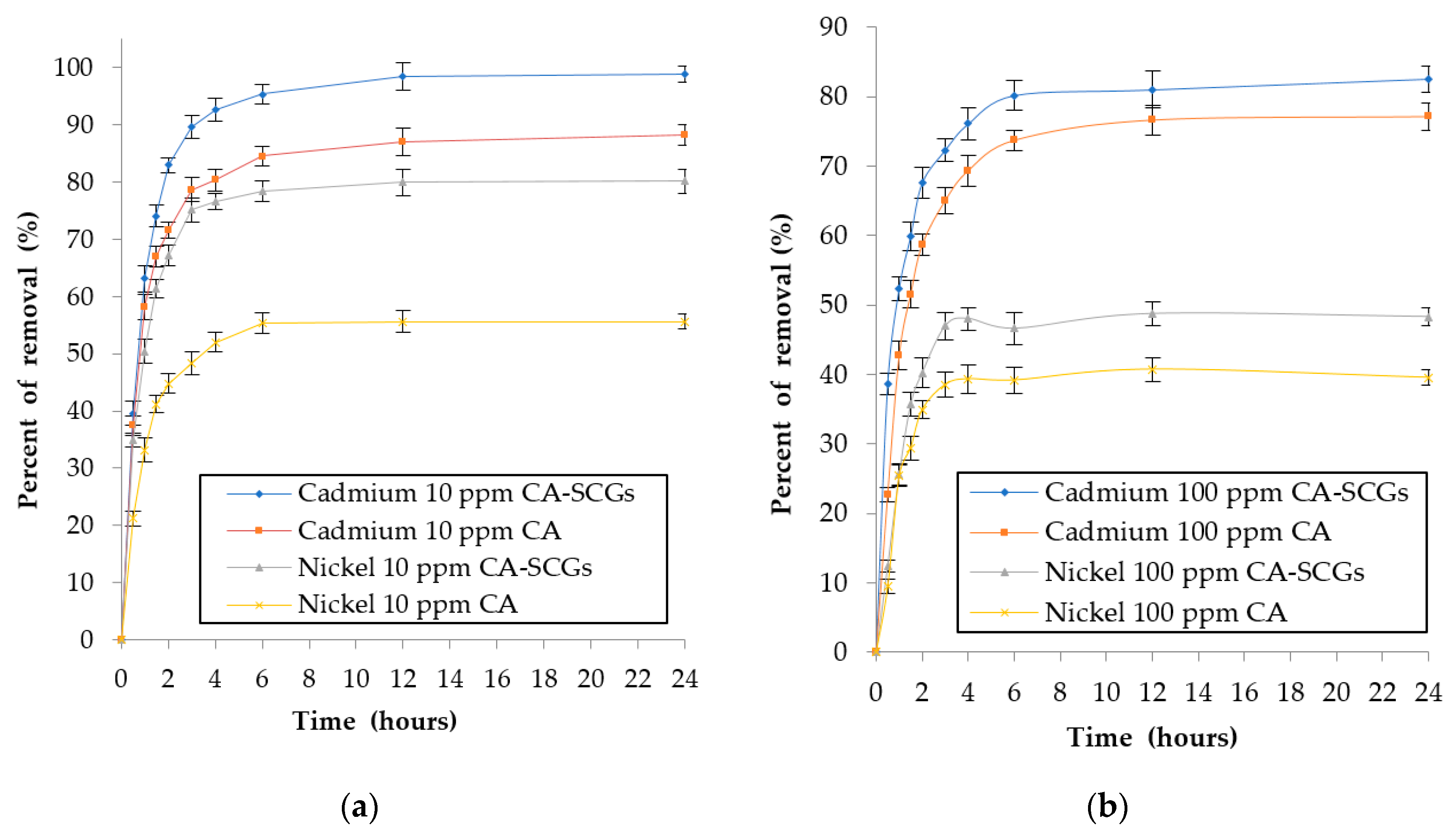
3.8. Kinetic Experiments
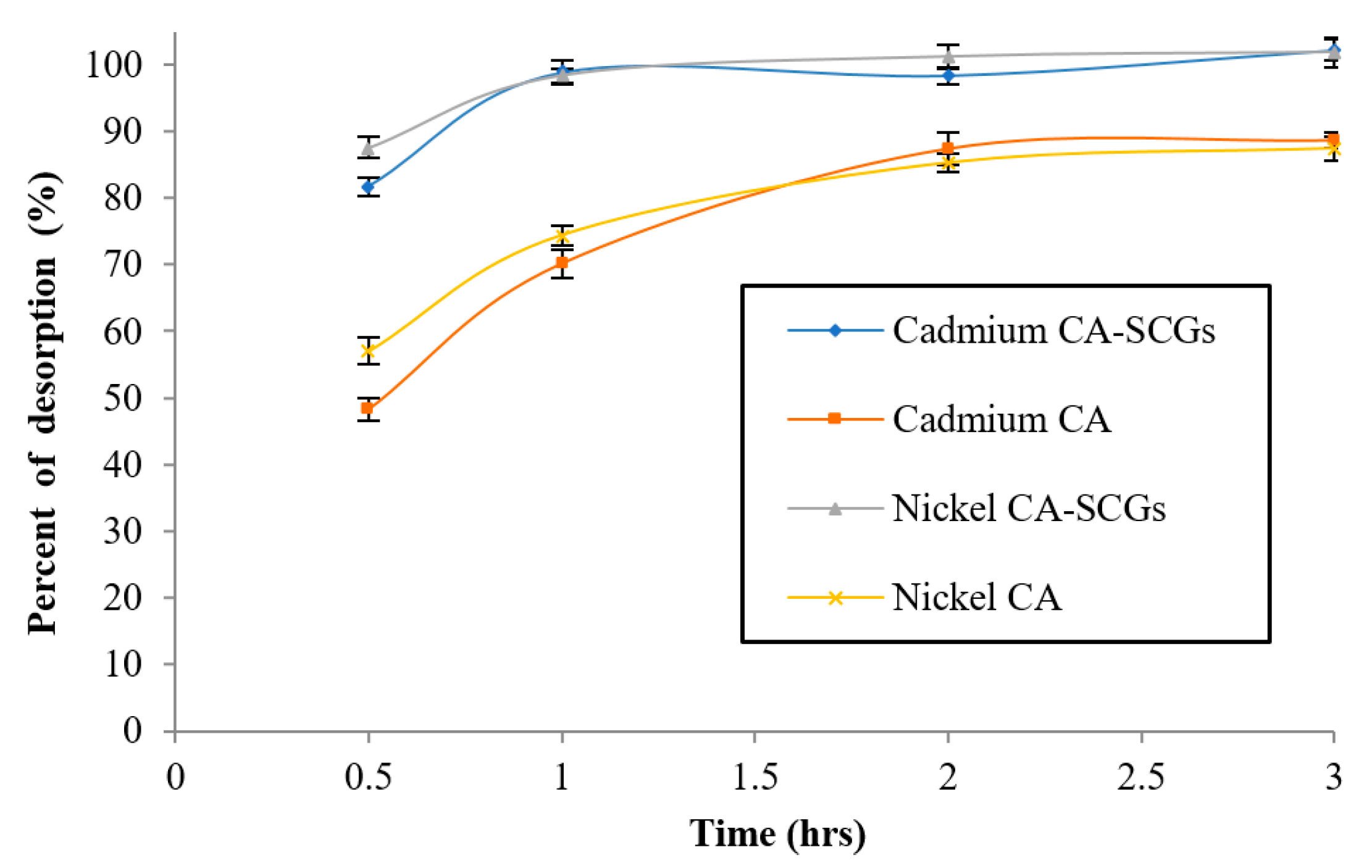
3.9. Adsorption/Desorption Studies
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tchounwou, P.B.; Yedjou, C.G.; Patlolla, A.K.; Sutton, D.J. Heavy metal toxicity and the environment. Exp. Suppl. 2012, 101, 133–164. [Google Scholar] [CrossRef] [PubMed]
- Olabemiwo, F.A.; Tawabini, B.S.; Patel, F.; Oyehan, T.A.; Khaled, M.; Laoui, T. Cadmium Removal from Contaminated Water Using Polyelectrolyte-Coated Industrial Waste Fly Ash. Bioinorg. Chem. Appl. 2017, 2017, 7298351. [Google Scholar] [CrossRef] [PubMed]
- Rafati Rahimzadeh, M.; Rafati Rahimzadeh, M.; Kazemi, S.; Moghadamnia, A.A. Cadmium toxicity and treatment: An update. Casp. J. Intern. Med. 2017, 8, 135–145. [Google Scholar] [CrossRef]
- Doll, R. Report of the International Committee on Nickel Carcinogenesis in Man. Scand. J. Work Environ. Health 1990, 16, 1–82. [Google Scholar] [CrossRef]
- Agency for Toxic Substances and Disease Registry (ATSDR). Toxicological Profile for Nickel (Update); Department of Public Health and Human Services, Public Health Service: Atlanta, GA, USA, 2005.
- Gruszecka-Kosowska, A.; Baran, P.; Wdowin, M.; Franus, W. Waste dolomite powder as an adsorbent of Cd, Pb(II), and Zn from aqueous solutions. Environ. Earth Sci. 2017, 76, 521. [Google Scholar] [CrossRef]
- Płaza, A.; Kołodyńska, D.; Hałas, P.; Gęca, M.; Franus, M.; Hubicki, Z. The zeolite modified by chitosan as an adsorbent for environmental applications. Adsorpt. Sci. Technol. 2017, 35, 834–844. [Google Scholar] [CrossRef] [Green Version]
- Franus, M.; Bandura, L.; Madej, J. Mono and Poly-Cationic Adsorption of Heavy Metals Using Natural Glauconite. Minerals 2019, 9, 470. [Google Scholar] [CrossRef]
- Pyrzynska, K. Removal of cadmium from wastewaters with low-cost adsorbents. J. Environ. Chem. Eng. 2019, 7, 102795. [Google Scholar] [CrossRef]
- Anastopoulos, I.; Karamesouti, M.; Mitropoulos, A.C.; Kyzas, G.Z. A review for coffee adsorbents. J. Mol. Liq. 2017, 229, 555–565. [Google Scholar] [CrossRef]
- De Gisi, S.; Lofrano, G.; Grassi, M.; Notarnicola, M. Characteristics and adsorption capacities of low-cost sorbents for wastewater treatment: A review. Sustain. Mater. Technol. 2016, 9, 10–40. [Google Scholar] [CrossRef] [Green Version]
- Jiménez-Zamora, A.; Pastoriza, S.; Rufián-Henares, J. Ángel Revalorization of coffee by-products. Prebiotic, antimicrobial and antioxidant properties. LWT Food Sci. Technol. 2015, 61, 12–18. [Google Scholar] [CrossRef]
- Vítěz, T.; Koutný, T.; Šotnar, M.; Chovanec, J. On the Spent Coffee Grounds Biogas Production. Acta Univ. Agric. Silvic. Mendel. Brun. 2016, 64, 1279–1282. [Google Scholar] [CrossRef] [Green Version]
- Azouaou, N.; Sadaoui, Z.; Djaafri, A.; Mokaddem, H. Adsorption of cadmium from aqueous solution onto untreated coffee grounds: Equilibrium, kinetics and thermodynamics. J. Hazard. Mater. 2010, 184, 126–134. [Google Scholar] [CrossRef] [PubMed]
- Papageorgiou, S.K.; Katsaros, F.K.; Kouvelos, E.P.; Nolan, J.W.; Le Deit, H.; Kanellopoulos, N.K. Heavy metal sorption by calcium alginate beads from Laminaria digitata. J. Hazard. Mater. 2006, 137, 1765–1772. [Google Scholar] [CrossRef]
- Wang, S.; Vincent, T.; Faur, C.; Guibal, E. Alginate and Algal-Based Beads for the Sorption of Metal Cations: Cu(II) and Pb(II). Int. J. Mol. Sci. 2016, 17, 1453. [Google Scholar] [CrossRef]
- Torres-Caban, R.; Vega-Olivencia, C.A.; Alamo-Nole, L.; Morales-Irizarry, D.; Roman-Velazquez, F.; Mina-Camilde, N. Removal of Copper from Water by Adsorption with Calcium-Alginate/Spent-Coffee-Grounds Composite Beads. Materials 2019, 12, 395. [Google Scholar] [CrossRef]
- Utomo, H.D.; Hunter, K.A. Adsorption of heavy metals by exhausted coffee grounds as a potential treatment method for waste waters. e-J. Surf. Sci. Nanotechnol. 2006, 4, 504–506. [Google Scholar] [CrossRef] [Green Version]
- Dávila-Guzmán, N.E.; de Jesús Cerino-Córdova, F.; Soto-Regalado, E.; Rangel-Mendez, J.R.; Díaz-Flores, P.E.; Garza-Gonzalez, M.T.; Loredo-Medrano, J.A. Copper Biosorption by Spent Coffee Ground: Equilibrium, Kinetics, and Mechanism. Clean Soil Air Water 2013, 41, 557–564. [Google Scholar] [CrossRef]
- Torres, E.; Mata, Y.N.; Blázquez, M.L.; Muñoz, J.A.; González, F.; Ballester, A. Gold and silver uptake and nanoprecipitation on calcium alginate beads. Langmuir 2005, 21, 7951–7958. [Google Scholar] [CrossRef]
- Li, Y.; Xia, B.; Zhao, Q.; Liu, F.; Zhang, P.; Du, Q.; Wang, D.; Li, D.; Wang, Z.; Xia, Y. Removal of copper ions from aqueous solution by calcium alginate immobilized kaolin. J. Environ. Sci. 2011, 23, 404–411. [Google Scholar] [CrossRef]
- Kołodyńska, D.; Krukowska, J.; Thomas, P. Comparison of sorption and desorption studies of heavy metal ions from biochar and commercial active carbon. Chem. Eng. J. 2017, 307, 353–363. [Google Scholar] [CrossRef]
- Jiao, Y.; Han, D.; Lu, Y.; Rong, Y.; Fang, L.; Liu, Y.; Han, R. Characterization of pine-sawdust pyrolytic char activated by phosphoric acid through microwave irradiation and adsorption property toward CDNB in batch mode. Desalin. Water Treat. 2017, 77, 247–255. [Google Scholar] [CrossRef]
- Schmid, T.; Messmer, A.; Yeo, B.S.; Zhang, W.; Zenobi, R. Towards chemical analysis of nanostructures in biofilms II: Tip-enhanced Raman spectroscopy of alginates. Anal. Bioanal. Chem. 2008, 391, 1907–1916. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, U.P. Analysis of Cellulose and Lignocellulose Materials by Raman Spectroscopy: A Review of the Current Status. Molecules 2019, 24, 1659. [Google Scholar] [CrossRef]
- Ballesteros, L.F.; Teixeira, J.A.; Mussatto, S.I. Chemical, Functional, and Structural Properties of Spent Coffee Grounds and Coffee Silverskin. Food Bioprocess Technol. 2014, 7, 3493. [Google Scholar] [CrossRef]
- Pujol, D.; Liu, C.; Gominho, J.; Olivella, M.; Fiol, N.; Villaescusa, I.; Pereira, H. The chemical composition of exhausted coffee waste. Ind. Crop. Prod. 2013, 50, 423–429. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, S.; Shan, X.-Q. Adsorption of metal ions on lignin. J. Hazard. Mater. 2008, 151, 134–142. [Google Scholar] [CrossRef]
- Algothmi, W.M.; Bandaru, N.M.; Yü, Y.; Shapter, J.G.; Ellis, A.V. Alginate–graphene oxide hybrid gel beads: An efficient copper adsorbent material. J. Colloid Interface Sci. 2013, 397, 32–38. [Google Scholar] [CrossRef]
- Baillot, R.; Deshayes, Y. Reliability Investigation of LED Devices for Public Light Applications; Elsevier: London, UK, 2017; pp. 43–106. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Hamdaoui, O.; Naffrechoux, E. Modeling of adsorption isotherms of phenol and chlorophenols onto granular activated carbonPart II. Models with more than two parameters. J. Hazard. Mater. 2007, 147, 401–411. [Google Scholar] [CrossRef]
- Günay, A.; Arslankaya, E.; Tosun, İ. Lead removal from aqueous solution by natural and pretreated clinoptilolite: Adsorption equilibrium and kinetics. J. Hazard. Mater. 2007, 146, 362–371. [Google Scholar] [CrossRef] [PubMed]
- Bolster, C.H.; Hornberger, G.M. On the Use of Linearized Langmuir Equations. Soil Sci. Soc. Am. J. 2008, 72, 1848. [Google Scholar] [CrossRef]
- Patterer, M.S.; Bavasso, I.; Sambeth, J.; Medici, F. Cadmium Removal from Acqueous Solution by Adsorption on Spent Coffee Grounds. Chem. Eng. Trans. 2017, 60, 157–162. [Google Scholar] [CrossRef]
- Al-Rub, F.; El-Naas, M.; Benyahia, F.; Ashour, I. Biosorption of nickel on blank alginate beads, free and immobilized algal cells. Process Biochem. 2004, 39, 1767–1773. [Google Scholar] [CrossRef]
- Attar, K.; Demey, H.; Bouazza, D.; Sastre, A.M. Sorption and Desorption Studies of Pb(II) and Ni(II) from Aqueous Solutions by a New Composite Based on Alginate and Magadiite Materials. Polymer 2019, 11, 340. [Google Scholar] [CrossRef]
- Nastaj, J.; Przewłocka, A.; Rajkowska-Myśliwiec, M. Biosorption of Ni(II), Pb(II) and Zn(II) on calcium alginate beads: Equilibrium, kinetic and mechanism studies. Pol. J. Chem. Technol. 2016, 18, 81–87. [Google Scholar] [CrossRef]
- Alfaro-Cuevas-Villanueva, R.; Hidalgo-Vázquez, A.R.; Cortés Penagos Cde, J.; Cortés-Martínez, R. Thermodynamic, kinetic, and equilibrium parameters for the removal of lead and cadmium from aqueous solutions with calcium alginate beads. Sci. World J. 2014, 2014, 647512. [Google Scholar] [CrossRef]
- Mahmood, Z.; Amin, A.; Zafar, U. Adsorption studies of cadmium ions on alginate–calcium carbonate composite beads. Appl. Water Sci. 2017, 7, 915. [Google Scholar] [CrossRef]
- Kwiatkowska-Marks, S.; Wojcik, M. Removal of Cadmium(II) from Aqueous Solutions by Calcium Alginate Beads. Sep. Sci. Technol. 2014, 49, 2204–2211. [Google Scholar] [CrossRef]
- Petrovič, A.; Simonič, M. Removal of heavy metal ions from drinking water by alginate-immobilised Chlorella sorokiniana. Int. J. Environ. Sci. Technol. 2016, 13, 1761–1780. [Google Scholar] [CrossRef]
- Bayramoğlu, G.; Tüzün, I.; Celik, G.; Yilmaz, M.; Arica, M.Y.; Arıca, M.Y. Biosorption of mercury(II), cadmium(II) and lead(II) ions from aqueous system by microalgae Chlamydomonas reinhardtii immobilized in alginate beads. Int. J. Miner. Process. 2006, 81, 35–43. [Google Scholar] [CrossRef]
- Ho, Y.; McKay, G. Pseudo-second order model for sorption processes. Process Biochem. 1999, 34, 451–465. [Google Scholar] [CrossRef]
- Hefne, J.A.; Mekhemer, W.K.; Alandis, N.M.; Aldayel, O.A.; Alajyan, T. Removal of silver (I) from Aqueous solutions by natural bentonite. JKAU Sci. 2010, 22, 155–176. [Google Scholar] [CrossRef]
- Jodra, Y.; Mijangos, F. Ion exchange selectivities of calcium alginate gels for heavy metals. Water Sci. Technol. 2001, 43, 237–244. [Google Scholar] [CrossRef]
- Davila-Guzman, N.E.; Cerino-Córdova, F.J.; Loredo-Cancino, M.; Rangel-Mendez, J.R.; Gómez-González, R.; Soto-Regalado, E. Studies of Adsorption of Heavy Metals onto Spent Coffee Ground: Equilibrium, Regeneration, and Dynamic Performance in a Fixed-Bed Column. Int. J. Chem. Eng. 2016, 2016, 9413879. [Google Scholar] [CrossRef]






| CA | CA–SCGs | CA After Adsorption | CA–SCGs After Adsorption | |||
|---|---|---|---|---|---|---|
| Ca2+ | Ca2+ | Ca2+/Ni2+ | Ca2+/Cd2+ | Ca2+/Ni2+ | Ca2+/Cd2+ | |
| 1 | 12.66 | 12.21 | 1.76/2.22 | 2.73/0.76 | 5.06/4.07 | 8.65/5.21 |
| 2 | 22.97 | 10.56 | 4.22/2.45 | 9.35/2.74 | 16.49/3.37 | 9.66/5.28 |
| 3 | 19.58 | 19.11 | 1.27/2.32 | 4.60/1.37 | 1.03/1.87 | 6.22/4.37 |
| 4 | 23.36 | 3.15 | 0.56/2.28 | 0.96/0.23 | 4.65/2.87 | 6.75/4.25 |
| 5 | 20.47 | 1.75 | 0.65/1.90 | 1.41/0.56 | 1.29/1.81 | 4.05/2.55 |
| 6 | 17.91 | 6.56 | 0.80/1.95 | 3.00/0.97 | 4.86/2.73 | 2.67/1.60 |
| Ave. | 19.49 | 8.38 | 1.54/2.19 | 3.68/1.11 | 5.56/2.79 | 6.33/3.88 |
| S.D. | 3.59 | 5.05 | 1.27/0.20 | 2.80/0.81 | 5.16/0.79 | 2.42/1.36 |
| Med. | 20.03 | 8.56 | 1.04/2.25 | 2.87/0.87 | 4.76/2.80 | 6.49/4.31 |
| M.A.D. | 2.53 | 4.49 | 0.43/0.14 | 1.60/0.41 | 1.89/0.75 | 2.30/0.94 |
| Langmuir (Cd2+) | SCGs | CA Beads | CA/SCGs Beads |
| qmax (mg/g) | 10.671 | 38.049 | 25.495 |
| bL (L/mg) | 2.415 | 0.031 | 0.101 |
| χ2 | 5.669 | 0.307 | 1.533 |
| Freundlich (Cd2+) | SCGs | CA Beads | CA/SCGs Beads |
| Kf (mg/g)(L/mg)(1/n) | 8.353 | 1.519 | 3.340 |
| 1/n | 0.054 | 0.752 | 0.563 |
| χ2 | 1.856 | 0.241 | 0.430 |
| Sips (Cd2+) | SCGs | CA Beads | CA/SCGs Beads |
| qmax (mg/g) | 12.752 | 38.650 | 91.184 |
| bs (L/mg) | 1.290 | 0.030 | 0.005 |
| 1/n | 0.453 | 0.993 | 0.633 |
| χ2 | 5.622 | 0.301 | 0.587 |
| Langmuir (Ni2+) | SCGs | CA Beads | CA/SCGs Beads |
| qmax (mg/g) | 5.608 | 14.668 | 12.320 |
| bL (L/mg) | 0.803 | 0.019 | 0.056 |
| χ2 | 0.818 | 0.063 | 0.191 |
| Freundlich (Ni2+) | SCGs | CA Beads | CA/SCGs Beads |
| Kf (mg/g)(L/mg)(1/n) | 2.854 | 0.508 | 1.299 |
| 1/n | 0.177 | 0.676 | 0.508 |
| χ2 | 0.115 | 0.137 | 0.0461 |
| Sips (Ni2+) | SCGs | CA Beads | CA/SCGs Beads |
| qmax (mg/g) | 7.813 | 14.224 | 20.958 |
| bs (L/mg) | 0.233 | 0.020 | 0.014 |
| 1/n | 0.391 | 1.015 | 0.698 |
| χ2 | 0.042 | 0.064 | 0.024 |
| Sorbents | pH | Sorption Capacity Cd2+ (mg/g) | Sorption Capacity Ni2+ (mg/g) | References |
|---|---|---|---|---|
| Free dead algal cells | - | - | 13.90 (L) | - |
| Blank alginate beads | - | - | 25.60 (L) | - |
| Immobilized dead algal cells | 5.0 | - | 31.30 (L) | [36] |
| Composite Based on Alginate and Magadiite | 4.0 | - | 44.36 (L) and 47.73 (S) | [37] |
| Alginate beads | 5.0 | - | 97.93 (L) | [38] |
| Alginate beads | 6.0–7.0 | 39.32 (L) | - | [39] |
| Alginate–calcium carbonate composite beads | 6.0 | 10.20 (L) | - | [40] |
| Alginate beads | 5.0 | 68.90 (L) | - | [41] |
| Alginate-immobilized Chlorella sorokiniana | 5.0 | 164.50 (L) | 86.49 (L) | [42] |
| Alginate beads | 5.0–6.0 | 31.40 (L) | - | - |
| Alginate-immobilized Chlamydomonas reinhardtii | 5.0–6.0 | 88.60 (L) | - | [43] |
| Alginate beads from Laminaria digitata | 4.5 | 130.77 (L) and 198.43(S) | - | [15] |
| Spent Coffee Grounds | - | 4.48 (L) | - | [35] |
| Spent Coffee Grounds | 5.0 | 11.60 (L) | [18] | |
| Spent Coffee Grounds | 6.0 | 10.67 (L) and 12.75 (S) | 5.61(L) and 7.81 (S) | [This work] |
| Alginate beads | 6.0 | 38.05 (L) and 38.65 (S) | 14.67 (L) and 14.22 (S) | [This work] |
| Alginate-Spent Coffee Grounds | 6.0 | 25.50 (L) and 91.18 (S) | 12.32 (L) and 20.96 (S) | [This work] |
| Adsorbent | R2 | qe Experimental (mg/g) | qe Calculated (mg/g) | k2 (g/mg-min) | h (mg/g-min) |
|---|---|---|---|---|---|
| CA–SCGs beads (Cd2+ 10 ppm) | 0.9992 | 1.8320 | 1.8702 | 0.0212 | 0.0742 |
| CA beads (Cd2+ 10 ppm) | 0.9994 | 1.7233 | 1.7844 | 0.0209 | 0.0665 |
| CA–SCGs beads (Ni2+ 10 ppm) | 0.9993 | 1.6240 | 1.6556 | 0.0271 | 0.0742 |
| CA beads (Ni2+ 10 ppm) | 0.9988 | 1.1400 | 1.1685 | 0.0304 | 0.0415 |
| CA–SCGs beads (Cd2+ 100 ppm) | 0.9994 | 16.8640 | 17.2414 | 0.0022 | 0.6536 |
| CA beads (Cd2+ 100 ppm) | 0.9981 | 15.2940 | 15.8730 | 0.0016 | 0.3953 |
| CA–SCGs beads (Ni2+ 100 ppm) | 0.9963 | 9.5436 | 9.8425 | 0.0033 | 0.3149 |
| CA beads (Ni2+ 100 ppm) | 0.9956 | 7.4822 | 7.7280 | 0.0047 | 0.2810 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Torres-Caban, R.; Vega-Olivencia, C.A.; Mina-Camilde, N. Adsorption of Ni2+ and Cd2+ from Water by Calcium Alginate/Spent Coffee Grounds Composite Beads. Appl. Sci. 2019, 9, 4531. https://doi.org/10.3390/app9214531
Torres-Caban R, Vega-Olivencia CA, Mina-Camilde N. Adsorption of Ni2+ and Cd2+ from Water by Calcium Alginate/Spent Coffee Grounds Composite Beads. Applied Sciences. 2019; 9(21):4531. https://doi.org/10.3390/app9214531
Chicago/Turabian StyleTorres-Caban, Roberto, Carmen A. Vega-Olivencia, and Nairmen Mina-Camilde. 2019. "Adsorption of Ni2+ and Cd2+ from Water by Calcium Alginate/Spent Coffee Grounds Composite Beads" Applied Sciences 9, no. 21: 4531. https://doi.org/10.3390/app9214531
APA StyleTorres-Caban, R., Vega-Olivencia, C. A., & Mina-Camilde, N. (2019). Adsorption of Ni2+ and Cd2+ from Water by Calcium Alginate/Spent Coffee Grounds Composite Beads. Applied Sciences, 9(21), 4531. https://doi.org/10.3390/app9214531





