Wireless Channel Models for Over-the-Sea Communication: A Comparative Study
Abstract
:1. Introduction
- Theoretical key concepts needed to understand channel models for over-the-sea radio wave propagation are briefly discussed.
- Channel models are examined in relation to their operational principles and key features.
- Channel models are classified based on their propagation type and the mobility of their transceiver platform.
- Channel models are compared with each other in terms of major characteristics, pros, and cons.
- The possibility of unique and novel applications and some possible future improvements of the channel models are addressed.
- Key challenging issues for modeling a wireless channel for over-the-sea communication and the research directions to design a new channel model are discussed.
2. Key Concepts in Modeling Over-the-Sea Channels
2.1. Important Definitions and Parameters
2.2. Measurements and Modeling of Propagation Loss
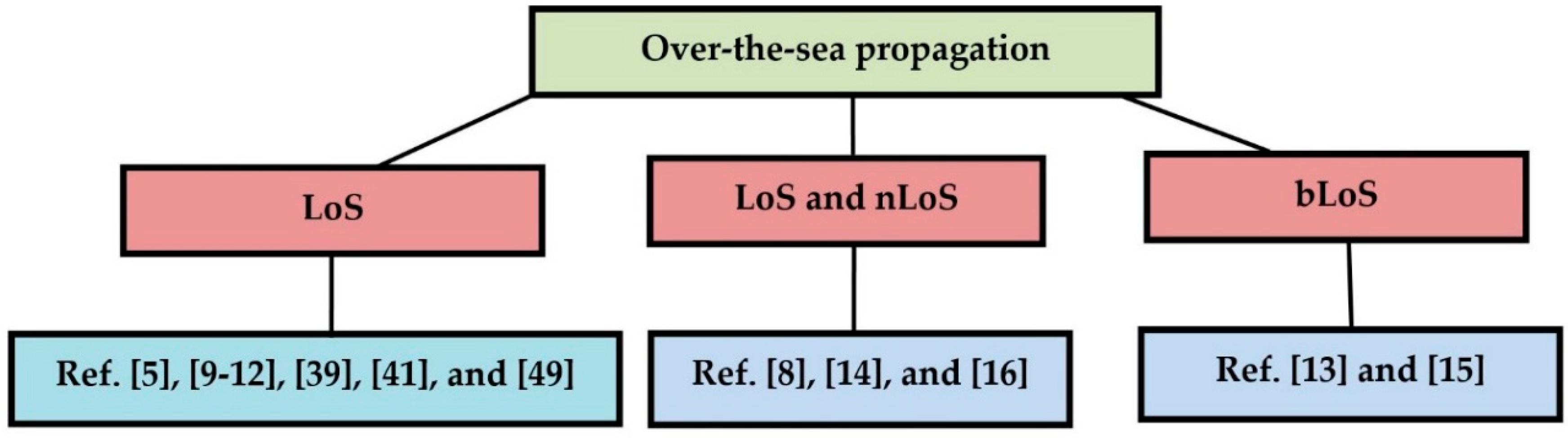
3. Classification of Over-the-Sea Channel Models
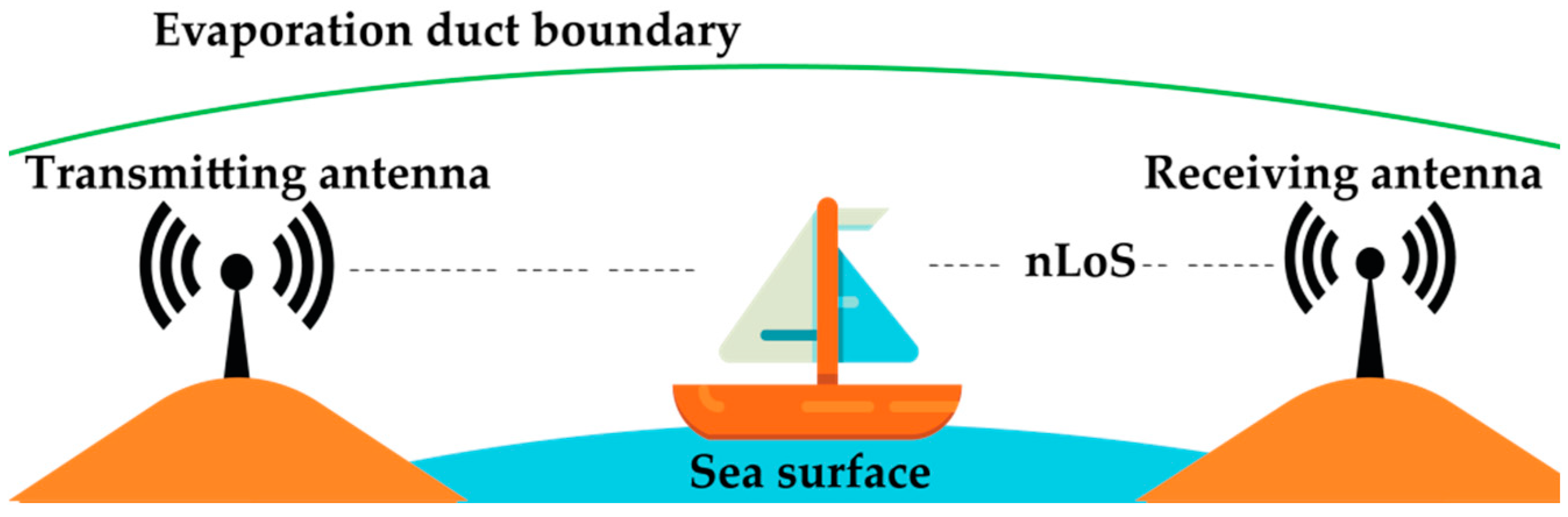
4. Channel Models for Over-the-Sea Wave Propagation
4.1. Wideband Channel Modeling for Over-the-Sea Wave Propagation
4.2. Marine Communication Channel Modeling Based on the Finite Difference Time Domain (FDTD)
4.3. Experimental Multipath Delay Profile of Mobile Radio Channels Over the Sea at 2 GHz
4.4. Modeling of Near-the-Sea Mobile Radio Wave Propagation at 5 GHz
4.5. Novel Maritime Channel Model Using Millimeter Radio Waves
4.6. Multipath Channel Model for Propagation of Radio Waves Over the Sea Surface
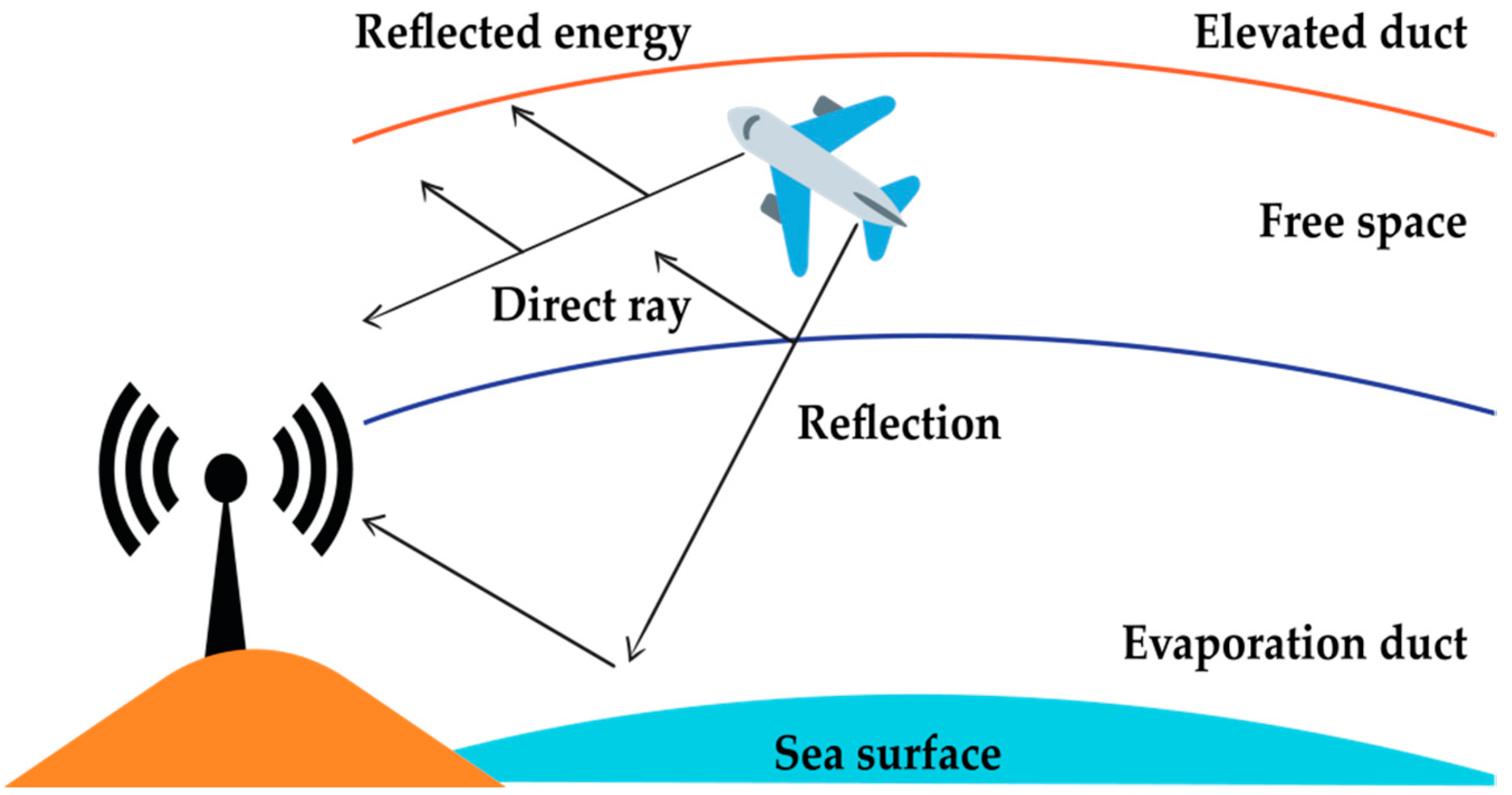
4.7. Channel Model for Surface Ducts
4.8. Channel Fading Margin for a Wireless Link
4.9. Over-the Sea Radio Links of Malaysian Shore Using the Evaporation Duct
4.10. High-Capacity and Long Range Microwave Over-the-Sea Link Propagation Using the Evaporation Duct
- Path loss = 141 dB
- Transmitted power = 27 dBm
- Antenna gain of the transmitter and receiver = 40 dB
- Bandwidth = 14 MHz
4.11. Multipath Delay Profile and Doppler Spread of Millimeter Radio Waves Over the Sea Surface
4.12. Ray-Tracing-Based Wireless Channel Modeling for Over-the-Sea Communication Near Diaoyu Islands
4.13. Measurement and Analysis on a Land-to-Ship Offshore Wireless Channel in 2.4 GHz
4.14. Research on Sea-Surface Ka-Band Stochastic Multipath Modeling
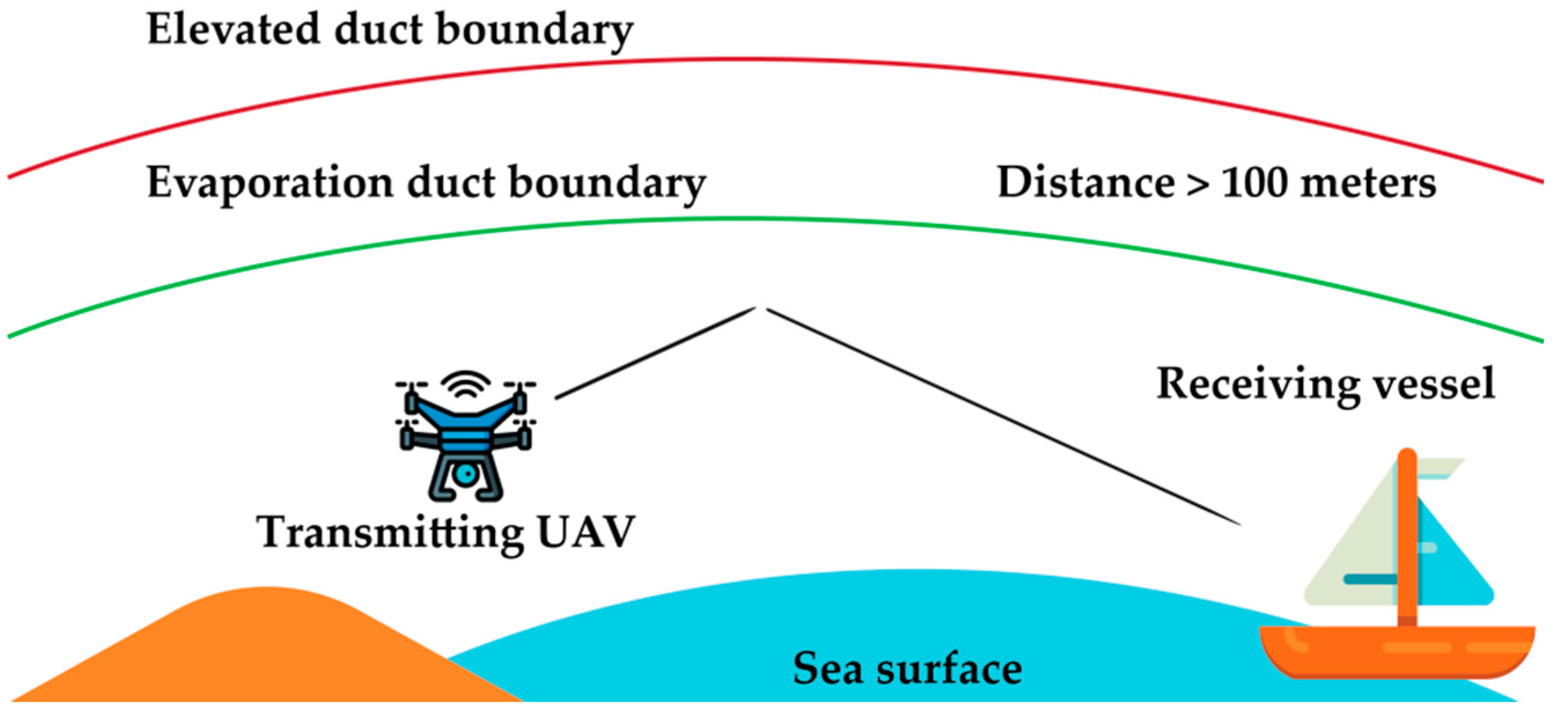
4.15. Modeling of a Channel Using the FDTD Method between UAVs and Sea Surface Vehicles
5. Comparison of the Channel Models
6. Possible Applications and Improvements
6.1. Applications of the Channel Models
6.2. Possibility of Unique and Novel Applications of the Channel Models
6.3. Possible Future Improvements of the Channel Models
6.4. Insights on Modeling the Over-the-Sea Wireless Channels
7. Challenging Issues and Research Directions
7.1. Challenging Issues
7.2. Furture Research Directions
8. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Balkees, P.A.S.; Sasidhar, K.; Rao, S. A survey based analysis of propagation models over the sea. In Proceedings of the 2015 International Conference on Advances in Computing, Communications and Informatics (ICACCI), Kochi, India, 10–13 August 2015. [Google Scholar]
- Habib, A.; Moh, S. A Survey on Channel Models for Radio Propagation over the Sea Surface. In Proceedings of the 10th International Conference on Internet (ICONI 2018), Phnom Penh, Cambodia, 1–3 December 2018. [Google Scholar]
- Lee, Y.; Meng, Y. Key Considerations in the Modeling of Tropical Maritime Microwave Attenuations. Int. J. Antennas Propag. 2015, 2015, 1–7. [Google Scholar] [CrossRef]
- Definition: Elevated Duct. Available online: https://www.its.bldrdoc.gov/fs-1037/dir-014/_1970.htm (accessed on 21 November 2018).
- Huang, F.; Liao, X.; Bai, Y. Multipath Channel Model for Radio Propagation over Sea Surface. Wirel. Pers. Commun. 2016, 90, 245–257. [Google Scholar] [CrossRef]
- Parsons, J. The Mobile Radio Propagation Channel; John Wiley: Chichester, UK, 2001. [Google Scholar]
- Lee, W. Mobile Communications Engineering, 1st ed.; McGraw-Hill: New York, NY, USA, 2012. [Google Scholar]
- Konstantinos, N.M.; Loulis, P.; Chronopoulos, M. Measurements and Wideband Channel Characterization for Over-the-sea Propagation. In Proceedings of the 2006 IEEE International Conference on Wireless and Mobile Computing, Networking and Communications, Montreal, QC, Canada, 19–21 June 2006. [Google Scholar]
- Timmins, I.; O’Young, S. Marine Communications Channel Modeling Using the Finite-Difference Time Domain Method. IEEE Trans. Veh. Technol. 2009, 58, 2626–2637. [Google Scholar] [CrossRef]
- Yang, K.; Roste, T.; Ekman, T. Experimental multipath delay profile of mobile radio channels over sea at 2 GHz. In Proceedings of the 2012 Loughborough Antennas and Propagations Conference, Loughborough, UK, 12–13 November 2012. [Google Scholar]
- Lee, Y.H.; Dong, F.; Meng, Y.S. Near Sea-Surface Mobile Radiowave Propagation at 5 GHz: Measurements and Modeling. Radioengineering 2014, 24, 824–830. [Google Scholar]
- Mehrnia, N.; Ozdemir, M.K. Novel maritime channel models for millimeter radiowaves. In Proceedings of the 24th International Conference on Software, Telecommunications and Computer Networks (SoftCOM), Split, Croatia, 21–23 September 2016. [Google Scholar]
- Dinc, E.; Akan, O. Channel Model for the Surface Ducts: Large-Scale Path-Loss, Delay Spread, and AOA. IEEE Trans. Antennas Propag. 2015, 63, 2728–2738. [Google Scholar] [CrossRef]
- Zaidi, K.; Jeoti, V.; Drieberg, M.; Awang, A.; Iqbal, A. Fading Characteristics in Evaporation Duct: Fade Margin for a Wireless Link in the South China Sea. IEEE Access 2018, 6, 11038–11045. [Google Scholar] [CrossRef]
- Iqbal, A.; Jeoti, V. Feasibility study of radio links using evaporation duct over sea off Malaysian shores. In Proceedings of the 2010 International Conference on Intelligent and Advanced Systems, Manilla, Philippines, 15–17 June 2010. [Google Scholar]
- Woods, G.; Ruxton, A.; Huddlestone-Holmes, C.; Gigan, G. High-Capacity, Long-Range, Over Ocean Microwave Link Using the Evaporation Duct. IEEE J. Ocean. Eng. 2009, 34, 323–330. [Google Scholar] [CrossRef]
- Mehrina, K.; Ozdemir, K.M. Multipath Delay Profile and Doppler Spread of Millimeter Radiowaves over the Sea Channel. In Proceedings of the 2017 IEEE International Black Sea Conference on Communications and Networking, Istanbul, Turkey, 5–8 June 2017. [Google Scholar]
- Wu, Y.; Gao, Z.; Canbin, C.; Huang, L.; Chiang, H.; Huang, Y.; Sun, H. Ray Tracing Based Wireless Channel Modeling over the Sea Surface Near Diaoyu Islands. In Proceedings of the 2015 First International Conference on Computational Intelligence Theory, Systems and Applications, Yilan, Taiwan, 10–12 December 2015. [Google Scholar]
- Lee, J.; Choi, J.; Lee, W.; Choi, J.; Kim, S. Measurement and Analysis on Land-to-Ship Offshore Wireless Channel in 2.4 GHz. IEEE Wirel. Commun. Lett. 2017, 6, 222–225. [Google Scholar] [CrossRef]
- Cao, X.; Jiang, T. Research on Sea Surface Ka-band Stochastic Multipath Channel Modeling. In Proceedings of the 2014 3rd Asia-Pacific Conference on Antennas and Propagation, Harbin, China, 26–29 July 2014. [Google Scholar]
- Shi, Z.; Xia, P.; Gao, Z.; Huang, L.; Chen, C. Modeling of Wireless Channel between UAV and Vessel using the FDTD method. In Proceedings of the 10th International Conference on Wireless Communications, Networking and Mobile Computing (WiCOM 2014), Beijing, China, 19–20 June 2015. [Google Scholar]
- Coker, A.; Straatemeier, L.; Rogers, T.; Valdez, P.; Cooksey, D.; Griendling, K. Maritime channel modeling and simulation for efficient wideband communications between autonomous Unmanned Surface Vehicles. In Proceedings of the 2013 OCEANS, San Diego, CA, USA, 23–27 September 2013. [Google Scholar]
- Zhao, X.; Huang, S.; Fan, H. Influence of sea surface roughness on the electromagnetic wave propagation in the duct environment. In Proceedings of the 2010 Second IITA International Conference on Geoscience and Remote Sensing, Qingdao, China, 28–31 August 2010. [Google Scholar]
- Choi, M.; Kim, B.; Kim, K.; Jeong, M.; Lee, S. Ship to Ship Maritime Communication for e-Navigation Using IEEE 802.16j. Adv. Sci. Technol. Lett. 2013, 28, 110–115. [Google Scholar] [CrossRef]
- Meng, Y.; Lee, Y. Measurements and Characterizations of Air-to-Ground Channel Over Sea Surface at C-Band with Low Airborne Altitudes. IEEE Trans. Veh. Technol. 2011, 60, 1943–1948. [Google Scholar] [CrossRef]
- Lee, Y.H.; Dong, F.; Meng, Y.S. Stand-Off Distances for Non-Line-Of-Sight Maritime Mobile Applications in 5 GHz Band. Prog. Electromagn. Res. B 2013, 54, 321–336. [Google Scholar] [CrossRef]
- Dong, F.; Lee, Y.H. Non-line-of-sight communication links over sea surface at 5.5 GHz. In Proceedings of the 2010 Microwave Conference Proceedings (APMC), Melbourne, Australia, 5–8 December 2011. [Google Scholar]
- Kane, Y. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar] [CrossRef]
- Alves, J.; Banner, M.; Young, I. Revisiting the Pierson–Moskowitz Asymptotic Limits for Fully Developed Wind Waves. J. Phys. Oceanogr. 2003, 33, 1301–1323. [Google Scholar] [CrossRef]
- Ohmori, S.; Miura, S. A fading reduction method for maritime satellite communications. IEEE Trans. Antennas Propag. 1983, 31, 184–187. [Google Scholar] [CrossRef]
- Sklar, B. Rayleigh fading channels in mobile digital communication systems. II. Mitigation. IEEE Commun. Mag. 1997, 35, 102–109. [Google Scholar] [CrossRef]
- Bennett, R.R. Satellite relay for unmanned air vehicle data. In Proceedings of the MILCOM 92 Conference Record, San Diego, CA, USA, 11–14 October 1992. [Google Scholar]
- Taflove, A.; Hagness, S. Computational Electrodynamics; Artech House: Boston, MA, USA, 2010. [Google Scholar]
- Yacoub, M. Foundations of Mobile Radio Engineering; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Elliott, W. Results of a VHF propagation study. IEEE Trans. Antennas Propag. 1981, 29, 808–811. [Google Scholar] [CrossRef]
- Budny, J. Strive to Thrive. Five-Volume Series. Series edited by Mickey Sarquis Publisher: Terrific Science Press. 2007. ISBN: 978-1-883822-42-2. Int. J. Toxicol. 2008, 27, 349–350. [Google Scholar] [CrossRef]
- Kinsman, B. Wind Waves; Dover: New York, NY, USA, 1984. [Google Scholar]
- Wireless EM Propagation Software—Wireless InSite—Remcom. Available online: https://www.remcom.com/wireless-insite-em-propagation-software/ (accessed on 21 November 2018).
- Haspert, K.; Tuley, M. Comparison of Predicted and Measured Multipath Impulse Responses. IEEE Trans. Aerosp. Electron. Syst. 2011, 47, 1696–1709. [Google Scholar] [CrossRef]
- Saleh, B.; Teich, M. Fundamentals of Photonics; Wiley: Chichester, UK, 2013. [Google Scholar]
- Patterson, W.L. Advanced refractive effects prediction system (AREPS). In Proceedings of the 2007 IEEE Radar Conference, Boston, MA, USA, 17–20 April 2007. [Google Scholar]
- Patterson, W.L. User’s Manual for Advanced Refractive Effects Prediction System (AREPS); Space and Naval Warfare Systems Center: San Diego, CA, USA, 2004; pp. 1–7. [Google Scholar]
- Method for Point-To-Area Predictions for Terrestrial Services in the Frequency Range 30 MHz to 3000 MHz. Available online: https://www.itu.int/rec/R-REC-P.1546/en (accessed on 12 January 2019).
- Kolmogorov-Smirnov test. Available online: https://ocw.mit.edu/courses/mathematics/18-443-statistics-for-applications-fall-2006/lecture-notes/lecture14.pdf (accessed on 12 January 2019).
- Chen, Y.; Georgiou, T. Stochastic Bridges of Linear Systems. Available online: https://arxiv.org/pdf/1407.3421.pdf (accessed on 12 January 2019).
- Rogers, L.; Williams, D. Diffusions, Markov Processes, and Martingales, 2nd ed.; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Chen, Z.; Wu, X.; Li, H.; Yang, N.; Chia, M. Considerations for Source Pulses and Antennas in UWB Radio Systems. IEEE Trans. Antennas Propag. 2004, 52, 1739–1748. [Google Scholar] [CrossRef]
- Cheng, D. Field and Wave Electromagnetics; Addison-Wesley: Reading, MA, USA, 1992. [Google Scholar]
- Measurements of Wind-Wave Growth and Swell Decay During the Joint North Sea Wave Project (JONSWAP). Available online: https://books.google.co.kr/books/about/Measurements_of_Wind_wave_Growth_and_Swe.html?id=UqSOPAAACAAJ&redir_esc=y (accessed on 12 January 2019).
- Bajwa, W.; Sayeed, A.; Nowak, R. Sparse Multipath Channels: Modeling and Estimation. In Proceedings of the 2009 IEEE 13th Digital Signal Processing Workshop and 5th IEEE Signal Processing Education Workshop, Marco Island, FL, USA, 4–7 January 2009. [Google Scholar]
- Saleh, A.; Valenzuela, R. A Statistical Model for Indoor Multipath Propagation. IEEE J. Sel. Areas Commun. 1987, 5, 128–137. [Google Scholar] [CrossRef] [Green Version]
- Karasawa, Y.; Shiokawa, T. Characteristics of L-band multipath fading due to sea surface reflection. IEEE Trans. Antennas Propag. 1984, 32, 618–623. [Google Scholar] [CrossRef]








| Channel Model | Used Frequency | Outstanding Features | Advantages | Limitations |
|---|---|---|---|---|
| Measurements and wideband channel characterization for over-the-sea propagation [8] | 1.9 GHz | • Supports mobile wireless vessel-to-vessel communication. • Implements a wideband radio channel. | • Measured data are authentic real-time data based on practical implementation. • Propagation environment includes both the sea and the land. | • Highly dependent on global positioning system (GPS). • Increased delay under non-line-of-sight (nLoS) propagation. • Multipath effect caused by fluctuating sea waves is ignored. |
| Marine communication channel modeling using the finite-difference time domain method [9] | Very high frequency (VHF) to 3 GHz | • Specially designed channel model for unmanned aerial vehicles (UAVs) to be deployed in an oceanic environment. • Applicable for very high frequencies up to 3 GHz. | • High channel accuracy due to finite difference time domain (FDTD) method. • Applicable to any sea-surface vehicle or sensor. • Prevents communication loss and reduces the probability of a collision. | • No particular deployment scenario has been elaborated. • Modeling process is performed through two-dimensional (2-D) approximation, which could have been better if it was performed using three-dimensional (3-D) approximation. |
| Experimental multipath delay profile of mobile radio channels over the sea [10] | 2 GHz | • Channel model is applicable for both short-range and long-range communication. | • Channel model is applicable for both mobile and fixed antennas. | • No measurements were undertaken for specular and diffuse multipath propagation. |
| Near-sea-surface mobile radio wave propagation at 5 GHz: measurements and modeling [11] | 5 GHz | • The only channel model in the literature so far to implement and compare three path loss models (two-ray, three-ray, and free space loss model (FSL)). • Both reflection and refraction are taken into account when modeling the channel. | • Works at very long distances, greater than 3000 m. • Measurement data are based on real-time application. | • The only channel parameter considered is the signal strength. • No consideration of the error rate. |
| Novel maritime channel models for millimeter radio waves [12] | 34 and 95 GHz | • Uses 5G candidate frequencies (35 and 94 GHz). • Modifies the two-ray model for the better prediction of path loss. | • Comparison results are shown for two different frequency bands. • Can predict the last peak of the path loss without showing any extra peak. | • Considers the sea surface as flat, without roughness. • The incident angles of the rays are not at all considered to be small. |
| Multipath channel model for radio wave propagation over the sea surface [5] | Applicable for multiple frequencies | • Applicable for ship-to-ship and ship-to-shore wireless communication over the sea. • Applied channel model under different carrier frequencies, transmission distances, and sea states. | • A global channel model applicable to all frequencies and geographic situations. • Considers both the reflection and refraction of rays. | • Coastline hills and the surrounding ships are not involved in channel modeling, thus making the channel model applicable only to the open sea. • Measured data are not based on real-time implementation. |
| Channel model for surface ducts [13] | 5–15 GHz | • Independent of polarization. • Channel model is symmetric. | • Reliable use of the RO method because the frequency used is more than 3 GHz. • Considers beyond non-line-of-sight (bLoS) links. | • No calculation presented of the complete fading margin. • Mobility of the transmitter and receiver is not mentioned. |
| Channel fading margin for a wireless link in the South China Sea [14] | 10.5 GHz | • Detailed analysis of the effects of both slow fading and fast fading. • Introducing the concept of complete fading margin, where both types of fading were incorporated. | • Consideration of both line-of-sight (LoS) and nLoS. • Fading margin is calculated both for short (50 km) and long distances (100 km). | • Fading margin is calculated only on the basis of the evaporation duct. Other types of atmospheric ducts are not considered. • No specification of the transmitter and receiver mobility. |
| Over-the-sea radio links of Malaysian shore using evaporation duct [15] | 1.7–25 GHz | • Supports over-the- sea bLoS communication links. • Supports mobility of the transceiver platforms. | • Experimental results were obtained for a wide range of frequencies (2.4, 5.8, 10.5, and 24.125). | • No elaborate discussion on path loss model. • Important parameters like mean excess delay, RMS delay spread, and channel fade margin are ignored. |
| High-capacity and long-range microwave over-the-sea link propagation using evaporation duct [16] | 10.6 GHz | • Supports ad hoc sensor network applications. • Antennas operate at optimal height for evaporation duct. | • Real life implementation of wave propagation mechanism using evaporation duct from sea to mainland. • Transmission of heavy multimedia (image and video) data is possible. | • Good amount of signal attenuation at the receiver end. • No consideration for channel fading. • Delay spread is not considered as a channel evaluation parameter. |
| Multipath delay profile and Doppler spread of millimeter radio waves [17] | 35 and 94 GHz | • Supports the data rate requirements for 5G. • Uses millimeter wave. • Implementable with multi-input multi-output (MIMO) systems. | •High rate for data transmission. • Tolerant to the effects of Doppler frequency. • Handled signal distortion caused by the mobility of the transceiver platform. | • Propagation loss equation was not assumed or formulated. • Considered LoS propagation only. |
| Ray-tracing-based wireless channel modeling for over-the-sea communication near Diaoyu islands [18] | 2.5 GHz | • Applies the ray tracing method. • The transceiver platforms can be set into hot air balloons. | • 3-D simulation of the sea environment. • Computation of the electric field intensity for both receiving and transmitting rays. | • Islands are huge barriers of wave propagation. No consideration of bLoS links to overcome those barriers. • Atmospheric duct height effects were ignored. • Fading characteristics of the channel were not explored. |
| Measurement and analysis of the land-to-ship offshore wireless channel in 2.4 GHz [19] | 2.4 GHz | • Comparison among the path loss models. • Use of empirical data to test the path loss models. | • Real-time implementation with the authentic author-collected data. • Considered both small- and large-scale fading. | • No experiments and calculations were conducted for fade margin. |
| Research on sea-surface Ka-band stochastic multipath modeling [20] | 26.5–40 GHz | • Used Ka-band for wave propagation. | •High data rate. • Small size terminal antenna. • Narrow beam. | • No specified path loss model. • Considered vertical polarization only for the incident rays. |
| Modeling of a channel using the FDTD method between UAV to sea surface vehicle [21] | Not specified | • Supports UAV to surface vessel communication links. | • Use of the FDTD method. | • Sea surface was assumed to be a perfectly conducting boundary, which is not realistic. • No specific carrier frequency was used. |
| Channel Model | Type of Propagation | Mobility of Transmitter | Mobility of Receiver | Considered Atmospheric Duct | Channel Parameters | Propagation Loss Model |
|---|---|---|---|---|---|---|
| Ref. [8] | Line-of-sight (LoS) and non-line-of-sight (nLoS) | Yes | No | Not considered | Propagation loss, RMS delay spread, and mean excess delay | Long-distance path loss model (eqn. (1)) |
| Ref. [9] | LoS | Yes | No | Not considered | Propagation loss, mean excess delay, and RMS delay spread | Basic path loss model (eqn. (8)) |
| Ref. [10] | LoS | Yes | Yes | Not considered | Mean excess delay, and RMS delay spread | Not mentioned |
| Ref. [11] | LoS | Yes | Yes | Evaporation duct | Propagation loss, transmitted power, transmitter height, and receiver height | three-ray path loss model (eqn. (4)) |
| Ref. [12] | LoS | Yes | No | Evaporation duct | Propagation loss, RMS delay spread, path loss, and received power | Modified two-ray path loss model (eqn. (19)) |
| Ref. [5] | LoS | Yes | No | Not considered | Propagation loss, power delay profile, and inter-symbol interference | Not mentioned |
| Ref. [13] | Beyond non-line-of-sight (bLoS) | Not mentioned | Not mentioned | Surface duct | Propagation loss, delay spread, and angle of arrival | Custom path loss model using (eqn. (22)) |
| Ref. [14] | LoS and nLoS | Not mentioned | Not mentioned | Evaporation duct | Propagation loss, channel fade margin, slow fading, and fast fading | Log normal path loss distribution |
| Ref. [15] | bLoS | Yes | Yes | Evaporation duct | Propagation loss | Not mentioned |
| Ref. [16] | nLoS and LoS | No | No | Evaporation duct | Propagation loss | Parabolic equation method |
| Ref. [17] | LoS | Yes | Yes | Not considered | Power delay profile, coherence time, bandwidth, and distance | Not mentioned |
| Ref. [18] | Not mentioned | Yes | Yes | Not considered | Propagation loss, time delay, and angle of arrival | Custom path loss model using (eqn. (35)) |
| Ref. [19] | LoS | Yes | No | Not considered | Propagation loss, and large- and small-scale fading | two-ray path loss model (eqn. (5)) |
| Ref. [20] | Not mentioned | Not mentioned | Not mentioned | Not considered | Time delay, phase delay, and amplitude gain | Not mentioned |
| Ref. [21] | LoS | Yes | Yes | Not considered | Propagation loss | Not mentioned |
| Channel Model | Antenna Type | Range | Bandwidth | Antenna Height | Transmitting Power |
|---|---|---|---|---|---|
| Ref. [8] | Omni-directional | 30 km | Not specified | 21.5 m | 30 dBm |
| Ref. [9] | Not specified | 100 m | Not specified | Not specified | Not specified |
| Ref. [10] | Omni-directional | Short range: 15 km Long range: 45 km | Not specified | For short range Transmitter: 6.4 m Receiver: 21 m For long range Transmitter: 9.5 m Receiver: 11.2 m | 27.2 dBm |
| Ref. [11] | Omni-directional | 18.52 km | Not specified | Transmitter: 3.5 m Receiver: 20 m | 23 dBm |
| Ref. [12] | Omni-directional | 2 km | 200 MHz | Transmitter: 5 m Receiver: 9.7m | 23 dBm |
| Ref. [5] | Dipole antenna | Not specified | Not specified | Not specified | Not specified |
| Ref. [13] | Not specified | 500 km | 20 MHz | Transmitter: 27 m Receiver: 27 m | 30 dBm |
| Ref. [14] | Gaussian | 100 km | Not specified | Transmitter: 4 m Receiver: 4 m | Not specified |
| Ref. [15] | Sin(x)/x | 100 km | Not specified | 5 m | 30 dBm |
| Ref. [16] | Parabolic dish antenna | 78 km | 14 MHz | Transmitter: 7 m Receiver: 7 m | 27 dBm |
| Ref. [17] | Omni-directional | 10 km | 200 MHz | Transmitter: 5.63 m Receiver: 20 m | 23 dBm |
| Ref. [18] | Omni-directional | Not specified | Not specified | Transmitter: 5 m Receiver: 100 m | Not specified |
| Ref. [19] | Omni-directional | 2 km | Not specified | Transmitter: 3 m Receiver: 4.5 m | 25 dBm |
| Ref. [20] | Not specified | Not specified | 0.95 MHz | Not specified | Not specified |
| Ref. [21] | Not specified | 10 m | Not specified | Transmitter: 40 m | Not specified |
| Channel Model | Application |
|---|---|
| Ref. [8] | Applicable to vessel-to-vessel communication system where both the transmitter and receiver are mobile. |
| Ref. [9] | UAV to surface vessel based communication. |
| Ref. [10] | Applicable to high-speed watercraft transmitter or receiver. |
| Ref. [11] | Channel model can be applied to a mobile vessel to sea shore based communication. |
| Ref. [12] | Applicable to the 5G network architecture. |
| Ref. [5] | Applicable for vessels in both smooth and rough sea surfaces. |
| Ref. [13] | Applicable to a scenario in which the transmitter and receiver have bLoS propagation. |
| Ref. [14] | A mathematical model for calculating the fading margin is applicable only for the South China Sea. |
| Ref. [15] | Applicable for over-the-sea communication in the deep sea. |
| Ref. [16] | Especially applicable for transmitting the data gathered from the ad hoc sensor nodes for environmental monitoring purposes. |
| Ref. [17] | Applicable for highly mobile water vehicles with high speed. |
| Ref. [18] | Applicable for airborne devices to sea vehicles. |
| Ref. [19] | Applicable for ship-to-shore communication. |
| Ref. [20] | Designed for applications requiring high data rate. |
| Ref. [21] | UAV to surface vessel based ocean surveillance. |
| Channel Model | Possible Future Improvements |
|---|---|
| Ref. [8] | Multipath effects of the sea surface may be incorporated into the channel model in the future to make it more reliable. |
| Ref. [9] | Elevated duct-based channel measurements may be included. |
| Ref. [10] | Diffuse and specular multipath propagations may be introduced in this channel model in the future. |
| Ref. [11] | The channel model can be improved by taking into account the error rate along with the signal strength as a parameter. |
| Ref. [12] | Sea roughness can be included as a channel modeling parameter. |
| Ref. [5] | A propagation loss model can be introduced. |
| Ref. [13] | A complete fading margin calculation may be introduced, consisting of slow and fast fading. |
| Ref. [14] | Technical validation of the mathematical idea should be introduced in the future. |
| Ref. [15] | Channel evaluation parameters, such as mean excess delay, fade margin, etc., can be introduced for a reliable design. |
| Ref. [16] | Channel evaluation parameters, such as mean excess delay, fade margin, etc., can be introduced for a reliable design. |
| Ref. [17] | Channel model can be integrated to support nLoS or bLoS propagations. |
| Ref. [18] | Channel model should be integrated with complex real time path loss model and duct effects. |
| Ref. [19] | Fade margin determination can be introduced in the model. |
| Ref. [20] | Fade margin determination can be introduced in the model. |
| Ref. [21] | Sea surface can be designed in a 3-D cross section. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Habib, A.; Moh, S. Wireless Channel Models for Over-the-Sea Communication: A Comparative Study. Appl. Sci. 2019, 9, 443. https://doi.org/10.3390/app9030443
Habib A, Moh S. Wireless Channel Models for Over-the-Sea Communication: A Comparative Study. Applied Sciences. 2019; 9(3):443. https://doi.org/10.3390/app9030443
Chicago/Turabian StyleHabib, Arafat, and Sangman Moh. 2019. "Wireless Channel Models for Over-the-Sea Communication: A Comparative Study" Applied Sciences 9, no. 3: 443. https://doi.org/10.3390/app9030443
APA StyleHabib, A., & Moh, S. (2019). Wireless Channel Models for Over-the-Sea Communication: A Comparative Study. Applied Sciences, 9(3), 443. https://doi.org/10.3390/app9030443






