PV Tracking Design Methodology Based on an Orientation Efficiency Chart
Abstract
1. Introduction
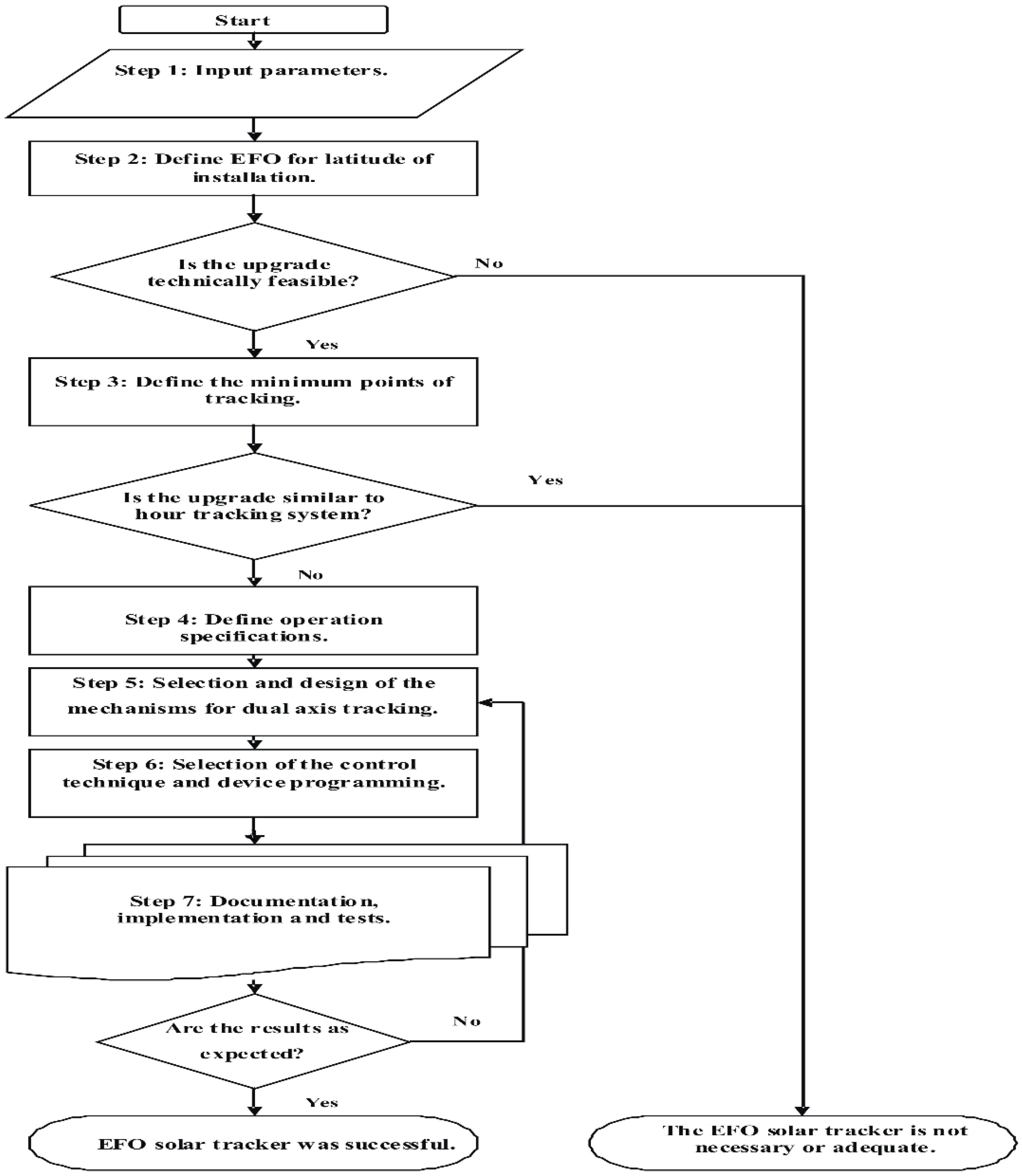
2. Materials and Methods
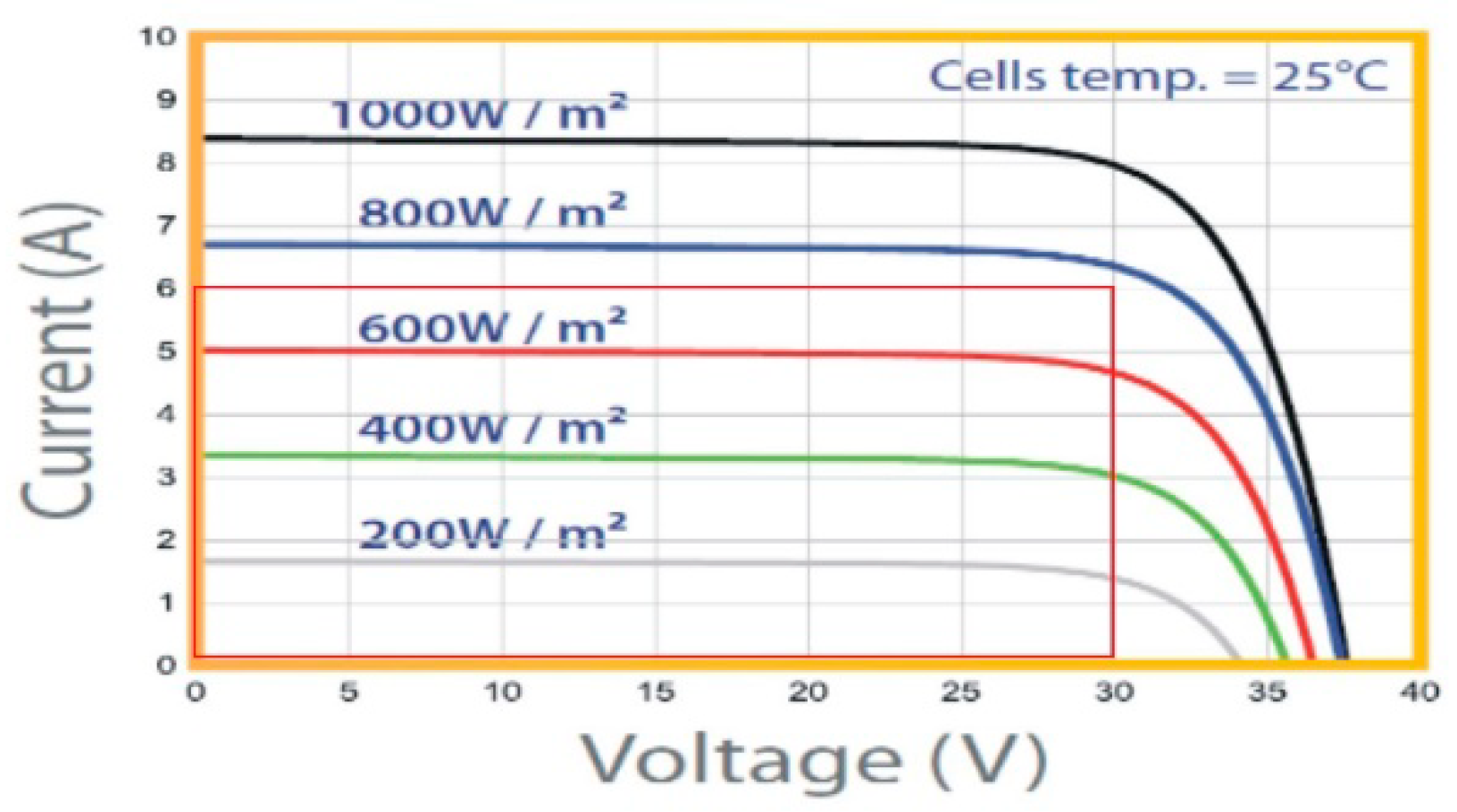
2.1. Input Parameters
2.2. EFO Chart of Installation Latitude
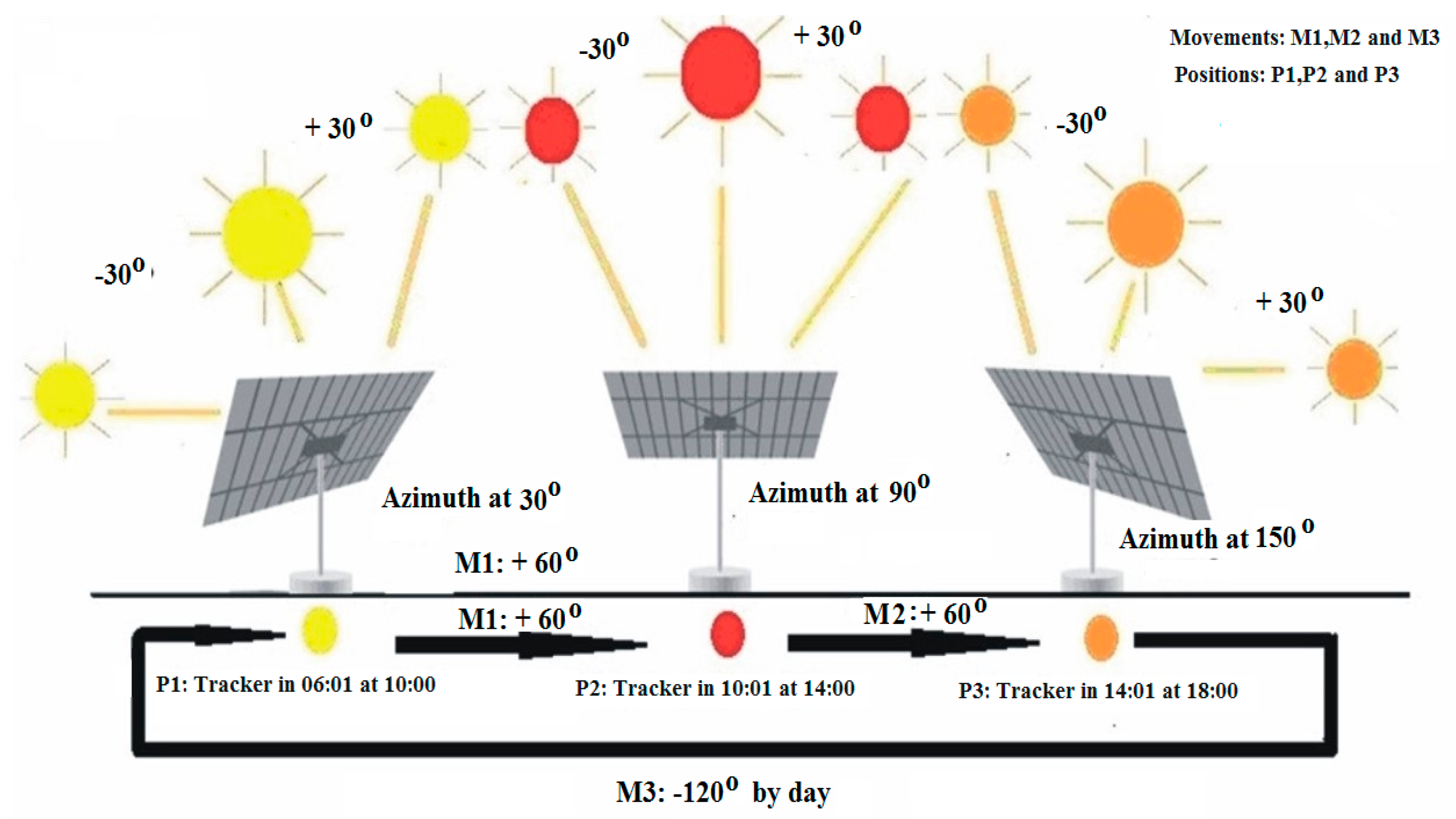
2.3. Points and Trajectory for Solar Tracking
2.4. Operation Specifications
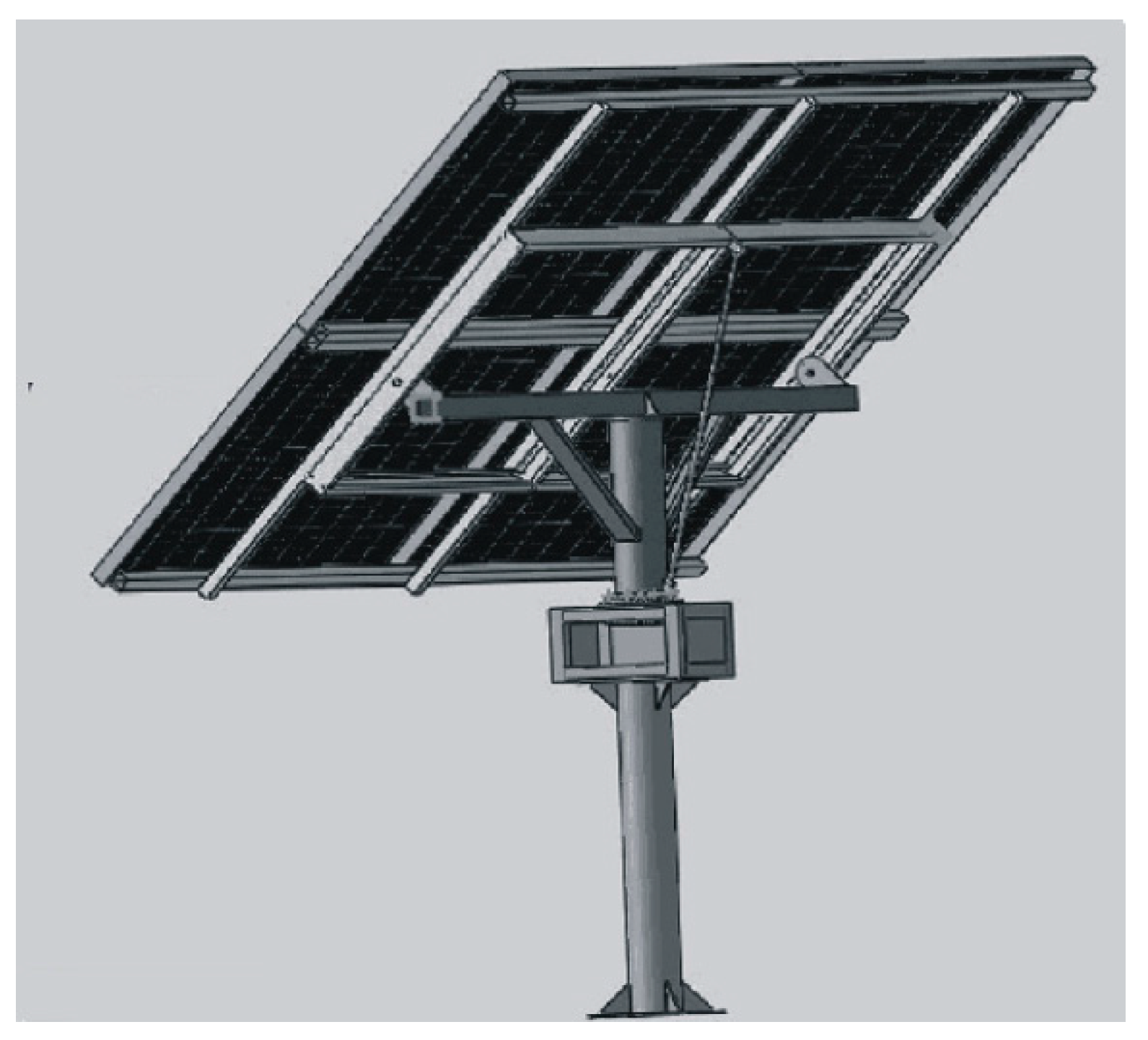
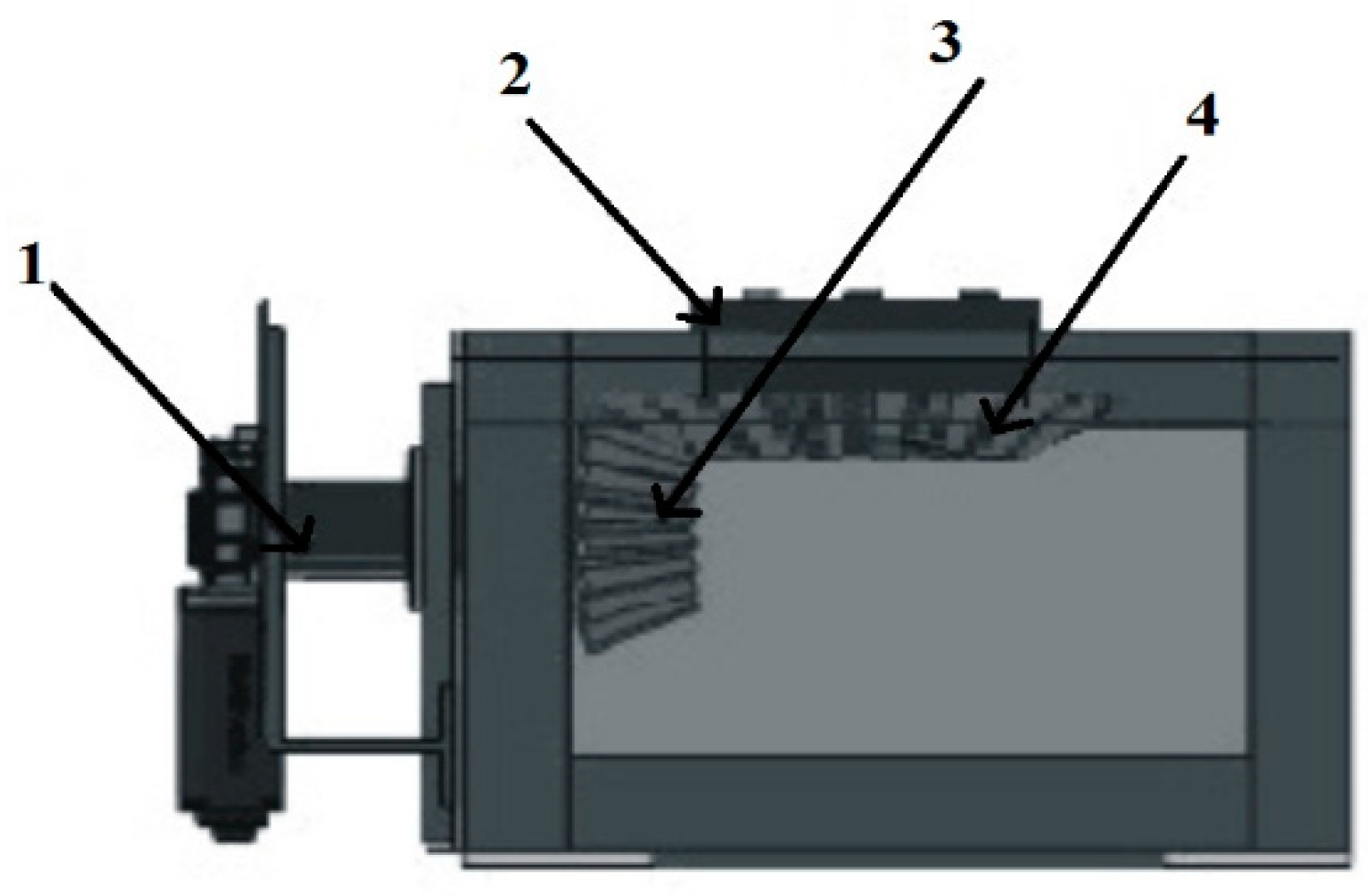
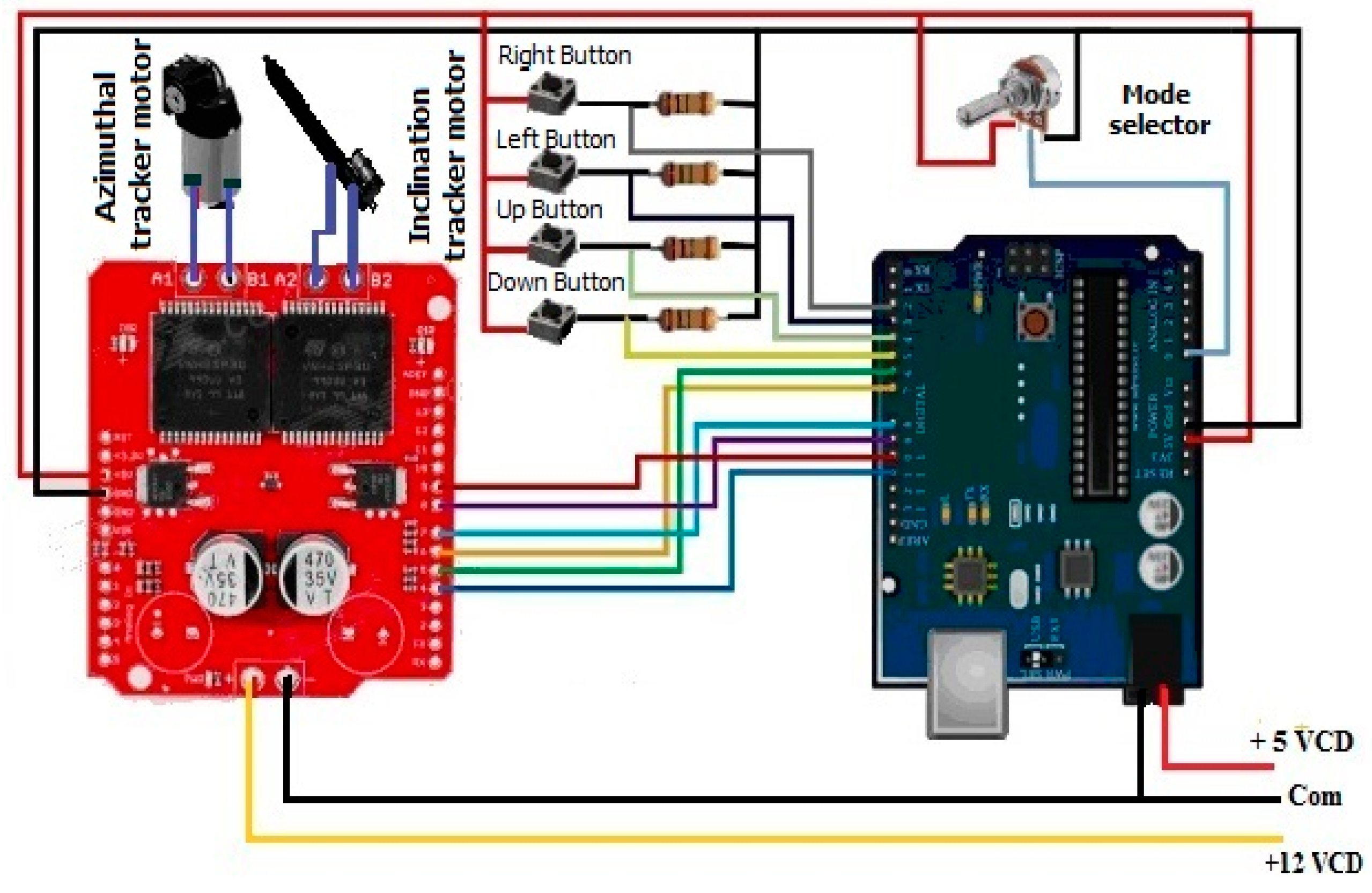
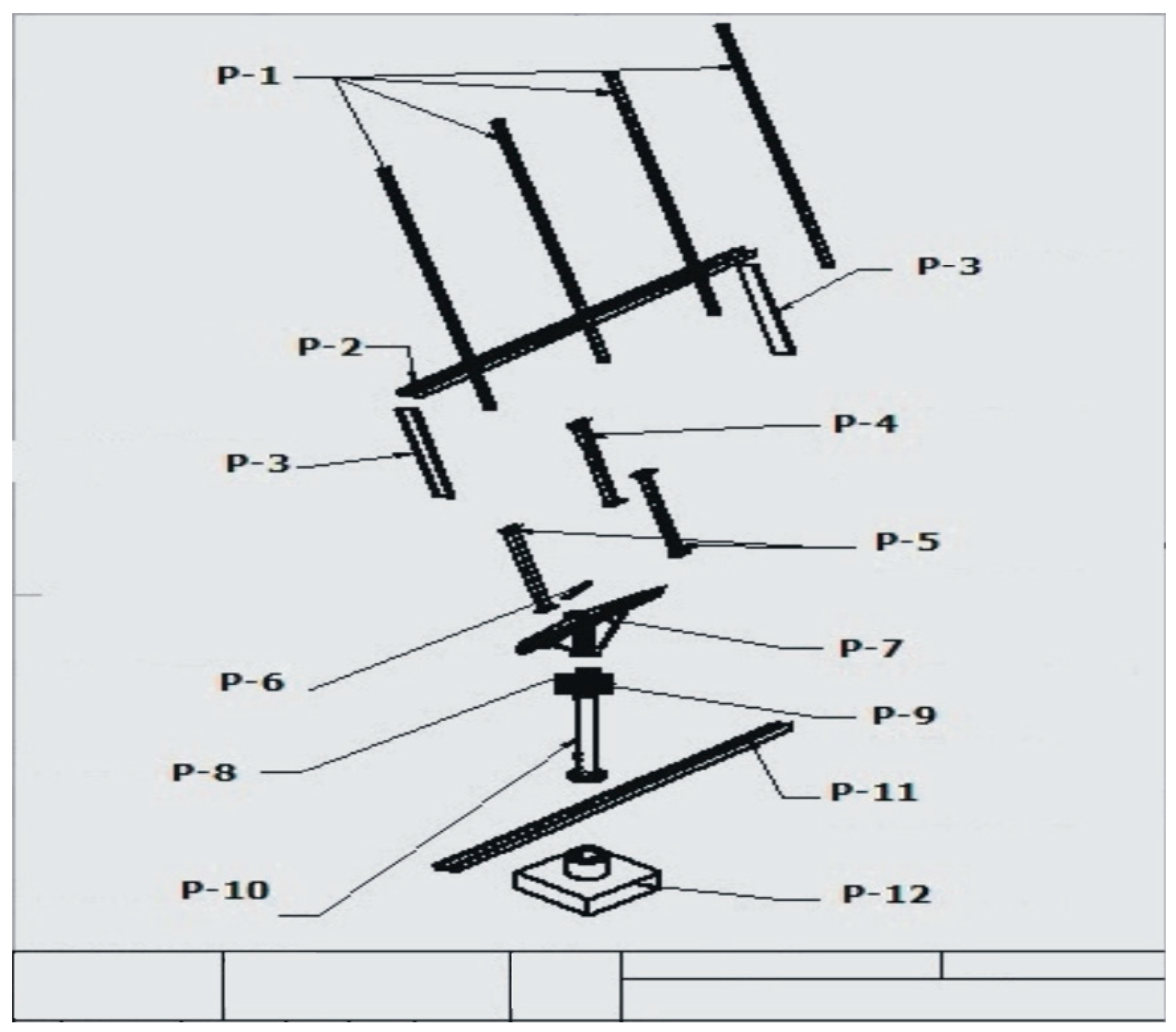
2.5. Selection and Design of the Mechanisms for Dual-Axis Tracking
2.6. Selection of the Control Technique and Device Programming
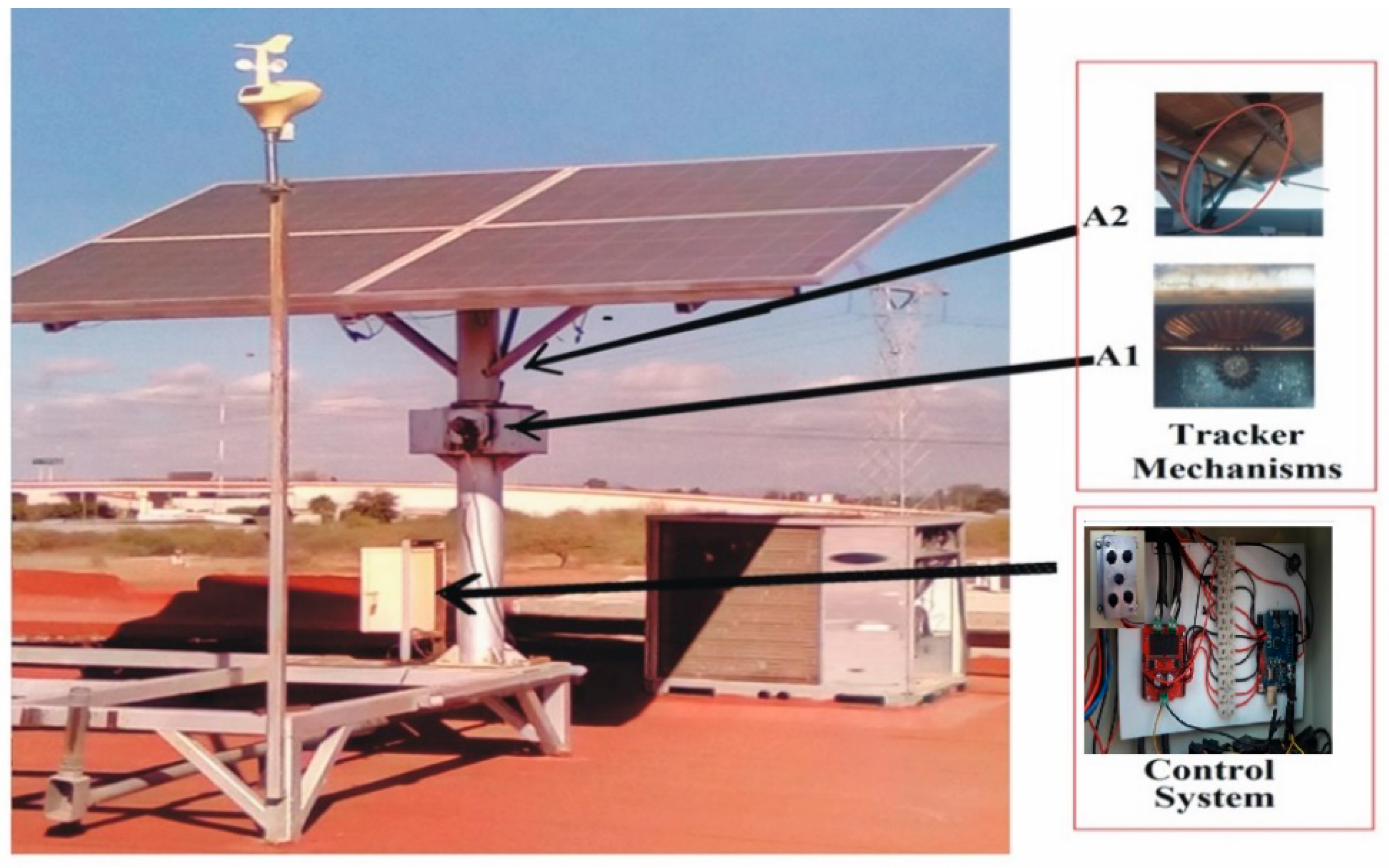
2.7. Documentation, Implementation, and Testing
2.7.1. Documentation
2.7.2. Implementation and Cost Reduction
2.7.3. Testing
3. Results
4. Conclusions
Supplementary Files
Supplementary File 1Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jaaz, A.H.; Sopian, K.; Gaaz, T.S. Study of the electrical and thermal performances of photovoltaic thermal collector-compound parabolic concentrated. Results Phys. 2018, 8, 949–954. [Google Scholar] [CrossRef]
- Song, S.; Hwang, S.; Ko, B.; Cha, S.; Jang, G. Novel Transient Power Control Schemes for BTB VSCs to Improve Angle Stability. Appl. Sci. 2018, 8, 1350. [Google Scholar] [CrossRef]
- Clifford, M.J.; Eastwood, D. Design of a novel passive solar tracker. Sol. Energy 2004, 77, 269–280. [Google Scholar] [CrossRef]
- Chong, K.K.; Wong, C.W.; Siaw, F.L.; Yew, T.K.; Liang Lau, S.L. Integration of an on-axis general sun-tracking formula in the algorithm of an open-loop sun-tracking system. Sensors 2009, 9, 7849–7865. [Google Scholar] [CrossRef] [PubMed]
- Hoffmann, F.M.; Molz, R.F.; Kothe, J.V.; Nara, E.O.; Tedesco, L.P.C. Monthly profile analysis based on a two-axis solar tracker proposal for photovoltaic panels. Renew. Energy 2018, 115, 750–759. [Google Scholar] [CrossRef]
- Visconti, P.; Costantini, P.; Orlando, C.; Lay-Ekuakille, A.; Cavalera, G. Software solution implemented on hardware system to manage and drive multiple bi-axial solar trackers by PC in photovoltaic solar plants. Measurement 2015, 76, 80–92. [Google Scholar] [CrossRef]
- Al-Mohamad, A. Efficiency improvements of photo-voltaic panels using a Sun tracking system. Appl. Energy 2004, 79, 345–354. [Google Scholar] [CrossRef]
- Hafez, A.Z.; Yousef, A.M.; Harag, N.M. Solar tracking systems: Technologies and trackers drive types—A review. Renew. Sustain. Energy Rev. 2018, 91, 754–782. [Google Scholar] [CrossRef]
- Seme, S.; Srpčič, G.; Kavšek, D.; Božičnik, S.; Letnik, T.; Praunseis, Z.; Hadžiselimović, M. Dual-axis photovoltaic tracking system e Design and experimental investigation. Energy 2017, 139, 1267–1274. [Google Scholar] [CrossRef]
- Cammarata, A. Optimized design of a large-workspace 2-DOF parallel robot for solar tracking systems. Mech. Machine Theory 2015, 83, 175–186. [Google Scholar] [CrossRef]
- Flores, D.; Palomino, S.; Lozada, N.; Luviano, A.; Chairez, I. Mechatronic design and implementation of a two axes sun tracking photovoltaic system driven by a robotic sensor. Mechatronics 2017, 47, 148–159. [Google Scholar] [CrossRef]
- González, J.; Palacios, C.; Flores, J. Analytical synthesis for four–bar mechanisms used in a pseudo–equatorial solar tracker. Ingeniería e Investigación 2013, 33, 55–60. [Google Scholar]
- Vaidya, V.; Wilson, D. Maximum power tracking in solar cell arrays using time-based reconfiguration. Renew. Energy 2013, 50, 74–81. [Google Scholar] [CrossRef]
- Nsengiyumva, W.; Chen, S.G.; Hu, L.; Chen, X. Recent advancements and challenges in Solar Tracking Systems (STS): A Review. Renew. Sustain. Energy Rev. 2018, 81, 250–279. [Google Scholar] [CrossRef]
- Shabani, M.; Mahmoudimehr, J. Techno-economic role of PV tracking technology in a hybrid PV hydro electric standalone power system. Appl. Energy 2018, 212, 84–108. [Google Scholar] [CrossRef]
- Fathabadi, H. Novel high efficient offline sensor less dual-axis solar tracker for using in photovoltaic systems and solar concentrators. Renew. Energy 2016, 95, 485–494. [Google Scholar] [CrossRef]
- Khadidja, B.; Dris, K.; Boubeker, A.; Noureddine, S. Optimisation of a Solar Tracker System for Photovoltaic Power Plants in Saharian region, Example of Ouargla. Energy Procedia 2014, 50, 610–618. [Google Scholar] [CrossRef]
- Tharamuttam, J.K.; Ng, A.K. Design and Development of an Automatic Solar Tracker. Energy Procedia 2017, 143, 629–634. [Google Scholar] [CrossRef]
- Zhong, H.; Li, G.; Tang, R.; Dong, W. Optical performance of inclined southenorth axis three-positions tracked solar panels. Energy 2011, 36, 1171–1179. [Google Scholar] [CrossRef]
- Ai, B.; Shen, H.; Ban, Q.; Ji, B.; Liao, X. Calculation of the hourly and daily radiation incident on three step tracking planes. Energy Convers. Manag. 2003, 44, 1999–2011. [Google Scholar] [CrossRef]
- Sumathi, V.; Jayapragash, R.; Bakshi, A.; Akella, P.K. Solar tracking methods to maximize PV system output—A review of the methods adopted in recent decade. Renew. Sustain. Energy Rev. 2017, 74, 130–138. [Google Scholar] [CrossRef]
- Huang, B.J.; Huang, Y.C.; Chen, G.Y.; Hsu, P.C.; Li, K. Improving Solar PV System Efficiency Using One-Axis 3-Position Sun Tracking. Energy Procedia 2013, 33, 280–287. [Google Scholar] [CrossRef]
- Haller, J.; Voswinckel, S.; Wesselak, V. The effect of quantum efficiencies on the optimum orientation of photovoltaic modules—A comparison between crystalline and thin film modules. Sol. Energy 2013, 88, 97–103. [Google Scholar] [CrossRef]
- Norton, R. Diseño de Maquinaria: Síntesis y Análisis de Máquinas y Mecanismos, 4th ed.; McGraw-Hill: México City, Mexico, 2009. [Google Scholar]
- Boletín Oficial del Estado, Sección HE 5: Contribución fotovoltaica mínima de energía eléctrica. Código Técnico de la Edificación. Available online: https://www.boe.es (accessed on 1 May 2018).
- Servicio Meteorológico Nacional. Available online: http: //smn.cna.gob.mx (accessed on 1 May 2018).
- Bio-Sol Trackers. Available online: http://www.bio-sol.net (accessed on 1 May 2018).
- Slewing Drive Manufactures. Available online: http://www.slew-ring.com (accessed on 1 May 2018).
- Industrial Machining. Available online: https://www.maquinadoseversteel.com/ (accessed on 1 May 2018).
- Singh, R.; Kumar, S.; Gehlot, A.; Pachauri, R. An imperative role of sun trackers in photovoltaic technology: A review. Renew. Sustain. Energy Rev. 2018, 82, 3263–3278. [Google Scholar] [CrossRef]
- Interempresas Energias. Available online: https://www.interempresas.net, (accessed on 7 February 2019).
- Ruelas, J.; Cota, A.; Ochoa, F.; Lucero, B.; Burgos, T.; Delfin, J.; Soto, A.; Tzab, J.; Lopez, J.; Nañez, J. Structural Design Methodology for Solar Concentrators Subjected to Wind Loads. Energy Procedia 2014, 57, 2872–2878. [Google Scholar] [CrossRef]
- Ambient Weather Stations. Available online: https://www.ambientweather.com, (accessed on 1 May 2018).
- Extech Instruments. Available online: http://www.extech.com (accessed on 1 May 2018).
- Energía Verde RMS. Available online: www.energiaverderms.com.mx (accessed on 1 May 2018).
- Kacira, M.; Simsek, M.; Babur, Y.; Demirkol, S. Determining optimum tilt angles and orientations of photovoltaic panels in Sanliurfa-Turkey, Renew. Energy 2009, 29, 1265–1275. [Google Scholar]
- Eke, R.; Senturk, A. Performance comparison of a double-axis sun tracking versus fixed PV system. Sol. Energy. 2012, 86, 2665–2672. [Google Scholar] [CrossRef]
- Bahrami, A.; Okoye, C.O.; Atikol, U. Technical and economic assessment of fixed, single and dual-axis tracking PV panels in low latitude countries. Renew. Energy 2017, 113, 563–579. [Google Scholar] [CrossRef]
- Serhan, M.; El-chaar, L. Two axes sun tracking system: Comparison with a fixed system. In Proceedings of the International Conference on Renewable Energies and Power Quality, Granada, Spain, 23–25 March 2010. [Google Scholar]





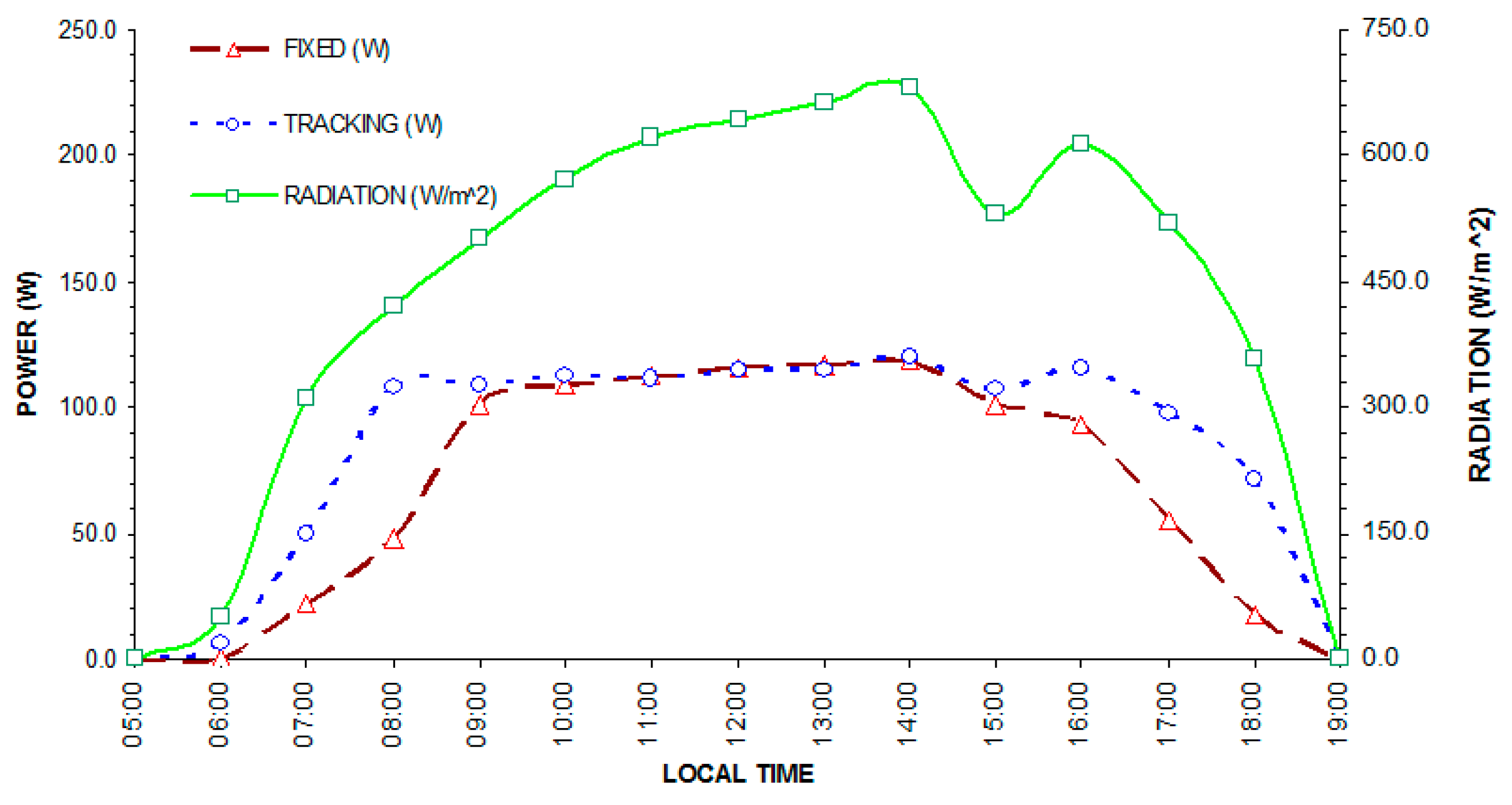
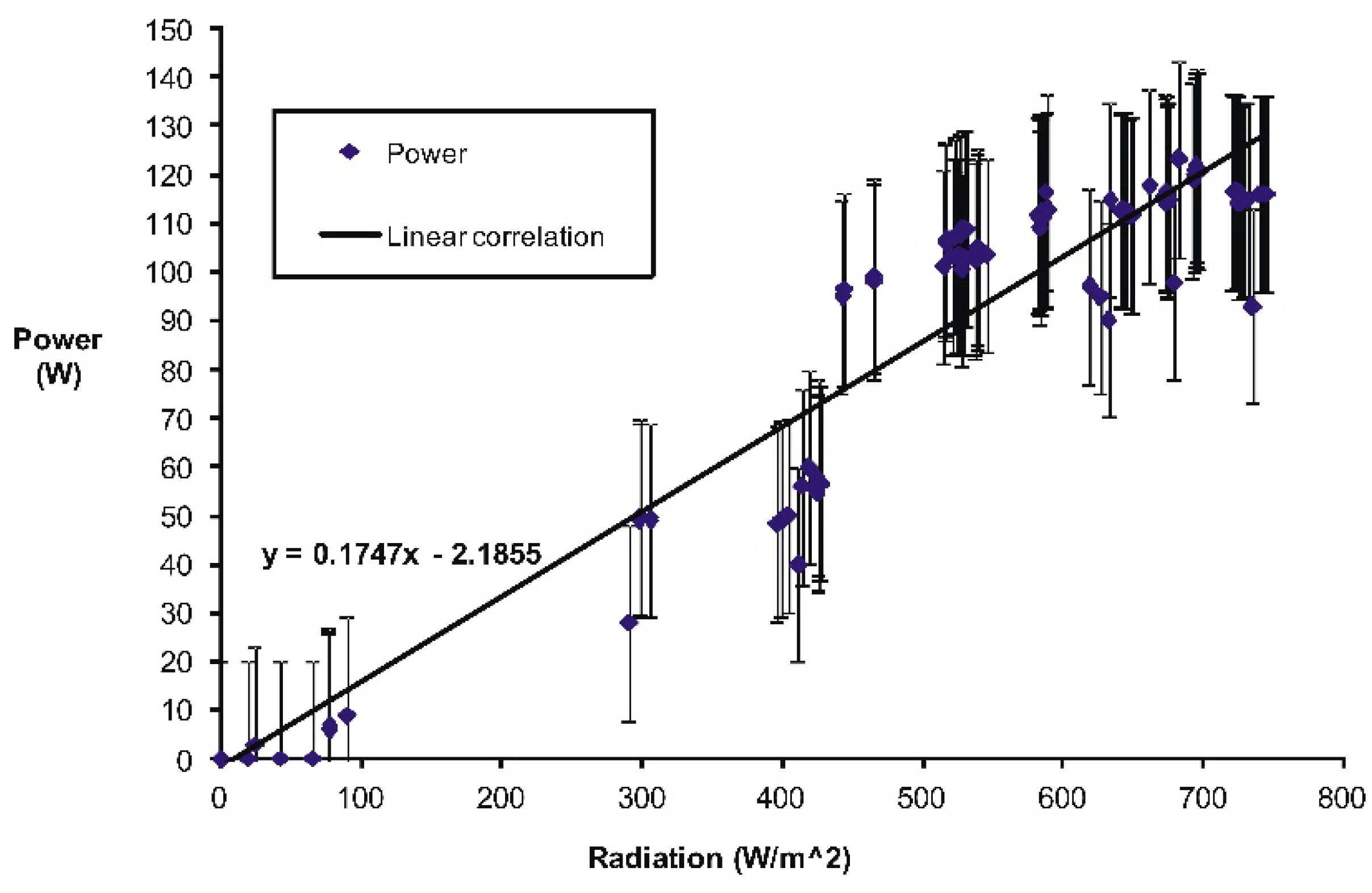
| Design Parameters | Value |
|---|---|
| Latitude | 27.5° |
| Efficiency as a function of the orientation (EFO) | 95–100% |
| Maximum wind speed | 33.3 m/s |
| Capacity | 1 kW |
| Cost | Lowest available |
| Position | Schedule of Tracking (Hours) | Azimuth (°) |
|---|---|---|
| P1 | 06:01 and 10:00 | 30 |
| P2 | 10:01 and 14:00 | 90 |
| P3 | 14:01 and 18:00 | 120 |
| Mechanism | Cost | Availability | Maintenance |
|---|---|---|---|
| Gear motor and linear actuator | L | H | M |
| Two slew drives | M | M | L |
| Two indexed motors | H | L | H |
| Device | Cost | Availability | Maintenance |
|---|---|---|---|
| Diligent field programmable gate array (FPGA) for Linux | L | H | L |
| Arduino microcontroller | L | H | L |
| Festo (programmable logic controller (PLC)) compact unit control | M | M | H |
| Industrial personal computer (PC) with output interfaces | H | L | L |
| Technique | Complexity | Knowledge |
|---|---|---|
| Grafcet | M | H |
| Petri nets | H | L |
| Flow diagrams | L | H |
| Register | Type | Latitude | Test Date | Efficiency Increment |
|---|---|---|---|---|
| Al-Mohamad [7] | Two photo sensors with balance shades | Damascus, Syria 33° N | Summer of 2000 | >20% |
| Serhan and EL-Chaar [39] | Four photo sensors | Libya 33° N | - | 20–28% |
| F.M. Hoffmant [5] | Four photo sensors | Santa Cruz, Brazil 29.7° S | From June to November of 2016 | 17.2–31.1% |
| This work | Open-loop EFO tracker | Cd. Obregon, Mexico 27.5° N | July of 2018 | 23.4% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruelas, J.; Muñoz, F.; Lucero, B.; Palomares, J. PV Tracking Design Methodology Based on an Orientation Efficiency Chart. Appl. Sci. 2019, 9, 894. https://doi.org/10.3390/app9050894
Ruelas J, Muñoz F, Lucero B, Palomares J. PV Tracking Design Methodology Based on an Orientation Efficiency Chart. Applied Sciences. 2019; 9(5):894. https://doi.org/10.3390/app9050894
Chicago/Turabian StyleRuelas, José, Flavio Muñoz, Baldomero Lucero, and Juan Palomares. 2019. "PV Tracking Design Methodology Based on an Orientation Efficiency Chart" Applied Sciences 9, no. 5: 894. https://doi.org/10.3390/app9050894
APA StyleRuelas, J., Muñoz, F., Lucero, B., & Palomares, J. (2019). PV Tracking Design Methodology Based on an Orientation Efficiency Chart. Applied Sciences, 9(5), 894. https://doi.org/10.3390/app9050894








