Alkali Ion Concentration Estimations in Cement Paste Pore Solutions

Featured Application

The testing procedure provided in this paper aims to estimate the available alkali content in cement-based materials, and it has a direct application in the construction materials field. Particularly, it should be used when the potential alkali–silica reactivities of aggregates could be expected.

Abstract

The alkalinity of the pore solution is of great interest for evaluating the rising of the alkali–silica reaction (ASR) when reactive amorphous silica is found in some aggregates in some cement-based composites. This reaction is not desirable because it generates swelling gel materials around the aggregates, which produce an expansive pressure inside concrete over time, and can cause the cracking of concrete, leading to serious structural problems. The purpose of this study is to develop a quick, easy and reliable method to estimate the available alkali concentrations in the pore solution of cement-based composites. The bound alkalis were initially calculated based on Taylor’s alkali distribution method. The proposed procedure to estimate the available alkalis content is a reliable method for use in construction and building composite materials.

1. Introduction

An important durability aspect of concrete structures is their resistance to the alkali–silica reaction (ASR) [1]. This is a deleterious process produced by several chemical reactions between amorphous silica present in some reactive aggregates and sodium, potassium, and hydroxyl ions, among others present in the concrete pore solution. Such reactions produce a gel that expands with the absorbed water. This reaction evolves over several steps [2]; the first one consists of the scission of siloxane bonds (Si-O-Si) in aggregate by hydroxyl ions to generate alkali silicate gel and silicic acid (silanol bonds), followed by the alkali–silica gel expansion due to the water absorption. Three conditions at least have to be satisfied for the concrete alkali–silica reaction (ASR) to take place. First, a high alkali amount must be in the concrete pore solution, second, an amorphous silica aggregate must be present in the concrete, and third, a high humidity level in the environment much be maintained over time, in order to ensure more than 80% relative humidity in the concrete pores [3].

The alkalis concentration in the pore solution of concrete is also a key parameter, because it is one of the major factors for the likelihood of the immobilization of contaminants with Portland cement, and on the reactivity enhancing of coal fly ash and ground granulated blast-furnace slag when are used as Supplementary Cementitious Materials (SCM). Also, the hydroxyl concentration in the pore solution, in equilibrium with the alkali concentration, was important, due to its influence on the passive layer formation around steel reinforcement, to prevent its corrosion. Thus, the partition of alkali ions in the cement paste hydrates and in the pore solution is of great importance.

Usually, atomic absorption spectrophotometry (AAS), flame emission spectrophotometry (FES) or flame photometry is the standard method for the determination of sodium and potassium in cement and concrete components [4,5,6,7].

Taylor [8] proposed an alkali partition theory, and a method for determining the alkali binding capacities of hydrated Portland cement paste. Therefore, part of the alkali ions are bound in the C-S-H gel and monosulphate phases (AF_m) in hydrated cement pastes and the others remain in the pore solution. Following this method, the moles of alkali ions released by the Portland cement hydration which become available for the pore solution could be predicted.

In this work, we develop a simple estimation procedure for the determination of the available sodium and potassium in the pore solution of concrete, based on the Taylor’s empirical theory [8]. The proposed procedure will allow predicting the development of alkali ion concentrations and in turn, the hydroxyl concentration in the pore solution.

This method considers the chemical composition of the Portland cement and the soluble alkali metal content. Then, the fixed alkalis can be calculated. The developed estimation procedure may be applied for the analysis of common Portland cement, CEM I, so it would be suitable for use in the procedure for routine classification of these materials with regard to their usage in engineered barriers for waste confinement design.

2. Materials and Methods

2.1. Common Portland Cements

Six common Portland cements CEM I, according to the European standard EN 197-1:2011 [9,10], were chosen in this study. All of them were provided by Spanish cement producers. One cement was a white cement (code 2), and another was a low-alkali cement (code 6). Also, two sulfate-resistant cements were selected (codes 3 and 4). Chemical analyses of SiO₂, Al₂O₃, Fe₂O₃, CaO, MgO, SO₃, and free lime were performed according to the European standard EN 196-2:2014 [4].

2.2. Total Alkali Determination

Total alkalis determination was performed by atomic absorption spectrophotometry (AAS), using a model SensAA Dual GBC Scientific Equipment on cement samples prepared according to the European standard EN 196-2:2014 [4].

2.3. Water-Soluble Alkali Determination

To determine water-soluble alkalis 25.0 g of sample were weighed and put into a 500 mL Erlenmeyer flask, and 250 mL of distilled water were added. The flask was stopped and then shacked for 10 min at room temperature (20–22 °C). Later, it was filtered through a Büchner funnel using a weak vacuum. Then, a 50 mL aliquot of the filtrate was transferred to a 100 mL volumetric flask and 0.5 mL of concentrated HCl and 9.0 mL of CaCl₂ stock solution (63,000 ppm or 6.3% CaO) were added to the 100 mL flask, and the solution was diluted to 100 mL.

Finally, the contents of each alkali, Na₂O and K₂O in the solution in the 100 mL flask was determined and calculated according to Equation (1):

Total water-soluble alkali, expressed as Na₂O (%) = A + E

(1)

The water-soluble sodium oxide, Na₂O (%), A, was calculated according to Equation (2). Water-soluble potassium oxide, K₂O (%), and C were calculated according to Equation (3) and the water-soluble sodium oxide equivalent, Na₂O_eq (%), E, was calculated according to Equation (4), considering the molecular ratio Na₂O to K₂O of 0.658.

A = B/(V × 10)

(2)

C = D/(V × 10)

(3)

E = C × 0.658

(4)

where:

• A = water-soluble sodium oxide, Na₂O (%).
• V = milliliters of original filtrate in the 100 mL flask.
• B = water-soluble sodium oxide, Na₂O (ppm) in the solution in the 100 mL flask.
• C = water-soluble potassium oxide, K₂O (%).
• D = water-soluble potassium oxide, K₂O (ppm) in the solution in the 100 mL flask.
• E = water-soluble sodium oxide equivalent, Na₂O_eq (%).

3. Results

3.1. Methodology for Estimating Alkali Ions in the Concrete Pore Solution

The chemical compositions of the seven common Portland cements are shown in

Table 1. Chemical compositions of cements determined according to EN 196-2 [4], and the total alkalis measured by atomic absorption spectrometry (%).

Cement	SiO2	Al2O3	Fe2O3	CaO	MgO	SO3	Free Lime	Na2Ot	K2Ot
CEM I 52.5 R	19.52	5.18	3.50	63.74	1.15	3.00	0.88	0.23	0.83
CEM I 52.5 R (white)	22.18	4.63	0.22	69.30	0.55	3.74	1.02	0.05	0.56
CEM I 42.5 R-SR 3	21.73	3.67	4.31	66.12	1.32	2.68	0.36	0.53	0.60
CEM I 52.5 R-SR 5	21.73	3.67	4.31	66.12	1.32	3.00	0.36	0.49	0.57
CEM I 42.5 R	21.29	5.36	2.36	64.15	4.02	3.22	0.69	0.33	0.88
CEM I 52.5 R (low-alkali)	22.16	4.14	3.99	66.35	1.81	2.53	0.42	0.14	0.52

. Clinker phases were calculated by using Bogue equations are shown in

Table 2. Clinker phases calculated using Bogue equations [12], and the theoretical phase composition assumed by Taylor [8], expressed as a percentage.

Clinker Phases Calculated Using Bogue Equations	C3S	C2S	C3A	C4AF	Other	C3S + C2S
Phase composition assumed by Taylor [8]	60	15	8	10	7	75
CEM I 52.5 R	67.80	4.83	7.79	10.64	8.94	72.63
CEM I 52.5 R (white)	77.93	4.81	11.90	0.67	4.69	82.74
CEM I 42.5 R-SR 3	71.81	8.14	2.42	13.10	4.53	79.95
CEM I 52.5 R-SR 5	71.81	8.14	2.42	13.10	4.53	79.95
CEM I 42.5 R	57.18	17.92	10.20	7.17	7.53	75.09
CEM I 52.5 R (low-alkali)	66.53	13.36	4.20	12.13	3.78	79.89

. According to Escalante-Garcia et al. [11], estimated Ca/Si ratios from QXDA, TMBC, and EDS are similar but higher than the Ca/Si ratio obtained by using Bogue equations. Thus, it can be assessed that the results that are obtained using the Bogue calculation showed lower Ca/Si ratios, since the alite predicted content in the cement were lower than the actual one [11]. Table 2 also shows the clinker phase composition assumed by Taylor in his work [8]. This is a very rough estimation that is only applicable for the cement, for which the chemical composition is not available. Therefore, the present estimation method takes into account the cement chemical composition, in order to get a reliable estimation of the pore solution alkalinity.

It is evident that the main clinker phase, C₃S, assumed by Taylor, was moderately reduced compared to that calculated in the present work. This is due to the higher amount of alite present in the modern Portland clinkers. Then, it is recommended that the calculated values of the clinker phase compositions for each cement.

The liquid phase contains several ions, where the alkali ions, K⁺ and Na⁺, and hydroxyl, and OH⁻ are usually the most dominant ions [13,14] in the pore solution. Both of them, K⁺ and Na⁺, are the most abundant cations in the pore solution, and the charge is mainly balanced by the hydroxyl ions.

Also, the alkalis released from the aggregates could increase the hydroxyl ion concentrations in the concrete [15,16]. In particular, the content of the alkali released is enhanced by the high alkalinity of the solution [15]. Also, for the concrete affected by ASR, alkalis are released with the accompanying dissolution of silicate anions [15]. Both are balanced with alkali metal ions. Also, aggregates may bind alkalis to some extent, which changes the pore solution alkalinity.

Lothenbach et al. [17] have proposed a thermodynamic model to study the effects of composition and temperature on the pore solution. They found that with longer ages of hydration, the ion concentrations in the pore solutions are similar at all temperatures, in general. However, the concentrations of sulfate and aluminum increased at high temperature. This fact leads to the promotion of ettringite solubility, which results in a decrease of the hydroxide ions in the pore solution. Nevertheless, the Portland cement pore solution was oversaturated with regard to ettringite, probably due to the low Al/Ca and Al/sulfate ratio, which undermines the precipitation process of ettringite [17].

Table 3. Water-soluble alkalis determined in the cement, which are considered as cations present in sulfate form (%).

Code	Cement	Na2Os	K2Os
1	CEM I 52.5 R	0.13	0.81
2	CEM I 52.5 R (white)	0.02	0.53
3	CEM I 42.5 R-SR 3	0.31	0.56
4	CEM I 52.5 R-SR 5	0.30	0.56
5	CEM I 42.5 R	0.20	0.87
6	CEM I 52.5 R (low-alkali)	0.06	0.51

shows the water-soluble alkalis, as determined by the method described in Section 2.3. According to Taylor [8], it is assumed that all of the water-soluble alkalis in cement are alkali cations that are present as sulfates (Table 3). Taylor proposed an assumed amount of 35% of the total weight of Na₂O in the cement, present as a sulfate, and an amount of 70% of the total weight of K₂O in the cement, present as sulfate, when the proportions are unknown. However, in this paper, an average of 53% of the total weight of Na₂O was Na₂O present as a sulfate in the cement, whereas 97% of the total weight of K₂O was K₂O present as a sulfate in the cement (

Table 4. Fractional distributions of the water-soluble alkalis (sulfate alkalis) in the total alkali content.

Code	Cement	Na2Ot	K2Ot	Na2Os	K2Os	Na2Os/Na2Ot (%)	K2Os/K2Ot (%)
1	CEM I 52.5 R	0.23	0.83	0.13	0.81	58	98
2	CEM I 52.5 R (white)	0.05	0.56	0.02	0.53	41	95
3	CEM I 42.5 R-SR 3	0.53	0.60	0.31	0.56	58	93
4	CEM I 52.5 R-SR 5	0.49	0.57	0.30	0.56	61	98
5	CEM I 42.5 R	0.33	0.88	0.20	0.87	61	99
6	CEM I 52.5 R (low-alkali)	0.14	0.52	0.06	0.51	40	99
Average percentage		-	-	-	-	53	97
Taylor´s average proposal (%) [8]		-	-	-	-	35	70
Minimum percentage		-	-	-	-	40	93
Maximum percentage		-	-	-	-	61	99

). The high variability with regard to the proportion of the soluble alkali cations against the total alkali cations suggests that it will be necessary, in the new proposed method, to use experimentally determined results.

Another source of error can be found in the testing method, because it is assumed that all the water-soluble alkali in the cement, mainly sulfates, will be dissolved. However, it could be possible that not all of the water-soluble alkalis in the cement were dissolved [18]. Then, the real amount of alkalis present as sulfates could be even higher. According to Taylor [8], most of the potassium normally is present as potassium sulfate whereas sodium was not the case.

Table 4 shows that sodium is bounded in cement paste hydrates in a higher extension than potassium, in agreement with other authors [16,19]. Whereas Taylor proposed that only about 70% of the potassium is water-soluble, in this research, almost all of the potassium is water-soluble. Also, he proposed a procedure for determining the alkali binding capacities of hydrated Portland cement paste.

Table 5. Fractional distribution of the total non-sulfate Na₂O and K₂O that are assumed to occur in the main clinker phases [8].

Non-Sulfate Alkalis Fraction	Alite	Belite	Aluminate	Ferrite
Non-sulfate Na2O fraction	0.44	0.17	0.36	0.03
Non-sulfate K2O fraction	0.29	0.41	0.27	0.03

gives the fractional distribution of the total non-sulfate Na₂O and K₂O that are assumed to occur in the main clinker phases, which have been considered in this work.

The contents of Na₂O and K₂O in each of the major phases were then calculated (

Table 6. Total non-soluble alkalis and fractions of Na₂O and K₂O bounded in the main clinker phases.

Na2O and K2O Fractions in Each Phase			0.44	0.29	0.17	0.41	0.36	0.27	0.03	0.03
CEMENT	Calculated Non-Soluble Alkalis (%)		C3S (%)		C2S (%)		C3A (%)		C4AF (%)
	Na2O	K2O	Na2O	K2O	Na2O	K2O	Na2O	K2O	Na2O	K2O
CEM I 52.5 R	0.10	0.02	0.04	0,01	0,02	0,01	0,03	0,01	0.00	0.00
CEM I 52.5 R (white)	0.03	0.03	0.01	0.01	0.01	0.01	0.01	0.01	0.00	0.00
CEM I 42.5 R-SR 3	0.23	0.04	0.10	0.01	0.04	0.02	0.08	0.01	0.01	0.00
CEM I 52.5 R-SR 5	0.19	0.01	0.08	0.00	0.03	0.00	0.07	0.00	0.01	0.00
CEM I 42.5 R	0.13	0.01	0.06	0.00	0.02	0.00	0.05	0.00	0.00	0.00
CEM I 52.5 R (low-alkali)	0.08	0.01	0.04	0.00	0.01	0.00	0.03	0.00	0.00	0.00
Average (%)	0.13	0.02	0.06	0.01	0.02	0.01	0.05	0.01	0.00	0.00
Maximum (%)	0.23	0.04	0.10	0.01	0.04	0.02	0.08	0.01	0.01	0.00
Minimum (%)	0.03	0.01	0.01	0.00	0.01	0.00	0.01	0.00	0.00	0.00
Taylor’s proposal (%)			0.17	0.14	0.27	0.79	1.05	0.97	0.06	0.07

) by using the fractional distribution that was proposed by Taylor, and given in Table 5.

Table 6 shows the total non-reactive alkalis that are calculated by subtracting the measured total alkalis content from the measured water-soluble alkalis, as well as the assumed distribution to occur in the main clinker phases.

Dalziel and Gutteridge [19] proposed an empirical formula (Equation (5)) to express the rates of reaction of alite, belite, aluminate, and ferrite. In this function, F is the fraction of the major clinker phases reacted at time T, in days, and k₁, k₂, and k₃ are constants, as shown in

Table 7. Constant values assumed in the empirical Equation (5), where F is the fraction that is reacted at time T (in days) [19].

F, the Fraction Reacted at Time T (in days)	k1	k2	k3
Fraction of hydrated alite	0.25	0.70	0.90
Fraction of hydrated belite	0.46	0.12	0.00
Fraction of hydrated aluminate	0.28	0.77	0.90
Fractions of hydrated ferrite	0.26	0.55	0.90
Bound water (fraction of 31.6 g/100 g cement)	0.25	0.69	0.90
Ca in the C-S-H and AFm phases (fraction of 791.0 mmol/100 g cement)	0.25	0.56	0.90

.

F = 1 − exp [−k₂(T − k₃)^k1]

(5)

Equation (5) was then used in the present work to obtain the fractions that were reacted for each clinker phase, C₃S, C₂S, C₃A, and C₄AF, bound water, and Ca in C-S-H and AF_m phases, at time T (in days) replacing the set of constants that are given in Table 7. Figure 1 illustrates the fractions reacted for the C₃S, C₂S, C₃A, and C₄AF clinker phases, bound water, and Ca uptake in the C-S-H and AF_m phases over time, as calculated with Equation (5).

Then, the fractions reacted for each clinker phase at 28 days was multiplied by the weight of Na₂O or K₂O in the phase, to obtain the weight of Na₂O or K₂O that was released, respectively.

Table 8. Weight of Na₂O or K₂O released from each main phase at 28 days.

Time (T, days)	28								Alkalis Released from the Main Clinker Phases
F the Fraction of the Major Clinker Phases Reacted at Time T	0.798		0.426		0.856		0.727
CEMENT	C3S (%)		C2S (%)		C3A (%)		C4AF (%)		Total (%)
	Na2O	K2O	Na2O	K2O	Na2O	K2O	Na2O	K2O	Na2O	K2O
CEM I 52.5 R	0.03	0.00	0.01	0.00	0.03	0.00	0.00	0.00	0.07	0.01
CEM I 52.5 R (white)	0.01	0.01	0.00	0.01	0.01	0.01	0.00	0.00	0.02	0.02
CEM I 42.5 R-SR 5	0.08	0.01	0.02	0.01	0.07	0.01	0.00	0.00	0.17	0.03
CEM I 52.5 R-SR 5	0.07	0.00	0.01	0.00	0.06	0.00	0.00	0.00	0.14	0.01
CEM I 42.5 R	0.05	0.00	0.01	0.00	0.04	0.00	0.00	0.00	0.10	0.01
CEM I 52.5 R (low-alkali)	0.03	0.00	0.01	0.00	0.03	0.00	0.00	0.00	0.06	0.00

presents the results obtained. The mass of Na₂O or K₂O that was released from the major clinker phases was added to that present as sulfates (Table 3), and the total alkalis present in the pore solution after 28 days are shown in

Table 9. Na₂O or K₂O in the pore solution (water-soluble plus released alkalis) at 28 days.

CEMENT	Na2O (%)	K2O (%)	Na2O (mmol)	K2O (mmol)
CEM I 52.5 R	0.21	0.82	3.33	8.74
CEM I 52.5 R (white)	0.04	0.55	0.69	5.84
CEM I 42.5 R-SR 5	0.47	0.59	7.65	6.22
CEM I 52.5 R-SR 5	0.44	0.57	7.15	6.02
CEM I 42.5 R	0.30	0.88	4.81	9.31
CEM I 52.5 R (low alkali)	0.12	0.52	1.92	5.50

, expressed as in units of millimoles.

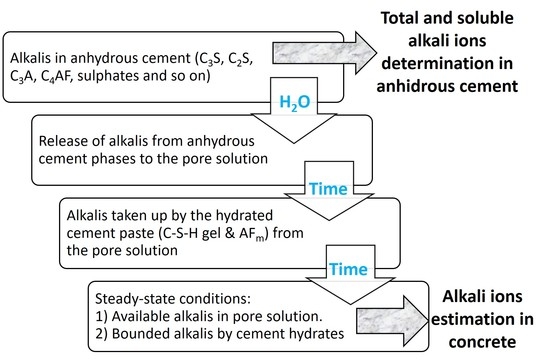
Finally, Figure 2 summarizes the procedure proposed in the present paper to estimate the alkali ion concentrations in the cement pore solution, which is a reliable method for use in engineered barriers for waste confinement design.

3.1.1. Volume of the Pore Solution

First, the volume of the bound water was calculated, multiplying 31.6 by the quantity F as in Equation (5), with k₁, k₂, and k₃ constants (shown in Table 7) of 0.25, 0.69, 0.90, respectively. For 28-days, the F-value was 0.793. Finally, the volume of the pore solution was calculated, subtracting the volume of the bound water from (100 × water–cement). The free water amount of the cement paste has very little influence on the determination of the free alkali metal ions when the water extraction method is used [20]. Therefore, determining this parameter was not necessary.

Volume of pore solution = V = (100 × 0.5) − (31.6 × 0.793) = 24.93 cm³

(6)

3.1.2. Amount of Calcium Present in the C-S-H and AF_m Phases

The amount of Ca present in the C-S-H and AF_m phases was also calculated by Equation (5), with k₁, k₂, and k₃ constant values (shown in Table 7) of 0.25, 0.56, 0.90, respectively. After 28 days, the F-value was 0.721.

The amount of alkali ions taken up by the C-S-H and AF_m phases depends on the amounts present, and the alkali-binding capacities of the mentioned hydration products. Knowledge of the alkali-binding capacities of such hydration productions will thus be useful when developing methods for predicting alkali and hydroxyl concentrations in the pore solution.

The alkali concentrations used by Hong and Glasser [21,22] for determining the alkali binding capacity were between 1 and 300 mmol/L for the C–S–H gel, and between 15 and 300 mmol/L for the C–A–S–H gel. However, the distribution ratio in real hydrated cement pastes could be significantly different from that obtained in synthetic pastes.

3.1.3. Na⁺ and K⁺ Concentrations in the Pore Solution

An alkali partition procedure was proposed, according to which part of the alkali ions are bound in the hydration products, also called C-S-H gel and AF_m phases and the others remain in the pore solution. From the mass balance, the quantity of the alkali cations released from the cement, m_released, is equal to that which is contained in the pore solution, mpore solution, plus that which is taken up by the hydration products, m_{taken up} (Equation (7)):

m_released = m_{pore solution} + m_{taken up}

(7)

The amount in solution is equal to the alkali concentration, [Na⁺] or [K⁺], which is assumed to be uniform, is multiplied by the volume of solution, V. The amount of Na⁺ or K⁺ that is taken up by the cement hydration products is assumed to be proportional to the amount of such hydration products, and also proportional to the Na⁺ or K⁺ concentration in solution. Therefore, their concentrations in the pore solution and in the hydration products were calculated by using Equations (8) and (9), respectively:

m_{pore solution} = [Na⁺ or K⁺] × V

(8)

m_{taken up} = b × P × [Na⁺ or K⁺]

(9)

where P is the amount of the hydration product that is able to take up alkali ions, divided by the same amount at complete hydration. Therefore, P is dimensionless. The constant b is named as the “binding factor” by Taylor [8]. Combining Equations (7)–(9), we obtain Equation (10) and (11).

m_released= m_{pore solution} + m_{taken up} = [Na⁺ or K⁺] × V + b × P × [Na⁺ or K⁺]

(10)

$$\left[{\mathrm{Na}}^{+} \mathrm{or} {\mathrm{K}}^{+}\right]=\frac{{\mathrm{m}}_{\mathrm{released}}}{\left[\mathrm{V}+\left(\mathrm{b}\times \mathrm{P}\right)\right]}$$

(11)

The “binding factor”, b, is equal to 31.0 cm³ for Na⁺ and 20.0 cm³ for K⁺, and it can be defined as an equivalent volume of pore solution. P is the quantity F that is obtained from Equation (5), equal to 0.721 at after 28 days. The volume of the pore solution, V, was calculated in Section 3.1, and it is equal to 24.93 cm³.

Finally, the amount in solution is equal to the alkali concentration, [Na⁺ or K⁺] at 28 days, which is shown in

Table 10. Na₂O or K₂O concentrations (mmol/L) in the pore solution at 28 days.

Code	Na2O (%)	K2O (%)	Na2O (mole)	K2O (mole)	Na2O (mmol)	K2O (mmol)	V (cm3)	b (Na+) cm3	b (K+) cm3	P	[Na+] mmol/L	[K+] mmol/L	[SO42−] mmol/L
1	0.21	0.82	0.0033	0.0087	3.33	8.74	24.93	31.0	20.0	0.721	0.0704	0.2221	0.0051
2	0.04	0.55	0.0007	0.0058	0.69	5.84	24.93	31.0	20.0	0.721	0.0146	0.1483	0.0016
3	0.47	0.59	0.0077	0.0062	7.65	6.22	24.93	31.0	20.0	0.721	0.1619	0.1579	0.0061
4	0.44	0.57	0.0071	0.0060	7.15	6.02	24.93	31.0	20.0	0.721	0.1512	0.1529	0.0055
5	0.30	0.88	0.0048	0.0093	4.81	9.31	24.93	31.0	20.0	0.721	0.1017	0.2365	0.0069
6	0.12	0.52	0.0019	0.0055	1.92	5.50	24.93	31.0	20.0	0.721	0.0407	0.1398	0.0020

.

Kawabata and Yamada [23] developed a model to estimate the pore solution alkalinity. They found that a reduction in pore solution alkalinity is the key factor for reducing ASR expansion. Also, they reported on the effect of the Ca/Si ratio, and the amount of C-S-H gel on the alkali uptake. The effect of the coal fly ash on the Portland cement paste is the formation of a higher amount of C-S-H gel, with a lower Ca/Si ratio. On the contrary, granulated blast-furnace slag leads to a higher amount of C-S-H gel, with a similar Ca/Si ratio than the plain Portland cement. Thus, the higher amount of C-S-H gel is, the higher alkali metal ions uptake. However, they can be released from the C-S-H gel in the long-term [23].

3.2. SO₄²⁻ and OH⁻ Concentrations in the Pore Solution

Sulfate concentration, [SO₄²⁻], was estimated from the Na⁺ and K⁺ concentrations, using the empirical equation found by Struble [24] (Equation (12)). The mean calculated concentration of SO₄²⁻ was 0.0045 mol/L, and the maximum value was 0.0069 mole/L (

Table 11. SO₄²⁻ and OH⁻ concentrations in the pore solution.

[SO42−] Calculated (mmol/L)	[OH−]Calculated from Charge Balance (mmol/L)	Na2Oequivalent (mole)	[OH−] Calculated from Helmuth Equation (mmol/L)	[OH−] Difference between Charge Balance and Helmuth Equation (mmol/L)	(%)
0.0051	0.2822	0.0091	0.2262	0.0561	19.87
0.0016	0.1597	0.0045	0.2231	−0.0633	−39.66
0.0061	0.3075	0.0117	0.2280	0.0796	25.88
0.0055	0.2930	0.0111	0.2275	0.0654	22.34
0.0069	0.3245	0.0109	0.2274	0.0971	29.93
0.0020	0.1765	0.0055	0.2238	−0.0472	−26.74

).

[SO₄²⁻] = 0.06 × ([Na⁺] + [K⁺])²

(12)

Then, hydroxyl concentration, [OH⁻], was calculated from charge balance, as shown in Equation (13) (Table 11):

[OH⁻] = [Na⁺] + [K⁺] − (2 × [SO₄²⁻])

(13)

Also, hydroxyl concentration, [OH⁻], was calculated from the empirical equation for predicting the hydroxyl ion concentrations of the pore solution of mature Portland cement pastes, developed by Helmuth et al. [25] (Equation (14)). In this last case, it is suggested that the calculated hydroxyl concentrations present an average error of about 27%, partially due to the equation for Na₂O_e concentration:

$$\left[{\mathrm{OH}}^{-}\right]=\frac{0.339\cdot \left[{\mathrm{Na}}_2{\mathrm{O}}_{\mathrm{e}}\right]}{water/cement}+0.022 \mathrm{mol}/\mathrm{L}$$

(14)

where [Na₂O_e] = [Na₂O] + 0.658 × [K₂O].

3.3. Relationship between the Water-Soluble Alkalis and the Major Clinker Phases

Figure 3 shows the relationship between the water-soluble alkalis obtained, following the procedure detailed in Section 2.3 (an extraction time of less than 30 min) and the C₃S. It is clear that sodium uptake was preferred. Also, it was found that the concentrations of both Na⁺ and K⁺ in the solution apparently decreased with increasing C₃S content in the clinker, particularly for K⁺. Therefore, the less alkali ions that are available for the pore solution, because more alkali ions are bound in the C–S–H gel. Also, C₃S hydration consumes less water than C₂S hydration, leaving more water available for the pore solution, resulting in a dilution of the alkali ions [18].

Bach et al. [26] found that the fraction of potassium uptake by low-pH cements is more than three times higher than that of sodium, disagreeing with the results shown in Figure 3. This significant difference was explained by the smaller solvated ionic radius of potassium, and because potassium ions can enter the C-S-H gel interlayer to compensate for the negative charges [26]. They also suggested that C-(A)-S-H is the phase that is mainly involved in the alkali retention by an effect of charge compensation. In addition, they reported a clear correlation between the dissolved alkali and alkali retention by C-S-H gel. In contrast, L’Hôpital et al. [27] did not find any difference between sodium and potassium uptake. They observed that the alkali uptake depends on the Ca/Si ratio in C-S-H and C-A-S-H gels. Thus, the lower the Ca/Si ratio is, the greater the uptake of alkali metal ions.

Different behaviors of C–S–H gel concerning the binding of Na⁺ and K⁺ are observed in Figure 3, where the effects of the alkali types in cement on the alkalinity of the pore solution is observed. The C–S–H gel binds less K⁺ than Na⁺, particularly at high concentrations, and more alkali ions remain in the solution [16]. Figure 4 shows the soluble alkali concentrations in the pore solution of hydrated Portland cement paste as a function of the amount of C₃S, C₂S, C₃S + C₂S, and CaO/SiO₂ ratio in the clinkers.

According to various national regulations and standards, the total alkali content in cement is regulated as Na₂O_equivalent. Therefore, the alkali types are not distinguished, and little attention is paid to the different types of alkalis, though the resultant alkalinity of the pore solution is quite different. Thus, the influence of mineral composition on the alkali concentrations was investigated using updated methods in this study. A water–cement ratio of 0.5 was considered. The C₃S content in the clinker ranged from 57.18% to 77.93%, while the C₂S content ranged from 4.81% to 17.92%, accordingly. These totaled 76% of the clinker content. The C₃A and C₄AF contents in the clinker ranged from 2.42% to 11%, and from 0.67% to 13.10%, respectively.

Figure 5 shows the relationship between the water-soluble alkalis of anhydrous cement and the water-soluble alkalis of hydrated cement paste after 28 days. In the case of the sodium ions, the trend was clearly linear (r² = 0.98); whereas in the case of potassium, this was less evident. From the analysis water-solubility data for alkalis, it can be concluded that sodium was significantly better bonded into the hydrated cement paste than potassium, as reported in the literature [8]. However, the large size of the solvated sodium ion hinders its uptake by interlayer sites of the C-S-H gel [26]. Alkali cations compete with calcium cations to compensate for the surface negative charge of the C-S-H gel. Bivalent cations present a higher electrostatic interaction; therefore, high amounts of calcium cations can hinder alkali uptake [28]. On the contrary, the pH increases at high alkali concentrations, and this enhances silanol site de-protonation, leading to a more negatively charged C-S-H gel surface. In addition, the high pH induces a lower calcium concentration in equilibrium with the C-S-H gel. Both occurrences cause an increase in alkali metal binding [28].

Some authors found an increase in alkali metal uptake in the presence of C-A-S-H gels, which was attributed to SiO₂ substitution by AlO₂⁻, and the need for the uptake of more positive cations, to maintain charge equilibrium [22]. On the contrary, some studies observed less alkali metal uptake in the C-A-S-H gels than in the C-S-H gels [29], while other studies found no significant difference in the systems, with or without aluminum [26].

Brouwers and Van Eijk [30] investigated the alkali binding capacities of hydrated Portland cement pastes following the same method proposed by Taylor [8], but they used a computer model to predict the amount of alkali ions released by the cement hydration. They also proposed that the C–S–H gel is the only hydration product that is able to bind alkalis, instead of the relative fraction of C–S–H and AF_m phases that are formed in the paste. Currently, it is considered that the alkali metal uptake by AF_m is much lower than that of the C-S-H gel. Given that, most researchers have focused on alkali uptake by the C-S-H gel. They also suggest that the constant values of alkali distribution ratios imply that the alkali-binding capacity of C–S–H gel is linearly proportional to the alkali concentrations in the pore solution; this was verified for Na⁺, but not for K⁺ [31]. Therefore, Chen and Browers [31] found a linear relationship between the molar concentration of Na⁺ in the C-S-H gel, and its concentration in the pore solution, with a proportionality constant of 0.45. The distribution ratio in cm³/g found by other authors was 0.39 [8,21]. In the present work, such a constant is 1.6. On the contrary, they did not find a similar relationship in the case of K⁺, and then, they proposed a non-linear relationship that is similar to a Freundlich isotherm, between the molar concentration of K⁺ in the C-S-H gel, and the molar concentration of K⁺ in the pore solution. In the present work, neither a linear relationship was found. Nevertheless, a good relationship was found between the total K₂O and K₂O/SiO₂ ratios (Figure 6). SiO₂ is determined by X-ray fluorescence (XRF) in the Portland cement. It was found that the total K₂O of the paste correlated well with the K₂O/SiO₂ ratio; increasing the total K₂O in the clinker appeared to increase the overall K₂O/SiO₂ ratio.

Finally, the numerical prediction was compared with water-soluble alkali determination, as determined in the pastes after 28 days.

4. Conclusions

A new method was developed and successfully applied for the estimation of the available alkalis in a concrete pore solution, using data for the total and soluble sodium and potassium in anhydrous cement, based on the method proposed by Taylor. Knowledge of the distributions of alkali ions in the solid phase and the solution is valuable for a better understanding of the development of the hydroxyl concentration in the pore solution of concrete. The total and soluble sodium concentration relationship was linear, whereas that for potassium was not linear. On the other hand, the hydroxyl concentration could be better estimated by the charge balance from the alkali and sulfate ion concentrations; therefore, empirical equations should be avoided.

The procedure proposed in this paper to estimate the available alkalis concentrations in the cement pore solution is a reliable method for use in engineered barriers for waste confinement designs.