Signal Strength Enhancement of Magnetostrictive Patch Transducers for Guided Wave Inspection by Magnetic Circuit Optimization
Abstract
:Featured Application
Abstract
1. Introduction
2. Theory
2.1. Magnetostrictive Equation
2.2. Enhancement with a Soft Magnetic Patch
3. Numerical Simulation
3.1. Simulation Setup
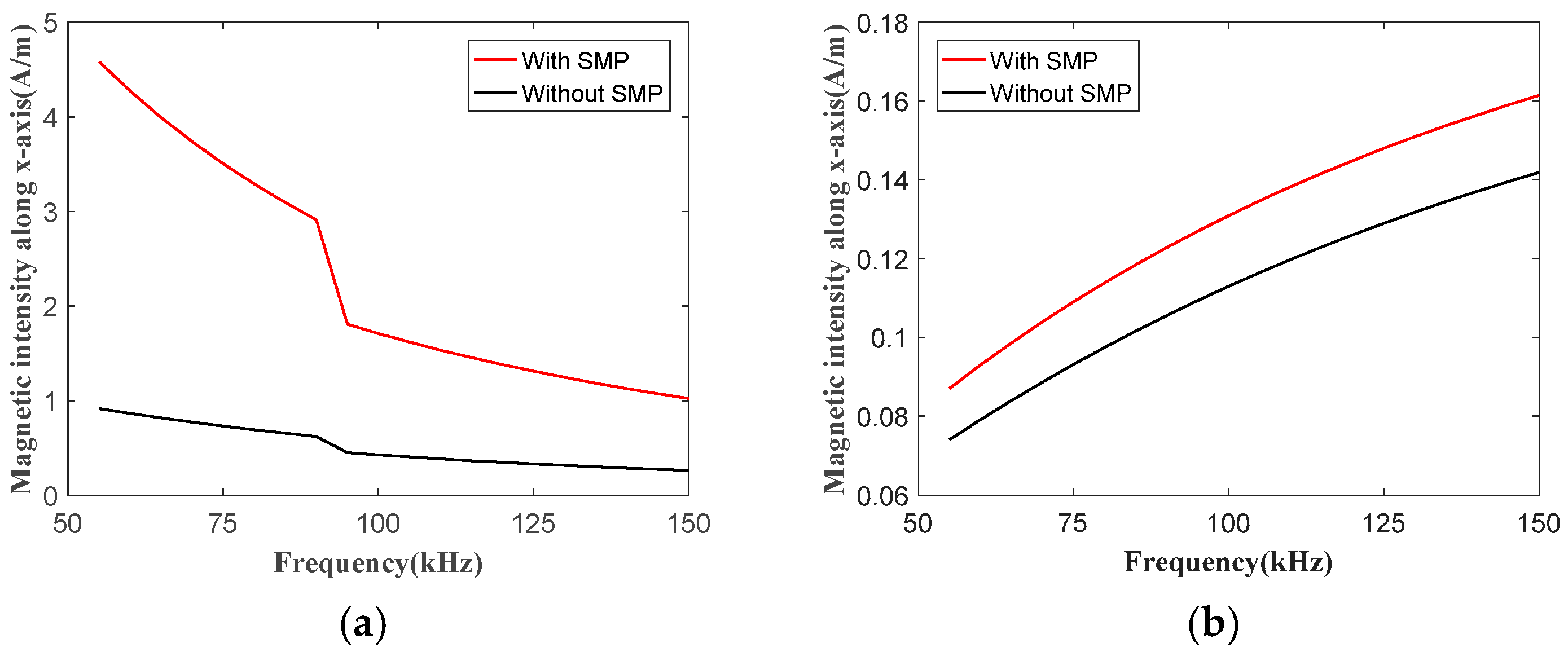
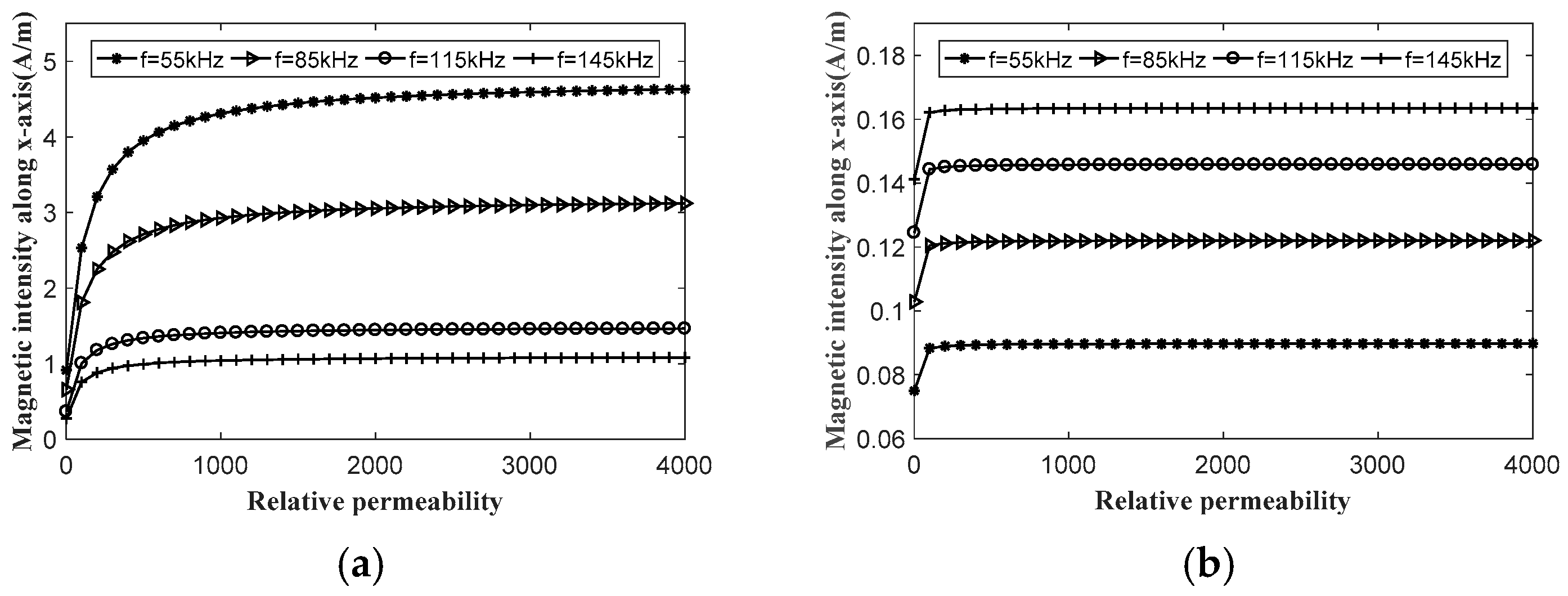
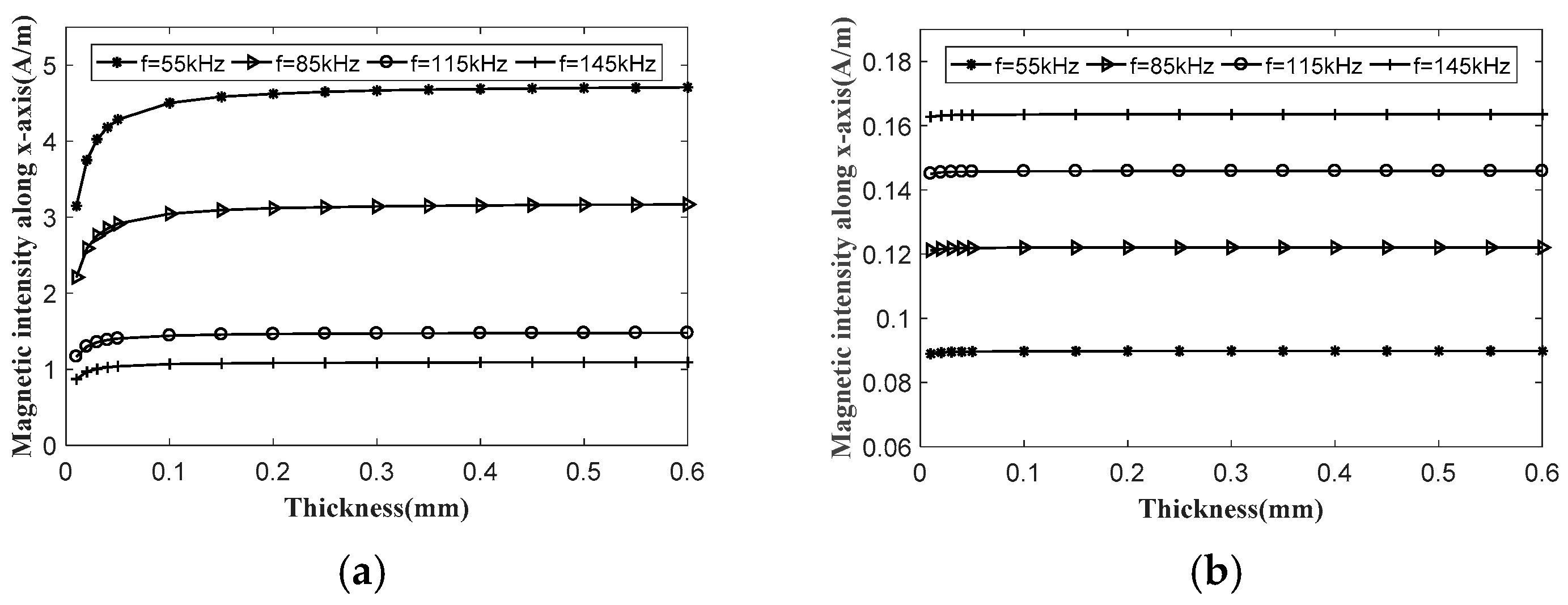
3.2. Simulation Verification and Factor Analysis
4. Experimental Investigation
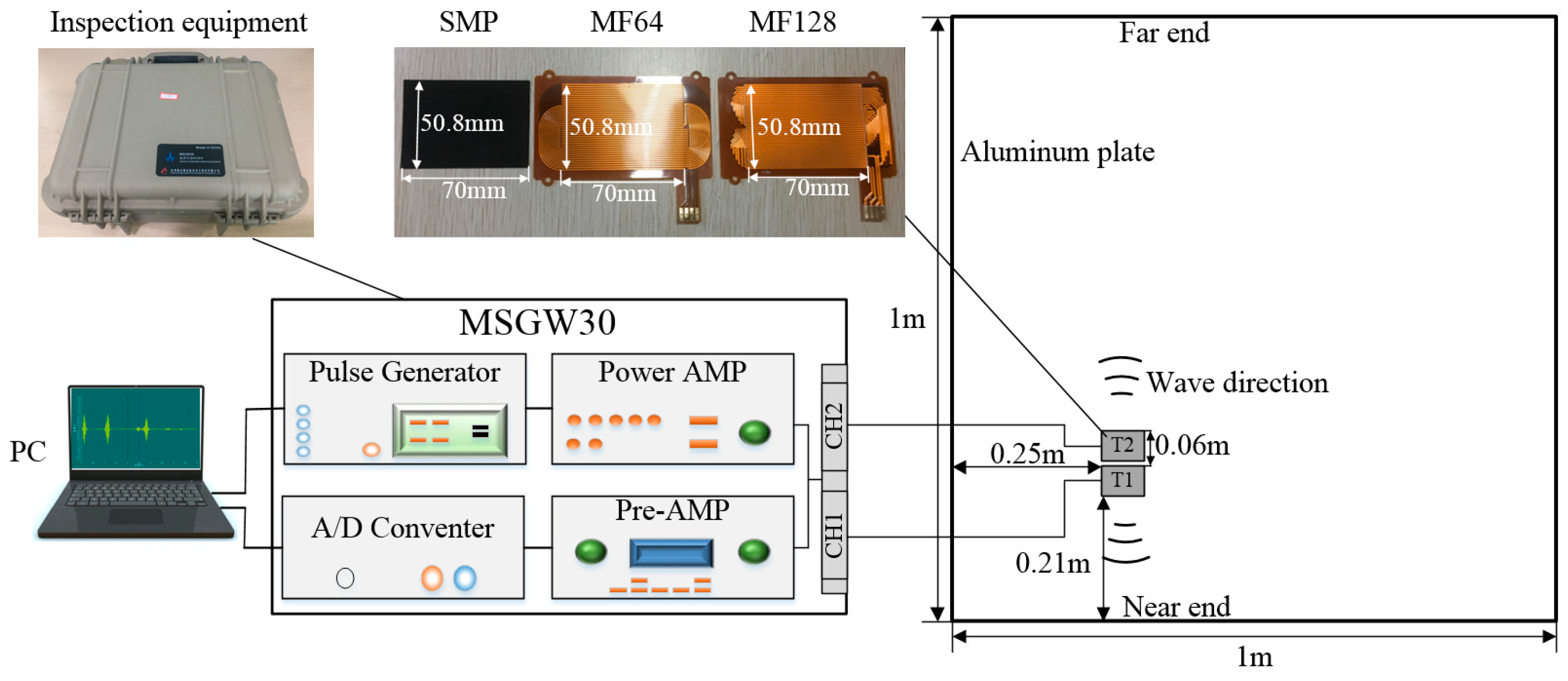
4.1. Experimental Setup
4.2. Results and Discussions
5. Conclusions
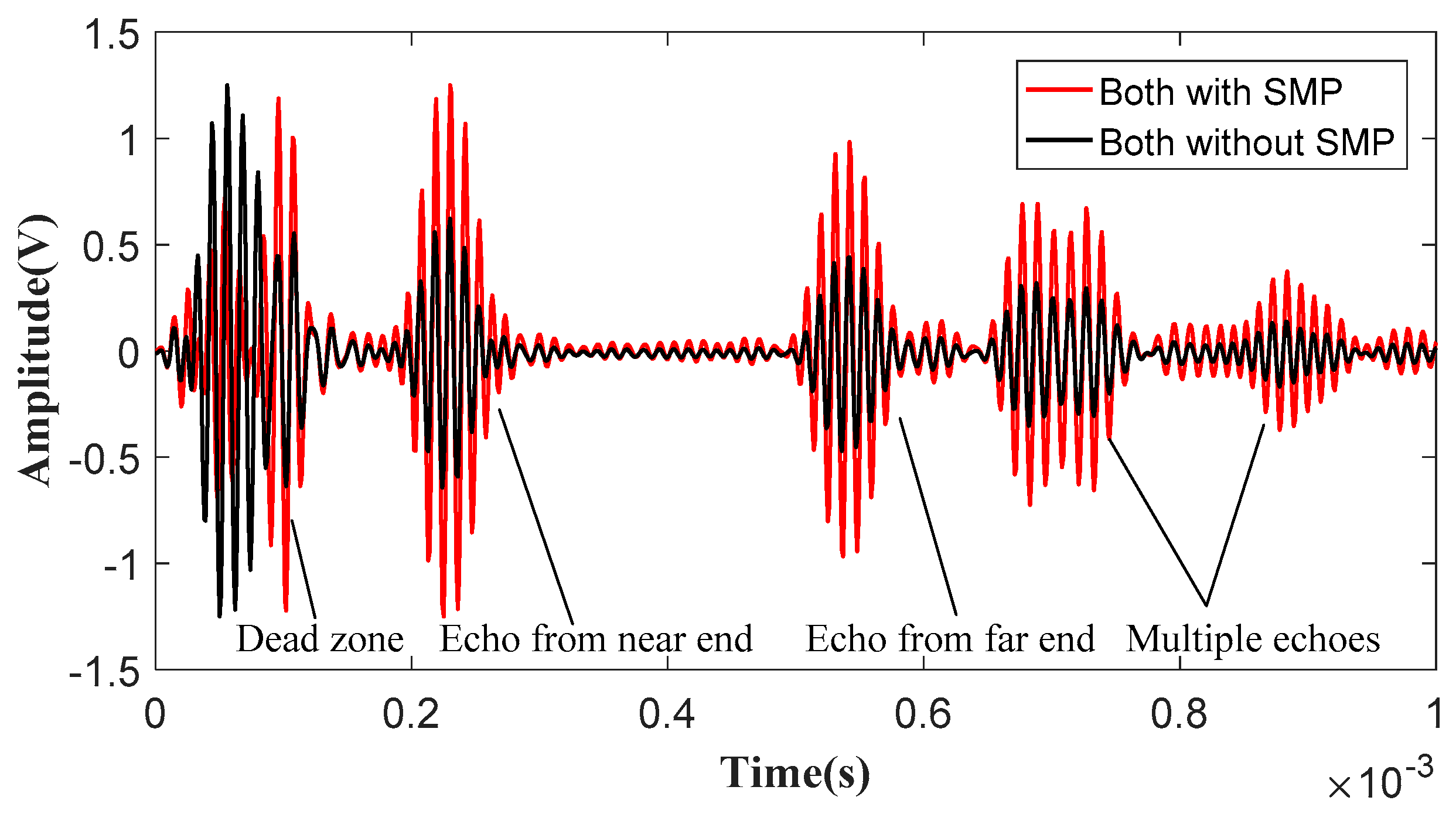
- The amplitude enhancement of the dynamic magnetic field in the magnetostrictive patch is beneficial for SH wave generation and the concentration of the induced magnetic field by the magnetostrictive patch in wave detection is good for the measurement by the coils.
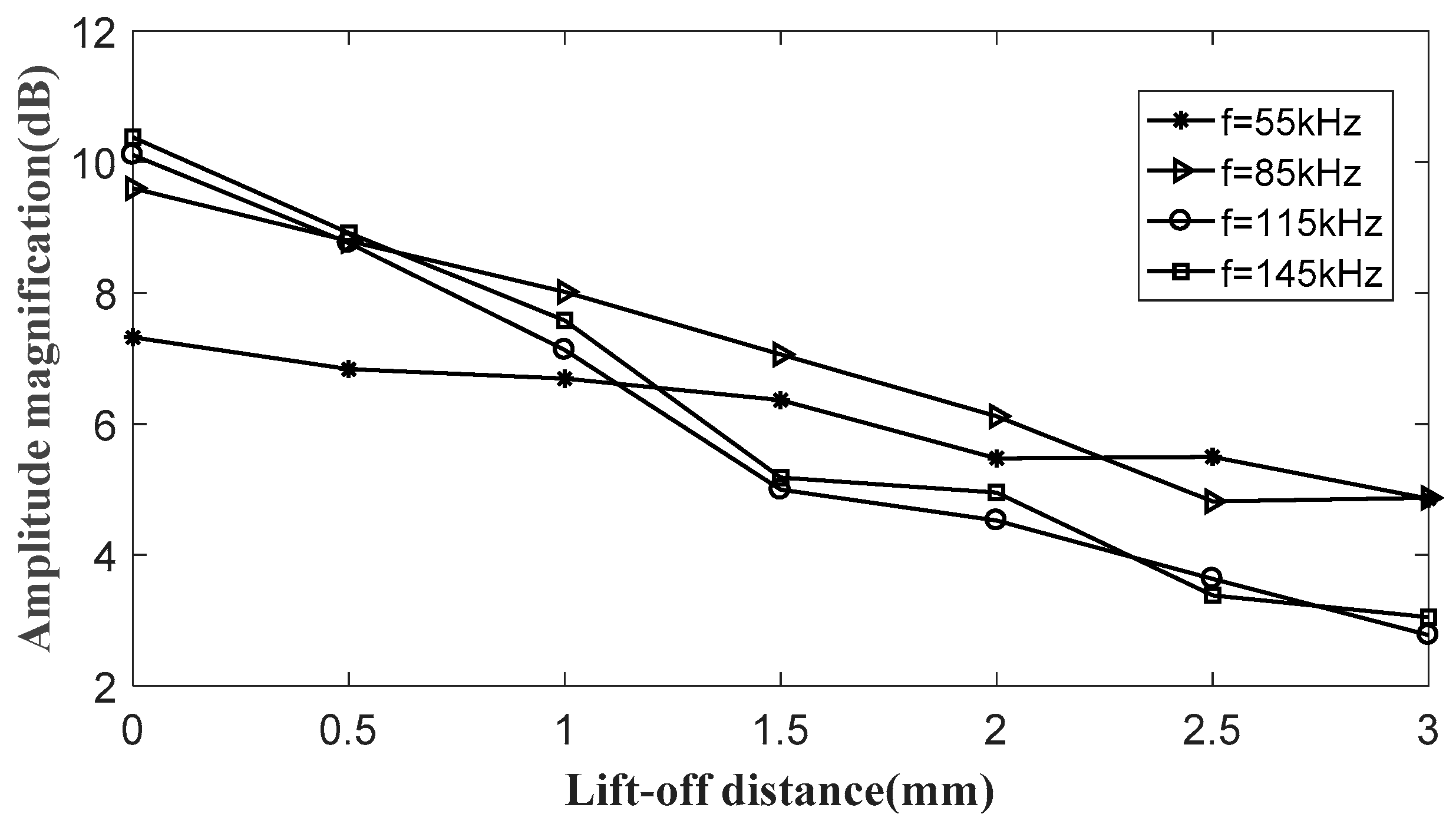
- The SMP can significantly decrease the resistance of the magnetic circuit for the dynamic magnetic field in wave generation and concentrates the induced magnetic field in wave detection. The relative permeability should be more than 2000 and the thickness should be over 0.15 mm. The increased distance between the SMP and the coils was bad for the improvement.
- Covered by the SMPs with the relative permeability of 2800, the MPTs provided a better performance in both processes of wave generation and detection compared to those without the SMPs. The improvement of the SMP in wave detection was more than that in wave generation. The largest magnification can be 12.7 dB when the transducer is used as an actuator and sensor at the same time.
- For ideal results, using the SMPs with the relative permeability of 2800, a thickness over 0.3 mm and close fitting between the SMP and coils is recommended.
Author Contributions
Funding
Conflicts of Interest
References
- Rose, J.L. Ultrasonic Guided Waves in Solid Media, 1st ed.; Cambridge University Press: Cambridge, UK, 2014; p. 1. ISBN 978-1-107-04895-9. [Google Scholar]
- Heinlein, S.; Cawley, P.; Vogt, T.K. Reflection of torsional T (0, 1) guided waves from defects in pipe bends. NDT E Int. 2018, 93, 57–63. [Google Scholar] [CrossRef]
- Leinov, E.; Lowe, M.J.S.; Cawley, P. Investigation of guided wave propagation and attenuation in pipe buried in sand. J. Sound Vib. 2015, 347, 96–114. [Google Scholar] [CrossRef] [Green Version]
- Hayashi, T.; Tamayama, C.; Murase, M. Wave structure analysis of guided waves in a bar with an arbitrary cross-section. Ultrasonics 2006, 44, 17–24. [Google Scholar] [CrossRef] [PubMed]
- Seung, H.M.; Park, C.I.; Kim, Y.Y. An omnidirectional shear-horizontal guided wave EMAT for a metallic plate. Ultrasonics 2016, 69, 58–66. [Google Scholar] [CrossRef] [PubMed]
- Hasanian, M.; Lissenden, C.J. Second order harmonic guided wave mutual interactions in plate: Vector analysis, numerical simulation, and experimental results. J. Appl. Phys. 2017, 122, 084901. [Google Scholar] [CrossRef]
- Yu, X.; Fan, Z.; Castaings, M.; Biateau, C. Feature guided wave inspection of bond line defects between a stiffener and a composite plate. NDT E Int. 2017, 89, 44–55. [Google Scholar] [CrossRef]
- Wu, J.; Tang, Z.; Lv, F.; Yang, K.; Yun, C.B. Ultrasonic guided wave-based switch rail monitoring using independent component analysis. Meas. Sci. Technol. 2018, 29, 115102. [Google Scholar] [CrossRef]
- Evans, M.; Lucas, A.; Ingram, I. The inspection of level crossing rails using guided waves. Constr. Build. Mater 2018, 179, 614–618. [Google Scholar] [CrossRef]
- Yao, W.; Sheng, F.; Wei, X.; Zhang, L.; Ynag, Y. Propagation characteristics of ultrasonic guided waves in continuously welded rail. Modern Phys. Lett. B 2017, 31, 1740075. [Google Scholar] [CrossRef] [Green Version]
- Nakamura, N.; Ogi, H.; Hirao, M. EMAT pipe inspection technique using higher mode torsional guided wave T (0, 2). NDT E Int. 2017, 87, 78–84. [Google Scholar] [CrossRef]
- Kogia, M.; Gan, T.H.; Balachandran, W.; Livadas, M.; Kappatos, V.; Szabo, I.; Mohimi, A.; Round, A. High temperature shear horizontal electromagnetic acoustic transducer for guided wave inspection. Sensors 2016, 16, 582. [Google Scholar] [CrossRef]
- Lee, J.R.; Chia, C.C.; Park, C.Y.; Jeong, H. Laser ultrasonic anomalous wave propagation imaging method with adjacent wave subtraction: Algorithm. Opt. Laser Technol. 2012, 44, 1507–1515. [Google Scholar] [CrossRef]
- Lee, J.R.; Chia, C.C.; Shin, H.J.; Park, C.Y.; Yoon, D.J. Laser ultrasonic propagation imaging method in the frequency domain based on wavelet transformation. Opt. Lasers Eng. 2011, 49, 167–175. [Google Scholar] [CrossRef]
- Sevillano, E.; Sun, R.; Perera, R. Damage detection based on power dissipation measured with PZT sensors through the combination of electro-mechanical impedances and guided waves. Sensors 2016, 16, 639. [Google Scholar] [CrossRef]
- Vinogradov, S.; Cobb, A.; Fisher, J. New Magnetostrictive Transducer Designs for Emerging Application Areas of NDE. Materials 2018, 11, 755. [Google Scholar] [CrossRef]
- Kwun, H.; Bartels, K.A. Magnetostrictive sensor technology and its applications. Ultrasonics 1998, 36, 171–178. [Google Scholar] [CrossRef]
- Wang, W.B.; Cao, S.Y.; Huang, W.M. Magnetostrive Materials and Devices, 1st ed.; Metallurgical Industry Press: Beijing, China, 2008; pp. 8–10. ISBN 9787502445324. (In Chinese) [Google Scholar]
- Kim, Y.Y.; Kwon, Y.E. Review of magnetostrictive patch transducers and applications in ultrasonic nondestructive testing of waveguides. Ultrasonics 2015, 62, 3–19. [Google Scholar] [CrossRef] [Green Version]
- Tzannes, N.S. Joule and Wiedemann effects-The simultaneous generation of longitudinal and torsional stress pulses in magnetostrictive materials. IEEE Trans. Sonics Ultrason. 1966, 13, 33–40. [Google Scholar] [CrossRef]
- Xu, J.; Wu, X.; Cheng, C.; Ben, A. A magnetic flux leakage and magnetostrictive guided wave hybrid transducer for detecting bridge cables. Sensors 2012, 12, 518–533. [Google Scholar] [CrossRef]
- Xu, J.; Wu, X.; Sun, P. Detecting broken-wire flaws at multiple locations in the same wire of prestressing strands using guided waves. Ultrasonics 2013, 53, 150–156. [Google Scholar] [CrossRef]
- Park, C.; Kim, Y.Y. Nonferromagnetic material inserted magnetostrictive patch bonding technique for torsional modal testing of a ferromagnetic cylinder. Rev. Sci. Instrum. 2010, 81, 035103. [Google Scholar] [CrossRef]
- Kumar, K.S.; Murthy, V.; Balasubramaniam, K. Improvement in the signal strength of magnetostrictive ultrasonic guided wave transducers for pipe inspection using a soft magnetic ribbon-based flux concentrator. Insight 2012, 54, 217–220. [Google Scholar] [CrossRef]
- Kim, H.W.; Cho, S.H.; Kim, Y.Y. Analysis of internal wave reflection within a magnetostrictive patch transducer for high-frequency guided torsional waves. Ultrasonics 2011, 51, 647–652. [Google Scholar] [CrossRef]
- Cho, S.H.; Lee, J.S.; Kim, Y.Y. Guided wave transduction experiment using a circular magnetostrictive patch and a figure-of-eight coil in nonferromagnetic plates. Appl. Phys. Lett. 2006, 88, 224101. [Google Scholar] [CrossRef]
- Yoo, B.; Na, S.M.; Flatau, A.B.; Pines, D.J. Ultrasonic guided wave sensing performance of a magnetostrictive transducer using Galfenol flakes-polymer composite patches. J. Appl. Phys. 2015, 117, 17A916. [Google Scholar] [CrossRef]
- Kim, Y.; Kim, Y.Y. A novel Terfenol-D transducer for guided-wave inspection of a rotating shaft. Sens. Actuators A Phys. 2007, 133, 447–456. [Google Scholar] [CrossRef]
- Cho, S.H.; Kim, H.W.; Kim, Y.Y. Megahertz-range guided pure torsional wave transduction and experiments using a magnetostrictive transducer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 1225–1229. [Google Scholar] [CrossRef]
- Park, C.I.; Cho, S.H.; Kim, Y.Y. Z-shaped magnetostrictive patch for efficient transduction of a torsional wave mode in a cylindrical waveguide. Appl. Phys. Lett. 2006, 89, 174103. [Google Scholar] [CrossRef]
- Park, C.I.; Kim, W.; Cho, S.H.; Kim, Y.Y. Surface-detached V-shaped yoke of obliquely bonded magnetostrictive strips for high transduction of ultrasonic torsional waves. Appl. Phys. Lett. 2005, 87, 224105. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, Z.; Lv, F.; Pan, X. Helical comb magnetostrictive patch transducers for inspecting spiral welded pipes using flexural guided waves. Ultrasonics 2017, 74, 1–10. [Google Scholar] [CrossRef]
- Kim, Y.G.; Moon, H.S.; Park, K.J.; Lee, J.K. Generating and detecting torsional guided waves using magnetostrictive sensors of crossed coils. NDT E Int. 2011, 44, 145–151. [Google Scholar] [CrossRef]
- Lee, J.S.; Kim, Y.Y.; Cho, S.H. Beam-focused shear-horizontal wave generation in a plate by a circular magnetostrictive patch transducer employing a planar solenoid array. Smart Mater. Struct. 2008, 18, 015009. [Google Scholar] [CrossRef]
- Huang, S.L.; Wang, K.; Zhao, W. Theory and Application of Electromagnetic Ultrasonic Guided Wave, 1st ed.; Tsinghua University Press: Beijing, China, 2013; p. 74. ISBN 978-7-302-33673-0. [Google Scholar]
- Zhang, X.W.; Tang, Z.F.; Lv, F.Z.; Pan, X.H. Excitation of axisymmetric and non-axisymmetric guided waves in elastic hollow cylinders by magnetostrictive transducers. J. Zhejiang Univ.-Sci. A 2016, 17, 215–229. [Google Scholar] [CrossRef]
- Yang, Q.; Li, Y.; Zhao, Z.; Zhu, L.; Luo, Y.; Zhu, J. Design of a 3-D rotational magnetic properties measurement structure for soft magnetic materials. IEEE Trans. Appl. Supercond. 2014, 24, 1–4. [Google Scholar] [CrossRef]
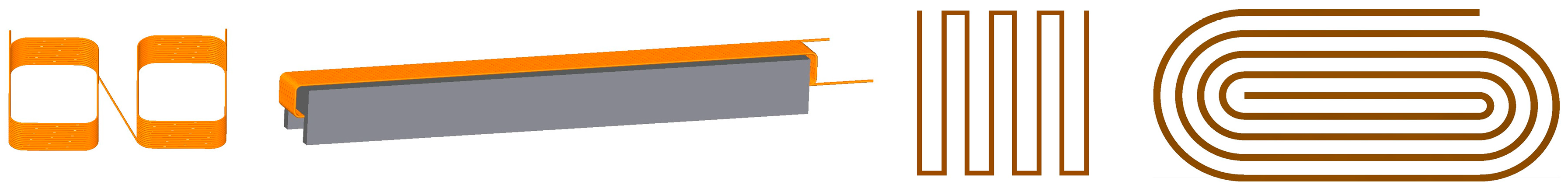



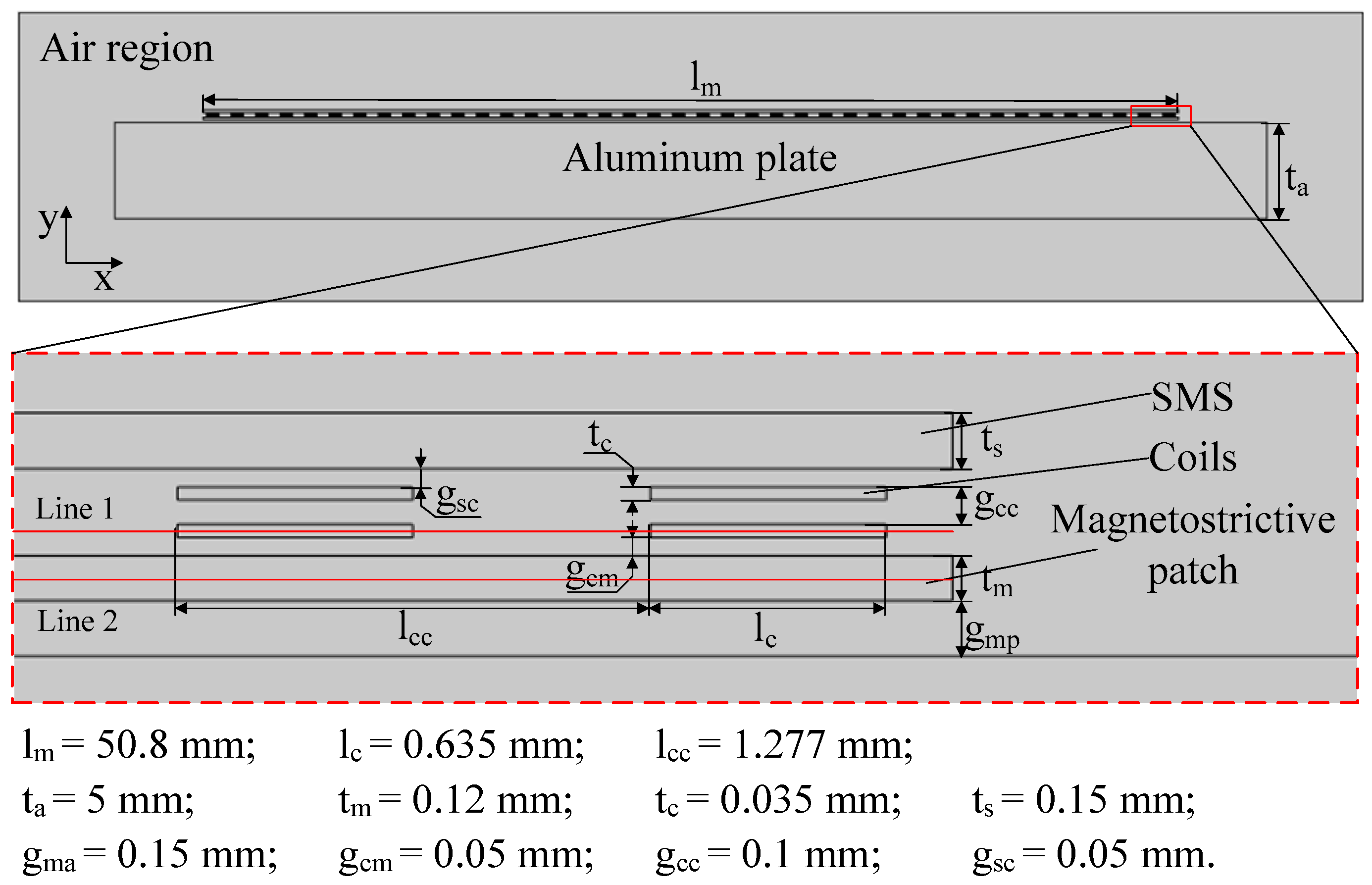
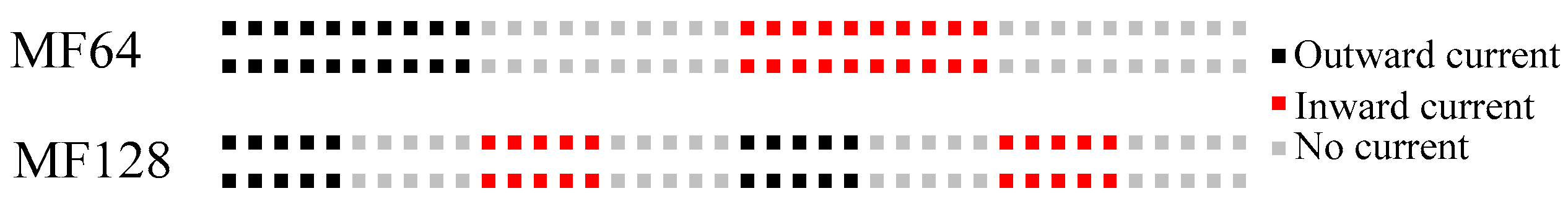

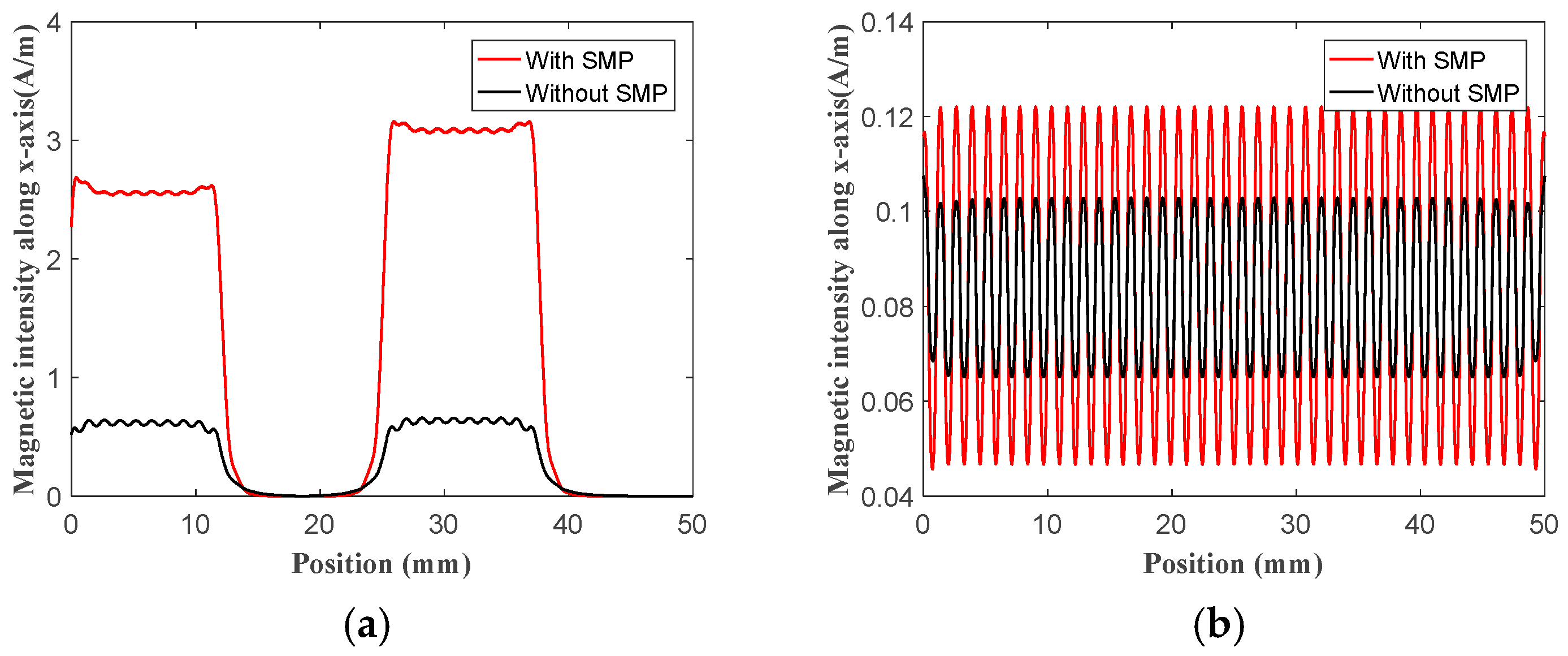



| Material Name | Air | Aluminum | Copper | Fe–Co Alloy | Mn–Zn Ferrite |
|---|---|---|---|---|---|
| Relative permeability | 1 | 1 | 1 | 2000 | 2800 |
| Conductivity (S/m) | 0 | 3.774 × 107 | 5.988 × 107 | 2.5 × 106 | 0.17 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Tang, Z.; Yang, K.; Lv, F. Signal Strength Enhancement of Magnetostrictive Patch Transducers for Guided Wave Inspection by Magnetic Circuit Optimization. Appl. Sci. 2019, 9, 1477. https://doi.org/10.3390/app9071477
Wu J, Tang Z, Yang K, Lv F. Signal Strength Enhancement of Magnetostrictive Patch Transducers for Guided Wave Inspection by Magnetic Circuit Optimization. Applied Sciences. 2019; 9(7):1477. https://doi.org/10.3390/app9071477
Chicago/Turabian StyleWu, Jianjun, Zhifeng Tang, Keji Yang, and Fuzai Lv. 2019. "Signal Strength Enhancement of Magnetostrictive Patch Transducers for Guided Wave Inspection by Magnetic Circuit Optimization" Applied Sciences 9, no. 7: 1477. https://doi.org/10.3390/app9071477
APA StyleWu, J., Tang, Z., Yang, K., & Lv, F. (2019). Signal Strength Enhancement of Magnetostrictive Patch Transducers for Guided Wave Inspection by Magnetic Circuit Optimization. Applied Sciences, 9(7), 1477. https://doi.org/10.3390/app9071477





