Size-Dependent Free Vibrations of FG Polymer Composite Curved Nanobeams Reinforced with Graphene Nanoplatelets Resting on Pasternak Foundations
Abstract
:1. Introduction
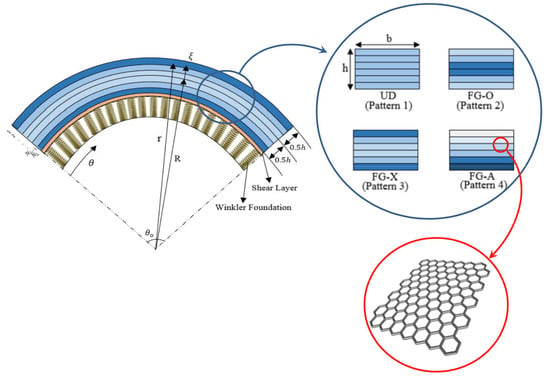
2. Effective Material Properties and Constitutive Relations
3. Solution Procedure
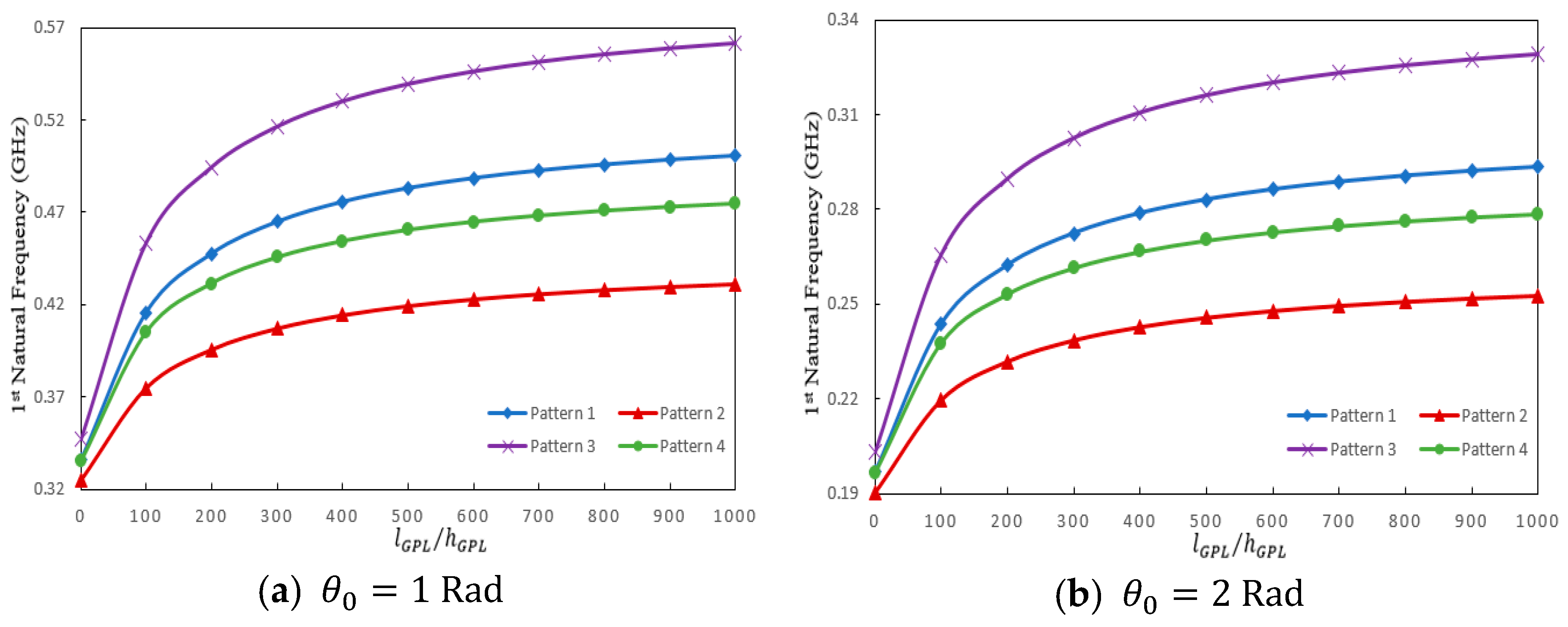
4. Numerical Results and Discussion
Comparison and Validation
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A
References
- Rafiee, M.A.; Rafiee, J.; Wang, Z.; Song, H.; Yu, Z.Z.; Koratkar, N. Enhanced Mechanical Properties ofnanocomposites at low graphene content. ACS Nano 2009, 3, 3884–3890. [Google Scholar] [CrossRef] [PubMed]
- Liang, J.; Wang, Y.; Huang, Y.; Ma, Y.; Liu, Z.; Cai, J. Letter to the Editor Electromagnetic interference shielding of graphene/epoxy composites. Carbon N. Y. 2008, 47, 922–925. [Google Scholar] [CrossRef]
- Shen, H.S.; Xiang, Y.; Lin, F. Nonlinear vibration of functionally graded graphene-reinforced composite laminated plates in thermal environments. Comput. Methods Appl. Mech. Eng. 2017, 319, 175–193. [Google Scholar] [CrossRef]
- Wang, Y.; Feng, C.; Zhao, Z.; Yang, J. Buckling of Graphene Platelet Reinforced Composite Cylindrical Shell with Cutout. Int. J. Struct. Stab. Dyn. 2018, 18, 1850040. [Google Scholar] [CrossRef]
- Song, M.; Kitipornchai, S.; Yang, J. Free and forced vibrations of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Struct. 2017, 159, 579–588. [Google Scholar] [CrossRef]
- Feng, C.; Kitipornchai, S.; Yang, J. Nonlinear free vibration of functionally graded polymer composite beams reinforced with graphene nanoplatelets (GPLs). Eng. Struct. 2017, 140, 110–119. [Google Scholar] [CrossRef]
- Sahmani, S.; Aghdam, M.M. Nonlocal strain gradient beam model for nonlinear vibration of prebuckled and postbuckled multilayer functionally graded GPLRC nanobeams. Compos. Struct. 2017, 179, 77–88. [Google Scholar] [CrossRef]
- Zhao, Z.; Feng, C.; Wang, Y.; Yang, J. Bending and vibration analysis of functionally graded trapezoidal nanocomposite plates reinforced with graphene nanoplatelets (GPLs). Compos. Struct. 2017, 180, 799–808. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Nonlinear vibration and postbuckling of functionally graded graphene reinforced porous nanocomposite beams. Compos. Sci. Technol. 2017, 142, 235–245. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Feng, C.; Zhao, Z.; Yang, J. Eigenvalue buckling of functionally graded cylindrical shells reinforced with graphene platelets (GPL). Compos. Struct. 2017. [Google Scholar] [CrossRef]
- Yang, B.; Kitipornchai, S.; Yang, Y.F.; Yang, J. 3D thermo-mechanical bending solution of functionally graded graphene reinforced circular and annular plates. Appl. Math. Model. 2017, 49, 69–86. [Google Scholar] [CrossRef] [Green Version]
- Kitipornchai, S.; Chen, D.; Yang, J. Free vibration and elastic buckling of functionally graded porous beams reinforced by graphene platelets. Mater. Des. 2017, 116, 656–665. [Google Scholar] [CrossRef]
- Song, M.; Yang, J.; Kitipornchai, S.; Zhu, W. Buckling and postbuckling of biaxially compressed functionally graded multilayer graphene nanoplatelet-reinforced polymer composite plates. Int. J. Mech. Sci. 2017, 131, 345–355. [Google Scholar] [CrossRef]
- Feng, C.; Kitipornchai, S.; Yang, J. Nonlinear bending of polymer nanocomposite beams reinforced with non-uniformly distributed graphene platelets (GPLs). Compos. Part B Eng. 2017, 110, 132–140. [Google Scholar] [CrossRef]
- Gholami, R.; Ansari, R. Large deflection geometrically nonlinear analysis of functionally graded multilayer graphene platelet-reinforced polymer composite rectangular plates. Compos. Struct. 2017, 180, 760–771. [Google Scholar] [CrossRef]
- Song, M.; Yang, J.; Kitipornchai, S. Bending and buckling analyses of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Part B Eng. 2018, 134, 106–113. [Google Scholar] [CrossRef]
- Hosseini, S.A.H.; Rahmani, O. Free vibration of shallow and deep curved FG nanobeam via nonlocal Timoshenko curved beam model. Appl. Phys. A Mater. Sci. Process 2016, 122, 169. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Barati, M.R. A nonlocal strain gradient refined beam model for buckling analysis of size-dependent shear-deformable curved FG nanobeams. Compos. Struct. 2017, 159, 174–182. [Google Scholar] [CrossRef]
- Rahmani, O.; Hosseini, S.A.H.; Hayati, H. Frequency analysis of curved nano-sandwich structure based on a nonlocal model. Mod. Phys. Lett. B 2016, 30, 1650136. [Google Scholar] [CrossRef]
- Hayati, H.; Hosseini, S.A.; Rahmani, O. Coupled twist–bending static and dynamic behavior of a curved single-walled carbon nanotube based on nonlocal theory. Microsyst. Technol. 2016. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Thermo-electro-mechanical bending behavior of sandwich nanoplate integrated with piezoelectric face-sheets based on trigonometric plate theory. Compos. Struct. 2017, 162, 108–122. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Effect of thermo-magneto-electro-mechanical fields on the bending behaviors of a three-layered nanoplate based on sinusoidal shear-deformation plate theory. J. Sandw. Struct. Mater. 2017. [Google Scholar] [CrossRef]
- Aya, S.A.; Tufekci, E. Modeling and analysis of out-of-plane behavior of curved nanobeams based on nonlocal elasticity. Compos. Part B Eng. 2017, 119, 184–195. [Google Scholar] [CrossRef]
- Hajianmaleki, M.; Qatu, M.S. Static and vibration analyses of thick, generally laminated deep curved beams with different boundary conditions. Compos. Part B Eng. 2012, 43, 1767–1775. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Nonlocal electro-thermo-mechanical analysis of a sandwich nanoplate containing a Kelvin–Voigt viscoelastic nanoplate and two piezoelectric layers. Acta Mech. 2017, 228, 475–493. [Google Scholar] [CrossRef]
- Arefi, M. Surface effect and non-local elasticity in wave propagation of functionally graded piezoelectric nano-rod excited to applied voltage. Appl. Math. Mech. 2016, 37, 289–302. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Transient sinusoidal shear deformation formulation of a size-dependent three-layer piezo-magnetic curved nanobeam. Acta Mech. 2017, 228, 3657–3674. [Google Scholar] [CrossRef]
- Romano, G.; Barretta, R. Comment on the paper “Exact solution of Eringen’s nonlocal integral model for bending of Bernoulli-Euler and Timoshenko beams” by meral Tuna & Mesut Kirca. Int. J. Eng. Sci. 2016, 109, 240–242. [Google Scholar] [CrossRef]
- Barretta, R.; Feo, L.; Luciano, R.; Marotti de Sciarra, F. Application of an enhanced version of the Eringen differential model to nanotechnology. Compos. Part B Eng. 2016, 96, 274–280. [Google Scholar] [CrossRef]
- Barretta, R.; Feo, L.; Luciano, R.; Marotti de Sciarra, F.; Penna, R. Functionally graded Timoshenko nanobeams: A novel nonlocal gradient formulation. Compos. Part B Eng. 2016, 100, 208–219. [Google Scholar] [CrossRef]
- Apuzzo, A.; Barretta, R.; Canadija, M.; Feo, L.; Luciano, R.; Marotti de Sciarra, F. A closed-form model for torsion of nanobeams with an enhanced nonlocal formulation. Compos. Part B Eng. 2017, 108, 315–324. [Google Scholar] [CrossRef]
- Barati, M.R.; Shahverdi, H. Vibration analysis of multi-phase nanocrystalline silicon nanoplates considering the size and surface energies of nanograins/nanovoids. Int. J. Eng. Sci. 2017, 119, 128–141. [Google Scholar] [CrossRef]
- Romano, G.; Barretta, R. Nonlocal elasticity in nanobeams: The stress-driven integral model. Int. J. Eng. Sci. 2017, 115, 14–27. [Google Scholar] [CrossRef]
- Romano, G.; Barretta, R.; Diaco, M.; Marotti de Sciarra, F. Constitutive boundary conditions and paradoxes in nonlocal elastic nano-beams. Int. J. Mech. Sci. 2017, 121, 151–156. [Google Scholar] [CrossRef]
- Farokhi, H.; Ghayesh, M.H. On the dynamics of imperfect shear deformable microplates. Int. J. Eng. Sci. 2018, 133, 264–283. [Google Scholar] [CrossRef]
- Barretta, R.; Ali Faghidian, S.; Marotti de Sciarra, F. Stress-driven nonlocal integral elasticity for axisymmetric nano-plates. Int. J. Eng. Sci. 2019, 136, 38–52. [Google Scholar] [CrossRef]
- Barretta, R.; Marotti de Sciarra, F. Constitutive boundary conditions for nonlocal strain gradient elastic nano-beams. Int. J. Eng. Sci. 2018, 130, 187–198. [Google Scholar] [CrossRef]
- Faghidian, S.A. Reissner stationary variational principle for nonlocal strain gradient theory of elasticity. Eur. J. Mech. A Solids 2018, 70, 115–126. [Google Scholar] [CrossRef]
- Asadi, H. Numerical simulation of the fluid-solid interaction for CNT reinforced functionally graded cylindrical shells in thermal environments. Compos. Struct. 2017, 138, 214–224. [Google Scholar] [CrossRef]
- Asadi, H.; Souri, M.; Wang, Q. A numerical study on flow-induced instabilities of supersonic FG-CNT reinforced composite flat panels in thermal environments. Compos. Struct. 2017, 171, 113–125. [Google Scholar] [CrossRef]
- Jouneghani, F.Z.; Dimitri, R.; Bacciocchi, M.; Tornabene, F. Free vibration analysis of functionally graded porous doubly-curved shells based on the First-order Shear Deformation Theory. Appl. Sci. 2017, 7, 1252. [Google Scholar] [CrossRef]
- Nejati, M.; Dimitri, R.; Tornabene, F.; Yas, M.H. Thermal buckling of nanocomposite stiffened cylindrical shells reinforced by functionally Graded wavy Carbon NanoTubes with temperature-dependent properties. Appl. Sci. 2017, 7, 1223. [Google Scholar] [CrossRef]
- Keleshteri, M.M.; Asadi, H.; Wang, Q. Postbuckling analysis of smart FG-CNTRC annular sector plates with surface-bonded piezoelectric layers using generalized differential quadrature method. Comput. Methods Appl. Mech. Eng. 2017, 325, 689–710. [Google Scholar] [CrossRef]
- Asadi, H.; Beheshti, A.R. On the nonlinear dynamic responses of FG-CNTRC beams exposed to aerothermal loads using thord-order piston theory. Acta Mech. 2018, 229, 2413–2430. [Google Scholar] [CrossRef]
- Keleshteri, M.M.; Asadi, H.; Wang, Q. On the snap-through instability of post-buckled FG-CNTRC rectangula plates with intergated piezoelectric layers. Comput. Method. Appl. Mech. Eng. 2018, 331, 53–71. [Google Scholar] [CrossRef]
- Malikan, M.; Dimitri, R.; Tornabene, F. Effect of sinusoidal corrugated geometries on the vibrational response of viscoelastic nanoplates. Appl. Sci. 2018, 8, 1432. [Google Scholar] [CrossRef]
- Jouneghani, F.Z.; Dimitri, R.; Tornabene, F. Structural response of porous FG nanobeams under hygro-thermo-mechanical loadings. Compos. Part B Eng. 2018, 152, 71–78. [Google Scholar] [CrossRef]
- Malikan, M.; Nguyen, V.B.; Tornabene, F. Damped forced vibration analysis of single-walled carbon nanotubes resting on viscoelastic foundation in thermal environment using nonlocal strain gradient theory. Eng. Sci. Technol. Int. J. 2018, 21, 778–786. [Google Scholar] [CrossRef]
- Jalaei, M.H.; Dimitri, R.; Tornabene, F. Dynamic stability of temperature-dependent graphene sheet embedded in an elastomeric medium. Appl. Sci. 2019, 9, 887. [Google Scholar] [CrossRef]
- Guzmán de Villoria, R.; Miravete, A. Mechanical model to evaluate the effect of the dispersion in nanocomposites. Acta Mater. 2007, 55, 3025–3031. [Google Scholar] [CrossRef]
- Shi, Z.; Zhang, T. Bending analysis of a piezoelectric curved actuator with a generally graded property for the piezoelectric parameter. Smart Mater. Struct. 2008, 17, 45018. [Google Scholar] [CrossRef]
- Arefi, M.; Rahimi, G.H. Application of shear deformation theory for two dimensional electro-elastic analysis of a FGP cylinder. Smart Struct. Syst. 2014, 13, 1–24. [Google Scholar] [CrossRef]
- Eringen, A.C. On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J Appl. Phys. 1983, 54, 4703. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Employing sinusoidal shear deformation plate theory for transient analysis of three layers sandwich nanoplate integrated with piezo-magnetic face-sheets. Smart Mater. Struct. 2016, 25, 115040. [Google Scholar] [CrossRef]
- Arefi, M.; Kiani, M.; Zenkour, A.M. Size-dependent free vibration analysis of a three-layered exponentially graded nano-/micro-plate with piezomagnetic face sheets resting on Pasternak’s foundation via MCST. J. Sandw. Struct. Mater. 2017, 1–32. [Google Scholar] [CrossRef]
- Apuzzo, A.; Barretta, R.; Faghidian, S.A.; Luciano, R.; Marotti de Sciarra, F. Nonlocal strain gradient exact solutions for functionally graded inflected nano-beams. Compos. Part B Eng. 2019, 164, 667–674. [Google Scholar] [CrossRef]
- Ganapathi, M.; Merzouki, T.; Polit, O. Vibration study of curved nanobeams based on nonlocal higher-order shear deformation theory using finite element approach. Compos. Struct. 2018, 184, 821–838. [Google Scholar] [CrossRef]





| Boundary Conditions | ||
|---|---|---|
| S-S | ||
| C-S | ||
| C-C |
| Ref. [17] | Present | Ref. [17] | Present | Ref. [17] | Present | Ref. [17] | Present | Ref. [17] | Present | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 10 | i = 1 | 4.56009 | 4.5739 | 4.35045 | 4.3630 | 4.1673 | 4.1788 | 4.00549 | 4.0161 | 3.86118 | 3.8710 |
| i = 2 | 23.7375 | 23.7852 | 20.0993 | 20.1213 | 17.7444 | 17.7547 | 16.0611 | 16.0652 | 14.7808 | 14.7813 | |
| i = 3 | 53.2817 | 53.3129 | 38.7745 | 38.7229 | 31.9762 | 31.9116 | 27.8325 | 27.7665 | 24.9704 | 24.9058 | |
| 20 | i = 1 | 4.66754 | 4.6710 | 4.45296 | 4.4563 | 4.2655 | 4.2687 | 4.09987 | 4.1029 | 3.95216 | 3.9551 |
| i = 2 | 25.0039 | 25.0155 | 21.1716 | 21.1794 | 18.6911 | 18.6969 | 16.9179 | 16.9226 | 15.5694 | 15.5734 | |
| i = 3 | 58.3285 | 58.3342 | 42.4472 | 42.4407 | 35.0050 | 34.9964 | 30.4689 | 30.4600 | 27.3356 | 27.3269 | |
| 50 | i = 1 | 4.72079 | 4.7215 | 4.50376 | 4.5045 | 4.31416 | 4.3150 | 4.14665 | 4.1475 | 3.99725 | 3.9981 |
| i = 2 | 25.5362 | 25.5382 | 21.6223 | 21.6251 | 19.0889 | 19.0919 | 17.2780 | 17.2810 | 15.9008 | 15.9037 | |
| i = 3 | 60.4005 | 60.4016 | 43.9551 | 43.9589 | 36.2484 | 36.2525 | 31.5512 | 31.5551 | 28.3067 | 28.3104 | |
| S-S Boundary Conditions | C-C Boundary Conditions | ||||
|---|---|---|---|---|---|
| Ref. [40] | Present | Ref. [40] | Present | ||
| 10 | 0 | 8.2006 | 8.2141 | 25.8678 | 26.0937 |
| 1 | 7.8236 | 7.8366 | 24.3792 | 24.4961 | |
| 2 | 7.4942 | 7.5057 | 23.1083 | 23.1601 | |
| 3 | 7.2032 | 7.2134 | 22.0084 | 22.0212 | |
| 4 | 6.9437 | 6.9527 | 21.0456 | 21.0353 | |
| 20 | 0 | 8.2922 | 8.2955 | 37.0381 | 37.0333 |
| 1 | 7.9110 | 7.9138 | 34.7887 | 34.7932 | |
| 2 | 7.5779 | 7.5803 | 32.8611 | 32.9158 | |
| 3 | 7.2837 | 7.2858 | 31.1899 | 31.3129 | |
| 4 | 7.0213 | 7.0231 | 29.7263 | 29.9234 | |
| Boundary Condition | Boundary Condition | |||||
|---|---|---|---|---|---|---|
| S-S | C-S | C-C | S-S | C-S | C-C | |
| 0.0 | 2.2360 | 6.9765 | 8.9273 | 2.3185 | 7.3241 | 9.3883 |
| 0.5 | 2.2358 | 6.9751 | 8.9258 | 2.3182 | 7.3227 | 9.3867 |
| 1.0 | 2.2349 | 6.9709 | 8.9214 | 2.3173 | 7.3183 | 9.3821 |
| 1.5 | 2.2336 | 6.9640 | 8.9140 | 2.3159 | 7.3111 | 9.3743 |
| 2.0 | 2.2316 | 6.9544 | 8.9037 | 2.3139 | 7.3010 | 9.3634 |
| 2.5 | 2.2292 | 6.9421 | 8.8905 | 2.3113 | 7.2880 | 9.3495 |
| 3.0 | 2.2262 | 6.9271 | 8.8744 | 2.3082 | 7.2723 | 9.3326 |
| 3.5 | 2.2226 | 6.9096 | 8.8555 | 2.3046 | 7.2539 | 9.3128 |
| 4.0 | 2.2186 | 6.8895 | 8.8339 | 2.3004 | 7.2328 | 9.2900 |
| Pattern of Multilayer | Boundary Condition | |||
|---|---|---|---|---|
| S-S | C-S | C-C | ||
| Pattern 1 | 2.5058 | 5.7430 | 6.9190 | |
| Pattern 2 | 2.3910 | 5.5456 | 6.7041 | |
| Pattern 3 | 2.6163 | 5.9357 | 7.1293 | |
| Pattern 4 | 2.4923 | 5.7200 | 6.8935 | |
| Pattern 1 | 2.4283 | 7.4648 | 9.5336 | |
| Pattern 2 | 2.3173 | 7.3183 | 9.3821 | |
| Pattern 3 | 2.5356 | 7.6117 | 9.6868 | |
| Pattern 4 | 2.4174 | 7.4543 | 9.5225 | |
| 3 | 3.5 | 4 | 4.5 | 5 | ||
|---|---|---|---|---|---|---|
| 10 | m = 1 | 53.6075 | 54.0087 | 54.3011 | 54.5226 | 54.6958 |
| m = 2 | 100.9296 | 102.9847 | 104.6266 | 105.9049 | 106.8881 | |
| m = 3 | 139.5596 | 142.0667 | 144.4766 | 146.7824 | 148.9747 | |
| 11 | m = 1 | 53.7027 | 54.0769 | 54.3521 | 54.5621 | 54.7271 |
| m = 2 | 102.6704 | 104.3787 | 105.7134 | 106.7410 | 107.5308 | |
| m = 3 | 144.1770 | 146.4964 | 148.7037 | 150.7876 | 152.7349 | |
| 12 | m = 1 | 53.7911 | 54.1410 | 54.4004 | 54.5996 | 54.7571 |
| m = 2 | 104.1203 | 105.5133 | 106.5874 | 107.4125 | 108.0505 | |
| m = 3 | 148.4306 | 150.5309 | 152.4963 | 154.3126 | 155.9658 | |
| 13 | m = 1 | 53.8735 | 54.2012 | 54.4461 | 54.6354 | 54.7858 |
| m = 2 | 105.3043 | 106.4268 | 107.2889 | 107.9545 | 108.4744 | |
| m = 3 | 152.2553 | 154.0912 | 155.7658 | 157.2676 | 158.5904 | |
| 14 | m = 1 | 53.9504 | 54.2578 | 54.4894 | 54.6695 | 54.8133 |
| m = 2 | 106.2589 | 107.1599 | 107.8544 | 108.3958 | 108.8240 | |
| m = 3 | 155.5631 | 157.0872 | 158.4328 | 159.6003 | 160.5981 | |
| 15 | m = 1 | 54.0224 | 54.3113 | 54.5305 | 54.7020 | 54.8397 |
| m = 2 | 107.0251 | 107.7501 | 108.3140 | 108.7588 | 109.1154 | |
| m = 3 | 158.2723 | 159.4621 | 160.4807 | 161.3424 | 162.0661 | |
| 0 | 1 | 2 | 3 | 4 | 5 | ||
|---|---|---|---|---|---|---|---|
| m = 1 | 2.6176 | 2.6163 | 2.6125 | 2.6061 | 2.5972 | 2.5859 | |
| m = 2 | 10.4115 | 10.3910 | 10.3302 | 10.2313 | 10.0974 | 9.9329 | |
| m = 3 | 22.9623 | 22.8610 | 22.5649 | 22.0960 | 21.4861 | 20.7716 | |
| m = 1 | 2.5369 | 2.5356 | 2.5319 | 2.5257 | 2.5171 | 2.5061 | |
| m = 2 | 10.3316 | 10.3112 | 10.2509 | 10.1527 | 10.0199 | 9.8564 | |
| m = 3 | 22.8853 | 22.7843 | 22.4891 | 22.0217 | 21.4138 | 20.7012 | |
| m = 1 | 2.4064 | 2.4053 | 2.4017 | 2.3958 | 2.3877 | 2.3773 | |
| m = 2 | 10.1994 | 10.1793 | 10.1197 | 10.0228 | 9.8916 | 9.7302 | |
| m = 3 | 22.7573 | 22.6568 | 22.3632 | 21.8983 | 21.2936 | 20.5849 | |
| m = 1 | 2.2318 | 2.2307 | 2.2274 | 2.2219 | 2.2143 | 2.2047 | |
| m = 2 | 10.0164 | 9.9967 | 9.9382 | 9.8429 | 9.7140 | 9.5555 | |
| m = 3 | 22.5790 | 22.4793 | 22.1878 | 21.7264 | 21.1261 | 20.4227 | |
| m = 1 | 2.0194 | 2.0184 | 2.0155 | 2.0105 | 2.0037 | 1.9949 | |
| m = 2 | 9.7847 | 9.7654 | 9.7082 | 9.6151 | 9.4891 | 9.3342 | |
| m = 3 | 22.3512 | 22.2524 | 21.9638 | 21.5067 | 20.9122 | 20.2157 | |
| m = 1 | 1.7765 | 1.7756 | 1.7730 | 1.7686 | 1.7626 | 1.7549 | |
| m = 2 | 9.5067 | 9.4879 | 9.4323 | 9.3418 | 9.2193 | 9.0687 | |
| m = 3 | 22.0750 | 21.9774 | 21.6921 | 21.2404 | 20.6529 | 19.9646 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arefi, M.; Bidgoli, E.M.-R.; Dimitri, R.; Tornabene, F.; Reddy, J.N. Size-Dependent Free Vibrations of FG Polymer Composite Curved Nanobeams Reinforced with Graphene Nanoplatelets Resting on Pasternak Foundations. Appl. Sci. 2019, 9, 1580. https://doi.org/10.3390/app9081580
Arefi M, Bidgoli EM-R, Dimitri R, Tornabene F, Reddy JN. Size-Dependent Free Vibrations of FG Polymer Composite Curved Nanobeams Reinforced with Graphene Nanoplatelets Resting on Pasternak Foundations. Applied Sciences. 2019; 9(8):1580. https://doi.org/10.3390/app9081580
Chicago/Turabian StyleArefi, Mohammad, Elyas Mohammad-Rezaei Bidgoli, Rossana Dimitri, Francesco Tornabene, and J. N. Reddy. 2019. "Size-Dependent Free Vibrations of FG Polymer Composite Curved Nanobeams Reinforced with Graphene Nanoplatelets Resting on Pasternak Foundations" Applied Sciences 9, no. 8: 1580. https://doi.org/10.3390/app9081580
APA StyleArefi, M., Bidgoli, E. M.-R., Dimitri, R., Tornabene, F., & Reddy, J. N. (2019). Size-Dependent Free Vibrations of FG Polymer Composite Curved Nanobeams Reinforced with Graphene Nanoplatelets Resting on Pasternak Foundations. Applied Sciences, 9(8), 1580. https://doi.org/10.3390/app9081580