Multi-Wavelength Digital-Phase-Shifting Moiré Based on Moiré Wavelength
Abstract
:Featured Application
Abstract
1. Introduction
2. Method
2.1. Digital Phase-Shifting Moiré
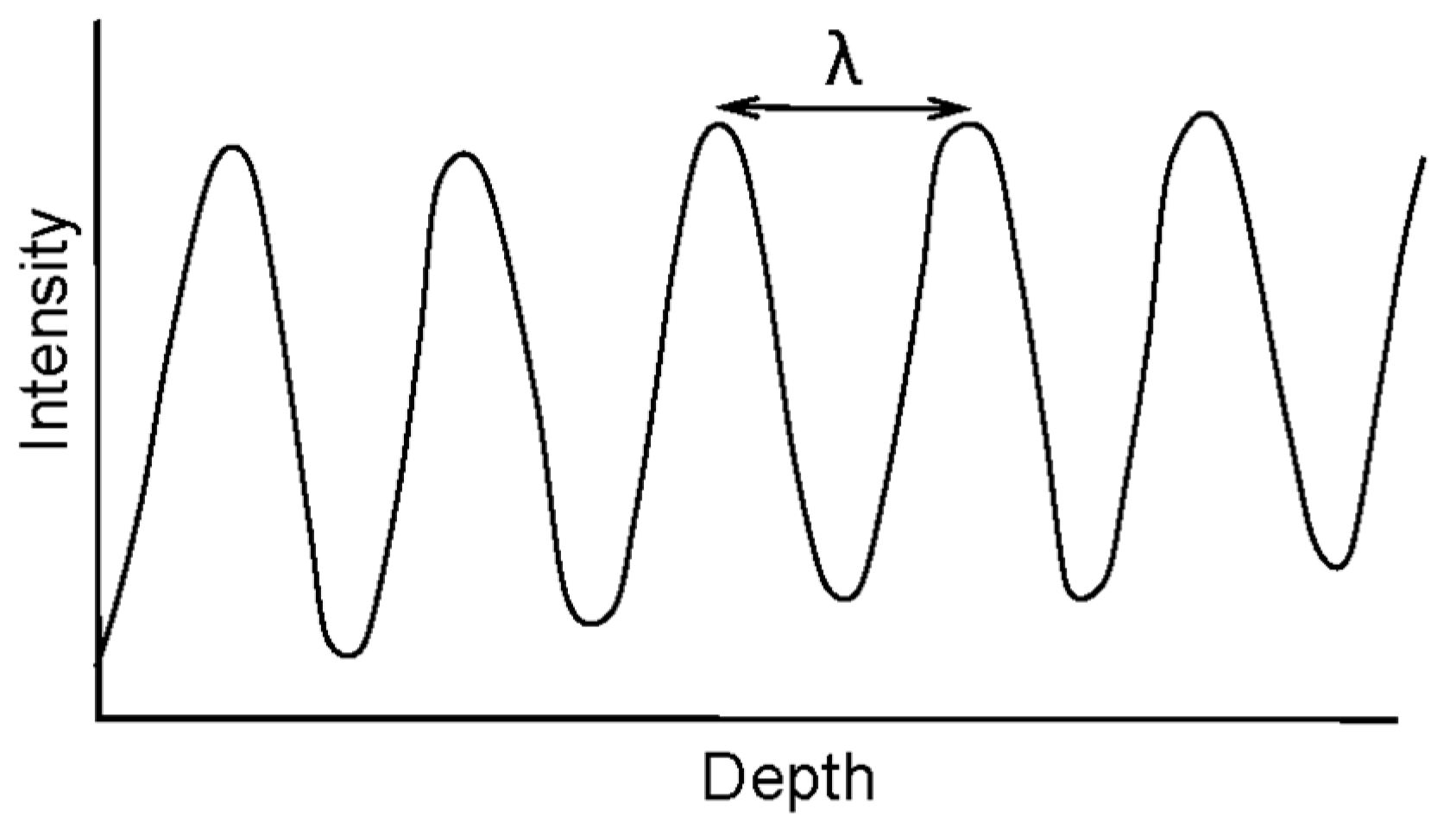
2.2. Computation of Moiré Wavelength in Moiré System Calibration
2.3. Digital-Moiré Temporal Phase Unwrapping
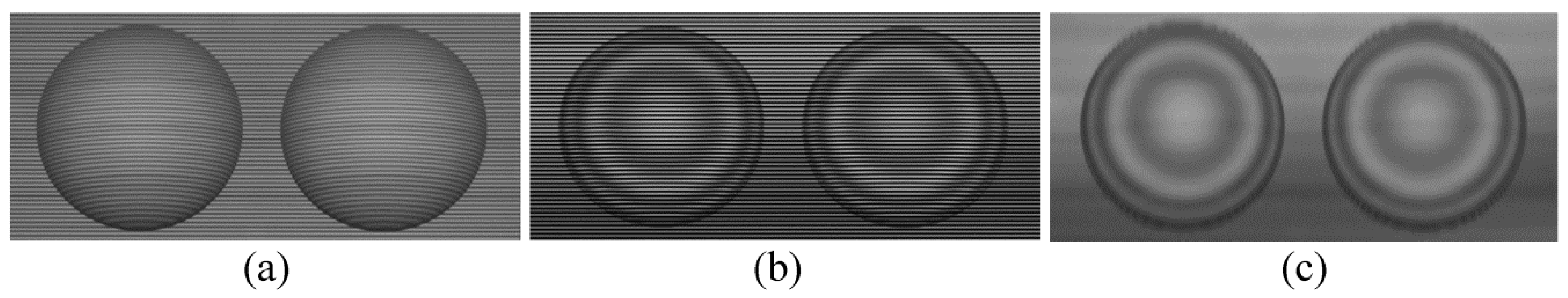
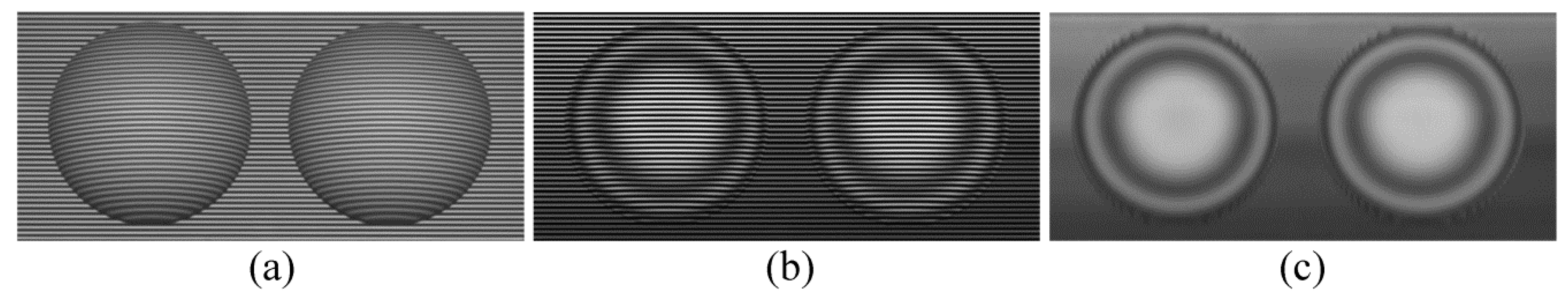
2.3.1. Two-Wavelength Phase-Unwrapping
2.3.2. Three-Wavelength Phase-Unwrapping
2.4. 3D Surface Reconstruction by Moiré-Wavelength and Height Refinement
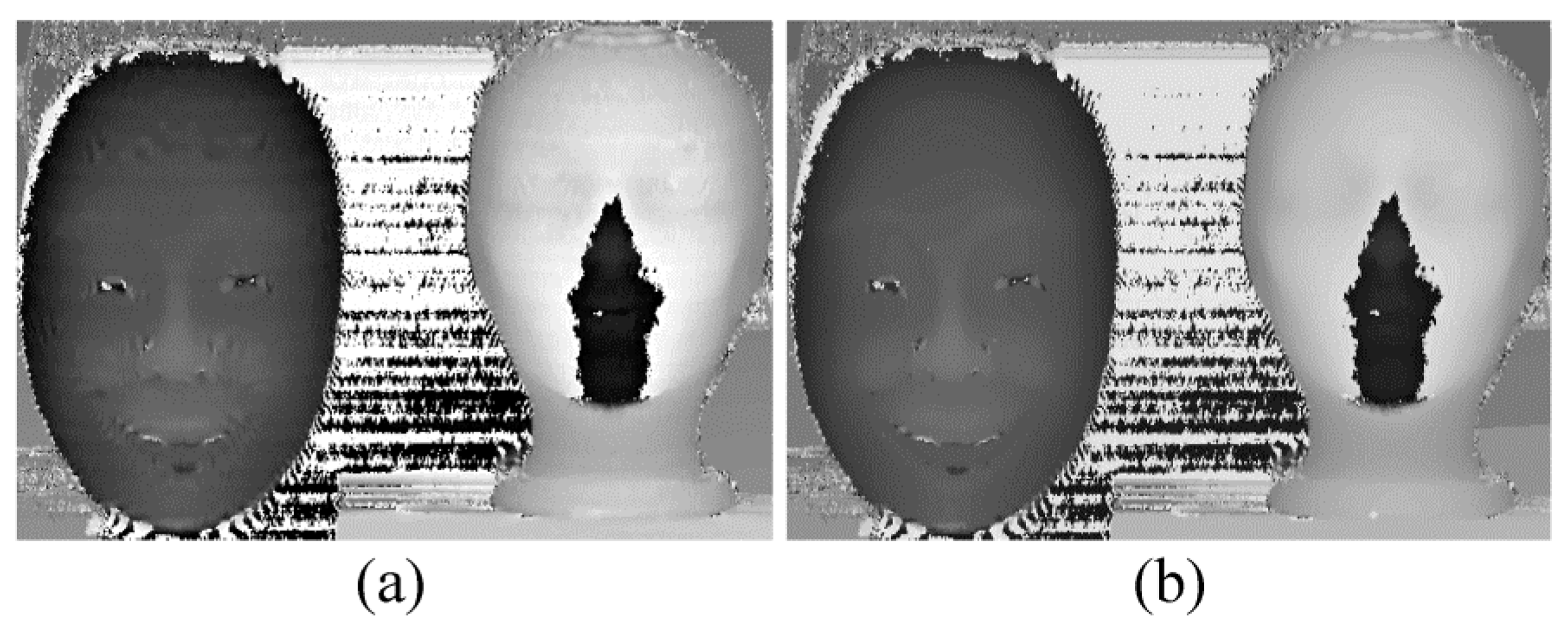
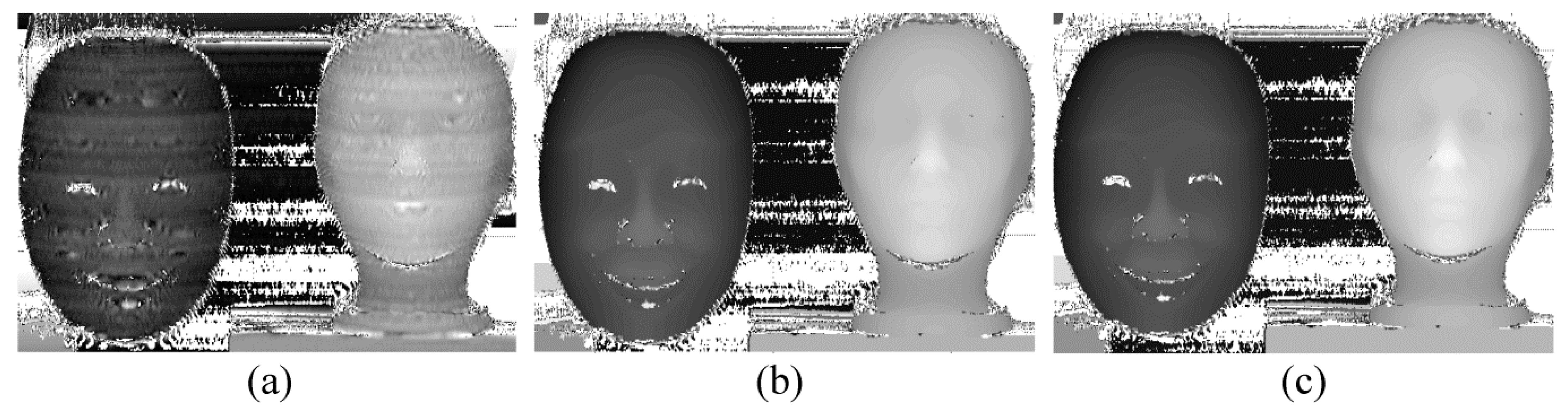
3. Experiments and Results
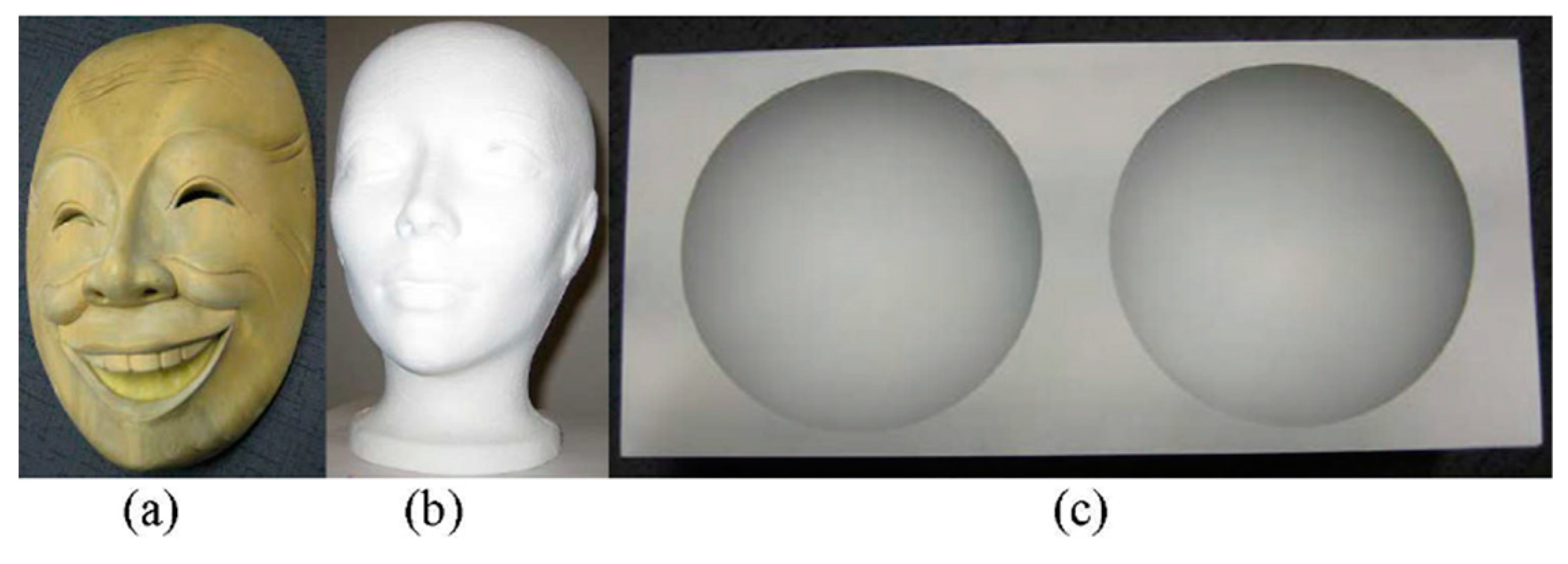
3.1. Experimental Setup
3.2. System Calibration
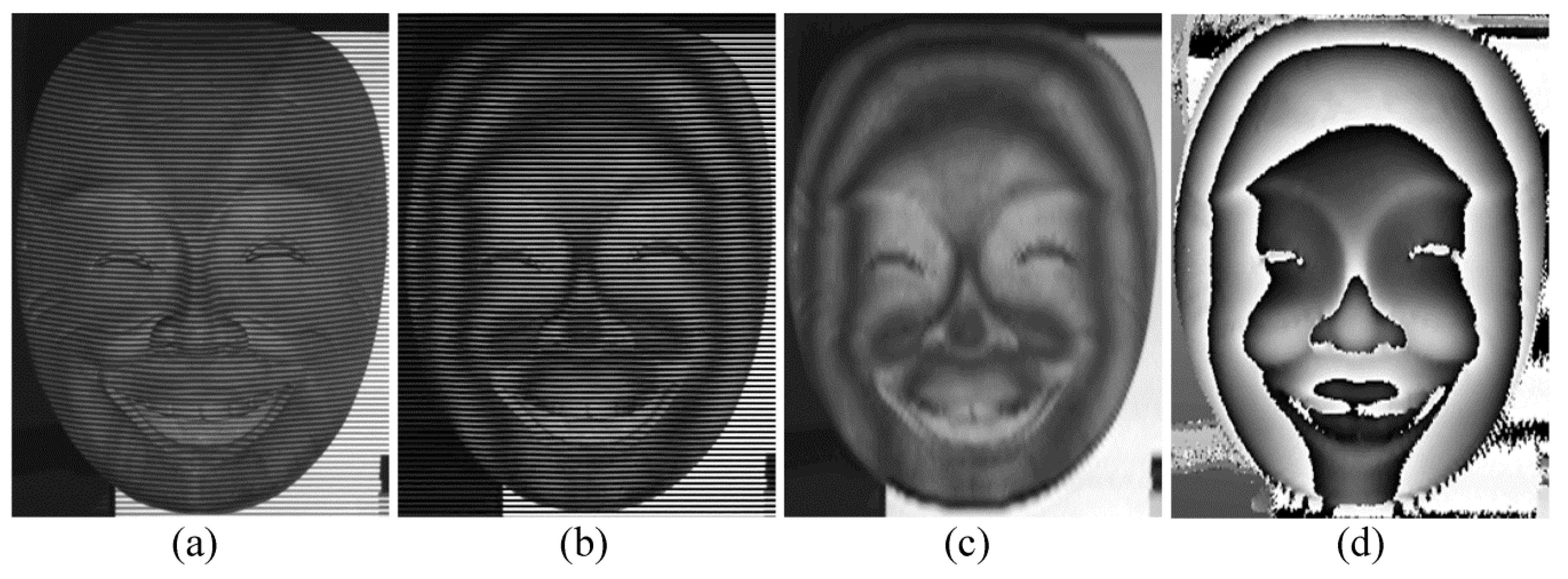
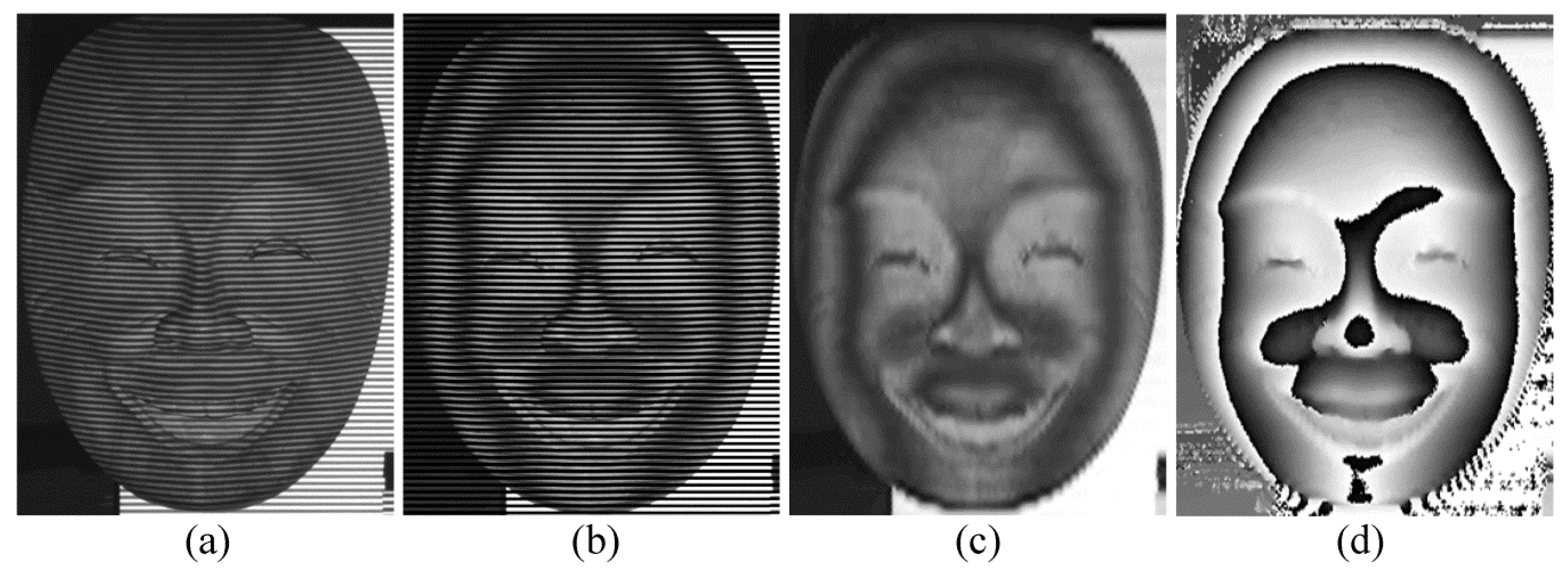
3.3. Measurement
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Gorthi, S.S.; Rastogi, P. Fringe projection techniques: Whither we are? Opt. Lasers Eng. 2010, 48, 133–140. [Google Scholar] [CrossRef] [Green Version]
- Hu, Q.; Harding, K.G. Conversion from phase map to coordinate: Comparison among spatial carrier, Fourier transform, and phase shifting methods. Opt. Lasers Eng. 2007, 45, 342–348. [Google Scholar] [CrossRef]
- Song, L.; Chang, Y.; Xi, J.; Guo, Q.; Zhu, X.; Li, X. Phase unwrapping method based on multiple fringe patterns without use of equivalent wavelengths. Opt. Commun. 2015, 355, 213–224. [Google Scholar] [CrossRef] [Green Version]
- Zuo, C.; Huang, L.; Zhang, M.; Chen, Q.; Asundi, A. Temporal phase unwrapping algorithms for fringe projection profilometry: A comparative review. Opt. Lasers Eng. 2016, 85, 84–103. [Google Scholar] [CrossRef]
- Goldstein, R.M.; Zebker, H.A.; Werner, C.L. Satellite radar interferometry: Two-dimensional phase unwrapping. Radio Sci. 1988, 23, 713–720. [Google Scholar] [CrossRef]
- Zappa, E.; Busca, G. Comparison of eight unwrapping algorithms applied to Fourier-transform profilometry. Opt. Lasers Eng. 2008, 46, 106–116. [Google Scholar] [CrossRef]
- Creath, K. Step height measurement using two-wavelength phase-shifting interferometry. Appl. Opt. 1987, 26, 2810–2816. [Google Scholar] [CrossRef] [PubMed]
- Saldner, H.O.; Huntley, J.M. Temporal phase unwrapping: Application to surface profiling of discontinuous objects. Appl. Opt. 1997, 36, 2770–2775. [Google Scholar] [CrossRef] [PubMed]
- Reich, C.; Ritter, R.; Thesing, J. White light heterodyne principle for 3D-measurement. In Proceedings of the SPIE 3100, Sensors, Sensor Systems, and Sensor Data Processing, Lasers and Optics in Manufacturing III, Munich, Germany, 16–20 June 1997; pp. 236–244. [Google Scholar]
- Cheng, Y.Y.; Wyant, J.C. Two-wavelength phase shifting interferometry. Appl. Opt. 1984, 23, 4539–4543. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Chen, W.; Tan, Y. Phase-unwrapping algorithm for the measurement of three-dimensional object shapes. Appl. Opt. 1994, 33, 4497–4500. [Google Scholar]
- Cheng, Y.Y.; Wyant, J.C. Multiple-wavelength phase-shifting interferometry. Appl. Opt. 1985, 24, 804–807. [Google Scholar] [CrossRef] [PubMed]
- Song, L.; Dong, X.; Xi, J.; Yu, Y.; Yang, C. A new phase unwrapping algorithm based on three wavelength phase shift profilometry method. Opt. Lasers Technol. 2013, 45, 319–329. [Google Scholar] [CrossRef]
- Geng, L.; Liu, Y.; Xiao, Z.; Wu, J.; Zhang, Y.; Yuan, F.; Yang, Z.; Gan, P.; Su, J.; Ye, K. Phase unwrapping method based on heterodyne three frequency non-equal step phase shift. In Proceedings of the International Conference on Image and Graphics 2015, Part III, Lecture Notes in Computer Science 9219, Tianjin, China, 13–16 August 2015; pp. 68–79. [Google Scholar]
- Towers, C.E.; Towers, D.P.; Jones, J.D.C. Optimum frequency selection in multi-frequency interferometry. Opt. Lett. 2003, 28, 887–889. [Google Scholar] [CrossRef] [PubMed]
- Towers, C.E.; Towers, D.P.; Jones, J.D.C. Absolute fringe order calculation using optimised multi- frequency selection in full-field profilometry. Opt. Lasers Eng. 2005, 43, 788–800. [Google Scholar] [CrossRef]
- Mohammadi, F.; Kofman, J. Single-frame digital phase-shifting 3D shape measurement using pixel-wise moiré-wavelength refinement. Opt. Lasers Eng. 2016, 78, 196–204. [Google Scholar] [CrossRef]
- Ryu, W.J.; Kang, Y.J.; Baik, S.H.; Kang, S.J. A study on the 3-D measurement by using digital projection moiré method. Optik 2008, 119, 453–458. [Google Scholar] [CrossRef]
- Mohammadi, F.; Kofman, J. Improved grid-noise removal in single-frame digital moiré 3D shape measurement. Opt. Lasers Eng. 2016, 86, 143–155. [Google Scholar] [CrossRef]
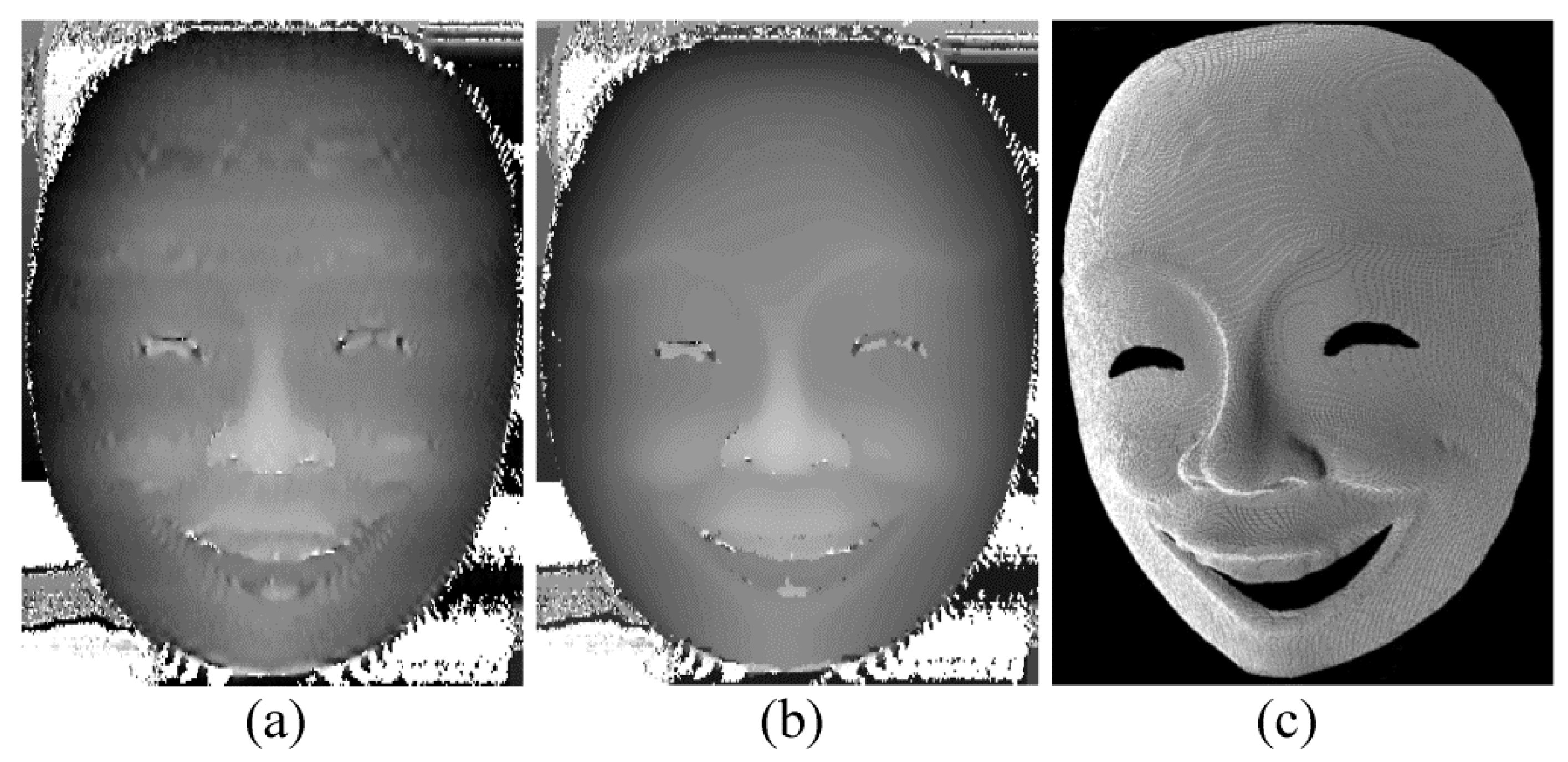
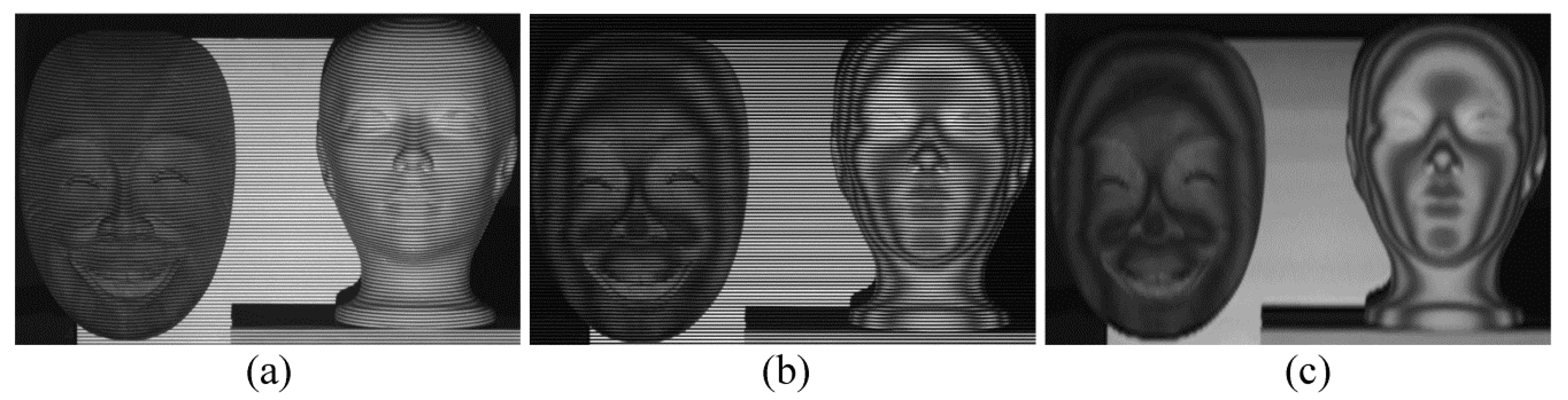
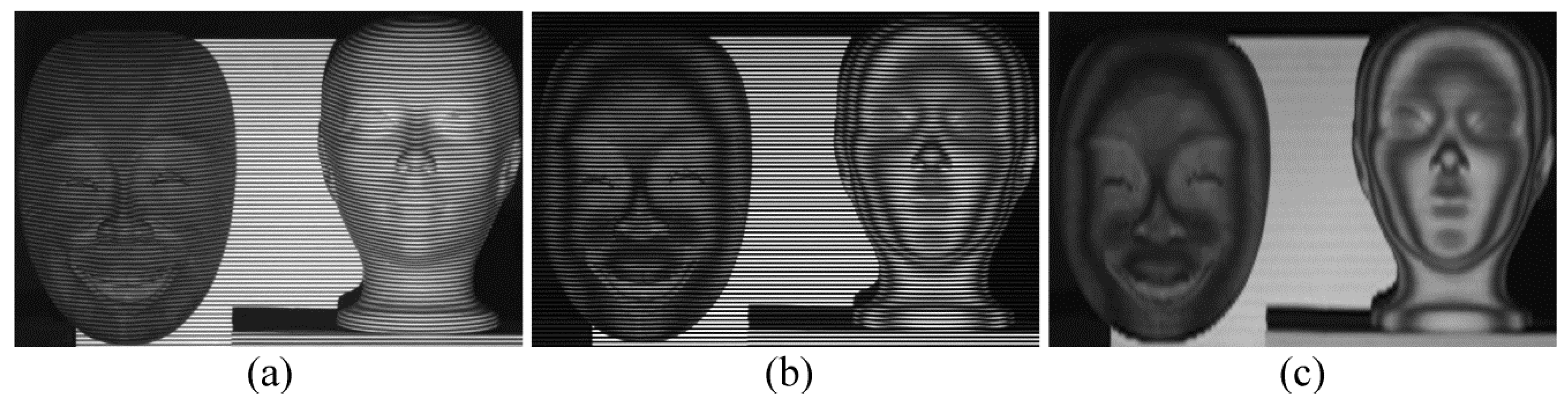
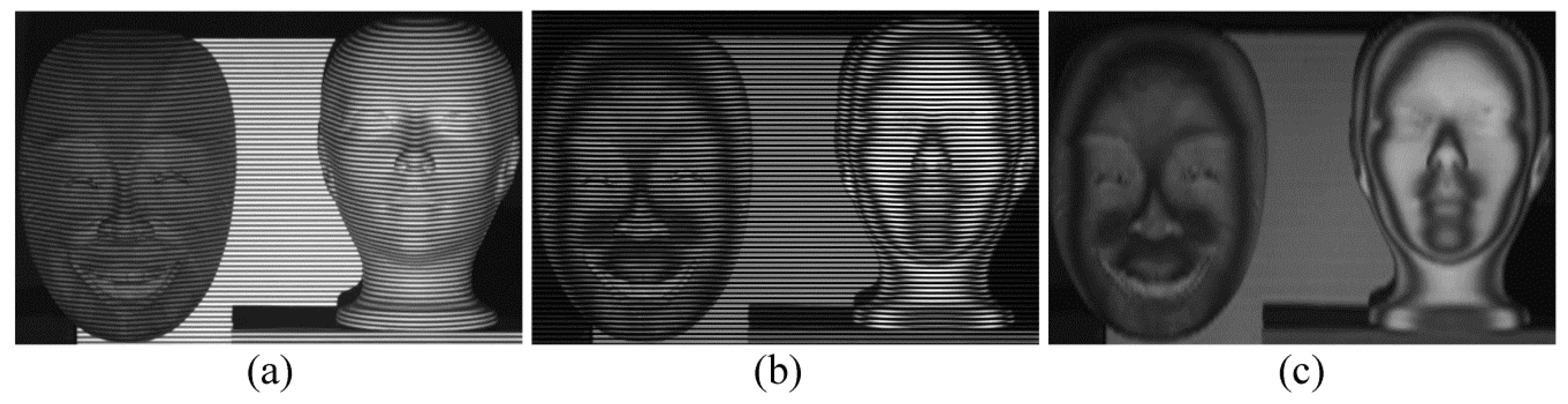






| Grid Pitch (pixels) | Max (mm) | ||
|---|---|---|---|
| 8 | 15.88 | 19.08 | 16.966 |
| 10 | 19.62 | 24.09 | 21.850 21.035 |
| 12 | 23.78 | 28.31 | 26.048 |
| 14 | 27.93 | 33.22 | 30.573 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammadi, F.; Kofman, J. Multi-Wavelength Digital-Phase-Shifting Moiré Based on Moiré Wavelength. Appl. Sci. 2019, 9, 1917. https://doi.org/10.3390/app9091917
Mohammadi F, Kofman J. Multi-Wavelength Digital-Phase-Shifting Moiré Based on Moiré Wavelength. Applied Sciences. 2019; 9(9):1917. https://doi.org/10.3390/app9091917
Chicago/Turabian StyleMohammadi, Fatemeh, and Jonathan Kofman. 2019. "Multi-Wavelength Digital-Phase-Shifting Moiré Based on Moiré Wavelength" Applied Sciences 9, no. 9: 1917. https://doi.org/10.3390/app9091917
APA StyleMohammadi, F., & Kofman, J. (2019). Multi-Wavelength Digital-Phase-Shifting Moiré Based on Moiré Wavelength. Applied Sciences, 9(9), 1917. https://doi.org/10.3390/app9091917




