Performance Comparison of Polymeric and Silica-Based Multi-Bed Pervaporation Membrane Reactors during Ethyl Levulinate Production
Abstract
:1. Introduction
2. Developing the CFD Model
- Isothermal condition;
- Steady state;
- Negligible film transport resistance at the interface of feed/membrane;
- Constant performance of membrane and catalyst without any deactivation or concentration polarization.
2.1. Governing Physical Equations
2.2. Chemical Kinetic Reactions
2.3. Chemical–Physical Properties
2.4. Boundary Conditions and Post-Processing Definitions
2.5. Numerical Method
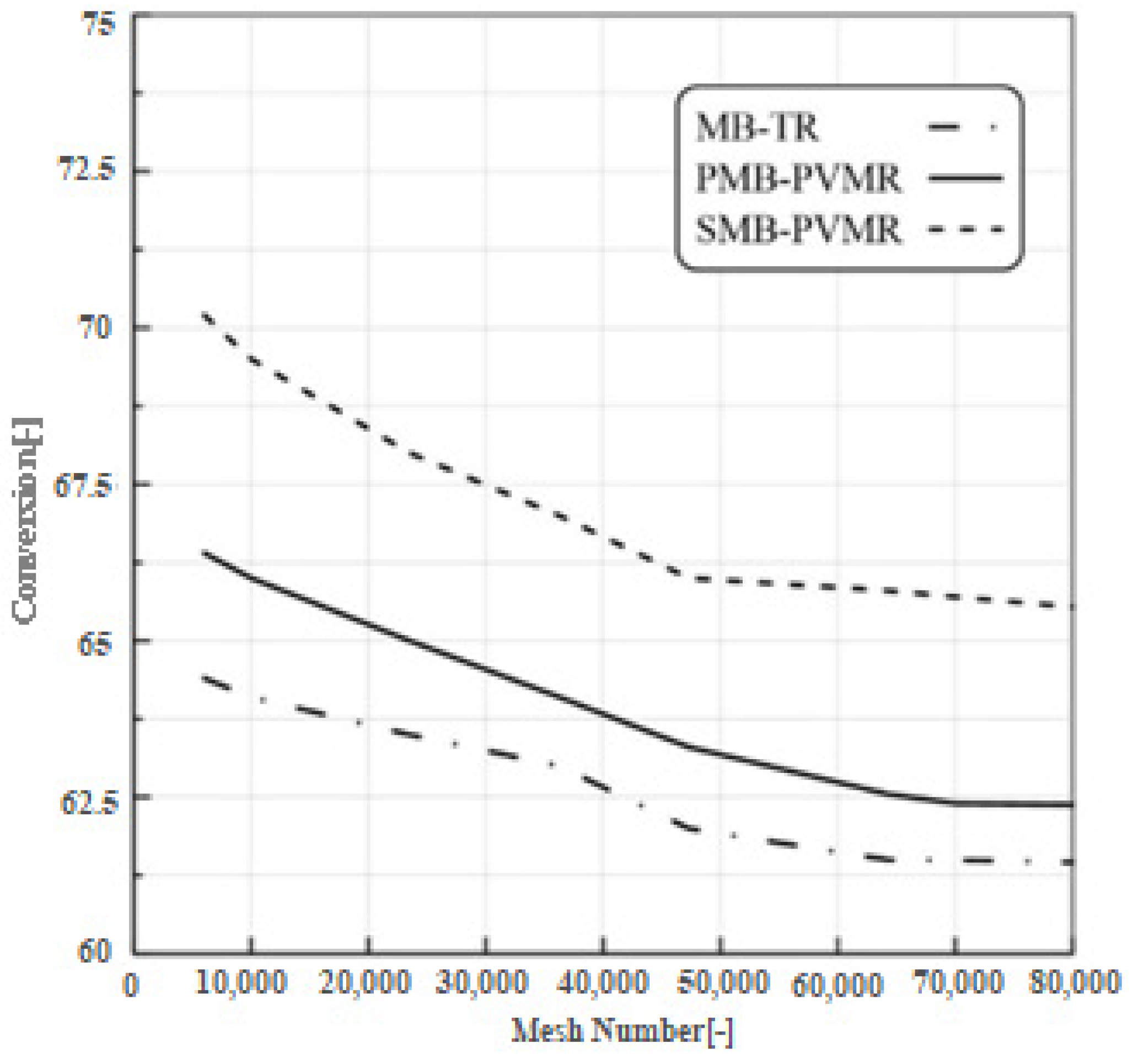
2.6. Mesh Independency
3. Results and Discussion
3.1. Model Validation
3.2. Evaluation of the Operating Parameter Effects
3.3. LA Conversion versus Different Operating Conditions
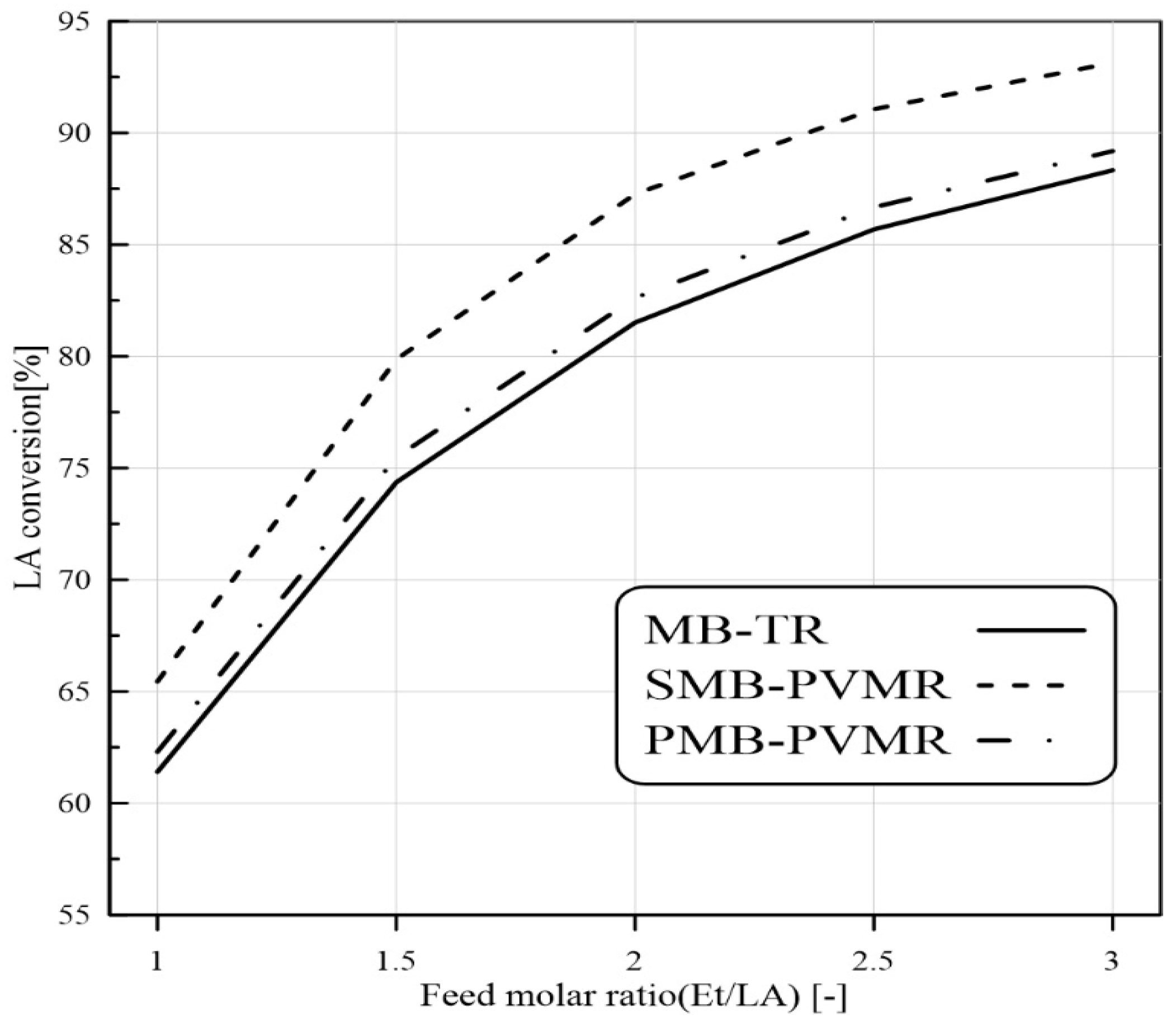
3.3.1. Feed Molar Ratio Effect
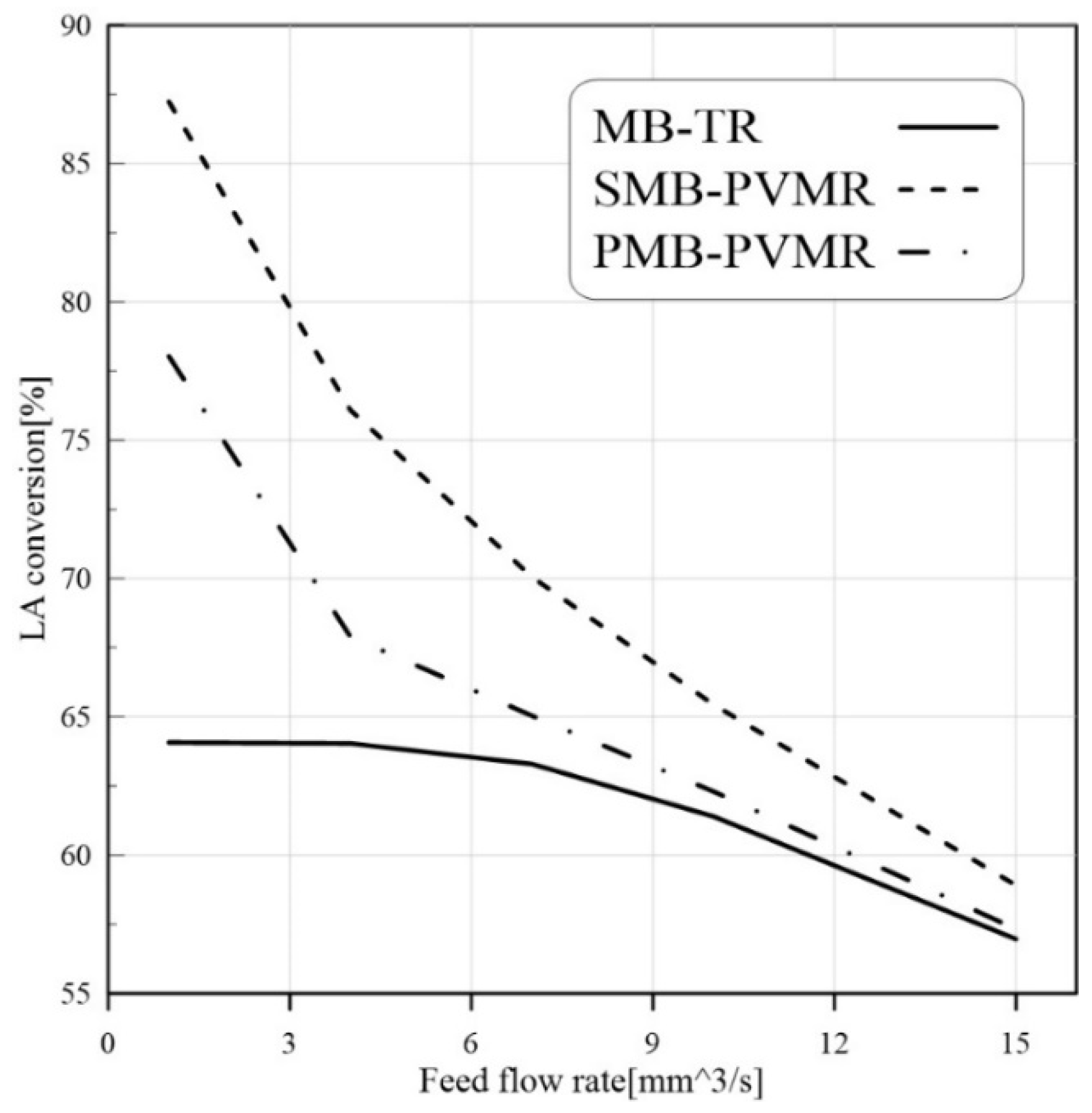
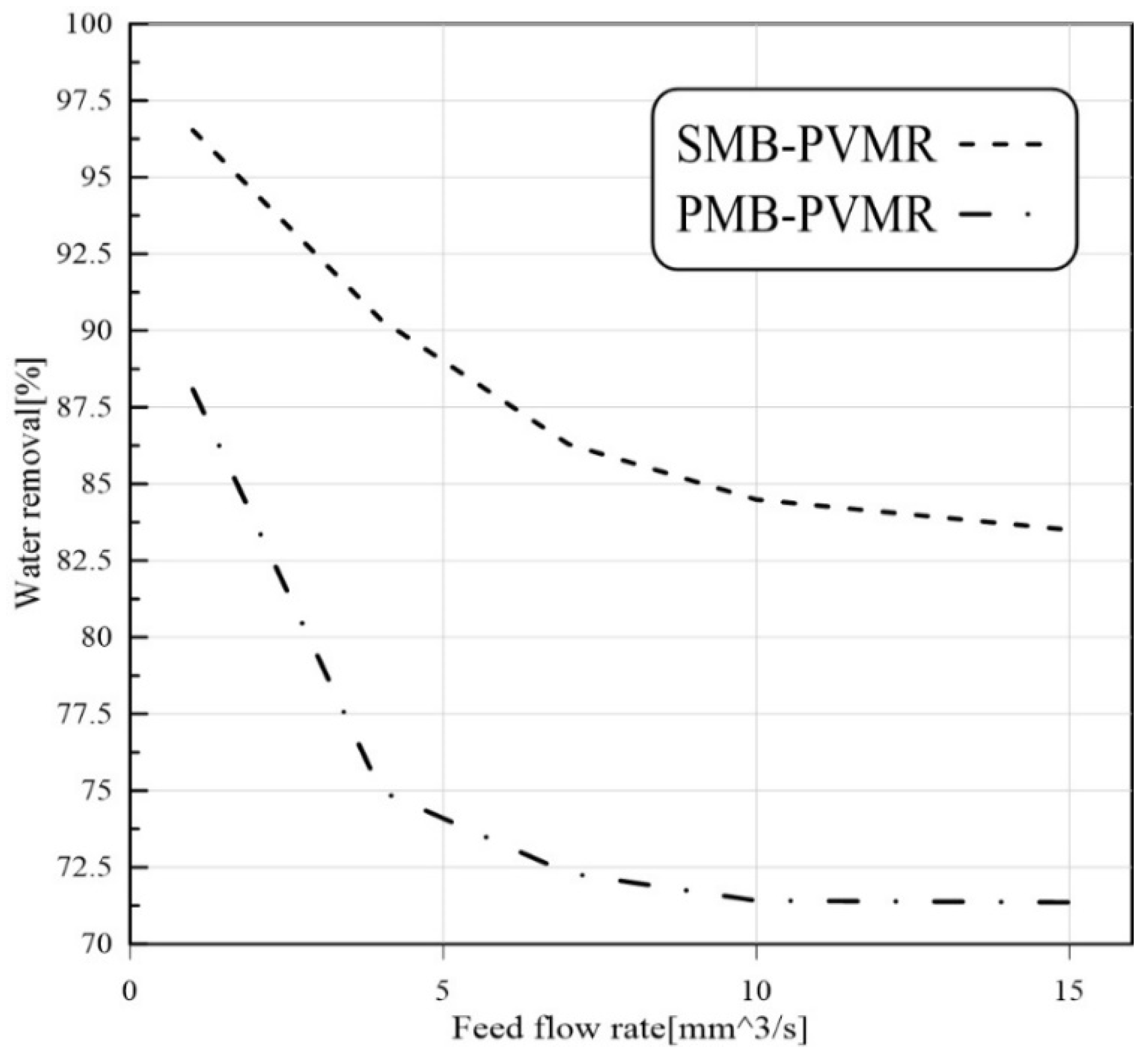
3.3.2. Feed Flow Rate Effect
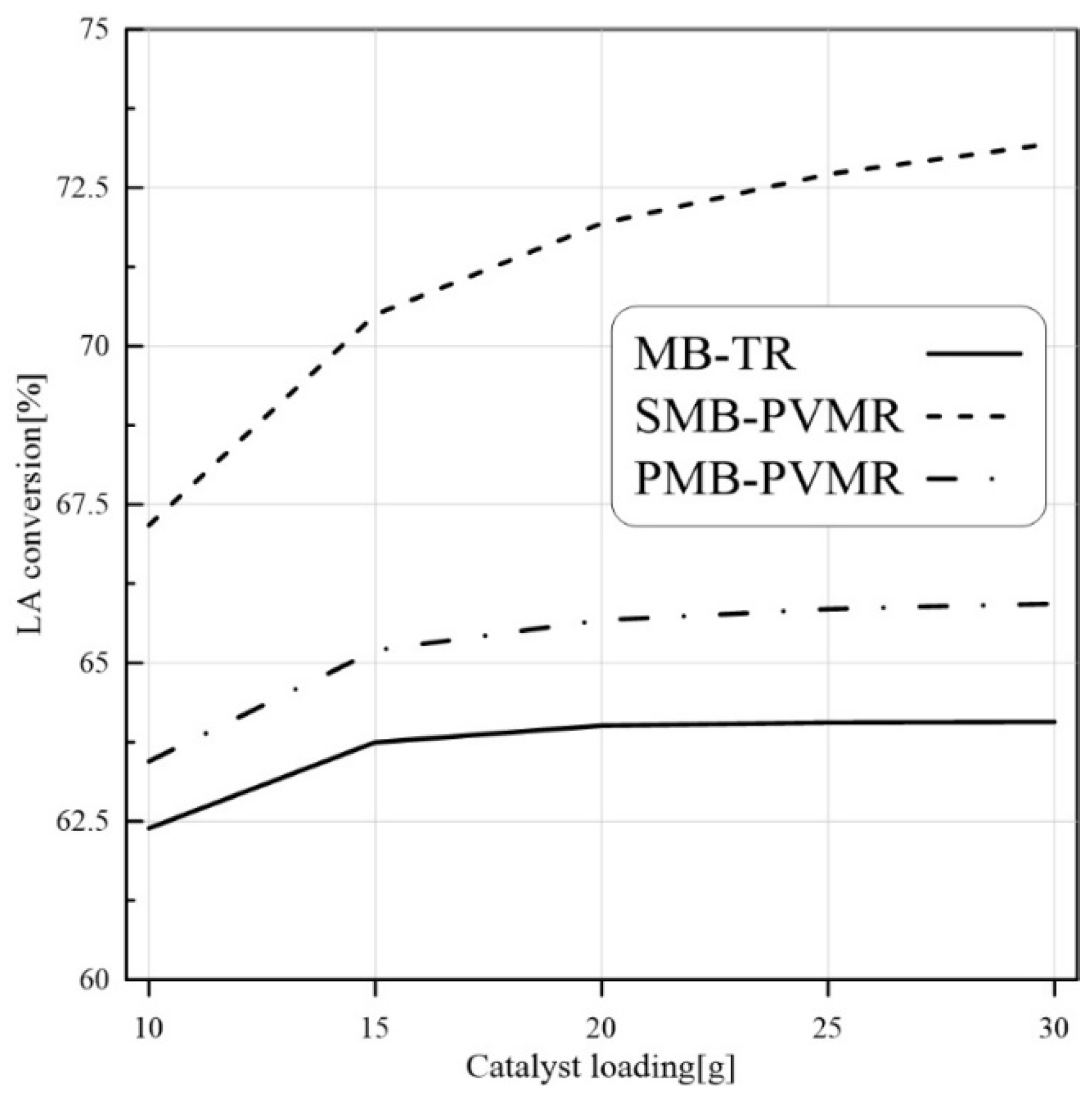
3.3.3. Catalyst Loading Effect
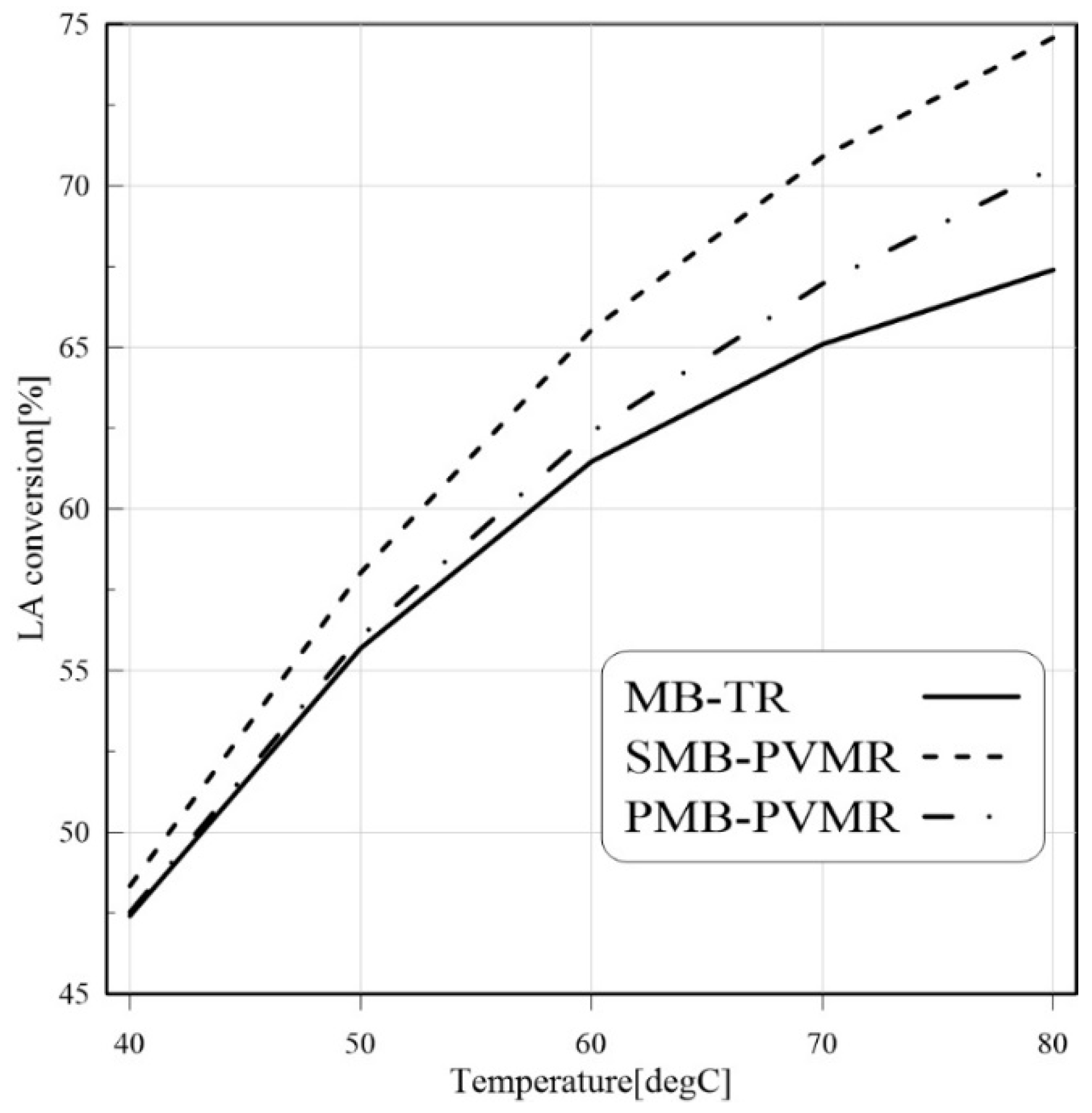
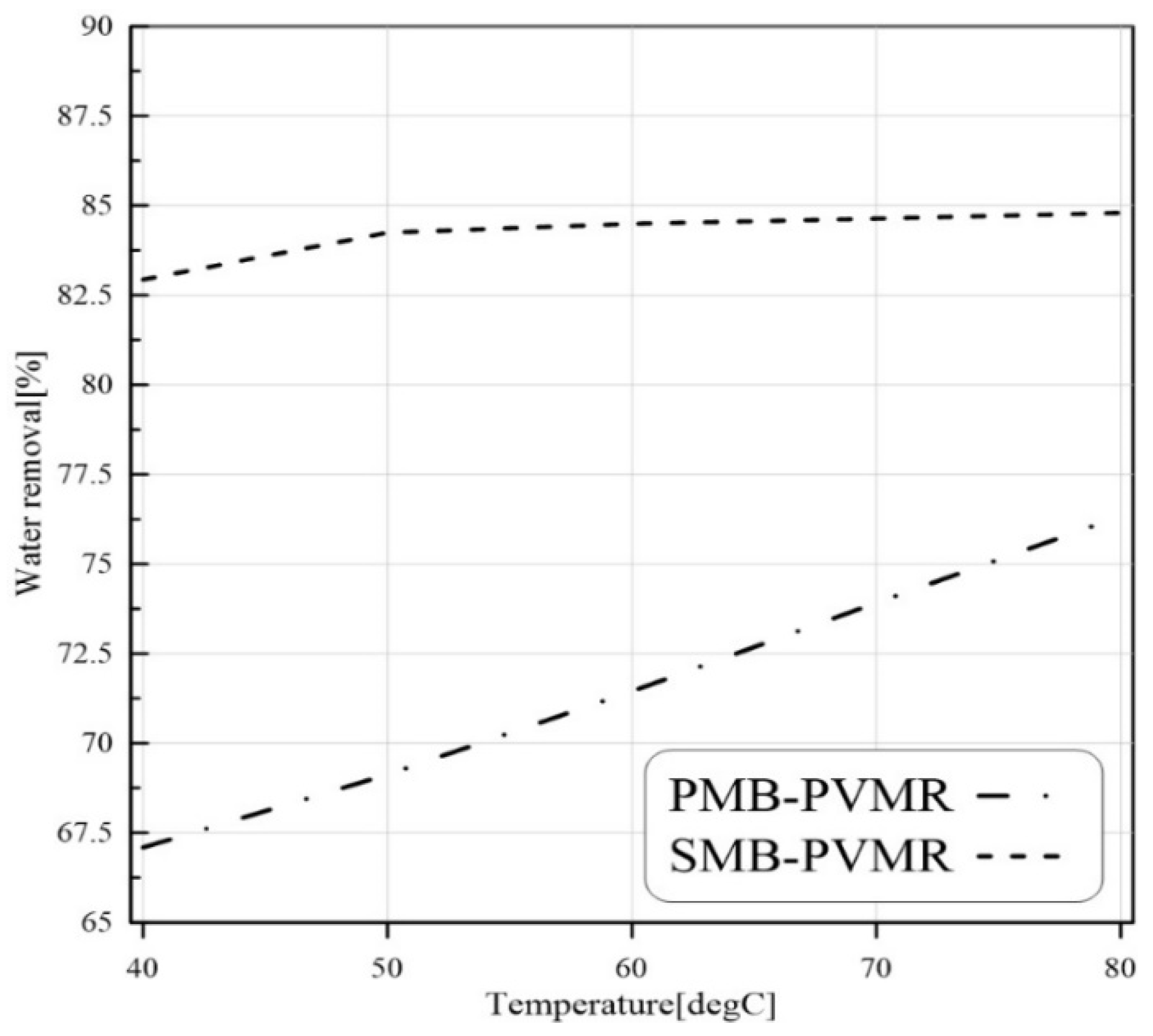
3.3.4. Temperature Effect
3.4. Water Removal at Different Operation Condition
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sadati Tilebon, S.M.; Ghasemzadeh, K.; Jafarharasi, N.; Vosoughi, P. An Overview on the Bioethanol Production using Membrane Technologies. Int. J. Membr. Sci. Technol. 2014, 1, 9–22. [Google Scholar]
- Ghasemzadeh, K.; Basile, A.; Sadati Tilebon, S.M. Membranes for IGCC power plants. In Integrated Membrane Systems and Processes; Basile, A., Charcosset, C., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 2016; pp. 256–283. [Google Scholar]
- Ghasemzadeh, K.; Sadati Tilebon, S.M.; Basile, A. Coproduction of electrical energy and methanol in IGCC Plants. In Methanol: Science and Engineering; Basile, A., Dalena, F., Eds.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 399–428. [Google Scholar]
- Ghasemzadeh, K.; Sadati Tilebon, S.M.; Basile, A. Technoeconomic Assessment of Polymeric, Ceramic, and Metallic Membrane Integration in an Advanced IGCC Process for CO2 Separation and Capture. In Current Trends and Future Developments on (Bio-) Membranes: Carbon Dioxide Separation/Capture by Using Membranes; Basile, A., Favvas, E.P., Eds.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 511–549. [Google Scholar]
- Gruber, P.R.; Kamm, M.; Kamm, B. Biorefineries: Industrial Processes and Products: Status Quo and Future Directions; Wiley-VCH: Hoboken, NJ, USA, 2006. [Google Scholar]
- Chang, C.; Xu, G.; Jiang, X. Production of ethyl levulinate by direct conversion of wheat straw in ethanol media. Bioresour. Technol. 2012, 121, 93–99. [Google Scholar] [CrossRef] [PubMed]
- Delgado, P.; Sanz, M.T.; Beltrán, S.; Núñez, L.A. Ethyl lactate production via esterification of lactic acid with ethanol combined with pervaporation. Chem. Eng. J. 2010, 165, 693–700. [Google Scholar] [CrossRef]
- Pereira, C.S.M.; Silva, V.M.T.M.; Pinho, S.P.; Rodrigues, A.E. Batch and continuous studies for ethyl lactate synthesis in a pervaporation membrane reactor. J. Memb. Sci. 2010, 361, 43–55. [Google Scholar] [CrossRef]
- Russo, V.; Hrobar, V.; Mäki-Arvela, P.; Eränen, K.; Sandelin, F.; Di Serio, M.; Salmi, T. Kinetics and modelling of levulinic acid esterification in batch and continuous reactors. Top Catal. 2018, 61, 1856–1865. [Google Scholar] [CrossRef]
- Ghasemzadeh, K.; Sadati Tilebon, S.M.; Basile, A. Modeling of Silica Membranes. In Current Trends and Future Developments on (Bio-) Membranes Silica Membranes: Preparation, Modelling, Application, and Commercialization; Basile, A., Ghasemzadeh, K., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 135–153. [Google Scholar]
- Ghasemzadeh, K.; Jalilnejad, E.; Sadati Tilebon, S.M. Hydrogen Production Technologies from Ethanol. In Ethanol: Science and Engineering; Basile, A., Iulianelli, A., Dalena, F., Veziroglu, T.N., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; pp. 307–340. [Google Scholar]
- Ghasemzadeh, K.; Tilebon, S.M.S.; Basile, A. Silica Membranes Application for Hydrogen Separation. In Current Trends and Future Developments on (Bio-) Membranes Silica Membranes: Preparation, Modelling, Application, and Commercialization; Basile, A., Ghasemzadeh, K., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 243–264. [Google Scholar]
- Tilebon, S.M.S.; Ghasemzadeh, K.; Basile, A. Microporous Graphene Membrane Reactors. In Current Trends and Future Developments on (Bio-) Membranes: Microporous Membranes and Membrane Reactors; Basile, A., Ghasemzadeh, K., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; pp. 357–375. [Google Scholar]
- Ghasemzadeh, K.; Khosravi, M.; Sadati Tilebon, S.M.; Aghaeinejad-Meybodi, A.; Basile, A. Theoretical evaluation of PdAg membrane reactor performance during biomass steam gasification for hydrogen production using CFD method. Int. J. Hydrogen Energy 2018, 43, 11719–11730. [Google Scholar] [CrossRef]
- Ghasemzadeh, K.; Tilebon, S.M.S.; Basile, A. Membrane technologies for exhaust gas cleaning and carbon capture and sequestration. In Current Trends and Future Developments on (Bio-) Membranes: Cogeneration Systems and Membrane Technology; Basile, A., Spazzafumo, G., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 97–123. [Google Scholar]
- Castricum, H.L.; Sah, A.; Kreiter, R.; Blank DH, A.; Ventec, J.F.; ten Elshof, J.E. Hydrothermally stable molecular separation membranes from organically linked silica. J. Mater. Chem. 2008, 18, 2150–2158. [Google Scholar] [CrossRef]
- Boffa, V.; Blank DH, A.; ten Elshof, J.E. Hydrothermal stability of microporous silica and niobia–silica membranes. J. Membr. Sci. 2008, 319, 256–263. [Google Scholar] [CrossRef]
- Fotou, G.P.; Lin, Y.S.; Pratsinis, S.E. Hydrothermal stability of pure and modified microporous silica membranes. J. Mater. Sci. 1995, 30, 2803–2808. [Google Scholar] [CrossRef]
- Chen, W.H.; Tsai, C.W.; Lin, Y.L.; Chein, R.Y.; Yu, C.T. Reaction phenomena of high-temperature water gas shift reaction in a membrane reactor. Fuel 2020, 199, 358–371. [Google Scholar] [CrossRef]
- Russo, V.; Rossano, C.; Salucci, E.; Tesser, R.; Salmi, T.; Di Serio, M. Intraparticle diffusion model to determine the intrinsic kinetics of ethyl levulinate synthesis promoted by Amberlyst-15. Chem. Eng. Sci. 2020, 228, 115974. [Google Scholar] [CrossRef]




| Retentate | Permeate |
|---|---|
| Continuity equation | Continuity equation |
| Momentum equation | Momentum equation |
| Species equation | Species equation |
| Parameter | Name | Value | Units |
|---|---|---|---|
| ΔH | Enthalpy | 15.14 | kJ/mol |
| E1 | Activation energy | 32.51 | kJ/mol |
| E2 | Activation energy | 39.38 | kJ/mol |
| Kref | Equilibrium cst | 3.18 | - |
| k1,0 | Rate constant | 5.74 × 10−14 | (m3/mol)2/s |
| k2,0 | Rate constant | 5.32 × 10−10 | (m3/mol)·(m3/kg)/s |
| Tref | Temperature ref | 333 | K |
| Coefficients | LA | Et | EtLA | H2O |
|---|---|---|---|---|
| Ai | 0.754 | 1.65 | 0.528 | 5.46 |
| Bi | 0.258 | 0.276 | 0.246 | 0.305 |
| Ci | 738 | 514 | 666 | 647 |
| Di | 0.220 | 0.233 | 0.286 | 0.081 |
| Coefficients | LA | Et | EtLA | H2O |
|---|---|---|---|---|
| Aµi | −12.873 | 7.874 | −1.3913 | −51.964 |
| Bµi | 2295.7 | 781.98 | 1034.8 | 3670.6 |
| Cµi | −0.043631 | −3.0418 | −1.4837 | 5.7331 |
| Dµi | 0 | 0 | 0 | −5.3495 × 10−29 |
| Eµi | 0 | 0 | 0 | 10 |
| Permeate Side | Retentate Side | Position |
|---|---|---|
| ------- | inflow | Z = 0 |
| outflow | outflow | Z = L |
| Water flux | Water flux | r = red line |
| r = green line |
| Operating Parameters | Temperature Effect | Feed Flow Rate Effect | Et/LA Molar Ratio | Catalyst Loading |
|---|---|---|---|---|
| Temperature (°C) | 40–80 | 333 | 333 | 333 |
| Feed flow rate (mm3/s) | 10 | 1–15 | 10 | 10 |
| Et/LA | 1 | 1 | 1–3 | 1 |
| Catalyst loading (g) | 8.6 | 8.6 | 8.6 | 10–30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghasemzadeh, K.; Ghahremani, M.; Jalilnejad, E.; Yousefi Amiri, T.; Basile, A. Performance Comparison of Polymeric and Silica-Based Multi-Bed Pervaporation Membrane Reactors during Ethyl Levulinate Production. Membranes 2022, 12, 1000. https://doi.org/10.3390/membranes12101000
Ghasemzadeh K, Ghahremani M, Jalilnejad E, Yousefi Amiri T, Basile A. Performance Comparison of Polymeric and Silica-Based Multi-Bed Pervaporation Membrane Reactors during Ethyl Levulinate Production. Membranes. 2022; 12(10):1000. https://doi.org/10.3390/membranes12101000
Chicago/Turabian StyleGhasemzadeh, Kamran, Milad Ghahremani, Elham Jalilnejad, Taher Yousefi Amiri, and Angelo Basile. 2022. "Performance Comparison of Polymeric and Silica-Based Multi-Bed Pervaporation Membrane Reactors during Ethyl Levulinate Production" Membranes 12, no. 10: 1000. https://doi.org/10.3390/membranes12101000
APA StyleGhasemzadeh, K., Ghahremani, M., Jalilnejad, E., Yousefi Amiri, T., & Basile, A. (2022). Performance Comparison of Polymeric and Silica-Based Multi-Bed Pervaporation Membrane Reactors during Ethyl Levulinate Production. Membranes, 12(10), 1000. https://doi.org/10.3390/membranes12101000







