Relationship between Countermovement Jump and Sprint Performance in Professional Football Players
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Procedures
2.2.1. Countermovement Jump (CMJ)
- Peak Propulsive Velocity (m/s);
- Peak Relative Propulsive Power (W/Kg);
- Peak Relative Propulsive Force (%);
- Relative Propulsive Net Impulse (Newton Second per Kilo (N.s/Kg);
- Peak Braking Velocity (m/s);
- Peak Relative Braking Power (W/Kg);
- Peak Relative Braking Force (%);
- Relative Braking Net Impulse (Newton Second per Kilo (N.s/Kg);
- Braking RFD (N/s);
- Time to Take-Off (s);
- Take-Off velocity (m/s);
- Impulse Ratio (Unitless; Propulsive Net Impulse/Braking Net Impulse);
- Jump Height (m).
2.2.2. Sprint Test
- Between 0 and 5 m;
- Between 5 and 10 m;
- Between 10 and 30 m.
- Velocity 0–5 m (4.81 m/s): Group 1a—faster, Group 2a—slower;
- Braking RFD (7077 N/s): Group 1b—higher braking RFD, Group 2b—lower braking RFD.
2.3. Statistical Analysis
3. Results
3.1. Comparison of Participants’ Basic Information
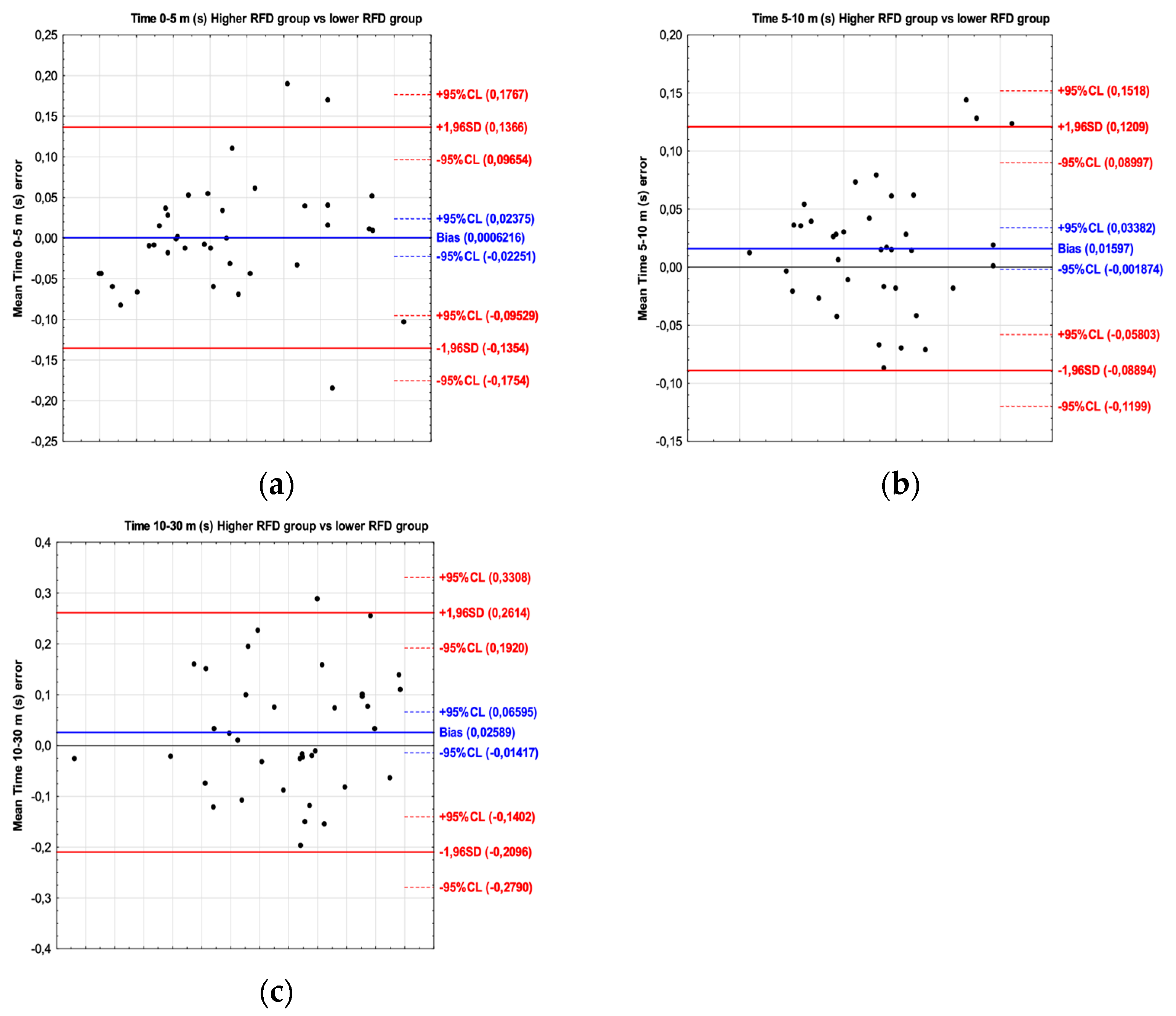
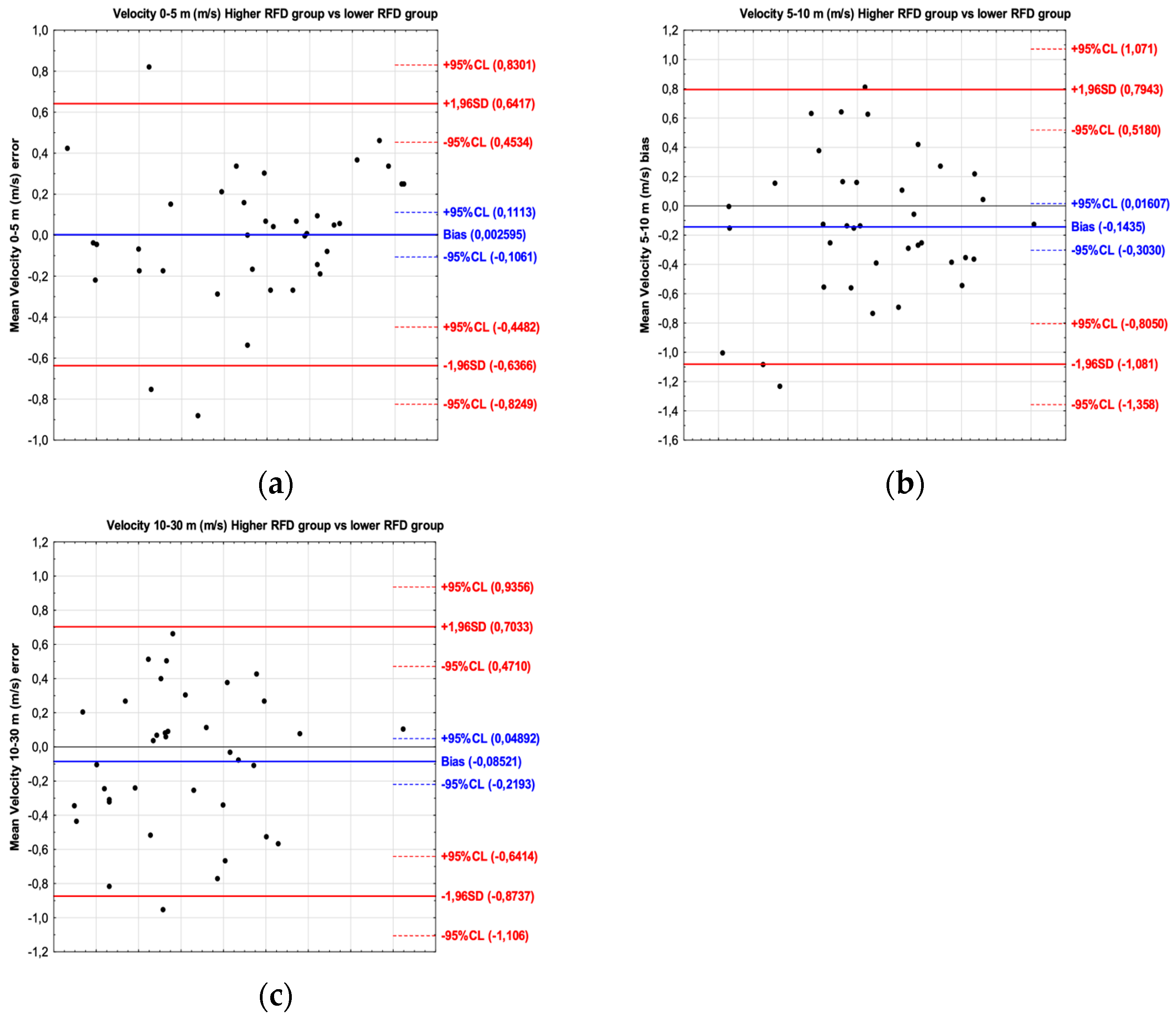
3.2. Comparison of Sprint Test Variables between Football Players with Higher and Lower Braking RFD
3.3. Comparison of CMJ Test between Faster and Slower Football Players
3.4. Relationship between CMJ and Sprint Test Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wisløff, U.; Castagna, C.; Helgerud, J.; Jones, R.; Hoff, J. Strong correlation of maximal squat strength with sprint performance and vertical jump height in elite soccer players. Br. J. Sports Med. 2004, 38, 285–288. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Nimphius, S.; Stone, M.H. The Importance of Muscular Strength in Athletic Performance. Sports Med. 2016, 46, 1419–1449. [Google Scholar] [CrossRef] [PubMed]
- Gabbett, T.J. Sprinting patterns of National Rugby League competition. J. Strength Cond. Res. 2012, 26, 121–130. [Google Scholar] [CrossRef] [PubMed]
- Cunniffe, B.; Proctor, W.; Baker, J.S.; Davies, B. An evaluation of the physiological demands of elite rugby union using Global Positioning System tracking software. J. Strength Cond. Res. 2009, 23, 1195–1203. [Google Scholar] [CrossRef] [PubMed]
- Stone, M.H.; Moir, G.; Glaister, M.; Sanders, R. How much strength is necessary? Phys. Ther. Sport 2002, 3, 88–96. [Google Scholar] [CrossRef]
- Aagaard, P.; Simonsen, E.B.; Andersen, J.L.; Magnusson, P.; Dyhre-Poulsen, P. Increased rate of force development and neural drive of human skeletal muscle following resistance training. J. Appl. Physiol. 2002, 93, 1318–1326. [Google Scholar] [CrossRef]
- Weyand, P.G.; Sandell, R.F.; Prime, D.N.; Bundle, M.W. The biological limits to running speed are imposed from the ground up. J. Appl. Physiol. 2010, 108, 950–961. [Google Scholar] [CrossRef]
- Clark, K.P.; Weyand, P.G. Are running speeds maximized with simple- spring stance mechanics? J. Appl. Physiol. 2014, 117, 604–615. [Google Scholar] [CrossRef] [PubMed]
- McBride, J.M.; Blow, D.; Kirby, T.J.; Haines, T.L.; Dayne, A.M.; Triplett, N.T. Relationship between maximal squat strength and five, ten, and forty yard sprint times. J. Strength Cond. Res. 2009, 23, 1633–1636. [Google Scholar] [CrossRef]
- Maffiuletti, N.A.; Aagaard, P.; Blazevich, A.J.; Folland, J.; Tillin, N.; Duchateau, J. Rate of force development: Physiological and methodological considerations. Eur. J. Appl. Physiol. 2016, 116, 1091–1116. [Google Scholar] [CrossRef]
- Tillin, N.A.; Pain, M.T.; Folland, J. Explosive force production during isometric squats correlates with athletic performance in rugby union players. J. Sports Sci. 2013, 31, 66–76. [Google Scholar] [CrossRef]
- Kale, M.; Aşçi, A.; Bayrak, C.; Açikada, C. Relationships among jumping performances and sprint parameters during maximum speed phase in sprinters. J. Strength Cond. Res. 2009, 23, 2272–2279. [Google Scholar] [CrossRef] [PubMed]
- Loturco, I.; D’Angelo, R.A.; Fernandes, V. Relationship between sprint ability and loaded/unloaded jump tests in elite sprinters. J. Strength Cond. Res. 2015, 29, 758–764. [Google Scholar] [CrossRef] [PubMed]
- Hori, N.; Newton, R.U.; Andrews, W.A.; Kawamori, N.; McGuigan, M.R.; Nosaka, K. Does performance of hang power clean differentiate performance of jumping, sprinting, and changing of direction? J. Strength Cond. Res. 2008, 22, 412–418. [Google Scholar] [CrossRef]
- Barr, M.J.; Sheppard, J.M.; Agar-Newman, D.J.; Newton, R.U. Transfer effect of strength and power training to the sprinting kinematics of international rugby players. J Strength Cond. Res. 2014, 28, 2585–2596. [Google Scholar] [CrossRef]
- Baker, D.G.; Newton, R.U. Comparison of lower body strength, power, acceleration, speed, agility, and sprint momentum to describe and compare playing rank among professional rugby league players. J. Strength Cond. Res. 2008, 22, 153–158. [Google Scholar] [CrossRef]
- Cronin, J.B.; Hansen, K.T. Strength and power predictors of sports speed. J. Strength Cond. Res. 2005, 19, 349–357. [Google Scholar] [CrossRef]
- Katja, T.; Coh, M. Relations between explosive strength, stiffness and sprinting performance of Slovenian sprinters. In Proceedings of the 8th Annual Congress of the ECCS, Salzburg, Austria, 9–12 July 2003; p. 133. [Google Scholar]
- Gimigliano, F.; Resmini, G.; Moretti, A.; Aulicino, M.; Gargiulo, F.; Gimigliano, A.; Liguori, S.; Paoletta, M.; Iolascon, G. Epidemiology of Musculoskeletal Injuries in Adult Athletes: A Scoping Review. Medicina 2021, 57, 1118. [Google Scholar] [CrossRef] [PubMed]
- Goes, R.A.; Lopes, L.R.; Cossich, V.R.A.; de Miranda, V.A.R.; Coelho, O.N.; do Carmo Bastos, R.; Domenis, L.A.M.; Guimarães, J.A.M.; Grangeiro-Neto, J.A.; Perini, J.A. Musculoskeletal injuries in athletes from five modalities: A cross-sectional study. BMC Musculoskelet Disord. 2020, 21, 122. [Google Scholar] [CrossRef]
- Cardoso-Marinho, B.; Barbosa, A.; Bolling, C.; Marques, J.P.; Figueiredo, P.; Brito, J. The perception of injury risk and prevention among football players: A systematic review. Front. Sports Act. Living 2022, 4, 1018752. [Google Scholar] [CrossRef]
- Badby, A.J.; Mundy, P.D.; Comfort, P.; Lake, J.P.; McMahon, J.J. The Validity of Hawkin Dynamics Wireless Dual Force Plates for Measuring Countermovement Jump and Drop Jump Variables. Sensors 2023, 23, 4820. [Google Scholar] [CrossRef]
- Lakens, D. Calculating and reporting effect sizes to facilitate cumulative science: A practical primer for t-tests and ANOVAs. Front. Psychol. 2013, 4, 863. [Google Scholar] [CrossRef]
- Bland, J.M.; Altman, D.G. Measurement error proportional to the mean. BMJ 1996, 313, 106. [Google Scholar] [CrossRef]
- Nahm, F.S. Receiver operating characteristic curve: Overview and practical use for clinicians. Korean J. Anesthesiol. 2022, 75, 25–36. [Google Scholar] [CrossRef]
- Duthie, G.M.; Pyne, D.B.; Marsh, D.J.; Hooper, S.L. Sprint patterns in rugby union players during competition. J. Strength Cond. Res. 2006, 20, 208–214. [Google Scholar] [CrossRef] [PubMed]
- Bissas, A.I.; Havenetidis, K. The use of various strength-power tests as predictors of sprint running performance. J. Sports Med. Phys. Fitness 2008, 48, 49–54. [Google Scholar] [PubMed]
- Smirniotou, A.; Katsikas, C.; Paradisis, G.; Argeitaki, P.; Zacharogiannis, E.; Tziortzis, S. Strength-power parameters as predictors of sprinting performance. J. Sports Med. Phys. Fitness 2008, 48, 447–454. [Google Scholar] [PubMed]
- Young, W.; McLean, B.; Ardagna, J. Relationship between strength qualities and sprinting performance. J. Sports Med. Phys. Fitness 1995, 35, 13–19. [Google Scholar]
- Weyand, P.G.; Sternlight, D.B.; Bellizzi, M.J.; Wright, S. Faster top running speeds are achieved with greater ground forces not more rapid leg movements. J. Appl. Physiol. 2000, 89, 1991–1999. [Google Scholar] [CrossRef]
- Styles, W.J.; Matthews, M.J.; Comfort, P. Effects of Strength Training on Squat and Sprint Performance in Soccer Players. J. Strength Cond. Res. 2016, 30, 1534–1539. [Google Scholar] [CrossRef]
- Comfort, P.; Haigh, A.; Matthews, M.J. Are changes in maximal squat strength during preseason training reflected in changes in sprint performance in rugby league players? J. Strength Cond. Res. 2012, 26, 772–776. [Google Scholar] [CrossRef] [PubMed]
- Rønnestad, B.R.; Nymark, B.S.; Raastad, T. Effects of in-season strength maintenance training frequency in professional soccer players. J. Strength Cond. Res. 2011, 25, 2653–2660. [Google Scholar] [CrossRef]
- Ronnestad, B.R.; Kvamme, N.H.; Sunde, A.; Raastad, T. Short-term effects of strength and plyometric training on sprint and jump performance in professional soccer players. J. Strength Cond. Res. 2008, 22, 773–780. [Google Scholar] [CrossRef] [PubMed]
- Sleivert, G.; Taingahue, M. The relationship between maximal jump-squat power and sprint acceleration in athletes. Eur. J. Appl. Physiol. 2004, 91, 46–52. [Google Scholar] [CrossRef] [PubMed]
- Morin, J.B.; Bourdin, M.; Edouard, P.; Peyrot, N.; Samozino, P.; Lacour, J.R. Mechanical determinants of 100-m sprint running performance. Eur. J. Appl. Physiol. 2012, 112, 3921–3930. [Google Scholar] [CrossRef]
- Jakobsen, M.D.; Sundstrup, E.; Randers, M.B. The effect of strength training, recreational soccer and running exercise on stretch-shortening cycle muscle performance during countermovement jumping. Hum. Mov. Sci. 2012, 31, 970–986. [Google Scholar] [CrossRef] [PubMed]
- Fristrup, B.; Krustrup, P.; Kristensen, K.H.; Rasmussen, S.; Aagaard, P. Test-retest reliability of lower limb muscle strength, jump and sprint performance tests in elite female team handball players. Eur. J. Appl. Physiol. 2024. [Google Scholar] [CrossRef]
- Berthoin, S.; Dupont, G.; Mary, P.; Gerbeaux, M. Predicting sprint kinematic parameters from anaerobic field tests in physical education students. J. Strength Cond. Res. 2001, 15, 75–80. [Google Scholar]
- Faccioni, A. Relationships between Selected Speed Strength Performance Tests and Temporal Variables of Maximal Running Velocity. Master’s Thesis, University of Canberra, Canberra, Australia, 1996. [Google Scholar]
| Outcome Measure | Group Velocity 0–5 m | Mean ± SD | p | Group Braking RFD | Mean ± SD | p |
|---|---|---|---|---|---|---|
| Body height (m) | 1a | 1.79 ± 0.04 | 1b | 1.89 ± 0.03 | ||
| 2a | 1.88 ± 0.05 | 0.87 | 2b | 1.80 ± 0.06 | 0.97 | |
| Body mass (kg) | 1a | 80.21 ± 5.54 | 1b | 83.30 ± 5.21 | ||
| 2a | 82.83 ± 6.01 | 0.97 | 2b | 79.45 ± 6.02 | 0.75 | |
| Age (years) | 1a | 22.3 ± 5.32 | 1b | 23.1 ± 3.25 | ||
| 2a | 24.7 ± 3.40 | 0.86 | 2b | 24.3 ± 3.30 | 0.92 |
| Outcome Measure | Group | n | Mean ± SD | p | ES | CV | SEM |
|---|---|---|---|---|---|---|---|
| Time 0–5 m (s) | 1b | 50 | 1.02 ± 0.05 | 0.48 | 0.16 | 4.90 | 0.007 |
| Time 0–5 m (s) | 2b | 37 | 1.01 ± 0.06 | 6.12 | 0.010 | ||
| Time 5–10 m (s) | 1b | 50 | 0.72 ± 0.03 | 0.01 | 0.60 | 4.30 | 0.004 |
| Time 5–10 m (s) | 2b | 37 | 0.74 ± 0.03 | 5.37 | 0.006 | ||
| Time 10–30 m (s) | 1b | 50 | 2.41 ± 0.08 | 0.04 | 0.44 | 3.34 | 0.011 |
| Time 10–30 m (s) | 2b | 37 | 2.45 ± 0.08 | 3.37 | 0.013 | ||
| Velocity 0–5 m (m/s) | 1b | 50 | 4.91 ± 0.23 | 0.40 | 0.17 | 4.75 | 0.033 |
| Velocity 0–5 m (m/s) | 2b | 37 | 4.96 ± 0.29 | 6.01 | 0.049 | ||
| Velocity 5–10 m (m/s) | 1b | 50 | 6.93 ± 0.29 | 0.01 | 0.53 | 4.24 | 0.041 |
| Velocity 5–10 m (m/s) | 2b | 37 | 6.76 ± 0.34 | 5.12 | 0.057 | ||
| Velocity 10–30 m (m/s) | 1b | 50 | 8.28 ± 0.27 | 0.04 | 0.43 | 3.34 | 0.039 |
| Velocity 10–30 m (m/s) | 2b | 37 | 8.16 ± 0.27 | 3.36 | 0.045 |
| Outcome Measure | Group | n | Mean ± SD | p | ES | CV(%) | SEM |
|---|---|---|---|---|---|---|---|
| Peak Propulsive Velocity (m/s) | 1a | 30 | 1.62 ± 0.14 | 0.03 | 0.50 | 8.78 | 0.02 |
| Peak Propulsive Velocity (m/s) | 2a | 57 | 1.67 ± 0.09 | 5.47 | 0.01 | ||
| Peak Relative Propulsive Power (W/Kg) | 1a | 30 | 51.8 ± 5.9 | 0.02 | 0.50 | 11.50 | 1.09 |
| Peak Relative Propulsive Power (W/Kg) | 2a | 57 | 54.8 ± 5.5 | 10.05 | 0.72 | ||
| Peak Relative Propulsive Force (%) | 1a | 30 | 259 ± 19 | 0.76 | 0.04 | 7.42 | 3.51 |
| Peak Relative Propulsive Force (%) | 2a | 57 | 260 ± 25 | 9.64 | 3.33 | ||
| Relative Propulsive Net Impulse (N.s/Kg) | 1b | 30 | 2.69 ± 0.21 | 0.01 | 0.52 | 7.85 | 0.03 |
| Relative Propulsive Net Impulse (N.s/Kg) | 2a | 57 | 2.79 ± 0.17 | 6.06 | 0.02 | ||
| Peak Braking Velocity (m/s) | 1a | 30 | −1.28 ± 0.24 | 0.22 | 0.30 | −18.88 | 0.04 |
| Peak Braking Velocity (m/s) | 2a | 57 | −1.36 ± 0.28 | −21.00 | 0.03 | ||
| Peak Relative Braking Power (W/Kg) | 1a | 30 | −18.8 ± 5.3 | 0.22 | 0.28 | −28.31 | 0.97 |
| Peak Relative Braking Power (W/Kg) | 2a | 57 | −20.7 ± 7.3 | −35.45 | 0.97 | ||
| Peak Relative Braking Force (%) | 1a | 30 | 247 ± 33 | 0.39 | 0.18 | 13.47 | 6.08 |
| Peak Relative Braking Force (%) | 2b | 57 | 253 ± 33 | 13.21 | 4.44 | ||
| Relative Braking Net Impulse (N.s/Kg) | 1a | 30 | 1.27 ± 0.24 | 0.21 | 0.30 | 18.87 | 0.04 |
| Relative Braking Net Impulse (N.s/Kg) | 2a | 57 | 1.35 ± 0.28 | 21.04 | 0.03 | ||
| Braking RFD (N/s) | 1a | 30 | 7340 ± 2731 | 0.43 | 0.18 | 37.21 | 498 |
| Braking RFD (N/s) | 2a | 57 | 7862 ± 3058 | 38.89 | 405 | ||
| Time to Take-Off (s) | 1a | 30 | 0.77 ± 0.08 | 0.25 | 0.27 | 11.29 | 0.01 |
| Time to Take-Off (s) | 2a | 57 | 0.80 ± 0.11 | 14.06 | 0.01 | ||
| Take-Off Velocity (m/s) | 1a | 30 | 2.67 ± 0.21 | 0.01 | 0.55 | 8.04 | 0.03 |
| Take-Off Velocity (m/s) | 2b | 57 | 2.77 ± 0.17 | 6.10 | 0.02 | ||
| Impulse Ratio (Propulsive Net Impulse/Braking Net Impulse) | 1a | 30 | 2.16 ± 0.41 | 0.94 | 0.02 | 19.03 | 0.07 |
| Impulse Ratio (Propulsive Net Impulse/Braking Net Impulse) | 2a | 57 | 2.15 ± 0.50 | 23.48 | 0.06 | ||
| Jump Height (m) | 1a | 30 | 0.36 ± 0.05 | 0.01 | 0.53 | 15.70 | 0.010 |
| Jump Height (m) | 2a | 57 | 0.39 ± 0.04 | 12.09 | 0.006 |
| Outcome Measure | Time 0–5 m (s) | Time 5–10 m (s) | Time 10–30 m (s) | Velocity 0–5 m (m/s) | Velocity 5–10 m (m/s) | Velocity 10–30 m (m/s) |
|---|---|---|---|---|---|---|
| Peak Propulsive Velocity (m/s) | −0.219 | −0.135 | −0.126 | 0.221 | 0.143 | 0.128 |
| Peak Relative Propulsive Power (W/Kg) | −0.210 | −0.282 | −0.377 | 0.211 | 0.285 | 0.373 |
| Peak Relative Propulsive Force (%) | −0.146 | −0.099 | −0.123 | 0.144 | 0.093 | 0.120 |
| Relative Propulsive Net Impulse (N.s/Kg) | −0.253 | −0.239 | −0.317 | 0.254 | 0.248 | 0.316 |
| Peak Braking Velocity (m/s) | 0.144 | 0.090 | 0.046 | −0.137 | −0.104 | −0.044 |
| Peak Relative Braking Power (W/Kg) | 0.143 | 0.113 | 0.078 | −0.136 | −0.122 | −0.075 |
| Peak Relative Braking Force (%) | −0.107 | −0.083 | −0.046 | 0.103 | 0.086 | 0.047 |
| Relative Braking Net Impulse (N.s/Kg) | −0.149 | −0.092 | −0.044 | 0.142 | 0.106 | 0.043 |
| Braking RFD (N/s) | −0.099 | −0.145 | −0.072 | 0.091 | 0.144 | 0.073 |
| Time to Take-Off (s) | −0.120 | 0.140 | 0.070 | 0.125 | −0.145 | −0.067 |
| Take-Off Velocity (m/s) | −0.263 | −0.241 | −0.309 | 0.265 | 0.250 | 0.308 |
| Impulse Ratio (Propulsive Net Impulse/Braking Net Impulse) | 0.012 | 0.002 | −0.102 | −0.005 | −0.014 | 0.103 |
| Jump Height (m) | −0.255 | −0.239 | −0.309 | 0.256 | 0.248 | 0.308 |
| Outcome Measure | Time 0–5 m (s) | Time 5–10 m (s) | Time 10–30 m (s) | Velocity 0–5 m (m/s) | Velocity 5–10 m (m/s) | Velocity 10–30 m (m/s) |
|---|---|---|---|---|---|---|
| Peak Propulsive Velocity (m/s) | −0.350 | −0.064 | 0.061 | 0.359 | 0.081 | −0.057 |
| Peak Relative Propulsive Power (W/Kg) | −0.281 | −0.341 | −0.420 | 0.280 | 0.356 | 0.421 |
| Peak Relative Propulsive Force (%) | −0.212 | −0.119 | −0.188 | 0.349 | 0.126 | 0.191 |
| Relative Propulsive Net Impulse (N.s/Kg) | −0.356 | −0.301 | −0.280 | 0.366 | 0.321 | 0.281 |
| Peak Braking Velocity (m/s) | 0.208 | −0.001 | −0.134 | −0.205 | −0.021 | 0.138 |
| Peak Relative Braking Power (W/Kg) | 0.178 | 0.030 | −0.134 | −0.179 | −0.050 | 0.140 |
| Peak Relative Braking Force (%) | −0.214 | 0.069 | 0.212 | 0.231 | −0.053 | −0.209 |
| Relative Braking Net Impulse (N.s/Kg) | −0.12 | −0.002 | 0.132 | 0.209 | 0.025 | −0.136 |
| Braking RFD (N/s) | −0.132 | 0.111 | 0.328 | 0.146 | −0.101 | −0.327 |
| Time to Take-Off (s) | −0.142 | −0.100 | 0.006 | 0.124 | 0.090 | −0.003 |
| Take-Off Velocity (m/s) | −0.367 | −0.302 | −0.269 | 0.365 | 0.322 | 0.270 |
| Impulse Ratio (Propulsive Net Impulse/Braking Net Impulse) | 0.030 | −0.146 | −0.293 | −0.029 | 0.134 | 0.299 |
| Jump Height (m) | −0.351 | −0.309 | −0.279 | 0.348 | 0.328 | 0.279 |
| Outcome Measure | Time 0–5 m (s) | Time 5–10 m (s) | Time 10–30 m (s) | Velocity 0–5 m (m/s) | Velocity 5–10 m (m/s) | Velocity 10–30 m (m/s) |
|---|---|---|---|---|---|---|
| Peak Propulsive Velocity (m/s) | −0.238 | 0.179 | −0.113 | 0.249 | −0.171 | 0.112 |
| Peak Relative Propulsive Power (W/Kg) | −0.122 | −0.394 | −0.346 | 0.124 | 0.392 | 0.340 |
| Peak Relative Propulsive Force (%) | −0.137 | 0.296 | 0.158 | 0.140 | −0.296 | −0.160 |
| Relative Propulsive Net Impulse (N.s/Kg) | −0.135 | −0.143 | −0.355 | 0.137 | 0.149 | 0.353 |
| Peak Braking Velocity (m/s) | 0.061 | −0.011 | −0.155 | −0.160 | 0.002 | 0.153 |
| Peak Relative Braking Power (W/Kg) | −0.143 | −0.364 | −0.333 | −0.144 | 0.357 | 0.328 |
| Peak Relative Braking Force (%) | −0.276 | −0.383 | −0.121 | 0.276 | 0.381 | 0.122 |
| Relative Braking Net Impulse (N.s/Kg) | −0.167 | 0.009 | −0.052 | 0.166 | −0.000 | 0.050 |
| Braking RFD (N/s) | −0.187 | 0.113 | 0.066 | 0.190 | −0.120 | −0.066 |
| Time to Take-Off (s) | −0.018 | 0.084 | −0.213 | 0.030 | −0.079 | 0.213 |
| Take-Off Velocity (m/s) | −0.144 | −0.146 | −0.352 | 0.146 | 0.152 | 0.349 |
| Impulse Ratio (Propulsive Net Impulse/Braking Net Impulse) | 0.070 | −0.050 | −0.103 | −0.068 | 0.043 | 0.102 |
| Jump Height (m) | −0.146 | −0.144 | −0.345 | 0.149 | 0.150 | 0.342 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oleksy, Ł.; Mika, A.; Kuchciak, M.; Stolarczyk, A.; Adamska, O.; Szczudło, M.; Kielnar, R.; Wolański, P.; Deszczyński, J.M.; Reichert, P. Relationship between Countermovement Jump and Sprint Performance in Professional Football Players. J. Clin. Med. 2024, 13, 4581. https://doi.org/10.3390/jcm13154581
Oleksy Ł, Mika A, Kuchciak M, Stolarczyk A, Adamska O, Szczudło M, Kielnar R, Wolański P, Deszczyński JM, Reichert P. Relationship between Countermovement Jump and Sprint Performance in Professional Football Players. Journal of Clinical Medicine. 2024; 13(15):4581. https://doi.org/10.3390/jcm13154581
Chicago/Turabian StyleOleksy, Łukasz, Anna Mika, Maciej Kuchciak, Artur Stolarczyk, Olga Adamska, Miłosz Szczudło, Renata Kielnar, Paweł Wolański, Jarosław Michał Deszczyński, and Paweł Reichert. 2024. "Relationship between Countermovement Jump and Sprint Performance in Professional Football Players" Journal of Clinical Medicine 13, no. 15: 4581. https://doi.org/10.3390/jcm13154581
APA StyleOleksy, Ł., Mika, A., Kuchciak, M., Stolarczyk, A., Adamska, O., Szczudło, M., Kielnar, R., Wolański, P., Deszczyński, J. M., & Reichert, P. (2024). Relationship between Countermovement Jump and Sprint Performance in Professional Football Players. Journal of Clinical Medicine, 13(15), 4581. https://doi.org/10.3390/jcm13154581









