Numerical Simulation and Verification of Seed-Filling Performance of Single-Bud Billet Sugarcane Seed-Metering Device Based on EDEM
Abstract
:1. Introduction
2. Materials and Methods
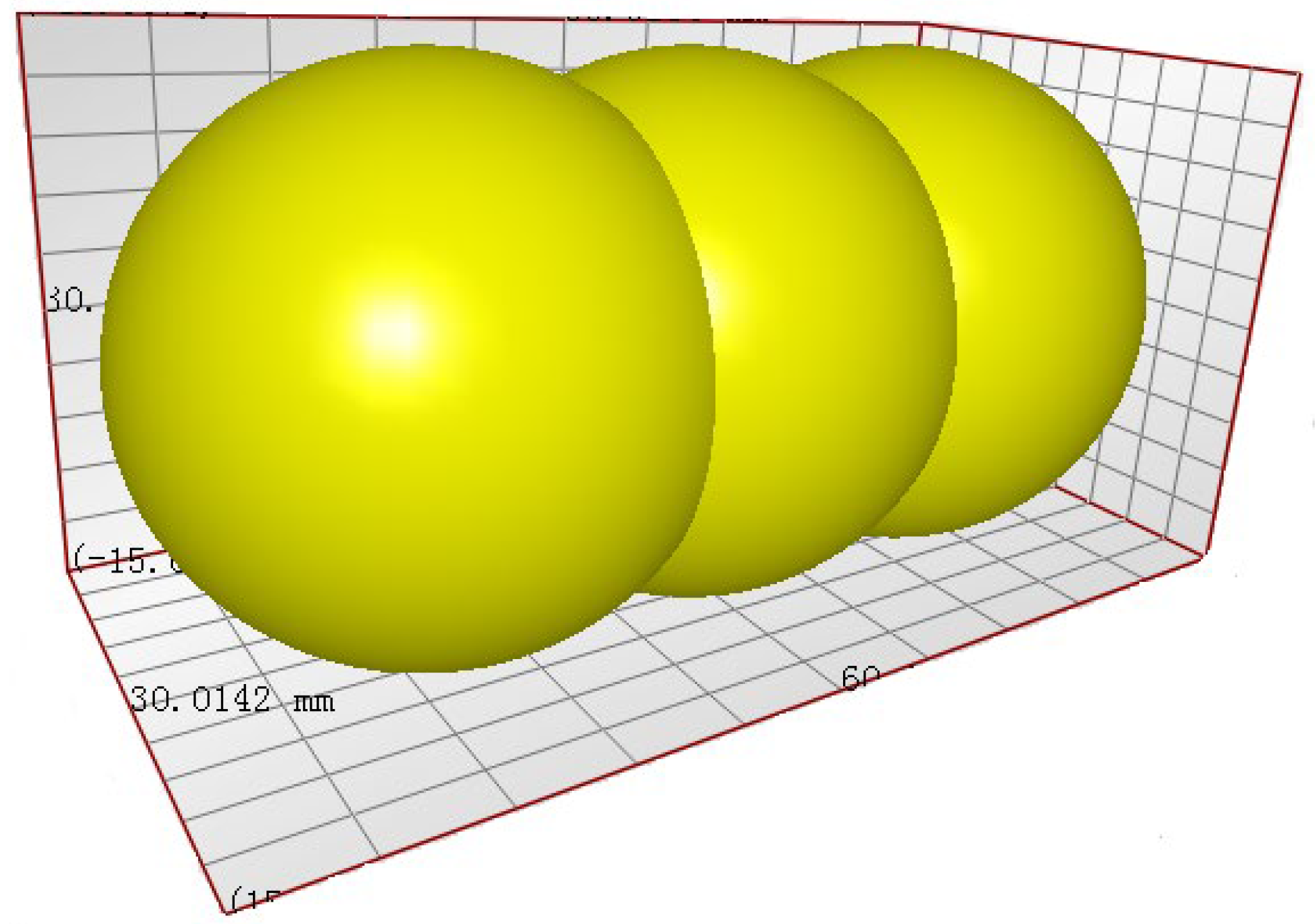
2.1. Particle Model of Single-Bud BILLET
2.2. Geometric Model of Single-Bud Billet Seed-Metering Device
2.3. Contact Model and Parameters Setting
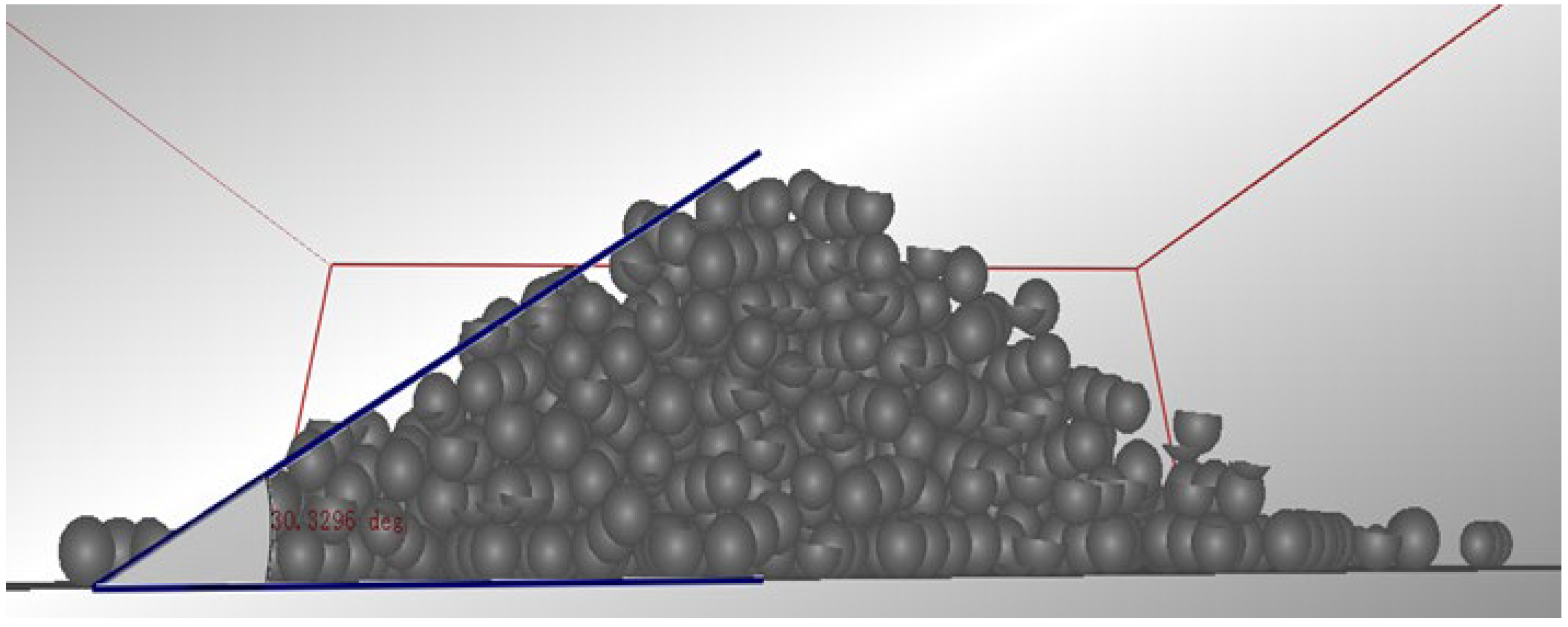
2.4. Angle of Repose Experiment
2.5. Seed-Filling Experiment
3. Results and Discussion
3.1. Analysis of the Angle of Repose Results
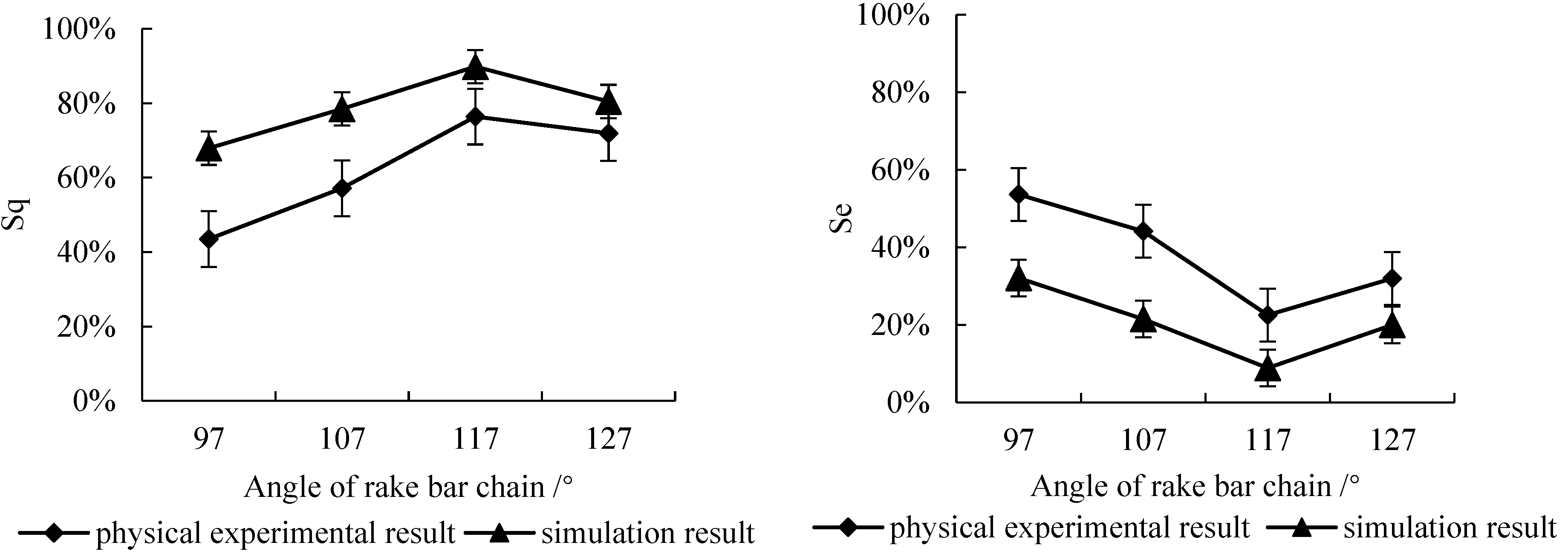
3.2. Analysis of the Seed-Filling Experiment Results
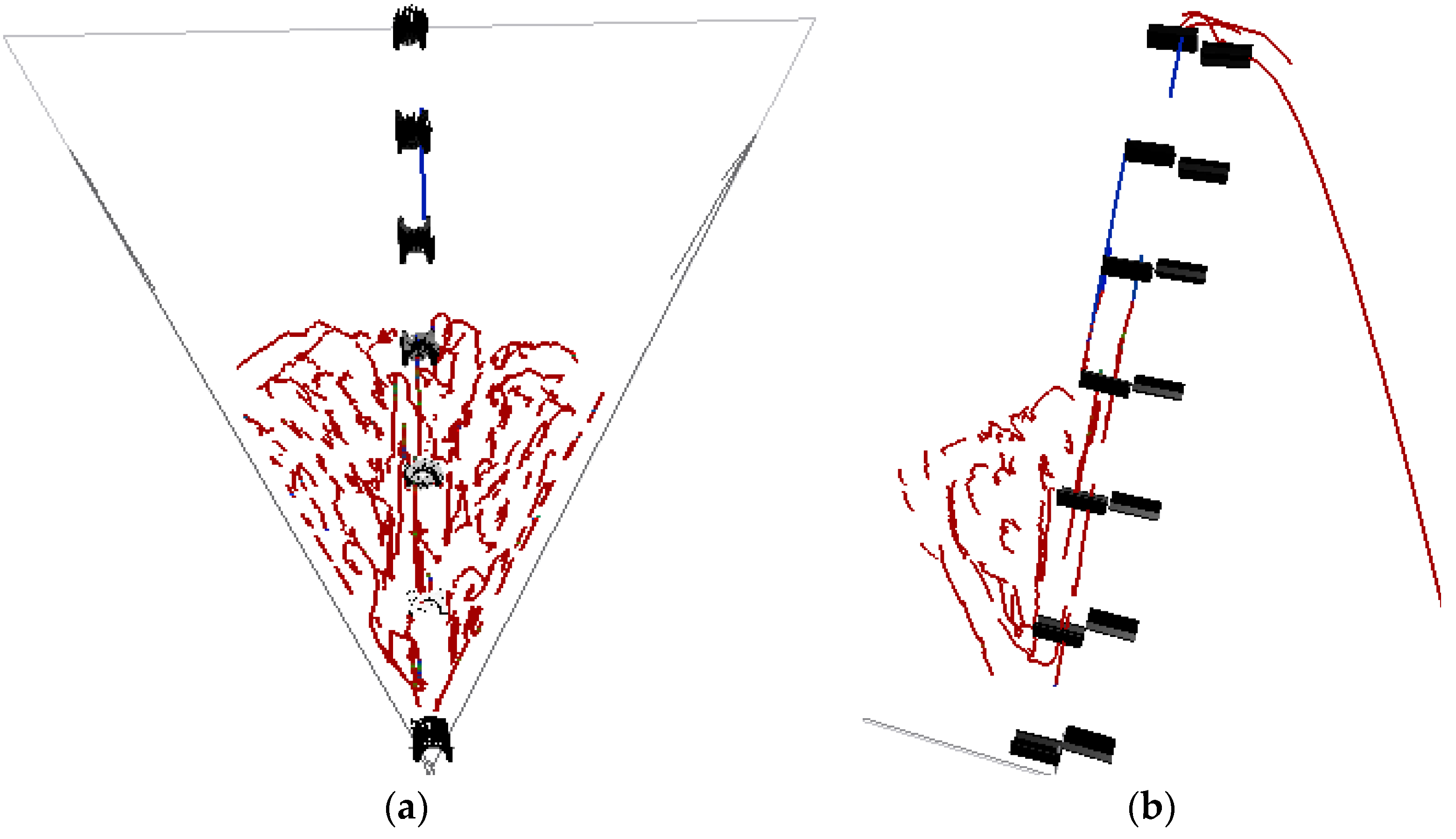
3.3. Motion of Single-Bud Billet in Seed-Metering Device Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Saengprachatanarug, K.; Wongpichet, S.; Ueno, M.; Taira, E. Comparative discharge and precision index of a sugar cane billet planter. Appl. Eng. Agric. 2016, 32, 561–567. [Google Scholar] [CrossRef]
- Wang, M.; Liu, Q.; Ou, Y.; Zou, X. Experimental Study of the Seed-Filling Uniformity of Sugarcane Single-Bud Billet Planter. Sugar Tech. 2021, 23, 827–837. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Zhou, L.; Yu, J.; Wang, Y.; Yan, D.; Yu, Y. A study on the modelling method of maize-seed particles based on the discrete element method. Powder Technol. 2020, 374, 353–376. [Google Scholar] [CrossRef]
- Owen, P.J.; Cleary, P.W. Prediction of screw conveyor performance using the Discrete Element Method (DEM). Powder Technol. 2009, 193, 274–288. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, X.; Wu, S.; Chen, C.; Wang, J.; Yuan, S.; Chen, B.; Li, P.; Xu, R. Numerical simulation of particle motion at cucumber straw grinding process based on EDEM. Int. J. Agric. Biol. Eng. 2020, 13, 227–235. [Google Scholar] [CrossRef]
- Shi, L.; Zhao, W.; Sun, B.; Sun, W. Determination of the coefficient of rolling friction of irregularly shaped maize particles by using discrete element method. Int. J. Agric. Biol. Eng. 2020, 13, 15–25. [Google Scholar] [CrossRef]
- Wang, Y.; Liang, Z.; Zhang, D.; Cui, T.; Shi, S.; Li, K.; Yang, L. Calibration method of contact characteristic parameters for corn seeds based on EDEM. Trans. Chin. Soc. Agric. Eng. 2016, 32, 36–42. [Google Scholar]
- Sun, J.; Chen, H.; Duan, J.; Liu, Z.; Zhu, Q. Mechanical properties of the grooved-wheel drilling particles under multivariate interaction influenced based on 3D printing and EDEM simulation. Comput. Electron. Agric. 2020, 172, 105329. [Google Scholar] [CrossRef]
- Yudao, L.I.; Shulun, X.I.N.G.; Shasha, L.I.; Liu, L.; Zhang, X.; Zhanhua, S.O.N.G.; Fade, L.I. Seeding performance simulations and experiments for a spoon-wheel type precision cottonseed-metering device based on EDEM. Mech. Eng. Sci. 2020, 2, 1–8. [Google Scholar] [CrossRef]
- Miao, Z.; Li, Z.; Xu, K.; Wu, L.; Su, N.; Song, G.; Liu, Y. The Numerical Simulation Analysis of Pinus sylvestris var. Mongolica seeds Vibration Situation Based on EDEM. Iop Conf. Ser. Earth Environ. Sci. 2019, 252, 052111. [Google Scholar] [CrossRef]
- Ghodki, B.M.; Goswami, T.K. DEM simulation of flow of black pepper seeds in cryogenic grinding system. J. Food Eng. 2017, 196, 36–51. [Google Scholar] [CrossRef]
- Horabik, J.; Parafiniuk, P.; Molenda, M. Stress profile in bulk of seeds in a shallow model silo as influenced by mobilisation of particle-particle and particle-wall friction: Experiments and DEM simulations. Powder Technol. 2018, 327, 320–334. [Google Scholar] [CrossRef]
- Li, H.; Zeng, S.; Luo, X.; Fang, L.; Liang, Z.; Yang, W. Design, DEM simulation, and field experiments of a novel precision seeder for dry direct-seeded rice with film mulching. Agriculture 2021, 11, 378. [Google Scholar] [CrossRef]
- Lu, F.; Ma, X.; Qi, L.; Tan, S.; Tan, Y.; Jiang, L.; Sun, G. Parameter optimization and experiment of vibration seed-uniforming device for hybrid rice based on discrete element method. Trans. Chin. Soc. Agric. Eng. 2016, 32, 17–25. [Google Scholar]
- He, Y.; Li, C.; Zhao, X.; Gao, Y.; Li, S.; Wang, X. Simulation analysis of the fertilizer ejecting device of corn fertilizer applicator based on EDEM. J. Phys. Conf. Ser. 2020, 1633, 012061. [Google Scholar] [CrossRef]
- Liu, J.S.; Gao, C.Q.; Nie, Y.J.; Yang, B.; Ge, R.Y.; Xu, Z.H. Numerical simulation of Fertilizer Shunt-Plate with uniformity based on EDEM software. Comput. Electron. Agric. 2020, 178, 105737. [Google Scholar] [CrossRef]
- Liu, T.; He, R.; Lu, J.; Zou, Y.; Zhao, M. Simulation and verification on seeding performance of nest hole wheel seed-metering device based on EDEM. J. South China Agric. Univ. 2016, 37, 126–132. [Google Scholar]
- Liu, Q.T.; Ou, Y.G.; Qing, S.L.; Chen, H.B. Failure tests of sugarcane stalks under torsion, compression and tension load. Trans. CSAE 2006, 6, 201–204. [Google Scholar]
- Fengwei, G.; Youqun, Z.; Feng, W.; Zhichao, H.; Lili, S. Simulation analysis and experimental validation of conveying device in uniform rrushed straw throwing and seed-sowing Machines using CFD-DEM coupled approach. Comput. Electron. Agric. 2022, 193, 106720. [Google Scholar] [CrossRef]
- Wang, M.; Liu, Q.; Ou, Y.; Zou, X. Determination of physical and mechanical properties of sugarcane single-bud billet. arXiv 2022, arXiv:2203.16916. Available online: https://arxiv.org/abs/2203.16916 (accessed on 31 March 2022).
- Chen, S.; Cao, S.; Gao, K.; Cao, W.; Cui, S.; Ma, J. Research on Wheat Modeling Method Based on EDEM. In Proceedings of the Journal of Physics. Conference Series (Online), Wetherby, UK, 6 February 2021; Volume 1798, p. 012048. [Google Scholar] [CrossRef]
- Guo, Z.; Chen, X.; Liu, H.; Guo, Q.; Guo, X.; Lu, H. Theoretical and experimental investigation on angle of repose of biomass–coal blends. Fuel 2014, 15, 131–139. [Google Scholar] [CrossRef]
- Matuttis, H.G.; Luding, S.; Herrmann, H.J. Discrete element simulations of dense packings and heaps made of spherical and non-spherical particles. Powder Technol. 2000, 109, 278–292. [Google Scholar] [CrossRef]
- Al-Hashemi, H.M.; Al-Amoudi, O.S. A review on the angle of repose of granular materials. Powder Technol. 2018, 1, 397–417. [Google Scholar] [CrossRef]
- Tan, Y.; Yu, Y.; Fottner, J.; Kessler, S. Automated measurement of the numerical angle of repose (aMAoR) of biomass particles in EDEM with a novel algorithm. Powder Technol. 2021, 388, 462–473. [Google Scholar] [CrossRef]
- Zhou, L.; Yu, J.; Liang, L.; Wang, Y.; Yu, Y.; Yan, D.; Sun, K.; Liang, P. DEM Parameter Calibration of Maize Seeds and the Effect of Rolling Friction. Processes 2021, 9, 914. [Google Scholar] [CrossRef]
- Wenzheng, L.; Jin, H.; Hongwen, L.; Xueqiang, L.I.; Kan, Z.H.; Zhongcai, W.E. Calibration of simulation parameters for potato minituber based on EDEM. Trans. Chin. Soc. Agric. Mach. 2018, 49, 125–135. [Google Scholar]
- Du, Y.; Cui, T.; Zhang, D.; Wei, Y.; Yang, R.; Wu, H. Establishment and Parameter Calibration of Broad Bean Seeds Simulation Particles in EDEM. In 2019 ASABE Annual International Meeting; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2019; Volume 1. [Google Scholar] [CrossRef]






| Materials | Parameter | Value |
|---|---|---|
| Billet | Poisson’s ratio | 0.344 |
| Shear modulus (Pa) | 1.08 × 107 | |
| Density (kg/m3) | 244.67 | |
| Steel | Poisson’s ratio | 0.3 |
| Shear modulus (Pa) | 7.9 × 1010 | |
| Density (kg/m3) | 7850 | |
| Billet–billet | Coefficient of restitution | 0.668 |
| Static friction coefficient | 0.352 | |
| Rolling friction coefficient | 0.026 | |
| Billet–steel | Coefficient of restitution | 0.572 |
| Static friction coefficient | 0.377 | |
| Rolling friction coefficient | 0.039 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Liu, Q.; Ou, Y.; Zou, X. Numerical Simulation and Verification of Seed-Filling Performance of Single-Bud Billet Sugarcane Seed-Metering Device Based on EDEM. Agriculture 2022, 12, 983. https://doi.org/10.3390/agriculture12070983
Wang M, Liu Q, Ou Y, Zou X. Numerical Simulation and Verification of Seed-Filling Performance of Single-Bud Billet Sugarcane Seed-Metering Device Based on EDEM. Agriculture. 2022; 12(7):983. https://doi.org/10.3390/agriculture12070983
Chicago/Turabian StyleWang, Meimei, Qingting Liu, Yinggang Ou, and Xiaoping Zou. 2022. "Numerical Simulation and Verification of Seed-Filling Performance of Single-Bud Billet Sugarcane Seed-Metering Device Based on EDEM" Agriculture 12, no. 7: 983. https://doi.org/10.3390/agriculture12070983
APA StyleWang, M., Liu, Q., Ou, Y., & Zou, X. (2022). Numerical Simulation and Verification of Seed-Filling Performance of Single-Bud Billet Sugarcane Seed-Metering Device Based on EDEM. Agriculture, 12(7), 983. https://doi.org/10.3390/agriculture12070983






