Design of a Gantry Crawler Multifunctional Operation Platform for Wine Grape Cultivation

Abstract

In response to prominent issues in existing wine grape cultivation, such as complex power machinery integration, inconvenient connection operations, and low efficiency of unilateral walking operations, a gantry track-mounted multifunctional operations platform for wine grapes was developed. The platform spans across grape trellises, enabling dual-sided operations in a single pass. Considering the high stability requirements for wine grape operations, the track walking device and hitch-lifting mechanism were developed. To accommodate the varying speed requirements of different operational stages, engine power and gearbox gear selection were calculated and selected. Structural strength analysis, steering performance analysis, and overall stability analysis of the machine were conducted. Field test results indicate a maximum operating speed of 3.85 km/h and a minimum turning radius of 3.95 m, meeting the needs of field operations. The maximum climbing angle is no more than 18°, and the machine experiences no lateral sliding or tipping when the maximum lateral tilt angle is below 28°. The hitch-lifting mechanism showed an average settlement of 1.58 mm when lifting to different heights, with a stability rate of 99.7%. This study demonstrates that the developed multifunctional operations platform can meet the field operation requirements of wine grape cultivation.

1. Introduction

Wine grapes are a type of perennially climbing economic crop and serve as the raw material for wine production. In China, the cultivation area has approached 800,000 hectares, generating direct economic benefits of over 9 billion RMB. The main cultivation regions include Ningxia, Xinjiang, and Shandong, all located north of the frost line. These areas are characterized by arid conditions, abundant sunlight, large diurnal temperature variations, and the need for winter soil covering to prevent frost damage, as well as spring soil clearing and vine lifting operations. Currently, over 20 different types of operational machinery are involved in the production of this crop, requiring tractors with varying power levels. The diverse and complex combinations of machinery lead to low operational efficiency, and there is a lack of multifunctional equipment capable of covering different processes. This results in high costs of mechanization, which has become a bottleneck for the rapid development of the industry [1].

In developed countries such as those in Europe and the United States, the cultivation techniques, operational standards, and research and development systems for wine grapes are relatively mature. Each individual operation has relatively mature grape mechanization equipment. Research on multifunctional platform-type equipment for grape cultivation is mainly based on the development of straddle tractors. These tractors have hydraulic lifting systems and attachment points at different positions on the chassis, allowing for the attachment of various types of tools to perform multiple operations in grape cultivation [2,3]. For example, the 6030 grape operation machine developed by OXBO in the United States, based on a straddle tractor, can quickly achieve plant protection and harvesting functions through a hydraulic telescopic mast. The Optimum carrier straddle tractor developed by PELLENC in France uses a modular multifunctional attachment technology to achieve pruning, plant protection, and harvesting functions [4]. The 3WZG-650 high-clearance crop protection machine developed by China Modern Agricultural Equipment Technology Co., Ltd. features wheel-type movement and a 0.9 m ground clearance, making it capable of performing crop protection tasks for tall crops. The 3WP4-1100 high-clearance plant protection machine developed by Shandong Wuzheng has a ground clearance of 1.75 m, uses full hydraulic drive, and has good field performance, making it suitable for plant protection operations in the later growth stages of tall crops [5]. The 3TG-1500 high-clearance crawler-type tea garden harvester, developed by Yunma Agricultural Machinery Manufacturing Co., Ltd., located in Yancheng City, Jiangsu Province, China. using a gantry straddle structure, achieves inter-row harvesting. Wu Yan from Ningxia University studied multifunctional grape operation machinery, designing a specialized operation chassis and hydraulic control system with a ground clearance of 2.5 m, achieving theoretical research on multifunctional grape operation equipment [6]. These studies provide valuable references for the design of gantry crawler-type multifunctional operation platforms for wine grapes.

This paper addresses the issues of the numerous types of operational equipment and the lack of multifunctional machinery for wine grape cultivation in China. Based on the cultivation model and agronomic requirements of wine grapes, a multifunctional operations platform spanning across grape trellises was designed. The design and selection of key components of the platform’s chassis were carried out, along with an analysis of the longitudinal and lateral operational stability of the entire machine. Field tests were conducted to verify the performance indicators and the rationality of the overall design. Furthermore, this study provides a novel solution for the mechanized operations of wine grapes. The development of new components can reduce the number of machines needed for grape cultivation. Dual-sided operation boosts efficiency and lowers costs, emphasizing the research’s importance and commercial value.

2. Materials and Methods

2.1. Job Requirements and Design Objectives

Cultivation of wine grapes differs from that of table grapes. To facilitate ventilation, light penetration, ease of field management, and mechanized operations, a single-arm trellis system is primarily used. The trellis poles are generally made of materials such as cement, metal, or wood and are erected in the middle of the field. The height of the trellis is typically between 1.5 and 1.8 m, with a row spacing of 3 m. The horizontal distance between poles within a row is generally 4 to 6 m, with a horizontal wire pulled every 50 cm vertically to facilitate grapevine climbing.

Since wine grape cultivation in China is located north of the freezing line, between latitudes 35° N and 45° N, winter temperatures are low. To prevent frost damage to the grapevines, they need to be buried under the soil during winter, and soil uncovering operations are carried out in spring. The soil uncovering depth ranges from 30 to 50 cm, requiring high power consumption for mechanized operations and posing significant operational challenges. To improve the efficiency of mechanized operations for wine grapes, a gantry straddle structure is used to simultaneously operate on both sides of a single row of grapevines. This differs from the traditional mechanized operation method using tractor-mounted equipment. This equipment can adapt to the field walking environment of wine grape cultivation and allows for quick attachment of functional components. The chassis uses a mechanical multi-speed transmission with sufficient power. It allows real-time adjustment of the machine’s walking speed based on component working conditions. Wine grape cultivation areas have diverse topography, requiring the power chassis to have high ground clearance and mobility during mechanized operations. Based on field surveys, this paper focuses on designing a multifunctional operation platform for wine grape cultivation areas with slopes of ≤15%. Considering the above operational requirements and actual conditions, the design objectives for the gantry crawler-type multifunctional operation platform for wine grapes are clarified, with specific parameters shown in

Table 1. Overall Design Target Parameters.

Parameter	Numerical Value/Form
Travel mechanism	Caterpillar type
Whole structure	Gantry cross-type
Gearbox form	Mechanical transmission
Engine type	Diesel engine
Minimum speed/(km/h)	1
Maximum climbing angle/°	≥10°
Inner span width/(m)	2.2
Inner span height/(m)	2

.

2.2. Structure and Working Principle of the Whole Machine

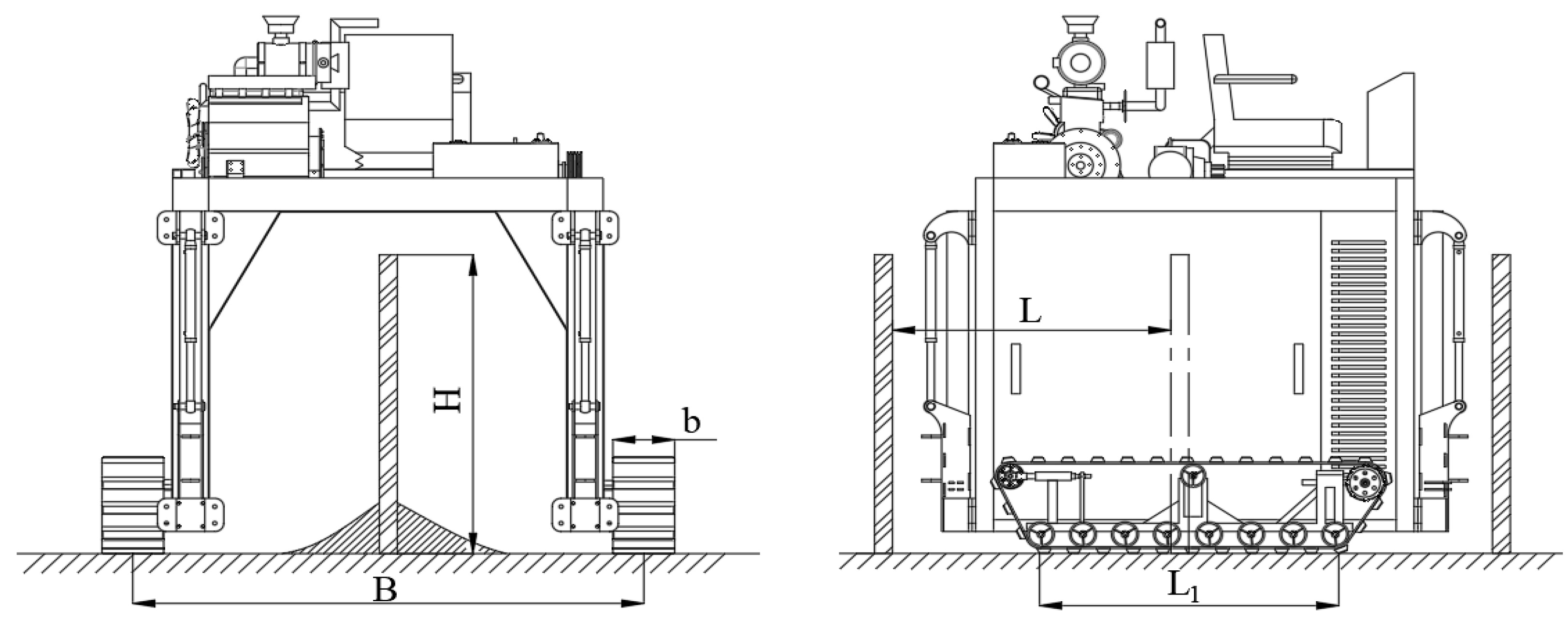
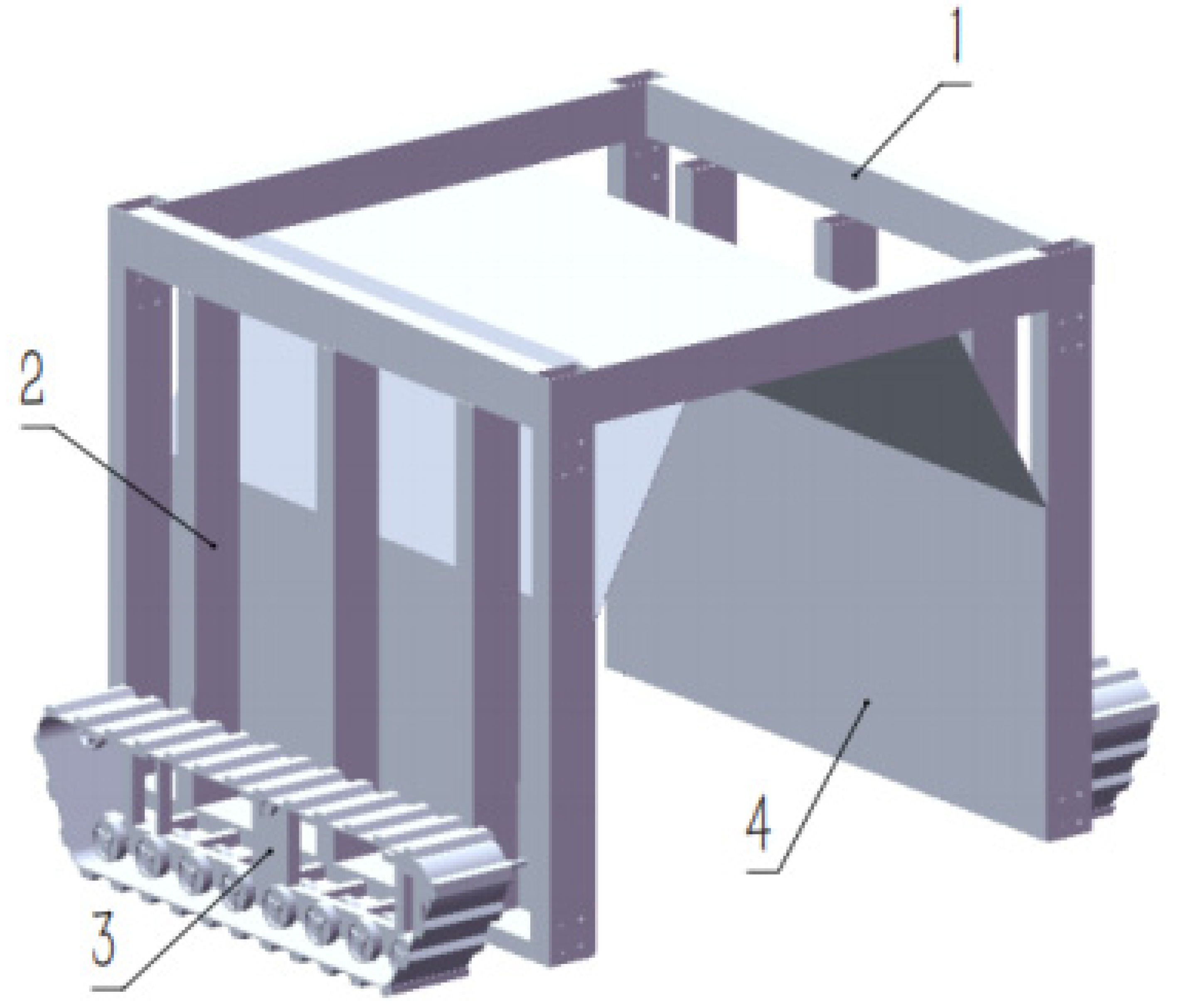
2.2.1. Overall Structure

The chassis of the gantry crawler-type multifunctional operating platform for wine grapes mainly consists of a frame, crawler walking device, engine, hydraulic oil tank, control console, hydraulic lifting hitch device, and operational components. The frame is equipped with a cab, control console, and transmission device. The hydraulic lifting hitch device is fitted with soil-clearing operational components and plant protection operational components for wine grapes, and additional pruning and weeding operational components can be attached later. The overall structure is shown in Figure 1.

2.2.2. Chassis Working Principle

The gantry crawler-type multifunctional platform for wine grapes has both mechanical and hydraulic power output systems. It powers the chassis crawler walking system and the multifunctional operational system separately. The entire machine uses a diesel engine as the power source. The output power is transmitted in two ways: through the clutch and transfer case. One connection leads to the gear transmission box, directly driving the main hydraulic pump. The pump is linked to the hydraulic valve cluster, which distributes hydraulic force to the cylinders and motors, powering the machine’s operational components. The other way is connected to the mechanical power take-off through the transfer case gear pair, and the power take-off transmits power to the gearbox via a belt pulley. The gearbox then distributes the power to the crawler drive wheels on both sides through long and short drive shafts, completing the power transmission of the chassis.

2.3. Design of Walking Device

2.3.1. Analysis of Operational Walking Environment

The operational environment is a wine grape plantation, as shown in Figure 2. The entire machine spans across the grapevine trellis, with the soil between the poles being loose and uneven, with ups and downs, making the field conditions relatively poor for walking. The internal height of the machine must exceed the height H of the trellises. If a wheeled walking device is used, it may become unbalanced and collide with the trellises, causing secondary soil compaction, which is unfavorable for subsequent soil clearing operations. To ensure better traction, lower ground pressure, and soil looseness, a track-mounted walking device was selected as the walking system for the machine. The following figure illustrates the operational mode of the machine. In the Figure 2, B is the track gauge, L₁ is the track support surface length, b is the track width, and H is the height of the grape trellis, which is 1.8 m. L is the width between adjacent grape trellises, which is 3 m.

2.3.2. Calculation of Main Parameters for the Walking Device

The key parameters determining the design and selection of the tracks include the track plate width, track support surface length, and track gauge [7]. The track plate width is determined by the average ground pressure; the larger the width, the smaller the average ground pressure, and the less soil compaction occurs [8]. The track support surface length is the length of the track in contact with the ground, and this length is directly proportional to the machine’s steering capability; the longer the length, the better the walking stability. The track gauge is the distance between the centers of the tracks on either side; the larger the gauge, the lower the center of gravity, making the machine less susceptible to tipping over. Based on actual working conditions, the calculation formulas for each parameter are as follows:

$$\{\begin{array}{l}b=(0.9\sim 1.1)\times 209\times \sqrt[3]{G}\\ L_1=(1.25\sim 1.5)\times \sqrt[3]{G}\\ B=(6.5\sim 7.5)\times b\end{array}$$

(1)

In the formula, b—the track width, in mm; G—total machine weight in kilograms, calculated using the SolidWorks mass evaluation module, resulting in an approximate machine mass of 4500 kg; L₁—the length of the track support surface, in mm; B—the track gauge, in mm; L—the total length of the track, in mm.

The parameters of the tracks determine the track selection. Considering the actual conditions in the vineyard and the substantial weight of the machine, the tracks need to provide good walking stability and low soil compaction, facilitating subsequent soil-clearing operations. According to Formula (1), the calculated values were taken at the upper limits to adapt to the soft soil conditions of the vineyard, resulting in b = 310 mm to 379 mm, with 379 mm selected; L₁ = 2 m to 2.4 m, with 2.4 m selected; and B = 2.4 m to 2.8 m, with 2.8 m selected.

2.4. Design and Calculation of Drive System

2.4.1. Design of Drive Scheme

The gantry-tracked multifunctional operation platform for wine grapes adopts a rigid frame cross-over structure. The entire machine’s control platform and transmission system are arranged directly above the vehicle body. The track drive wheel is connected to the upper transmission system via a chain. The transmission system is located at the rear side of the top of the vehicle body. The engine and transfer case are arranged vertically. The power is output through the power take-off on one side of the transfer case and is transmitted by the drive shaft and pulley, then delivered to the gearbox to drive the track walking device. The hydraulic transmission system is located on one side of the vehicle body and controls each hydraulic cylinder and hydraulic motor through a hydraulic valve group. This transmission scheme design is beneficial for the spatial layout of the vehicle body, reducing the length of the vehicle body and ensuring the stability and maneuverability of the vehicle [9,10,11], as shown in Figure 3.

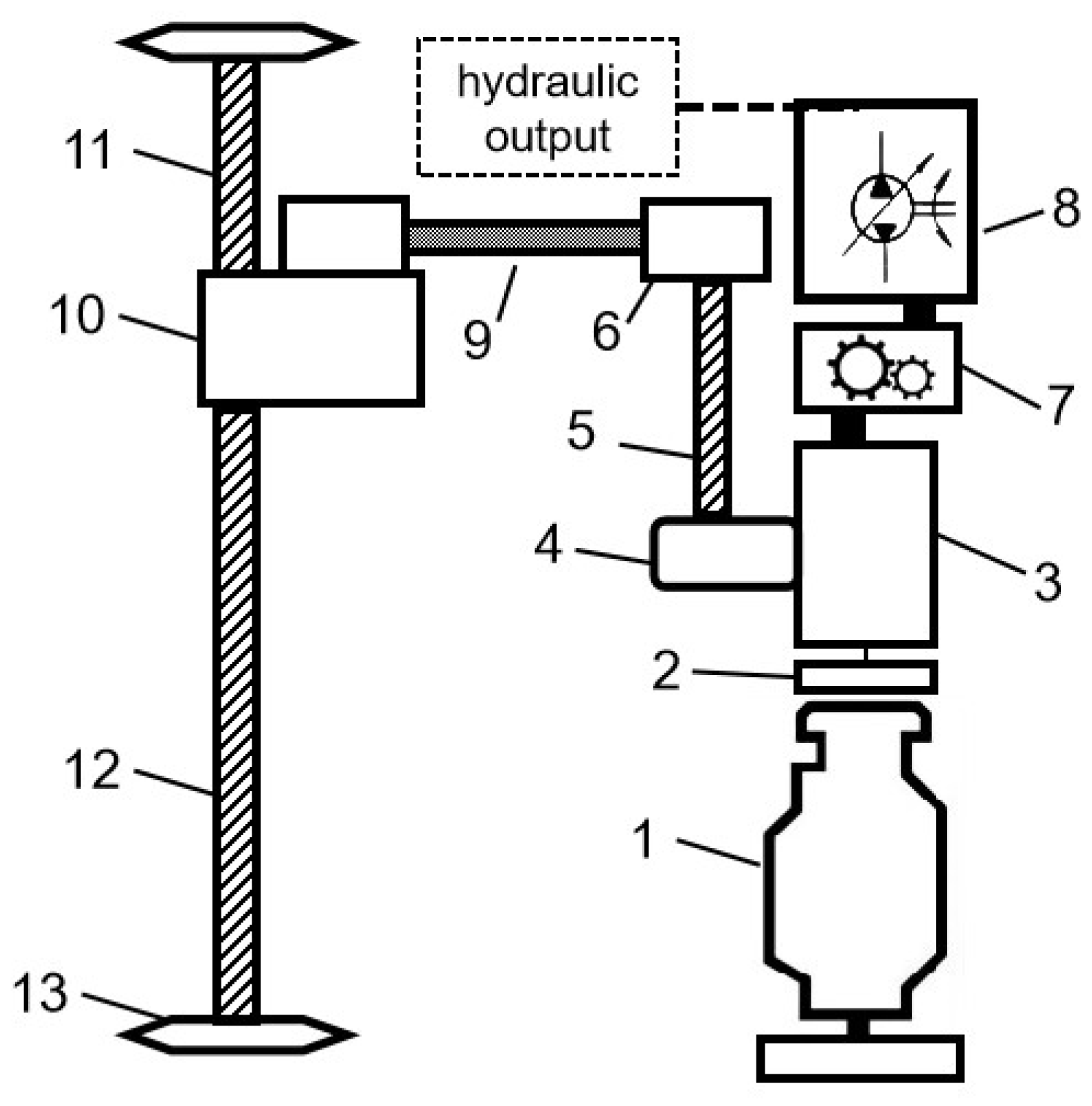
2.4.2. Hydraulic System Principle

The hydraulic system schematic is shown in Figure 4. The overall hydraulic system is controlled in three circuits: the walking control oil circuit, the lifting control oil circuit, and the rotation control oil circuit. Hydraulic power is provided by a hydraulic pump. The walking control oil circuit consists of a speed control valve, a three-position, four-way solenoid valve, a check valve, a flow divider, a pressure-reducing valve, and a hydraulic motor. The lifting control oil circuit consists of a telescopic hydraulic cylinder, a throttle valve, a check valve, a three-position, four-way solenoid valve, and a pressure-reducing valve. The rotation control oil circuit consists of a hydraulic motor, a throttle valve, a check valve, a three-position, four-way solenoid valve, and a pressure-reducing valve. By controlling the three-position, four-way solenoid valve, the hydraulic motor can rotate forward and backward, and the telescopic hydraulic cylinder can move up and down. The overall oil circuit controls the pressure through the pressure-reducing valve, and the overflow valve protects the main oil circuit’s safety. The speed control valve is primarily used to reduce the hydraulic impact caused by action changes during the machine’s walking operation, and the check valve ensures the independent operation of the oil circuit to avoid interference.

2.4.3. Steering Performance Analysis

When the two tracks perform differential movement driven by the gearbox, one track is braked, resulting in a zero linear speed, and steering is completed relying on the drive of the other track [12]. The turning radius is related to the track gauge and track width. The steering of the track is directed from the side with a non-zero speed to the side with a zero speed. The radius r of the inner track’s turn is related to the track support surface length L1 through a trigonometric function relationship. R is the turning radius of the entire machine. The schematic diagram of the machine’s differential steering is shown in Figure 5.

Without considering the external sliding and skidding of the tracked chassis, the trigonometric formula for calculating the turning radius of the entire machine is as follows:

$$\{\begin{array}{l}\theta =arctg{\displaystyle \frac{B+0.5b}{L_1}}\\ R={\displaystyle \frac{L_1}{\cos \theta }}\end{array}$$

(2)

In the formula, B—the track gauge, taken as 2.9 m; b—the track width, taken as 0.35 m; L₁—the length of the track support surface, taken as 2.4 m; R—the turning radius of the entire machine.

When the machine makes a stationary turn, the outer track rotates around the inner track, with the inner track’s linear speed being zero. The minimum turning radius of the entire machine, calculated using Formula (2), is 3.8 m.

2.4.4. Engine Calculation and Selection

The selection of the engine is based on the maximum power consumption. Among the various operations, the soil clearing operation is the most power-intensive as it involves earthwork, making it the basis for calculating the overall machine power. During the soil clearing process, the soil must first be loosened and thrown out through a mechanical rotary tilling action, followed by the use of plow blades to excavate the loosened soil and deposit it on both sides of the grapevines. Hence, the machine’s operating power P_S is the sum of the power consumption for soil clearing P_q and the power required for walking on the maximum slope P_z. The actual power transmitted by the engine to the output shaft P_A is the ratio of the machine’s operating power P_S to the transmission efficiency η. The calculation formula is as follows:

$$P_A={\displaystyle \frac{P_S}{\eta }}={\displaystyle \frac{P_q+P_z}{\eta }}$$

(3)

In the formula, P_A—the actual power transmitted by the engine to the output shaft; P_S—the machine’s operating power; Pq—the power consumed by clearing soil; Pz—the required power when walking on the maximum slope; η—transmission efficiency of the track-mounted chassis, η = 0.87.

Power Requirements for Soil Clearing Operations: The gantry track-mounted self-propelled multifunctional operations platform is a dual-sided operational device. According to the “Agricultural Machinery Design Manual”, the power consumption for soil excavation by the dual-sided soil clearing machine’s plow blade is calculated as follows:

$$\{\begin{array}{l}{P}_q=P_l+P_x\\ P_l=2{\displaystyle \frac{Kabn}{{\eta }_T}}\times u_{\min }\\ P_x=2Fv\\ F=\sqrt{{\left(mg\right)}^2+{({\displaystyle \frac{m{v}^2}{r}})}^2}\end{array}$$

(4)

In the formula, P_l—power consumption for soil excavation by the plow blade of the soil clearing machine; P_x—power consumption of the rotary thrower; n-Number of supporting plows, n = 1, η_T—utilization factor of drawbar pull, η_T = 0.9; a-Design tillage depth, a = 0.25; b-Single plow width, b = 0.5; K-Ploughing specific resistance of soil, K = 35 kpa; u_min-Minimum operating speed, u_min = 1 km/h; F-force exerted by the thrower on the soil.

Based on measurements, the rotary thrower of the soil-clearing machine has a diameter of 60 cm (radius 30 cm) and a rotational speed of 286 r/min. The soil bulk density in the vineyard is 1.3 g/cm³, with a soil moisture content of 23%, resulting in a unit thrower mass of 21.6 kg and a thrower linear speed of 8.88 m/s. According to the above Formula (4), the force exerted by the thrower on the soil is F = 5835.84 N. The power consumption for soil excavation by the plow blade is P_l = 10KW, and the power required for the rotary thrower is P_x = 105 KW, resulting in a total power consumption for soil clearing of P_q = 115 KW.

Power Requirements for Field Transfer: Based on the machine’s full-load speed characteristics, when the maximum slope is 15%, the maximum power required for the machine’s operation is the sum of the power consumption due to rolling resistance on flat ground, air resistance, and maximum slope rolling resistance. The calculation formula is as follows:

$$\{\begin{array}{l}{P}_z=P_f+P_w+P_i\\ P_f={\displaystyle \frac{mgfu}{3600}}\\ P_w={\displaystyle \frac{C_{d}A{u}^3}{76140}}\\ P_i={\displaystyle \frac{mgu\alpha }{3600}}\end{array}$$

(5)

In the formula, P_z—the maximum power required for the whole machine operation; P_f—the rolling resistance of the whole machine on flat ground consumes power; P_w—air resistance horsepower; P_i—the maximum climbing rolling resistance consumes power; u—the maximum speed of the whole machine, u = 4 km/h; m—the quality of the whole machine; the quality of the whole machine using SolidWorks quality evaluation module to calculate the quality of the whole machine is about 4500 kg; g—gravity acceleration, g = 9.8 m/s²; f—coefficient of rolling resistance, f = 0.02; C_d—coefficient of air resistance, C_d = 0.9; A—the forward windward area of the whole machine, A = 3.6 m²; α—maximum climbing degree, take 15%, about 8°.

According to the above Formula (5), the power consumption due to rolling resistance on flat ground is P_f = 0.98 KW, air resistance power consumption is P_w = 0.002 KW, and maximum slope rolling resistance power consumption is Pi = 7.35 KW, resulting in a total maximum power requirement for operation P_z = 8.4 KW.

In summary, based on the calculation Formulas (3)–(5), the power required by the machine P_A = 123.4 KW. The actual power transmitted by the engine to the output shaft is calculated to be 141 kW, equivalent to approximately 189 horsepower. To ensure a power reserve, the selected engine power should be slightly greater than the calculated requirement [13,14]. Therefore, a Yuchai YCS06200-62 type inline six-cylinder water-cooled diesel engine with 200 horsepower, a maximum torque of 750 N·m, a rated speed of 2100 rpm, and a displacement of 6.2 L was selected. According to the “Handbook of Mechanical Design” [15]., the corresponding hydraulic pump provides a pressure of approximately 18 MPa.

2.4.5. Selection and Calculation of the Transmission

Due to the compact space layout of the overall power system located at the rear upper part of the multifunctional operation platform, it is necessary to select and calculate the transmission. First, the transmission ratio range must be determined, typically defined by the ratio of the highest gear to the lowest gear. The highest gear is generally the direct gear, with the transfer case input transmission ratio assumed to be 1. At the lowest speed, the transmission ratio is maximum, calculated as follows:

$$i_{\min }=0.377{\displaystyle \frac{rn}{i_g{u}_{\max }}}$$

(6)

In the formula, u_max—the maximum speed of the whole machine; r—the driving wheel pitch circle radius; n—rated engine speed; i_g—the transmission ratio of the transfer case; i_min—the minimum transmission ratio of the gearbox.

Based on the design of the gantry crawler self-propelled multifunctional grape operation platform, the maximum driving speed should not be less than 4 km/h, with the engine’s rated speed at 2100 r/min, the drive wheel pitch circle radius at 0.12 m, and the transfer case transmission ratio at 1. From the above Formula (6), the lowest gear transmission ratio of the transmission is i_min = 10.6, while the highest gear transmission ratio, which is the transfer case transmission ratio, is i_g = 1, meaning the input speed is directly transferred to the drive shaft. To improve the overall power characteristics and fuel economy and to ensure smoother power transitions, the number of gears selected should be ≥6. The calculation formula for the transmission ratios of each gear is:

$$i_m=i_{m-1}\sqrt[6]{{\displaystyle \frac{i_{\max }}{i_{\min }}}}$$

(7)

Using the Formula (7), the transmission ratios for each gear are shown in

Table 2. Transmission ratio of each gear of the transmission box.

Name	Numerical Value
Gear	1	2	3	4	5	6
Transmission ratio	5.7	4.2	3.3	2.7	2.2	1
Fast C6J76TA	5.7	3.5	2.3	1.45	1	0.8
Theoretical velocity (km/h)	1	1.3	1.7	2.1	2.6	4

.

In summary, the theoretical calculation for the lowest gear transmission ratio of the gantry crawler self-propelled multifunctional grape operation platform is 5.7, with the highest gear transmission ratio being 1 and the number of gears being 6. In actual selection, the highest gear transmission ratio is greater than the theoretical calculation. The Fast C6J76TA manual transmission is selected, with the lowest gear transmission ratio at 5.7, the highest gear transmission ratio at 0.8, and the number of gears at 6, with a maximum input torque of 760 N·m, meeting the operational requirements.

2.5. Design of Hanging Lifting Mechanism

The crawler self-propelled grape operation platform is equipped with four sets of hanging lifting mechanisms. By attaching different operational function components, it can perform various tasks, such as soil-clearing operations. The functional components are attached via pin holes on the sliding sleeve, which moves up and down along the guide rail. One side of the sliding sleeve is fixedly connected to a hydraulic cylinder, which is externally connected to a hydraulic pump. The sliding sleeve is lifted or lowered by changes in flow and pressure. The two ends of the hydraulic lifting mechanism are mechanically connected to the multifunctional operation platform [16], as shown in Figure 6.

The lifting mechanism is connected to a hydraulic distribution valve, with the oil supply pressure provided by the hydraulic pump. The components are lifted or lowered as needed for the operation. The lifting force calculation begins by using the SolidWorks mass evaluation module to analyze the mass of each operational component. The heaviest component weighs approximately 558 kg, translating to 5468.4 N. Based on the hydraulic lifting requirements, a hydraulic cylinder with an inner diameter of D = 40 mm is selected as the piston rod diameter. According to the engine selection calculations, a 200-horsepower diesel engine has a rated speed of 2100 rpm. Referring to the “Handbook of Mechanical Design” [15], the corresponding gear hydraulic pump’s rated oil supply pressure is 18 MPa. After pressure reduction through the hydraulic distribution valve and the relief valve, the oil supply pressure is 8 MPa. The lifting force of the hydraulic cylinder is calculated using the following formula:

$$F=0.785D^{2}P\psi {\eta }_1\ge G_0$$

(8)

In the formula, D—inner diameter of the hydraulic cylinder, D = 50 mm; G₀—the actual weight of the hydraulic cylinder to be lifted, G₀ = 5468.4 N; ψ—hydraulic cylinder load rate, ψ = 0.7; η₁—the total efficiency of the hydraulic cylinder, η₁ = 1; P—feed pressure, P = 8 MPa.

From the above Formula (8), the lifting force of the hydraulic cylinder is calculated to be 10,990 N, which is greater than the actual required lifting weight of 5468.4 N. Therefore, the selected hydraulic cylinder meets the lifting requirements during operation and satisfies the design needs.

When calculating the lifting force of the hydraulic cylinder, it is necessary to check the maximum allowable stress on the hydraulic cylinder wall thickness. 45# steel was selected as the cylinder material. According to the “Handbook of Mechanical Design” [15], the cylinder has an inner diameter of 50 mm and a wall thickness of 9 mm, with the allowable stress for 45# steel being [σ] ≥ 120 MPa. The formula for calculating the maximum allowable stress on the hydraulic cylinder wall thickness is as follows:

$${\sigma }_{\max }={\displaystyle \frac{P_{y}D}{2\delta }}={\displaystyle \frac{1.5PD}{2\delta }}<\left[\sigma \right]$$

(9)

In the formula, σ_max—maximum allowable stress of the hydraulic cylinder; P_y—test pressure of the hydraulic cylinder, P_y = 1.5P; δ—wall thickness of the hydraulic cylinder, which is 9 mm according to the “Handbook of Mechanical Design” [15].

Using the above Formula (9), the maximum allowable stress of the hydraulic cylinder is calculated to be 33.3 MPa. The calculated maximum allowable stress on the hydraulic cylinder wall thickness is less than the allowable stress for 45# steel, which is 120 MPa. Therefore, the hydraulic cylinder design is reasonable and can withstand the operational load, making the design of the hitch-lifting mechanism feasible.

2.6. Chassis Structure Design

The design of the chassis structure is a multifaceted system engineering task. While meeting operational needs, it must also comply with technical indicators that affect chassis performance, such as stiffness and strength. Based on the agronomic model of wine grape cultivation, the entire machine adopts a gantry-style chassis structure for design layout. The walking device connects the suspension system with the tracked walking device. The cab, transmission system, and hydraulic control system are located on top of the vehicle, while the working devices are positioned at the four corners of the vehicle. These are mechanically connected by separate hydraulic lifting devices. To ensure a reasonable overall structure while considering both structural strength and lightweight factors, the chassis material selected is Q235 structural steel [17,18]. Different sizes of rectangular steel tubes are welded horizontally and vertically to manufacture the chassis. Since the entire machine straddles the grape trellis to facilitate passage without interference, the internal clear height is set at 2.1 m and the clear width at 2 m. The structural design is shown in Figure 7.

Since the transmission and control components of the machine are located above the frame, assuming a uniform load and symmetrical structure on both sides, the center of mass of the entire machine is positioned along the central axis of the vehicle body. By taking moments about the tracks on both sides and simplifying the frame as a single beam under concentrated load, the entire load is positioned at the exact center of this beam. Using the SolidWorks mass evaluation module, the maximum mass exerted on the top of the vehicle body is approximately m = 1054 kg. The formula for calculating the maximum bending stress of the beam is:

$${\sigma }_{\max }={\displaystyle \frac{M_{\max }}{K}}={\displaystyle \frac{3P{L}_{AB}}{2b{h}^2}}<\left[\sigma \right]$$

(10)

In the formula, δ_max—maximum allowable bending stress of frame; M_max—maximum bending moment of frame; K—anti-bending moment interface coefficient; P—simplify the maximum force of the beam, P = mg = 10.3 KN; L_AB—simplify the length of beam, taken as 2405 mm; b—cross-section width of beam, taken as 100 mm; h—cross-section height of beam, taken as 200 mm.

Using the above Formula (10), the maximum bending stress of the chassis is calculated to be 9.27 MPa. The allowable bending stress of Q235 is 235 MPa. The calculated bending stress is far less than the allowable bending stress of Q235. Therefore, the strength design of the vehicle chassis is reasonable and can withstand operational loads. The chassis structure design scheme is feasible.

3. Results and Discussion

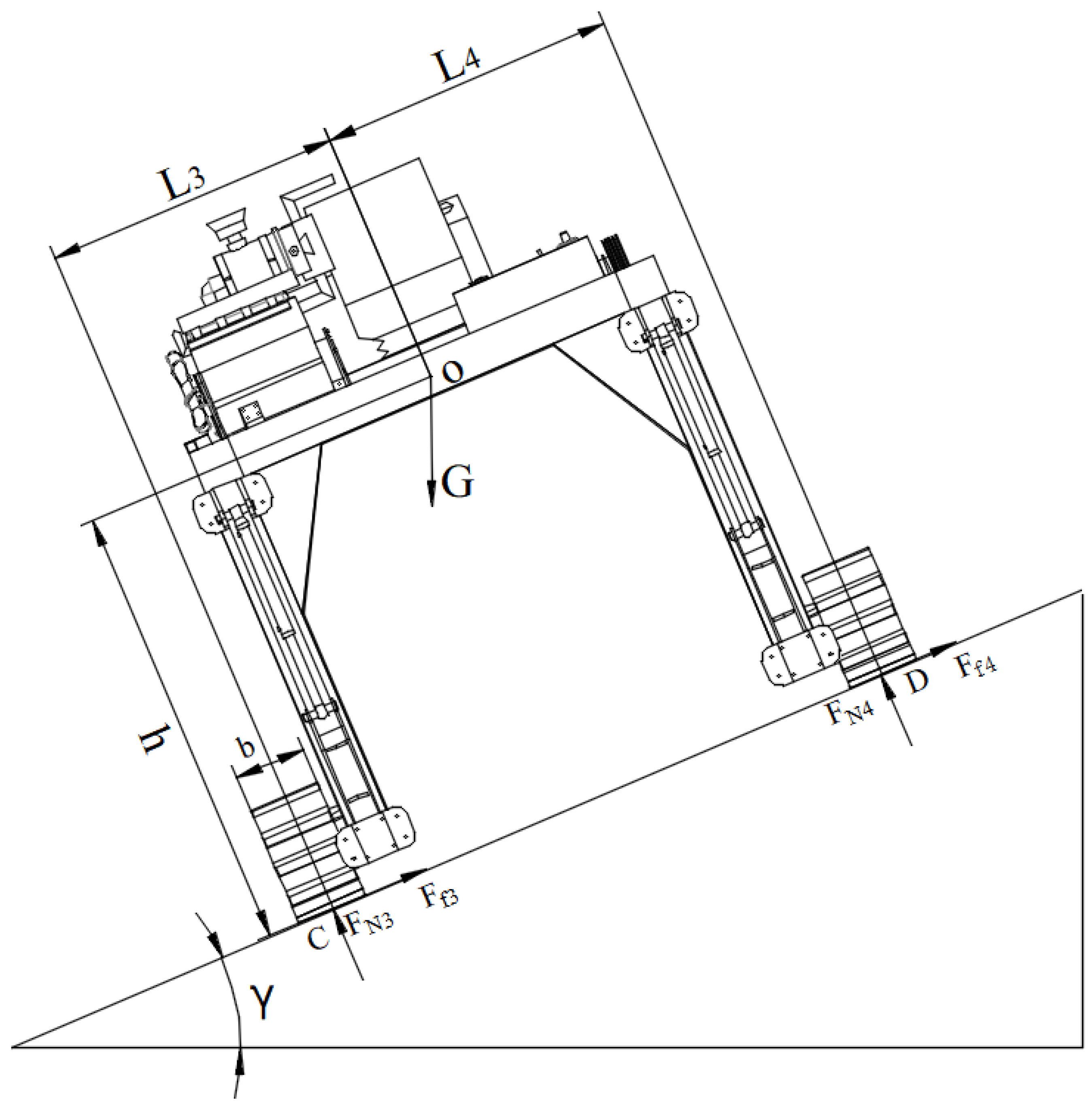
3.1. Whole Machine Stability Analysis

Due to the high center of gravity of the gantry-tracked self-propelled grape operation platform, it is necessary to analyze the stability of the entire machine to prevent tipping during operations or when driving on slopes. According to the direction of slope driving, stability analysis can be divided into longitudinal driving stability analysis and lateral driving stability analysis [19]. Since the machine uses tracked operation and drives at a constant speed when going uphill or downhill, the walking speed is relatively slow. Air resistance and mechanical losses in track transmission can be ignored in the stability analysis [20]. The main forces acting on the machine during stable driving on a slope include the gravity of the entire machine, the frictional resistance of the slope surface, and the normal reaction force supported by the slope surface [21].

3.1.1. Longitudinal Driving Stability Analysis

Longitudinal driving stability refers to the risk of the machine tipping forward or backward on slopes, depending on the support from the front and rear track wheels. The uphill limit tipping angle α and the downhill limit tipping angle β are used as evaluation indicators for longitudinal driving stability [22]. As shown in Figure 8.

When the gantry-tracked self-propelled grape operation platform drives uphill or downhill at a constant speed, external factors can be ignored, assuming it is stationary on the slope. As shown in Figure 8a, taking the moment at support point A, a mechanical equilibrium equation is established:

$$\{\begin{array}{l}{F}_{N1}+F_{N2}=G\cos \alpha \\ F_{f1}+F_{f2}=G\sin \alpha \\ Gh\sin \alpha +F_{N2}(L_1+L_2)=G{L}_{1}COS\alpha \end{array}$$

(11)

In the formula, F_N1—the supporting force of point A supporting wheel; F_N2—the supporting force of point B supporting wheel; F_f1—friction of the point A supporting wheel; F_f2—friction of the point B supporting wheel; h—the vertical distance from the center of gravity to the ground, using SolidWorks software (2020 version) to measure the center of gravity of the whole machine, h = 2.1 m; L₁—the horizontal distance from the center of gravity to point A; L₂—the horizontal distance from the center of gravity to point B; α, β—uphill and downhill limit tipping angle.

When driving uphill, the support force at the rear wheel (point A) is much greater than the support force at the front wheel (point B). When the support force at point B is zero (F_N2 = 0), the support force of the slope on the entire machine falls above point A, and the machine may tip longitudinally. At this point, the uphill limit tipping angle α is as follows:

$$\alpha =\arctan {\displaystyle \frac{L_1}{h}}$$

(12)

Similarly, the downhill limit tipping state of the machine is shown in Figure 8b, and the downhill limit tipping angle β is as follows:

$$\beta =\arctan {\displaystyle \frac{L_2}{h}}$$

(13)

Based on Equations (11)–(13), the uphill tipping limit angle α depends on two factors. The first is the horizontal distance from the center of gravity to point A. The second is the vertical distance from the center of gravity to the ground. Similarly, the downhill tipping limit angle β also depends on two factors. The first is the horizontal distance from the center of gravity to point B. The second is the vertical distance from the center of gravity to the ground. The larger the horizontal distances from the center of gravity to points A and B, the greater the uphill and downhill limit tipping angles, and the greater the machine’s ability to resist tipping. Using SolidWorks software to measure the horizontal distances from the center of gravity to points A and B, L₁ is 819.5 mm and L2 is 840.9 mm. The calculated uphill limit tipping angle α is 20.8°, and the downhill limit tipping angle β is 21.8°, both greater than the design requirement of 10°. Thus, the longitudinal driving stability requirements of the gantry-tracked self-propelled grape operation platform on slopes are met.

3.1.2. Lateral Driving Stability Analysis

Lateral driving stability refers to the risk of the machine tipping sideways. This depends on the tracks on both sides when driving or operating along the contour lines of a slope. The lateral limit tipping angle γ and the lateral limit sliding angle γ₁ are used as evaluation indicators for lateral driving stability [23], as shown in Figure 9.

When the gantry-tracked self-propelled grape operation platform drives at a constant speed on a lateral slope, external factors can be ignored, assuming it is stationary on the slope. As shown in Figure 9, taking the moment at support point C, a mechanical equilibrium equation is established:

$$\{\begin{array}{l}{F}_{N3}+F_{N4}=G\cos \gamma \\ F_{f3}+F_{f4}=G\sin \gamma \\ Gh\sin \gamma +F_{N4}(L_3+L_4)=G{L}_{3}COS\gamma \end{array}$$

(14)

In the formula, F_N3—the supporting force of the point C supporting wheel; F_N4—the supporting force of the point D supporting wheel; F_f3—friction force of the point C supporting wheel; F_f4—friction force of the D point supporting wheel; L₃—the horizontal distance from the center of gravity to point C; L₄—the horizontal distance from the center of gravity to point D.

When the machine is driving on a slope, the support force on the lower side is much greater than the support force on the higher side. When the support force at point D is zero (F_N4 = 0), the support force of the slope on the entire machine falls above point C, and the machine may tip laterally. At this point, the lateral limit tipping angle γ is as follows:

$$\gamma =\arctan {\displaystyle \frac{L_3}{h}}$$

(15)

When driving on a lateral slope, the machine may also experience sliding. To prevent lateral sliding, according to the force analysis of the machine’s lateral stability, lateral sliding should not occur if the following condition is met:

$$F_{f3}+F_{f4}=G\sin {\gamma }_1\le \mu G\cos {\gamma }_1$$

(16)

In the formula, μ is the ground adhesion coefficient, taken as 0.6 [14]. The maximum lateral limit sliding angle γ₁ can be calculated as:

$${\gamma }_1\le \arctan \mu$$

(17)

According to Formulas (14)–(17), when the machine operates or travels along the contour line of a slope, the lateral critical tipping angle γ is related to the horizontal distance from the center of gravity to the supporting point of the track on the lower side. The maximum critical sliding angle γ₁ is related to the ground adhesion coefficient. The greater the horizontal distance from the center of gravity to the supporting point of the track on the lower side, the larger the lateral critical tipping angle γ and the ground adhesion coefficient μ, thereby increasing the machine’s ability to resist lateral tipping and sliding. Using SolidWorks software, the horizontal distance from the center of gravity to point C was measured, resulting in L₃ being 1400 mm. The calculations show that the lateral critical tipping angle γ of the machine is 33.4°, and the maximum critical sliding angle γ₁ is 30.9°. To prevent lateral sliding, the machine’s lateral critical tipping angle γ should be less than the maximum critical sliding angle γ₁. Therefore, when γ ≤ 30.9°, the operation is relatively stable and no side slip or rollover will occur. When γ ≥ 33.4°, the entire vehicle will roll over horizontally.

3.2. Field Test

3.2.1. Maximum Driving Speed Test

According to the design and selection of the gearbox, the machine has six gears. As shown in Figure 10, a straight test field was selected, and the highest gear (6th gear) was engaged. The machine entered the test field at full throttle. The maximum driving speed of the machine was measured by driving back and forth over a distance of 100 m. A stopwatch was used to measure the time taken for a single trip of 100 m, and the average value was used to determine the maximum driving speed.

According to

Table 3. Test results of maximum driving speed in each gear.

Gear	Time of Use (Min)	Theoretical Velocity (km/h)	Actual Velocity (km/h)	Percentage of Consolidation of Deviation
Gear 1	8.8	1	0.68	32%
Gear 2	6.4	1.3	0.94	27.69%
Gear 3	4.2	1.7	1.42	16.74%
Gear 4	3.2	2.1	1.83	12.86%
Gear 5	2.8	2.6	2.12	18.46%
Gear 6	1.5	4	3.85	3.75%

, the selection of the engine and gear positions for the gantry track-mounted self-propelled grape operations platform is reasonable, enabling the machine to perform both walking and operational tasks. During the tests, the speeds and times for gears 1 through 6 were measured. The actual speed for the lowest gear (1st gear) was 0.68 km/h, and the actual speed for the highest gear (6th gear) was 3.85 km/h. Due to mechanical and slippage losses in the track-mounted chassis, the actual tested speeds were lower than the theoretically calculated speeds. As observed from the speed variations in

Table 4. Minimum turning radius test results.

Diversion	Angle	Testing Radius (m)	Mean Value (m)	Minimum Turning Radius (m)
Levoversion	120°	3.8	3.9	3.95
	240°	4.0
	360°	3.9
Dextroversion	120°	3.9	4.0
	240°	4.1
	360°	4.0

, the higher the traveling speed, the lower the deviation rate, which indicates better speed stability.

3.2.2. Minimum Turning Radius Test

A dry, hard, and level field with a slope ≤3% was selected for testing, as shown in Figure 11. The machine entered the test field at the lowest gear speed, performing differential braking on both tracks to conduct left and right circular movements at the lowest gear speed, completing one full circle. The turning radius was measured at every 120° interval, and the average value was calculated.

From Table 4, it can be seen that the track design of the gantry crawler self-propelled grape operation platform is reasonable, with an average minimum turning radius of 3.95 m. The designed track driving device can meet the differential braking turning requirements in the field. Compared with the theoretical calculation, the actual measured turning radius is slightly larger due to track slippage and skid motion, but it can still meet the turning needs of vineyard field operations.

3.2.3. Longitudinal Driving Stability

Test A slope ranging from 5° to 25° with a length of 30 m was selected in the test field, with an on-site wind speed ≤1.5 m/s. The machine entered the slope at the lowest gear speed and increased the gear according to the driving conditions. The contact between the front support points of the tracks and the slope was observed, and a safety device to prevent longitudinal tipping was set up at the front of the machine. The climbing angle was measured using an angle-measuring instrument. When there was a gap between the front support points of the tracks and the slope, the machine stopped advancing, and the maximum angle was measured, as shown in Figure 12.

From

Table 5. Longitudinal driving stability test results.

Gradient	Passing Distance (mm)	Left Track Ground Clearance (mm)	Right Track Ground Clearance (mm)
5°	10	0	0
10°	10	0	0
13°	10	0	0
18°	10	1.5	0
19°	3	3	5

, it can be seen that the machine climbed the slope sequentially from the minimum to the maximum slope at the lowest gear speed. The field climbing test showed that the machine could climb a 10° slope, but when the climbing angle exceeded 18°, a gap appeared between the track support surface and the slope. Therefore, the maximum climbing angle was determined to be 18°, which is slightly smaller than the theoretical calculation but meets the stability requirements for field slope operations.

3.2.4. Lateral Driving Stability

Test A slope ranging from 20° to 35° with a length of 20 m was selected in the test field. The machine drove along the contour lines of the slope at different angles, observing the ground clearance of the track on the high side of the slope. When both tracks were in close contact with the slope and successfully passed the preset slope, the slope angle was measured using an angle-measuring instrument. A safety device to prevent longitudinal tipping was set up on the side of the machine, as shown in Figure 13.

From

Table 6. Lateral driving stability test results.

Gradient	Passing Distance (mm)	Low Side Track Clearance (mm)	The High Side of the Track Has Ground Clearance (mm)
20°	10	0	0
22°	10	0	0
24°	10	0	0
26°	10	0	0
28°	2	0	2

, it can be seen that the machine entered the slope at the lowest gear speed. The field lateral stability test showed that when the machine drove to a 28° slope, a gap appeared between the track support surface and the slope on the high side of the slope. The maximum lateral driving angle was determined to be less than 28°. When the slope angle was 26°, the lateral driving stability requirements were met, which is slightly smaller than the theoretical calculation.

3.2.5. Hitch Lifting Capacity

The test of the hitch lifting capacity is the determination of the maximum lifting capacity of the hydraulic cylinder. A level test field was selected, as shown in Figure 14. The heaviest operating component was hitched to the sliding sleeve, and loading and lifting were performed at six equally spaced points within the full lifting stroke, including the highest and lowest points. The vertical height of the loading points was measured, and the change in vertical height was observed every 10 min within 30 min.

From

Table 7. The test results of the lifting capacity of the hanging device.

Improve the Quality (kg)	Cylinder Stroke (mm)	10 Min Height Change (mm)	20 Min Height Change (mm)	30 Min Height Change (mm)	Total Settlement Change (mm)	Average Settlement (mm)
558	0	0	0	0	0	1.58
	150	0	0.5	1	1.5
	250	0	0.8	1.2	2
	350	0	0.6	0.8	1.4
	450	0.1	0.7	1.1	1.9
	550	0.3	1	1.4	2.7

, it can be seen that the lifting capacity test was performed with the heaviest operating component, the soil-clearing rotary plow device, which weighs 558 kg. The designed hitch device can complete the lifting operation with a reasonable structural design. The maximum stroke of the hitch hydraulic cylinder is 550 mm, with an average cumulative settlement of 1.58 mm within 30 min and lifting height stability of 99.7%, meeting the lifting requirements for the hitched operating components.

4. Conclusions

(1)

For the cultivation of wine grapes using a vertical trellis system, traditional operations powered by tractors often result in a wide range of equipment types and low efficiency. To address this issue, a multifunctional operation platform based on a gantry mechanical structure has been developed. This involved the design and calculation of key components, and a prototype was trial-manufactured. The equipment is capable of performing bilateral operations simultaneously, achieving twice the efficiency of traditional machinery. With the development of additional operational components, this platform not only boosts efficiency but also reduces the mechanical investment costs for grape growers.

(2)

The power from the diesel engine is transmitted using a transfer case combined with a power take-off unit. The walking device uses a track system with a track width of 379 mm, a track support surface length of 2.4 m, and a track gauge of 2.8 m, providing good ground pressure distribution without causing secondary soil compaction. Considering that frost protection and soil clearing operations are the most power-consuming tasks, the total power requirement for the machine was determined to be 141 kW. A 6-speed gearbox was selected based on calculations to meet the speed requirements for different operational stages.

(3)

Field performance tests showed that the gantry track-mounted self-propelled wine grape operations platform has a maximum traveling speed of 3.85 km/h, a minimum turning radius of 3.95 m, a maximum longitudinal climbing angle of 18°, and a maximum lateral angle of 26°. The average settlement of the heaviest operational component over 30 min was 1.58 mm, with lifting height stability reaching 99.7%, thus meeting the requirements for field operations.