Re-Evaluation of Fatigue Thickness Effect Based on Fatigue Test Database
Abstract
:1. Introduction
- (1)
- Introduce the concept of thickness correction factors by Gurney and in current standards and then relate these factors to general physical or statistical causes.
- (2)
- Present relevant types of typical weld geometry for S-N curve classifications and test data grouping.
- (3)
- Conduct a literature review of thickness correction factors for the associated joint types and in general terms.
- (4)
- Review the fatigue test database from tests conducted in the last 40 years and select test data with sufficient data integrity for further statistical analysis.
- (5)
- Conduct statistical analysis of fatigue strength with different thickness groups and different S-N curve categories to gain insight into the uncertainties of fatigue strength associated with individual thickness.
2. Literature Review
2.1. Overview of Thickness Effect and Correction
- Size effect and effective thickness.
- Revision of the power exponent k.
- Statistical: Thicker plates can have a higher probability of imperfection to start crack initiation.
- Fabrication: Residual stress of a thicker plate is higher due to more restraint.
- Geometry: The main geometric cause is that a thicker plate has a lower stress gradient across the thickness than a thinner plate. The other two causes can be that a thicker plate may have a relatively smaller radius (ai = ai, but ai/t1 < ai/t2, [8]) of the welding profile at the weld toe, and a thicker section can introduce a plane strain state. Due to the presence of a steeper stress gradient in a thinner section, a small crack (notch) close to the surface of a small specimen will experience a lower strain than a small crack (notch) close to the surface of a thick specimen, for the same stress at the surface. Overall, the geometry effect was considered as the dominant cause of the thickness effect.
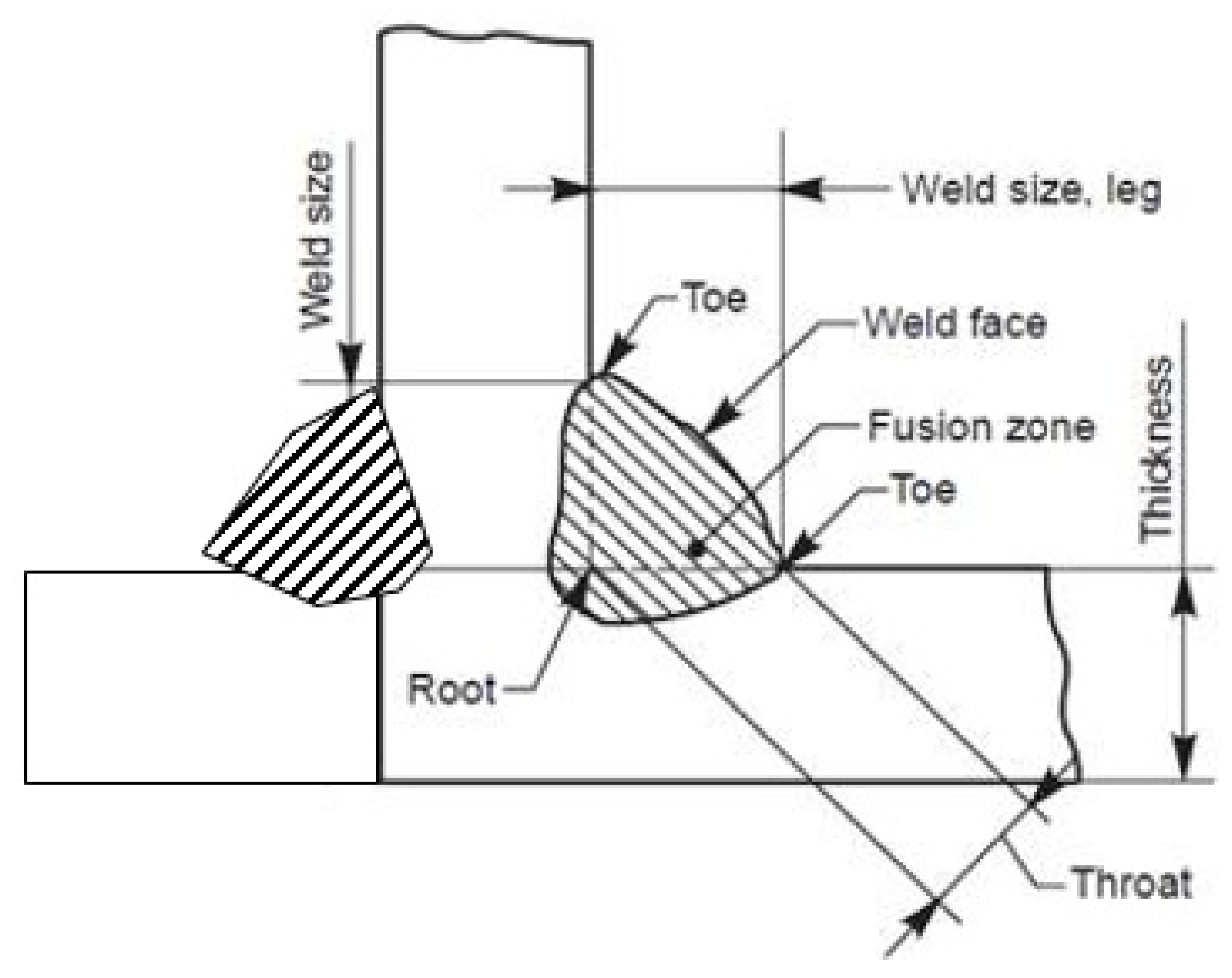
2.2. Typical Joints in Current Standards
- T-joints.
- Transverse butt-welded joints.
- Tubular joints.
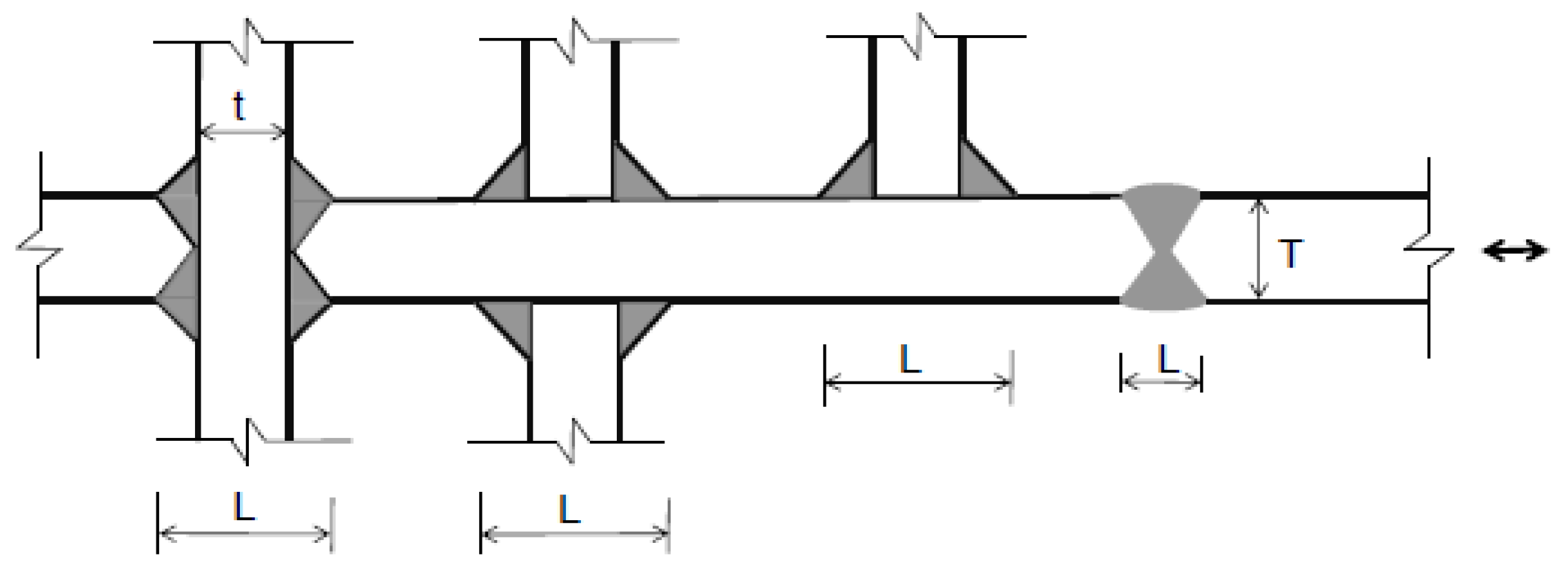
2.2.1. T-Joints
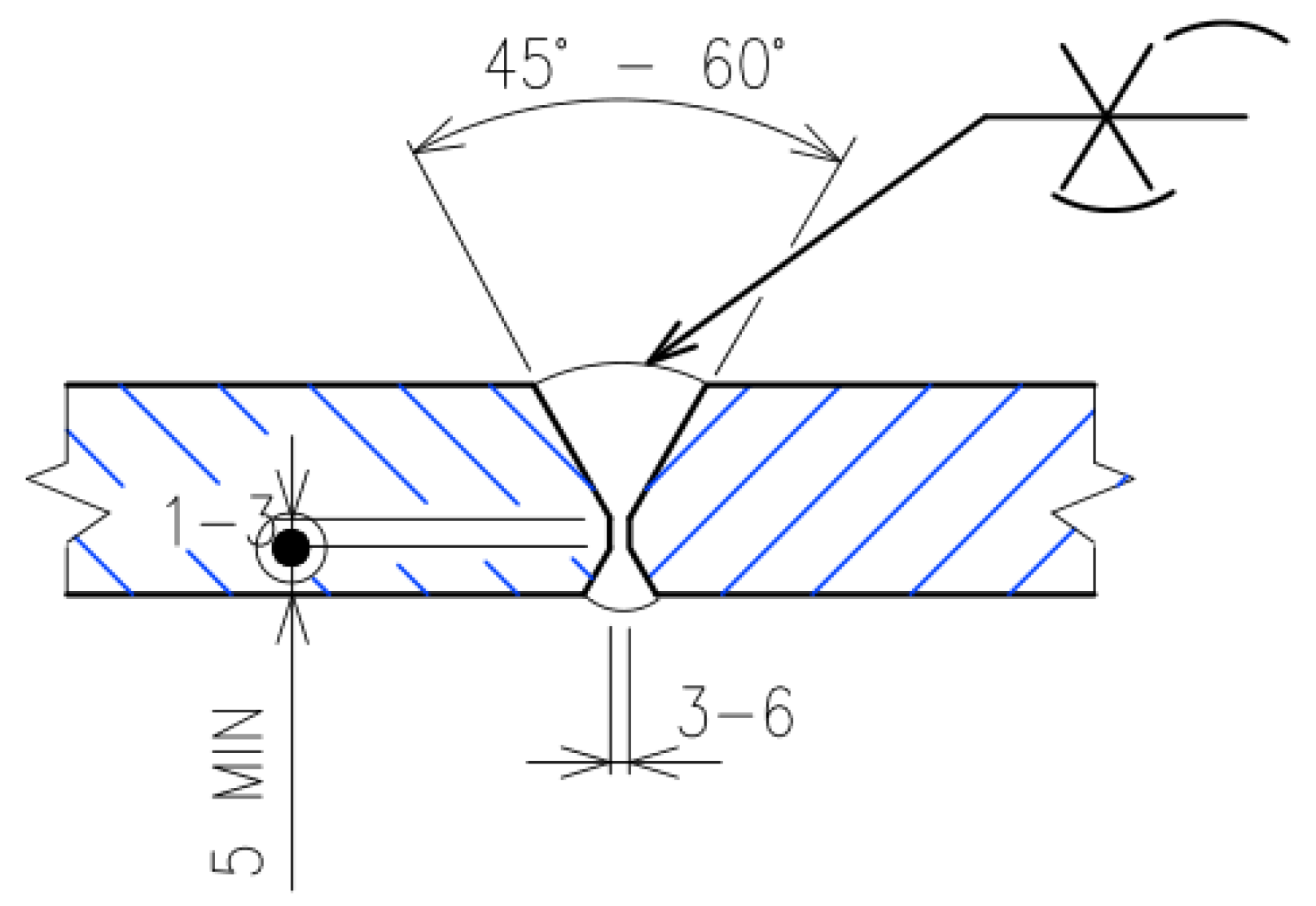
2.2.2. Transverse Butt-Welded Joints
2.2.3. Tubular Joints
2.3. General Uncertianties of Thickness Correction Factors
- Not all factors affecting fatigue strength are scalable or proportionable, such as corrosion effect.
- The basis for scale may not be the thickness alone, as weld width and attachment length will also contribute, known as the size effect.
- The scale can be different for different weld classes and not uniform for the same S-N category.
2.4. Effective Thickness for Size Effect
2.5. Revision of Power Exponent k
3. Fatigue S-N Database
- The quality of each individual test is satisfactory, i.e., certified weld procedure and weld quality.
- All entries in the database are based on clearly recorded test parameters, such as:
- Definition of loading, e.g., R-ratio, CA/VA loading, frequency;
- Material;
- Type/geometry and thickness of specimen;
- Condition of cathodic protection;
- Condition of corrosive environment;
- Fabrication details, e.g., weld profile, treatment etc.;
- Temperature.
- Definition of failure criteria and the associated number of cycles.
- Type of stress range, i.e., hotspot stress or nominal stress.
3.1. Literature Review of Fatigue Test Programmes
- HSE RPFG [30]
- UKOSRP I and II (Corrosive Environment) [19]
- Other studies showed a more marked reduction of fatigue life under free corrosion.
- The UKOSRP results were the only ones suggesting CP completely restored fatigue life.
- 0.93 for K butt weld.
- 0.88 for longitudinal fillet welds.
- TWI-SINTEF/UCL/Nordic Program/US Highway [17]
- University of Waterloo [25]
- FATHOMS [38]
- Vaessen et al. [18]
- Olafsson [39]
- Huang et al. [32]
- Various Studies from Pedersen [8]
- Li et al. [34]
- Maddox et al. [34]
- Kawasaki Steel Corp. [23]
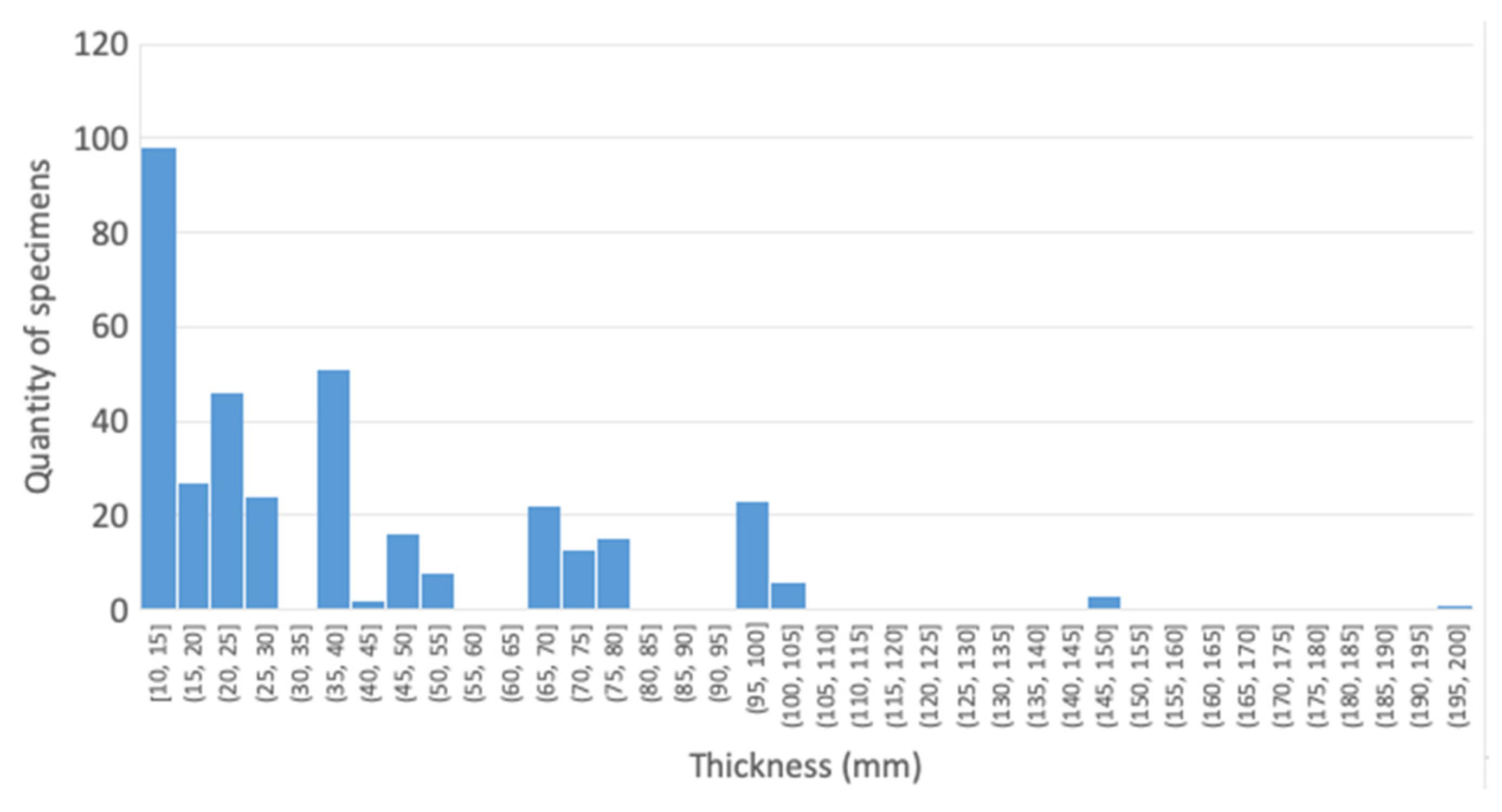
3.2. Collated Fatigue Database
4. Evaluation of Thickness Effect
4.1. Analysis Method
4.2. Data Analysis
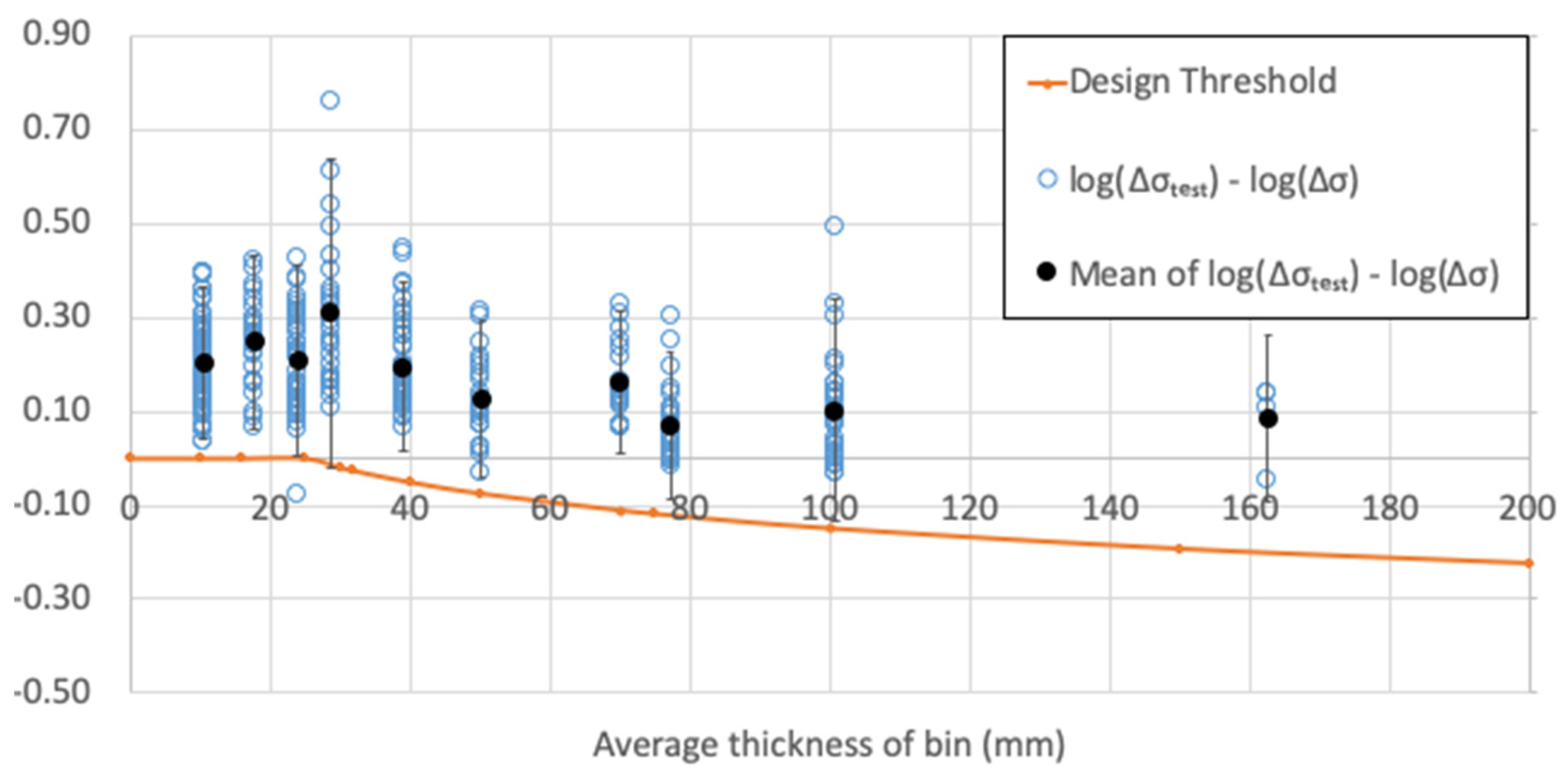
4.2.1. T-Joints
In Air
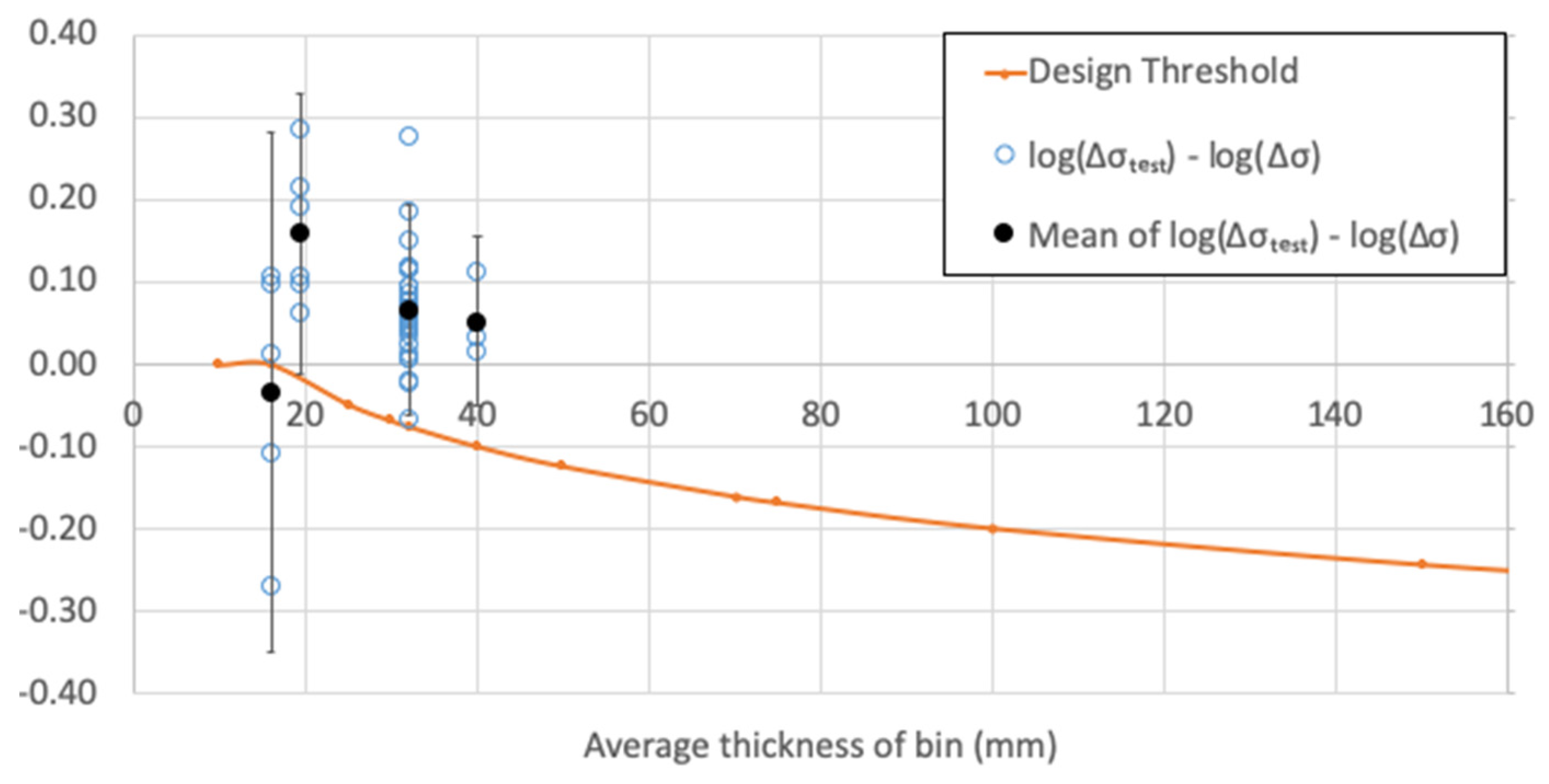
- The mean fatigue strengths start decreasing beyond the reference thickness of 25 mm but appear to level off after 50 mm with increasing thickness;
- With increasing thickness, the lower bound of test data, corresponding to mean –2 × standard deviation, decreases in general but is higher than the design threshold. Consideration should also be given for the statistical uncertainties due to the lack of data in some data groups;
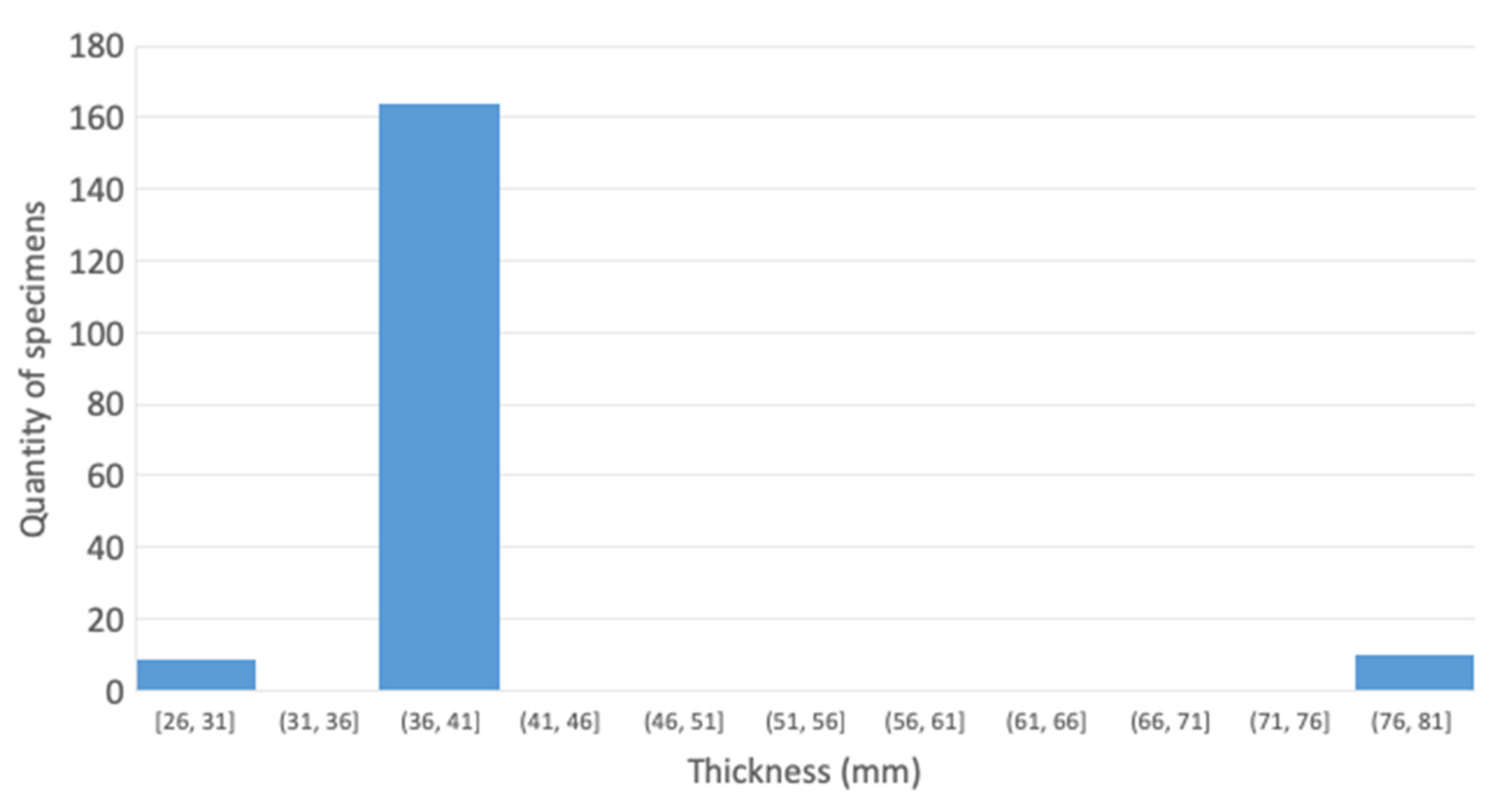
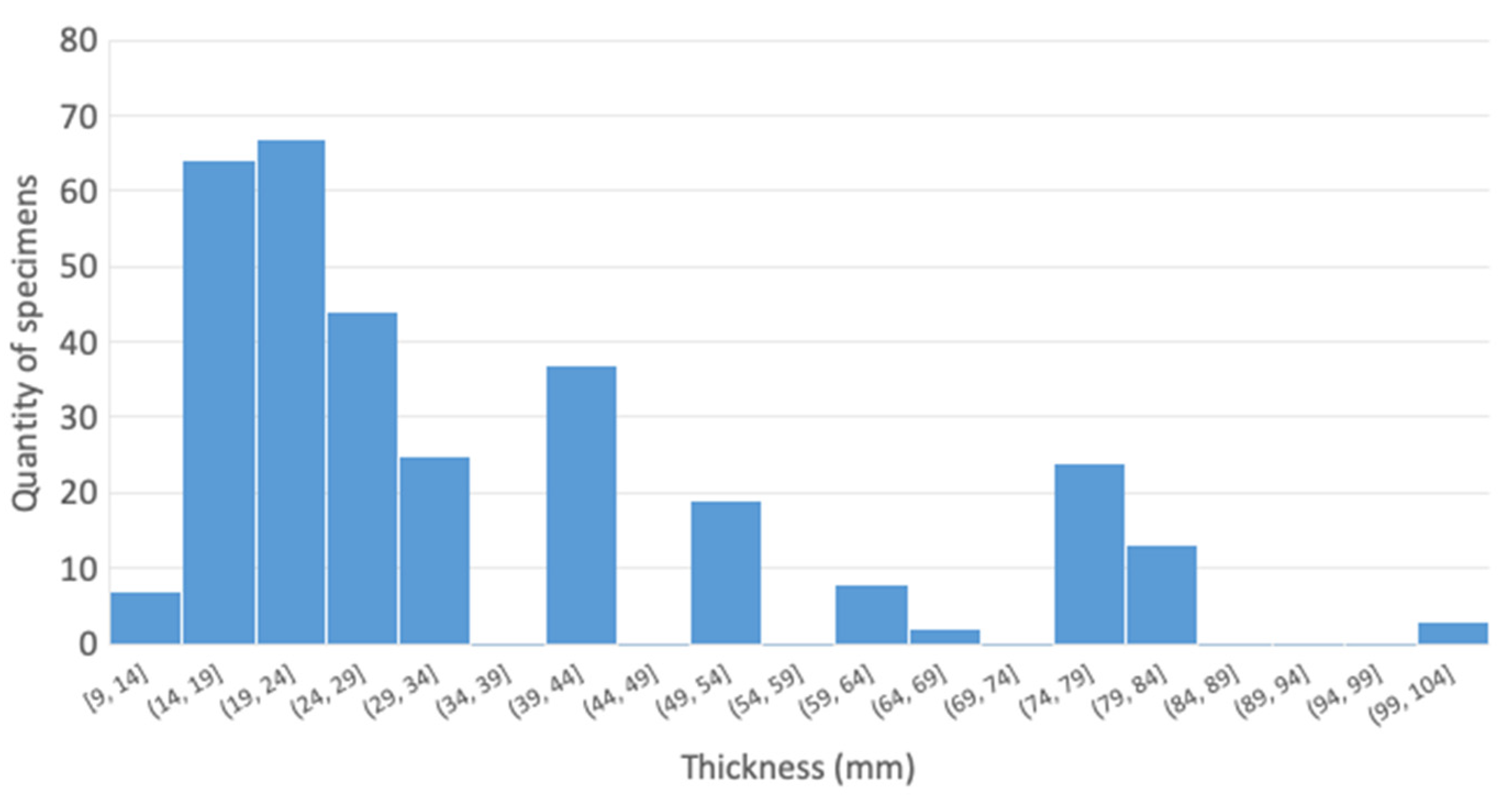
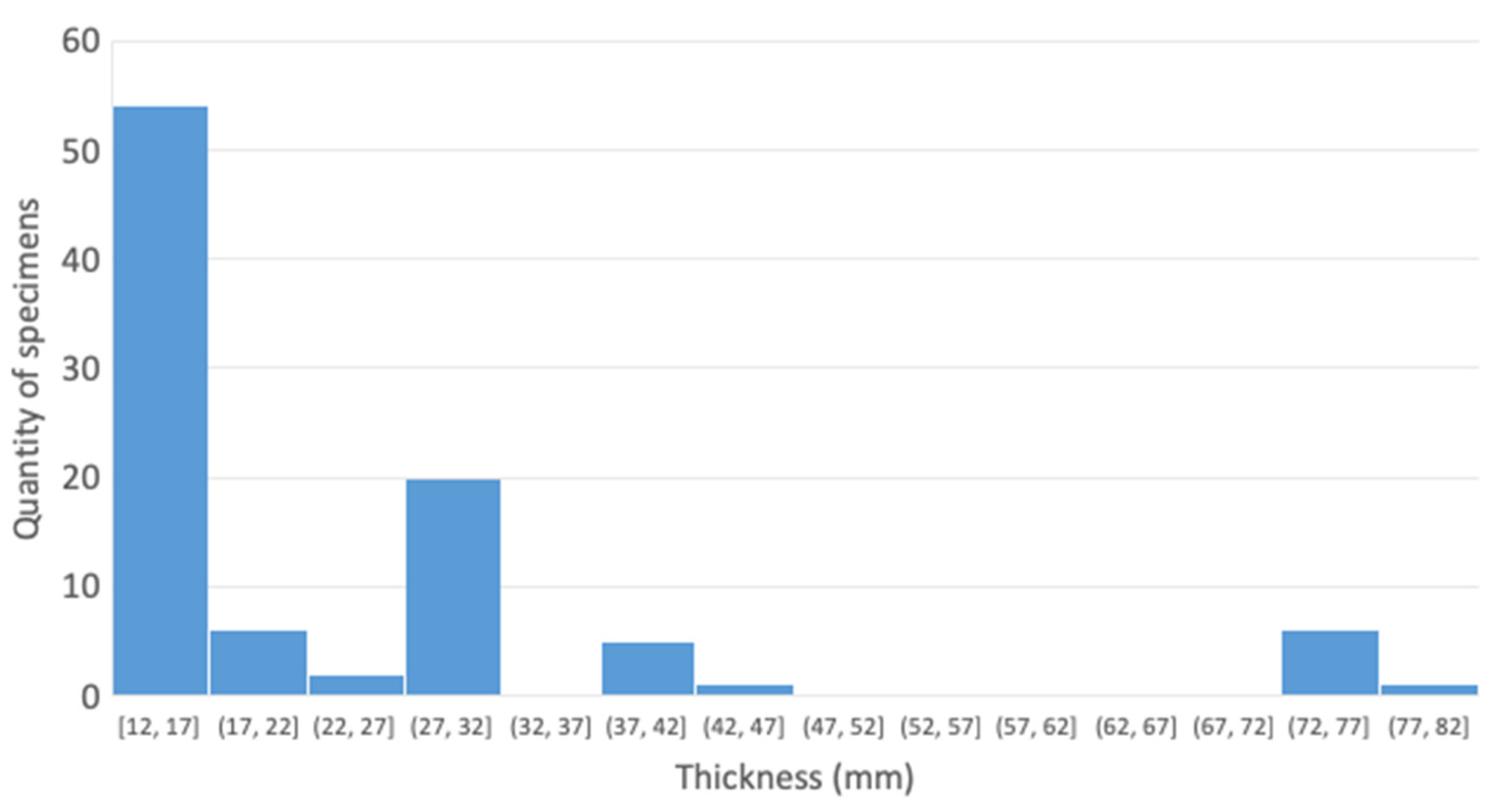
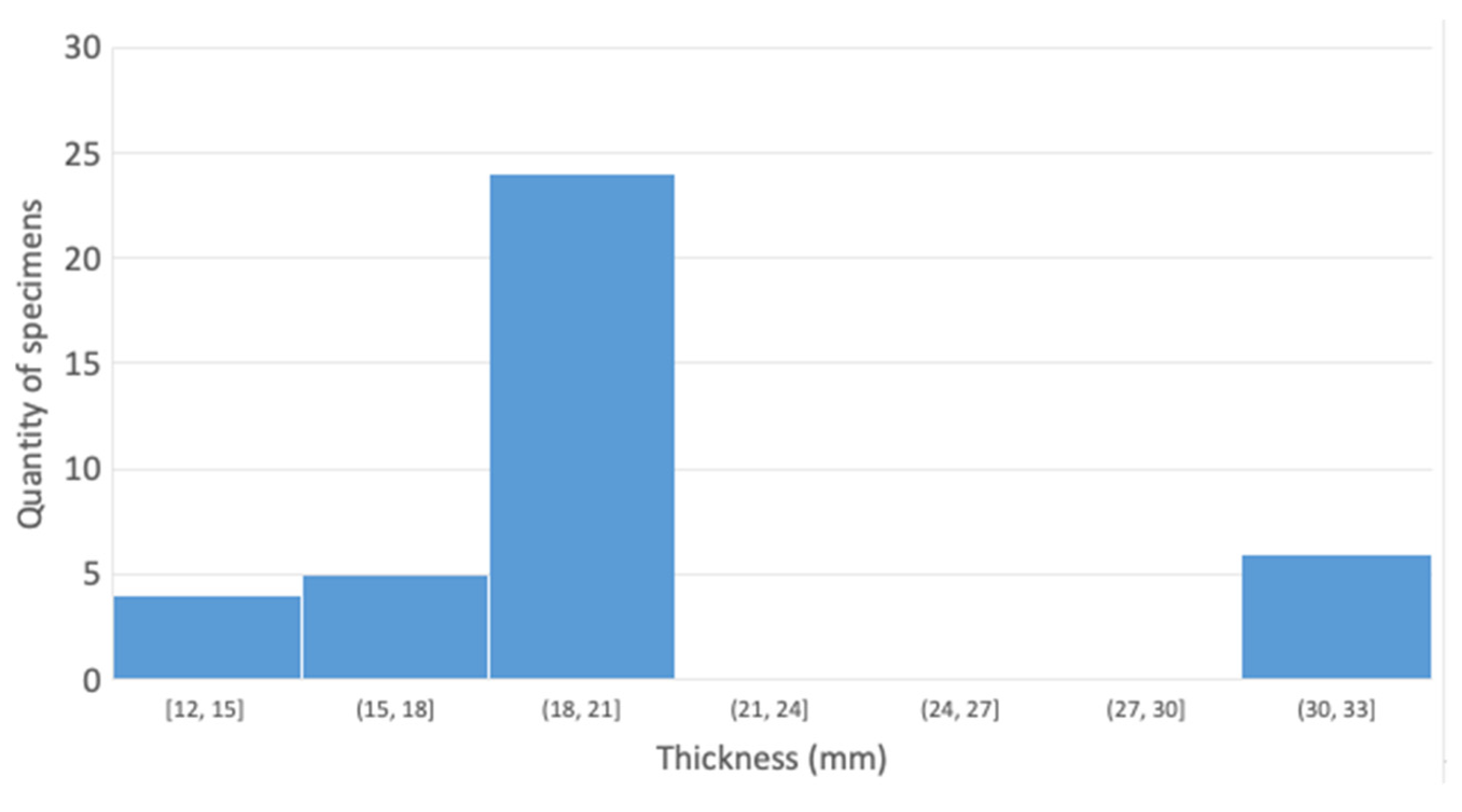
- Test data are concentrated in the small thickness range (T <50 mm), but there are still sizable amounts of data in the thickness range greater than 50 mm.
Cathodic Protection
- With increasing thickness, the few data groups do not indicate that the mean fatigue strength follows a monotonic relation. This may imply that the thickness effect may not exist or does not play a dominant role, where other factors may affect the performance to a greater extent compared to the in-air condition;
- With increasing thickness, the fatigue strength at a lower bound is consistently higher than the design threshold, meaning the design curve is conservative;
- A lack of test results imposes large uncertainties at the system level as well as the local level in the first group and the third group.
Free Corrosion
- With increasing thickness, the mean fatigue strength does not follow a monotonic relation. This may imply that thickness effect may not exist or does not play a dominant role, where other factors may affect the performance to a greater extent compared to the in-air condition;
- With increasing thickness, the fatigue strength at the lower bound is consistently higher than the design threshold, meaning the design curve is conservative, and much more than that for the in-air condition;
- A lack of test results imposes large uncertainties in the higher thickness region.
4.2.2. Transverse Butt-Welded Joints
In Air
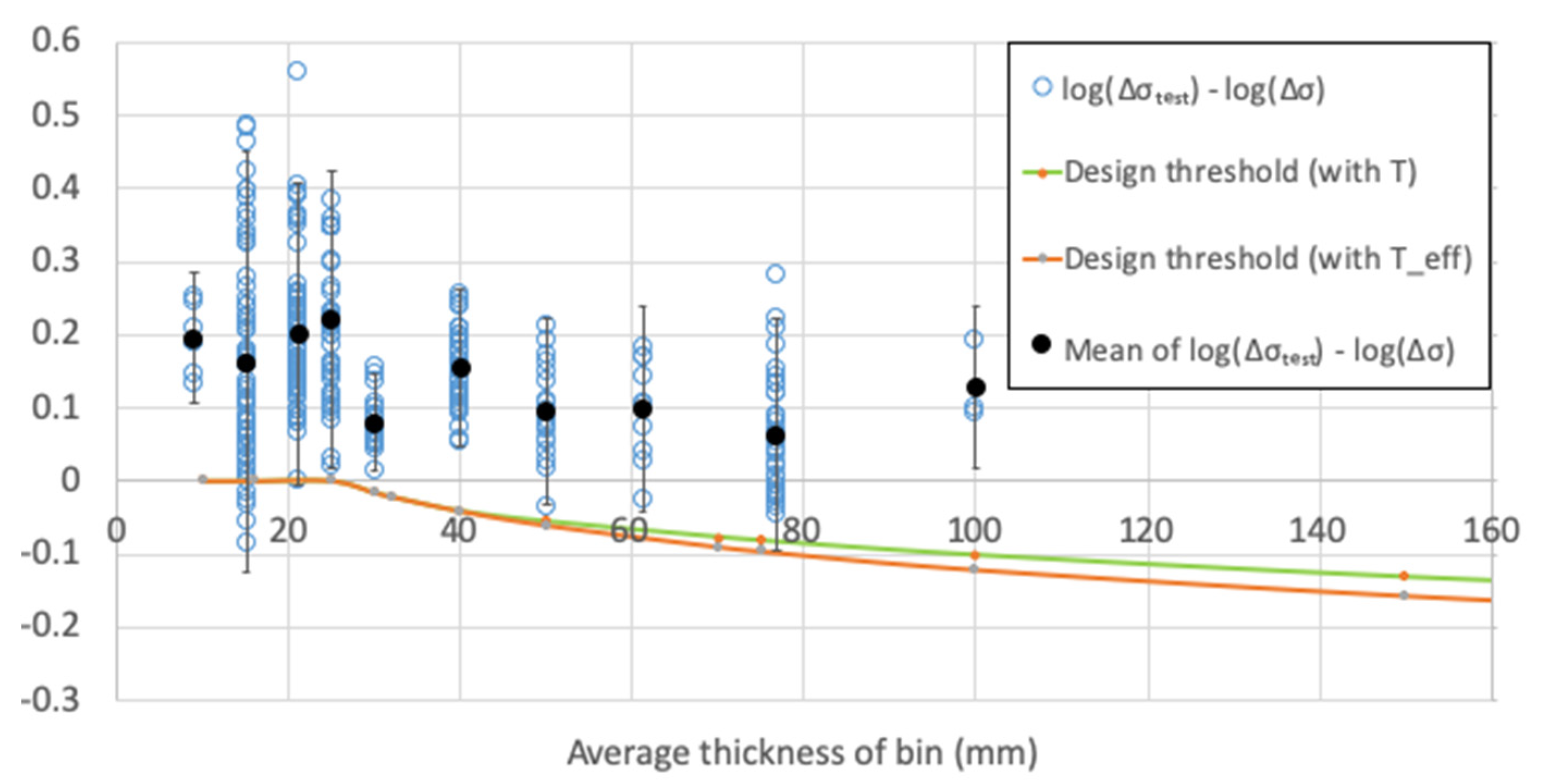
- With increasing thickness after the reference thickness, the mean fatigue strengths initially drop but level off after 50 mm;
- With increasing thickness after the reference thickness, the fatigue strengths at the lower bound are mostly above the design threshold, meaning that the design curve is conservative;
- Test data are concentrated in the small thickness range (T ≤ 40 mm);
- As the attachment length (L) for the double-sided transverse butt weld can be taken to be equal to the plate thickness if the weld prep profile is 45°, the effective thickness can be made smaller. Conservatism at a higher thickness can be reduced, as shown by the green line in Figure 12.
Cathodic Protection
- The mean fatigue strengths increase against the increase in thickness;
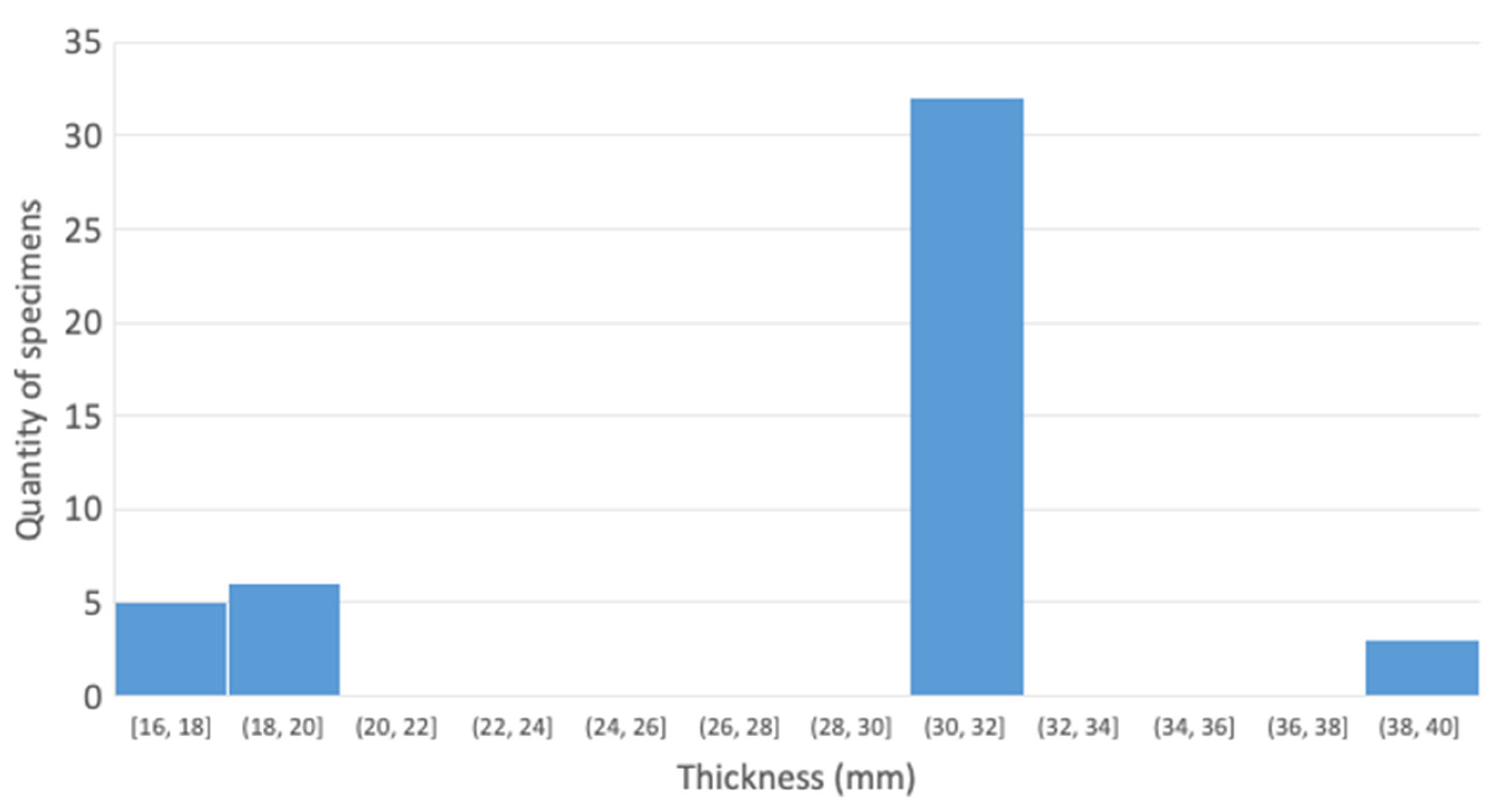
- There is a lack of data in the high thickness region, thus statistical uncertainty may be high.
Free Corrosion
- The mean fatigue strengths decrease against the increase in thickness;
- There is a lack of data in all thickness regions, thus statistical uncertainty may be high.
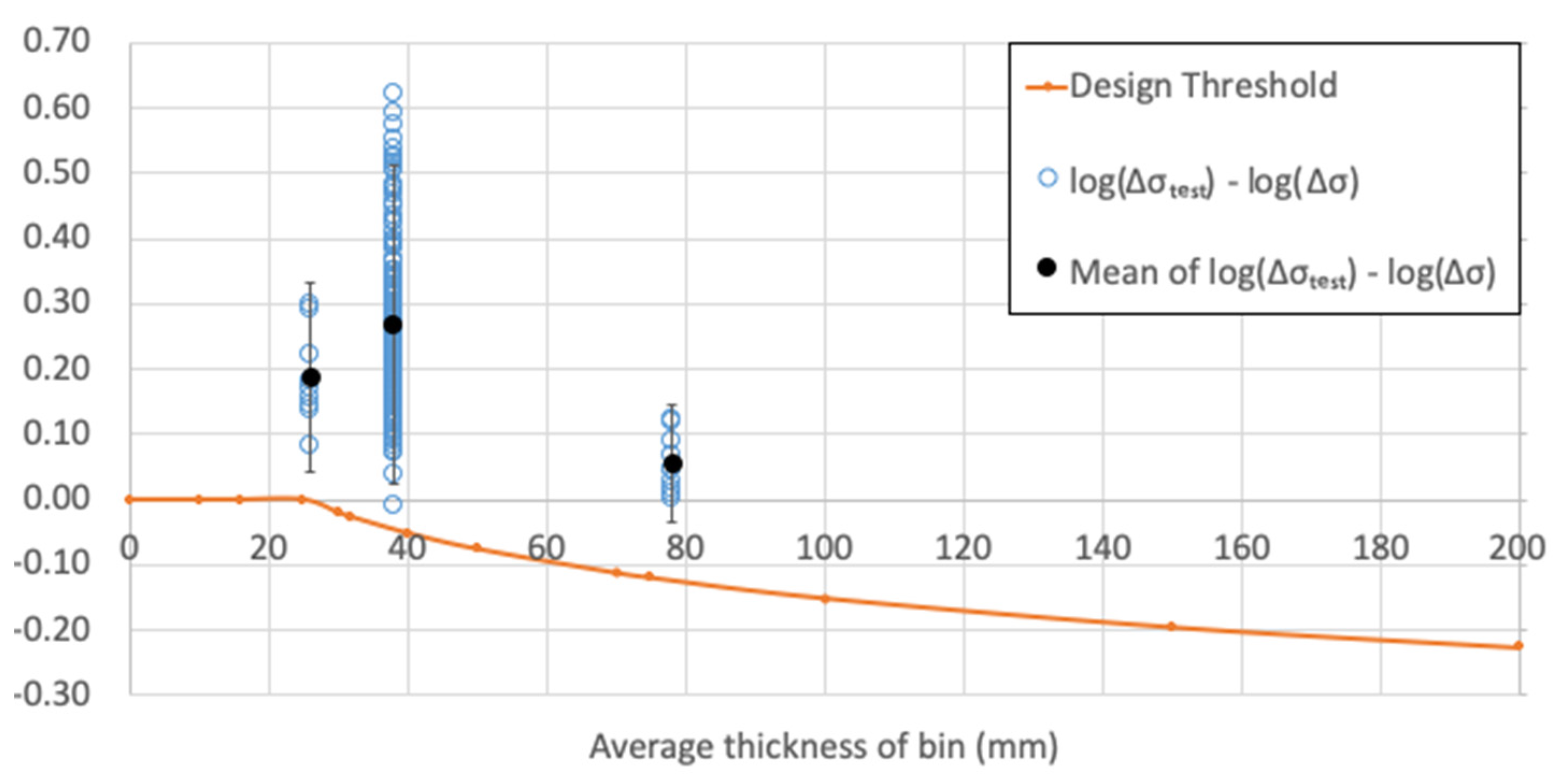
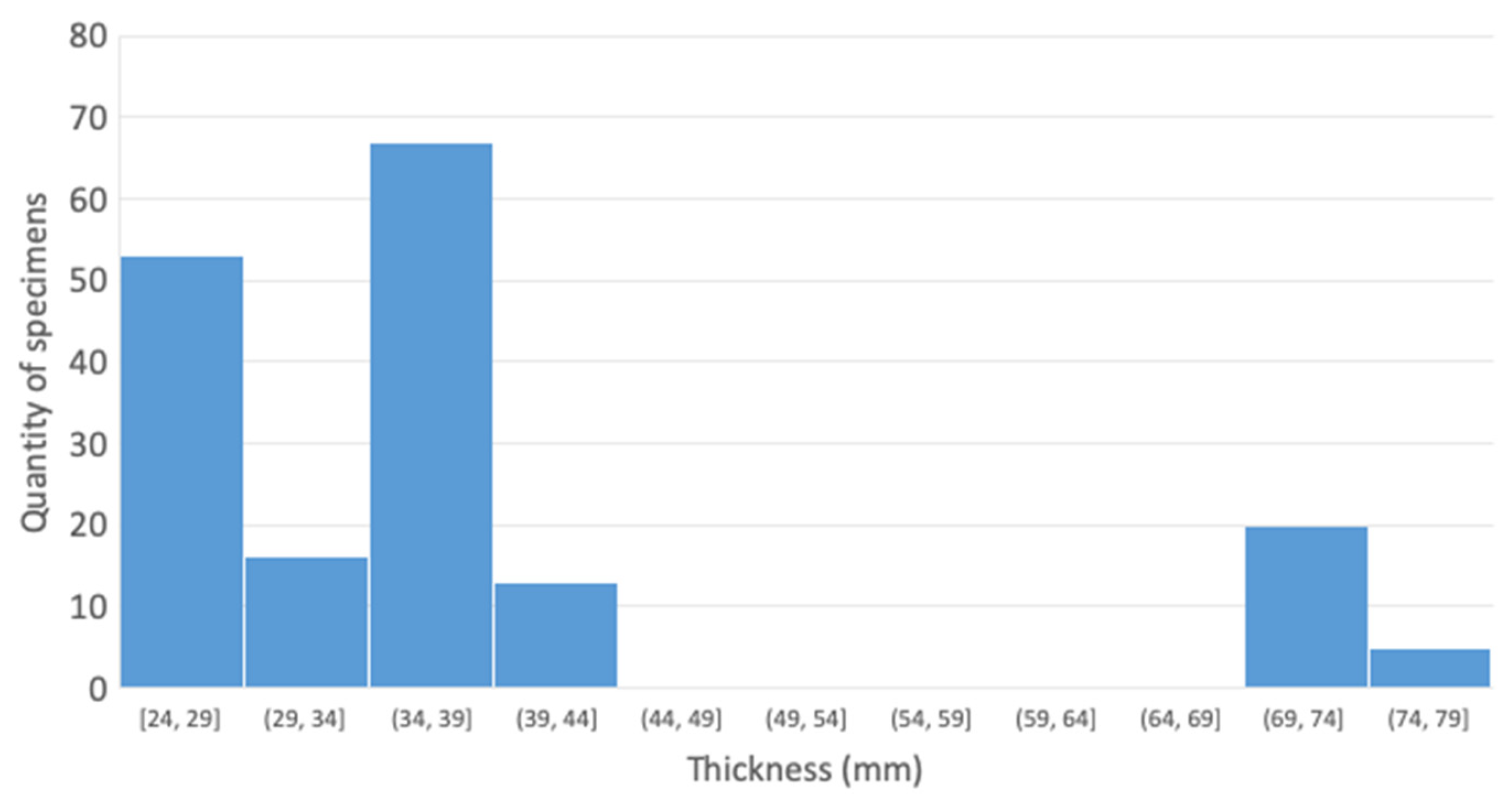
4.2.3. Tubular Joints
In Air
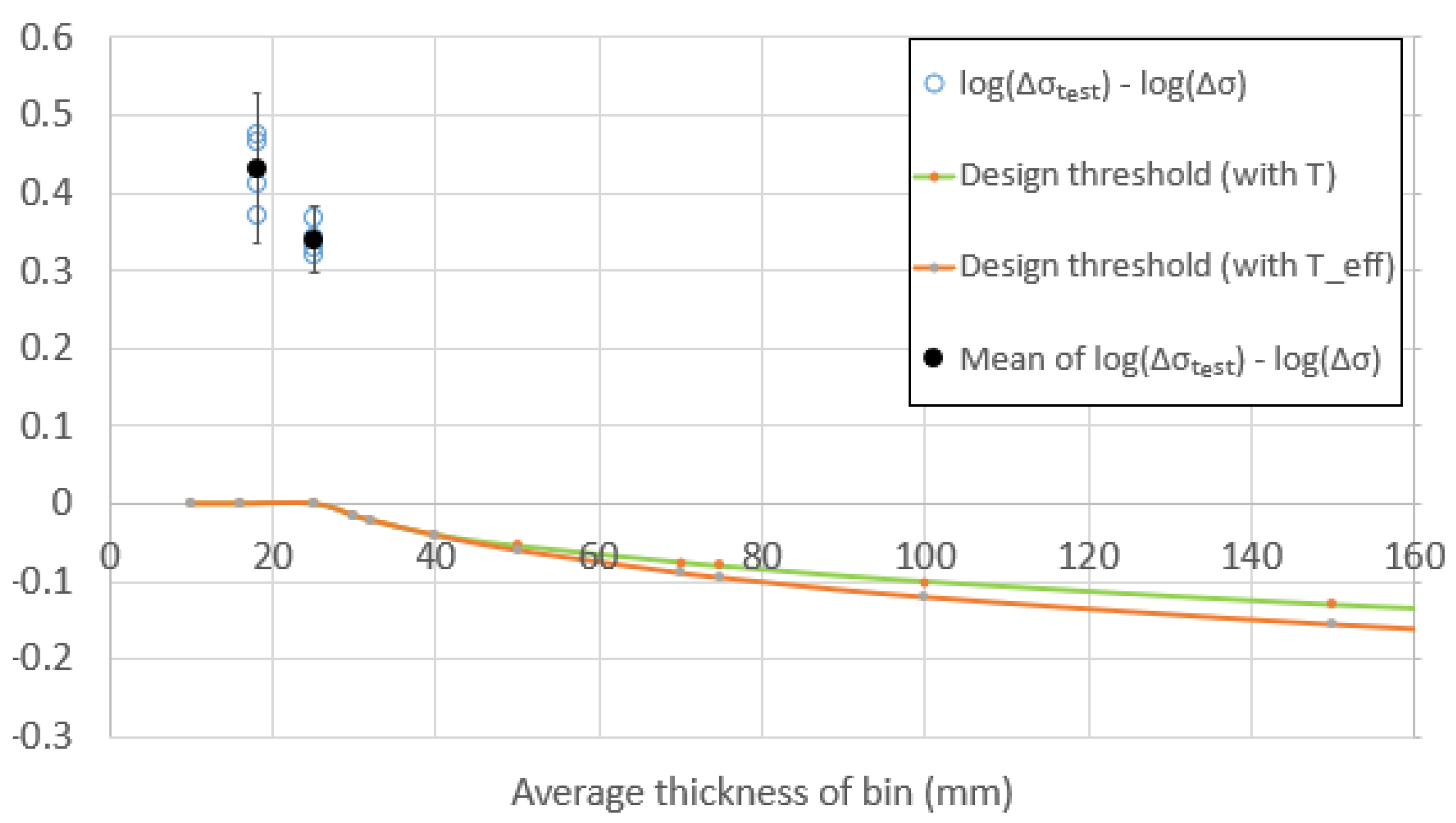
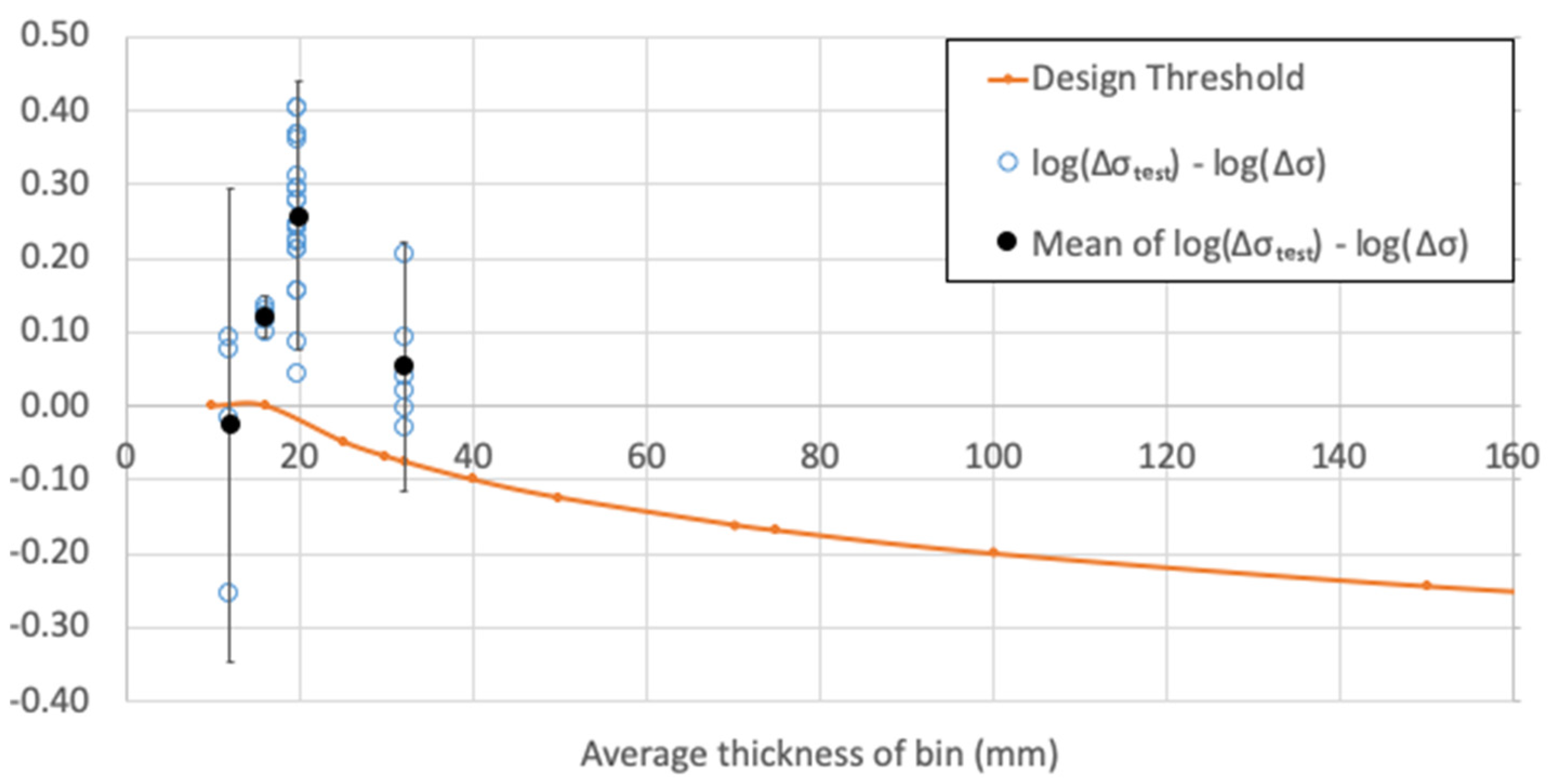
- As the lower bound for some high thickness bins lies below the design threshold, the design curve is not entirely at the conservative side according to these data. This phenomenon may be due to a number of reasons, one of which could be the statistical bias because of lack of data;
- The mean fatigue strength of the last two groups seemed to level off at the higher thickness range.
Cathodic Protection
- The mean fatigue strengths decrease against the increase in thickness, despite the first bin of data;
- Except the first bin of data, the lower bound of the rest of the data fits the design threshold quite well before 32 mm, but is slightly conservative after 32 mm. This may be due to the statistical uncertainty of the last bin at 40 mm that contains only three data entries.
Free Corrosion
- Most of the data entries fall above the design threshold, despite the first bin of data at 12 mm, which has a larger dispersion. This phenomenon could be induced by the outlier data entries;
- There is a lack of data in most thickness regions, especially for higher thicknesses.
5. Discussion
- The decrease in fatigue strength is less severe than predicted using the general thickness correction factor when the thickness is greater than 40 mm in a non-corrosive environment. This trend appears to agree with the proposed modification in the latest DNVGL-RP-C203 [4], as shown in the recent work by Lotsberg [13] which modifies this formula to consider the size effect. However, the size effect equation cannot be used for evaluation with the current test dataset because of a lack of information on the additional dimensions, such as the attachment length L in the original test conditions;
- For the limited data available for corrosion fatigue tests, analysis shows the current standards are on the conservative side, but with varying degrees of conservatism in different thickness ranges. This phenomenon can be attributed to the following:
- ○
- Statistical uncertainties due to the number of the data points;
- ○
- There are multiple factors affecting corrosion fatigue in each of the different tests, hence greater scatter. A cleaned data base with uniform attributes may help, but the quantity of data will be significantly reduced for each of the groups;
- ○
- The non-proportional aspect of corrosion fatigue may be dominant which diminishes the effects of thickness.
- The mean fatigue strength levels off when thickness is greater than 40 mm in a non-corrosive environment;
- Although there is high statistical uncertainty due to a lack of data for thicker specimens, fatigue strength based on this database gives slightly less conservative values than the current standard in a non-corrosive environment,
- The size effect equation can improve the design standard, especially for high-thickness transverse butt-welded joints;
- Due to a lack of corrosion fatigue data, a more quantitative thickness effect cannot be illustrated, but most of the existing data are on the conservative side.
- The mean fatigue strength decreases with increasing thickness for in-air and CP conditions, supporting the theory of fatigue strength reduction with increasing thickness.
- The fatigue strength characteristic values based on the in-air samples are close to the design strength from standards.
- There is wide variation of data spread for fatigue in a corrosive environment from the available limited data points.
6. Conclusions
- There is good agreement of thickness correction factors between the DNVGL-RP-C203 values and the characteristic values, defined as the mean + 2 standard deviation, obtained from data analysis for in-air conditions, for T-joints and transverse butt-welded joints with thicknesses up to 100 mm. Further extrapolation beyond 100 mm will yield conservative results. However, only two groups of data are available with t > 100 mm.
- For corrosion fatigue with CP for T-joints and transverse butt-welded joints, the thickness correction factors in DNVGL-RP-C203 are generally conservative even with relatively thin thicknesses of t = 25 mm. Even greater conservatism has been found for the FC condition for these joint types. It is therefore inferred that the thickness correction effects are overestimated in current standards for the corrosive fatigue predictions.
- For tubular joints and for in-air conditions, the data follow the trend of a decrease in mean fatigue strength with increasing thickness, though the agreement with design values is less when the dispersion is considered. For corrosion fatigue when t > 16 mm, though the prediction is generally conservative, the agreement between design values and data is even poorer. There is high level of statistical uncertainties due to a lack of sufficient data.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ABS | American Bureau of Shipping |
| API | American Petroleum Institute |
| ASTM | American Society for Testing and Materials |
| CA | Constant Amplitude |
| CP | Cathodic Protection |
| DNVGL | Det Norske Veritas Germanischer Lloyd |
| FC | Free Corrosion |
| FCAW | Flux-cored Arc Welding |
| HSE | Health and Safety Executive (UK) |
| IACS | International Association of Classification Societies |
| IIW | International Institute of Welding |
| N/A | Not Available |
| OWT | Offshore Wind Turbine |
| PWHT | Post-Weld Heat Treatment |
| SAW | Submerged Arc Welding |
| S-N | Stress Range—Number of Cycles |
| TIG | Gas Tungsten Arc Welding |
| TWI | The Welding Institute |
| UCL | University College London |
| UKOSRP | UK Offshore Steels Research Project |
| VA | Variable Amplitude |
References
- Zhao, W. Structural Reliability of Fatigue and Fracture under Random Loading. Ph.D. Thesis, University of London, London, UK, 1989. [Google Scholar]
- Sørensen, J. Reliability assessment of wind turbines. In Proceedings of the 12th International Conference on Applications of Statistics and Probability in Civil Engineering, Vancouver, CA, USA, 12–15 July 2015. [Google Scholar] [CrossRef]
- Zhao, W.; Stacey, A.; Prakash, P. probabilistic models of uncertainties in fatigue and fracture reliability analysis. In Proceedings of the 21st International Conference on Offshore Mechanics and Arctic Engineering, Oslo, Norway, 23–28 June 2002; Volume 3. [Google Scholar] [CrossRef]
- DNVGL. (DNVGL-RP-C203). Fatigue Design of Offshore Steel Structures; Det Norske Veritas: Oslo, Norway, 2020. [Google Scholar]
- Gurney, T.R. Fatigue of Welded Structures, 2nd ed.; Cambridge University Press: Cambridge, UK, 1979. [Google Scholar]
- IIW. Recommendations for Fatigue Design of Welded Joints and Components, IIW-1823-07; International Institute of Welding: Paris, France, 2008. [Google Scholar]
- ISO. Petroleum and Natural Gas Industries- Fixed Steel Offshore Structures (ISO 19902); International Organization for Standardization (ISO): Geneva, Switzerland, 2007. [Google Scholar]
- Pedersen, M.M. Thickness effect in fatigue of welded butt joints: A review of experimental works. Int. J. Steel Struct. 2019, 19, 1930–1938. [Google Scholar] [CrossRef]
- API. API Recommended Practice: Recommended Practice for Planning, Designing, and Constructing Fixed Offshore Platforms–Load and Resistance Factor Design (API-RP-2A-LRFD); American Petroleum Institute (API): Washington, DC, USA, 2019. [Google Scholar]
- ABS. Guide for Fatigue Assessment of Offshore Structures (ABS-115); American Bureau of Shipping (ABS): Houston, TX, USA, 2020. [Google Scholar]
- Gurney, T.R. The influence of thickness on fatigue of welded joints-10years on (a review of British work). In Proceedings of the 8th International Conference on Offshore Mechanics and Arctic Engineering, The Hague, The Netherlands, 19–23 March 1989; Volume III, pp. 1–8. [Google Scholar]
- International Association of Classification Societies (IACS). Harmonized Common Structural Rules for Oil Tankers and Bulk Carriers; IACS: London, UK, 2014. [Google Scholar]
- Lotsberg, I. Assessment of the size effect for use in design standards for fatigue analysis. Int. J. Fatigue 2014, 66, 86–100. [Google Scholar] [CrossRef]
- Haagensen, P.J. Influence of Notch Severity on Size Effects in Welded Joints and Notched Components. In Proceedings of the International Conference of Weld Failures (Weldtech 88), London, UK, 21–24 November 1988. [Google Scholar]
- Berge, S. On the effect of plate thickness in fatigue of welds. Eng. Fract. Mech. 1985, 21, 423–435. [Google Scholar] [CrossRef]
- Cole, I.S.; Vittori, O.; Cerretti, G. Cathodic protection and thickness effect: A final conclusion. In Proceedings of the 3rd Offshore and Polar Engineering Conference, Singapore, 6–11 June 1993; pp. 309–316. [Google Scholar]
- OTO 99 058. Fatigue Design Curves for Welded Joints in Air & Seawater Under Variable Amplitude Loading; Health and Safety Executive (HSE): Bootle, Merseyside, UK, 2000. [Google Scholar]
- Vaessen, G.; de Back, J.; van Leeuwen, J. fatigue behavior of welded steel joints in air and seawater. J. Pet. Technol. 1982, 34, 440–446. [Google Scholar] [CrossRef]
- OTH 92 392. A Survey of Some Recent Corrosion Fatigue Tests on Welded Joints in Steel Plate; Health and Safety Executive (HSE): Bootle, Merseyside, UK, 1994. [Google Scholar]
- Dijkstra, O.D.; de Back, J. Fatigue strength of Tubular T- and X-Joints. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 5–8 May 1980. [Google Scholar] [CrossRef]
- Gibstein, M.B. Fatigue strength of welded tubular joints tested at Det norske Veritas laboratories. In Proceedings of the Conference on Steel in Marine Structures, Paris, France, 5–8 October 1981. Paper No 8.4. [Google Scholar]
- Lourenssen, A.A.; Dijkstra, O.D. Fatigue tests on large post weld heat treated and as welded tubular T-Joints. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 1982. [Google Scholar] [CrossRef]
- Narumoto, A.; Akahide, K.; Kikukawa, S.; Kawai, Y.; Hashimoto, O. Corrosion fatigue strength of high-strength low alloy steels for offshore structures. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6–9 May 1985. [Google Scholar] [CrossRef]
- Dover, W.D.; Wilson, T.J. Corrosion Fatigue of Tubular Welded Joints. Advances in Fracture Research, Proceedings of the Sixth International Conference on Fracture ICF6; Valluri, S.R., Ed.; Pergamon Press: New Delhi, India, 1984; pp. 2505–2512. [Google Scholar]
- Vosikovosky, O.; Bell, R.; Burns, D.J.; Monaupt, U.H. Effects of Cathodic Protection and Thickness on Corrosion Fatigue Life of Welded Plate T-Joints. In Proceedings of the Conference on Steels in Marine Structures, Delft, The Netherlands, 15–18 June 1987. [Google Scholar]
- Gerald, J.; Bignonnet, A.; Lieurade, H.P.; Lecoq, H. Corrosion Fatigue Tests on High Strength Steel Tubular X Nodes with Improved Welds. In Steel in Marine Structures, Proceedings of the third International ECSC Offshore Conference on Steel in Marine Structures (SIMS ‘87), Delft, The Netherlands, 15–18 June 1987; Elsevier Science Publishers: Amsterdam, The Netherlands, 1987; pp. 455–463. [Google Scholar]
- Kerr, J.; Holmes, R.; Brown, G.M. Fatigue of Large Tubular Joints Subjected to Cathodic Protection. In Steel in Marine Structures, Proceedings of the third International ECSC Offshore Conference on Steel in Marine Structures (SIMS ’87), Delft, The Netherlands, June 1987; Elsevier Science Publishers: Amsterdam, The Netherlands, 1987; pp. 479–487. [Google Scholar]
- Zhou, M.; Yao, M.; Shi, L. Improvement of Fatigue Strength of Tubular T Joint in Offshore Platforms Due to Postweld Treatment; China Ocean Engineering, China Ocean Press: Shanghai, China, 1990; Volume 4, pp. 189–198. [Google Scholar]
- Ohta, A.; Mawari, T.; Suzuki, N. Evaluation of effect of plate thickness on fatigue strength of butt welded joints by a test maintaining maximum stress at yield strength. Eng. Fract. Mech. 1990, 37, 987–993. [Google Scholar] [CrossRef]
- OTH 92 390. Background to New Fatigue Guidance for Steel Joints and Connections in Offshore Structures; OTH 92 390; Health and Safety Executive (HSE): Bootle, Merseyside, UK, 1999. [Google Scholar]
- Raghava, G. Corrosion Fatigue Behaviour of Cathodically Protected Stiffened Steel Tubular T Joints. Ph.D. Thesis, Anna University, Chennai, India, 1998. [Google Scholar]
- Huang, X.; Wang, Z. Durability method on corrosion fatigue performance of AH 32 steel. Frat. Integrità Strutt. 2019, 13, 481–490. [Google Scholar] [CrossRef]
- Lee, J.K.; Yoon, T.Y.; Chang, S.P. The fatigue performance of buttwelded joint with thick plates. Res. Inst. Ind. Sci. Technol. 2003, 17, 249–255. [Google Scholar]
- Li, Y.; Hou, B. Corrosion fatigue of welded joints of steel for marine platform. Indian J. Eng. Mater. Sci. 2006, 13, 467–470. [Google Scholar]
- Maddox, S.J.; Zhang, Y. Comparison of fatigue of girth-welds in full-scale pipes and small-scale strip specimens. In Proceedings of the 27th International Conference on Offshore Mechanics and Arctic Engineering, Estoril, Portugal, 15–20 June 2008. [Google Scholar] [CrossRef]
- Kim, K.N.; Lee, S.H.; Jung, K.S. Evaluation of factors affecting the fatigue behaviour of butt welded joints using SM520C-TMC steel. Int. J. Steel Struct. 2009, 9, 185–193. [Google Scholar] [CrossRef]
- Polezhayeva, H.; Badger, C. Effect of plate thickness on fatigue strength of base material and butt welded specimens made from EH40 steel thick plates: Phase 1. In Proceedings of the Nineteenth International Offshore and Polar Engineering Conference (ISOPE), Osaka, Japan, 21–26 June 2009; pp. 366–373. [Google Scholar]
- EUR 24214. Fatigue Behaviour of High-Strength Steel-Welded Joints in Offshore and Marine Systems; EUR 24214: Luxembourg, 2010. [Google Scholar]
- Olafsson, O.M. Improved Design Basis of Welded Joints in Seawater. Ph.D. Thesis, Technical University of Denmark, Lyngby, Denmark, 2016. [Google Scholar]
- Kang, S.K. Thickness Effect of Fatigue on butt weld joints. In Proceedings of the TSCF 2016 Shipbuilders Meeting, Korean Register, Busan, Korea, 26–27 October 2016. [Google Scholar]
- Murthy, D.S.R. Corrosion Fatigue Behaviour of Steel Tubular Joints of Offshore Structures. Ph.D. Thesis, Anna University, Madras, India, 1993. [Google Scholar]





| Standard | F Curve | D Curve | T Curve | |||
|---|---|---|---|---|---|---|
| tref (mm) | k | tref (mm) | k | tref (mm) | k | |
| DNVGL-RP-C203 [4] | 25 | 0.25 | 25 | 0.2 | 16 | 0.25 |
| API-RP-2A [7] | N/A | N/A | N/A | N/A | 16 | 0.25 |
| ISO 19902 [6] | 16 | 0.25 | 16 | 0.25 | 16 | 0.25 |
| ABS 115 [10] | 22 | 0.25 | 22 | 0.25 | 32 | 0.25 |
| Programme Name | Joint Type | CP Condition | Number of CA Tests | Year |
|---|---|---|---|---|
| US Highway [17] | T-joint | In-air | 71 | 1974–1993 |
| Vaessen et al. [18] | T-joint | In-air/FC | 75 (In-air: 42, FC: 33) | 1979 |
| UKOSRP I [19] | T-joint | CP/FC | 71 (CP: 32, FC: 39) | 1980 |
| Dijkstra and de Back [20] | Tubular | CP/FC | 4 (CP: 1, FC: 4) | 1980 |
| Gibstein [21] | Tubular | CP | 2 | 1981 |
| Lourenssen et al. [22] | Tubular | FC | 2 | 1982 |
| Kawasaki Steel Corp. [23] | T-joint | In-air/FC | 32 (In-air: 16, FC: 16) | 1985 |
| UKOSRP II [19] | T-Joint | In-air/CP/FC | 176 (In-air: 16, CP: 132, FC: 28) | 1986 |
| Dover et al. [24] | Tubular | CP | 4 | 1986 |
| University of Waterloo [25] | T-joint | CP/FC | 30 (CP: 20, FC: 10) | 1987 |
| Gerald et al. [26] | Tubular | CP | 2 | 1987 |
| Kerr et al. [27] | Tubular | CP | 4 | 1987 |
| Zhou et al. [28] | Tubular | FC | 4 | 1990 |
| Ohta et al. [29] | Transverse butt-welded joint | In-air | 16 | 1990 |
| TWI/SINTEF [17] | Tubular | In-air/CP | 14 (In-air:8, CP: 6) | 1993 |
| Nordic Program [17] | T-joint | In-air | 35 | 1993 |
| HSE RPFG [30] | T-joint | In-air | 146 | 1995 |
| HSE RPFG [30] | Tubular | In-air/FC | 120 (In-air: 94, FC: 26) | 1995 |
| SERC-M [31] | Tubular | In-air, FC | 5 (In-air: 1, FC: 4) | N/A |
| Huang et al. [32] | T-joint | In-air/CP | 77 (In-air: 29, CP: 48) | 2006 |
| Lee et al. [33] | Transverse butt-welded joint | In-air | 11 | 2003 |
| Li et al. [34] | Transverse butt-welded joint | In-air/CP/FC | 29 (In-air: 7, CP: 14, FC: 8) | 2006 |
| Maddox et al. [35] | Transverse butt-welded joint | In-air | 34 | 2008 |
| Kim et al. [36] | Transverse butt-welded joint | In-air | 34 | 2009 |
| Polezhayeva et al. [37] | Transverse butt-welded joint | In-air | 7 | 2009 |
| FATHOMS [38] | Transverse butt-welded joint | In-air/CP | 78 (In-air: 52, CP: 17) | 2010 |
| Olafsson [39] | Transverse butt-welded joint | In-air/CP | 108 (In-air: 89, CP: 19) | 2016 |
| Kang [40] | Transverse butt-welded joint | In-air | 54 | 2016 |
| Programme Name | Thickness (mm) | Treatment | Loading Frequency (Hz) | R-Ratio |
|---|---|---|---|---|
| US Highway [17] | 10 | As welded | N/A | N/A |
| Vaessen et al. [18] | 40, 70 | As welded/PWHT/Ground/Plasma dressed/TIG | In air: 5 Seawater: 0.2 | −1/0/0.1 |
| UKOSRP I [19] | 38 | As welded/PWHT/Ground/Plasma dressed/TIG | In air: 5 Seawater: 0.17 | −1/0 |
| Dijkstra and de Back [20] | 16, 32 | As welded | 0.2 | −1/0 |
| Gibstein [21] | 16 | As welded | N/A | −1 |
| Lourenssen et al. [22] | 32 | As welded/PWHT | 0.2 | 0 |
| Kawasaki Steel Corp. [23] | 30 | As welded/TIG | 0.167 | N/A |
| UKOSRP II [19] | 38 | As welded/PWHT/Epoxy coated/Toe ground/Hammer peened/Shot peened | In air: 5 Seawater: 0.17 | −1/−0.5/0/0.5 |
| Dover et al. [24] | 16 | As welded | 0.17 | 0.1/0.3 |
| University of Waterloo [25] | 26, 78 | As welded | 0.2 | 0.05 |
| Gerald et al. [26] | 40 | As welded | 0.5 | 0.1 |
| Kerr et al. [27] | 32 | As welded | 0.167 | 0 |
| Zhou et al. [28] | 19.1 | As welded/TIG | 0.2 | −1 |
| Ohta et al. [29] | 9, 40 | As welded | N/A | 0 |
| TWI/SINTEF [17] | 32 | As welded | N/A | N/A |
| Nordic Program [17] | 12, 16 | As welded | N/A | N/A |
| HSE RPFG [30] | 16 to 200 | As welded | 0.167–0.4 | 0/0.05/0.1 |
| HSE RPFG [30] | 16 to 200 | As welded/Toe Ground/PWHT | 0.167–0.4 | −1/0/0.1 |
| SERC-M [31] | 12 | N/A | 2 | −1/0 |
| Huang et al. [32] | 24 | Dogbone specimen | 0.5–1 | N/A |
| Lee et al. [33] | 40, 75, 100 | As welded | 5–15 | 0.1 |
| Li et al. [34] | 18–25 | As welded | 0.5 | −1 |
| Maddox et al. [34] | 22 | As welded | 3–8 and 25–30 | −0.01 to 0.83 |
| Kim et al. [36] | 20, 40, 60, 80 | As welded/FCAW/SAW | 6–15 | 0.1 |
| Polezhayeva et al. [37] | 22, 66 | As welded | 0.5 or 10 | 0.1 |
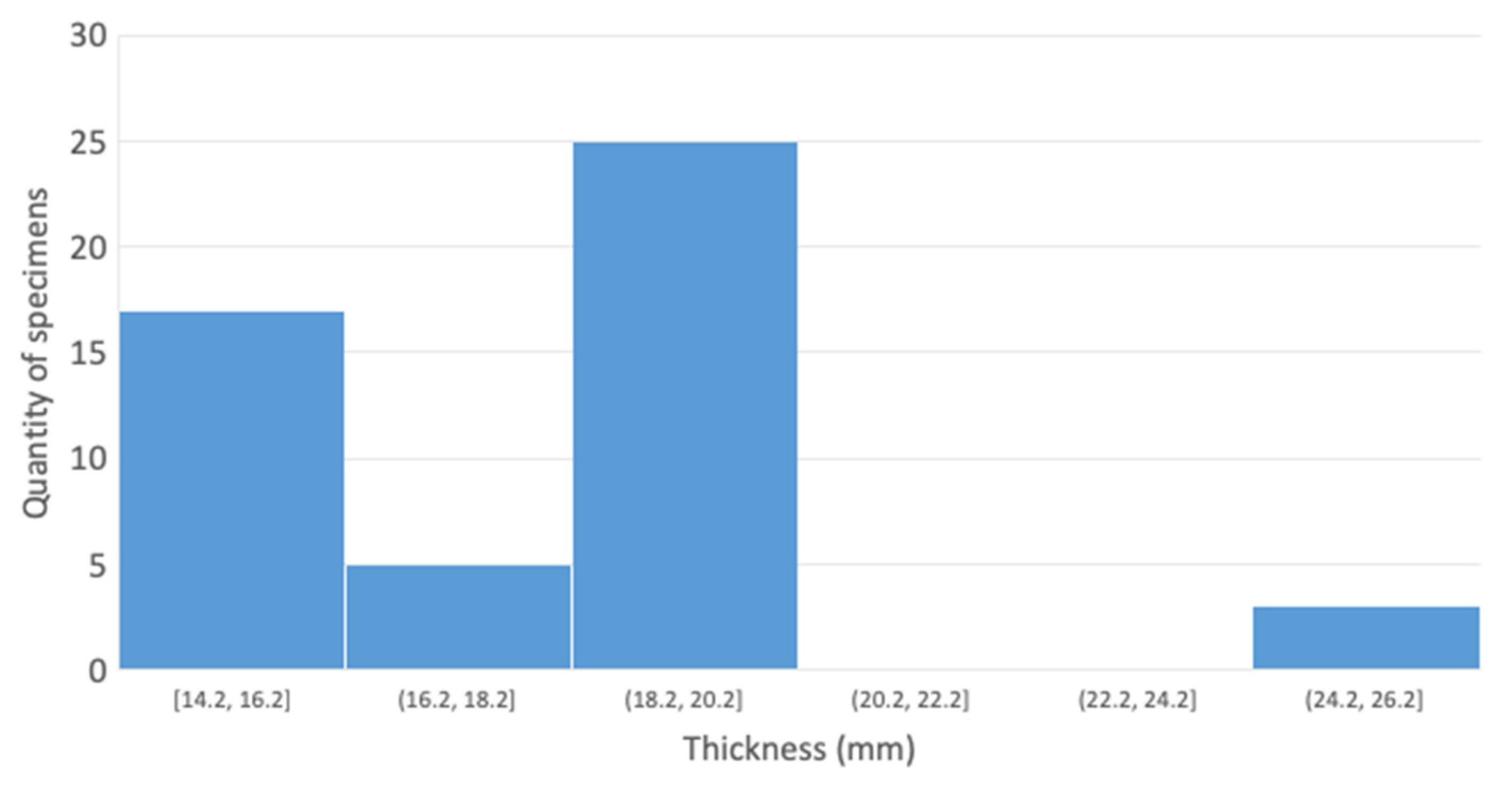
| FATHOMS [38] | 14.2,15,16 | As welded/FCAW/Laser hybrid | N/A | 0.1/0.5 |
| Olafsson [39] | 20 to 40 | As welded/Laser hybrid | 6, 8, 10 | 0.5 |
| Kang [40] | 25, 50, 75 | As welded | N/A | 0.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, W.; Hsu, W.-T. Re-Evaluation of Fatigue Thickness Effect Based on Fatigue Test Database. J. Mar. Sci. Eng. 2020, 8, 895. https://doi.org/10.3390/jmse8110895
Zhao W, Hsu W-T. Re-Evaluation of Fatigue Thickness Effect Based on Fatigue Test Database. Journal of Marine Science and Engineering. 2020; 8(11):895. https://doi.org/10.3390/jmse8110895
Chicago/Turabian StyleZhao, Wangwen, and Wei-Ting Hsu. 2020. "Re-Evaluation of Fatigue Thickness Effect Based on Fatigue Test Database" Journal of Marine Science and Engineering 8, no. 11: 895. https://doi.org/10.3390/jmse8110895
APA StyleZhao, W., & Hsu, W.-T. (2020). Re-Evaluation of Fatigue Thickness Effect Based on Fatigue Test Database. Journal of Marine Science and Engineering, 8(11), 895. https://doi.org/10.3390/jmse8110895





