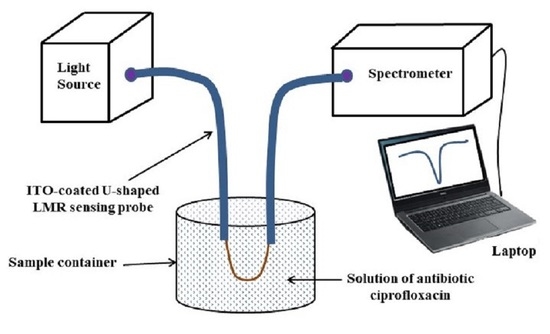
Design Considerations of an ITO-Coated U-Shaped Fiber Optic LMR Biosensor for the Detection of Antibiotic Ciprofloxacin
Abstract
:1. Introduction
2. Theory
2.1. Dispersion Properties
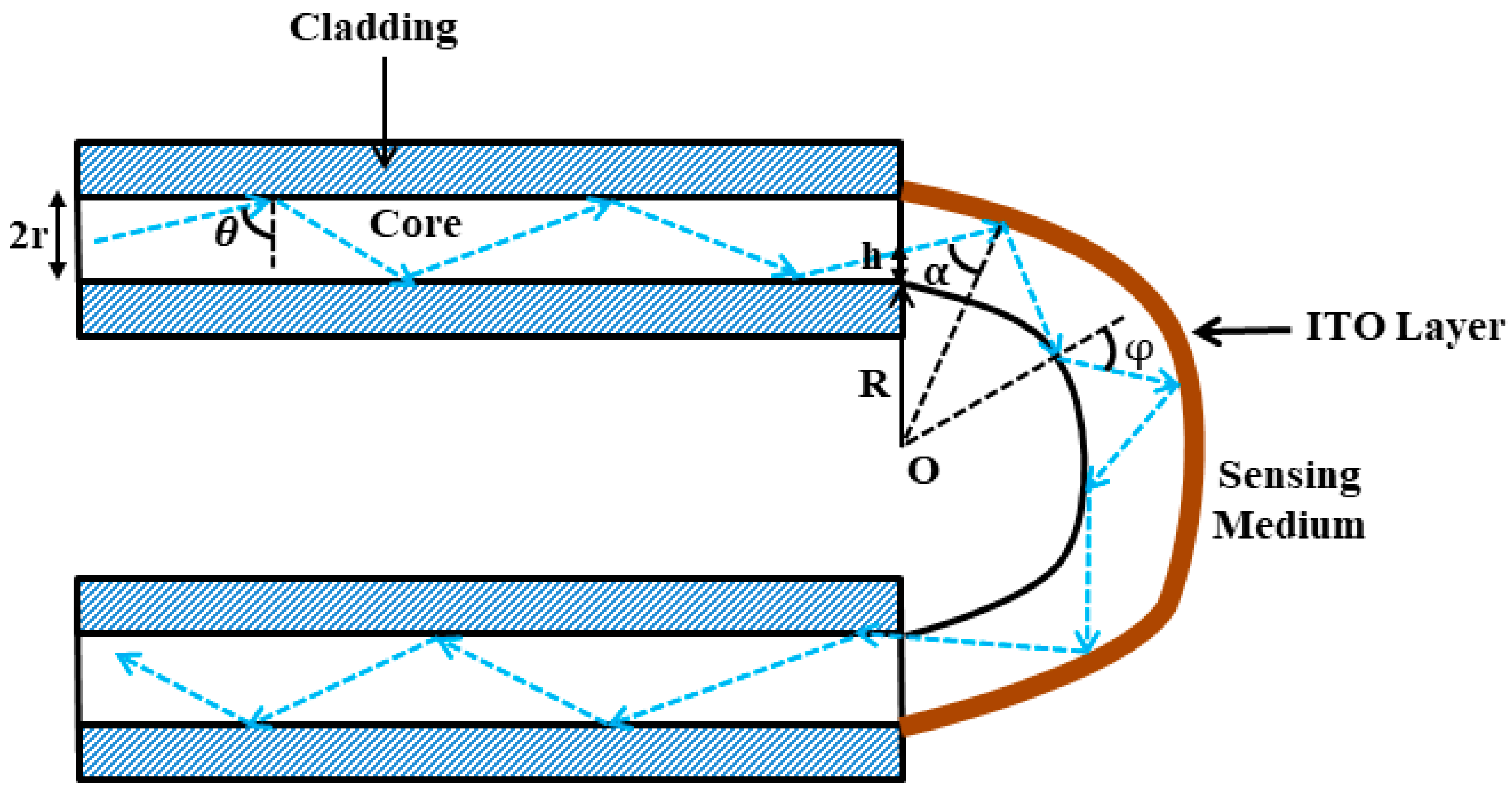
2.2. Estimation of Angles Inside the U-Shaped Region
2.3. Transmitted Power in the Case of a U-Shaped Fiber
2.4. Sensing Parameters of the U-Shaped Fiber Optic LMR Sensor
3. Numerical Results and Discussion
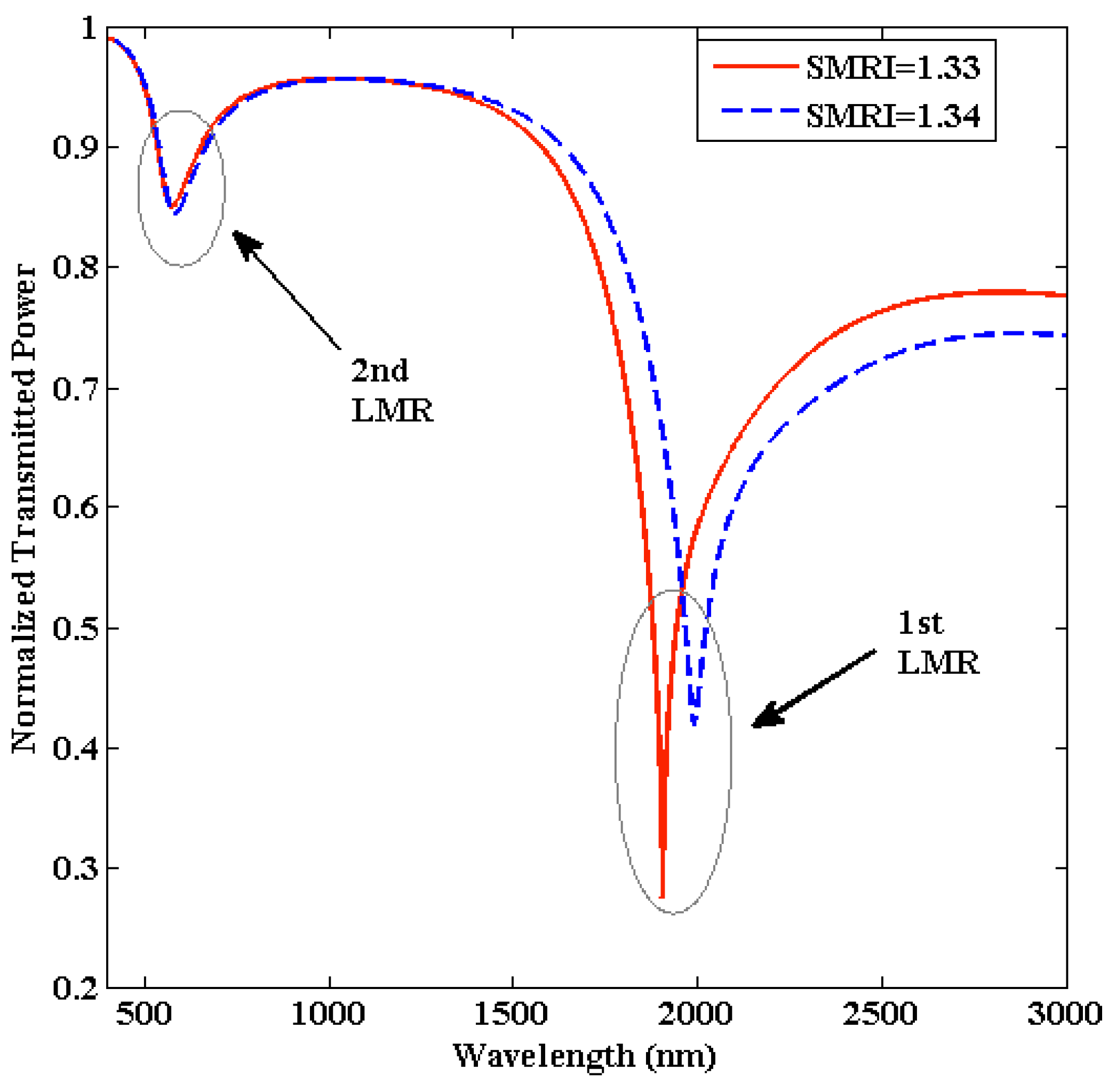
3.1. Shift in LMR Curves
3.2. Optimization of the ITO film Thickness (d)
3.3. Optimization of the Sensing Region Length (L)
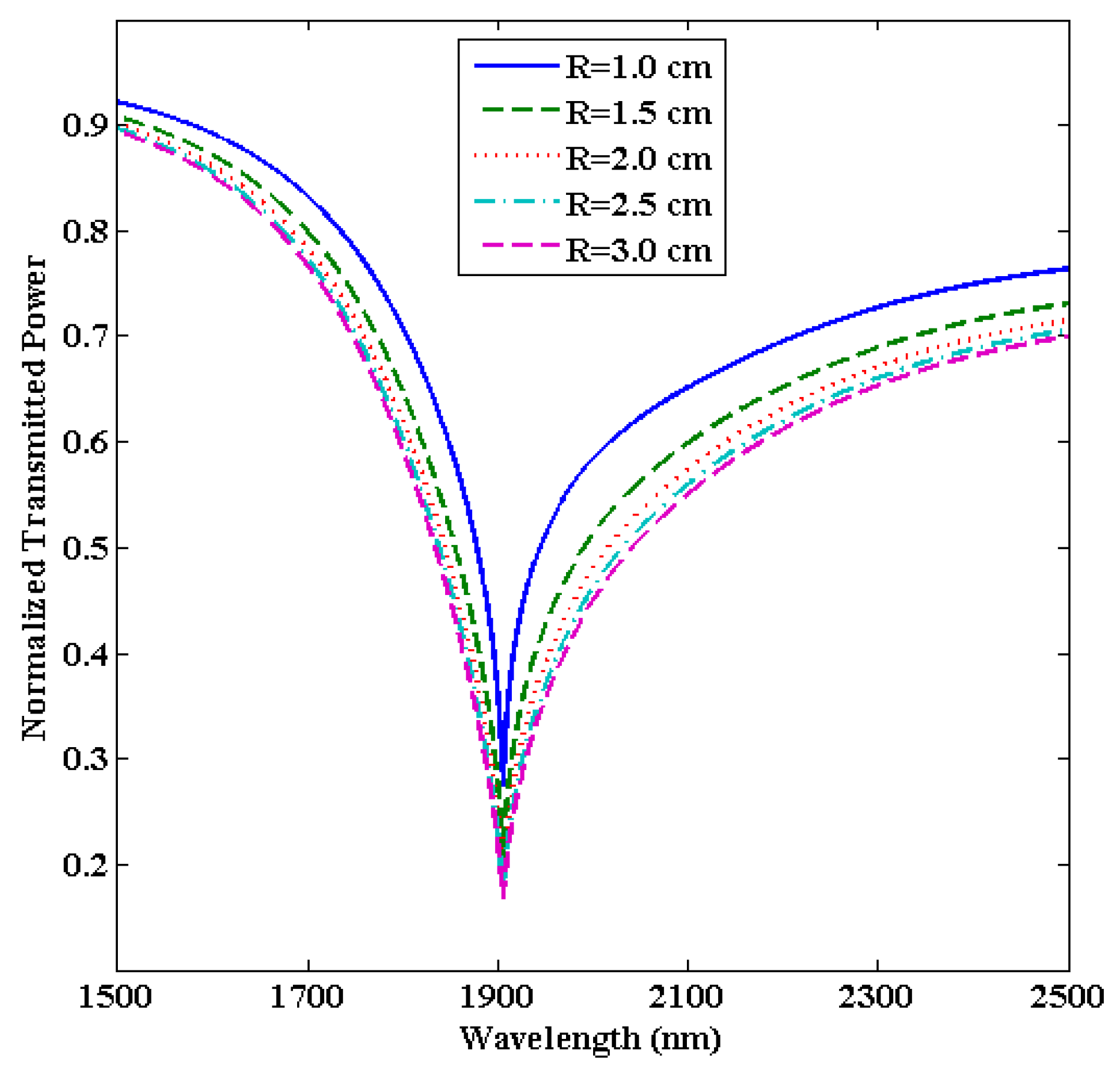
3.4. Optimization of the Bending Radius (R)
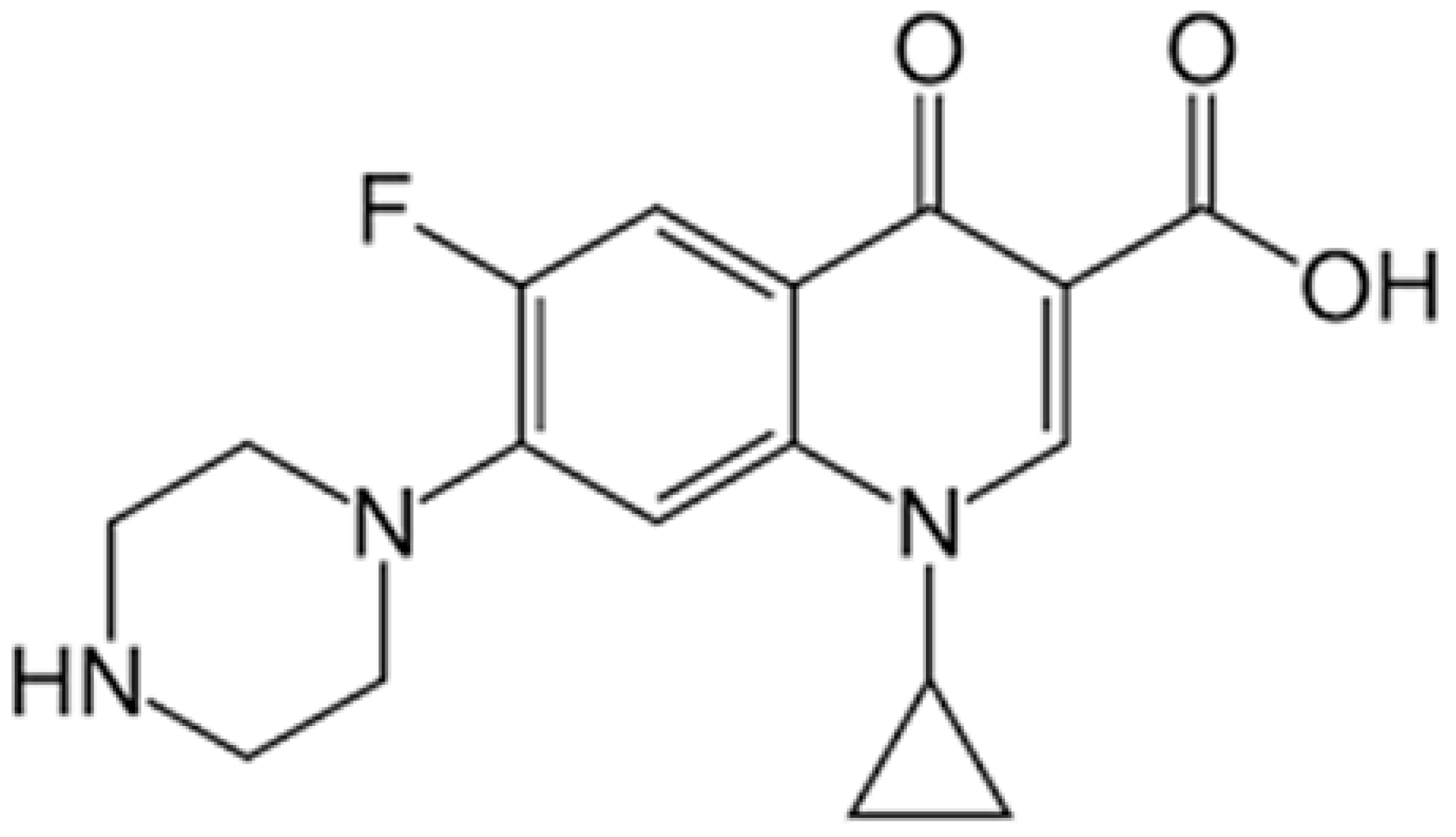
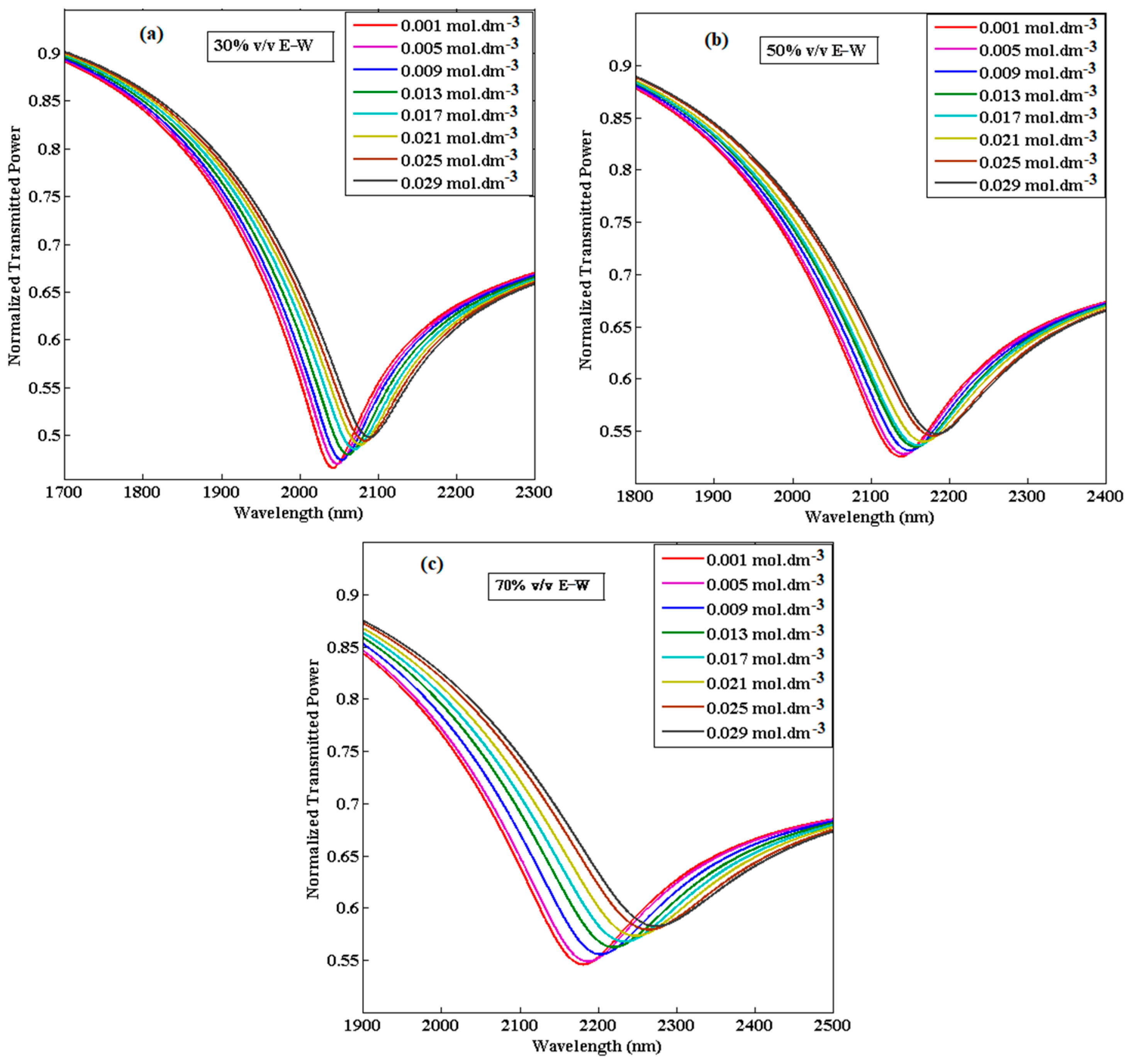
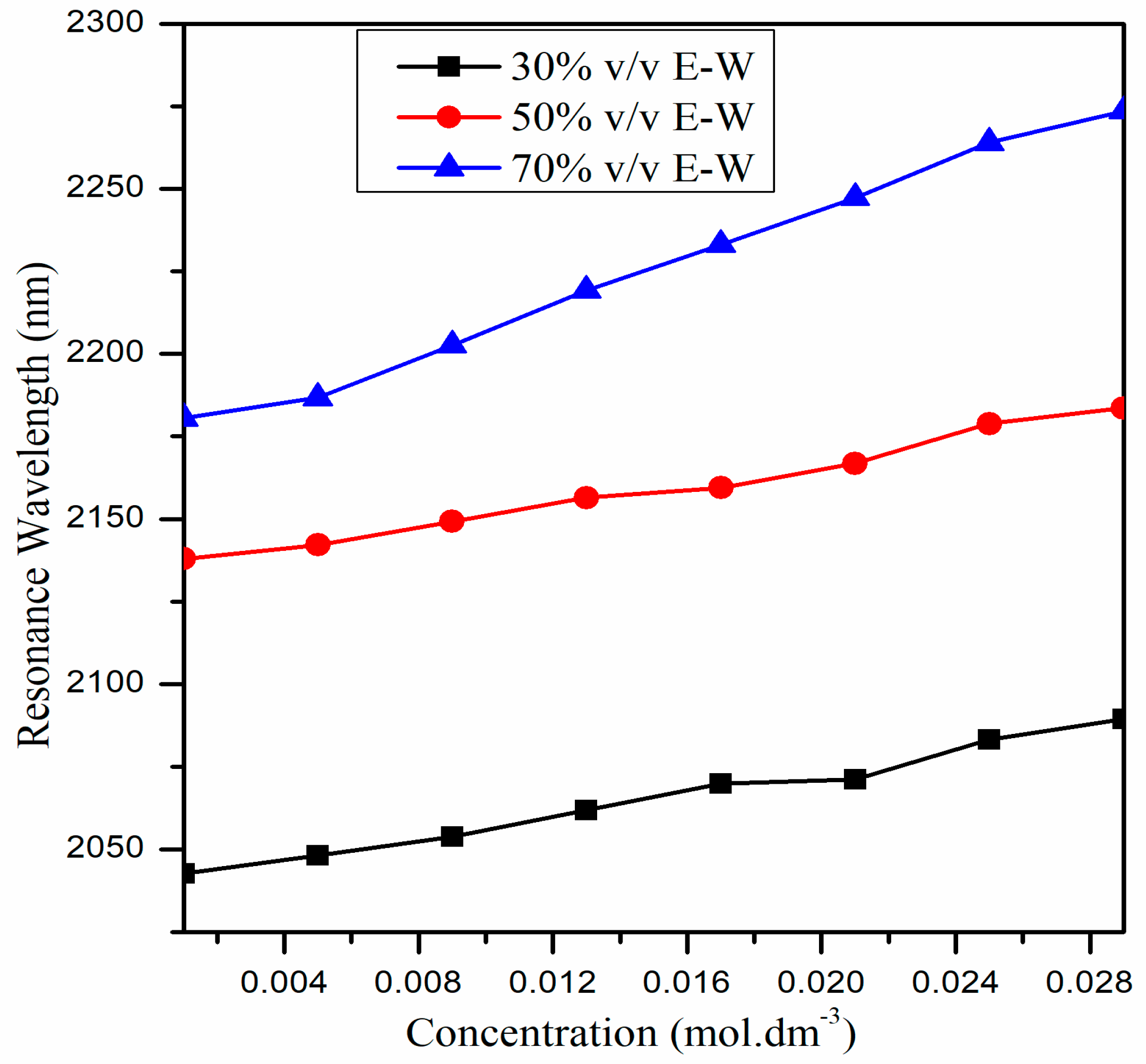
3.5. Detection of the Antibiotic Ciprofloxacin (CIP)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kovalakova, P.; Cizmas, L.; McDonald, T.J.; Marsalek, B.; Feng, M.; Sharma, V.K. Occurrence and toxicity of antibiotics in the aquatic environment: A review. Chemosphere 2020, 251, 126351. [Google Scholar]
- Zhang, H.; Liu, P.; Feng, Y.; Yang, F. Fate of antibiotics during wastewater treatment and antibiotic distribution in the effluent-receiving waters of the Yellow Sea, northern China. Mar. Pollut. Bull. 2013, 73, 282–290. [Google Scholar] [CrossRef] [PubMed]
- Danner, M.C.; Robertson, A.; Behrends, V.; Reiss, J. Antibiotic pollution in surface fresh waters: Occurrence and effects. Sci. Total Environ. 2019, 664, 793–804. [Google Scholar] [PubMed]
- Gomez, C.L.; Marinov, D.; Sanseverino, I.; Navarro, C.A.; Niegowska, M.; Porcel, R.E.; Lettieri, T. Selection of Substances for the 3rd Watch List under the Water Framework Directive; Publications Office of the European Union: Luxembourg, 2020; ISBN 978-92-76-19425-5. [Google Scholar] [CrossRef]
- Azriouil, M.; Matrouf, M.; Ettadili, F.E.; Laghrib, F.; Farahi, A.; Saqrane, S.; Bakasse, M.; Lahrich, S.; El Mhammedi, M.A. Recent trends on electrochemical determination of antibiotic Ciprofloxacin in biological fluids, pharmaceutical formulations, environmental resources and foodstuffs: Direct and indirect approaches. Food Chem. Toxicol. 2022, 168, 113378. [Google Scholar]
- Yan, C.; Yang, Y.; Zhou, J.; Liu, M.; Nie, M.; Shi, H.; Gu, L. Antibiotics in the surface water of the Yangtze estuary: Occurrence, distribution and risk assessment. Environ. Pollut. 2013, 175, 22–29. [Google Scholar]
- Martinez, J.L. Environmental pollution by antibiotics and by antibiotic resistance determinants. Environ. Pollut. 2009, 157, 2893–2902. [Google Scholar] [PubMed]
- Segura, P.A.; Takada, H.; Correa, J.A.; El Saadi, K.; Koike, T.; Onwona-Agyeman, S.; Ofosu-Anim, J.; Sabi, E.B.; Wasonga, O.V.; Mghalu, J.M.; et al. Global occurrence of anti-infectives in contaminated surface waters: Impact of income inequality between countries. Environ. Int. 2015, 80, 89–97. [Google Scholar] [CrossRef]
- Abramova, A.A.; Isakov, V.G.; Grakhova, E.V.; Nepogodin, A.M. Methods for detection of antibiotics in urban wastewater. IOP Conf. Ser. Mater. Sci. Eng. 2020, 862, 062059. [Google Scholar] [CrossRef]
- Chen, T.; Liu, Y.; Lu, J.; Xing, J.; Li, L.; Liu, T.; Xue, Q. Highly efficient detection of ciprofloxacin in water using a nitrogen-doped carbon electrode fabricated through plasma modification. New J. Chem. 2019, 43, 15169–15176. [Google Scholar] [CrossRef]
- Ahmed, S.; Ning, J.; Peng, D.; Chen, T.; Ahmad, I.; Ali, A.; Lei, Z.; Shabbir, M.A.B.; Cheng, G.; Yuan, Z. Current advances in immunoassays for the detection of antibiotics residues: A review. Food Agric. Immunol. 2020, 31, 268–290. [Google Scholar] [CrossRef]
- Díaz-Quiroz, C.A.; Hernandez-Chavez, J.F.; Ulloa-Mercado, G.; Gortáres-Moroyoqui, P.; Martínez-Macías, R.; Meza-Escalante, E.; Serrano-Palacios, D. Simultaneous quantification of antibiotics in wastewater from pig farms by capillary electrophoresis. J. Chromatogr. B 2018, 1092, 386–393. [Google Scholar] [CrossRef] [PubMed]
- Kim, C.; Ryu, H.D.; Chung, E.G.; Kim, Y. Determination of 18 veterinary antibiotics in environmental water using high-performance liquid chromatography-q-orbitrap combined with on-line solid-phase extraction. J. Chromatogr. B 2018, 1084, 158–165. [Google Scholar] [CrossRef] [PubMed]
- Tabrizchi, M.; ILbeigi, V. Detection of explosives by positive corona discharge ion mobility spectrometry. J. Hazard. Mater. 2010, 176, 692–696. [Google Scholar] [CrossRef]
- Blasco, C.; Corcia, A.D.; Pico, Y. Determination of tetracyclines in multi-specie animal tissues by pressurized liquid extraction and liquid chromatography-tandem mass spectrometry. Food Chem. 2009, 116, 1005–1012. [Google Scholar] [CrossRef]
- Moros, J.; Laserna, J.J. New Raman-Laser-Induced Breakdown Spectroscopy Identity of Explosives Using Parametric Data Fusion on an Integrated Sensing Platform. Anal. Chem. 2011, 83, 6275–6285. [Google Scholar] [CrossRef]
- Zeng, Y.; Chang, F.; Liu, Q.; Duan, L.; Li, D.; Zhang, H. Recent Advances and Perspectives on the Sources and Detection of Antibiotics in Aquatic Environments. J. Anal. Methods Chem. 2022, 2022, 5091181. [Google Scholar] [CrossRef]
- Liu, C.; Li, B.; Liu, M.; Mao, S. Demand, status, and prospect of antibiotics detection in the environment. Sens. Actuators B Chem. 2022, 369, 132383. [Google Scholar] [CrossRef]
- Wang, X.D.; Wolfbeis, O.S. Fiber-optic chemical sensors and biosensors (2015–2019). Anal. Chem. 2019, 92, 397–430. [Google Scholar] [CrossRef]
- Bianchi, L.; Mooney, R.; Cornejo, Y.; Hyde, C.; Schena, E.; Berlin, J.M.; Aboody, K.; Saccomandi, P. Fiber Bragg grating sensors-based thermometry of gold nanorod-enhanced photothermal therapy in tumor model. IEEE Sens. J. 2021, 22, 11297–11306. [Google Scholar] [CrossRef]
- Bianchi, L.; Korganbayev, S.; Orrico, A.; Landro, M.D.; Saccomandi, P. Quasi-distributed fiber optic sensor-based control system for interstitial laser ablation of tissue: Theoretical and experimental investigations. Biomed. Opt. Exp. 2021, 12, 2841–2858. [Google Scholar] [CrossRef]
- Korganbayev, S.; Landro, M.D.; Wolf, A.; Tosi, D.; Saccomandi, P. Tilted Fiber Bragg grating measurements during laser ablation of hepatic tissues: Quasi-distributed temperature reconstruction and cladding mode resonances analysis. IEEE Sens. J. 2022, 22, 15999–16007. [Google Scholar] [CrossRef]
- Correia, R.; James, S.; Lee, S.W.; Morgan, S.P.; Korposh, S. Biomedical application of optical fibre sensors. J. Opt. 2018, 20, 073003. [Google Scholar] [CrossRef]
- Sahota, J.K.; Gupta, N.; Dhawan, D. Fiber Bragg grating sensors for monitoring of physical parameters: A comprehensive review. Opt. Eng. 2020, 59, 060901. [Google Scholar] [CrossRef]
- Joe, H.E.; Yun, H.; Jo, S.H.; Jun, M.B.G.; Min, B.K. A review on optical fiber sensors for environmental monitoring. Int. J. Precis. Eng. Manuf. 2018, 5, 173–191. [Google Scholar] [CrossRef]
- Vikas; Gupta, S.; Tejavath, K.; Verma, R.K. Urea detection using bio-synthesized gold nanoparticles: An SPR/LSPR based sensing approach realized on optical fiber. Opt. Quantum Electron. 2020, 52, 278. [Google Scholar] [CrossRef]
- Vikas; Yadav, M.K.; Kumar, P.; Verma, R.K. Detection of adulteration in pure honey utilizing Ag-graphene oxide coated fiber optic SPR probes. Food Chem. 2020, 332, 127346. [Google Scholar] [CrossRef]
- Vikas; Verma, R.K. On the application of few layer Ti3C2 MXene on fiber optic SPR sensor for performance enhancement. Eur. Phys. J. D 2021, 75, 1–11. [Google Scholar] [CrossRef]
- Korposh, S.; Chianella, I.; Guerreiro, A.; Caygill, S.; Piletsky, S.; James, S.W.; Tatam, R.P. Selective vancomycin detection using optical fibre long period gratings functionalised with molecularly imprinted polymer nanoparticles. Analyst 2014, 139, 2229–2236. [Google Scholar] [CrossRef] [Green Version]
- Chaudhari, P.P.; Chau, L.K.; Tseng, Y.T.; Huang, C.J.; Chen, Y.L. A fiber optic nanoplasmonic biosensor for the sensitive detection of ampicillin and its analogs. Microchim. Acta. 2020, 187, 396. [Google Scholar] [CrossRef]
- Huang, Q.D.; Lv, C.H.; Yuan, X.L.; He, M.; Lai, J.P.; Sun, H. A novel fluorescent optical fiber sensor for highly selective detection of antibiotic ciprofloxacin based on replaceable molecularly imprinted nanoparticles composite hydrogel detector. Sens. Actuators B Chem. 2021, 328, 129000. [Google Scholar] [CrossRef]
- Wei, Z.; Cheng, X.; Li, J.; Wang, G.; Mao, J.; Zhao, J.; Lou, X. Ultrasensitive evanescent wave optical fiber aptasensor for online, continuous, type-specific detection of sulfonamides in environmental water. Anal. Chim. Acta. 2022, 1233, 340505. [Google Scholar] [CrossRef] [PubMed]
- Vikas; Mishra, S.K.; Mishra, A.K.; Saccomandi, P.; Verma, R.K. Recent Advances in Lossy Mode Resonance-Based Fiber Optic Sensors: A Review. Micromachines 2022, 13, 1921. [Google Scholar] [CrossRef] [PubMed]
- Marciniak, M.; Grzegorzewski, J.; Szustakowski, M. Analysis of lossy mode cut-off conditions in planar waveguides with semiconductor guiding layer. IEE Proc. J. 1993, 140, 247–252. [Google Scholar] [CrossRef]
- Arregui, F.J.; Villar, I.D.; Corres, J.M.; Goicoechea, J.; Zamarreño, C.R.; Elosua, C.; Hernaez, M.; Rivero, P.J.; Socorro, A.B.; Urrutia, A.; et al. Fiber-optic lossy mode resonance sensors. Procedia Eng. 2014, 87, 3–8. [Google Scholar] [CrossRef] [Green Version]
- Golant, E.I.; Golant, K.M. Fields and modes in thin film coated optical waveguides. In Proceedings of the 2019 PhotonIcs & Electromagnetics Research Symposium—Spring (PIERS-Spring), Rome, Italy, 17–20 June 2019; pp. 725–732. [Google Scholar] [CrossRef]
- Golant, E.I.; Pashkovskii, A.B.; Golant, K.M. Lossy mode resonance in an etched-out optical fiber taper covered by a thin ITO layer. Appl. Opt. 2020, 59, 9254–9258. [Google Scholar] [CrossRef] [PubMed]
- Villar, I.D.; Zamarreño, C.R.; Hernaez, M.; Arregui, F.J.; Matias, I.R. Lossy mode resonance generation with indium-tin-oxide-coated optical fibers for sensing applications. J. Light. Technol. 2010, 28, 111–117. [Google Scholar] [CrossRef] [Green Version]
- Vikas; Walia, K.; Verma, R.K. Lossy mode resonance-based uniform core tapered fiber optic sensor for sensitivity enhancement. Commun. Theor. Phys. 2020, 72, 095502. [Google Scholar] [CrossRef]
- Zamarreno, C.R.; Sanchez, P.; Hernaez, M.; Villar, I.D.; Valdivielso, C.F.; Matias, I.R. Sensing properties of indium oxide coated optical fiber devices based on lossy mode resonances. IEEE Sens. J. 2012, 12, 151–155. [Google Scholar] [CrossRef]
- Hernaez, M.; Villar, I.D.; Zamarreno, C.R.; Arregui, F.J.; Matias, I.R. Optical fiber refractometers based on lossy mode resonances supported by TiO2 coatings. Appl. Opt. 2010, 49, 3980–3985. [Google Scholar] [CrossRef]
- Sanchez, P.; Zamarreno, C.R.; Hernaez, M.; Matias, I.R.; Arregui, F.J. Optical fiber refractometers based on Lossy Mode Resonances by means of SnO2 sputtered coatings. Sens. Actuators B Chem. 2014, 202, 154–159. [Google Scholar] [CrossRef]
- Usha, S.P.; Mishra, S.K.; Gupta, B.D. Fiber optic hydrogen sulfide gas sensors utilizing ZnO thin film/ZnO nanoparticles: A comparison of surface plasmon resonance and lossy mode resonance. Sens. Actuators B Chem. 2015, 218, 196–204. [Google Scholar] [CrossRef]
- Paliwal, N.; John, J. Lossy mode resonance (LMR) based fiber optic sensors: A review. IEEE Sens. J. 2015, 15, 5361–5371. [Google Scholar] [CrossRef]
- Korotcenkov, G. Metal oxides for solid-state gas sensors: What determine our choice. Mater. Sci. Eng. B 2007, 139, 1–23. [Google Scholar] [CrossRef]
- Grundmann, M.; Frenzel, H.; Lajn, A.; Lorenz, M.; Schein, F.; Wenckstern, H.V. Transparent semiconducting oxides: Materials and devices. Phys. Status Solidi A 2010, 207, 1437–1449. [Google Scholar] [CrossRef]
- Chopra, K.L.; Das, S.R. Why thin film in solar cells. In Thin Film Solar Cells; Springer: Boston, MA, USA, 1983; pp. 1–18. [Google Scholar] [CrossRef]
- Wan, C.; Qu, A.; Deng, L.; Liu, X.; Wu, C. Preparation of an electrochemical biosensor based on indium tin oxide and its performance in detecting antibiotic resistance genes. Microchem. J. 2022, 182, 107953. [Google Scholar] [CrossRef]
- Yeh, S.H.; Huang, M.S.; Huang, C.H. Electrochemical sensors for sulfamethoxazole detection based on graphene oxide/graphene layered composite on indium tin oxide substrate. J. Taiwan Inst. Chem. Eng. 2022, 131, 104155. [Google Scholar] [CrossRef]
- Smietana, M.; Niedzialkowski, P.; Bialobrzeska, W.; Burnat, D.; Sezemsky, P.; Koba, M.; Stranak, V.; Siuzdak, K.; Ossowski, T.; Bogdanowicz, R. Study on combined optical and electrochemical analysis using indium-tin-oxide-coated optical fiber sensor. Electroanalysis 2019, 31, 398–404. [Google Scholar] [CrossRef]
- Nix, D.; DeVito, J.M. Ciprofloxacin and norfloxacin, two fluoroquinolone antimicrobials. Clin. Pharm. 1987, 6, 105–117. [Google Scholar]
- Zhang, Z.; Wu, T.; Zhou, H.; Jiang, C.; Wang, Y. 3D flower-shaped BiOI encapsulated in molecularly imprinted polymer for hypersensitivity to norfloxacin. Microchem. J. 2021, 164, 106017. [Google Scholar] [CrossRef]
- Littlejohn, D.; Lucas, D.; Han, L. Bent silica fiber evanescent absorption sensors for near infrared spectroscopy. Appl. Spectrosc. 1999, 53, 845–849. [Google Scholar] [CrossRef]
- Khijwania, S.J.; Gupta, B.D. Fiber optic evanescent field absorption sensor: Effect of fiber parameters and geometry of the probe. Opt. Quantum Electron. 1999, 31, 625–636. [Google Scholar] [CrossRef]
- Sai, V.V.; Kundu, T.; Mukherji, S. Novel U-bent fiber optic probe for localized surface plasmon resonance based biosensor. Biosens. Bioelectron. 2009, 24, 2804–2809. [Google Scholar] [CrossRef] [PubMed]
- Srivastava, S.K.; Arora, V.; Sapra, S.; Gupta, B.D. Localized surface plasmon resonance-based fiber optic U-shaped biosensor for the detection of blood glucose. Plasmonics 2012, 7, 261–268. [Google Scholar] [CrossRef]
- Vikas; Verma, R.K. Design considerations of a surface plasmon resonance (SPR) based tapered fiber optic bio-sensing probe with graphene-MoS2 over layers”. Opt. Int. J. Light Electron Opt. 2019, 180, 330–343. [Google Scholar] [CrossRef]
- Rhodes, C.; Cerruti, M.; Efremenko, A.; Losego, M.; Aspnes, D.E.; Maria, J.P.; Franzen, S. Dependence of plasmon polaritons on the thickness of indium tin oxide thin films. J. Appl. Phys. 2008, 103, 093108. [Google Scholar] [CrossRef]
- Deosarkar, S.D.; Birajdar, S.S.; Sawale, R.T.; Pawar, M.P.; Thakre, A.M. Density and Optical Properties of {Ciprofloxacin Hydrochloride + Aqueous- Ethanol} Mixtures at 30 °C. J. Thermodyn. 2016, 2016, 1575836. [Google Scholar] [CrossRef] [Green Version]
- Koohyar, F.; Rostami, A.A.; Chaichi, M.J.; Kiani, F. Refractive indices, viscosities, and densities for L-cysteine hydrochloride monohydrate+ D-sorbitol + water, and glycerol+ D-sorbitol+ water in the temperature range between T = 303.15 K and T = 323.15 K. J. Solution Chem. 2011, 40, 1361–1370. [Google Scholar] [CrossRef]
- Tan, A.J.; Ng, S.M.; Stoddart, P.R.; Chua, H.S. Theoretical model and design considerations of U-shaped fiber optic sensors: A review. IEEE Sens. J. 2020, 20, 14578–14589. [Google Scholar] [CrossRef]
- Paliwal, N.; Punjabi, N. Design and fabrication of lossy mode resonance based U-shaped fiber optic refractometer utilizing dual sensing phenomenon. J. Light. Technol. 2016, 34, 4186–4193. [Google Scholar] [CrossRef]
- Vikas; Verma, R.K. Sensitivity enhancement of a lossy mode resonance based tapered fiber optic sensor with an optimum taper profile. J. Phys. D 2018, 51, 415302. [Google Scholar] [CrossRef]
- Li, S.; Gao, L.; Zou, C.; Xie, W.; Wei, Y.; Tian, C.; Wang, Z.; Liang, F.; Xiang, Y.; Yang, Q. A Polarization-Independent Fiber-Optic SPR Sensor. Sensors 2018, 18, 3204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, Y.; Wei, T.; Dong, W.; Huang, C.; Zhang, K.; Sun, Y.; Chen, X.; Dai, N. Near-perfect infrared absorption from dielectric multilayer of plasmonic aluminum-doped zinc oxide. Appl. Phys. Lett. 2013, 102, 213117. [Google Scholar] [CrossRef]
- Shah, K.; Sharma, N.K.; Sajal, V. SPR based fiber optic sensor with bi layers of indium tin oxide and platinum: A theoretical evaluation. Optik 2017, 135, 50–56. [Google Scholar] [CrossRef]
- Punjabi, N.; Satija, J.; Mukherji, S. Evanescent wave absorption based fiber-optic sensor-cascading of bend and tapered geometry for enhanced sensitivity. In Sensing Technology: Current Status and Future Trends III; Springer: Cham, Switzerland, 2015; pp. 25–45. [Google Scholar] [CrossRef]
- Lopez, S.; Villar, I.D.; Zamarreño, C.R.; Hernaez, M.; Arregui, F.J.; Matias, I.R. Optical fiber refractometers based on indium tin oxide coatings fabricated by sputtering. Opt. Lett. 2012, 37, 28–30. [Google Scholar] [CrossRef]
- Zamarreño, C.R.; Sanchez, P.; Hernaez, M.; Villar, I.D.; Valdivielso, C.F.; Matias, I.R.; Arregui, F.J. Dual-peak resonance-based optical fiber refractometers. IEEE Photonics Technol. Lett. 2010, 22, 1778–1780. [Google Scholar] [CrossRef]
- Ozcariz, A.; Azamar, D.A.P.; Zamarreno, C.R.; Dominguez, R.; Arregui, F.J. Aluminum doped zinc oxide (AZO) coated optical fiber LMR refractometers-An experimental demonstration. Sens. Actuators B Chem. 2019, 281, 698–704. [Google Scholar] [CrossRef]
- Paliwal, N.; John, J. Theoretical modeling and investigations of AZO coated LMR based fiber optic tapered tip sensor utilizing an additional TiO2 layer for sensitivity enhancement. Sens. Actuators B Chem. 2017, 238, 1–8. [Google Scholar] [CrossRef]
- Tien, C.L.; Lin, H.L.; Su, S.H. High sensitivity refractive index sensor by D-shaped fibers and titanium dioxide nanofilm. Soft Matter Photon. 2018, 2018, 2303740. [Google Scholar] [CrossRef] [Green Version]
- Ozcariz, A.; Dominik, M.; Smietana, M.; Zamarreno, C.R.; Villar, I.D.; Arregui, F.J. Lossy mode resonance optical sensors based on indium-gallium-zinc oxide thin film. Sens. Actuators A Phys. 2019, 290, 20–27. [Google Scholar] [CrossRef]
- Ozcariz, A. Development of copper oxide thin film for lossy mode resonance-based optical fiber sensor. Multidiscip. Digit. Publ. Inst. Proc. 2018, 2, 893. [Google Scholar]
- Xie, T.; He, Y.; Zhang, H.; Xu, Y. Highly sensitive surface plasmon resonance sensor based on graphene-coated U-shaped fiber. Plasmonics 2021, 16, 205–213. [Google Scholar] [CrossRef]
- Zhang, C.; Li, Z.; Jiang, S.Z.; Li, C.H.; Xu, S.C.; Yu, J.; Li, Z.; Wang, M.H.; Liu, A.H.; Man, B.Y. U-shaped fiber optic SPR sensor based on graphene/AgNPs. Sens. Actuators B Chem. 2017, 251, 127–133. [Google Scholar] [CrossRef]






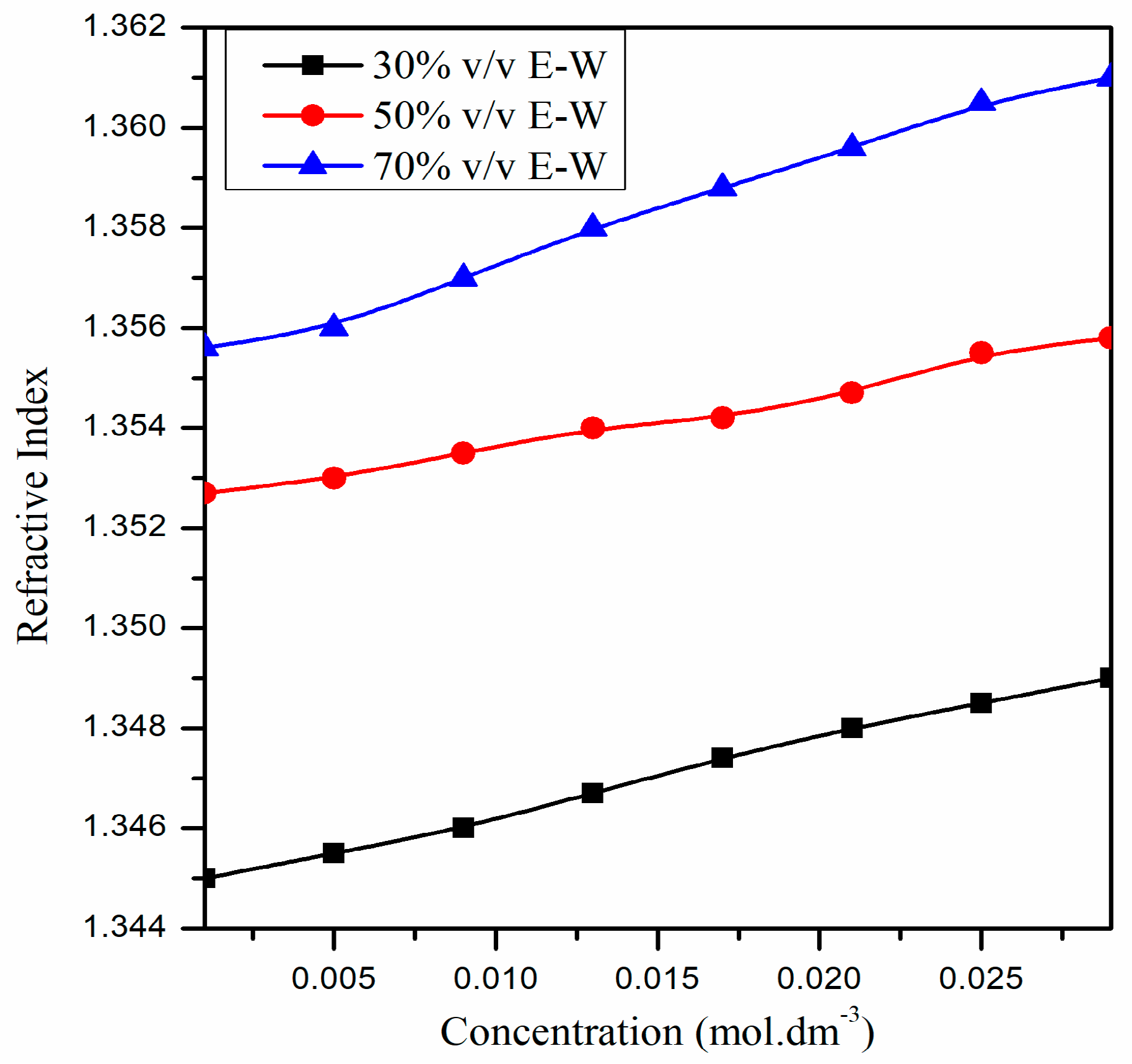
| Concentration of CIP Hydrochloride (mol∙dm−3) | Refractive Index (n) | ||
|---|---|---|---|
| 30% v/v E–W | 50% v/v E–W | 70% v/v E–W | |
| 0.001 | 1.3450 | 1.3527 | 1.3556 |
| 0.005 | 1.3455 | 1.3530 | 1.3560 |
| 0.009 | 1.3460 | 1.3535 | 1.3570 |
| 0.013 | 1.3467 | 1.3540 | 1.3580 |
| 0.017 | 1.3474 | 1.3542 | 1.3588 |
| 0.021 | 1.3480 | 1.3547 | 1.3596 |
| 0.025 | 1.3485 | 1.3555 | 1.3605 |
| 0.029 | 1.3490 | 1.3558 | 1.3610 |
| ITO Thickness (nm) | Performance Parameters | ||
|---|---|---|---|
| Sensitivity (nm/RIU) | FWHM (nm) | FOM (RIU−1) | |
| 60 | 4850 | 99.3 | 48.84 |
| 70 | 6220 | 102.9 | 60.44 |
| 80 | 7500 | 109.4 | 68.55 |
| 90 | 8570 | 93.3 | 91.85 |
| 100 | 9340 | 102.0 | 91.56 |
| 110 | 9980 | 144.7 | 68.97 |
| Type of LMR/SPR Configuration | Theoretical/Experimental | Maximum Sensitivity (nm/RIU) | Figure of Merit (RIU−1) | References |
|---|---|---|---|---|
| Uniform fiber core + ITO | Experimental | 1221 | - | Lopez et al. [68] |
| Uniform fiber core + In2O3 | Experimental | 4068 | - | Zamarreno et al. [69] |
| Uniform fiber core + AZO | Experimental | 2280 | - | Ozcariz et al. [70] |
| Tapered fiber core + AZO/TiO2 | Theoretical | 9000 | - | Paliwal et al. [71] |
| D-shaped fiber + TiO2 | Experimental | 4122 | - | Tien et al. [72] |
| D-shaped fiber + IGZO | Experimental | 12,929 | 396.20 | Ozcariz et al. [73] |
| Uniform fiber core + CuO | Experimental | 7324 | - | Ozcariz et al. [74] |
| U-shaped fiber core + gold + graphene | Theoretical | 15,000 | 33.59 | Xie et al. [75] |
| U-shaped fiber core + graphene + silver NPs | Experimental | 1198 | - | Zhang et al. [76] |
| U-shaped fiber core + ITO | Theoretical | 17,209 | 91.42 | Present work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vikas; Saccomandi, P. Design Considerations of an ITO-Coated U-Shaped Fiber Optic LMR Biosensor for the Detection of Antibiotic Ciprofloxacin. Biosensors 2023, 13, 362. https://doi.org/10.3390/bios13030362
Vikas, Saccomandi P. Design Considerations of an ITO-Coated U-Shaped Fiber Optic LMR Biosensor for the Detection of Antibiotic Ciprofloxacin. Biosensors. 2023; 13(3):362. https://doi.org/10.3390/bios13030362
Chicago/Turabian StyleVikas, and Paola Saccomandi. 2023. "Design Considerations of an ITO-Coated U-Shaped Fiber Optic LMR Biosensor for the Detection of Antibiotic Ciprofloxacin" Biosensors 13, no. 3: 362. https://doi.org/10.3390/bios13030362
APA StyleVikas, & Saccomandi, P. (2023). Design Considerations of an ITO-Coated U-Shaped Fiber Optic LMR Biosensor for the Detection of Antibiotic Ciprofloxacin. Biosensors, 13(3), 362. https://doi.org/10.3390/bios13030362