Enhancing the Accuracy of Measuring DEP Force Applied on Cells by Considering the Friction Effect
Abstract
:1. Introduction
2. Materials and Methods
2.1. Theory and Modeling
2.2. The Geometry of the Electrodes and the Microchannel
2.3. Simulation
2.4. Cell and Device Preparation
| Properties | WBC Buffer | Sperm Buffer | WBC | Sperm | Reference |
|---|---|---|---|---|---|
| Working frequency (MHz) | - | - | 0.8 | 1 | - |
| Radius * (µm) | - | - | 3.8 | 2.5 | [45,46] |
| Membrane thickness (nm) | - | - | 7 | NK ** | [47] |
| Permittivity | 78 | 78 | 104 | NK ** | [46] |
| Membrane permittivity | - | - | 12 | NK ** | [48] |
| Conductivity (µS/cm) | 800 | 140 | 650 | NK ** | [46] |
| Membrane conductivity (nS/cm) | - | - | 10 | NK ** | [46] |
| Density (kg/m3) | - | - | 1019 | 1100 | [49,50] |
| Viscosity (mPa.s) | 1.2 | 1.2 | - | - | [51] |
2.5. Fabricating of the Electrodes and the Microchannel
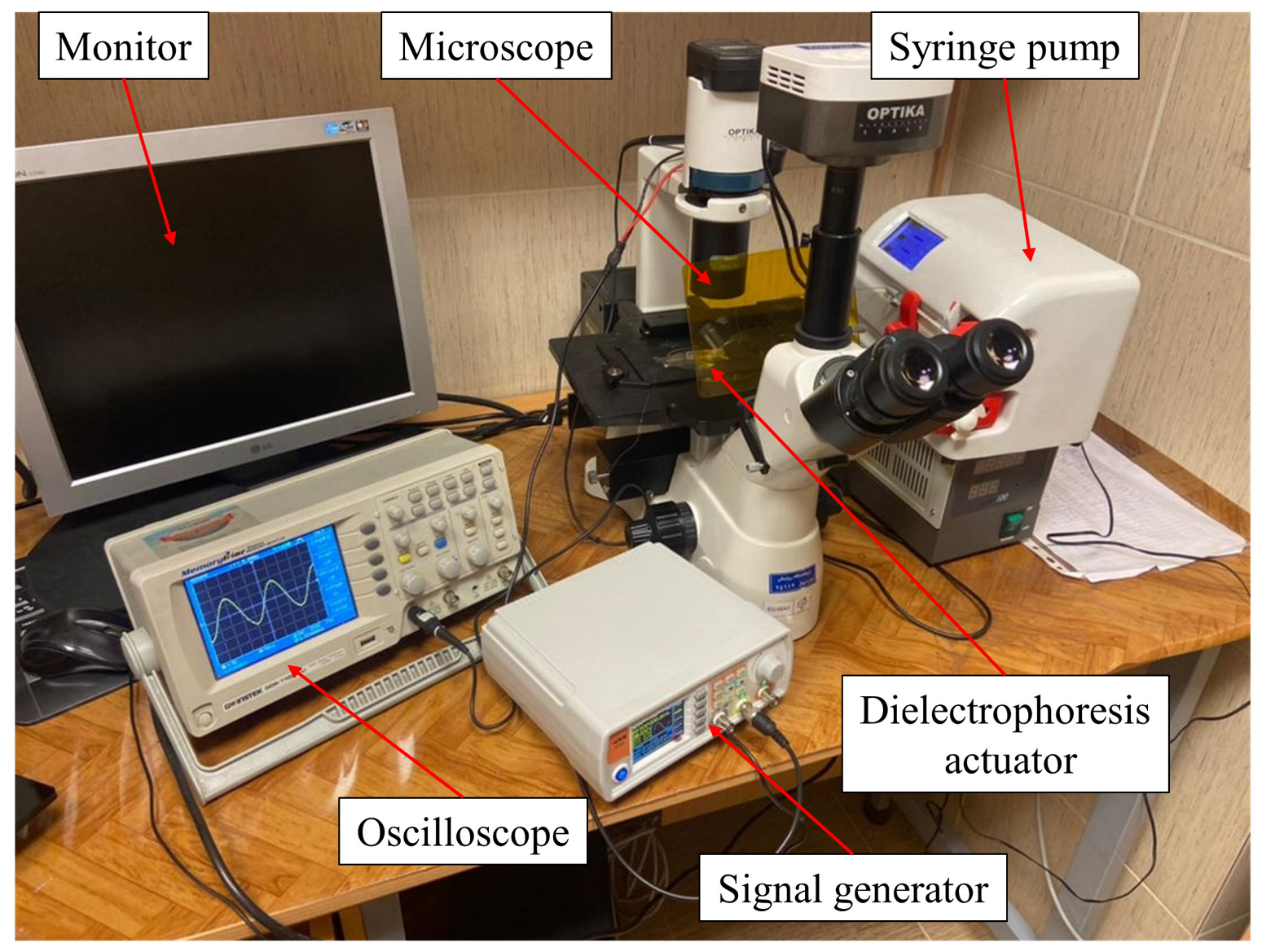
2.6. Laboratory Setup
3. Results and Discussion
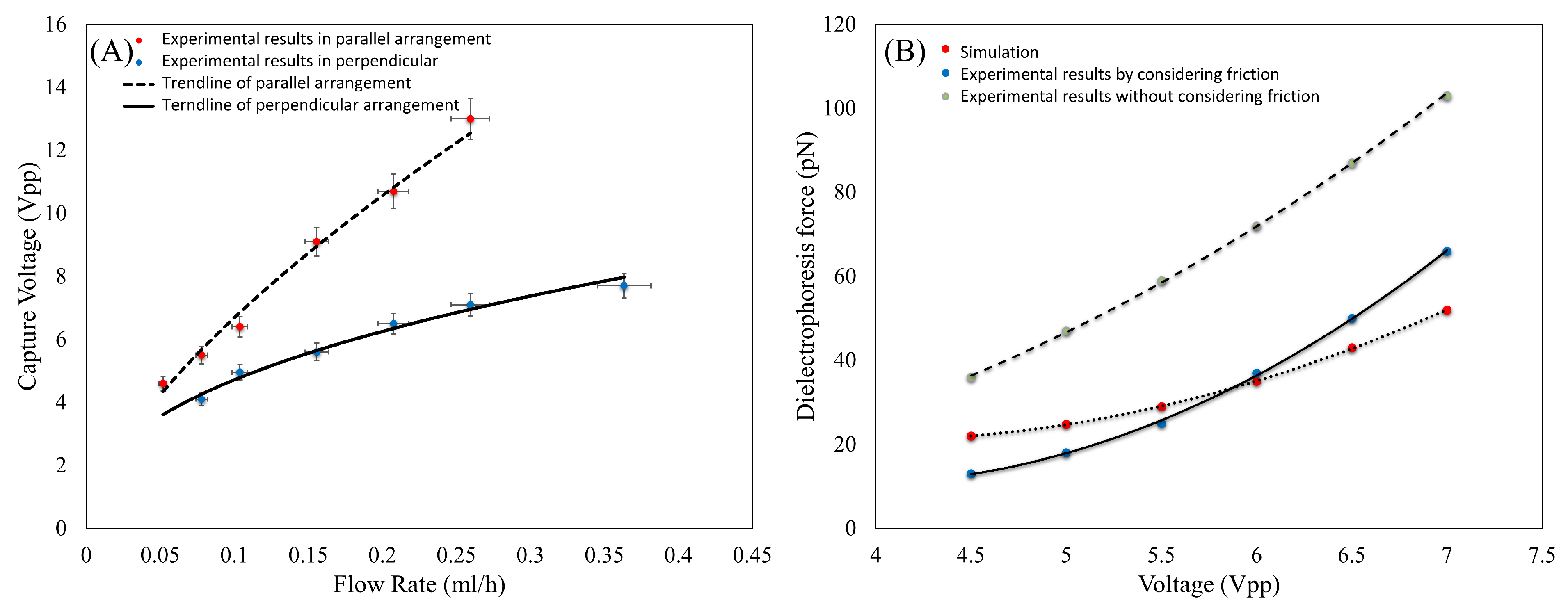
3.1. Simulation Results
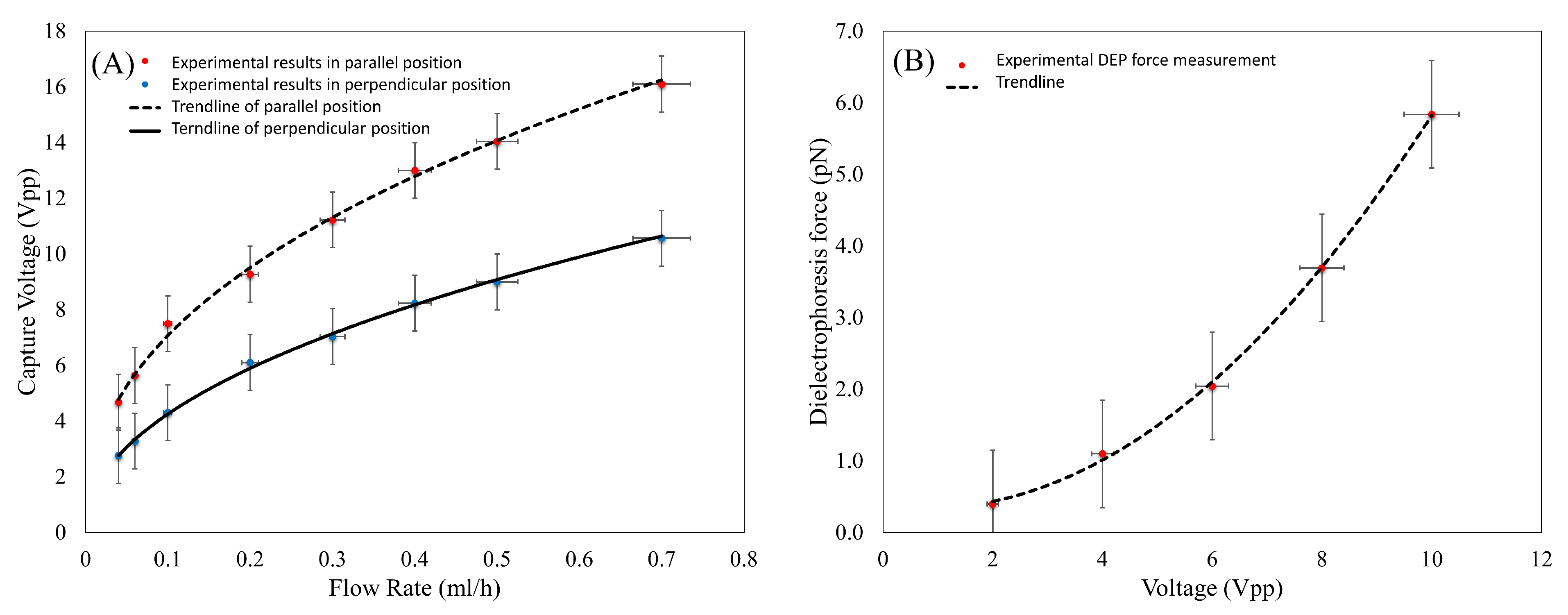
3.2. Experimental DEP Force Measurement
3.3. Validation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brugo-Olmedo, S.; Chillik, C.; Kopelman, S. Definition and causes of infertility. Reprod. Biomed. Online 2001, 2, 41–53. [Google Scholar] [CrossRef] [PubMed]
- Zini, A.; Finelli, A.; Phang, D.; Jarvi, K. Influence of semen processing technique on human sperm DNA integrity. Urology 2000, 56, 1081–1084. [Google Scholar] [CrossRef] [PubMed]
- Sarbandi, I.R.; Lesani, A.; Zand, M.M.; Nosrati, R. Rheotaxis-based sperm separation using a biomimicry microfluidic device. Sci. Rep. 2021, 11, 18327. [Google Scholar] [CrossRef] [PubMed]
- Whitesides, G.M. The origins and the future of microfluidics. Nature 2006, 442, 368–373. [Google Scholar] [CrossRef]
- Reyes, D.R.; Iossifidis, D.; Auroux, P.-A.; Manz, A. Micro Total Analysis Systems. Introduction, Theory, and Technology. Anal. Chem. 2002, 74, 2623–2636. [Google Scholar] [CrossRef]
- Xie, L.; Ma, R.; Han, C.; Su, K.; Zhang, Q.; Qiu, T.; Wang, L.; Huang, G.; Qiao, J.; Wang, J.; et al. Integration of Sperm Motility and Chemotaxis Screening with a Microchannel-Based Device. Clin. Chem. 2010, 56, 1270–1278. [Google Scholar] [CrossRef]
- Pérez-Cerezales, S.; Laguna-Barraza, R.; De Castro, A.C.; Sánchez-Calabuig, M.-J.; Cano-Oliva, E.; De Castro-Pita, F.J.; Montoro-Buils, L.; Pericuesta, E.; Fernández-González, R.; Gutiérrez-Adán, A. Sperm selection by thermotaxis improves ICSI outcome in mice. Sci. Rep. 2018, 8, 2902. [Google Scholar] [CrossRef]
- Ainsworth, C.J.; Nixon, B.; Aitken, R.J. The electrophoretic separation of spermatozoa: An analysis of genotype, surface carbohydrate composition and potential for capacitation. Int. J. Androl. 2011, 34, e422–e434. [Google Scholar] [CrossRef]
- Simon, L.; Murphy, K.; Aston, K.I.; Emery, B.R.; Hotaling, J.M.; Carrell, D.T. Micro-electrophoresis: A noninvasive method of sperm selection based on membrane charge. Fertil. Steril. 2015, 103, 361–366.e3. [Google Scholar] [CrossRef]
- Esfahani, M.H.N.; Deemeh, M.R.; Tavalaee, M.; Sekhavati, M.H.; Gourabi, H. Zeta Sperm Selection Improves Pregnancy Rate and Alters Sex Ratio in Male Factor Infertility Patients: A Double-Blind, Randomized Clinical Trial. Int. J. Fertil. Steril. 2016, 10, 253–260. [Google Scholar] [CrossRef]
- De Wagenaar, B.; Dekker, S.; de Boer, H.L.; Bomer, J.G.; Olthuis, W.; Berg, A.V.D.; Segerink, L.I. Towards microfluidic sperm refinement: Impedance-based analysis and sorting of sperm cells. Lab Chip 2016, 16, 1514–1522. [Google Scholar] [CrossRef] [PubMed]
- Zaman, M.A.; Padhy, P.; Wu, M.; Ren, W.; Jensen, M.A.; Davis, R.W.; Hesselink, L. Controlled Transport of Individual Microparticles Using Dielectrophoresis. Langmuir 2023, 39, 101–110. [Google Scholar] [CrossRef] [PubMed]
- Riccardi, M.; Martin, O.J.F. Electromagnetic Forces and Torques: From Dielectrophoresis to Optical Tweezers. Chem. Rev. 2022, 123, 1680–1711. [Google Scholar] [CrossRef] [PubMed]
- Gascoyne, P.R.C.; Noshari, J.; Anderson, T.J.; Becker, F.F. Isolation of rare cells from cell mixtures by dielectrophoresis. Electrophoresis 2009, 30, 1388–1398. [Google Scholar] [CrossRef]
- Sadeghian, H.; Hojjat, Y.; Soleimani, M. Interdigitated electrode design and optimization for dielectrophoresis cell separation actuators. J. Electrost. 2017, 86, 41–49. [Google Scholar] [CrossRef]
- Li, D.; Yu, W.; Zhou, T.; Li, M.; Song, Y.; Li, D. Conductivity-difference-enhanced DC dielectrophoretic particle separation in a microfluidic chip. Analyst 2022, 147, 1106–1116. [Google Scholar] [CrossRef]
- Coll De Peña, A.; Mohd Redzuan, N.H.; Abajorga, M.K.; Hill, N.; Thomas, J.A.; Lapizco-Encinas, B.H. Analysis of Bacteriophages with Insulator-Based Dielectrophoresis. Micromachines 2019, 10, 450. [Google Scholar] [CrossRef]
- Khoshmanesh, K.; Nahavandi, S.; Baratchi, S.; Mitchell, A.; Kalantar-Zadeh, K. Dielectrophoretic platforms for bio-microfluidic systems. Biosens. Bioelectron. 2011, 26, 1800–1814. [Google Scholar] [CrossRef]
- Fuhr, G.; Muller, T.; Baukloh, V.; Lucas, K. High-frequency electric field trapping of individual human spermatozoa. Hum. Reprod. 1998, 13, 136–141. [Google Scholar] [CrossRef]
- Garcia, M.M.; Ohta, A.T.; Walsh, T.J.; Vittinghof, E.; Lin, G.; Wu, M.C.; Lue, T.F. A Noninvasive, Motility Independent, Sperm Sorting Method and Technology to Identify and Retrieve Individual Viable Nonmotile Sperm for Intracytoplasmic Sperm Injection. J. Urol. 2010, 184, 2466–2472. [Google Scholar] [CrossRef]
- Rosales-Cruzaley, E.; Cota-Elizondo, P.A.; Sanchez, D.; Lapizco-Encinas, B.H. Sperm cells manipulation employing dielectrophoresis. Bioprocess Biosyst. Eng. 2012, 36, 1353–1362. [Google Scholar] [CrossRef]
- Huang, H.-Y.; Kao, W.-L.; Wang, Y.-W.; Yao, D.-J. Using a Dielectrophoretic Microfluidic Biochip Enhanced Fertilization of Mouse Embryo in Vitro. Micromachines 2020, 11, 714. [Google Scholar] [CrossRef]
- Koh, J.B.Y. Marcos Effect of dielectrophoresis on spermatozoa. Microfluid. Nanofluidics 2014, 17, 613–622. [Google Scholar] [CrossRef]
- Wongtawan, T.; Dararatana, N.; Thongkittidilok, C.; Kornmatitsuk, S.; Oonkhanond, B. Enrichment of bovine X-sperm using microfluidic dielectrophoretic chip: A proof-of- concept study. Heliyon 2020, 6, e05483. [Google Scholar] [CrossRef] [PubMed]
- Hughes, M.; Morgan, H. Measurement of Bacterial Flagellar Thrust by Negative Dielectrophoresis. Biotechnol. Prog. 1999, 15, 245–249. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Du, H.; Chen, D.; Shu, C. Analysis of dielectrophoretic electrode arrays for nanoparticle manipulation. Comput. Mater. Sci. 2004, 30, 320–325. [Google Scholar] [CrossRef]
- Tathireddy, P.; Choi, Y.-H.; Skliar, M. Particle AC electrokinetics in planar interdigitated microelectrode geometry. J. Electrost. 2008, 66, 609–619. [Google Scholar] [CrossRef]
- Morgan, H.; Izquierdo, A.G.; Bakewell, D.; Green, N.G.; Ramos, A. The dielectrophoretic and travelling wave forces generated by interdigitated electrode arrays: Analytical solution using Fourier series. J. Phys. D Appl. Phys. 2001, 34, 1553. [Google Scholar] [CrossRef]
- Hong, S.; Kim, C.; Song, H.; An, S.; Yoon, H.; Jhe, W. Measuring Dielectrophoresis Force for Metallic and Non-metallic Particle Manipulations via a Quartz Tuning Fork Atomic Force Microscope. J. Korean Phys. Soc. 2019, 75, 1021–1027. [Google Scholar] [CrossRef]
- Jeon, H.-J.; Lee, H.; Yoon, D.S.; Kim, B.-M. Dielectrophoretic force measurement of red blood cells exposed to oxidative stress using optical tweezers and a microfluidic chip. Biomed. Eng. Lett. 2017, 7, 317–323. [Google Scholar] [CrossRef]
- Imasato, H.; Yamakawa, T. Measurement of dielectrophoretic force by employing controllable gravitational force. J. Electrophor. 2008, 52, 1–8. [Google Scholar] [CrossRef]
- Voldman, J.; Braff, R.A.; Toner, M.; Gray, M.L.; Schmidt, M.A. Holding Forces of Single-Particle Dielectrophoretic Traps. Biophys. J. 2001, 80, 531–542. [Google Scholar] [CrossRef] [PubMed]
- Jones, T.B. Electromechanics of Particles; Cambridge University Press: New York, NY, USA, 1995; ISBN 9780521431965. [Google Scholar]
- Abd Rahman, N.; Ibrahim, F.; Yafouz, B. Dielectrophoresis for Biomedical Sciences Applications: A Review. Sensors 2017, 17, 449. [Google Scholar] [CrossRef] [PubMed]
- Acheson, D.J. Elementary Fluid Dynamics. J. Acoust. Soc. Am. 1991, 89, 3020. [Google Scholar] [CrossRef]
- Bruus, H. Lecture Notes Theoretical Microfluidics. Physics 2008, 18, 363. [Google Scholar]
- Nascimento, J.M.; Shi, L.Z.; Meyers, S.; Gagneux, P.; Loskutoff, N.M.; Botvinick, E.L.; Berns, M. The use of optical tweezers to study sperm competition and motility in primates. J. R. Soc. Interface 2008, 5, 297–302. [Google Scholar] [CrossRef]
- Nosrati, R.; Vollmer, M.; Eamer, L.; Gabriel, M.C.S.; Zeidan, K.; Zini, A.; Sinton, D. Rapid selection of sperm with high DNA integrity. Lab Chip 2014, 14, 1142–1150. [Google Scholar] [CrossRef]
- Shuchat, S.; Park, S.; Kol, S.; Yossifon, G. Distinct and independent dielectrophoretic behavior of the head and tail of sperm and its potential for the safe sorting and isolation of rare spermatozoa. Electrophoresis 2019, 40, 1606–1614. [Google Scholar] [CrossRef]
- Liu, C.; Xue, C.; Sun, J.; Hu, G. A generalized formula for inertial lift on a sphere in microchannels. Lab Chip 2016, 16, 884–892. [Google Scholar] [CrossRef]
- Farajpour, D. A review on the mechanics of inertial microfluidics. J. Comput. Appl. Mech. 2021, 52, 168–192. [Google Scholar] [CrossRef]
- Green, N.G.; Ramos, A.; González, A.; Morgan, H.; Castellanos, A. Fluid flow induced by nonuniform ac electric fields in electrolytes on microelectrodes. III. Observation of streamlines and numerical simulation. Phys. Rev. E 2002, 66, 026305. [Google Scholar] [CrossRef] [PubMed]
- Tada, S.; Seki, Y. Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device. Fluids 2022, 7, 263. [Google Scholar] [CrossRef]
- Younglai, E.; Holt, D.; Brown, P.; Jurisicova, A.; Casper, R. Sperm swim-up techniques and DNA fragmentation. Hum. Reprod. 2001, 16, 1950–1953. [Google Scholar] [CrossRef] [PubMed]
- Maree, L.; Du Plessis, S.; Menkveld, R.; Van Der Horst, G. Morphometric dimensions of the human sperm head depend on the staining method used. Hum. Reprod. 2010, 25, 1369–1382. [Google Scholar] [CrossRef]
- Yang, J.; Huang, Y.; Wang, X.; Wang, X.-B.; Becker, F.F.; Gascoyne, P.R. Dielectric Properties of Human Leukocyte Subpopulations Determined by Electrorotation as a Cell Separation Criterion. Biophys. J. 1999, 76, 3307–3314. [Google Scholar] [CrossRef]
- Siani, O.Z.; Sojoodi, M.; Targhi, M.Z.; Movahedin, M. Blood Particle Separation Using Dielectrophoresis in A Novel Microchannel: A Numerical Study. Cell J. 2019, 22, 218–226. [Google Scholar] [CrossRef]
- Huang, Y.; Wang, X.-B.; Gascoyne, P.R.; Becker, F.F. Membrane dielectric responses of human T-lymphocytes following mitogenic stimulation. Biochim. et Biophys. Acta (BBA) Biomembr. 1999, 1417, 51–62. [Google Scholar] [CrossRef]
- Barnkob, R.; Augustsson, P.; Magnusson, C.; Lilja, H.; Laurell, T.; Bruus, H. Measuring Density and Compressibility of White Blood Cells and Prostate Cancer Cells by Microchannel Acoustophoresis. 15th International Conference on Miniaturized Systems for Chemistry and Life Sciences 2011. MicroTAS 2011, 1, 127–129. [Google Scholar]
- Malvezzi, H.; Sharma, R.; Agarwal, A.; Abuzenadah, A.M.; Abu-Elmagd, M. Sperm quality after density gradient centrifugation with three commercially available media: A controlled trial. Reprod. Biol. Endocrinol. 2014, 12, 121. [Google Scholar] [CrossRef]
- Swindells, J.F.; Snyder, C.F.; Hardy, R.C.; Golden, P.E. Viscosities of Sucrose Solutions at Various Temperatures: Tables of Recalculated Values. Suppl. Natl. Bur. Stand. Circ. 1958, 440, 1–7. [Google Scholar]
- Ghomian, T.; Hihath, J. Review of Dielectrophoretic Manipulation of Micro and Nanomaterials: Fundamentals, Recent Developments, and Challenges. IEEE Trans. Biomed. Eng. 2023, 70, 27–41. [Google Scholar] [CrossRef] [PubMed]
- Huang, H.-Y.; Huang, Y.-H.; Kao, W.-L.; Yao, D.-J. Embryo formation from low sperm concentration by using dielectrophoretic force. Biomicrofluidics 2015, 9, 022404. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khouzestani, A.; Hojjat, Y.; Tavalaee, M.; Sadeghian, H.; Nasr-Esfahani, M.H. Enhancing the Accuracy of Measuring DEP Force Applied on Cells by Considering the Friction Effect. Biosensors 2023, 13, 540. https://doi.org/10.3390/bios13050540
Khouzestani A, Hojjat Y, Tavalaee M, Sadeghian H, Nasr-Esfahani MH. Enhancing the Accuracy of Measuring DEP Force Applied on Cells by Considering the Friction Effect. Biosensors. 2023; 13(5):540. https://doi.org/10.3390/bios13050540
Chicago/Turabian StyleKhouzestani, Alireza, Yousef Hojjat, Marziyeh Tavalaee, Hesam Sadeghian, and Mohammad Hossein Nasr-Esfahani. 2023. "Enhancing the Accuracy of Measuring DEP Force Applied on Cells by Considering the Friction Effect" Biosensors 13, no. 5: 540. https://doi.org/10.3390/bios13050540
APA StyleKhouzestani, A., Hojjat, Y., Tavalaee, M., Sadeghian, H., & Nasr-Esfahani, M. H. (2023). Enhancing the Accuracy of Measuring DEP Force Applied on Cells by Considering the Friction Effect. Biosensors, 13(5), 540. https://doi.org/10.3390/bios13050540




