Advances in Stabilization and Enrichment of Shallow Nitrogen-Vacancy Centers in Diamond for Biosensing and Spin-Polarization Transfer
Abstract
1. Introduction
2. Basic Physics of NV Centers

3. Charge Stabilization by Doping and Surface Termination
4. Laser-Induced Charge Switching
5. Applications
5.1. Charge-Conversion-Based Applications
5.2. Applications That Require Stabilization of Charges
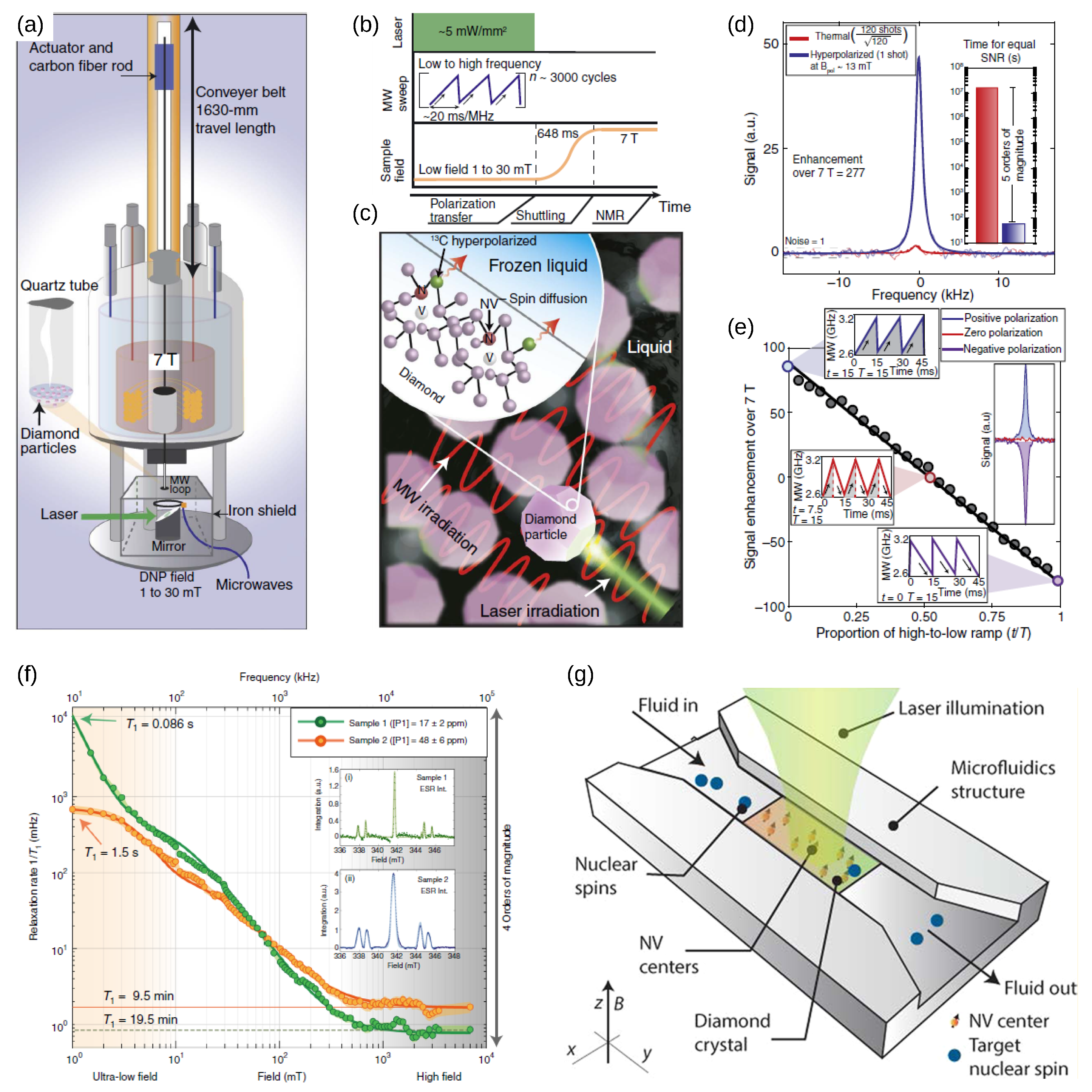
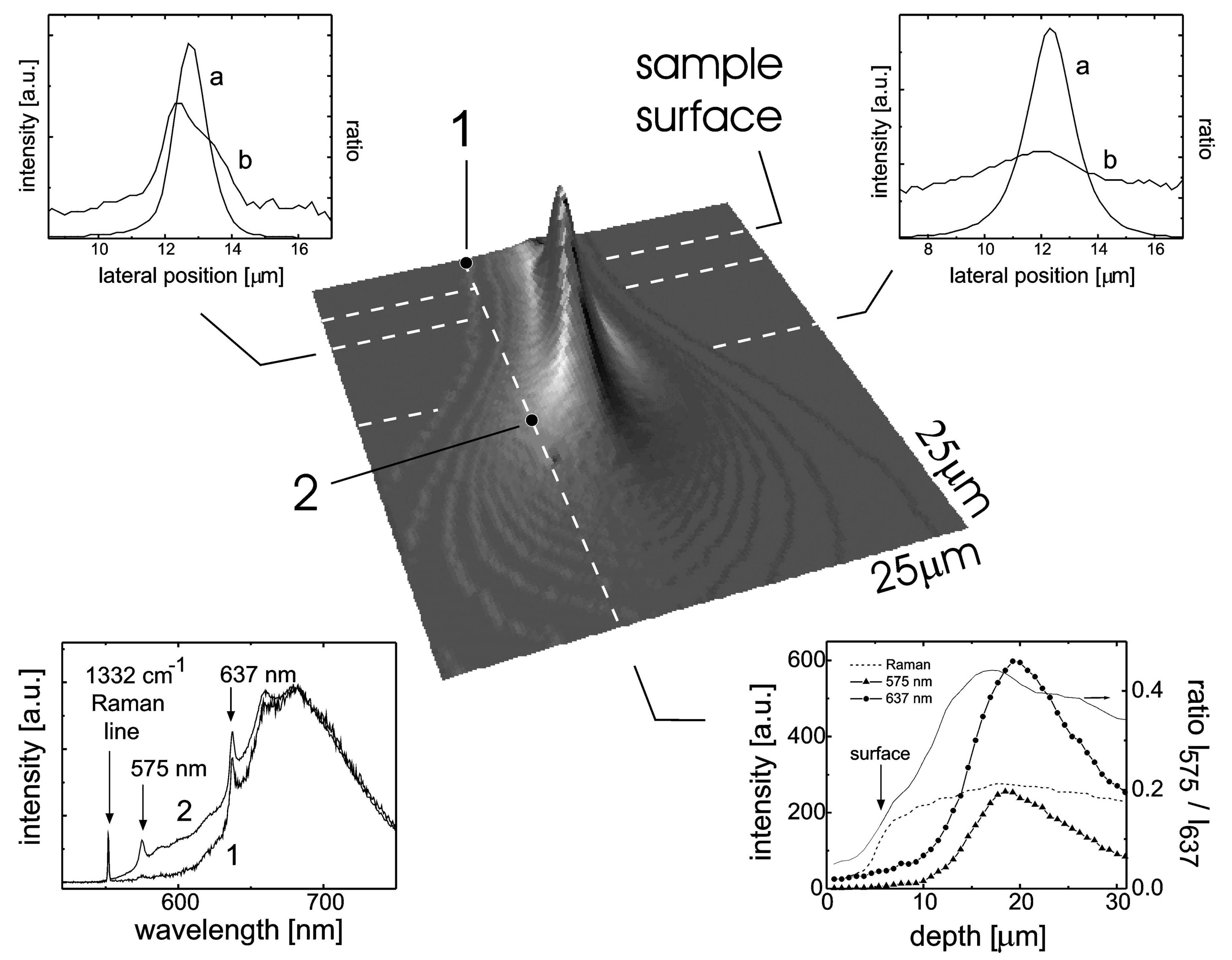
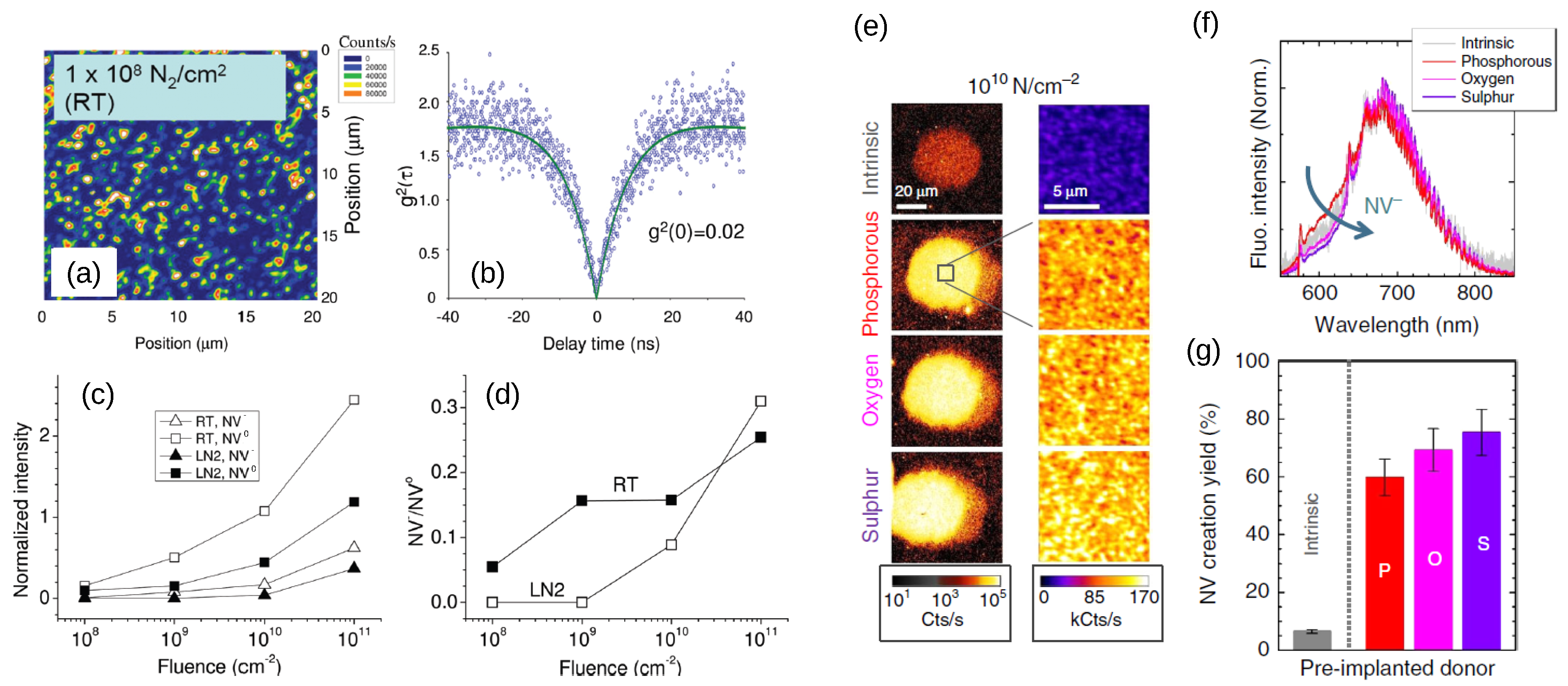
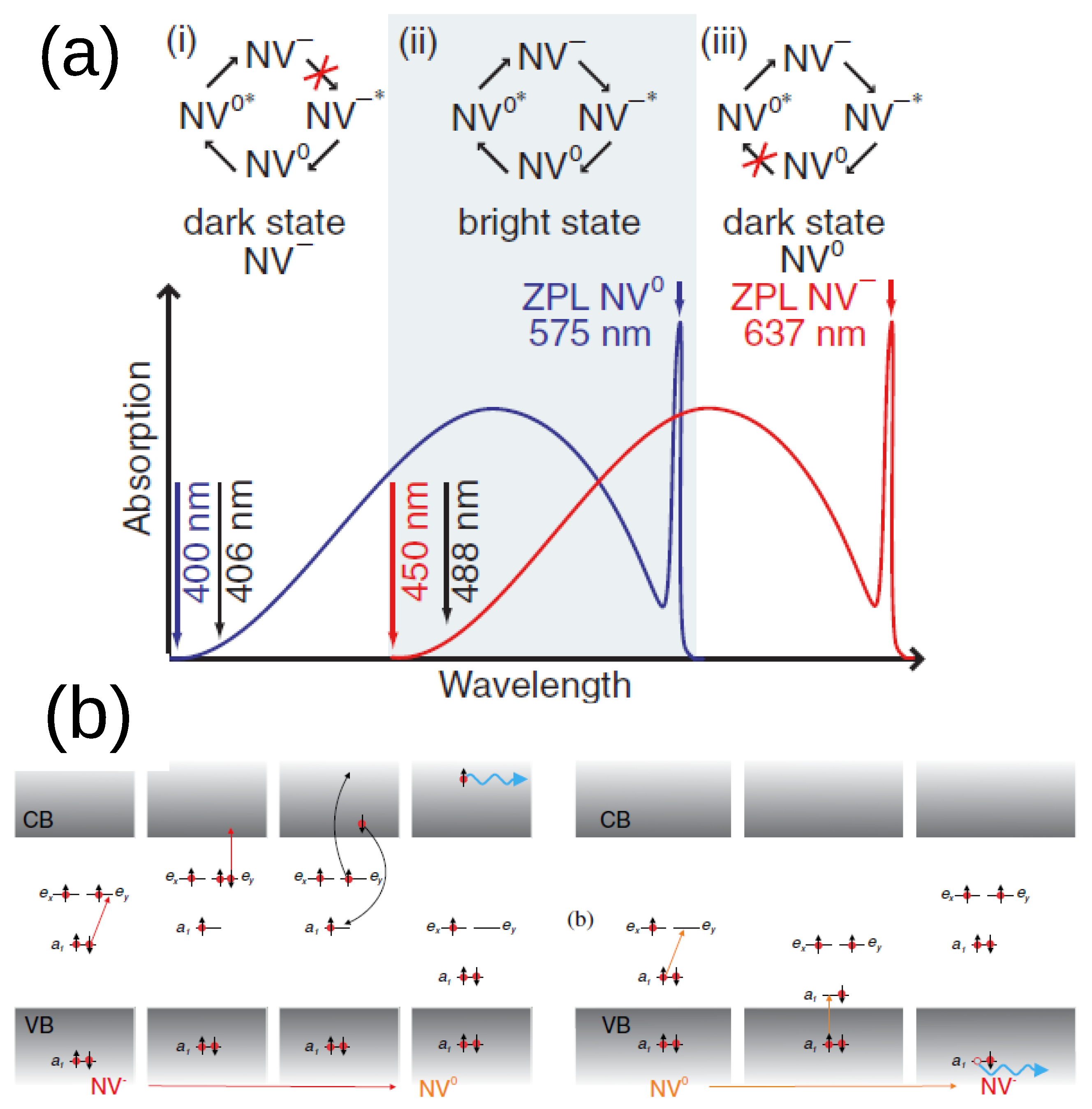
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kurtsiefer, C.; Mayer, S.; Zarda, P.; Weinfurter, H. Stable solid-state source of single photons. Phys. Rev. Lett. 2000, 85, 290. [Google Scholar] [CrossRef] [PubMed]
- Beveratos, A.; Kühn, S.; Brouri, R.; Gacoin, T.; Poizat, J.P.; Grangier, P. Room temperature stable single-photon source. Eur. Phys. J. D-At. Mol. Opt. Plasma Phys. 2002, 18, 191–196. [Google Scholar] [CrossRef]
- Babinec, T.M.; Hausmann, B.J.; Khan, M.; Zhang, Y.; Maze, J.R.; Hemmer, P.R.; Lončar, M. A diamond nanowire single-photon source. Nat. Nanotechnol. 2010, 5, 195–199. [Google Scholar] [CrossRef]
- Schröder, T.; Gädeke, F.; Banholzer, M.J.; Benson, O. Ultrabright and efficient single-photon generation based on nitrogen-vacancy centres in nanodiamonds on a solid immersion lens. New J. Phys. 2011, 13, 055017. [Google Scholar] [CrossRef]
- Balasubramanian, G.; Neumann, P.; Twitchen, D.; Markham, M.; Kolesov, R.; Mizuochi, N.; Isoya, J.; Achard, J.; Beck, J.; Tissler, J.; et al. Ultralong spin coherence time in isotopically engineered diamond. Nat. Mater. 2009, 8, 383–387. [Google Scholar] [CrossRef]
- Stanwix, P.L.; Pham, L.M.; Maze, J.R.; Le Sage, D.; Yeung, T.K.; Cappellaro, P.; Hemmer, P.R.; Yacoby, A.; Lukin, M.D.; Walsworth, R.L. Coherence of nitrogen-vacancy electronic spin ensembles in diamond. Phys. Rev. B 2010, 82, 201201. [Google Scholar] [CrossRef]
- Davis, H.C.; Ramesh, P.; Bhatnagar, A.; Lee-Gosselin, A.; Barry, J.F.; Glenn, D.R.; Walsworth, R.L.; Shapiro, M.G. Mapping the microscale origins of magnetic resonance image contrast with subcellular diamond magnetometry. Nat. Commun. 2018, 9, 131. [Google Scholar] [CrossRef]
- Schloss, J.M.; Barry, J.F.; Turner, M.J.; Walsworth, R.L. Simultaneous broadband vector magnetometry using solid-state spins. Phys. Rev. Appl. 2018, 10, 034044. [Google Scholar] [CrossRef]
- Iwasaki, T.; Naruki, W.; Tahara, K.; Makino, T.; Kato, H.; Ogura, M.; Takeuchi, D.; Yamasaki, S.; Hatano, M. Direct nanoscale sensing of the internal electric field in operating semiconductor devices using single electron spins. ACS Nano 2017, 11, 1238–1245. [Google Scholar] [CrossRef] [PubMed]
- Forneris, J.; Tchernij, S.D.; Traina, P.; Moreva, E.; Skukan, N.; Jakšić, M.; Grilj, V.; Bosia, F.; Enrico, E.; Amato, G.; et al. Mapping the Local Spatial Charge in Defective Diamond by Means of N-V Sensors—A Self-Diagnostic Concept. Phys. Rev. Appl. 2018, 10, 014024. [Google Scholar] [CrossRef]
- Kucsko, G.; Maurer, P.C.; Yao, N.Y.; Kubo, M.; Noh, H.J.; Lo, P.K.; Park, H.; Lukin, M.D. Nanometre-scale thermometry in a living cell. Nature 2013, 500, 54–58. [Google Scholar] [CrossRef]
- Singam, S.K.; Nesladek, M.; Goovaerts, E. Nitrogen-vacancy nanodiamond based local thermometry using frequency-jump modulation. Nanotechnology 2019, 31, 105501. [Google Scholar] [CrossRef] [PubMed]
- Barone, F.C.; Marcinkiewicz, C.; Li, J.; Sternberg, M.; Lelkes, P.I.; Dikin, D.A.; Bergold, P.J.; Gerstenhaber, J.A.; Feuerstein, G. Pilot study on biocompatibility of fluorescent nanodiamond-(NV)-Z∼ 800 particles in rats: Safety, pharmacokinetics, and bio-distribution (part III). Int. J. Nanomed. 2018, 13, 5449. [Google Scholar] [CrossRef] [PubMed]
- Moore, L.; Yang, J.; Lan, T.T.H.; Osawa, E.; Lee, D.K.; Johnson, W.D.; Xi, J.; Chow, E.K.H.; Ho, D. Biocompatibility assessment of detonation nanodiamond in non-human primates and rats using histological, hematologic, and urine analysis. ACS Nano 2016, 10, 7385–7400. [Google Scholar] [CrossRef]
- van der Laan, K.; Hasani, M.; Zheng, T.; Schirhagl, R. Nanodiamonds for in vivo applications. Small 2018, 14, 1703838. [Google Scholar]
- Chipaux, M.; van der Laan, K.J.; Hemelaar, S.R.; Hasani, M.; Zheng, T.; Schirhagl, R. Nanodiamonds and their applications in cells. Small 2018, 14, 1704263. [Google Scholar] [CrossRef] [PubMed]
- Han, K.Y.; Kim, S.K.; Eggeling, C.; Hell, S.W. Metastable dark states enable ground state depletion microscopy of nitrogen vacancy centers in diamond with diffraction-unlimited resolution. Nano Lett. 2010, 10, 3199–3203. [Google Scholar] [CrossRef]
- Chen, X.; Zou, C.; Gong, Z.; Dong, C.; Guo, G.; Sun, F. Subdiffraction optical manipulation of the charge state of nitrogen vacancy center in diamond. Light. Sci. Appl. 2015, 4, e230. [Google Scholar] [CrossRef]
- Li, S.; Chen, X.d.; Zhao, B.W.; Dong, Y.; Zou, C.W.; Guo, G.C.; Sun, F.W. Optical far-field super-resolution microscopy using nitrogen vacancy center ensemble in bulk diamond. Appl. Phys. Lett. 2016, 109, 111107. [Google Scholar] [CrossRef]
- Moosa, B.; Fhayli, K.; Li, S.; Julfakyan, K.; Ezzeddine, A.; Khashab, N.M. Applications of nanodiamonds in drug delivery and catalysis. J. Nanosci. Nanotechnol. 2014, 14, 332–343. [Google Scholar] [CrossRef]
- Lim, D.G.; Prim, R.E.; Kim, K.H.; Kang, E.; Park, K.; Jeong, S.H. Combinatorial nanodiamond in pharmaceutical and biomedical applications. Int. J. Pharm. 2016, 514, 41–51. [Google Scholar] [CrossRef]
- Perevedentseva, E.; Lin, Y.C.; Jani, M.; Cheng, C.L. Biomedical applications of nanodiamonds in imaging and therapy. Nanomedicine 2013, 8, 2041–2060. [Google Scholar] [CrossRef]
- Liu, Y.Y.; Chang, B.M.; Chang, H.C. Nanodiamond-enabled biomedical imaging. Nanomedicine 2020, 15, 1599–1616. [Google Scholar] [CrossRef]
- Kaufmann, S.; Simpson, D.A.; Hall, L.T.; Perunicic, V.; Senn, P.; Steinert, S.; McGuinness, L.P.; Johnson, B.C.; Ohshima, T.; Caruso, F.; et al. Detection of atomic spin labels in a lipid bilayer using a single-spin nanodiamond probe. Proc. Natl. Acad. Sci. USA 2013, 110, 10894–10898. [Google Scholar] [CrossRef]
- Ermakova, A.; Pramanik, G.; Cai, J.M.; Algara-Siller, G.; Kaiser, U.; Weil, T.; Tzeng, Y.K.; Chang, H.C.; McGuinness, L.; Plenio, M.B.; et al. Detection of a few metallo-protein molecules using color centers in nanodiamonds. Nano Lett. 2013, 13, 3305–3309. [Google Scholar] [CrossRef]
- Gorrini, F.; Giri, R.; Avalos, C.; Tambalo, S.; Mannucci, S.; Basso, L.; Bazzanella, N.; Dorigoni, C.; Cazzanelli, M.; Marzola, P.; et al. Fast and sensitive detection of paramagnetic species using coupled charge and spin dynamics in strongly fluorescent nanodiamonds. ACS Appl. Mater. Interfaces 2019, 11, 24412–24422. [Google Scholar] [CrossRef]
- Ajoy, A.; Bissbort, U.; Lukin, M.D.; Walsworth, R.L.; Cappellaro, P. Atomic-scale nuclear spin imaging using quantum-assisted sensors in diamond. Phys. Rev. X 2015, 5, 011001. [Google Scholar] [CrossRef]
- Sushkov, A.O.; Lovchinsky, I.; Chisholm, N.; Walsworth, R.L.; Park, H.; Lukin, M.D. Magnetic resonance detection of individual proton spins using quantum reporters. Phys. Rev. Lett. 2014, 113, 197601. [Google Scholar] [CrossRef] [PubMed]
- Fischer, R.; Jarmola, A.; Kehayias, P.; Budker, D. Optical polarization of nuclear ensembles in diamond. Phys. Rev. B 2013, 87, 125207. [Google Scholar] [CrossRef]
- King, J.P.; Jeong, K.; Vassiliou, C.C.; Shin, C.S.; Page, R.H.; Avalos, C.E.; Wang, H.J.; Pines, A. Room-temperature in situ nuclear spin hyperpolarization from optically pumped nitrogen vacancy centres in diamond. Nat. Commun. 2015, 6, 8965. [Google Scholar] [CrossRef] [PubMed]
- Pezzagna, S.; Naydenov, B.; Jelezko, F.; Wrachtrup, J.; Meijer, J. Creation efficiency of nitrogen-vacancy centres in diamond. New J. Phys. 2010, 12, 065017. [Google Scholar] [CrossRef]
- Andrich, P.; Li, J.; Liu, X.; Heremans, F.J.; Nealey, P.F.; Awschalom, D.D. Microscale-resolution thermal mapping using a flexible platform of patterned quantum sensors. Nano Lett. 2018, 18, 4684–4690. [Google Scholar] [CrossRef]
- Trusheim, M.E.; Li, L.; Laraoui, A.; Chen, E.H.; Bakhru, H.; Schröder, T.; Gaathon, O.; Meriles, C.A.; Englund, D. Scalable fabrication of high purity diamond nanocrystals with long-spin-coherence nitrogen vacancy centers. Nano Lett. 2014, 14, 32–36. [Google Scholar] [CrossRef] [PubMed]
- McCloskey, D.J.; Dontschuk, N.; Broadway, D.A.; Nadarajah, A.; Stacey, A.; Tetienne, J.P.; Hollenberg, L.C.; Prawer, S.; Simpson, D.A. Enhanced widefield quantum sensing with nitrogen-vacancy ensembles using diamond nanopillar arrays. ACS Appl. Mater. Interfaces 2020, 12, 13421–13427. [Google Scholar] [CrossRef] [PubMed]
- Gorrini, F.; Dorigoni, C.; Olivares-Postigo, D.; Giri, R.; Aprà, P.; Picollo, F.; Bifone, A. Long-lived ensembles of shallow NV–centers in flat and nanostructured diamonds by photoconversion. ACS Appl. Mater. Interfaces 2021, 13, 43221–43232. [Google Scholar] [CrossRef] [PubMed]
- Hauf, M.; Grotz, B.; Naydenov, B.; Dankerl, M.; Pezzagna, S.; Meijer, J.; Jelezko, F.; Wrachtrup, J.; Stutzmann, M.; Reinhard, F.; et al. Chemical control of the charge state of nitrogen-vacancy centers in diamond. Phys. Rev. B 2011, 83, 081304. [Google Scholar] [CrossRef]
- Bluvstein, D.; Zhang, Z.; Jayich, A.C.B. Identifying and mitigating charge instabilities in shallow diamond nitrogen-vacancy centers. Phys. Rev. Lett. 2019, 122, 076101. [Google Scholar] [CrossRef]
- Yuan, Z.; Fitzpatrick, M.; Rodgers, L.V.; Sangtawesin, S.; Srinivasan, S.; De Leon, N.P. Charge state dynamics and optically detected electron spin resonance contrast of shallow nitrogen-vacancy centers in diamond. Phys. Rev. Res. 2020, 2, 033263. [Google Scholar] [CrossRef]
- Manson, N.; Harrison, J. Photo-ionization of the nitrogen-vacancy center in diamond. Diam. Relat. Mater. 2005, 14, 1705–1710. [Google Scholar] [CrossRef]
- Beha, K.; Batalov, A.; Manson, N.B.; Bratschitsch, R.; Leitenstorfer, A. Optimum photoluminescence excitation and recharging cycle of single nitrogen-vacancy centers in ultrapure diamond. Phys. Rev. Lett. 2012, 109, 097404. [Google Scholar] [CrossRef]
- Aslam, N.; Waldherr, G.; Neumann, P.; Jelezko, F.; Wrachtrup, J. Photo-induced ionization dynamics of the nitrogen vacancy defect in diamond investigated by single-shot charge state detection. New J. Phys. 2013, 15, 013064. [Google Scholar] [CrossRef]
- Schreyvogel, C.; Polyakov, V.; Wunderlich, R.; Meijer, J.; Nebel, C. Active charge state control of single NV centres in diamond by in-plane Al-Schottky junctions. Sci. Rep. 2015, 5, 12160. [Google Scholar] [CrossRef] [PubMed]
- Meara, C.J.; Rayson, M.J.; Briddon, P.R.; Goss, J.P. Density functional theory study on magnetically detecting positively charged nitrogen-vacancy center in diamond. Phys. Rev. B 2019, 100, 104108. [Google Scholar] [CrossRef]
- Karim, A.; Lyskov, I.; Russo, S.P.; Peruzzo, A. Bright ab initio photoluminescence of NV+ in diamond. J. Appl. Phys. 2021, 130, 234402. [Google Scholar] [CrossRef]
- Davies, G. The effect of nitrogen impurity on the annealing of radiation damage in diamond. J. Phys. C Solid State Phys. 1972, 5, 2534. [Google Scholar] [CrossRef]
- Davies, G.; Hamer, M. Optical studies of the 1.945 eV vibronic band in diamond. Proc. R. Soc. Lond. A Math. Phys. Sci. 1976, 348, 285–298. [Google Scholar]
- Fuchs, G.; Dobrovitski, V.; Toyli, D.; Heremans, F.; Weis, C.; Schenkel, T.; Awschalom, D. Excited-state spin coherence of a single nitrogen–vacancy centre in diamond. Nat. Phys. 2010, 6, 668–672. [Google Scholar] [CrossRef]
- Gruber, A.; Drabenstedt, A.; Tietz, C.; Fleury, L.; Wrachtrup, J.; Borczyskowski, C.v. Scanning confocal optical microscopy and magnetic resonance on single defect centers. Science 1997, 276, 2012–2014. [Google Scholar] [CrossRef]
- Doherty, M.; Dolde, F.; Fedder, H.; Jelezko, F.; Wrachtrup, J.; Manson, N.; Hollenberg, L. Theory of the ground-state spin of the NV- center in diamond. Phys. Rev. B 2012, 85, 205203. [Google Scholar] [CrossRef]
- Schirhagl, R.; Chang, K.; Loretz, M.; Degen, C.L. Nitrogen-vacancy centers in diamond: Nanoscale sensors for physics and biology. Annu. Rev. Phys. Chem. 2014, 65, 83–105. [Google Scholar] [CrossRef]
- Rondin, L.; Tetienne, J.P.; Hingant, T.; Roch, J.F.; Maletinsky, P.; Jacques, V. Magnetometry with nitrogen-vacancy defects in diamond. Rep. Prog. Phys. 2014, 77, 056503. [Google Scholar] [CrossRef]
- Balasubramanian, G.; Chan, I.; Kolesov, R.; Al-Hmoud, M.; Tisler, J.; Shin, C.; Kim, C.; Wojcik, A.; Hemmer, P.R.; Krueger, A.; et al. Nanoscale imaging magnetometry with diamond spins under ambient conditions. Nature 2008, 455, 648–651. [Google Scholar] [CrossRef]
- Maze, J.R.; Stanwix, P.L.; Hodges, J.S.; Hong, S.; Taylor, J.M.; Cappellaro, P.; Jiang, L.; Dutt, M.G.; Togan, E.; Zibrov, A.; et al. Nanoscale magnetic sensing with an individual electronic spin in diamond. Nature 2008, 455, 644–647. [Google Scholar] [CrossRef] [PubMed]
- Collins, A.; Lawson, S. Cathodoluminescence studies of isotope shifts associated with localised vibrational modes in synthetic diamond. J. Phys. Condens. Matter 1989, 1, 6929. [Google Scholar] [CrossRef]
- Mita, Y. Change of absorption spectra in type-Ib diamond with heavy neutron irradiation. Phys. Rev. B 1996, 53, 11360. [Google Scholar] [CrossRef] [PubMed]
- Davies, G. Dynamic Jahn-Teller distortions at trigonal optical centres in diamond. J. Phys. C Solid State Phys. 1979, 12, 2551. [Google Scholar] [CrossRef]
- Collins, A.T. The characterisation of point defects in diamond by luminescence spectroscopy. Diam. Relat. Mater. 1992, 1, 457–469. [Google Scholar] [CrossRef]
- Lu, H.C.; Lo, J.I.; Peng, Y.C.; Cheng, B.M. Photoluminescence of diamond containing nitrogen vacancy defects as a sensor of temperature upon exposure to vacuum-and extreme-ultraviolet radiation. Phys. Chem. Chem. Phys. 2020, 22, 26982–26986. [Google Scholar] [CrossRef]
- Subedi, S.D.; Fedorov, V.V.; Peppers, J.; Martyshkin, D.V.; Mirov, S.B.; Shao, L.; Loncar, M. Laser spectroscopic characterization of negatively charged nitrogen-vacancy (NV-) centers in diamond. Opt. Mater. Express 2019, 9, 2076–2087. [Google Scholar] [CrossRef]
- Deák, P.; Aradi, B.; Kaviani, M.; Frauenheim, T.; Gali, A. Formation of NV centers in diamond: A theoretical study based on calculated transitions and migration of nitrogen and vacancy related defects. Phys. Rev. B 2014, 89, 075203. [Google Scholar] [CrossRef]
- Iakoubovskii, K.; Adriaenssens, G.; Nesladek, M. Photochromism of vacancy-related centres in diamond. J. Phys. Condens. Matter 2000, 12, 189. [Google Scholar] [CrossRef]
- Acosta, V.M.; Bauch, E.; Ledbetter, M.P.; Santori, C.; Fu, K.M.; Barclay, P.E.; Beausoleil, R.G.; Linget, H.; Roch, J.F.; Treussart, F.; et al. Diamonds with a high density of nitrogen-vacancy centers for magnetometry applications. Phys. Rev. B 2009, 80, 115202. [Google Scholar] [CrossRef]
- Martin, J.; Wannemacher, R.; Teichert, J.; Bischoff, L.; Köhler, B. Generation and detection of fluorescent color centers in diamond with submicron resolution. Appl. Phys. Lett. 1999, 75, 3096–3098. [Google Scholar] [CrossRef]
- Santori, C.; Barclay, P.E.; Fu, K.M.C.; Beausoleil, R.G. Vertical distribution of nitrogen-vacancy centers in diamond formed by ion implantation and annealing. Phys. Rev. B 2009, 79, 125313. [Google Scholar] [CrossRef]
- Greentree, A.D.; Olivero, P.; Draganski, M.; Trajkov, E.; Rabeau, J.R.; Reichart, P.; Gibson, B.C.; Rubanov, S.; Huntington, S.T.; Jamieson, D.N.; et al. Critical components for diamond-based quantum coherent devices. J. Phys. Condens. Matter 2006, 18, S825. [Google Scholar] [CrossRef]
- Feng, F.; Zhang, W.; Zhang, J.; Lou, L.; Zhu, W.; Wang, G. Optimizing the density of nitrogen implantation for generating high-density NV center ensembles for quantum sensing. Eur. Phys. J. D 2019, 73, 202. [Google Scholar] [CrossRef]
- Orwa, J.; Santori, C.; Fu, K.; Gibson, B.; Simpson, D.; Aharonovich, I.; Stacey, A.; Cimmino, A.; Balog, P.; Markham, M.; et al. Engineering of nitrogen-vacancy color centers in high purity diamond by ion implantation and annealing. J. Appl. Phys. 2011, 109, 083530. [Google Scholar] [CrossRef]
- Ofori-Okai, B.; Pezzagna, S.; Chang, K.; Loretz, M.; Schirhagl, R.; Tao, Y.; Moores, B.; Groot-Berning, K.; Meijer, J.; Degen, C. Spin properties of very shallow nitrogen vacancy defects in diamond. Phys. Rev. B 2012, 86, 081406. [Google Scholar] [CrossRef]
- Osterkamp, C.; Scharpf, J.; Pezzagna, S.; Meijer, J.; Diemant, T.; Jürgen Behm, R.; Naydenov, B.; Jelezko, F. Increasing the creation yield of shallow single defects in diamond by surface plasma treatment. Appl. Phys. Lett. 2013, 103, 193118. [Google Scholar] [CrossRef]
- Waldermann, F.; Olivero, P.; Nunn, J.; Surmacz, K.; Wang, Z.; Jaksch, D.; Taylor, R.; Walmsley, I.; Draganski, M.; Reichart, P.; et al. Creating diamond color centers for quantum optical applications. Diam. Relat. Mater. 2007, 16, 1887–1895. [Google Scholar] [CrossRef]
- Martin, J.; Grebner, W.; Sigle, W.; Wannemacher, R. Confocal microscopy of color center distributions in diamond. J. Lumin. 1999, 83, 493–497. [Google Scholar] [CrossRef]
- Jadidi, M.F.; Özer, H.Ö.; Goel, S.; Kilpatrick, J.I.; McEvoy, N.; McCloskey, D.; Donegan, J.F.; Cross, G.L. Distribution of shallow NV centers in diamond revealed by photoluminescence spectroscopy and nanomachining. Carbon 2020, 167, 114–121. [Google Scholar] [CrossRef]
- Naydenov, B.; Reinhard, F.; Lämmle, A.; Richter, V.; Kalish, R.; D’Haenens-Johansson, U.F.; Newton, M.; Jelezko, F.; Wrachtrup, J. Increasing the coherence time of single electron spins in diamond by high temperature annealing. Appl. Phys. Lett. 2010, 97, 242511. [Google Scholar] [CrossRef]
- Gaebel, T.; Domhan, M.; Wittmann, C.; Popa, I.; Jelezko, F.; Rabeau, J.; Greentree, A.; Prawer, S.; Trajkov, E.; Hemmer, P.R.; et al. Photochromism in single nitrogen-vacancy defect in diamond. Appl. Phys. B 2006, 82, 243–246. [Google Scholar] [CrossRef]
- Zaitsev, A.; Moe, K.; Wang, W. Defect transformations in nitrogen-doped CVD diamond during irradiation and annealing. Diam. Relat. Mater. 2018, 88, 237–255. [Google Scholar] [CrossRef]
- Barry, J.F.; Schloss, J.M.; Bauch, E.; Turner, M.J.; Hart, C.A.; Pham, L.M.; Walsworth, R.L. Sensitivity optimization for NV-diamond magnetometry. Rev. Mod. Phys. 2020, 92, 015004. [Google Scholar] [CrossRef]
- Vaddiraju, S.; Eaton-Magana, S.; Chaney, J.A.; Sunkara, M.K. UPS of boron-sulfur Co-doped, n-type diamond. Electrochem. Solid-State Lett. 2004, 7, G331. [Google Scholar] [CrossRef]
- Doi, Y.; Fukui, T.; Kato, H.; Makino, T.; Yamasaki, S.; Tashima, T.; Morishita, H.; Miwa, S.; Jelezko, F.; Suzuki, Y.; et al. Pure negatively charged state of the NV center in n-type diamond. Phys. Rev. B 2016, 93, 081203. [Google Scholar] [CrossRef]
- Watanabe, A.; Nishikawa, T.; Kato, H.; Fujie, M.; Fujiwara, M.; Makino, T.; Yamasaki, S.; Herbschleb, E.; Mizuochi, N. Shallow NV centers augmented by exploiting n-type diamond. Carbon 2021, 178, 294–300. [Google Scholar] [CrossRef]
- Lühmann, T.; John, R.; Wunderlich, R.; Meijer, J.; Pezzagna, S. Coulomb-driven single defect engineering for scalable qubits and spin sensors in diamond. Nat. Commun. 2019, 10, 4956. [Google Scholar] [CrossRef]
- Hayashi, K.; Yamanaka, S.; Watanabe, H.; Sekiguchi, T.; Okushi, H.; Kajimura, K. Investigation of the effect of hydrogen on electrical and optical properties in chemical vapor deposited on homoepitaxial diamond films. J. Appl. Phys. 1997, 81, 744–753. [Google Scholar] [CrossRef]
- Kawarada, H. Hydrogen-terminated diamond surfaces and interfaces. Surf. Sci. Rep. 1996, 26, 205–259. [Google Scholar] [CrossRef]
- Sowers, A.; Ward, B.; English, S.; Nemanich, R. Field emission properties of nitrogen-doped diamond films. J. Appl. Phys. 1999, 86, 3973–3982. [Google Scholar] [CrossRef]
- Ristein, J. Surface transfer doping of diamond. J. Phys. D Appl. Phys. 2006, 39, R71. [Google Scholar] [CrossRef]
- Karaveli, S.; Gaathon, O.; Wolcott, A.; Sakakibara, R.; Shemesh, O.A.; Peterka, D.S.; Boyden, E.S.; Owen, J.S.; Yuste, R.; Englund, D. Modulation of nitrogen vacancy charge state and fluorescence in nanodiamonds using electrochemical potential. Proc. Natl. Acad. Sci. USA 2016, 113, 3938–3943. [Google Scholar] [CrossRef] [PubMed]
- Stacey, A.; Dontschuk, N.; Chou, J.P.; Broadway, D.A.; Schenk, A.K.; Sear, M.J.; Tetienne, J.P.; Hoffman, A.; Prawer, S.; Pakes, C.I.; et al. Evidence for primal sp2 defects at the diamond surface: Candidates for electron trapping and noise sources. Adv. Mater. Interfaces 2019, 6, 1801449. [Google Scholar] [CrossRef]
- Sangtawesin, S.; Dwyer, B.L.; Srinivasan, S.; Allred, J.J.; Rodgers, L.V.; De Greve, K.; Stacey, A.; Dontschuk, N.; O’Donnell, K.M.; Hu, D.; et al. Origins of diamond surface noise probed by correlating single-spin measurements with surface spectroscopy. Phys. Rev. X 2019, 9, 031052. [Google Scholar] [CrossRef]
- Fu, K.M.; Santori, C.; Barclay, P.; Beausoleil, R. Conversion of neutral nitrogen-vacancy centers to negatively charged nitrogen-vacancy centers through selective oxidation. Appl. Phys. Lett. 2010, 96, 121907. [Google Scholar] [CrossRef]
- Cui, S.; Hu, E.L. Increased negatively charged nitrogen-vacancy centers in fluorinated diamond. Appl. Phys. Lett. 2013, 103, 051603. [Google Scholar] [CrossRef]
- Kawai, S.; Yamano, H.; Sonoda, T.; Kato, K.; Buendia, J.J.; Kageura, T.; Fukuda, R.; Okada, T.; Tanii, T.; Higuchi, T.; et al. Nitrogen-terminated diamond surface for nanoscale NMR by shallow nitrogen-vacancy centers. J. Phys. Chem. C 2019, 123, 3594–3604. [Google Scholar] [CrossRef]
- Giri, R.; Jensen, R.H.; Khurana, D.; Bocquel, J.; Radko, I.P.; Lang, J.; Osterkamp, C.; Jelezko, F.; Berg-Sorensen, K.; Andersen, U.L.; et al. Charge stability and charge-state-based spin readout of shallow nitrogen-vacancy centers in diamond. arXiv 2022, arXiv:2208.14154. [Google Scholar]
- Haruyama, M.; Okigawa, Y.; Okada, M.; Nakajima, H.; Okazaki, T.; Kato, H.; Makino, T.; Yamada, T. Charge stabilization of shallow nitrogen-vacancy centers using graphene/diamond junctions. Appl. Phys. Lett. 2023, 122, 141601. [Google Scholar] [CrossRef]
- Rondin, L.; Dantelle, G.; Slablab, A.; Grosshans, F.; Treussart, F.; Bergonzo, P.; Perruchas, S.; Gacoin, T.; Chaigneau, M.; Chang, H.C.; et al. Surface-induced charge state conversion of nitrogen-vacancy defects in nanodiamonds. Phys. Rev. B 2010, 82, 115449. [Google Scholar] [CrossRef]
- Shanley, T.W.; Martin, A.A.; Aharonovich, I.; Toth, M. Localized chemical switching of the charge state of nitrogen-vacancy luminescence centers in diamond. Appl. Phys. Lett. 2014, 105, 063103. [Google Scholar] [CrossRef]
- Shenderova, O.A.; Shames, A.I.; Nunn, N.A.; Torelli, M.D.; Vlasov, I.; Zaitsev, A. Synthesis, properties, and applications of fluorescent diamond particles. J. Vac. Sci. Technol. B Nanotechnol. Microelectron. Mater. Process. Meas. Phenom. 2019, 37, 030802. [Google Scholar]
- Torelli, M.D.; Nunn, N.A.; Shenderova, O.A. A perspective on fluorescent nanodiamond bioimaging. Small 2019, 15, 1902151. [Google Scholar] [CrossRef]
- Basso, L.; Cazzanelli, M.; Orlandi, M.; Miotello, A. Nanodiamonds: Synthesis and application in sensing, catalysis, and the possible connection with some processes occurring in space. Appl. Sci. 2020, 10, 4094. [Google Scholar] [CrossRef]
- Nunn, N.; Torelli, M.; McGuire, G.; Shenderova, O. Nanodiamond: A high impact nanomaterial. Curr. Opin. Solid State Mater. Sci. 2017, 21, 1–9. [Google Scholar] [CrossRef]
- Terada, D.; Segawa, T.F.; Shames, A.I.; Onoda, S.; Ohshima, T.; Ōsawa, E.; Igarashi, R.; Shirakawa, M. Monodisperse five-nanometer-sized detonation nanodiamonds enriched in nitrogen-vacancy centers. ACS Nano 2019, 13, 6461–6468. [Google Scholar] [CrossRef]
- Tallaire, A.; Brinza, O.; De Feudis, M.; Ferrier, A.; Touati, N.; Binet, L.; Nicolas, L.; Delord, T.; Hétet, G.; Herzig, T.; et al. Synthesis of loose nanodiamonds containing nitrogen-vacancy centers for magnetic and thermal sensing. ACS Appl. Nano Mater. 2019, 2, 5952–5962. [Google Scholar] [CrossRef]
- Alkahtani, M.; Hemmer, P. Charge stability of nitrogen-vacancy color centers in organic nanodiamonds. Opt. Mater. Express 2020, 10, 1224–1231. [Google Scholar] [CrossRef]
- Narayan, J.; Bhaumik, A. Novel synthesis and properties of pure and NV-doped nanodiamonds and other nanostructures. Mater. Res. Lett. 2017, 5, 242–250. [Google Scholar] [CrossRef]
- Cazzanelli, M.; Basso, L.; Cestari, C.; Bazzanella, N.; Moser, E.; Orlandi, M.; Piccoli, A.; Miotello, A. Fluorescent Nanodiamonds Synthesized in One-Step by Pulsed Laser Ablation of Graphite in Liquid-Nitrogen. C 2021, 7, 49. [Google Scholar] [CrossRef]
- Su, L.J.; Fang, C.Y.; Chang, Y.T.; Chen, K.M.; Yu, Y.C.; Hsu, J.H.; Chang, H.C. Creation of high density ensembles of nitrogen-vacancy centers in nitrogen-rich type Ib nanodiamonds. Nanotechnology 2013, 24, 315702. [Google Scholar] [CrossRef]
- Laube, C.; Oeckinghaus, T.; Lehnert, J.; Griebel, J.; Knolle, W.; Denisenko, A.; Kahnt, A.; Meijer, J.; Wrachtrup, J.; Abel, B. Controlling the fluorescence properties of nitrogen vacancy centers in nanodiamonds. Nanoscale 2019, 11, 1770–1783. [Google Scholar] [CrossRef]
- Reineck, P.; Trindade, L.F.; Havlik, J.; Stursa, J.; Heffernan, A.; Elbourne, A.; Orth, A.; Capelli, M.; Cigler, P.; Simpson, D.A.; et al. Not all fluorescent nanodiamonds are created equal: A comparative study. Part. Part. Syst. Charact. 2019, 36, 1900009. [Google Scholar] [CrossRef]
- Sumikura, H.; Hirama, K.; Nishiguchi, K.; Shinya, A.; Notomi, M. Highly nitrogen-vacancy doped diamond nanostructures fabricated by ion implantation and optimum annealing. APL Mater. 2020, 8, 031113. [Google Scholar] [CrossRef]
- Petráková, V.; Taylor, A.; Kratochvílová, I.; Fendrych, F.; Vacík, J.; Kučka, J.; Štursa, J.; Cígler, P.; Ledvina, M.; Fišerová, A.; et al. Luminescence of nanodiamond driven by atomic functionalization: Towards novel detection principles. Adv. Funct. Mater. 2012, 22, 812–819. [Google Scholar] [CrossRef]
- Kratochvílová, I.; Šebera, J.; Ashcheulov, P.; Golan, M.; Ledvina, M.; Míčová, J.; Mravec, F.; Kovalenko, A.; Zverev, D.; Yavkin, B.; et al. Magnetical and optical properties of nanodiamonds can be tuned by particles surface chemistry: Theoretical and experimental study. J. Phys. Chem. C 2014, 118, 25245–25252. [Google Scholar] [CrossRef]
- Reineck, P.; Lau, D.W.; Wilson, E.R.; Fox, K.; Field, M.R.; Deeleepojananan, C.; Mochalin, V.N.; Gibson, B.C. Effect of surface chemistry on the fluorescence of detonation nanodiamonds. ACS Nano 2017, 11, 10924–10934. [Google Scholar] [CrossRef]
- Keremidarska, M.; Ganeva, A.; Mitev, D.; Hikov, T.; Presker, R.; Pramatarova, L.; Krasteva, N. Comparative study of cytotoxicity of detonation nanodiamond particles with an osteosarcoma cell line and primary mesenchymal stem cells. Biotechnol. Biotechnol. Equip. 2014, 28, 733–739. [Google Scholar] [CrossRef] [PubMed]
- Dhomkar, S.; Jayakumar, H.; Zangara, P.R.; Meriles, C.A. Charge dynamics in near-surface, variable-density ensembles of nitrogen-vacancy centers in diamond. Nano Lett. 2018, 18, 4046–4052. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.D.; Zou, C.L.; Sun, F.W.; Guo, G.C. Optical manipulation of the charge state of nitrogen-vacancy center in diamond. Appl. Phys. Lett. 2013, 103, 013112. [Google Scholar] [CrossRef]
- Giri, R.; Dorigoni, C.; Tambalo, S.; Gorrini, F.; Bifone, A. Selective measurement of charge dynamics in an ensemble of nitrogen-vacancy centers in nanodiamond and bulk diamond. Phys. Rev. B 2019, 99, 155426. [Google Scholar] [CrossRef]
- Siyushev, P.; Pinto, H.; Vörös, M.; Gali, A.; Jelezko, F.; Wrachtrup, J. Optically controlled switching of the charge state of a single nitrogen-vacancy center in diamond at cryogenic temperatures. Phys. Rev. Lett. 2013, 110, 167402. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.D.; Zhou, L.M.; Zou, C.L.; Li, C.C.; Dong, Y.; Sun, F.W.; Guo, G.C. Spin depolarization effect induced by charge state conversion of nitrogen vacancy center in diamond. Phys. Rev. B 2015, 92, 104301. [Google Scholar] [CrossRef]
- Neukirch, L.P.; Gieseler, J.; Quidant, R.; Novotny, L.; Vamivakas, A.N. Observation of nitrogen vacancy photoluminescence from an optically levitated nanodiamond. Opt. Lett. 2013, 38, 2976–2979. [Google Scholar] [CrossRef] [PubMed]
- Ji, P.; Dutt, M.G. Charge state dynamics of the nitrogen vacancy center in diamond under 1064-nm laser excitation. Phys. Rev. B 2016, 94, 024101. [Google Scholar] [CrossRef]
- Meirzada, I.; Hovav, Y.; Wolf, S.; Bar-Gill, N. Negative charge enhancement of near-surface nitrogen vacancy centers by multicolor excitation. Phys. Rev. B 2018, 98, 245411. [Google Scholar] [CrossRef]
- Wee, T.L.; Tzeng, Y.K.; Han, C.C.; Chang, H.C.; Fann, W.; Hsu, J.H.; Chen, K.M.; Yu, Y.C. Two-photon excited fluorescence of nitrogen-vacancy centers in proton-irradiated type Ib diamond. J. Phys. Chem. A 2007, 111, 9379–9386. [Google Scholar] [CrossRef]
- Ji, P.; Balili, R.; Beaumariage, J.; Mukherjee, S.; Snoke, D.; Dutt, M.G. Multiple-photon excitation of nitrogen vacancy centers in diamond. Phys. Rev. B 2018, 97, 134112. [Google Scholar] [CrossRef]
- Hopper, D.A.; Grote, R.R.; Exarhos, A.L.; Bassett, L.C. Near-infrared-assisted charge control and spin readout of the nitrogen-vacancy center in diamond. Phys. Rev. B 2016, 94, 241201. [Google Scholar] [CrossRef]
- Manson, N.B.; Hedges, M.; Barson, M.S.; Ahlefeldt, R.; Doherty, M.W.; Abe, H.; Ohshima, T.; Sellars, M.J. NV-–N+ pair centre in 1b diamond. New J. Phys. 2018, 20, 113037. [Google Scholar] [CrossRef]
- Giri, R.; Gorrini, F.; Dorigoni, C.; Avalos, C.; Cazzanelli, M.; Tambalo, S.; Bifone, A. Coupled charge and spin dynamics in high-density ensembles of nitrogen-vacancy centers in diamond. Phys. Rev. B 2018, 98, 045401. [Google Scholar] [CrossRef]
- Wildanger, D.; Patton, B.R.; Schill, H.; Marseglia, L.; Hadden, J.; Knauer, S.; Schönle, A.; Rarity, J.G.; O’Brien, J.L.; Hell, S.W.; et al. Solid Immersion Facilitates Fluorescence Microscopy with Nanometer Resolution and Sub-Ångström Emitter Localization. Adv. Mater. 2012, 24, OP309–OP313. [Google Scholar] [CrossRef]
- Hsiao, W.W.W.; Hui, Y.Y.; Tsai, P.C.; Chang, H.C. Fluorescent nanodiamond: A versatile tool for long-term cell tracking, super-resolution imaging, and nanoscale temperature sensing. Accounts Chem. Res. 2016, 49, 400–407. [Google Scholar] [CrossRef]
- Laporte, G.; Psaltis, D. STED imaging of green fluorescent nanodiamonds containing nitrogen-vacancy-nitrogen centers. Biomed. Opt. Express 2016, 7, 34–44. [Google Scholar] [CrossRef]
- Prabhakar, N.; Peurla, M.; Koho, S.; Deguchi, T.; Näreoja, T.; Chang, H.C.; Rosenholm, J.M.; Hänninen, P.E. STED-TEM correlative microscopy leveraging nanodiamonds as intracellular dual-contrast markers. Small 2018, 14, 1701807. [Google Scholar] [CrossRef]
- Hsieh, F.J.; Chen, Y.W.; Huang, Y.K.; Lee, H.M.; Lin, C.H.; Chang, H.C. Correlative light-electron microscopy of lipid-encapsulated fluorescent nanodiamonds for nanometric localization of cell surface antigens. Anal. Chem. 2018, 90, 1566–1571. [Google Scholar] [CrossRef]
- Mi, Z.; Chen, C.B.; Tan, H.Q.; Dou, Y.; Yang, C.; Turaga, S.P.; Ren, M.; Vajandar, S.K.; Yuen, G.H.; Osipowicz, T.; et al. Quantifying nanodiamonds biodistribution in whole cells with correlative iono-nanoscopy. Nat. Commun. 2021, 12, 4657. [Google Scholar] [CrossRef]
- Krečmarová, M.; Gulka, M.; Vandenryt, T.; Hrubý, J.; Fekete, L.; Hubík, P.; Taylor, A.; Mortet, V.; Thoelen, R.; Bourgeois, E.; et al. A label-free diamond microfluidic DNA sensor based on active nitrogen-vacancy center charge state control. ACS Appl. Mater. Interfaces 2021, 13, 18500–18510. [Google Scholar] [CrossRef] [PubMed]
- Petrakova, V.; Rehor, I.; Stursa, J.; Ledvina, M.; Nesladek, M.; Cigler, P. Charge-sensitive fluorescent nanosensors created from nanodiamonds. Nanoscale 2015, 7, 12307–12311. [Google Scholar] [CrossRef] [PubMed]
- Raabova, H.; Chvatil, D.; Cigler, P. Diamond nano-optode for fluorescent measurements of pH and temperature. Nanoscale 2019, 11, 18537–18542. [Google Scholar] [CrossRef] [PubMed]
- Bian, K.; Zheng, W.; Zeng, X.; Chen, X.; Stöhr, R.; Denisenko, A.; Yang, S.; Wrachtrup, J.; Jiang, Y. Nanoscale electric-field imaging based on a quantum sensor and its charge-state control under ambient condition. Nat. Commun. 2021, 12, 2457. [Google Scholar] [CrossRef]
- Dolde, F.; Fedder, H.; Doherty, M.W.; Nöbauer, T.; Rempp, F.; Balasubramanian, G.; Wolf, T.; Reinhard, F.; Hollenberg, L.C.; Jelezko, F.; et al. Electric-field sensing using single diamond spins. Nat. Phys. 2011, 7, 459–463. [Google Scholar] [CrossRef]
- Olivares-Postigo, D.; Gorrini, F.; Bitonto, V.; Ackermann, J.; Giri, R.; Krueger, A.; Bifone, A. Divergent Effects of Laser Irradiation on Ensembles of Nitrogen-Vacancy Centers in Bulk and Nanodiamonds: Implications for Biosensing. Nanoscale Res. Lett. 2022, 17, 95. [Google Scholar] [CrossRef]
- Li, C.; Soleyman, R.; Kohandel, M.; Cappellaro, P. SARS-CoV-2 quantum sensor based on nitrogen-vacancy centers in diamond. Nano Lett. 2021, 22, 43–49. [Google Scholar] [CrossRef]
- Barton, J.; Gulka, M.; Tarabek, J.; Mindarava, Y.; Wang, Z.; Schimer, J.; Raabova, H.; Bednar, J.; Plenio, M.B.; Jelezko, F.; et al. Nanoscale dynamic readout of a chemical redox process using radicals coupled with nitrogen-vacancy centers in nanodiamonds. ACS Nano 2020, 14, 12938–12950. [Google Scholar] [CrossRef]
- Kuwahata, A.; Kitaizumi, T.; Saichi, K.; Sato, T.; Igarashi, R.; Ohshima, T.; Masuyama, Y.; Iwasaki, T.; Hatano, M.; Jelezko, F.; et al. Magnetometer with nitrogen-vacancy center in a bulk diamond for detecting magnetic nanoparticles in biomedical applications. Sci. Rep. 2020, 10, 2483. [Google Scholar] [CrossRef]
- Jayakumar, H.; Lozovoi, A.; Daw, D.; Meriles, C.A. Long-term spin state storage using ancilla charge memories. Phys. Rev. Lett. 2020, 125, 236601. [Google Scholar] [CrossRef]
- Gardill, A.; Kemeny, I.; Cambria, M.C.; Li, Y.; Dinani, H.T.; Norambuena, A.; Maze, J.R.; Lordi, V.; Kolkowitz, S. Probing charge dynamics in diamond with an individual color center. Nano Lett. 2021, 21, 6960–6966. [Google Scholar] [CrossRef] [PubMed]
- Jayakumar, H.; Henshaw, J.; Dhomkar, S.; Pagliero, D.; Laraoui, A.; Manson, N.B.; Albu, R.; Doherty, M.W.; Meriles, C.A. Optical patterning of trapped charge in nitrogen-doped diamond. Nat. Commun. 2016, 7, 12660. [Google Scholar] [CrossRef]
- Lozovoi, A.; Daw, D.; Jayakumar, H.; Meriles, C. Dark defect charge dynamics in bulk chemical-vapor-deposition-grown diamonds probed via nitrogen vacancy centers. Phys. Rev. Mater. 2020, 4, 053602. [Google Scholar] [CrossRef]
- Taylor, J.M.; Cappellaro, P.; Childress, L.; Jiang, L.; Budker, D.; Hemmer, P.; Yacoby, A.; Walsworth, R.; Lukin, M. High-sensitivity diamond magnetometer with nanoscale resolution. Nat. Phys. 2008, 4, 810–816. [Google Scholar] [CrossRef]
- Zhu, Q.; Guo, H.; Chen, Y.; Wu, D.; Zhao, B.; Wang, L.; Zhang, Y.; Zhao, R.; Du, F.; Tang, J.; et al. Method to estimate the sensing properties of nitrogen-riched diamonds in sensors based on ensemble nitrogen vacancy color centers. Jpn. J. Appl. Phys. 2018, 57, 110309. [Google Scholar] [CrossRef]
- Edmonds, A.M.; Hart, C.A.; Turner, M.J.; Colard, P.O.; Schloss, J.M.; Olsson, K.S.; Trubko, R.; Markham, M.L.; Rathmill, A.; Horne-Smith, B.; et al. Characterisation of CVD diamond with high concentrations of nitrogen for magnetic-field sensing applications. Mater. Quantum Technol. 2021, 1, 025001. [Google Scholar] [CrossRef]
- Barry, J.F.; Turner, M.J.; Schloss, J.M.; Glenn, D.R.; Song, Y.; Lukin, M.D.; Park, H.; Walsworth, R.L. Optical magnetic detection of single-neuron action potentials using quantum defects in diamond. Proc. Natl. Acad. Sci. USA 2016, 113, 14133–14138. [Google Scholar] [CrossRef]
- Webb, J.L.; Troise, L.; Hansen, N.W.; Olsson, C.; Wojciechowski, A.M.; Achard, J.; Brinza, O.; Staacke, R.; Kieschnick, M.; Meijer, J.; et al. Detection of biological signals from a live mammalian muscle using an early stage diamond quantum sensor. Sci. Rep. 2021, 11, 2412. [Google Scholar] [CrossRef]
- Le Sage, D.; Arai, K.; Glenn, D.R.; DeVience, S.J.; Pham, L.M.; Rahn-Lee, L.; Lukin, M.D.; Yacoby, A.; Komeili, A.; Walsworth, R.L. Optical magnetic imaging of living cells. Nature 2013, 496, 486–489. [Google Scholar] [CrossRef]
- McCoey, J.M.; Matsuoka, M.; de Gille, R.W.; Hall, L.T.; Shaw, J.A.; Tetienne, J.P.; Kisailus, D.; Hollenberg, L.C.; Simpson, D.A. Quantum magnetic imaging of iron biomineralization in teeth of the chiton Acanthopleura hirtosa. Small Methods 2020, 4, 1900754. [Google Scholar] [CrossRef]
- McGuinness, L.P.; Yan, Y.; Stacey, A.; Simpson, D.A.; Hall, L.T.; Maclaurin, D.; Prawer, S.; Mulvaney, P.; Wrachtrup, J.; Caruso, F.; et al. Quantum measurement and orientation tracking of fluorescent nanodiamonds inside living cells. Nat. Nanotechnol. 2011, 6, 358–363. [Google Scholar] [CrossRef] [PubMed]
- Feng, X.; Leong, W.H.; Xia, K.; Liu, C.F.; Liu, G.Q.; Rendler, T.; Wrachtrup, J.; Liu, R.B.; Li, Q. Association of nanodiamond rotation dynamics with cell activities by translation-rotation tracking. Nano Lett. 2021, 21, 3393–3400. [Google Scholar] [CrossRef]
- King, J.P.; Coles, P.J.; Reimer, J.A. Optical polarization of C 13 nuclei in diamond through nitrogen vacancy centers. Phys. Rev. B 2010, 81, 073201. [Google Scholar] [CrossRef]
- Fischer, R.; Bretschneider, C.O.; London, P.; Budker, D.; Gershoni, D.; Frydman, L. Bulk nuclear polarization enhanced at room temperature by optical pumping. Phys. Rev. Lett. 2013, 111, 057601. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.J.; Shin, C.S.; Avalos, C.E.; Seltzer, S.J.; Budker, D.; Pines, A.; Bajaj, V.S. Sensitive magnetic control of ensemble nuclear spin hyperpolarization in diamond. Nat. Commun. 2013, 4, 1940. [Google Scholar] [CrossRef]
- Ajoy, A.; Liu, K.; Nazaryan, R.; Lv, X.; Zangara, P.R.; Safvati, B.; Wang, G.; Arnold, D.; Li, G.; Lin, A.; et al. Orientation-independent room temperature optical 13C hyperpolarization in powdered diamond. Sci. Adv. 2018, 4, eaar5492. [Google Scholar] [CrossRef]
- London, P.; Scheuer, J.; Cai, J.M.; Schwarz, I.; Retzker, A.; Plenio, M.B.; Katagiri, M.; Teraji, T.; Koizumi, S.; Isoya, J.; et al. Detecting and polarizing nuclear spins with double resonance on a single electron spin. Phys. Rev. Lett. 2013, 111, 067601. [Google Scholar] [CrossRef]
- Schwartz, I.; Scheuer, J.; Tratzmiller, B.; Müller, S.; Chen, Q.; Dhand, I.; Wang, Z.Y.; Müller, C.; Naydenov, B.; Jelezko, F.; et al. Robust optical polarization of nuclear spin baths using Hamiltonian engineering of nitrogen-vacancy center quantum dynamics. Sci. Adv. 2018, 4, eaat8978. [Google Scholar] [CrossRef]
- Healey, A.; Hall, L.; White, G.; Teraji, T.; Sani, M.A.; Separovic, F.; Tetienne, J.P.; Hollenberg, L. Polarization transfer to external nuclear spins using ensembles of nitrogen-vacancy centers. Phys. Rev. Appl. 2021, 15, 054052. [Google Scholar] [CrossRef]
- Abrams, D.; Trusheim, M.E.; Englund, D.R.; Shattuck, M.D.; Meriles, C.A. Dynamic nuclear spin polarization of liquids and gases in contact with nanostructured diamond. Nano Lett. 2014, 14, 2471–2478. [Google Scholar] [CrossRef]
- Tetienne, J.P.; Hall, L.; Healey, A.; White, G.; Sani, M.A.; Separovic, F.; Hollenberg, L. Prospects for nuclear spin hyperpolarization of molecular samples using nitrogen-vacancy centers in diamond. Phys. Rev. B 2021, 103, 014434. [Google Scholar] [CrossRef]
- Eills, J.; Budker, D.; Cavagnero, S.; Chekmenev, E.Y.; Elliott, S.J.; Jannin, S.; Lesage, A.; Matysik, J.; Meersmann, T.; Prisner, T.; et al. Spin Hyperpolarization in Modern Magnetic Resonance. Chem. Rev. 2023, 123, 1417–1551. [Google Scholar] [CrossRef] [PubMed]
- Parker, A.J.; Jeong, K.; Avalos, C.E.; Hausmann, B.J.; Vassiliou, C.C.; Pines, A.; King, J.P. Optically pumped dynamic nuclear hyperpolarization in C 13-enriched diamond. Phys. Rev. B 2019, 100, 041203. [Google Scholar] [CrossRef]
- Terblanche, C.J.; Reynhardt, E.C.; Van Wyk, J.A. 13C Spin–Lattice Relaxation in Natural Diamond: Zeeman Relaxation at 4.7 T and 300 K Due to Fixed Paramagnetic Nitrogen Defects. Solid State Nucl. Magn. Reson. 2001, 20, 1–22. [Google Scholar] [CrossRef] [PubMed]
- Ajoy, A.; Safvati, B.; Nazaryan, R.; Oon, J.; Han, B.; Raghavan, P.; Nirodi, R.; Aguilar, A.; Liu, K.; Cai, X.; et al. Hyperpolarized relaxometry based nuclear T 1 noise spectroscopy in diamond. Nat. Commun. 2019, 10, 5160. [Google Scholar] [CrossRef]

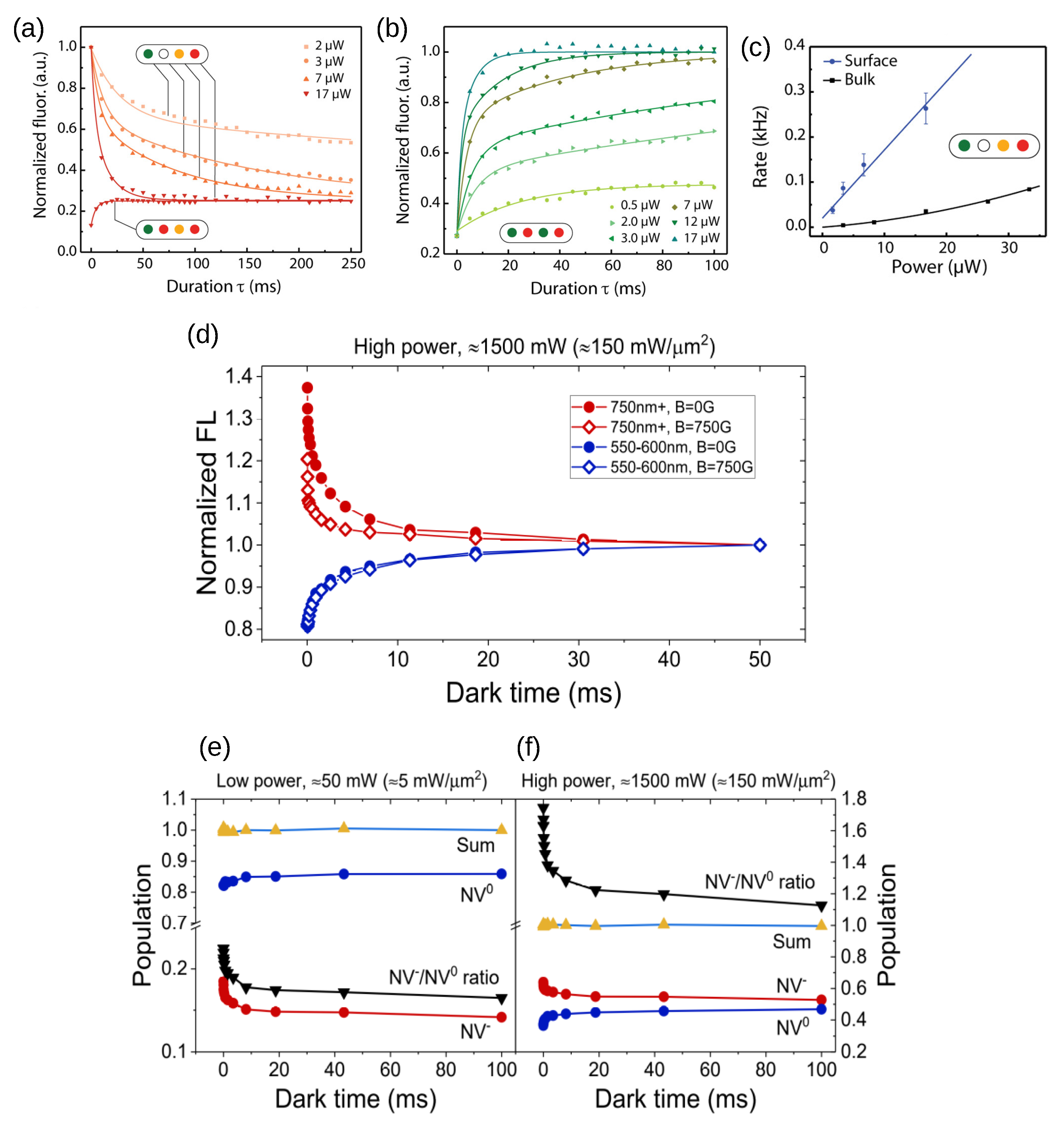
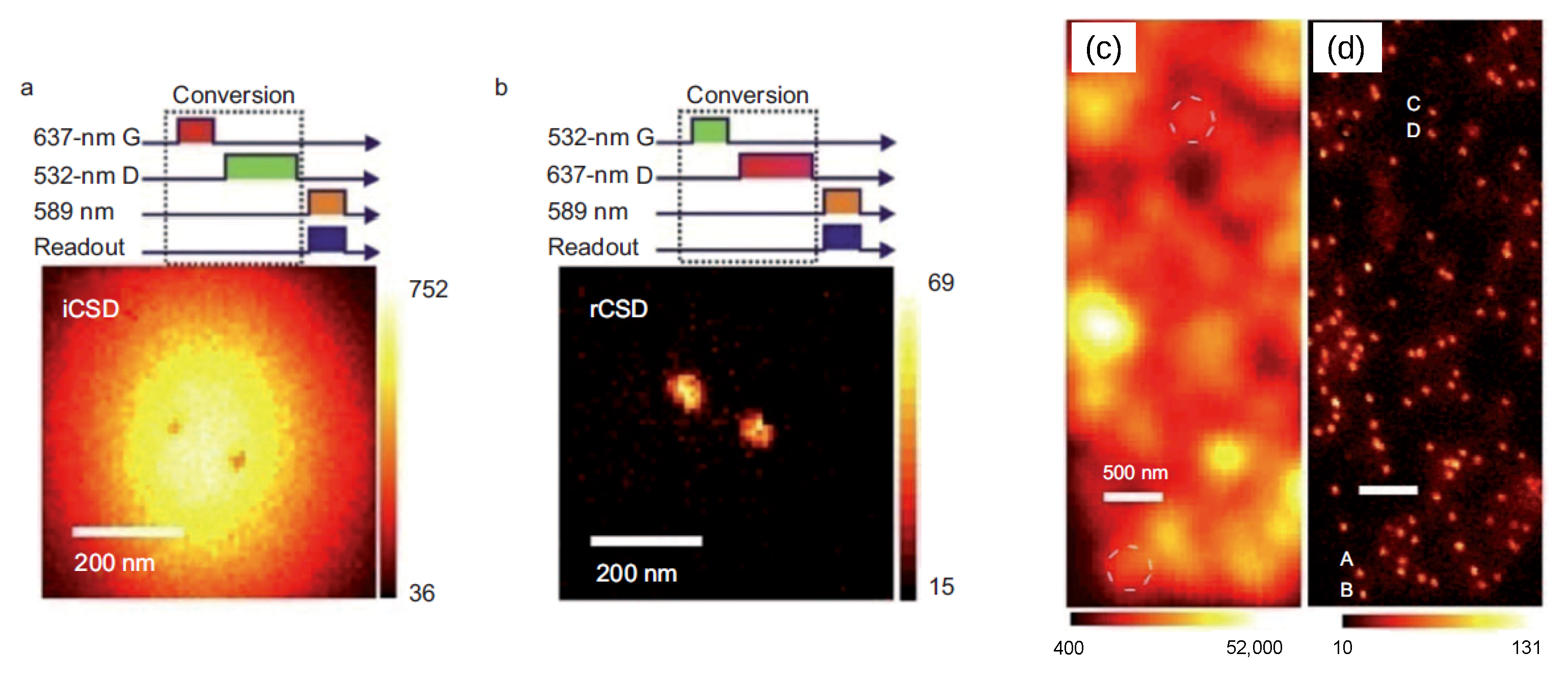
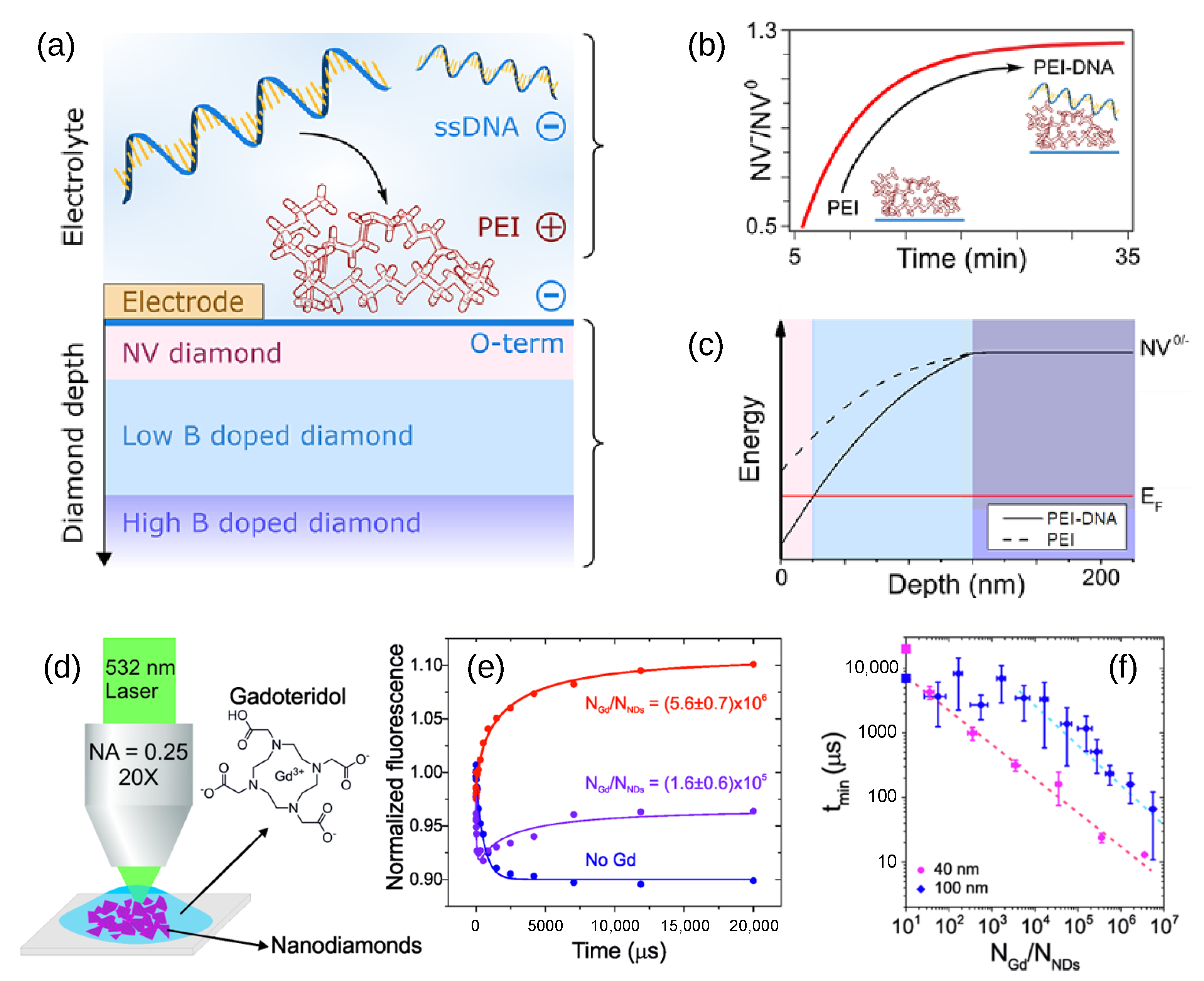
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gorrini, F.; Bifone, A. Advances in Stabilization and Enrichment of Shallow Nitrogen-Vacancy Centers in Diamond for Biosensing and Spin-Polarization Transfer. Biosensors 2023, 13, 691. https://doi.org/10.3390/bios13070691
Gorrini F, Bifone A. Advances in Stabilization and Enrichment of Shallow Nitrogen-Vacancy Centers in Diamond for Biosensing and Spin-Polarization Transfer. Biosensors. 2023; 13(7):691. https://doi.org/10.3390/bios13070691
Chicago/Turabian StyleGorrini, Federico, and Angelo Bifone. 2023. "Advances in Stabilization and Enrichment of Shallow Nitrogen-Vacancy Centers in Diamond for Biosensing and Spin-Polarization Transfer" Biosensors 13, no. 7: 691. https://doi.org/10.3390/bios13070691
APA StyleGorrini, F., & Bifone, A. (2023). Advances in Stabilization and Enrichment of Shallow Nitrogen-Vacancy Centers in Diamond for Biosensing and Spin-Polarization Transfer. Biosensors, 13(7), 691. https://doi.org/10.3390/bios13070691




