Molecular Dynamics Simulation of Dislocation Plasticity Mechanism of Nanoscale Ductile Materials in the Cold Gas Dynamic Spray Process
Abstract
1. Introduction
2. Computational Approach
3. Results and Discussion
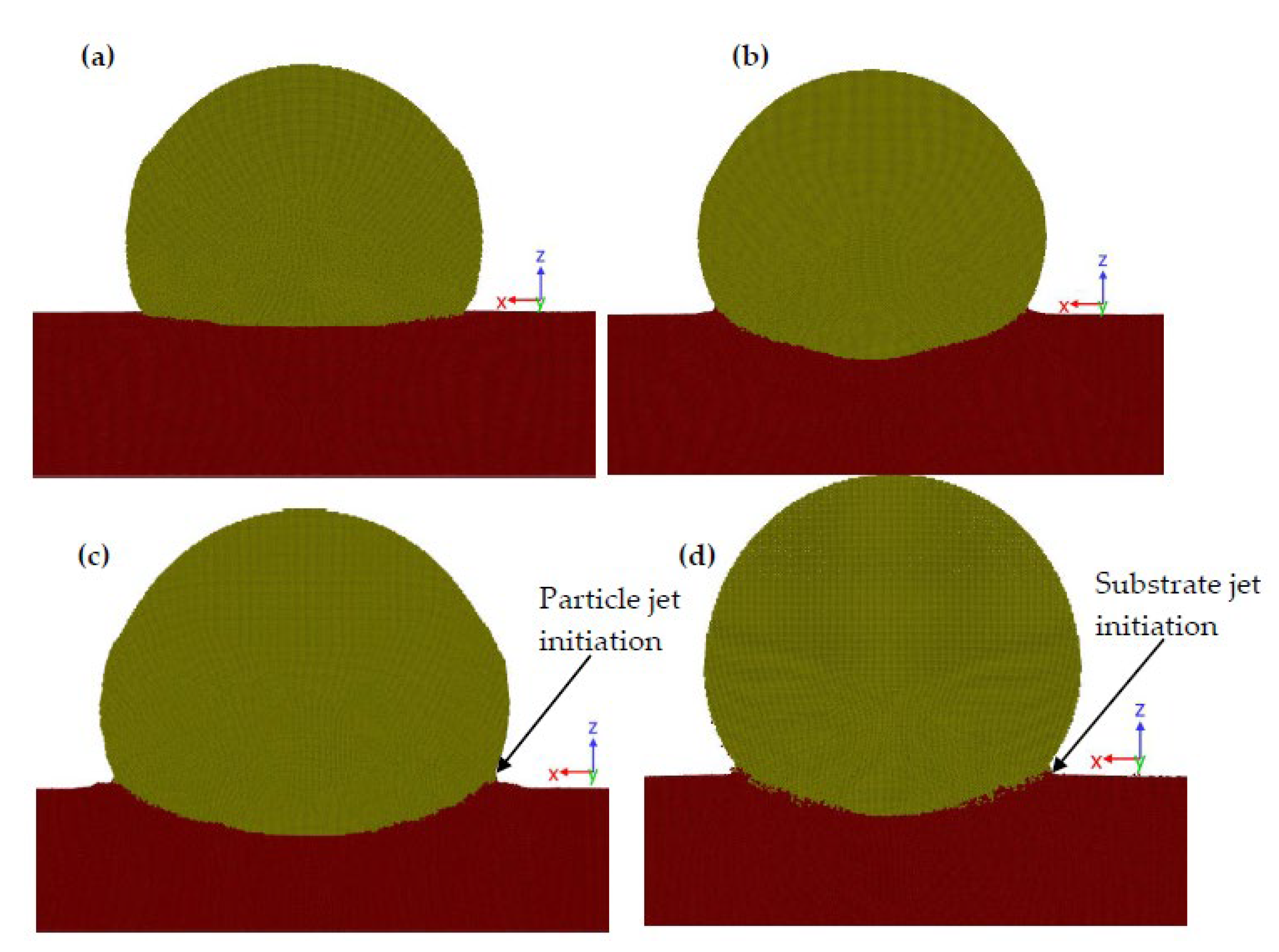
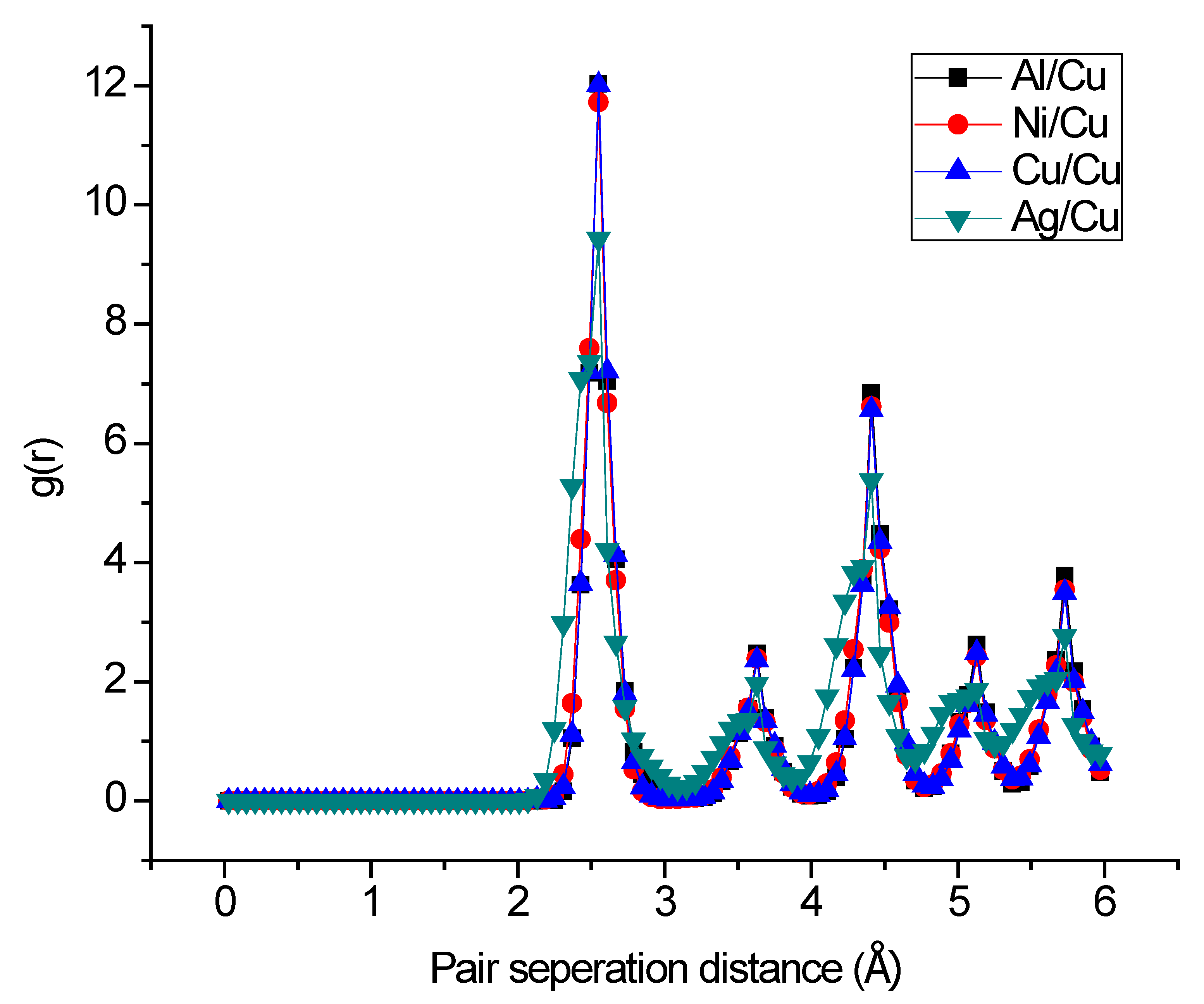
3.1. Atomic Structure Evolution and Material Jet Initiation
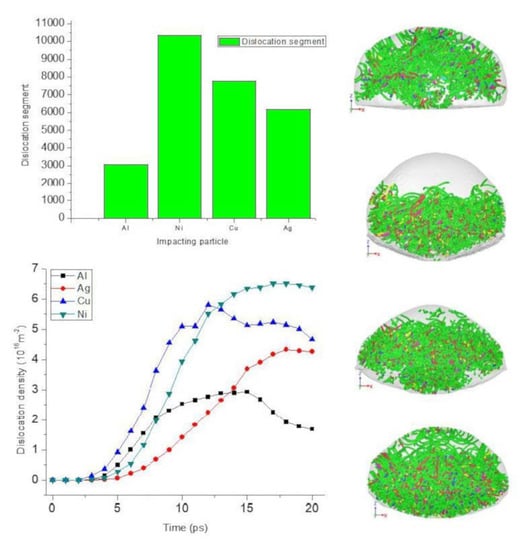
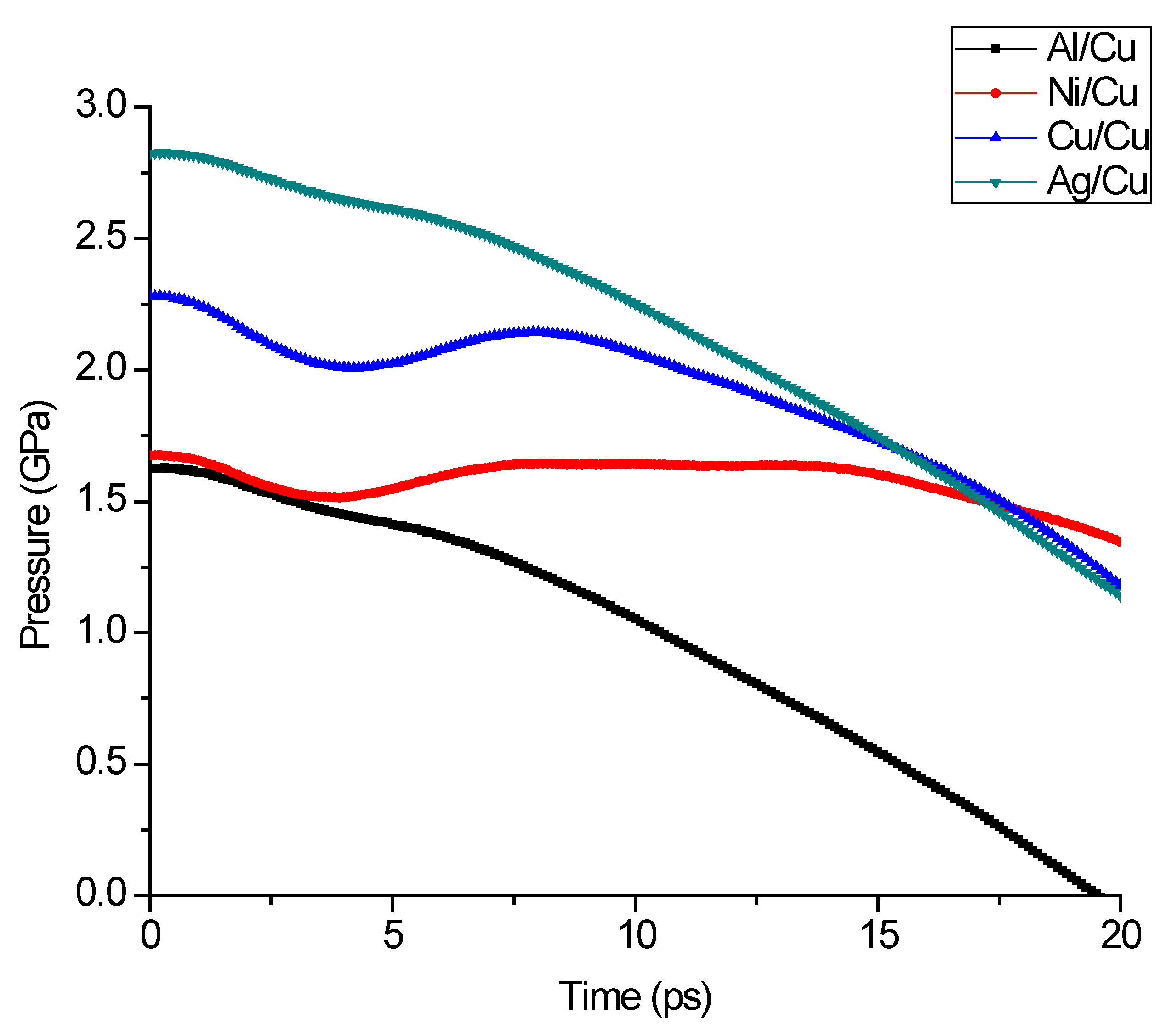
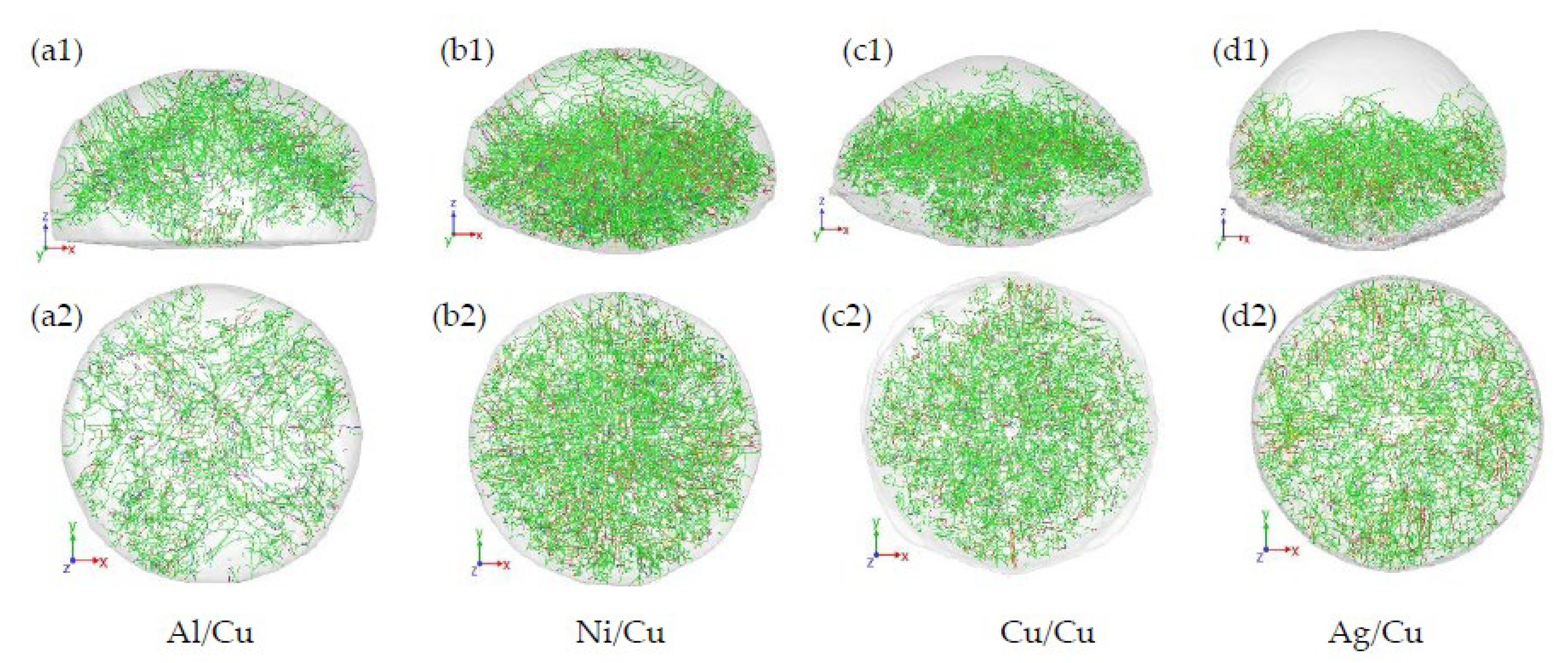
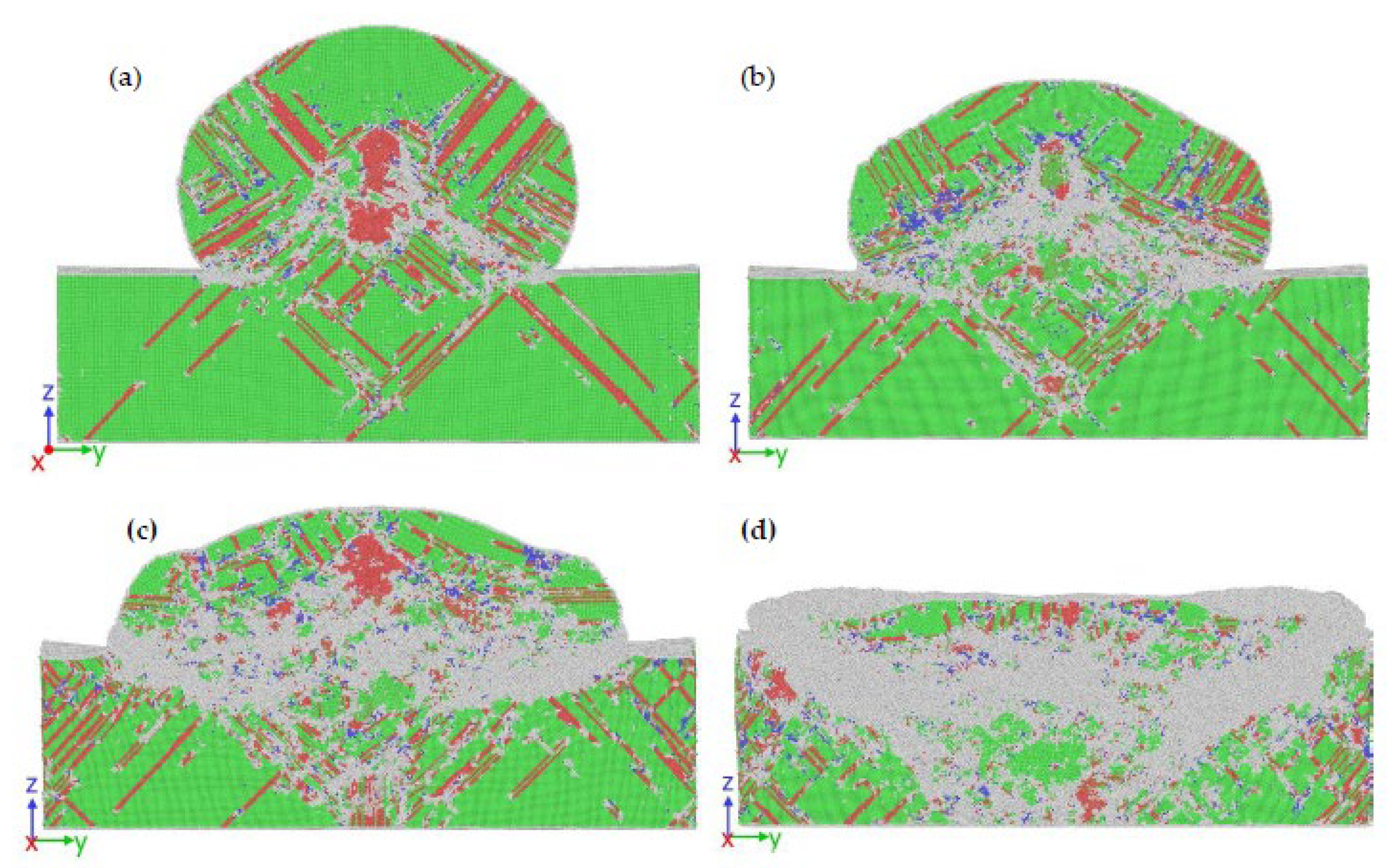
3.2. Material Dislocation Plasticity
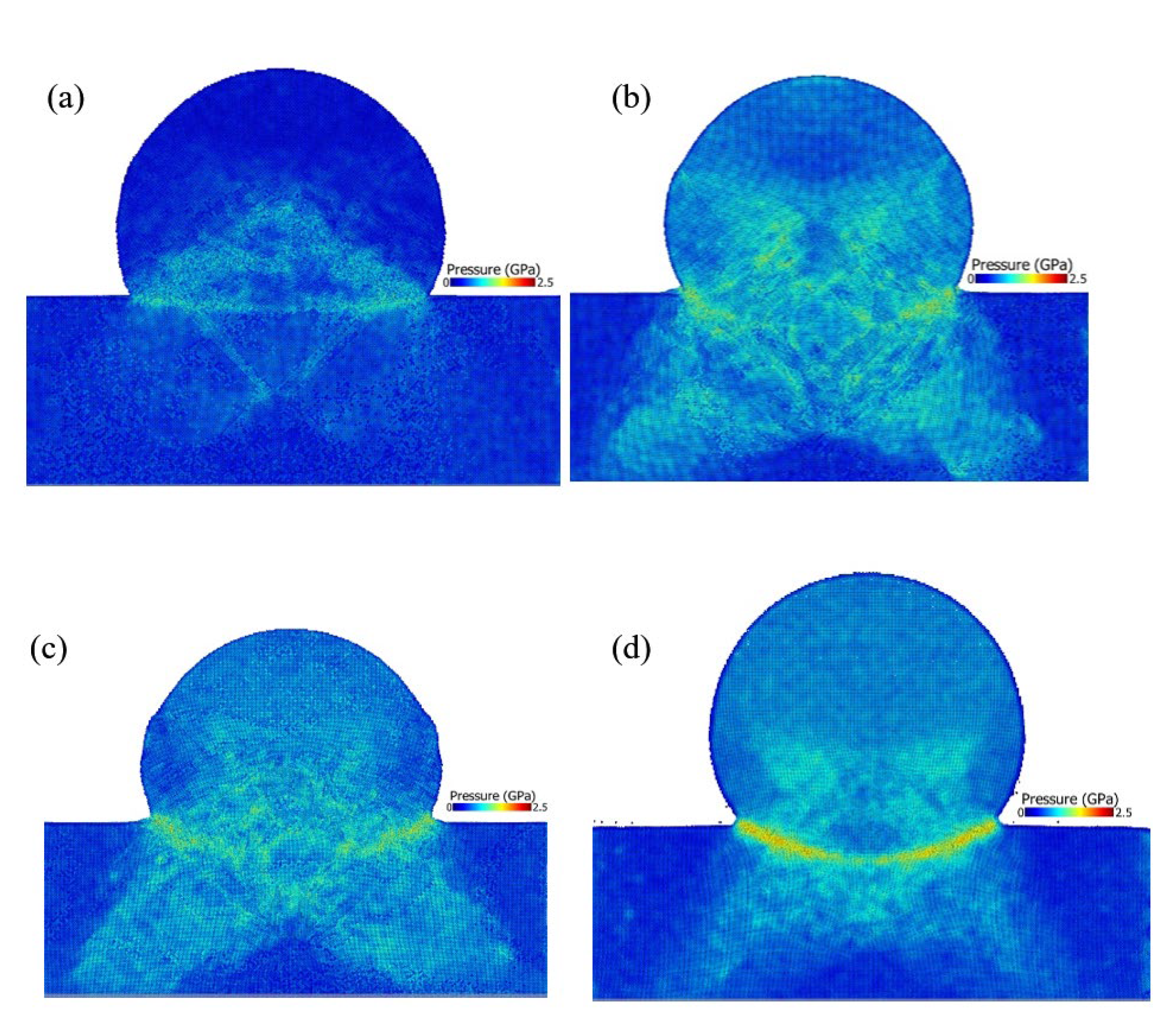
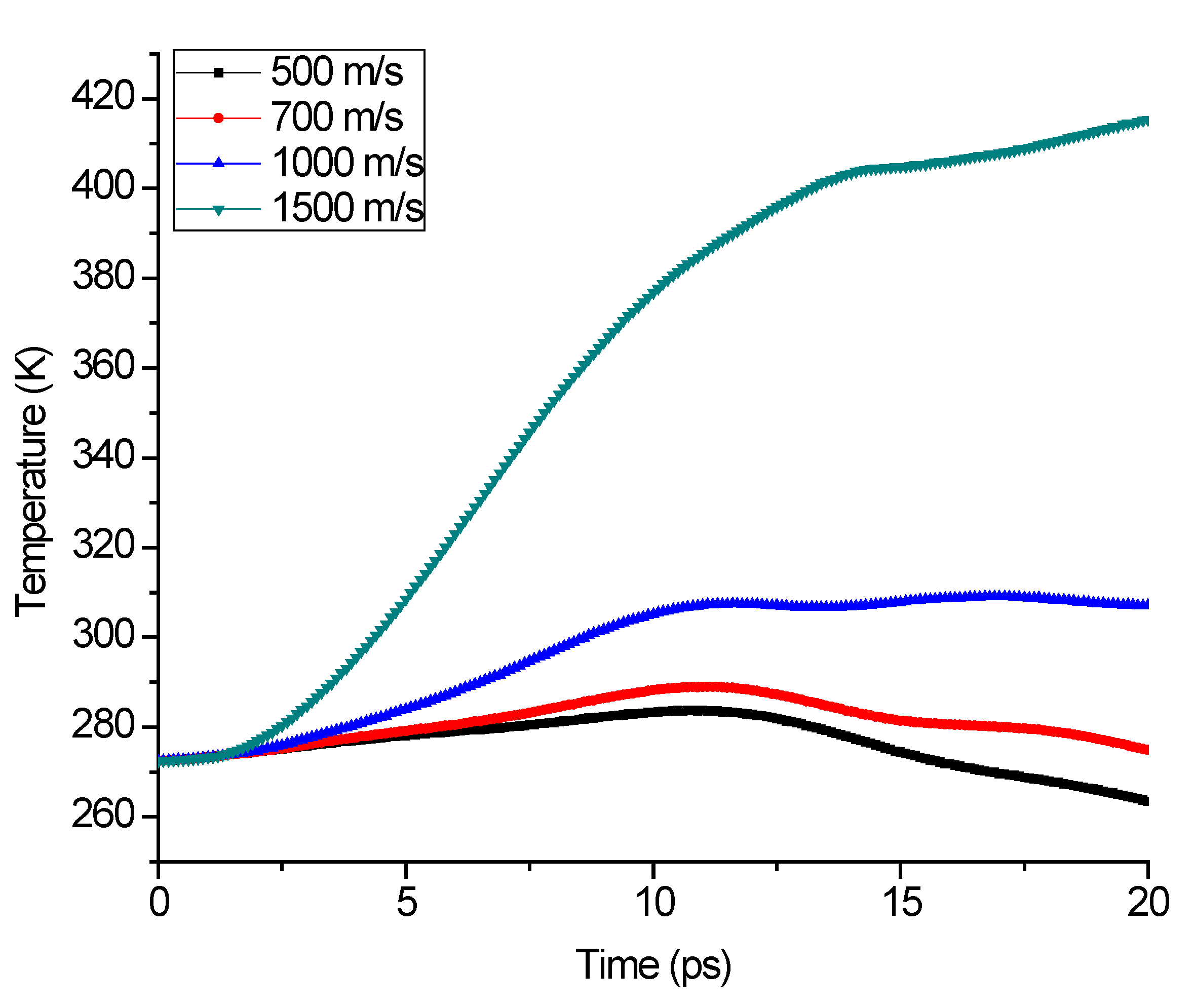
3.3. Particle Impact Velocity Effect on Microstructure Evolution
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ganesan, A.; Affi, J.; Yamada, M.; Fukumoto, M. Bonding behavior studies of cold sprayed copper coating on the PVC polymer substrate. Surf. Coat. Technol. 2012, 207, 262–269. [Google Scholar] [CrossRef]
- Khodabakhshi, F.; Marzbanrad, B.; Jahed, H.; Gerlich, A. Interfacial bonding mechanisms between aluminum and titanium during cold gas spraying followed by friction-stir modification. Appl. Surf. Sci. 2018, 462, 739–752. [Google Scholar] [CrossRef]
- Lu, J.; Zhang, H.; Chen, Y.; Zhao, X.; Guo, F.; Xiao, P. Effect of microstructure of a NiCoCrAlY coating fabricated by high-velocity air fuel on the isothermal oxidation. Corros. Sci. 2019, 159. [Google Scholar] [CrossRef]
- Raoelison, R.; Xie, Y.; Sapanathan, T.; Planche, M.; Kromer, R.; Costil, S.; Langlade, C. Cold gas dynamic spray technology: A comprehensive review of processing conditions for various technological developments till to date. Addit. Manuf. 2018, 19, 134–159. [Google Scholar] [CrossRef]
- Bagherifard, S.; Monti, S.; Zuccoli, M.V.; Riccio, M.; Kondás, J.; Guagliano, M. Cold spray deposition for additive manufacturing of freeform structural components compared to selective laser melting. Mater. Sci. Eng. A 2018, 721, 339–350. [Google Scholar] [CrossRef]
- Oyinbo, S.T.; Jen, T.-C. Investigation of the process parameters and restitution coefficient of ductile materials during cold gas dynamic spray (CGDS) using finite element analysis. Addit. Manuf. 2020, 31, 100986. [Google Scholar] [CrossRef]
- Oyinbo, S.; Jen, T.-C. A comparative review on cold gas dynamic spraying processes and technologies. Manuf. Rev. 2019, 6, 25. [Google Scholar] [CrossRef]
- Irissou, E.; Legoux, J.-G.; Ryabinin, A.N.; Jodoin, B.; Moreau, C. Review on Cold Spray Process and Technology: Part I—Intellectual Property. J. Therm. Spray Technol. 2008, 17, 495–516. [Google Scholar] [CrossRef]
- Van Steenkiste, T.; Smith, J.; Teets, R. Aluminum coatings via kinetic spray with relatively large powder particles. Surf. Coat. Technol. 2002, 154, 237–252. [Google Scholar] [CrossRef]
- Assadi, F.H.; Gartner, T.; Stoltenhoff, H.K. Bonding mechanism in cold gas spraying. Acta Mater. 2003, 51, 4379–4394. [Google Scholar] [CrossRef]
- Grujicic, M.; Zhao, C.; Tong, C.; Derosset, W.; Helfritch, D. Analysis of the impact velocity of powder particles in the cold-gas dynamic-spray process. Mater. Sci. Eng. A 2004, 368, 222–230. [Google Scholar] [CrossRef]
- Nasim, M.; Vo, T.; Mustafi, L.; Kim, B.; Lee, C.S.; Chu, W.-S.; Chun, D.-M. Deposition mechanism of graphene flakes directly from graphite particles in the kinetic spray process studied using molecular dynamics simulation. Comput. Mater. Sci. 2019, 169. [Google Scholar] [CrossRef]
- Rafaja, D.; Schucknecht, T.; Klemm, V.; Paul, A.; Berek, H. Microstructural characterisation of titanium coatings deposited using cold gas spraying on Al2O3 substrates. Surf. Coat. Technol. 2009, 203, 3206–3213. [Google Scholar] [CrossRef]
- Grujicic, M.; Saylor, J.; Beasley, D.; Derosset, W.; Helfritch, D. Computational analysis of the interfacial bonding between feed-powder particles and the substrate in the cold-gas dynamic-spray process. Appl. Surf. Sci. 2003, 219, 211–227. [Google Scholar] [CrossRef]
- Zhang, D.; Shipway, P.; McCartney, D. Cold Gas Dynamic Spraying of Aluminum: The Role of Substrate Characteristics in Deposit Formation. J. Therm. Spray Technol. 2005, 14, 109–116. [Google Scholar] [CrossRef]
- Kim, D.-Y.; Park, J.-J.; Lee, J.-G.; Kim, D.; Tark, S.J.; Ahn, S.; Yun, J.H.; Gwak, J.; Yoon, K.H.; Chandra, S.; et al. Cold Spray Deposition of Copper Electrodes on Silicon and Glass Substrates. J. Therm. Spray Technol. 2013, 22, 1092–1102. [Google Scholar] [CrossRef]
- King, P.C.; Zahiri, S.; Jahedi, M.; Friend, J. Aluminium coating of lead zirconate titanate—A study of cold spray variables. Surf. Coat. Technol. 2010, 205, 2016–2022. [Google Scholar] [CrossRef]
- Hussain, T.; McCartney, D.; Shipway, P. Bonding between aluminium and copper in cold spraying: Story of asymmetry. Mater. Sci. Technol. 2012, 28, 1371–1378. [Google Scholar] [CrossRef]
- Oyinbo, S.; Jen, T.-C. Feasibility of numerical simulation methods on the Cold Gas Dynamic Spray (CGDS) Deposition process for ductile materials. Manuf. Rev. 2020, 7, 24. [Google Scholar] [CrossRef]
- Seo, D.; Sayar, M.; Ogawa, K. SiO2 and MoSi2 formation on Inconel 625 surface via SiC coating deposited by cold spray. Surf. Coat. Technol. 2012, 206, 2851–2858. [Google Scholar] [CrossRef]
- Burlacov, I.; Jirkovský, J.; Kavan, L.; Ballhorn, R.; Heimann, R.B. Cold gas dynamic spraying (CGDS) of TiO2 (anatase) powders onto poly(sulfone) substrates: Microstructural characterisation and photocatalytic efficiency. J. Photochem. Photobiol. A Chem. 2007, 187, 285–292. [Google Scholar] [CrossRef]
- Amirthan, G.; Yamada, M.; Fukumoto, M. Cold Spray Coating Deposition Mechanism on the Thermoplastic and Thermosetting Polymer Substrates. J. Therm. Spray Technol. 2013, 22, 1275–1282. [Google Scholar] [CrossRef]
- Lupoi, R.; O’Neill, W. Deposition of metallic coatings on polymer surfaces using cold spray. Surf. Coat. Technol. 2010, 205, 2167–2173. [Google Scholar] [CrossRef]
- Zhou, X.; Chen, A.; Liu, J.; Wu, X.; Li, Y. Preparation of metallic coatings on polymer matrix composites by cold spray. Surf. Coat. Technol. 2011, 206, 132–136. [Google Scholar] [CrossRef]
- King, P.C.; Poole, A.J.; Horne, S.; De Nys, R.; Gulizia, S.; Jahedi, M.Z. Embedment of copper particles into polymers by cold spray. Surf. Coat. Technol. 2013, 216, 60–67. [Google Scholar] [CrossRef]
- Alhulaifi, A.S.; Buck, G.A.; Arbegast, W.J. Numerical and Experimental Investigation of Cold Spray Gas Dynamic Effects for Polymer Coating. J. Therm. Spray Technol. 2012, 21, 852–862. [Google Scholar] [CrossRef]
- Robitaille, F.; Yandouzi, M.; Hind, S.; Jodoin, B. Metallic coating of aerospace carbon/epoxy composites by the pulsed gas dynamic spraying process. Surf. Coat. Technol. 2009, 203, 2954–2960. [Google Scholar] [CrossRef]
- Champagne, V.K.; Helfritch, D.; Leyman, P.; Grendahl, S.; Klotz, B. Interface Material Mixing Formed by the Deposition of Copper on Aluminum by Means of the Cold Spray Process. J. Therm. Spray Technol. 2005, 14, 330–334. [Google Scholar] [CrossRef]
- Ko, K.; Choi, J.; Lee, H. Intermixing and interfacial morphology of cold-sprayed Al coatings on steel. Mater. Lett. 2014, 136, 45–47. [Google Scholar] [CrossRef]
- Yin, S.; Cavaliere, P.; Aldwell, B.; Jenkins, R.; Liao, H.; Li, W.; Lupoi, R. Cold spray additive manufacturing and repair: Fundamentals and applications. Addit. Manuf. 2018, 21, 628–650. [Google Scholar] [CrossRef]
- Moridi, A.; Gangaraj, S.; Guagliano, M.; Dao, M. Cold spray coating: Review of material systems and future perspectives. Surf. Eng. 2014, 30, 369–395. [Google Scholar] [CrossRef]
- Xie, Y.; Yin, S.; Chen, C.; Planche, M.-P.; Liao, H.; Lupoi, R. New insights into the coating/substrate interfacial bonding mechanism in cold spray. Scr. Mater. 2016, 125, 1–4. [Google Scholar] [CrossRef]
- Grujicic, M.; Zhao, C.; Derosset, W.; Helfritch, D. Adiabatic shear instability based mechanism for particles/substrate bonding in the cold-gas dynamic-spray process. Mater. Des. 2004, 25, 681–688. [Google Scholar] [CrossRef]
- Hassani-Gangaraj, M.; Veysset, D.; Champagne, V.K.; Nelson, K.A.; Schuh, C.A. Adiabatic shear instability is not necessary for adhesion in cold spray. Acta Mater. 2018, 158, 430–439. [Google Scholar] [CrossRef]
- Murr, L.E.; Esquivel, E.V. Observations of common microstructural issues associated with dynamic deformation phenomena: Twins, microbands, grain size effects, shear bands, and dynamic recrystallization. J. Mater. Sci. 2004, 39, 1153–1168. [Google Scholar] [CrossRef]
- Rahmati, S.; Zúñiga, A.; Jodoin, B.; Veiga, R. Deformation of copper particles upon impact: A molecular dynamics study of cold spray. Comput. Mater. Sci. 2020, 171, 109219. [Google Scholar] [CrossRef]
- Oyinbo, S.; Jen, T.-C.; Aasa, S.A.; Abegunde, O.; Zhu, Y. Development of palladium nanoparticles deposition on a copper substrate using a molecular dynamic (MD) simulation: A cold gas dynamic spray process. Manuf. Rev. 2020, 7, 29. [Google Scholar] [CrossRef]
- Bae, G.; Kumar, S.; Yoon, S.; Kang, K.; Na, H.; Kim, H.-J.; Lee, C. Bonding features and associated mechanisms in kinetic sprayed titanium coatings. Acta Mater. 2009, 57, 5654–5666. [Google Scholar] [CrossRef]
- Oyinbo, S.T.; Jen, T.-C.; Zhu, Y.; Ajiboye, J.S.; Ismail, S.O. Atomistic simulations of interfacial deformation and bonding mechanism of Pd-Cu composite metal membrane using cold gas dynamic spray process. Vacuum 2020, 182, 109779. [Google Scholar] [CrossRef]
- Wang, Q.; Birbilis, N.; Zhang, M.-X. Interfacial structure between particles in an aluminum deposit produced by cold spray. Mater. Lett. 2011, 65, 1576–1578. [Google Scholar] [CrossRef]
- Guetta, S.; Berger, M.-H.; Borit, F.; Guipont, V.; Jeandin, M.; Boustie, M.; Ichikawa, Y.; Sakaguchi, K.; Ogawa, K. Influence of Particle Velocity on Adhesion of Cold-Sprayed Splats. J. Therm. Spray Technol. 2009, 18, 331–342. [Google Scholar] [CrossRef]
- Henao, J.; Concustell, A.; Dosta, S.; Bolelli, G.; Cano, I.; Lusvarghi, L.; Guilemany, J. Deposition mechanisms of metallic glass particles by Cold Gas Spraying. Acta Mater. 2017, 125, 327–339. [Google Scholar] [CrossRef]
- Oyinbo, S.T.; Jen, T.-C. Molecular dynamics investigation of temperature effect and surface configurations on multiple impacts plastic deformation in a palladium-copper composite metal membrane (CMM): A cold gas dynamic spray (CGDS) process. Comput. Mater. Sci. 2020, 185, 109968. [Google Scholar] [CrossRef]
- Fukumoto, M.; Mashiko, M.; Yamada, M.; Yamaguchi, E. Deposition Behavior of Copper Fine Particles onto Flat Substrate Surface in Cold Spraying. J. Therm. Spray Technol. 2009, 19, 89–94. [Google Scholar] [CrossRef]
- Zhao, P.; Zhang, Q.; Guo, Y.; Liu, H.; Deng, Z. Atomic simulation of crystal orientation effect on coating surface generation mechanisms in cold spray. Comput. Mater. Sci. 2020, 184, 109859. [Google Scholar] [CrossRef]
- Salehinia, I.; Lawrence, S.K.; Bahr, D. The effect of crystal orientation on the stochastic behavior of dislocation nucleation and multiplication during nanoindentation. Acta Mater. 2013, 61, 1421–1431. [Google Scholar] [CrossRef]
- Basu, S.; Wang, Z.; Saldana, C. Anomalous evolution of microstructure and crystallographic texture during indentation. Acta Mater. 2016, 105, 25–34. [Google Scholar] [CrossRef]
- Oyinbo, S.; Jen, T.-C. A Molecular Dynamics Investigation of the Temperature Effect on the Mechanical Properties of Selected Thin Films for Hydrogen Separation. Membranes 2020, 10, 241. [Google Scholar] [CrossRef]
- Hassani-Gangaraj, M.; Veysset, D.; Nelson, K.A.; Schuh, C.A. In-situ observations of single micro-particle impact bonding. Scr. Mater. 2018, 145, 9–13. [Google Scholar] [CrossRef]
- King, P.C.; Zahiri, S.H.; Jahedi, M. Microstructural Refinement within a Cold-Sprayed Copper Particle. Met. Mater. Trans. A 2009, 40, 2115–2123. [Google Scholar] [CrossRef]
- Irissou, E.; Legoux, J.-G.; Arsenault, B.; Moreau, C. Investigation of Al-Al2O3 Cold Spray Coating Formation and Properties. J. Therm. Spray Technol. 2007, 16, 661–668. [Google Scholar] [CrossRef]
- King, P.C.; Busch, C.; Kittel-Sherri, T.; Jahedi, M.; Gulizia, S. Interface melding in cold spray titanium particle impact. Surf. Coat. Technol. 2014, 239, 191–199. [Google Scholar] [CrossRef]
- Schöner, C.; Pöschel, T. Orientation-dependent properties of nanoparticle impact. Phys. Rev. E 2018, 98, 022902. [Google Scholar] [CrossRef] [PubMed]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2009, 18. [Google Scholar] [CrossRef]
- Braga, C.; Travis, K.P. A configurational temperature Nosé-Hoover thermostat. J. Chem. Phys. 2005, 123, 134101. [Google Scholar] [CrossRef]
- Hassani-Gangaraj, M.; Veysset, D.; Champagne, V.K.; Nelson, K.A.; Schuh, C.A. Response to Comment on Adiabatic shear instability is not necessary for adhesion in cold spray. Scr. Mater. 2019, 162, 515–519. [Google Scholar] [CrossRef]
- Li, W.-Y.; Gao, W. Some aspects on 3D numerical modeling of high velocity impact of particles in cold spraying by explicit finite element analysis. Appl. Surf. Sci. 2009, 255, 7878–7892. [Google Scholar] [CrossRef]
- Ghelichi, R.; Bagherifard, S.; Guagliano, M.; Verani, M. Numerical simulation of cold spray coating. Surf. Coat. Technol. 2011, 205, 5294–5301. [Google Scholar] [CrossRef]
- Zhou, X.W.; Johnson, R.A.; Wadley, H.N.G. Misfit-energy-increasing dislocations in vapor-deposited CoFe/NiFe multilayers. Phys. Rev. B 2004, 69, 1–10. [Google Scholar] [CrossRef]
- Guo, Y.-B.; Xu, T.; Li, M. Generalized type III internal stress from interfaces, triple junctions and other microstructural components in nanocrystalline materials. Acta Mater. 2013, 61, 4974–4983. [Google Scholar] [CrossRef]
- Guo, Y.-B.; Xu, T.; Li, M. Hierarchical dislocation nucleation controlled by internal stress in nanocrystalline copper. Appl. Phys. Lett. 2013, 102, 241910. [Google Scholar] [CrossRef]
- Faken, D.; Jónsson, H. Systematic analysis of local atomic structure combined with 3D computer graphics. Comput. Mater. Sci. 1994, 2, 279–286. [Google Scholar] [CrossRef]
- Honeycutt, J.D.; Andersen, H.C. Molecular dynamics study of melting and freezing of small Lennard-Jones clusters. J. Phys. Chem. 1987, 91, 4950–4963. [Google Scholar] [CrossRef]
- Stukowski, A.; Bulatov, V.V.; Arsenlis, A. Automated identification and indexing of dislocations in crystal interfaces. Model. Simul. Mater. Sci. Eng. 2012, 20. [Google Scholar] [CrossRef]
- Dear, J.P.; Field, J.E. High-speed photography of surface geometry effects in liquid/solid impact. J. Appl. Phys. 1988, 63, 1015–1021. [Google Scholar] [CrossRef]
- Field, J.E.; Dear, J.P.; Ogren, J.E. The effects of target compliance on liquid drop impact. J. Appl. Phys. 1989, 65, 533–540. [Google Scholar] [CrossRef]
- Li, W.Y.; Yin, S.; Wang, X.-F. Numerical investigations of the effect of oblique impact on particle deformation in cold spraying by the SPH method. Appl. Surf. Sci. 2010, 256, 3725–3734. [Google Scholar] [CrossRef]
- Rokni, M.R.; Zarei-Hanzaki, A.; Abedi, H. Microstructure evolution and mechanical properties of back extruded 7075 aluminum alloy at elevated temperatures. Mater. Sci. Eng. A 2012, 532, 593–600. [Google Scholar] [CrossRef]
- Arabgol, Z.; Vidaller, M.V.; Assadi, H.; Gärtner, F.; Klassen, T. Influence of thermal properties and temperature of substrate on the quality of cold-sprayed deposits. Acta Mater. 2017, 127, 287–301. [Google Scholar] [CrossRef]
- Hull, D.; Bacon, D.J. Introduction to Dislocation, 4th ed.; Woburn: Butterworth, MA, USA, 2001. [Google Scholar]
- Rokni, M.R.; Widener, C.A.; Champagne, V. Microstructural stability of ultrafine grained cold sprayed 6061 aluminum alloy. Appl. Surf. Sci. 2014, 290, 482–489. [Google Scholar] [CrossRef]
- Cheng, K.; Zhang, L.; Lu, C.; Tieu, K. Coupled grain boundary motion in aluminium: The effect of structural multiplicity. Sci. Rep. 2016, 6, 25427. [Google Scholar] [CrossRef] [PubMed]
- Zou, Y.; Goldbaum, D.; Szpunar, J.A.; Yue, S. Microstructure and nanohardness of cold-sprayed coatings: Electron backscattered diffraction and nanoindentation studies. Scr. Mater. 2010, 62, 395–398. [Google Scholar] [CrossRef]
- Rokni, M.R.; Widener, C.; Crawford, G.; West, M. An investigation into microstructure and mechanical properties of cold sprayed 7075 Al deposition. Mater. Sci. Eng. A 2015, 625, 19–27. [Google Scholar] [CrossRef]
- Rokni, M.; Widener, C.; Crawford, G. Microstructural evolution of 7075 Al gas atomized powder and high-pressure cold sprayed deposition. Surf. Coat. Technol. 2014, 251, 254–263. [Google Scholar] [CrossRef]
- Li, X.; Wei, Y.; Yang, W.; Gao, H. Competing grain-boundary- and dislocation-mediated mechanisms in plastic strain recovery in nanocrystalline aluminum. Proc. Natl. Acad. Sci. USA 2009, 106, 16108–16113. [Google Scholar] [CrossRef]
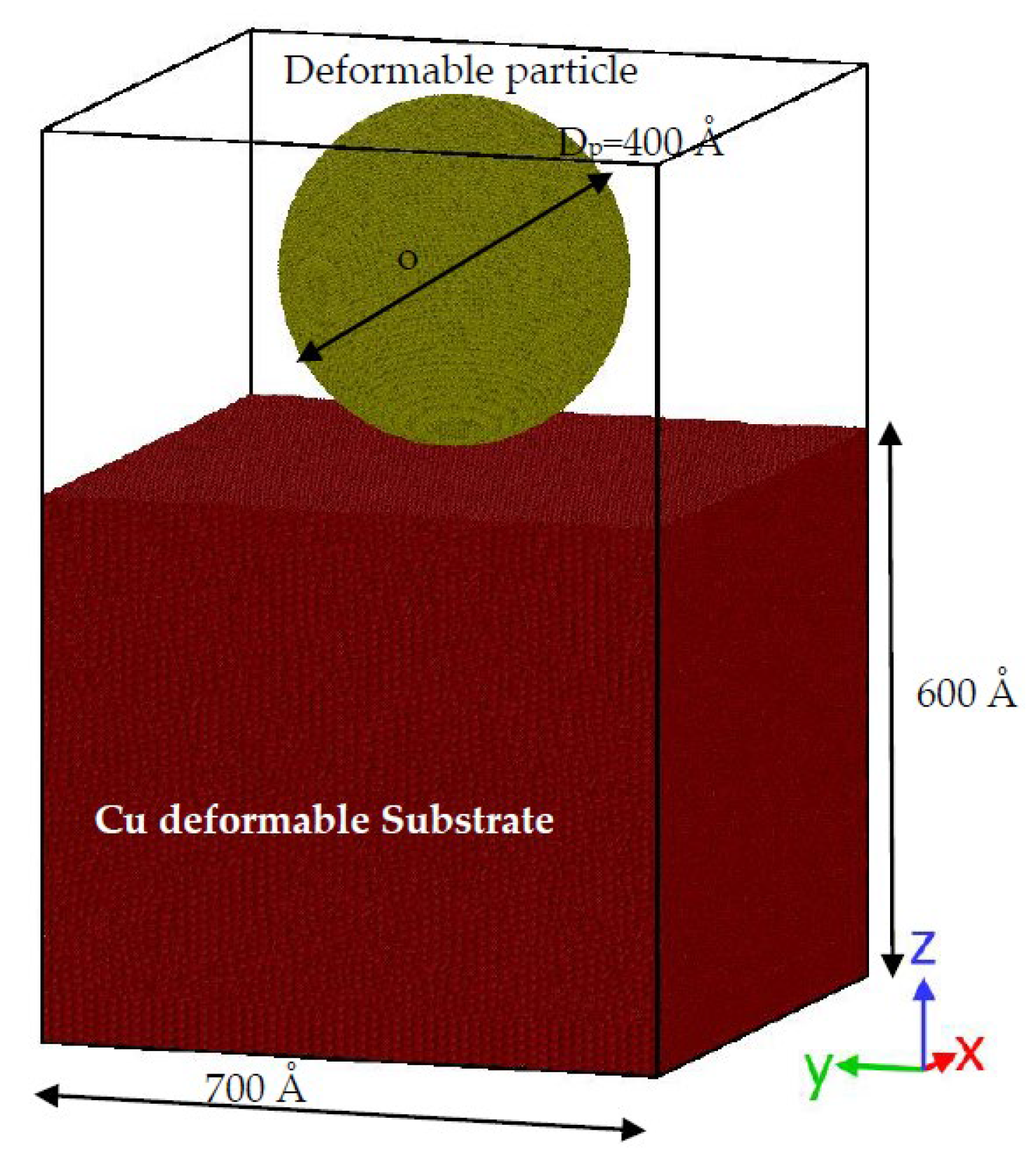






| Properties | Particle | Substrate |
|---|---|---|
| Material | Cu (1,908,733 atoms) Al (1,352,275 atoms) Ni (2,051,820 atoms) Ag (1,316,685 atoms) | Cu (19,873,953 atoms) |
| Dimensions | 400 Å Diameter | 700 Å × 700 Å × 600 Å |
| Initial impact velocity | 500, 700, 1000, 1500 m/s | - |
| Temperature | 273 K | 300 K |
| Crystal orientation | [1 0 0], [0 1 0], [0 0 1] in x-, y-, and z-directions | |
| Force field | EAM/alloy | |
| Time step | 0.001 ps (1.0 fs) | |
| Equilibration time | 20 ps | |
| Dynamics time | 20 ps | |
| Boundary condition | p p p | |
| Initial stand-off distance | 20 Å | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Temitope Oyinbo, S.; Jen, T.-C. Molecular Dynamics Simulation of Dislocation Plasticity Mechanism of Nanoscale Ductile Materials in the Cold Gas Dynamic Spray Process. Coatings 2020, 10, 1079. https://doi.org/10.3390/coatings10111079
Temitope Oyinbo S, Jen T-C. Molecular Dynamics Simulation of Dislocation Plasticity Mechanism of Nanoscale Ductile Materials in the Cold Gas Dynamic Spray Process. Coatings. 2020; 10(11):1079. https://doi.org/10.3390/coatings10111079
Chicago/Turabian StyleTemitope Oyinbo, Sunday, and Tien-Chien Jen. 2020. "Molecular Dynamics Simulation of Dislocation Plasticity Mechanism of Nanoscale Ductile Materials in the Cold Gas Dynamic Spray Process" Coatings 10, no. 11: 1079. https://doi.org/10.3390/coatings10111079
APA StyleTemitope Oyinbo, S., & Jen, T.-C. (2020). Molecular Dynamics Simulation of Dislocation Plasticity Mechanism of Nanoscale Ductile Materials in the Cold Gas Dynamic Spray Process. Coatings, 10(11), 1079. https://doi.org/10.3390/coatings10111079