Study on Key Mechanical Parameters of High-Strength Grouting Material
Abstract
:1. Introduction
2. Experiments
2.1. Materials
2.2. Experiment Design
2.3. Methods
3. Results and Discussion
3.1. Relationship between Axial Compressive Strength and Cubic Compressive Strength of HSGM
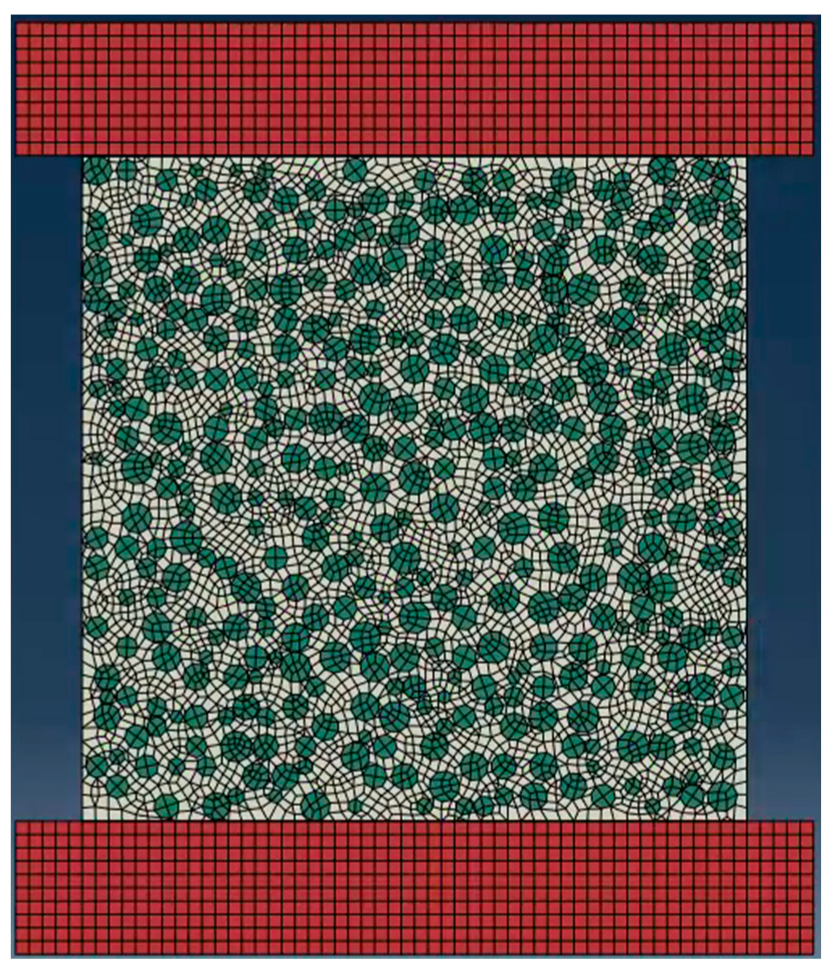
3.1.1. Finite Element Modeling
3.1.2. Formula for the Relationship between Axial Compressive Strength and Cubic Compressive Strength of HSGM
3.2. Relationship between Elastic Modulus and Cubic Compressive Strength of HSGM
3.2.1. Derivation of Elastic Modulus Model
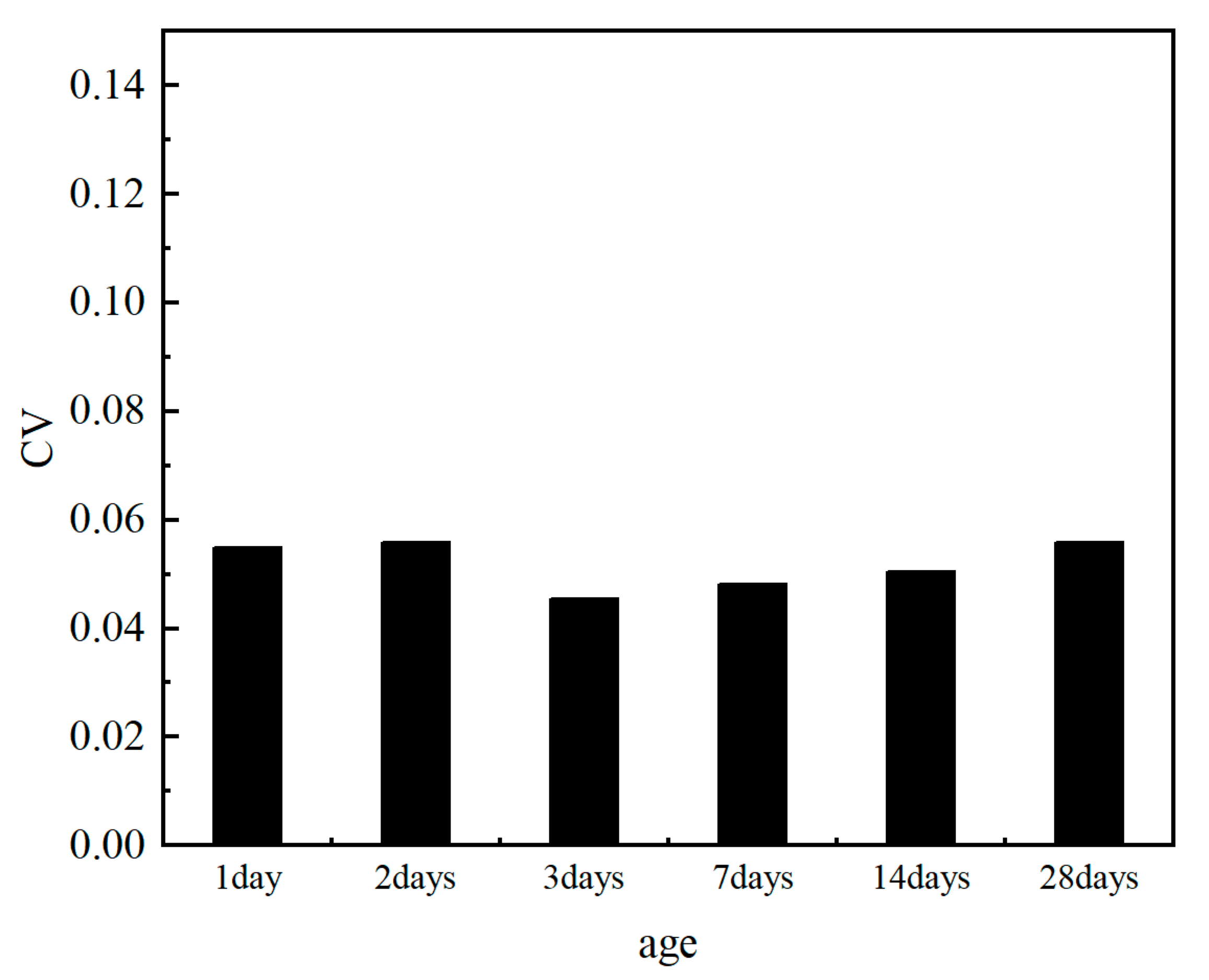
3.2.2. Test Verification of Elastic Modulus
3.3. Selection of Poisson’s Ratio
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Su, J.; Yang, Z.; Fang, Z. Size Effect of Concrete Compressive Strength with Different Aggregate Composition. Adv. Concr. Struct. 2009, 400–402, 831–835. [Google Scholar] [CrossRef]
- Lu, J.; Chen, J.; Gan, G.; Xu, F. Study on the Preparation of New Type High Capability and None Shrinkage Cement-based Grouting Material. Mater. Rev. 2016, 30, 123–129. [Google Scholar]
- Wang, D.; Zhang, Y.; Sun, X.; Gao, J. Development of ultra-high performance cement-based grouting material and its research & application in offshore wind power foundation construction. In Proceedings of the 2020 Fib Symposium: Concrete Structures for Resilient Society, Shanghai, China, 22–24 November 2020; pp. 36–45. [Google Scholar]
- Li, Z.X.; Chen, T.; Wang, X. Behavior of flat grouted connections subjected to lateral pressure and vertical load. Constr. Build. Mater. 2019, 212, 329–341. [Google Scholar] [CrossRef]
- Fu, T.; Ren, X.Q.; Li, Y.; Wang, K.; Zhu, Z.X. Experimental study on the mechanical behavior of HTRB600E steel sleeve grouting based on UHPC. Case Stud. Constr. Mater. 2022, 17, e01656. [Google Scholar] [CrossRef]
- Wang, X.; Chen, T.; Zhao, Q.; Yuan, G.K.; Liu, J.C. Fatigue Evaluation of Grouted Connections under Bending Moment in Offshore Wind Turbines Based on ABAQUS Scripting Interface. Int. J. Steel Struct. 2016, 16, 1149–1159. [Google Scholar] [CrossRef]
- Chen, T.; Cao, C.C.; Zhang, C.H.; Wang, X.; Chen, K.; Yuan, G.K. Numerical modeling and parametric analysis of grouted connections under axial loading. Thin-Walled Struct. 2020, 154, 106880. [Google Scholar] [CrossRef]
- GB/T50081-2019; Standard of Test Methods for Physical and Mechanical Properties of Concrete. Ministry of Housing and Urban-Rural Development of the PRC, State Administration for Market Regulation: Beijing, China, 2019.
- Cheng, S.-H.; Ren, Z.-G.; Li, P.-P.; Shangguan, J.-Y. Numerical Simulation of Concrete Aggregates with Arbitrary Shapes Based on ANSYS/LS-DYNA. J. Wuhan Univ. Technol. 2014, 36, 89–94. [Google Scholar] [CrossRef]
- Yang, Q.W.; Du, S.G. Prediction of concrete cubic compressive strength using ANN based size effect model. Comput. Mater. Contin. 2015, 47, 217–236. [Google Scholar]
- Kun, Z.; Bai Ru, L.; Xun An, Z.; Yi Hong, W.; Zhan, Q. Research on weibull distribution theory for cubic compressive strength test method of raw earth materials with different curing methods and time. Key Eng. Mater. 2021, 896, 129–140. [Google Scholar] [CrossRef]
- Su, J.; Zhang, J.; Zhou, C.B.; Fang, Z. Size Effect on the Cubic Compressive Strength of Reactive Powder Concrete. Rilem Proc. 2016, 105, 585–589. [Google Scholar]
- Zhou, J.J.; Pan, J.L.; Leung, C.K.Y.; Li, Z.J. Experimental study on mechanical behavior of high performance concrete under multi-axial compressive stress. Sci. China Technol. Sic. 2014, 57, 2514–2522. [Google Scholar] [CrossRef]
- He, X.X.; Lin, S.Y.; Zhen, X.C. Experimental Study on Size Effect of Compressive Strength and Deformation Properties of Large Size Fly Ash Concrete. Key Eng. Mater. 2011, 477, 319–324. [Google Scholar] [CrossRef]
- Bandeira, M.V.V.; Zydeck, R.C.; Kosteski, L.E.; Marangon, E. Concrete axial compressive strength with different loading directions and boundary conditions. Materia 2020, 25. [Google Scholar] [CrossRef]
- FaghihKhorasani, F.; Kabir, M.Z.; AhmadiNajafabad, M.; Ghavami, K. Predicting compressive stress-strain curves of structural adobe cubes based on Acoustic Emission (AE) hits and Weibull distribution. Int. J. Struct. Integr. 2019, 10, 766–791. [Google Scholar] [CrossRef]
- Xiong, M.X.; Liew, J.Y.R.; Wang, Y.B.; Xiong, D.X.; Lai, B.L. Effects of coarse aggregates on physical and mechanical properties of C170/185 ultra-high strength concrete and compressive behaviour of CFST columns. Constr. Build. Mater. 2020, 240, 117967. [Google Scholar] [CrossRef]
- Abid, M.; Hou, X.M.; Zheng, W.Z.; Hussain, R.R. Effect of Fibers on High-Temperature Mechanical Behavior and Microstructure of Reactive Powder Concrete. Materials 2019, 12, 329. [Google Scholar] [CrossRef] [Green Version]
- Ji, J.; Kang, W.; Jiang, L.Q.; Li, Y.H.; Ren, H.G.; Hao, S.X.; He, L.J.; Lin, Y.B.; Yu, C.Y. Mechanical Behavior of Reactive Powder Concrete Made From Local Material Subjected to Axial Pressure. Front. Mater. 2021, 8, 737646. [Google Scholar] [CrossRef]
- Bandeira, M.V.V.; Torre, K.R.L.; Kosteski, L.E.; Marangon, E.; Riera, J.D. Influence of contact friction in compression tests of concrete samples. Constr. Build. Mater. 2022, 317, 125811. [Google Scholar] [CrossRef]
- Wu, H.M.; Liu, K.; Yang, F.; Shen, B.; Ma, K.J.; Zhang, J.Y.; Liu, B. Experimental Mechanical Properties and Numerical Simulation of C80 Concrete with Different Contents of Stone Powder. Materials 2022, 15, 3282. [Google Scholar] [CrossRef]
- Li, M.; Hao, H.; Cui, J.; Hao, Y.F. Numerical investigation of the failure mechanism of cubic concrete specimens in SHPB tests. Def. Technol 2022, 18, 1–11. [Google Scholar] [CrossRef]
- Qiu, Y.R.; Zhang, G.X.; Yin, X.N. Parameters inversion of mortar in concrete considering the hardening process based on meso-mechanics. In Proceedings of the Third Technical Conference on Hydraulic Engineering, Hongkong, China, 13–14 December 2014; pp. 85–92. [Google Scholar]
- He, Z.H.; Du, S.G.; Chen, D. Microstructure of ultra high performance concrete containing lithium slag. J. Hazard. Mater. 2018, 353, 35–43. [Google Scholar] [CrossRef] [PubMed]
- Yang, C.C.; Huang, R. Double inclusion model for approximate elastic moduli of concrete material. Cem. Concr. Res. 1996, 26, 83–91. [Google Scholar] [CrossRef]
- Krishnya, S.; Elakneswaran, Y.; Yoda, Y. Proposing a three-phase model for predicting the mechanical properties of mortar and concrete. Mater. Today Commun. 2021, 29, 102858. [Google Scholar] [CrossRef]
- Mina, A.L.; Trezos, K.G.; Petrou, M.F. Optimizing the Mechanical Properties of Ultra-High-Performance Fibre-Reinforced Concrete to Increase Its Resistance to Projectile Impact. Materials 2021, 14, 5098. [Google Scholar] [CrossRef]
- Yang, H.Q.; Rao, M.J.; Dong, Y. Influence study of extra-large stone limited size and content on full-graded concrete properties. Constr. Build. Mater. 2016, 127, 774–783. [Google Scholar] [CrossRef]
- Aili, A.; Vandamme, M.; Torrenti, J.M.; Masson, B.; Sanahuja, J. Time evolutions of non-aging viscoelastic poisson’s ratio of concrete and implications for creep of C-S-H. Cem. Concr. Res. 2016, 90, 144–161. [Google Scholar] [CrossRef]








| - | Density | Specific Surface Area | 1-Day Compressive Strength | 3-Day Compressive Strength | 7-Day Compressive Strength | 28-Day Compressive Strength |
|---|---|---|---|---|---|---|
| P.II52.5 Portland cement | 3.02 g/cm3 | 394 m2/kg | 23.1 MPa | 39.3 MPa | 50.8 MPa | 57.9 MPa |
| - | Apparent Density | Bulk Density | Tight Bulk Density | Stone Powder Content | Water Absorption |
|---|---|---|---|---|---|
| Fine aggregate | 2700 kg/m3 | 1490 kg/m3 | 1590 kg/m3 | 1.5% | 1.2% |
| - | Appearance | Moisture Content | pH | Bulk Density | Water Reduction Rate |
|---|---|---|---|---|---|
| Water reducing agent | Pale yellow to white | 0.5% | 7.2 | 450 kg/m3 | 28% |
| No. | Water-Binder Ratio | Sand-Binder Ratio | Water Reducing Agent Content | Test Content |
|---|---|---|---|---|
| 1 | 0.2 | 1.15 | 0.4% | Axial compressive strength, cubic compressive strength and Possion’s ratio at 1 day, 2 days, 3 days, 7 days, 14 days and 28 days, three times |
| 2 | 0.2 | 1.75 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 3 | 0.2 | 1.55 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 4 | 0.2 | 1.35 | 0.4% | Elastic modulus, cubic compressive strength and Possion’s ratio at 7 days |
| 5 | 0.2 | 1.15 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 6 | 0.2 | 0.95 | 0.4% | Elastic modulus, cubic compressive strength and Possion’s ratio at 7 days |
| 7 | 0.2 | 0.75 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 8 | 0.2 | 0.55 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 9 | 0.2 | 0.35 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| 10 | 0.2 | 0 | 0.4% | Elastic modulus and cubic compressive strength at 7 days |
| - | Less Than 5 mm | 5 mm–10 mm | 10 mm–15 mm | 15 mm–20 mm | Totol |
|---|---|---|---|---|---|
| Grouting material | 42% | / | / | / | 42% |
| Concrete | 20% | 9% | 7% | 6% | 38% |
| No. | Sand to Paste Ratios | Volume Contents of Aggregate | Cubic Strength/MPa | Elastic Modulus/GPa |
|---|---|---|---|---|
| 1 | 1.75 | 52% | 114.7 | 42.1 |
| 2 | 1.55 | 49% | 115.2 | 43.5 |
| 3 | 1.35 | 46% | 109.1 | 42.2 |
| 4 | 1.15 | 42% | 115.3 | 40.0 |
| 5 | 0.95 | 37% | 115.4 | 39.3 |
| 6 | 0.75 | 32% | 117.6 | 37.3 |
| 7 | 0.55 | 25% | 116.1 | 33.4 |
| 8 | 0.35 | 18% | 114.5 | 28.5 |
| 9 | 0 | 0% | 116.5 | 27.0 |
| No. | Compressive Poisson’s Ratio | Tensile Poisson’s Ratio |
|---|---|---|
| 1 | 0.244 | 0.271 |
| 2 | 0.242 | 0.259 |
| 3 | 0.241 | 0.257 |
| 4 | 0.243 | 0.256 |
| 5 | 0.242 | 0.255 |
| 6 | 0.240 | 0.257 |
| 7 | 0.239 | 0.243 |
| 8 | 0.245 | 0.259 |
| 9 | 0.242 | 0.259 |
| 10 | 0.244 | 0.271 |
| 11 | 0.239 | 0.267 |
| 12 | 0.251 | 0.237 |
| 13 | 0.247 | 0.259 |
| 14 | 0.259 | 0.242 |
| 15 | 0.248 | 0.234 |
| 16 | 0.257 | 0.246 |
| 17 | 0.248 | 0.236 |
| 18 | 0.254 | 0.244 |
| 19 | 0.273 | 0.240 |
| 20 | 0.266 | 0.261 |
| average value | 0.248 | 0.253 |
| standard deviation | 0.009 | 0.011 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Cao, B.; Zhang, H.; Zhang, X.; He, Y. Study on Key Mechanical Parameters of High-Strength Grouting Material. Coatings 2023, 13, 440. https://doi.org/10.3390/coatings13020440
Zhang Y, Cao B, Zhang H, Zhang X, He Y. Study on Key Mechanical Parameters of High-Strength Grouting Material. Coatings. 2023; 13(2):440. https://doi.org/10.3390/coatings13020440
Chicago/Turabian StyleZhang, Yueran, Baoyong Cao, Heng Zhang, Xiong Zhang, and Yan He. 2023. "Study on Key Mechanical Parameters of High-Strength Grouting Material" Coatings 13, no. 2: 440. https://doi.org/10.3390/coatings13020440
APA StyleZhang, Y., Cao, B., Zhang, H., Zhang, X., & He, Y. (2023). Study on Key Mechanical Parameters of High-Strength Grouting Material. Coatings, 13(2), 440. https://doi.org/10.3390/coatings13020440





