Analysis of the Relationship between APS Thermal Spray Parameters of Ni5Al Particles and Coating Characteristics
Abstract
:1. Introduction
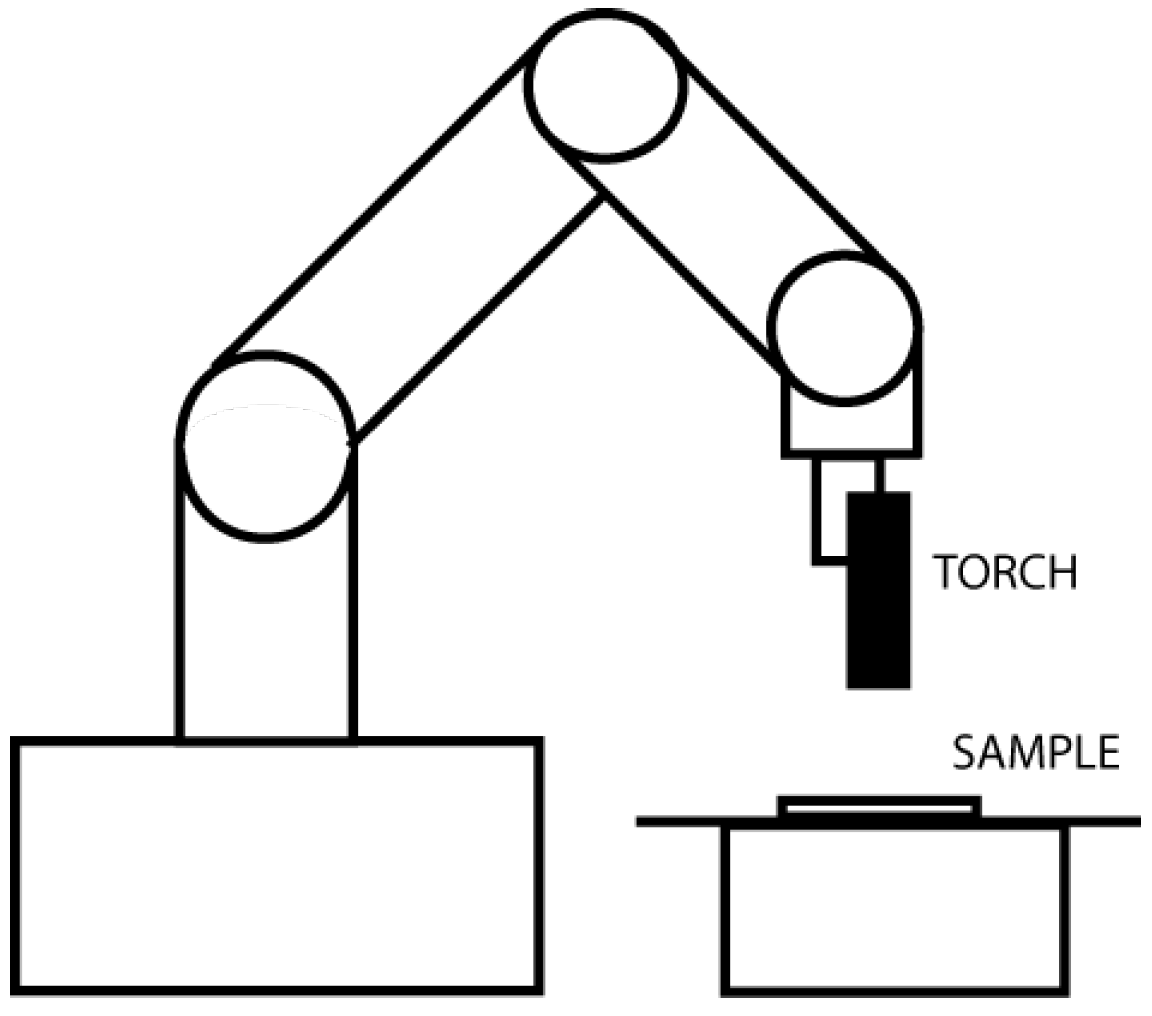
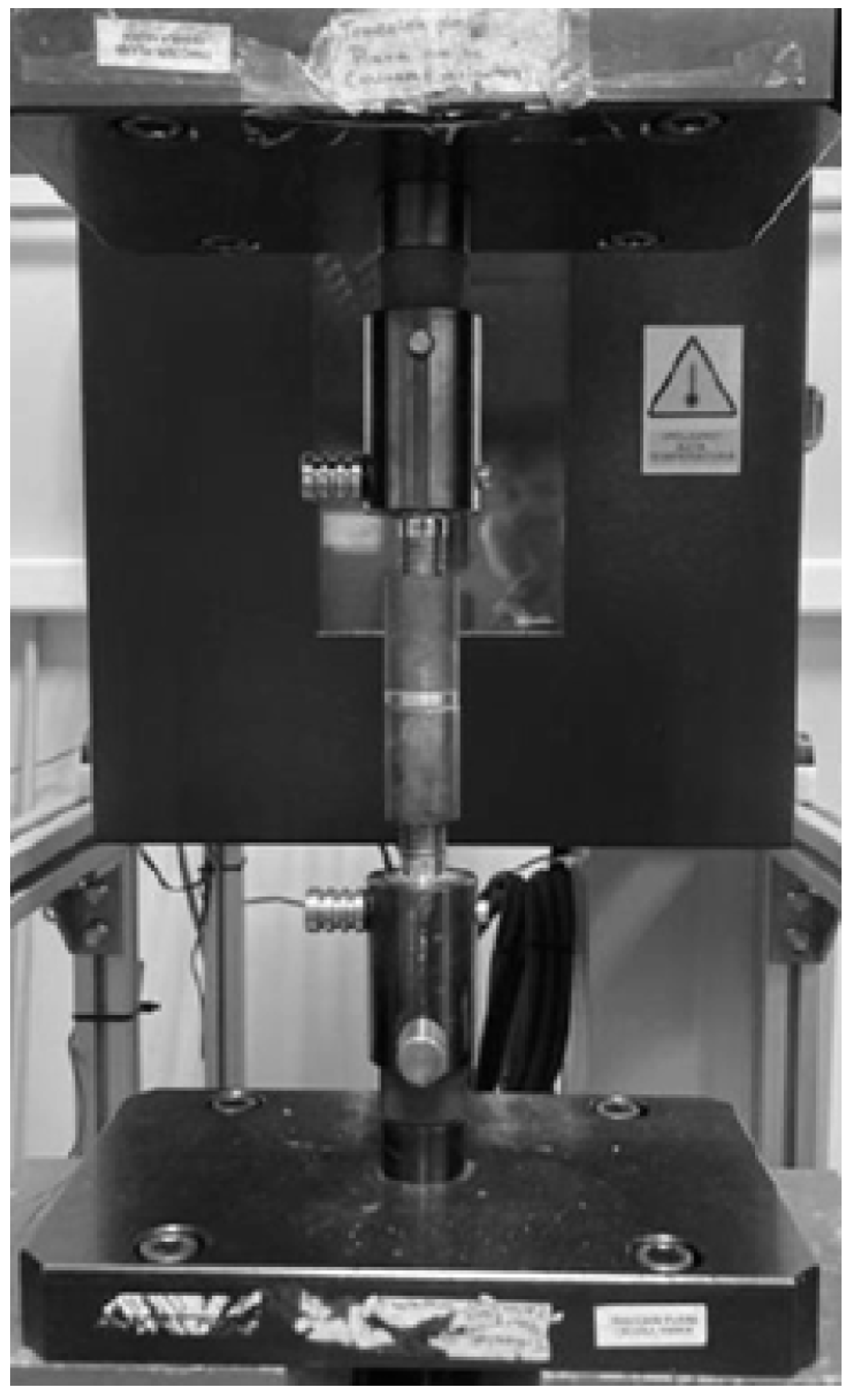
2. Materials and Methodology
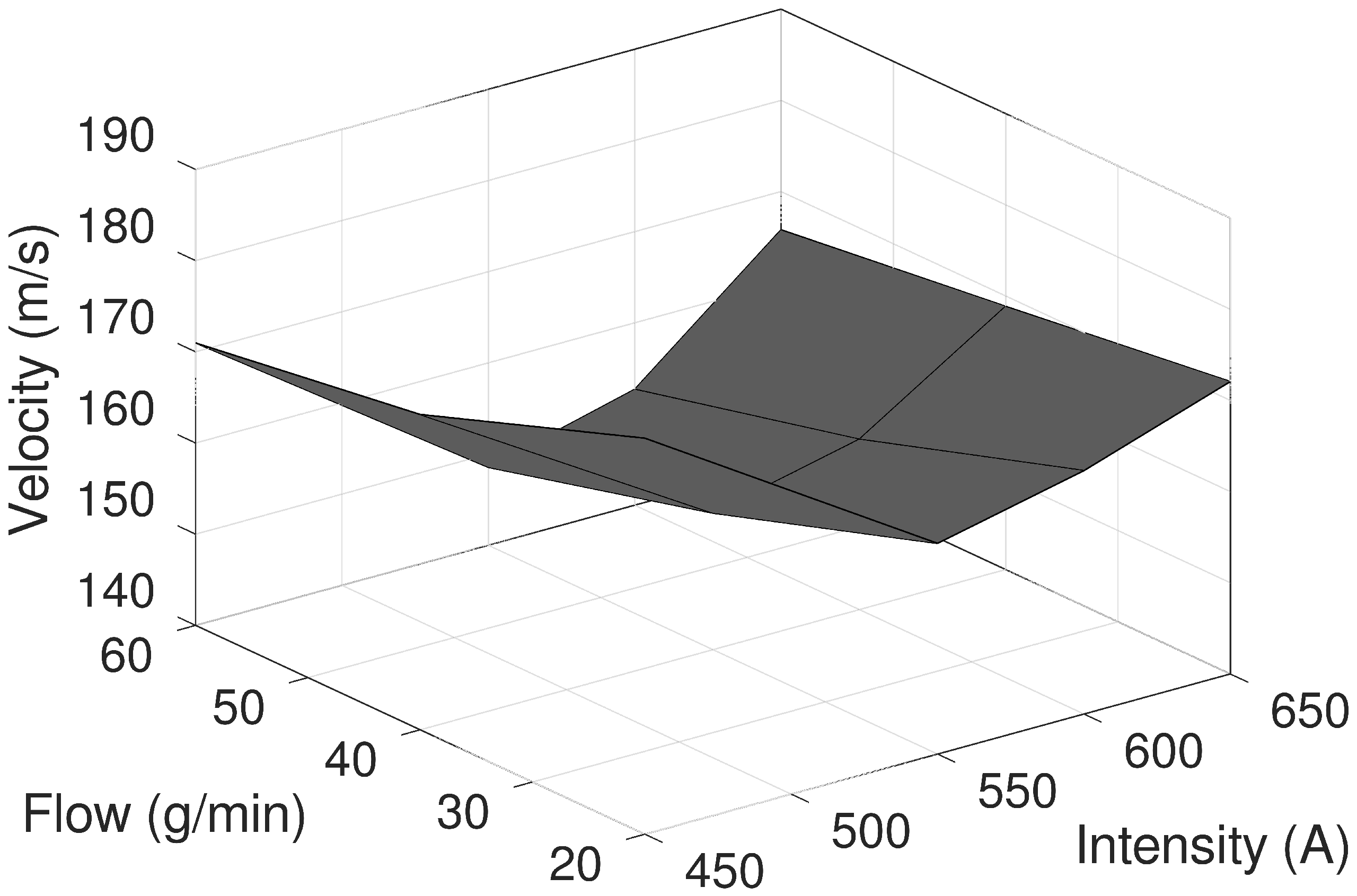
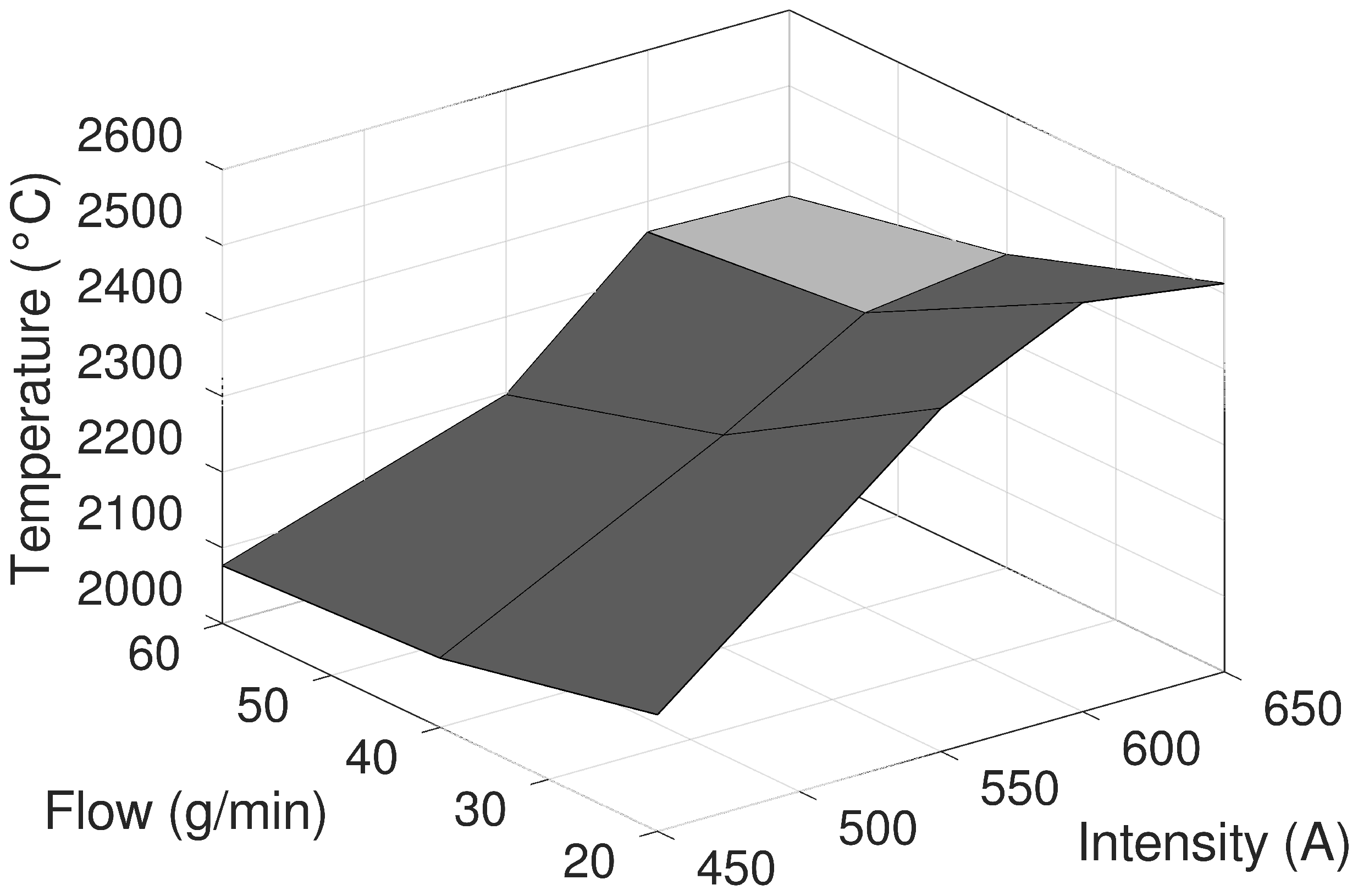
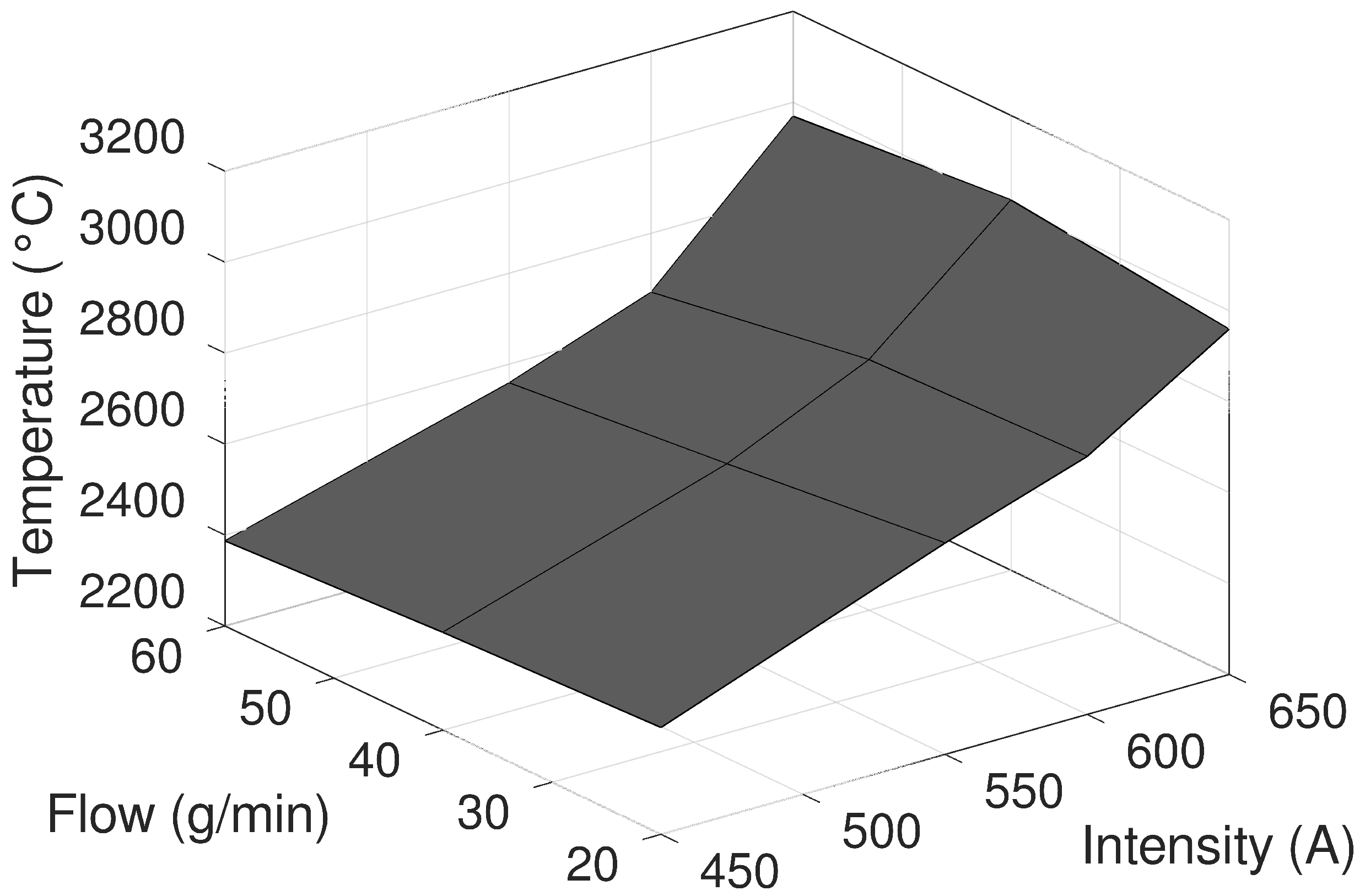
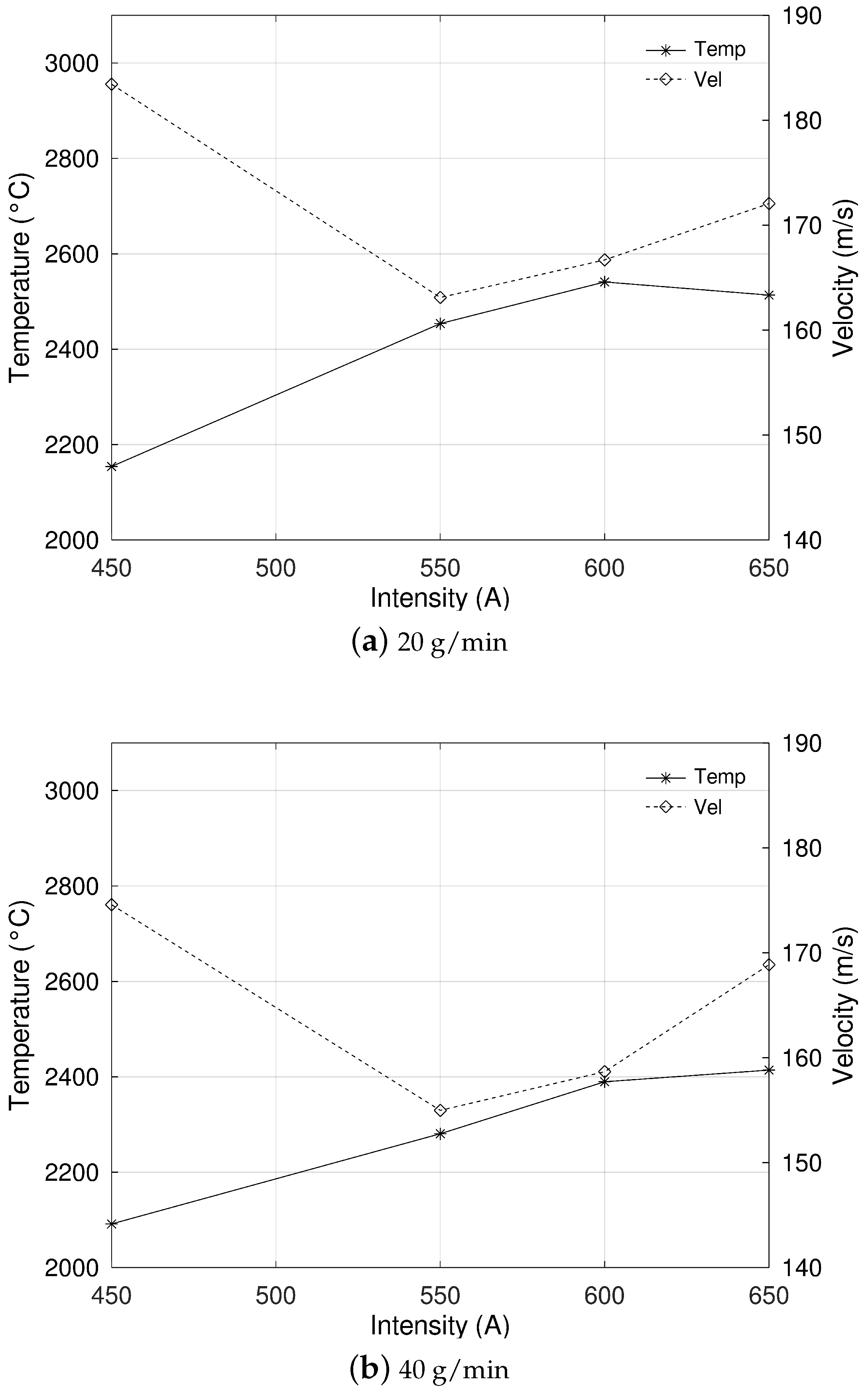
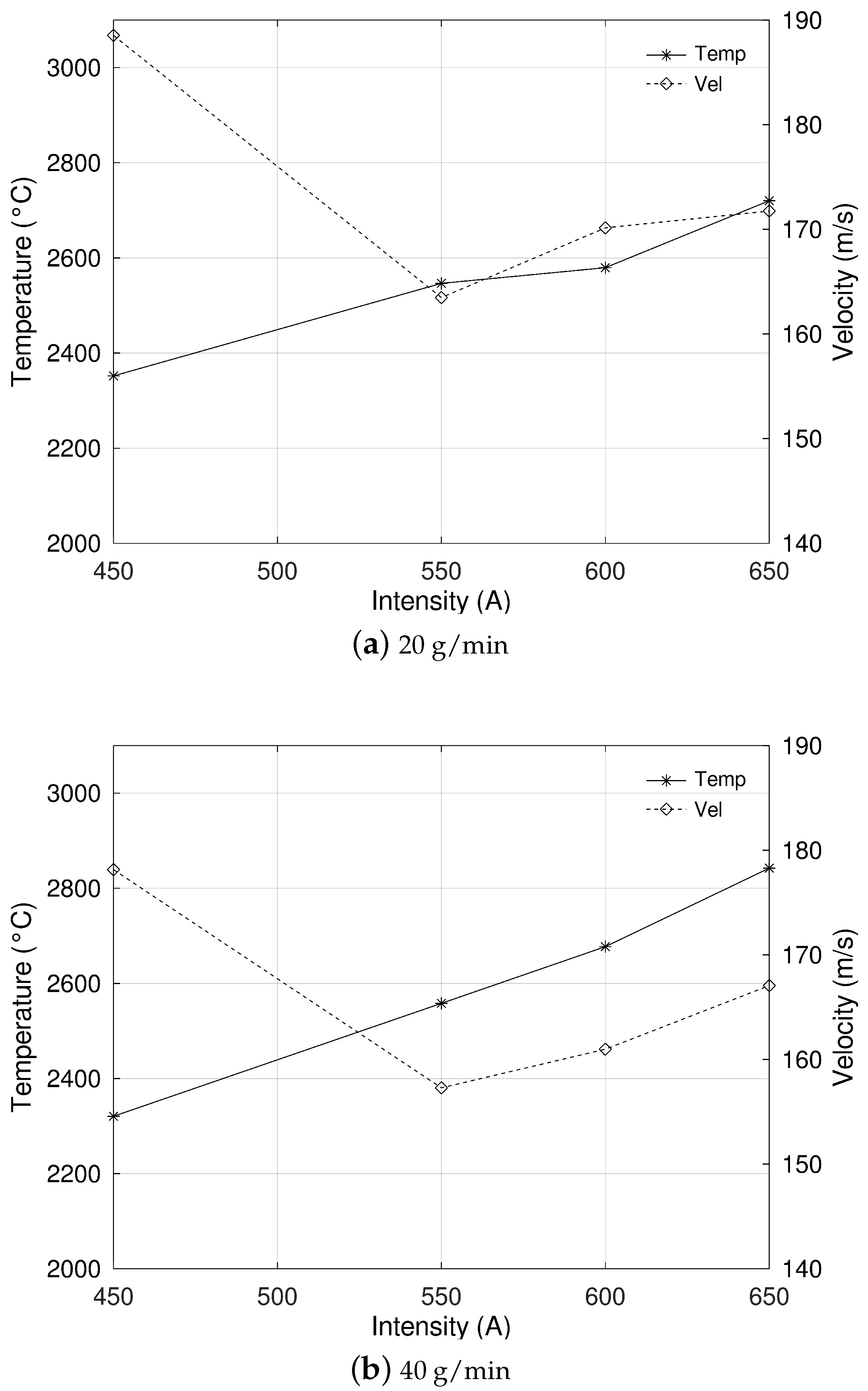
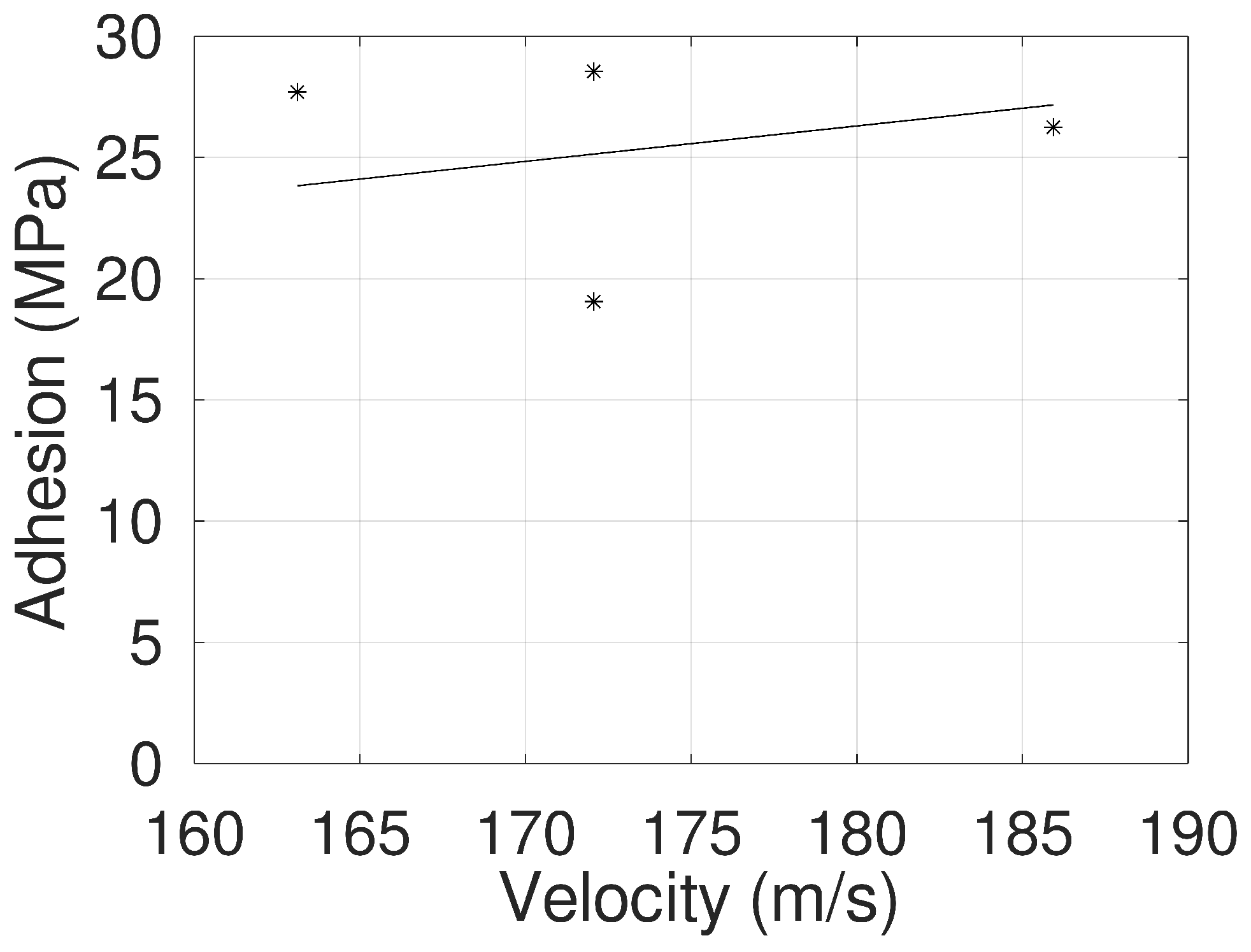
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| APS | Atmospheric Plasma Spray |
| DoE | Design of experiments |
| ANN | Artificial Neural Network Modelling |
| Ni5Al | Nickel-Aluminium |
References
- Chandra, S.; Fauchais, P. Formation of solid splats during thermal spray deposition. J. Therm. Spray Technol. 2009, 18, 148–180. [Google Scholar] [CrossRef]
- Dhiman, R.; McDonald, A.G.; Chandra, S. Predicting splat morphology in a thermal spray process. Surf. Coatings Technol. 2007, 201, 7789–7801. [Google Scholar] [CrossRef]
- Fauchais, P.; Vardelle, M.; Goutier, S. Atmospheric plasma spraying evolution since the sixties through modeling, measurements and sensors. Plasma Chem. Plasma Process. 2017, 37, 601–626. [Google Scholar] [CrossRef]
- Tanaka, Y.; Fukumoto, M. Investigation of dominating factors on flattening behavior of plasma sprayed ceramic particles. Surf. Coatings Technol. 1999, 120, 124–130. [Google Scholar] [CrossRef]
- Tanaka, Y.; Fukumoto, M. Influence of solidification and wetting on flattening behavior of plasma sprayed ceramic particles. In Proceedings of the ISAEM 2000: 2nd International Symposium on Designing, Processing and Properties of Advanced Engineering Materials, Guilin, China, 2000; pp. 518–523. [Google Scholar]
- Fukumoto, M.; Huang, Y. Flattening mechanism in thermal sprayed nickel particle impinging on flat substrate surface. J. Therm. Spray Technol. 1999, 8, 427–432. [Google Scholar] [CrossRef]
- Jiang, X.; Wan, Y.; Herman, H.; Sampath, S. Role of condensates and adsorbates on substrate surface on fragmentation of impinging molten droplets during thermal spray. Thin Solid Film. 2001, 385, 132–141. [Google Scholar] [CrossRef]
- Pershin, V.; Lufitha, M.; Chandra, S.; Mostaghimi, J. Effect of substrate temperature on adhesion strength of plasma-sprayed nickel coatings. J. Therm. Spray Technol. 2003, 12, 370. [Google Scholar] [CrossRef]
- Okumus, S.C. Microstructural and mechanical characterization of plasma sprayed Al2O3–TiO2 composite ceramic coating on Mo/cast iron substrates. Mater. Lett. 2005, 59, 3214–3220. [Google Scholar] [CrossRef]
- Syed, A.; Denoirjean, A.; Hannoyer, B.; Fauchais, P.; Denoirjean, P.; Khan, A.; Labbe, J. Influence of substrate surface conditions on the plasma sprayed ceramic and metallic particles flattening. Surf. Coatings Technol. 2005, 200, 2317–2331. [Google Scholar] [CrossRef]
- Fukumoto, M.; Yamaguchi, T.; Yamada, M.; Yasui, T. Splash splat to disk splat transition behavior in plasma-sprayed metallic materials. J. Therm. Spray Technol. 2007, 16, 905–912. [Google Scholar] [CrossRef]
- McDonald, A.; Moreau, C.; Chandra, S. Thermal contact resistance between plasma-sprayed particles and flat surfaces. Int. J. Heat Mass Transf. 2007, 50, 1737–1749. [Google Scholar] [CrossRef]
- Tekmen, C.; Tsunekawa, Y.; Okumiya, M. Effect of plasma spray parameters on in-flight particle characteristics and in situ alumina formation. Surf. Coatings Technol. 2008, 203, 223–228. [Google Scholar] [CrossRef]
- Yin, Z.; Tao, S.; Zhou, X.; Ding, C. Particle in-flight behavior and its influence on the microstructure and mechanical properties of plasma-sprayed Al2O3 coatings. J. Eur. Ceram. Soc. 2008, 28, 1143–1148. [Google Scholar] [CrossRef]
- Zhang, C.; Li, C.J.; Liao, H.; Planche, M.P.; Li, C.X.; Coddet, C. Effect of in-flight particle velocity on the performance of plasma-sprayed YSZ electrolyte coating for solid oxide fuel cells. Surf. Coatings Technol. 2008, 202, 2654–2660. [Google Scholar] [CrossRef]
- Zhang, C.; Kanta, A.F.; Li, C.X.; Li, C.J.; Planche, M.P.; Liao, H.; Coddet, C. Effect of in-flight particle characteristics on the coating properties of atmospheric plasma-sprayed 8 mol% Y2O3–ZrO2 electrolyte coating studying by artificial neural networks. Surf. Coatings Technol. 2009, 204, 463–469. [Google Scholar] [CrossRef]
- Mantry, S.; Jha, B.; Mandal, A.; Mishra, D.; Mishra, B.; Chakraborty, M. Influence of in-flight particle state diagnostics on properties of plasma sprayed YSZ-CeO2 nanocomposite coatings. Int. J. Smart Nano Mater. 2014, 5, 207–216. [Google Scholar] [CrossRef]
- Xiao, Y.; Ren, E.; Hu, M.; Liu, K. Effect of particle in-flight behavior on the microstructure and fracture toughness of YSZ TBCs prepared by plasma spraying. Coatings 2018, 8, 309. [Google Scholar] [CrossRef]
- Pierlot, C.; Pawlowski, L.; Bigan, M.; Chagnon, P. Design of experiments in thermal spraying: A review. Surf. Coatings Technol. 2008, 202, 4483–4490. [Google Scholar] [CrossRef]
- Heimann, R.B. Better quality control: Stochastic approaches to optimize properties and performance of plasma-sprayed coatings. J. Therm. Spray Technol. 2010, 19, 765–778. [Google Scholar] [CrossRef]
- Guessasma, S.; Montavon, G.; Coddet, C. Neural computation to predict in-flight particle characteristic dependences from processing parameters in the APS process. J. Therm. Spray Technol. 2004, 13, 570–585. [Google Scholar] [CrossRef]
- Forghani, S.; Ghazali, M.; Muchtar, A.; Daud, A. Mechanical properties of plasma sprayed nanostructured TiO2 coatings on mild steel. Ceram. Int. 2014, 40, 7049–7056. [Google Scholar] [CrossRef]
- Karthikeyan, S.; Balasubramanian, V.; Rajendran, R. Developing empirical relationships to estimate porosity and microhardness of plasma-sprayed YSZ coatings. Ceram. Int. 2014, 40, 3171–3183. [Google Scholar] [CrossRef]
- Batra, R.C.; Taetragool, U. Numerical techniques to find optimal input parameters for achieving mean particles’ temperature and axial velocity in atmospheric plasma spray process. Sci. Rep. 2020, 10, 21483. [Google Scholar] [CrossRef] [PubMed]
- Chadha, S.; Jefferson-Loveday, R.; Hussain, T. Modelling Knudsen number effects in suspension high velocity oxy fuel thermal spray. Int. J. Heat Mass Transf. 2020, 152, 119454. [Google Scholar] [CrossRef]
- Oerlikon Metco. SF-0001.5-Real-Time Sensor Technology; Oerlikon Metco: Pfaeffikon, Switzerland, 2022. [Google Scholar]
- Tecnar. User Manual Accuraspray; Tecnar: Saint-Bruno-de-Montarville, QC, Canada, 2021. [Google Scholar]
- Mauer, G.; Vaßen, R.; Stöver, D. Plasma and particle temperature measurements in thermal spray: Approaches and applications. J. Therm. Spray Technol. 2011, 20, 391–406. [Google Scholar] [CrossRef]
- Akbarnozari, A.; Amiri, S.; Moreau, C.; Bamber, O.; Gernon, J.; Choquet, M.; Pouliot, L. Improvement of Online Diagnostic System to Monitor In-Flight Particles in Thermal Spray Processes. In Proceedings of the ITSC2019, ASM International, Yokohama, Japan, 25–26 May 2019; pp. 957–964. [Google Scholar]
- Gougeon, P. A new sensor for on-line diagnostics of particles under thermal spraying conditions. Adv. Process. Tech. Part. Mater. 1994, 6, 199. [Google Scholar]
- Wei, P.; Wei, Z.; Zhao, G.; Bai, Y.; Tan, C. Effect of processing parameters on plasma jet and in-flight particles characters in supersonic plasma spraying. High Temp. Mater. Process. 2016, 35, 775–786. [Google Scholar] [CrossRef]










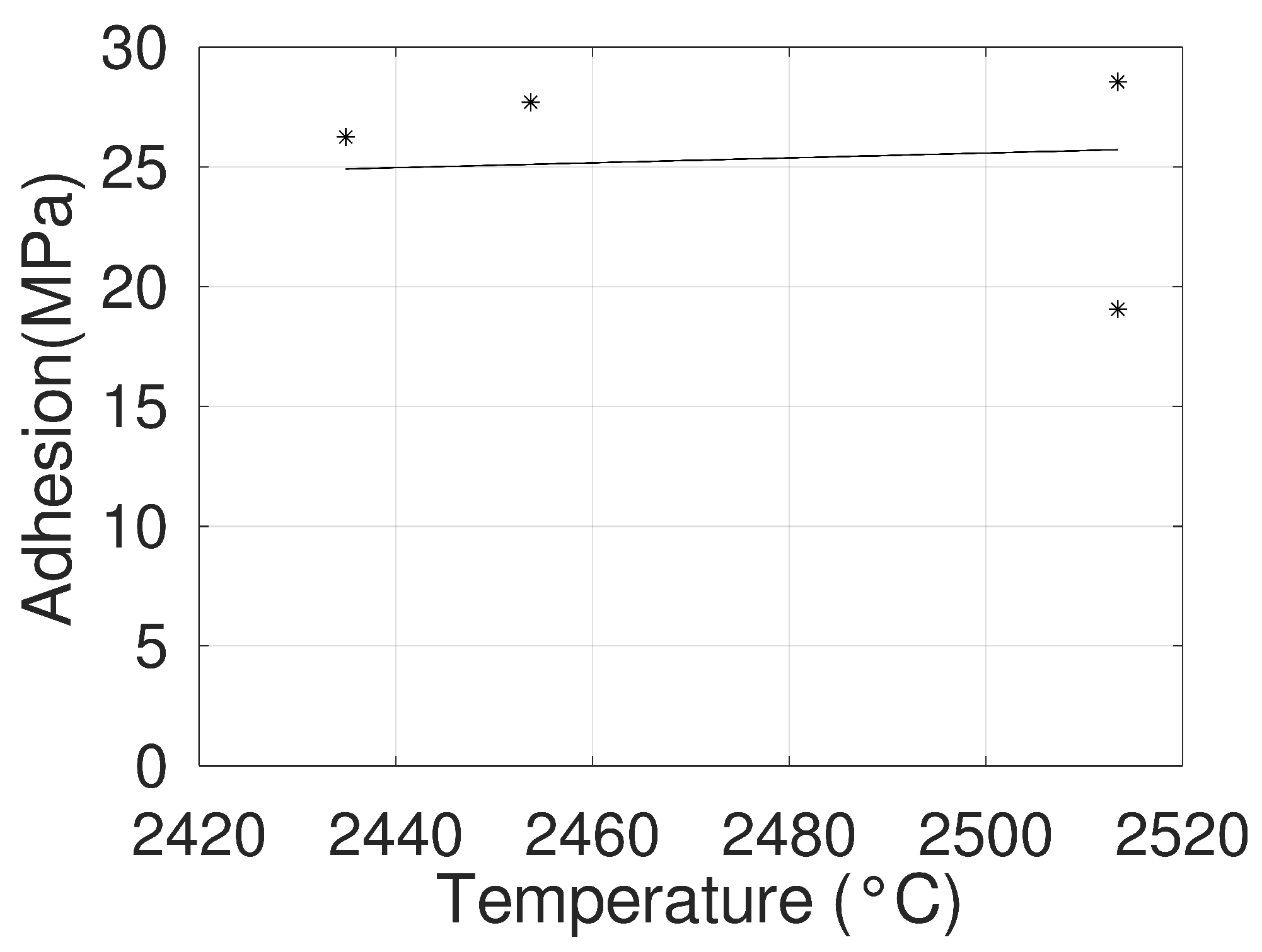

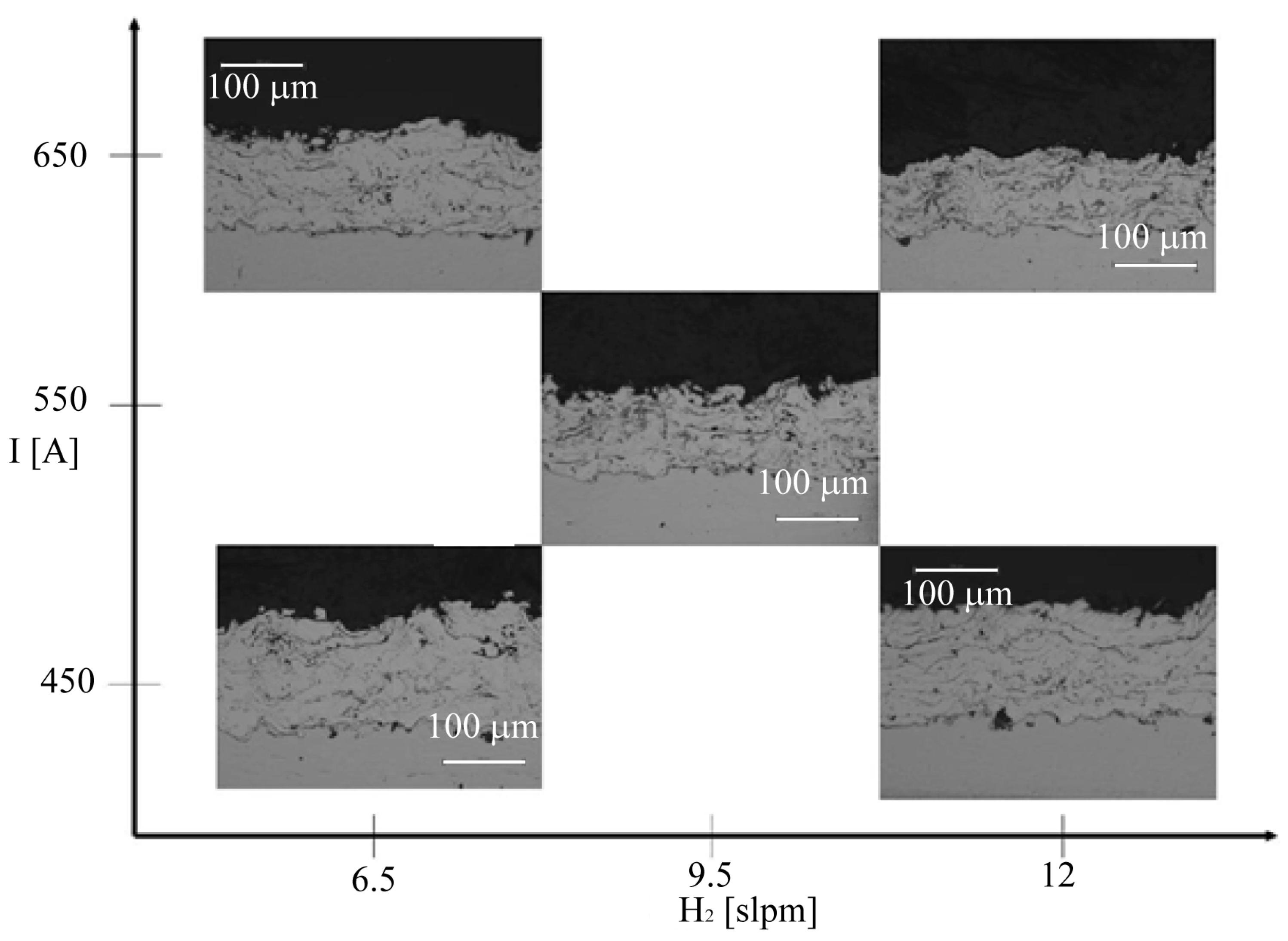
| Parameter | Values | |||
|---|---|---|---|---|
| Current (A) | 450 | 550 | 600 | 650 |
| flow rate (slpm) | 6.5 | 9.5 | 12 | |
| Powder flow rate (g/min) | 20 | 40 | 60 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernández Roiz, A.; Moreno, J.A.; Jorde Cerezo, G.; Solano, J. Analysis of the Relationship between APS Thermal Spray Parameters of Ni5Al Particles and Coating Characteristics. Coatings 2023, 13, 1564. https://doi.org/10.3390/coatings13091564
Fernández Roiz A, Moreno JA, Jorde Cerezo G, Solano J. Analysis of the Relationship between APS Thermal Spray Parameters of Ni5Al Particles and Coating Characteristics. Coatings. 2023; 13(9):1564. https://doi.org/10.3390/coatings13091564
Chicago/Turabian StyleFernández Roiz, Adrián, José Andrés Moreno, Guillermo Jorde Cerezo, and Joaquín Solano. 2023. "Analysis of the Relationship between APS Thermal Spray Parameters of Ni5Al Particles and Coating Characteristics" Coatings 13, no. 9: 1564. https://doi.org/10.3390/coatings13091564
APA StyleFernández Roiz, A., Moreno, J. A., Jorde Cerezo, G., & Solano, J. (2023). Analysis of the Relationship between APS Thermal Spray Parameters of Ni5Al Particles and Coating Characteristics. Coatings, 13(9), 1564. https://doi.org/10.3390/coatings13091564






