Mid-Infrared (MIR) Complex Refractive Index Spectra of Polycrystalline Copper-Nitride Films by IR-VASE Ellipsometry and Their FIB-SEM Porosity
Abstract
:1. Introduction
2. Experimental Procedure
3. Results and Discussion
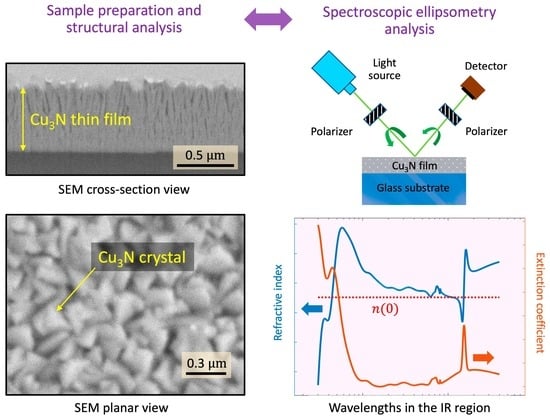
3.1. FIB-SEM Microscopy Study
3.2. UV-MIR Ellipsometric Analysis
3.3. FTIR and Raman Analysis
3.4. Using the Sub-Gap Wemple–DiDomenico Single-Oscillator Dispersion Model
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jiang, A.; Qi, M.; Xiao, J. Preparation, structure, properties, and application of copper nitride (Cu3N) thin films: A review. J. Mater. Sci. Technol. 2018, 34, 1467–1473. [Google Scholar] [CrossRef]
- Zakutayev, A. Design of nitride semiconductors for solar energy conversion. J. Mater. Chem. A 2016, 4, 6742–6754. [Google Scholar] [CrossRef]
- Borsa, D.; Boerma, D. Growth, structural and optical properties of Cu3N films. Surf. Sci. 2004, 548, 95–105. [Google Scholar] [CrossRef]
- Rodríguez-Tapiador, M.I.; Merino, J.; Jawhari, T.; Muñoz-Rosas, A.L.; Bertomeu, J.; Fernández, S. Impact of the RF Power on the Copper Nitride Films Deposited in a Pure Nitrogen Environment for Applications as Eco-Friendly Solar Absorber. Materials 2023, 16, 1508. [Google Scholar] [CrossRef] [PubMed]
- Márquez, E.; Blanco, E.; García-Gurrea, M.; Cintado Puerta, M.; Domínguez de la Vega, M.; Ballester, M.; Mánuel, J.; Rodríguez-Tapiador, M.; Fernández, S. Optical Properties of Reactive RF Magnetron Sputtered Polycrystalline Cu3N Thin Films Determined by UV/Visible/NIR Spectroscopic Ellipsometry: An Eco-Friendly Solar Light Absorber. Coatings 2023, 13, 1148. [Google Scholar] [CrossRef]
- Paredes, P.; Rauwel, E.; Rauwel, P. Surveying the Synthesis, Optical Properties and Photocatalytic Activity of Cu3N Nanomaterials. Nanomaterials 2022, 12, 2218. [Google Scholar] [CrossRef]
- Caskey, C.M.; Richards, R.M.; Ginley, D.S.; Zakutayev, A. Thin film synthesis and properties of copper nitride, a metastable semiconductor. Mater. Horizons 2014, 1, 424–430. [Google Scholar] [CrossRef]
- Chen, S.C.; Huang, S.Y.; Sakalley, S.; Paliwal, A.; Chen, Y.H.; Liao, M.H.; Sun, H.; Biring, S. Optoelectronic properties of Cu3N thin films deposited by reactive magnetron sputtering and its diode rectification characteristics. J. Alloy. Compd. 2019, 789, 428–434. [Google Scholar] [CrossRef]
- Chen, Y.H.; Lee, P.I.; Sakalley, S.; Wen, C.K.; Cheng, W.C.; Sun, H.; Chen, S.C. Enhanced electrical properties of copper nitride films deposited via high power impulse magnetron sputtering. Nanomaterials 2022, 12, 2814. [Google Scholar] [CrossRef]
- Ohring, M. Materials Science of Thin Films: Depositon and Structure; Elsevier: New York, NY, USA, 2001. [Google Scholar]
- Wang, J.; Chen, J.; Yuan, X.; Wu, Z.; Miao, B.; Yan, P. Copper nitride (Cu3N) thin films deposited by RF magnetron sputtering. J. Cryst. Growth 2006, 286, 407–412. [Google Scholar] [CrossRef]
- Yuan, X.; Yan, P.; Liu, J. Preparation and characterization of copper nitride films at various nitrogen contents by reactive radio-frequency magnetron sputtering. Mater. Lett. 2006, 60, 1809–1812. [Google Scholar] [CrossRef]
- Hadian, F.; Rahmati, A.; Movla, H.; Khaksar, M. Reactive DC magnetron sputter deposited copper nitride nano-crystalline thin films: Growth and characterization. Vacuum 2012, 86, 1067–1072. [Google Scholar] [CrossRef]
- Khalaf, M.K.; Saud, N.; Muhammed, M.S. Plasma Diagnostics and Characterizations of Reactive Magnetron Sputtered Copper Nitride Thin Films. In Proceedings of the IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2020; Volume 928, p. 072029. [Google Scholar]
- Wilczopolska, M.; Nowakowska-Langier, K.; Okrasa, S.; Skowronski, L.; Minikayev, R.; Strzelecki, G.W.; Chodun, R.; Zdunek, K. Synthesis of copper nitride layers by the pulsed magnetron sputtering method carried out under various operating conditions. Materials 2021, 14, 2694. [Google Scholar] [CrossRef] [PubMed]
- Tompkins, H.G.; Hilfiker, J.N. Spectroscopic Ellipsometry: Practical Application to Thin Film Characterization; Momentum Press: New York, NY, USA, 2015. [Google Scholar]
- Márquez, E.; Blanco, E.; García-Vázquez, C.; Díaz, J.; Saugar, E. Spectroscopic ellipsometry study of non-hydrogenated fully amorphous silicon films deposited by room-temperature radio-frequency magnetron sputtering on glass: Influence of the argon pressure. J. Non Cryst. Solids 2020, 547, 120305. [Google Scholar] [CrossRef]
- Thornton, J.A. High Rate Thick Film Growth. Annu. Rev. Mater. Res. 1977, 7, 239–260. [Google Scholar] [CrossRef]
- Jellison, G.E., Jr.; Modine, F.A. Parameterization of the optical functions of amorphous materials in the interband region. Appl. Phys. Lett. 1996, 69, 371. [Google Scholar] [CrossRef]
- Yu, W.; Zhao, J.; Jin, C. Simultaneous softening of Cu3N phonon modes along the T2 line under pressure: A first-principles calculation. Phys. Rev. B 2005, 72, 214116. [Google Scholar] [CrossRef]
- Urbach, F. The Long-Wavelength Edge of Photographic Sensitivity and of the Electronic Absorption of Solids. Phys. Rev. 1953, 92, 1324. [Google Scholar] [CrossRef]
- Cody, G. Urbach edge of crystalline and amorphous silicon: A personal review. J. Non-Cryst. Solids 1992, 141, 3–15. [Google Scholar] [CrossRef]
- Ugur, E.; Ledinský, M.; Allen, T.G.; Holovský, J.; Vlk, A.; De Wolf, S. Life on the Urbach Edge. J. Phys. Chem. Lett. 2022, 13, 7702–7711. [Google Scholar] [CrossRef]
- Figueira, C.; Del Rosario, G.; Pulgliese, D.; Rodriguez-Tapiador, M.; Fernández, S. Effect of Argon on the Properties of Copper Nirtide Fabricated by Magnetron Sputtering for the Next Generation of Solar Absorbers. Materials 2022, 15, 8973. [Google Scholar] [CrossRef] [PubMed]
- Reddy, K.V.S.; Uthanna, S. Effect of sputtering power on the physical properties of Cu3N films ormed by DC magnetron sputtering. Synth. React. Inorg. Met.Org. Nano Met. Chem. 2007, 37, 393–395. [Google Scholar] [CrossRef]
- Wemple, S.; DiDomenico, M., Jr. Optical dispersion and the structure of solids. Phys. Rev. Lett. 1969, 23, 1156. [Google Scholar] [CrossRef]
- Wemple, S.; DiDomenico, M., Jr. Behavior of the electronic dielectric constant in covalent and ionic materials. Phys. Rev. B 1971, 3, 1338. [Google Scholar] [CrossRef]
- Wemple, S. Refractive-index behavior of amorphous semiconductors and glasses. Phys. Rev. B 1973, 7, 3767. [Google Scholar] [CrossRef]
- Wemple, S. Optical oscillator strengths and excitation energies in solids, liquids, and molecules. J. Chem. Phys. 1977, 67, 2151–2168. [Google Scholar] [CrossRef]
- Alyousef, H.A.; Hassan, A.; Zakaly, H.M. Exploring the impact of substrate placement on Cu3N thin films as a solar cell window layer: Structural and optical attributes. Mater. Today Commun. 2023, 35, 106183. [Google Scholar] [CrossRef]
- Tanaka, K. Optical properties and photoinduced changes in amorphous AsS films. Thin Solid Film. 1980, 66, 271–279. [Google Scholar] [CrossRef]
- Borah, D.J.; Mostako, A. Investigation on dispersion parameters of Molybdenum Oxide thin films via Wemple–DiDomenico (WDD) single oscillator model. Appl. Phys. A 2020, 126, 818. [Google Scholar] [CrossRef]




| Sample | N Flux | Ar Flux | Partial N | Deposition | Total Oscillators | Sample | Ellipsometry Sample | Microscopy Sample | Urbach |
|---|---|---|---|---|---|---|---|---|---|
| ID | (sccm) | (sccm) | Pressure | Time (min) | (TL + Gaussian) | Thickness (nm) | Roughness (nm) | Roughness (nm) | Energy (meV) |
| #1360 | 20 | 10 | 0.8 | 60 | 12 (1 + 11) | 430 | 42 | 19 | 96 |
| #1460 | 20 | 0 | 1.0 | 60 | 9 (1 + 8) | 333 | 22 | 7 | 176 |
| #1490 | 20 | 0 | 1.0 | 90 | 7 (1 + 6) | 610 | 46 | 20 | 242 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Márquez, E.; Blanco, E.; Mánuel, J.M.; Ballester, M.; García-Gurrea, M.; Rodríguez-Tapiador, M.I.; Fernández, S.M.; Willomitzer, F.; Katsaggelos, A.K. Mid-Infrared (MIR) Complex Refractive Index Spectra of Polycrystalline Copper-Nitride Films by IR-VASE Ellipsometry and Their FIB-SEM Porosity. Coatings 2024, 14, 5. https://doi.org/10.3390/coatings14010005
Márquez E, Blanco E, Mánuel JM, Ballester M, García-Gurrea M, Rodríguez-Tapiador MI, Fernández SM, Willomitzer F, Katsaggelos AK. Mid-Infrared (MIR) Complex Refractive Index Spectra of Polycrystalline Copper-Nitride Films by IR-VASE Ellipsometry and Their FIB-SEM Porosity. Coatings. 2024; 14(1):5. https://doi.org/10.3390/coatings14010005
Chicago/Turabian StyleMárquez, Emilio, Eduardo Blanco, José M. Mánuel, Manuel Ballester, Marcos García-Gurrea, María I. Rodríguez-Tapiador, Susana M. Fernández, Florian Willomitzer, and Aggelos K. Katsaggelos. 2024. "Mid-Infrared (MIR) Complex Refractive Index Spectra of Polycrystalline Copper-Nitride Films by IR-VASE Ellipsometry and Their FIB-SEM Porosity" Coatings 14, no. 1: 5. https://doi.org/10.3390/coatings14010005
APA StyleMárquez, E., Blanco, E., Mánuel, J. M., Ballester, M., García-Gurrea, M., Rodríguez-Tapiador, M. I., Fernández, S. M., Willomitzer, F., & Katsaggelos, A. K. (2024). Mid-Infrared (MIR) Complex Refractive Index Spectra of Polycrystalline Copper-Nitride Films by IR-VASE Ellipsometry and Their FIB-SEM Porosity. Coatings, 14(1), 5. https://doi.org/10.3390/coatings14010005