A Simulation Study on the Effect of Supersonic Ultrasonic Acoustic Streaming on Solidification Dendrite Growth Behavior During Laser Cladding Based on Boundary Coupling
Abstract
1. Introduction
2. Materials and Methodology
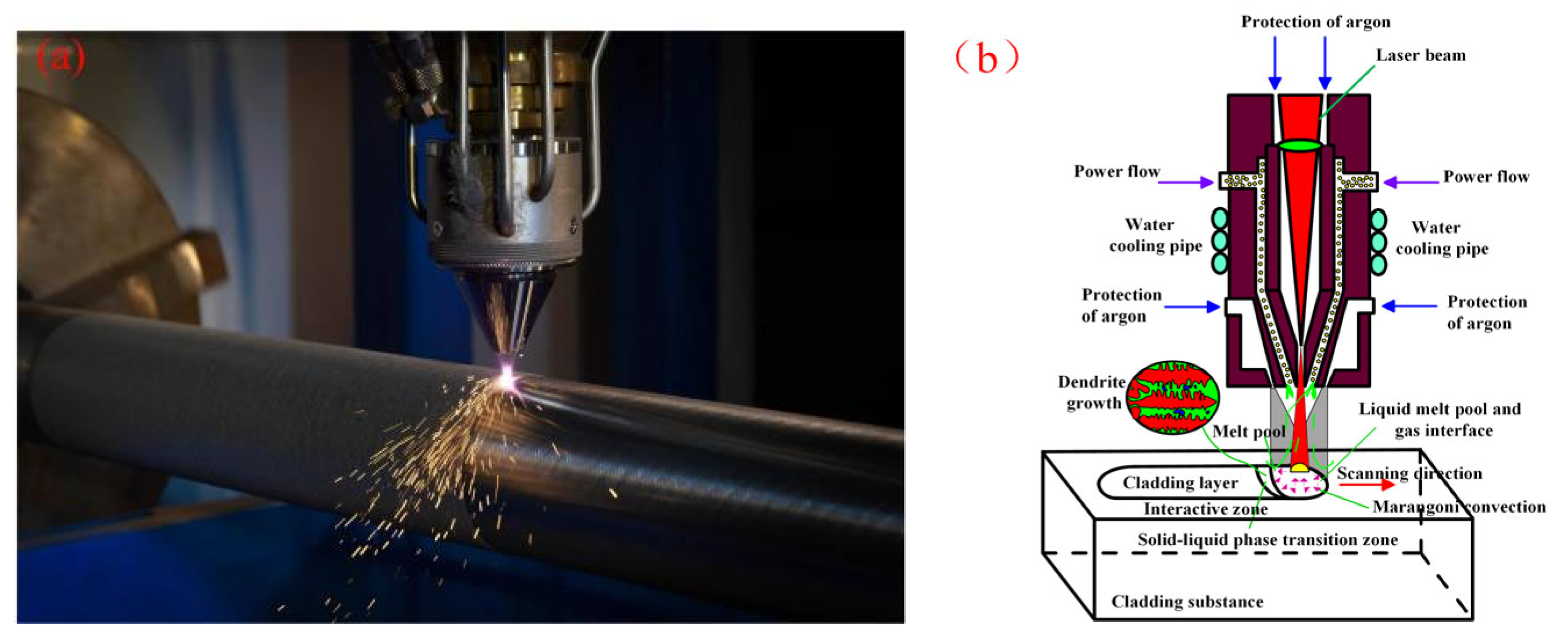
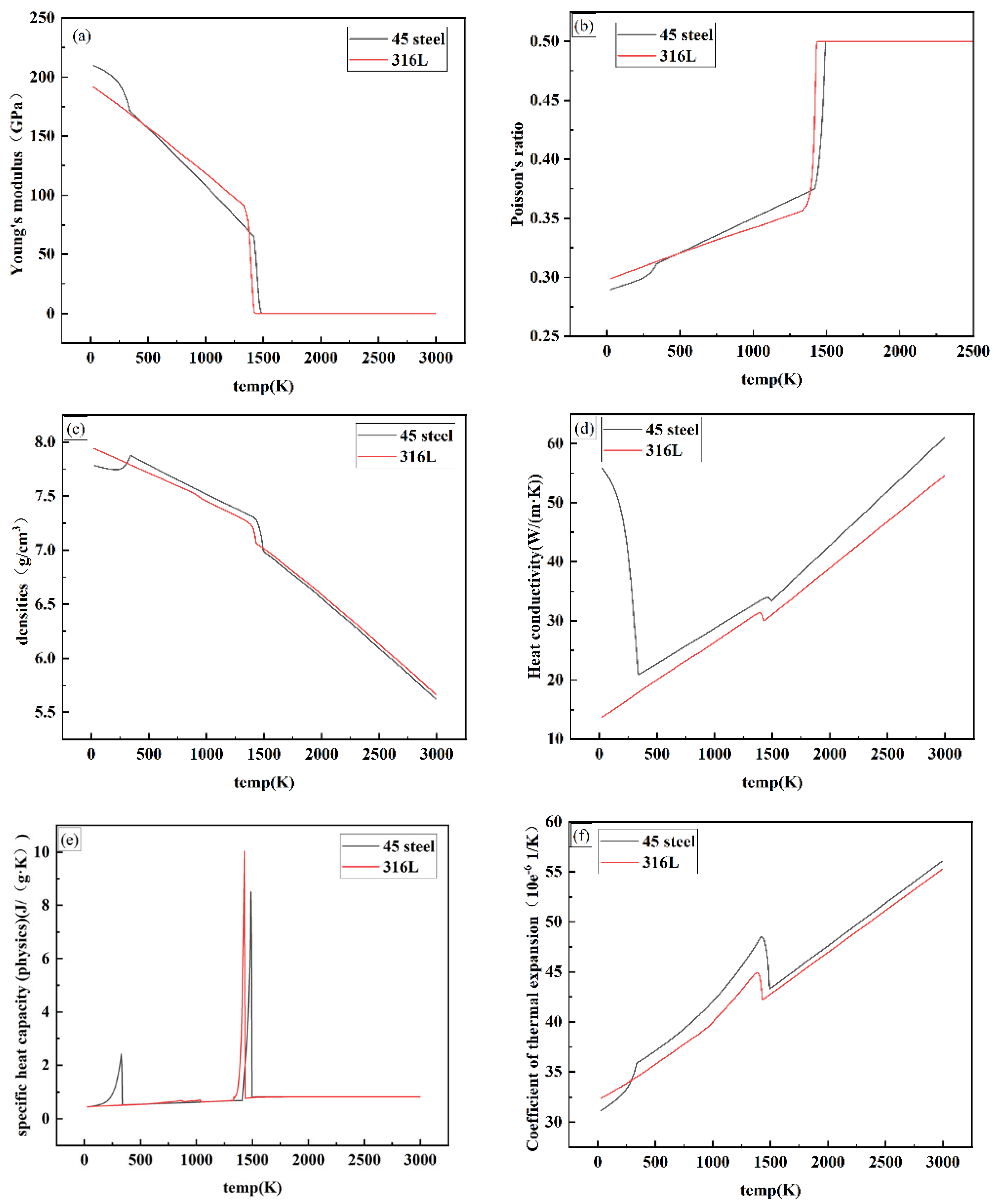
2.1. Material Parameterization for Laser Cladding
2.2. Governing Equation for Laser Cladding
- (1)
- The energy density of the laser heat source acting on the melt has a Gaussian distribution;
- (2)
- The fluid in the melt pool is an incompressible Newtonian fluid;
- (3)
- The melt metal flow in the melt pool is laminar;
- (4)
- The impact caused by momentum when the powder enters the melt pool is ignored;
- (5)
- The loss of heat dissipation caused by the high temperature of the melt is ignored;
- (6)
- The process of powder addition to the substrate follows a Gaussian distribution;
- (7)
- The absorption and reflection of the laser energy of the powder are linearly related to the amount of powder delivery.
2.3. Ultrasonic Vibration Theory
2.4. Acoustic Streaming Effect
2.5. Phase Field-Controlling Equations
2.6. Anisotropy of Interfacial Growth
2.7. Establishment of Quantitative Binary Alloy Phase Field Model
3. Selection for Microscopic Boundary
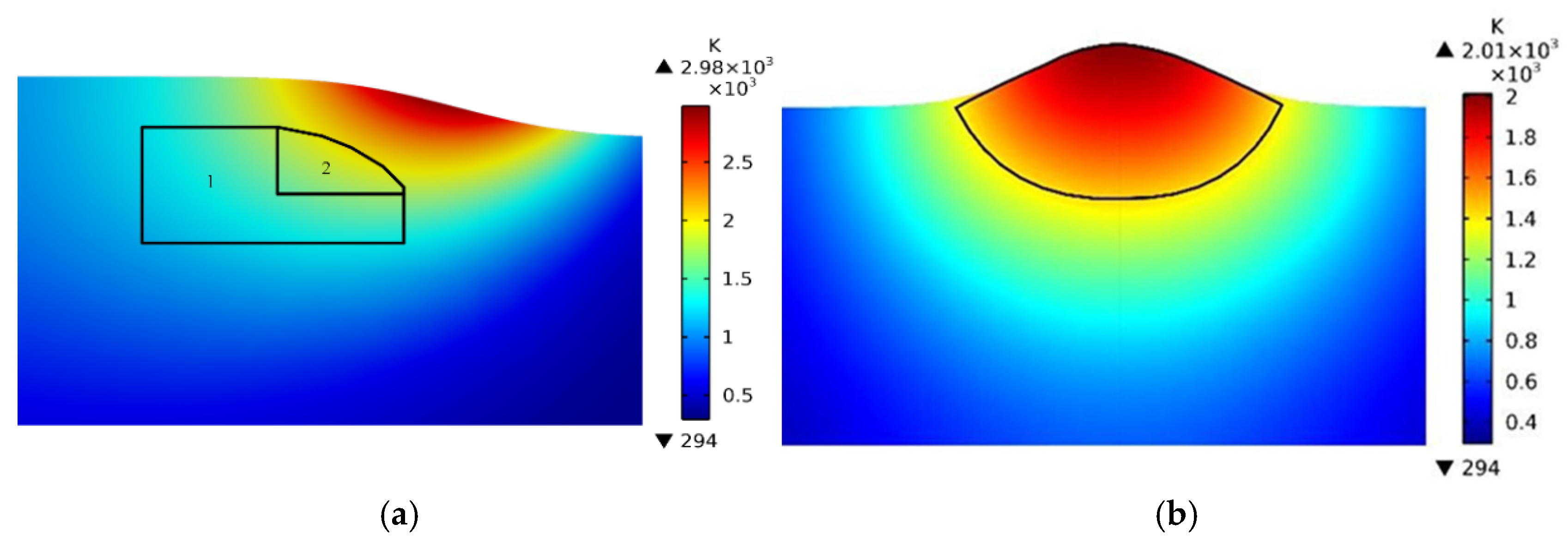
3.1. Temperature Field Boundary Selection
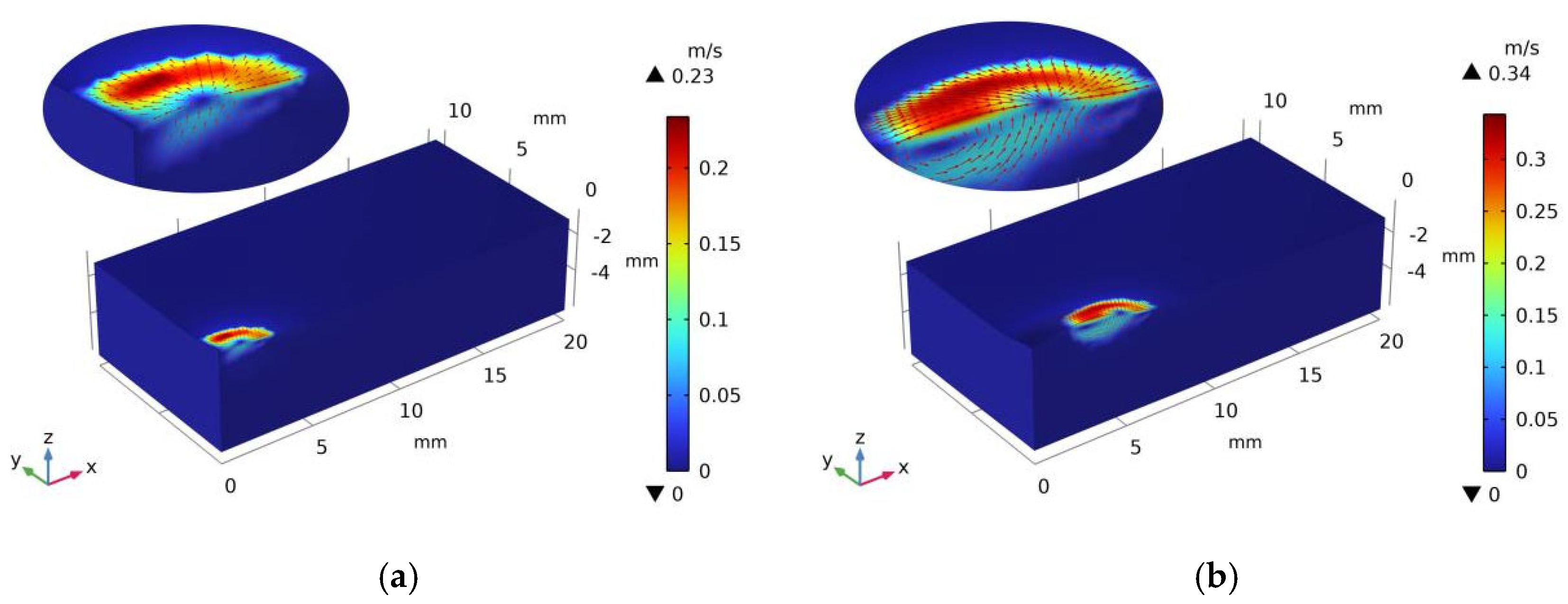
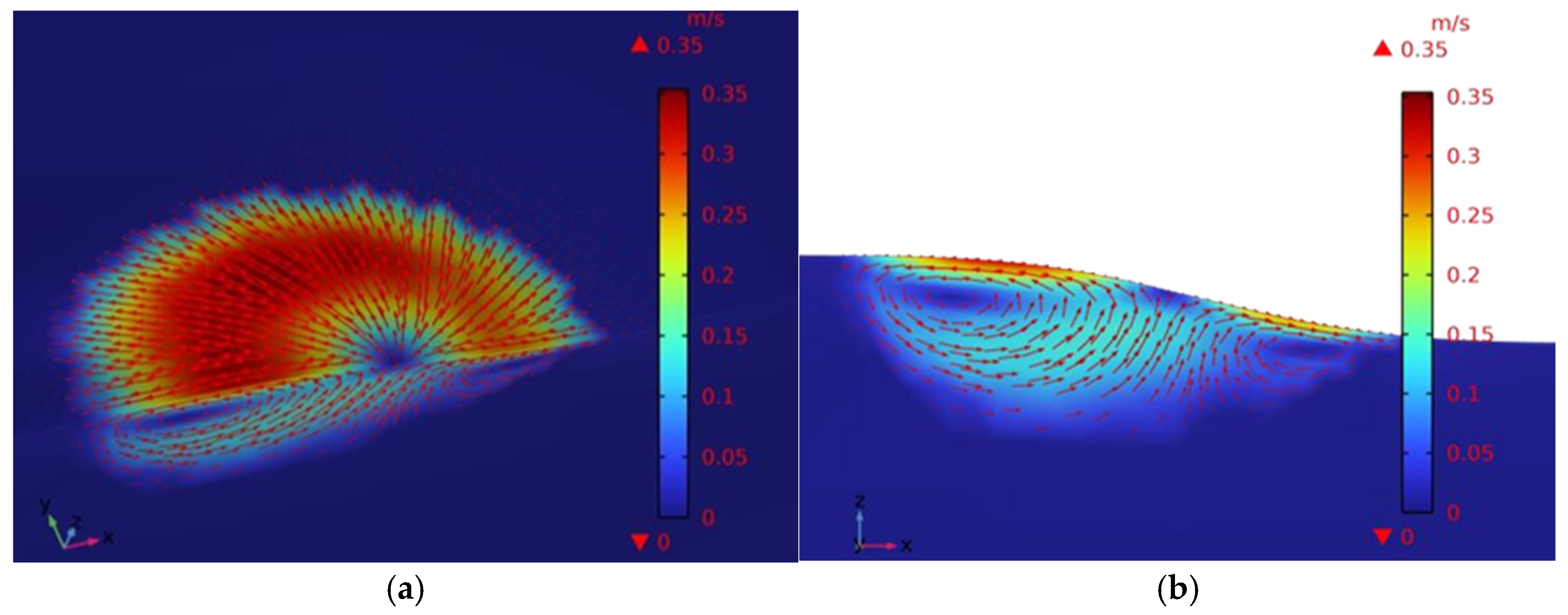
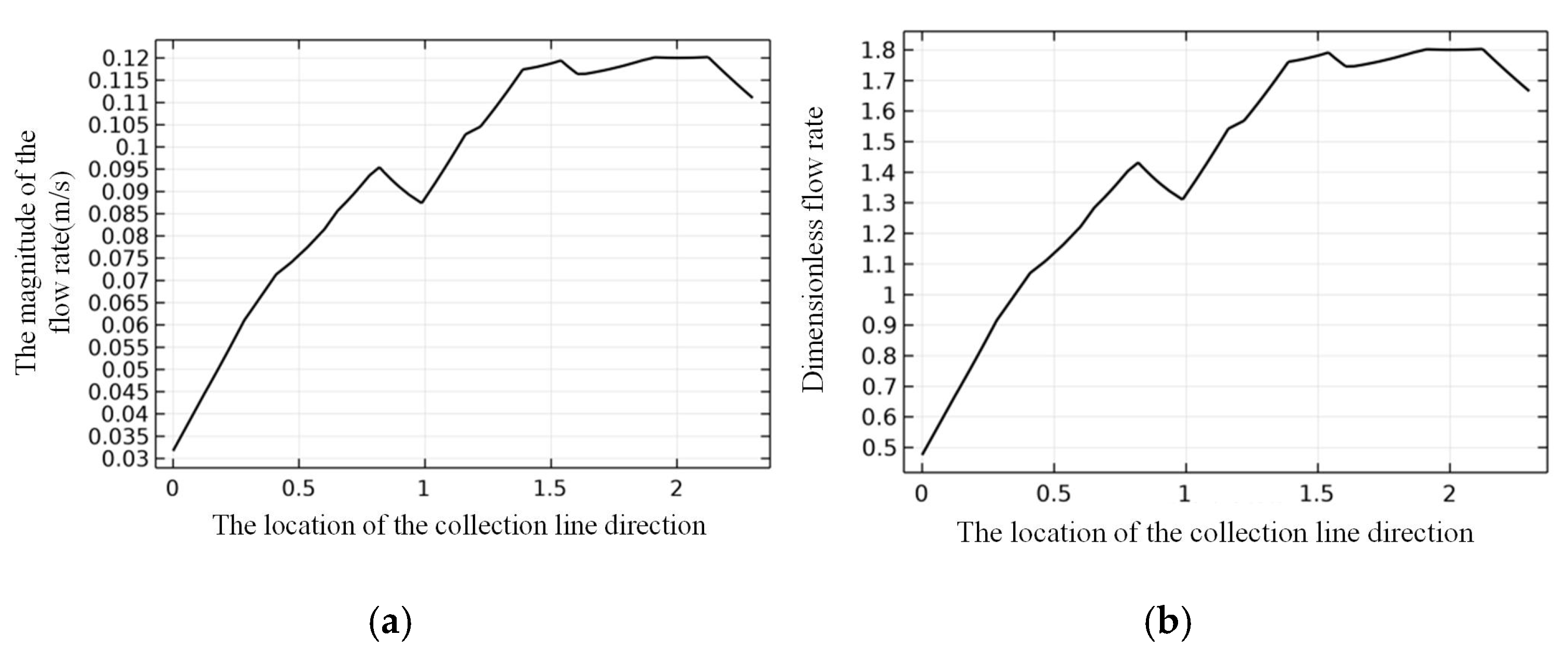
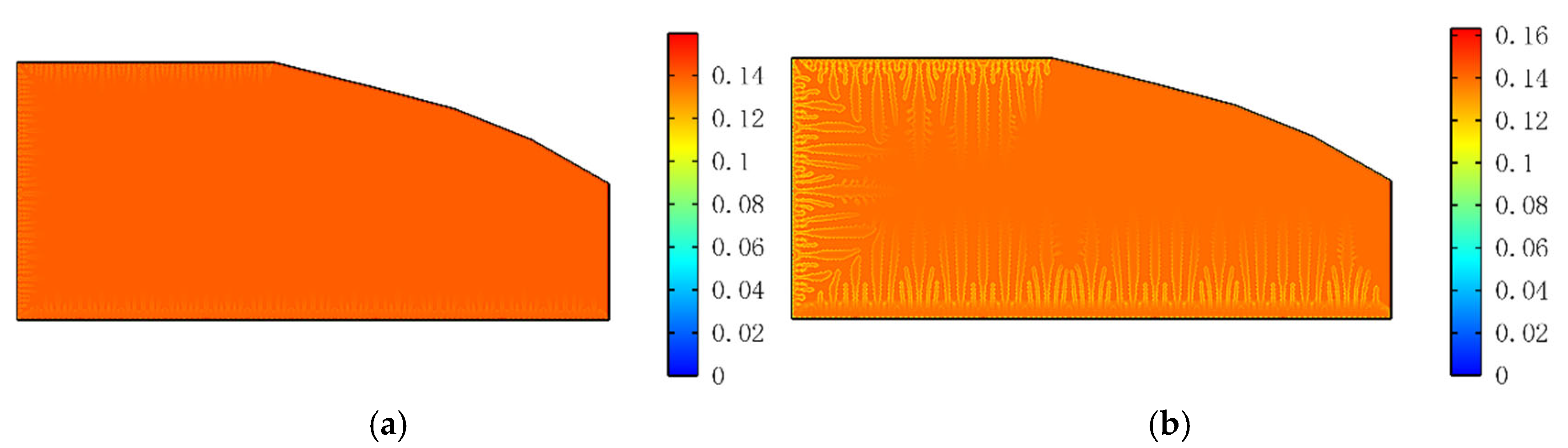
3.2. Flow Field Boundary Extraction Under Silent Flow
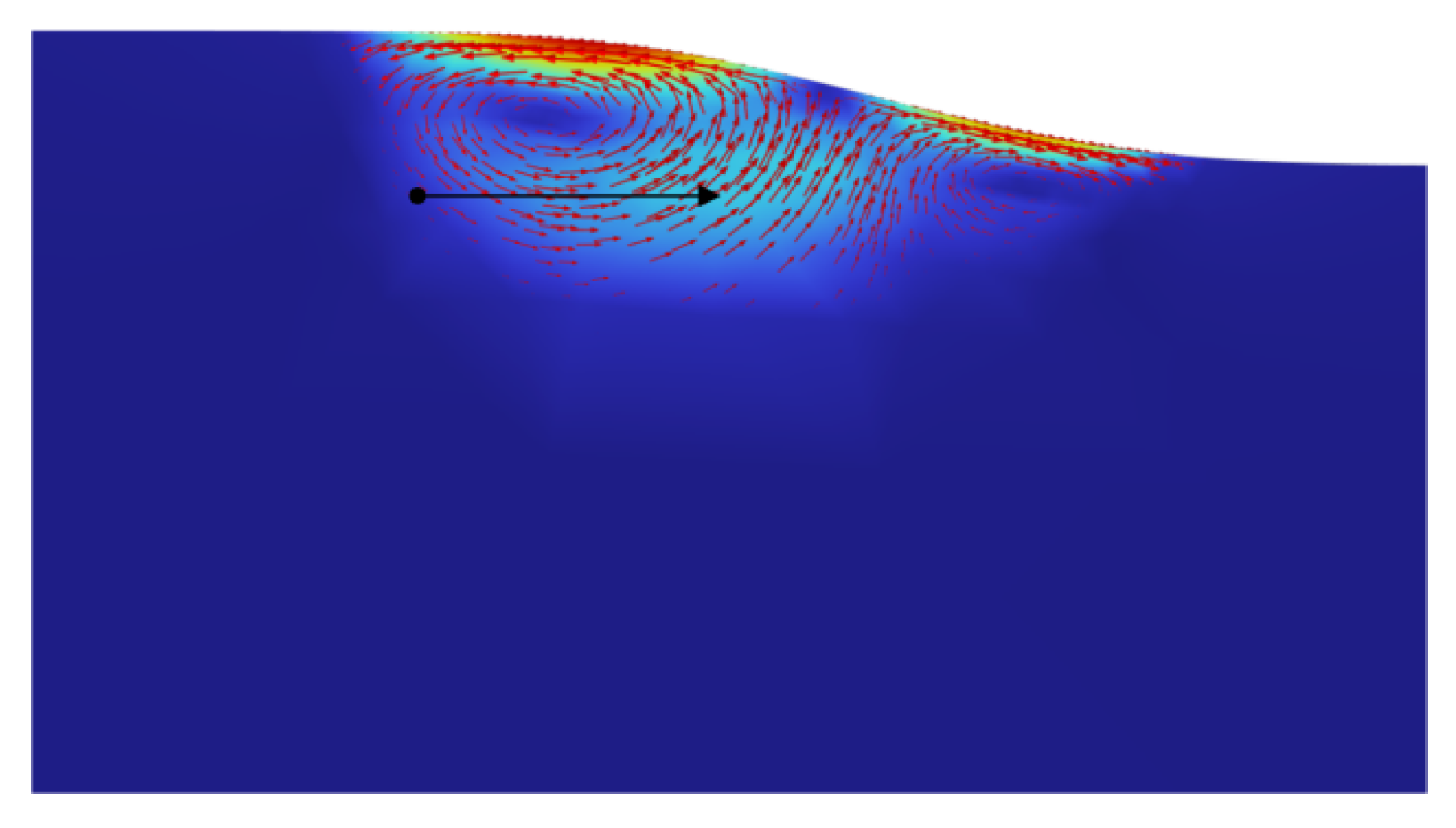
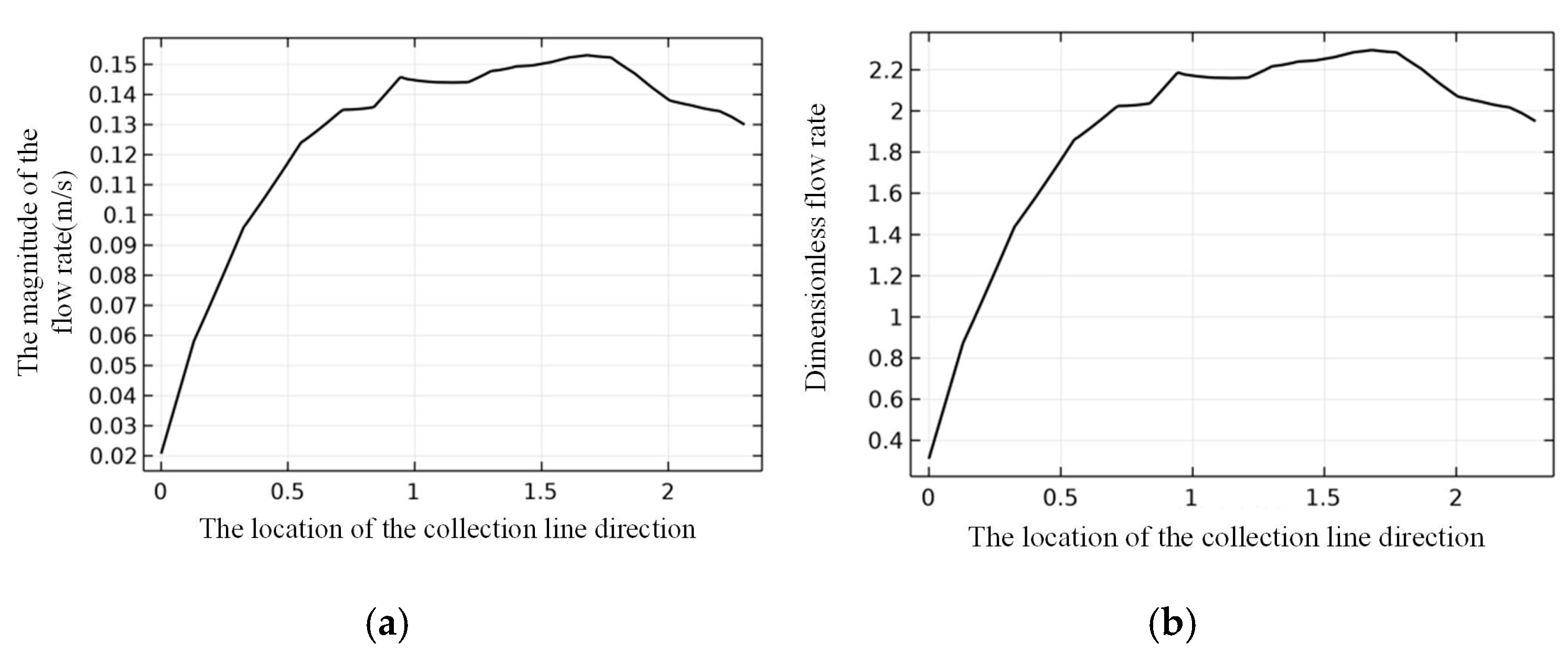
3.3. Flow Field Boundary Extraction Under Acoustic Flow
4. Results and Discussion
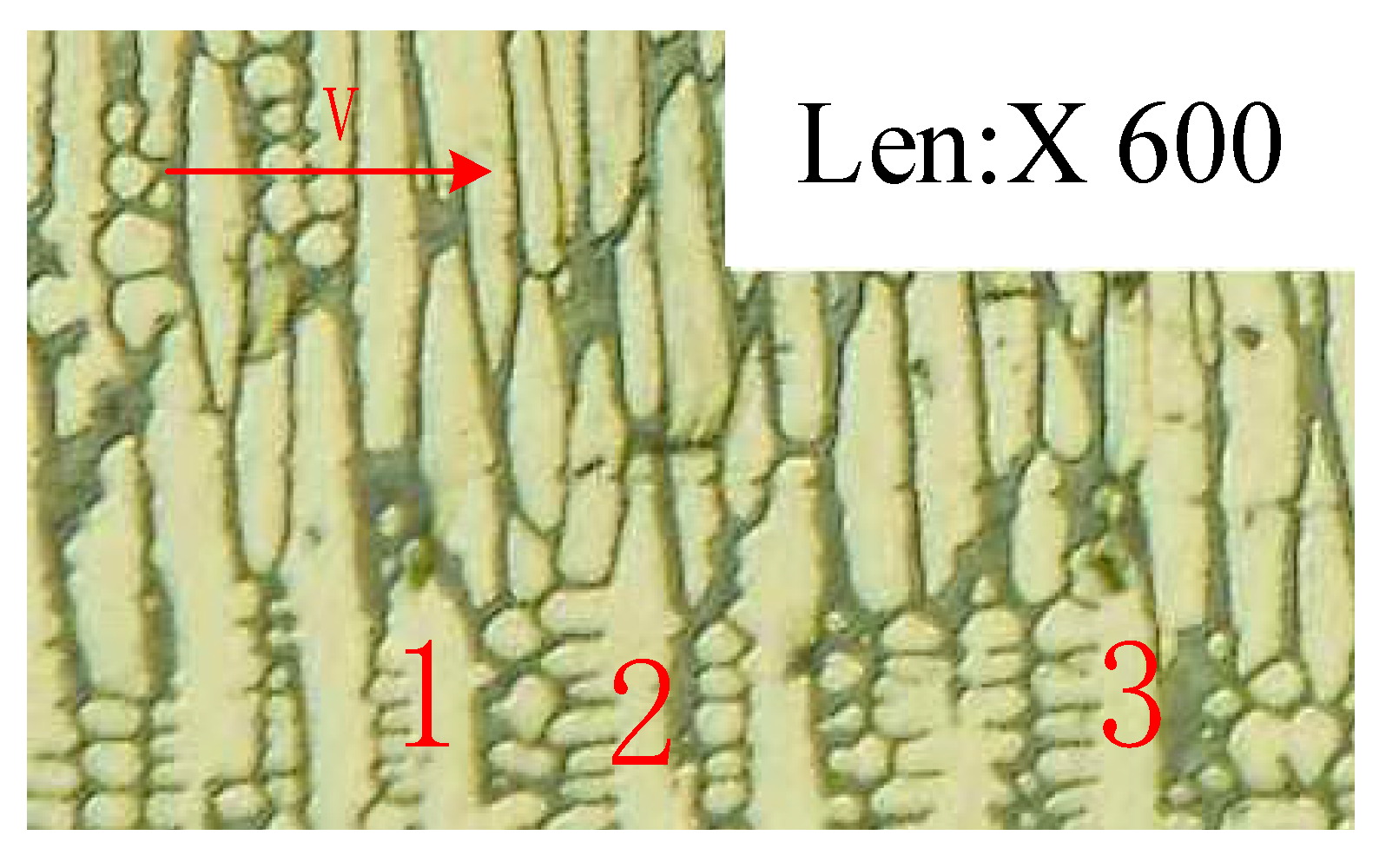
4.1. Dendrite Growth Behavior of Laser Cladding Without Flow Field
4.2. Growth Behavior of Convective Dendrites Under Silent Flow
4.3. Growth Behavior of Convective Dendrites Under Acoustic Flow
5. Conclusions
- (1)
- The numerical simulation results of coupling a static flow field and a sound flow field show that melt flow changes the heat and mass transfer behavior at the solidification interface and also changes the growth morphology of dendrites. The dendrite growth is inhibited under parallel concurrent flow, and the dendrite at the original vertical interface under shear flow inclines to the oncoming side. The dendrite tilt in the flow field is as follows: influenced by convection, the dendrite tilts to the oncoming side, and the tilt angle is small. Due to the influence of convection and the orientation of oncoming dendrites (the dendrites that are inclined to the oncoming side due to competitive growth), the dendrites are inclined to the oncoming side, and the inclination angle is large. Influenced by convection and consequent dendrite orientation (the dendrites that grow inclined to the consequent side due to competitive growth), the dendrites grow inclined to the consequent side, and the inclination angle is large.
- (2)
- Acoustic flow increases the flow velocity of the melt pool, increases the dendrite tilt angle to the onstream side, and promotes the growth of secondary dendrite arms on the onstream side. At the same time, the melt flow is more uniform, the solute distribution of dendrite growth is improved, and the enrichment and segregation of secondary elements between dendrites are reduced.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Valente, C.; Morgado, T.; Sharma, N. LASER cladding—A post processing technique for coating, repair and re-manufacturing. In Materials Forming, Machining and Post Processing; Springer: Berlin/Heidelberg, Germany, 2020; pp. 231–249. [Google Scholar] [CrossRef]
- Zhu, L.; Xue, P.; Lan, Q.; Meng, G.; Ren, Y.; Yang, Z.; Xu, P.; Liu, Z. Recent research and development status of laser cladding: A review. Opt. Laser Technol. 2021, 138, 106915. [Google Scholar] [CrossRef]
- Siddiqui, A.A.; Dubey, A.K. Recent trends in laser cladding and surface alloying. Opt. Laser Technol. 2021, 134, 106619. [Google Scholar] [CrossRef]
- Qi, K.; Yang, Y.; Sun, R.; Hu, G.; Lu, X.; Li, J.; Liang, W.; Jin, K.; Xiong, L. Effect of magnetic field on crack control of Co-based alloy laser cladding. Opt. Laser Technol. 2021, 141, 107129. [Google Scholar] [CrossRef]
- Campbell, J. Effects of vibration during solidification. Int. Met. Rev. 1981, 26, 71–108. [Google Scholar] [CrossRef]
- Ji, F.; Qin, X.; Hu, Z.; Xiong, X.; Ni, M.; Wu, M. Influence of ultrasonic vibration on molten pool behavior and deposition layer forming morphology for wire and arc additive manufacturing. Int. Commun. Heat Mass Transf. 2022, 130, 105789. [Google Scholar] [CrossRef]
- Wei, X.; Li, X.; Zhang, L.; Lv, Q. Effect of in-situ ultrasonic impact treatment on flow and solidification behavior of laser metal deposition: By finite element simulation. Int. J. Heat Mass Transf. 2022, 192, 122914. [Google Scholar] [CrossRef]
- Wang, Z.; Jiang, F.; Guo, C.; Xing, X.; Yang, Z.; Li, H.; Liu, C.; Xu, D.; Jiang, G.; Konovalov, S. Effects of ultrasonic vibration on microstructure and mechanical properties of 1Cr12Ni3MoVN alloy fabricated by directed energy deposition. Ultrasonics 2023, 132, 106989. [Google Scholar] [CrossRef]
- Zhuang, D.-D.; Du, B.; Zhang, S.-H.; Tao, W.-W.; Wang, Q.; Shen, H.-B. Effect and action mechanism of ultrasonic assistance on microstructure and mechanical performance of laser cladding 316L stainless steel coating. Surf. Coat. Technol. 2022, 433, 128122. [Google Scholar] [CrossRef]
- Song, H.; Jiang, F.; Guo, C.; Sun, Q.; Li, H.; Yang, Z.; Li, L.; Diao, M.; Zhang, Z. Effect of ultrasonic vibration on the microstructure and microhardness of laser cladding Fe-based crystalline/amorphous composite coatings. Mater. Lett. 2023, 335, 133780. [Google Scholar] [CrossRef]
- Ma, G.; Yan, S.; Wu, D.; Miao, Q.; Liu, M.; Niu, F. Microstructure evolution and mechanical properties of ultrasonic assisted laser clad yttria stabilized zirconia coating. Ceram. Int. 2017, 43, 9622–9629. [Google Scholar] [CrossRef]
- Geng, R.W.; Du, J.; Wei, Z.Y.; Zhao, G.X. An adaptive-domain-growth method for phase field simulation of dendrite growth in arc preheated fused-coating additive manufacturing. J. Phys. Conf. Ser. 2018, 1063, 012077. [Google Scholar] [CrossRef]
- Wu, L.; Zhang, J. Phase field simulation of dendritic solidification of Ti-6Al-4V during additive manufacturing process. Jom 2018, 70, 2392–2399. [Google Scholar] [CrossRef]
- Zhang, Y.; Jung, Y.-G.; Zhang, J. Phase field modeling of microstructure evolution in selective laser melting-manufactured titanium alloy. In Multiscale Modeling of Additively Manufactured Metals; Elsevier: Amsterdam, The Netherlands, 2020; pp. 141–154. [Google Scholar] [CrossRef]
- Sun, D.; Liu, J.; Li, Q. Phase-field method simulation of microstructure evolution at the bottom of melt pool in coaxial laser cladding. Zhongguo Jiguang (Chin. J. Lasers) 2013, 40, 0403005-6. [Google Scholar] [CrossRef]
- Li, A.; Wang, Z.; Sun, Z. Effect of Ultrasonic Vibration on Microstructure and Fluidity of Aluminum Alloy. Materials 2023, 16, 4110. [Google Scholar] [CrossRef] [PubMed]
- Lian, G.; Yue, K.; Zeng, J.; Feng, M.; Lan, R.; Kong, L. Microstructures and Properties of NbC-Reinforced Ni-Based Coatings Synthesized In Situ by Ultrasonic Vibration-Assisted Laser Cladding. Materials 2023, 16, 1704. [Google Scholar] [CrossRef] [PubMed]
- Lv, J.; Zhou, J.; Zhang, T.; Meng, X.; Li, P.; Huang, S. Microstructure and Wear Properties of IN718/WC Composite Coating Fabricated by Ultrasonic Vibration-Assisted Laser Cladding. Coatings 2022, 12, 412. [Google Scholar] [CrossRef]
- Han, X.; Li, C.; Chen, X.; Jia, S. Numerical simulation and experimental study on the composite process of submerged arc cladding and laser cladding. Surf. Coat. Technol. 2022, 439, 128432. [Google Scholar] [CrossRef]
- Lugscheider, E.; Bolender, H.; Krappitz, H. Laser cladding of paste bound hardfacing alloys. Surf. Eng. 1991, 7, 341–344. [Google Scholar] [CrossRef]
- Fellowes, F.; Steen, W.; Coley, K. Ceramic coatings for high temperature corrosion resistance by laser processing. Key Eng. Mater. 1990, 46, 435–446. [Google Scholar] [CrossRef]
- Zhou, J. Stress Evolution and Control in Laser Deposition Manufacturing; Shenyang University of Aeronautics and Astronautics: Shenyang, China, 2016; pp. 15–20. [Google Scholar]
- Kumar, S.; Wu, C.; Padhy, G.; Ding, W. Application of ultrasonic vibrations in welding and metal processing: A status review. J. Manuf. Process. 2017, 26, 295–322. [Google Scholar] [CrossRef]
- Humphrey, V.F. Ultrasound and matter—Physical interactions. Prog. Biophys. Mol. Biol. 2007, 93, 195–211. [Google Scholar] [CrossRef] [PubMed]
- Yao, Y.; Pan, Y.; Liu, S. Power ultrasound and its applications: A state-of-the-art review. Ultrason. Sonochemistry 2020, 62, 104722. [Google Scholar] [CrossRef] [PubMed]
- Khavari, M.; Priyadarshi, A.; Hurrell, A.; Pericleous, K.; Eskin, D.; Tzanakis, I. Characterization of shock waves in power ultrasound. J. Fluid Mech. 2021, 915, R3. [Google Scholar] [CrossRef]
- Wells, P.N.T. Physics of ultrasound. In Ultrasonic Exposimetry; CRC Press: Boca Raton, FL, USA, 2020; pp. 9–45. [Google Scholar]
- Čáp, I.; Čápová, K.; Smetana, M.; Borik, Š. Mechanical Waves. Electromagn. Acoust. Waves Bioeng. Appl. 2021, 57. [Google Scholar] [CrossRef]
- Li, Y.; Chen, C.; Yi, R. Recent development of ultrasonic brazing. Int. J. Adv. Manuf. Technol. 2021, 114, 27–62. [Google Scholar] [CrossRef]
- Sui, D.; Han, Q. Ultrasound-assisted cast-on method: Obtaining high-quality metallurgical bonds between a bare steel insert and A354 aluminum alloy within a composite casting. J. Mater. Process. Technol. 2023, 311, 117783. [Google Scholar] [CrossRef]
- Sonia, P.; Jain, J.K.; Saxena, K.K. Influence of ultrasonic vibration assistance in manufacturing processes: A Review. Mater. Manuf. Process. 2021, 36, 1451–1475. [Google Scholar] [CrossRef]
- Li, Q.; Jiang, W.; Xu, Y.; Yu, L.; Niu, Y.; Fan, Z. Development of prominent bonding strength in Al/Mg bimetal composites prepared by ultrasonic vibration-assisted compound casting: Effects of ultrasonic powers. J. Mater. Sci. Technol. 2024, 197, 78–93. [Google Scholar] [CrossRef]
- Kardyś, W.; Milewski, A.; Kogut, P.; Kluk, P. Universal ultrasonic generator for welding. Acta Phys. Pol. A 2013, 124, 456–458. [Google Scholar] [CrossRef]
- Faraday, M. On a peculiar class of acoustical figures; and on certain forms assumed by groups of particles upon vibrating elastic surfaces. Abstr. Pap. Print. Philos. Trans. R. Soc. Lond. 1837, 3, 49–51. [Google Scholar] [CrossRef]
- Liu, F. Numerical Study of Acoustic Flow Effects Based on Weakly Compressible Equations; Huazhong University of Science and Technology: Wuhan, China, 2017; Available online: https://kns.cnki.net/kcms2/article/abstract?v=k15566fjT2nTsAZ-sqgee1b4BNxqOltVHx21XJoEUw7bNtCOqYzYSWGERnYH1MGfAoo32d2CVyVqb7TdCmWLtSUN93VzIXQj1lYU5uVmM_Hzi9kZCJlF38DF6folUvNOKTZCHIcld6KhkMLOxU5seiLSTLBDGvQ0_pZHlW84BOl_hRDi7iviXVxPNsbtKYh492Im8E5NtMg=&uniplatform=NZKPT&language=CHS (accessed on 27 September 2024).
- Rayleigh, J.W.S.B. The Theory of Sound; Macmillan: New York, NY, USA, 1896. [Google Scholar]
- Eckart, C. Vortices and streams caused by sound waves. Phys. Rev. 1948, 73, 68. [Google Scholar] [CrossRef]
- Pierce, A.D. Mathematical theory of wave propagation. In Handbook of Acoustics; Springer: Berlin/Heidelberg, Germany, 1998; pp. 21–37. [Google Scholar]
- Schuster, G. Basic Principles of Wave Propagation. 2011. Available online: http://csim.kaust.edu.sa/files/Short.Courses/BasicSeismology.pdf (accessed on 27 September 2024).
- Muller, P.B.; Barnkob, R.; Jensen, M.J.H.; Bruus, H. A numerical study of microparticle acoustophoresis driven by acoustic radiation forces and streaming-induced drag forces. Lab Chip 2012, 12, 4617–4627. [Google Scholar] [CrossRef] [PubMed]
- Qian, Z. Nonlinear Acoustics; Science Press: New York, NY, USA, 2009. [Google Scholar]
- Hou, C. Simulation of NiCu Alloys by Phase Field Method and Study of Related Models; North Central University: Minneapolis, MN, USA, 2016; Available online: https://kns.cnki.net/kcms2/article/abstract?v=k15566fjT2m4VwbB8a1o4IdKakPlMIzF8joXRahBIhL_5XhhSFPkad11WvqKa_FJezOgySqm4QSXJcE79ykPa_At_Ug7gIaCbQ_eP9U7oaWdVF8dLQp3yUzpGDkroGPvsOBwK_1o5SaUJLGIZwa8dHdRoCedj99BYXvDzw2bW6f3wvG-dzzijx1bv8Rye7RoBnBpraSmSh4=&uniplatform=NZKPT&language=CHS (accessed on 27 September 2024).
- Boettinger, W.J.; Warren, J.A.; Beckermann, C.; Karma, A. Phase-field simulation of solidification. Annu. Rev. Mater. Res. 2002, 32, 163–194. [Google Scholar] [CrossRef]
- Beckermann, C.; Diepers, H.-J.; Steinbach, I.; Karma, A.; Tong, X. Modeling melt convection in phase-field simulations of solidification. J. Comput. Phys. 1999, 154, 468–496. [Google Scholar] [CrossRef]
- Alexiades, V. Mathematical Modeling of Melting and Freezing Processes; Routledge: London, UK, 2018. [Google Scholar] [CrossRef]
- Acharya, R.; Sharon, J.A.; Staroselsky, A. Prediction of microstructure in laser powder bed fusion process. Acta Mater. 2017, 124, 360–371. [Google Scholar] [CrossRef]










| Elemental | C | Si | Mn | Cr | Ni | Cu | P | S | Fe |
|---|---|---|---|---|---|---|---|---|---|
| Quantity contained | 0.45 | 0.27 | 0.65 | 0.02 | 0 | 0.01 | 0.016 | 0.007 | Bal |
| Elemental | C | Si | Mn | Cr | Ni | P | S | Mo | Fe |
|---|---|---|---|---|---|---|---|---|---|
| Quantity contained | 0.08 | 1.00 | 2.00 | 16~18 | 13 | 0.045 | 0.002 | 2~3 | Bal |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, X.; Zhan, H.; Li, C.; Wang, X.; Liu, J.; Li, S.; Sun, Q.; Kong, F. A Simulation Study on the Effect of Supersonic Ultrasonic Acoustic Streaming on Solidification Dendrite Growth Behavior During Laser Cladding Based on Boundary Coupling. Coatings 2024, 14, 1381. https://doi.org/10.3390/coatings14111381
Han X, Zhan H, Li C, Wang X, Liu J, Li S, Sun Q, Kong F. A Simulation Study on the Effect of Supersonic Ultrasonic Acoustic Streaming on Solidification Dendrite Growth Behavior During Laser Cladding Based on Boundary Coupling. Coatings. 2024; 14(11):1381. https://doi.org/10.3390/coatings14111381
Chicago/Turabian StyleHan, Xing, Hao Zhan, Chang Li, Xuan Wang, Jiabo Liu, Shuchao Li, Qian Sun, and Fanhong Kong. 2024. "A Simulation Study on the Effect of Supersonic Ultrasonic Acoustic Streaming on Solidification Dendrite Growth Behavior During Laser Cladding Based on Boundary Coupling" Coatings 14, no. 11: 1381. https://doi.org/10.3390/coatings14111381
APA StyleHan, X., Zhan, H., Li, C., Wang, X., Liu, J., Li, S., Sun, Q., & Kong, F. (2024). A Simulation Study on the Effect of Supersonic Ultrasonic Acoustic Streaming on Solidification Dendrite Growth Behavior During Laser Cladding Based on Boundary Coupling. Coatings, 14(11), 1381. https://doi.org/10.3390/coatings14111381






