Is It Better for a Publisher to Release an Audiobook after Its E-Book Version?
Abstract
1. Introduction
2. Literature Review
2.1. Literature Related to Digital Products
2.2. Literature Related to the Book Industry
2.3. Our Contributions to the Related Literature
3. Model Development
3.1. Notation and Assumptions
3.2. Demand Analysis for E-Book and Audiobook in SR Model
3.3. Demand Analysis for E-Book and Audiobook in LR Model
- (1)
- If , there are
- (2)
- If , there are
- (3)
- If , there are:
- (4)
- If , there are
4. Strategy Analysis of Publisher
4.1. Publisher’s Optimal Pricing Strategy under SR Model
4.2. Publisher’s Optimal Pricing Strategy under LR Model
- (1)
- If , there are , , , , ,
- (2)
- If , there are , can be any value in the interval , , , , .
- (1)
- If , there are , can be any value in the interval , , , , ;
- (2)
- If , there are , can be any value in the interval , , , , .
4.3. Publisher’s Optimal Choice
- 1.
- If , releasing a book in its audible and e-book versions simultaneously is the optimal choice of the publisher. The corresponding optimal pricing for the books, the demand for the e-book and the audiobook, and the publisher’s optimal profit are, respectively, as follows:
- 2.
- If , releasing a book in its audible version after its e-book version is the optimal choice of the publisher. The corresponding optimal pricing for the books, the demand for the e-book and the audiobook, and the publisher’s optimal profit are, respectively, as follows:
5. Numerical Analysis
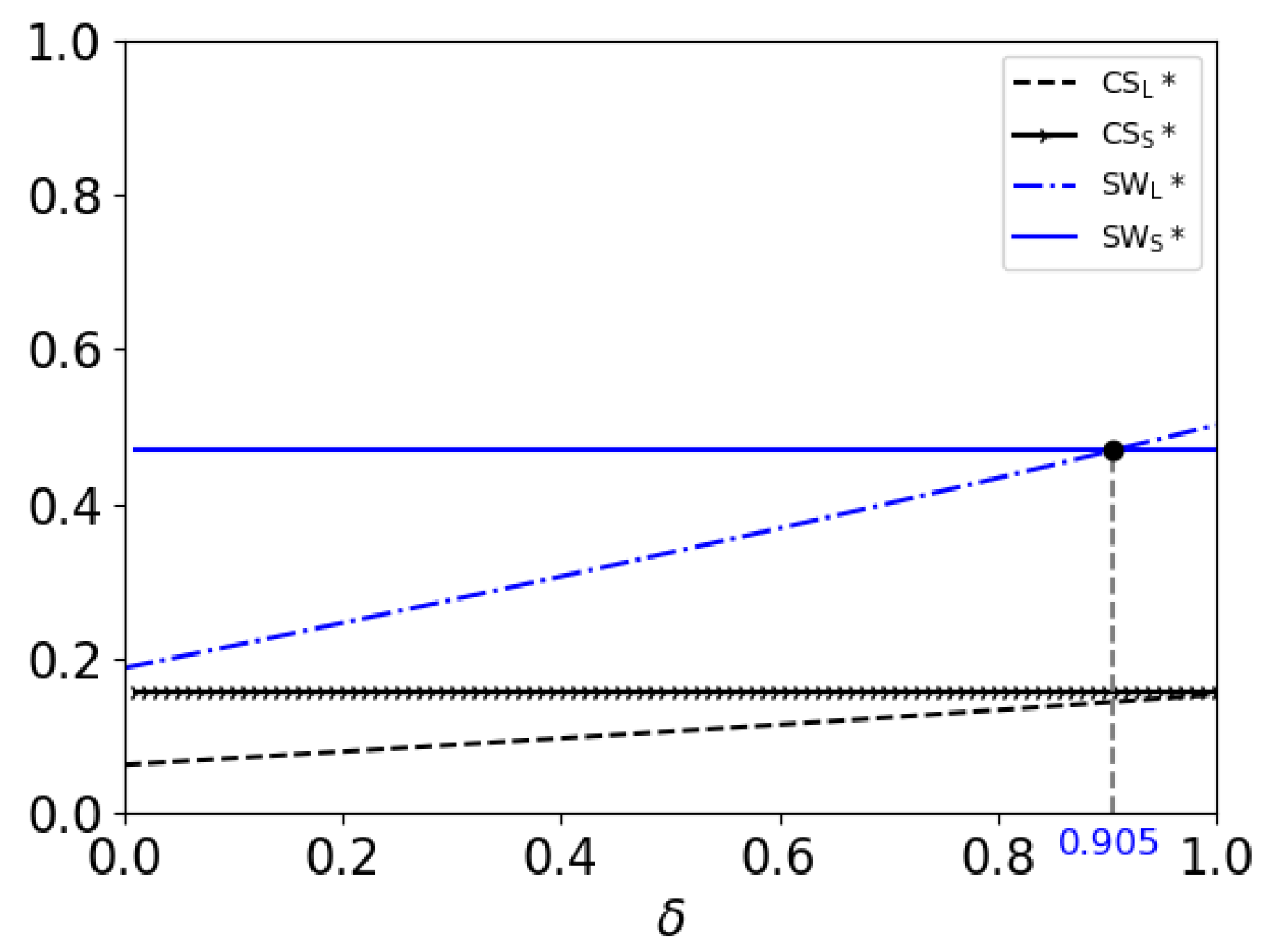
5.1. Effects of Discount Factor
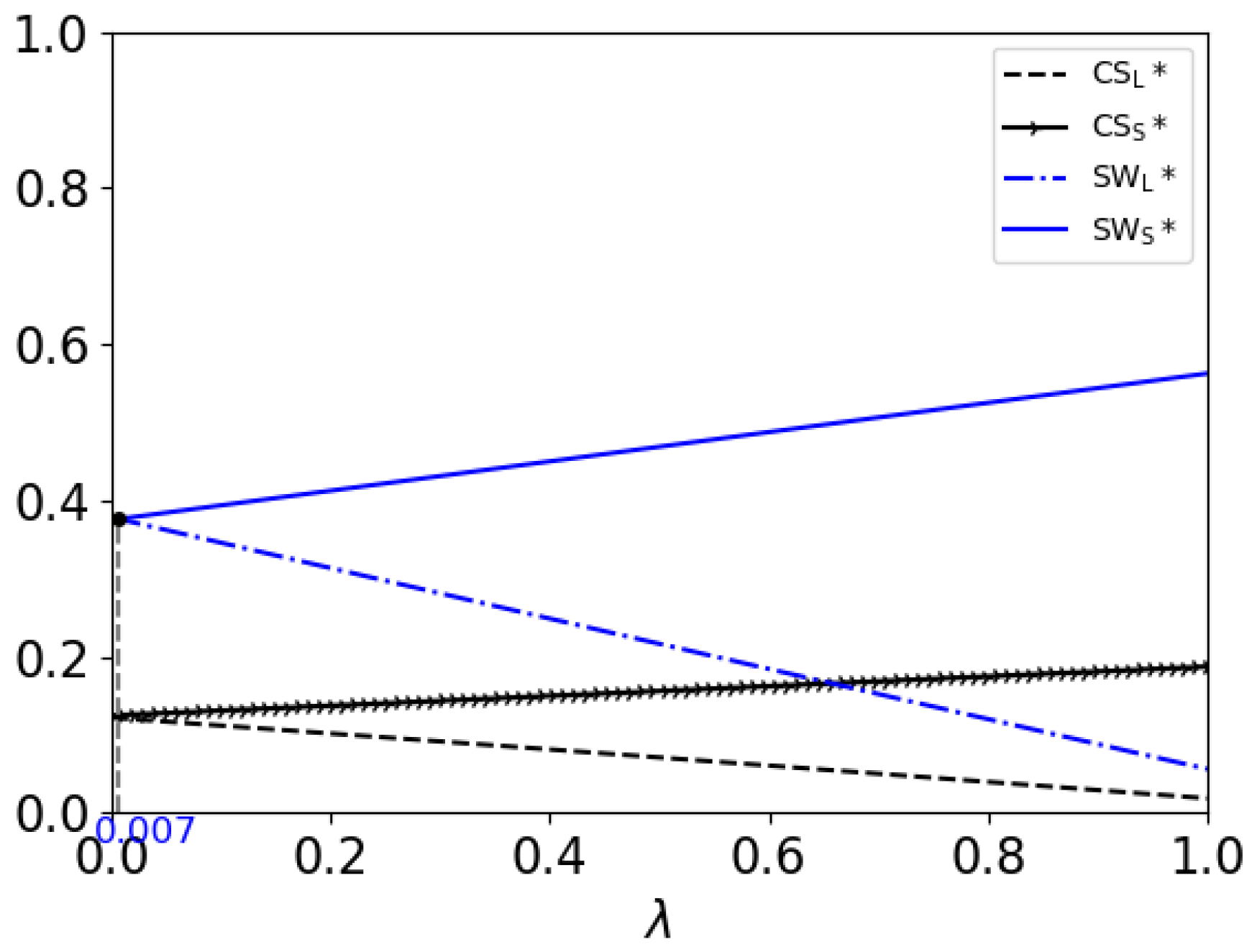
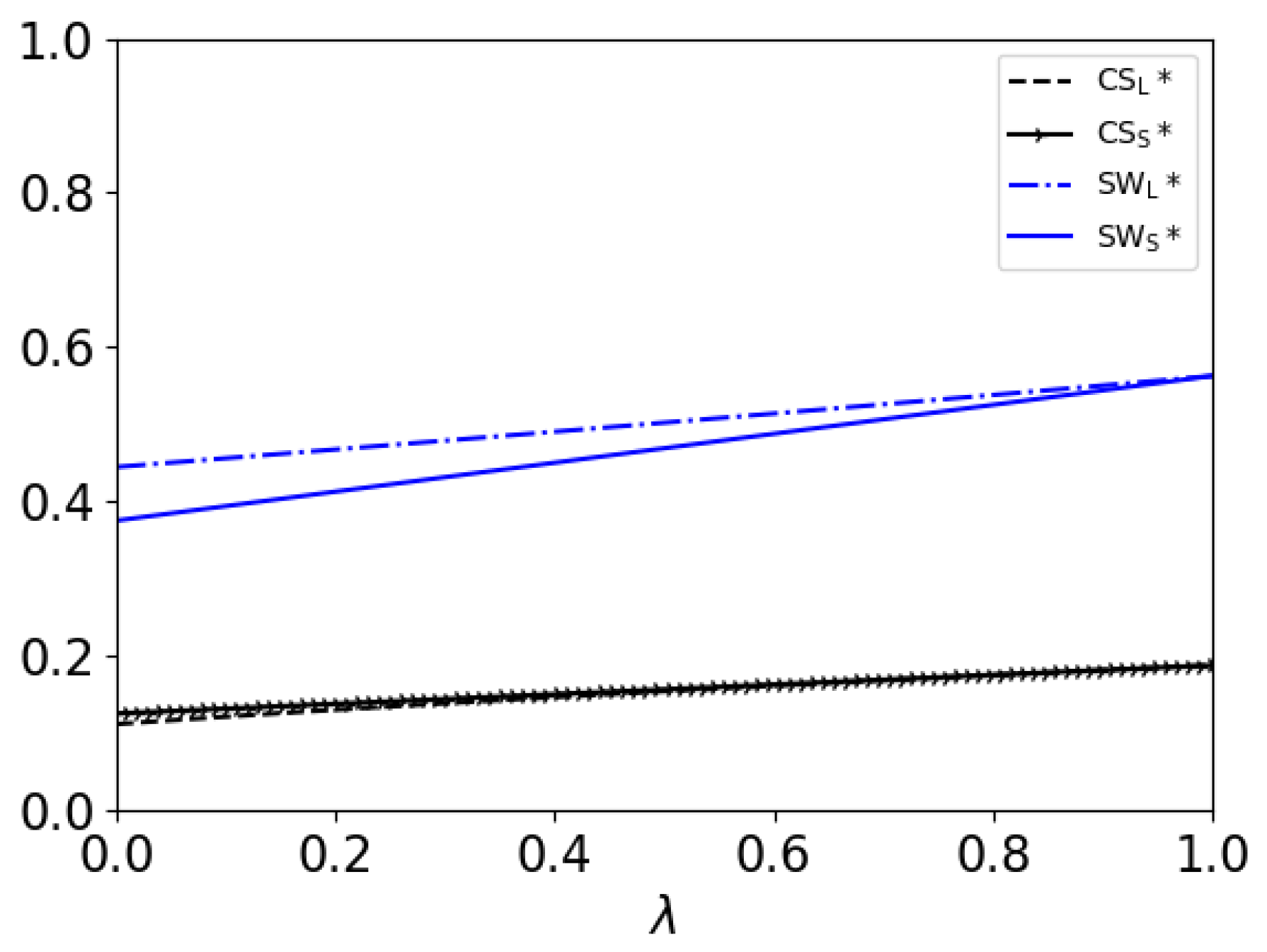
5.2. Effects of Percentage of High-Value Consumers
- 1.
- The consumer surplus in the SR model is higher than that in the LR model. This result is the same as that in Section 5.1.
- 2.
- When , and both decrease with ; when , and both increase with . In addition, and both increase with .This result shows that in the LR model, as the percentage of high-value consumers increases, the consumer surplus and the social welfare will both decrease if the consumers’ patience is not strong enough; but if the consumers have enough patience, both the firm and consumers can benefit. In the SR model, the firm and consumers can both benefit from a higher percentage of high-value consumers.
- 3.
- When , the social welfare in the SR model is higher than that in the LR model if ; otherwise, if , the social welfare in the LR model is higher. When , the social welfare in the SR model is higher than that in the LR model if ; otherwise, if , the social welfare in the LR model is higher. When , the social welfare in the LR model is higher than that in the SR model.From Figure 2, we can see that in the LR model, the patience of the consumers helps to improve the social welfare. But, the percentage of high-value consumers hurts the social welfare when the patience of the consumers is not strong enough. Thus, as the patience of the consumers increases, the condition for the percentage of high-value consumers, under which the social welfare is higher in the LR model, will be less strict.
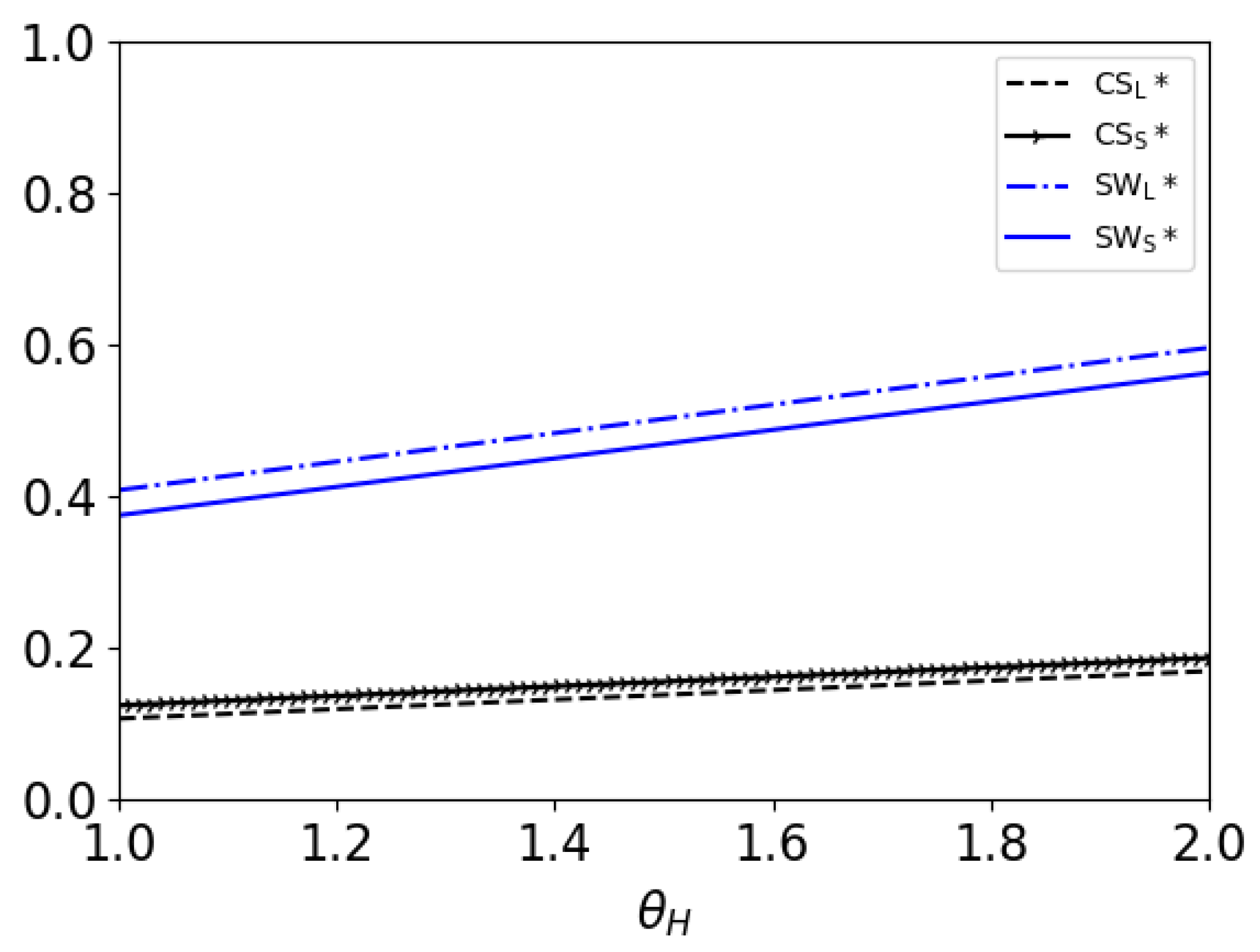
5.3. Effects of High-Value Consumers’ Acceptance Level of Audiobook
- 1.
- and both increase with . As the above analysis shows, a higher acceptance level results in a higher price of the audiobook, thus the publisher can obtain more profit from the audiobook. Therefore, he can obtain more total profits.
- 2.
- When , ; when , . From the above analysis, it can be seen that a stronger patience of the consumers helps the monopolist to choose the LR model. When the consumers are less patient, the monopolist prefers the SR model. But when the patience of the consumers is strong enough, the monopolist prefers the LR model. This result agrees with Theorem 3.
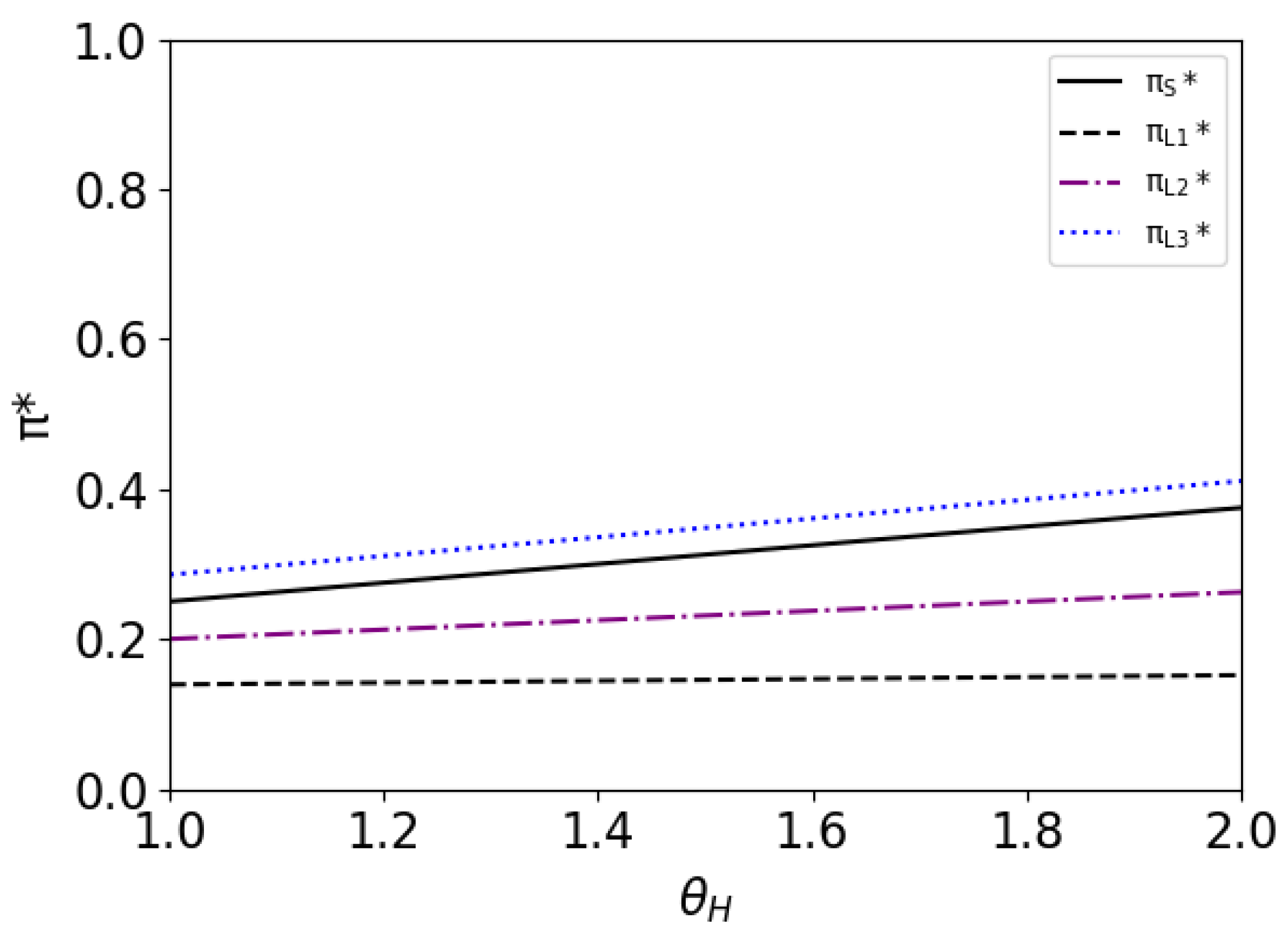
- 1.
- The consumer surplus in the SR model is higher than that in the LR model. This result is the same as those in Section 5.1 and Section 5.2. This means that no matter how these three factors () change, this result always holds.
- 2.
- and , and all increase with .This result shows that as the acceptance level of high-value consumers increases, the consumers can obtain more surplus. Further, as shown in Theorem 2, the publisher’s profit will also rise with the acceptance level. Thus, a higher acceptance level will result in higher social welfare.
- 3.
- When , the social welfare in the SR model is higher than that in the LR model; when , the social welfare in the LR model is higher than that in the SR model.
6. Discussion
7. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of Demand for the E-Book and the Audiobook under the SR Model
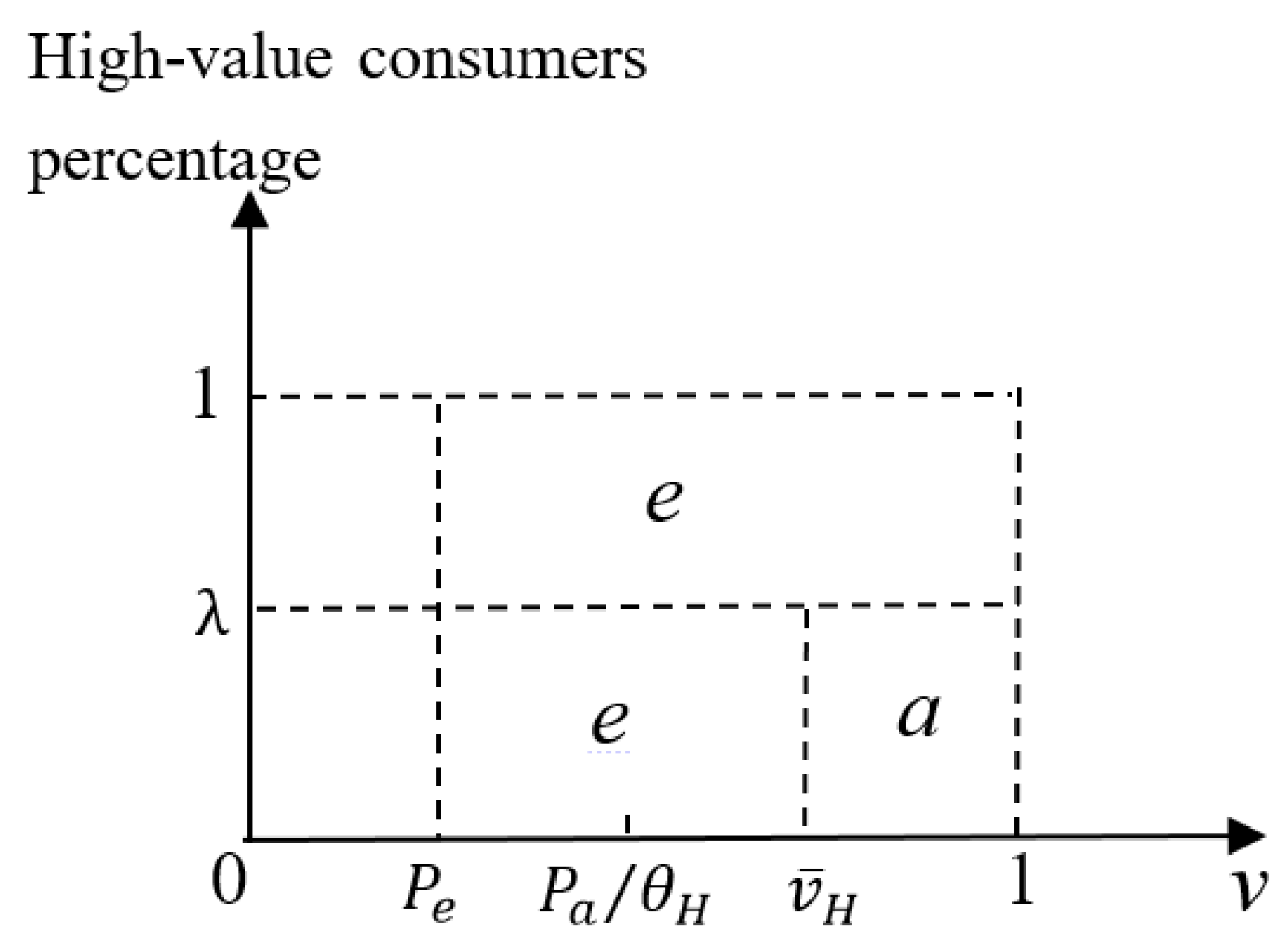
Appendix B. Derivation of Demand for the E-Book and the Audiobook in the Second Stage under LR Model
Appendix C
Appendix D
Appendix E
Appendix F
Appendix G
Appendix H
Appendix I
References
- Audio Publishers Association. Audiobook Publishers Report Tenth Straight Year of Double-Digit Growth. 2022. Available online: https://www.audiopub.org/uploads/pdf/Sales-and-Consumer-Surveys-Press-Release-2022.pdf (accessed on 16 January 2024).
- IBISWorld. E-Book Publishing Industry in the US -Market Research Report. 2022. Available online: https://www.ibisworld.com/united-states/market-research-reports/e-book-publishing-industry/ (accessed on 16 January 2024).
- Chen, Y.N.; Png, I. Information Goods Pricing and Copyright Enforcement: Welfare Analysis. Inf. Syst. Res. 2003, 14, 107–123. [Google Scholar] [CrossRef]
- Sundararajan, A. Managing Digital Piracy: Pricing and Protection. Inf. Syst. Res. 2004, 15, 287–308. [Google Scholar] [CrossRef]
- Jain, S. Digital Piracy: A Competitive Analysis. Mark. Sci. 2008, 27, 610–626. [Google Scholar]
- Khouja, M.; Rajagopalan, H.K. Can Piracy Lead to Higher Prices in the Music and Motion Picture Industries? J. Oper. Res. Soc. 2009, 60, 372–383. [Google Scholar]
- Kogan, K.; Ozinci, Y.; Perlman, Y. Containing Piracy with Product Pricing, Updating and Protection Investments. Int. J. Prod. Econ. 2013, 144, 468–478. [Google Scholar] [CrossRef]
- Wu, D.; Nan, G.; Li, M. Optimal Software Upgrade Strategy: Should We Sell Products or Premium Services in the Presence of Piracy? Electron. Commer. Res. Appl. 2018, 28, 219–229. [Google Scholar]
- Aversa, P.; Hervas-Drane, A.; Evenou, M. Business Model Responses to Digital Piracy. Calif. Manag. Rev. 2019, 61, 30–58. [Google Scholar] [CrossRef]
- Zhang, L.; Fan, L.; Peng, H.; Zhang, Y. Optimal Piracy Control and Pricing Strategies Considering Quality Degradation: The Effects of Policy Instruments. Commer. Res. Appl. 2021, 48, 1–14. [Google Scholar]
- Chai, Y.X.; Ren, J.F.; Zhang, J.Q. The Impacts of Digital Content Piracy and Copyright Protection Policies when Consumers are Loss Averse. J. Ind. Manag. Optim. 2022, 18, 3587–3612. [Google Scholar]
- Jin, C.; Wu, C.A.; Lahiri, A. Piracy and Bundling of Information Goods. J. Manag. Inf. Syst. 2022, 39, 906–933. [Google Scholar]
- Kim, B.; Park, S.E.; Straub, D.W. Pay-What-You-Want Pricing in the Digital Product Marketplace: A Feasible Alternative to Piracy Prevention? Inf. Syst. Res. 2022, 33, 784–793. [Google Scholar] [CrossRef]
- Serenko, A. Antecedents and Consequences of Explicit and Implicit Attitudes toward Digital Piracy. Inf. Manag. 2022, 59, 1–16. [Google Scholar]
- Sundararajan, A. Nonlinear Pricing of Information Goods. Manag. Sci. 2004, 50, 1660–1673. [Google Scholar]
- Yu, A.; Hu, Y.; Fan, M. Pricing Strategies for Tied Digital Contents and Devices. Decis. Support Syst. 2011, 51, 405–412. [Google Scholar]
- Li, M.; Feng, H.; Chen, F. Optimal Versioning and Pricing of Information Products with Considering or not Common Valuation of Customers. Comput. Ind. Eng. 2012, 63, 173–183. [Google Scholar] [CrossRef]
- Zhang, Z.; Nan, G.; Li, M.; Tan, Y. Duopoly Pricing Strategy for Information Products with Premium Service: Free Product or Bundling? J. Manag. Inf. Syst. 2016, 33, 260–295. [Google Scholar] [CrossRef]
- Dou, Y.; Hu, Y.J.; Wu, D.J. Selling or Leasing? Pricing Information Goods with Depreciation of Consumer Valuation. Inf. Syst. Res. 2017, 28, 582–602. [Google Scholar] [CrossRef]
- Zhang, L. Protection in DRM and Pricing Strategies in a Two-echelon Digital Product Supply Chain. Int. J. Syst. Sci. Oper. Logist. 2019, 6, 356–367. [Google Scholar] [CrossRef]
- Cheng, H.K.; Li, S.; Liu, Y. Optimal Software Free Trial Strategy: Limited Version, Time-locked, or Hybrid? Prod. Oper. Manag. 2015, 24, 504–517. [Google Scholar] [CrossRef]
- Xu, B.; Yao, Z.; Tang, P. Pricing Strategies for Information Products with Network Effects and Complementary Services in a Duopolistic Market. Int. J. Prod. Res. 2018, 56, 4243–4263. [Google Scholar]
- Catapano, R.; Shennib, F.; Levav, J. Preference Reversals Between Digital and Physical Goods. J. Mark. Res. 2022, 59, 353–373. [Google Scholar] [CrossRef]
- Maxim, A.; Maxim, A. The Role of E-books in Reshaping the Publishing Industry. Procedia Soc. Behav. Sci. 2012, 62, 1046–1050. [Google Scholar]
- Bounie, D.; Eang, B.; Sirbu, M.; Waelbroeck, P. Superstars and Outsiders in Online Markets: An Empirical Analysis of Books. Electron. Commer. Res. Appl. 2013, 12, 52–59. [Google Scholar] [CrossRef]
- Gilbert, R.J. E-books: A Tale of Digital Disruption. J. Econ. Perspect. 2015, 29, 165–184. [Google Scholar] [CrossRef]
- Luo, J.; Zhang, H.; Li, H. Pricing Strategies in Online Book Industry: A Comparative Study. Inf. Syst. E-Bus. Manag. 2018, 16, 791–816. [Google Scholar] [CrossRef]
- Chen, H.; Hu, Y.J.; Smith, M.D. The Impact of E-book Distribution on Print Sales: Analysis of a Natural Experiment. Manag. Sci. 2019, 65, 19–31. [Google Scholar] [CrossRef]
- Li, H. Intertemporal Price Discrimination with Complementary Products: E-Books and E-Readers. Manag. Sci. 2019, 65, 2665–2694. [Google Scholar] [CrossRef]
- Srivastava, N.; Mishra, A.; Dwivedi, Y.K. Investigating Antecedents of Adoption Intention for Audiobook Applications. J. Comput. Inf. Syst. 2022, 62, 1–13. [Google Scholar] [CrossRef]
- Hao, L.; Fan, M. An Analysis of Pricing Models in the Electronic Book Market. MIS Q. 2014, 38, 1017–1032. [Google Scholar] [CrossRef]
- Tan, Y.; Carrillo, J.E. Strategic Analysis of the Agency Model for Digital Goods. Prod. Oper. Manag. 2017, 26, 724–741. [Google Scholar] [CrossRef]
- Johnson, J.P. The Agency Model and MFN Clauses. Rev. Econ. Stud. 2017, 84, 1–47. [Google Scholar] [CrossRef]
- Lu, Q.; Shi, V.; Huang, J. Who Benefit from Agency Model: A Strategic Analysis of Pricing Models in Distribution Channels of Physical Books and E-books. Eur. J. Oper. Res. 2018, 264, 1074–1091. [Google Scholar]
- Zhang, L.; Fan, L. Strategic Analysis of E-book Pricing Models in the Presence of Network Externalities. Eur. J. Int. Manag. 2024, 22, 80–103. [Google Scholar] [CrossRef]
- Zhang, W. Game Theory and Information Economics; Shanghai People’s Publishing House: Shanghai, China, 2006. [Google Scholar]
- Myatt, D.P.; Wallace, C. Cournot Competition and the Social Value of Information. J. Econ. Theory 2015, 158, 466–506. [Google Scholar] [CrossRef]
- Goldsticker, R.P.; Agrrawal, P. The Effects of Blending Primary and Diluted Eps Data. Financ. Anal. J. 1999, 55, 51–60. [Google Scholar]





| Consumer’s valuation of the e-book. | |
| High-value consumers’ acceptable level for the audiobook. | |
| Low-value consumers’ acceptable level for the audiobook. | |
| Percentage of high-value consumers. | |
| The model where publisher releases the audiobook and the e-book simultaneously. | |
| The model where publisher releases the audiobook after the e-book. | |
| Retail price of the e-book under the SR model. | |
| Retail price of the e-book in stage i under the LR model, . | |
| Retail price of the audiobook. | |
| Consumer’s utility if she buys the e-book under the SR model. | |
| Low-value consumer’s utility if she buys the audiobook. | |
| High-value consumer’s utility if she buys the audiobook. | |
| Consumer’s utility if she buys the e-book in stage i under the LR model, . | |
| Discount factor of the e-book under the LR model. | |
| Valuation threshold of high-value consumer who is indifferent between buying the audiobook and the e-book under SR model. | |
| Valuation threshold of low-value consumer who is indifferent between buying the audiobook and the e-book under the SR model. | |
| Valuation threshold of high-value consumer who is indifferent between buying the audiobook and the e-book in the second stage under LR model. | |
| Valuation threshold of low-value consumer who is indifferent between buying the audiobook and the e-book in the second stage under the LR model. | |
| Demand for the e-book under the SR model. | |
| Demand for the e-book in stage i under the LR model, . | |
| Demand for the audiobook. | |
| Total profit of the publisher. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L. Is It Better for a Publisher to Release an Audiobook after Its E-Book Version? Systems 2024, 12, 33. https://doi.org/10.3390/systems12010033
Zhang L. Is It Better for a Publisher to Release an Audiobook after Its E-Book Version? Systems. 2024; 12(1):33. https://doi.org/10.3390/systems12010033
Chicago/Turabian StyleZhang, Linlan. 2024. "Is It Better for a Publisher to Release an Audiobook after Its E-Book Version?" Systems 12, no. 1: 33. https://doi.org/10.3390/systems12010033
APA StyleZhang, L. (2024). Is It Better for a Publisher to Release an Audiobook after Its E-Book Version? Systems, 12(1), 33. https://doi.org/10.3390/systems12010033






