A Sustainable Energy Distribution Configuration for Microgrids Integrated to the National Grid Using Back-to-Back Converters in a Renewable Power System
Abstract
:1. Introduction
2. Materials and Methods
2.1. Microgrid
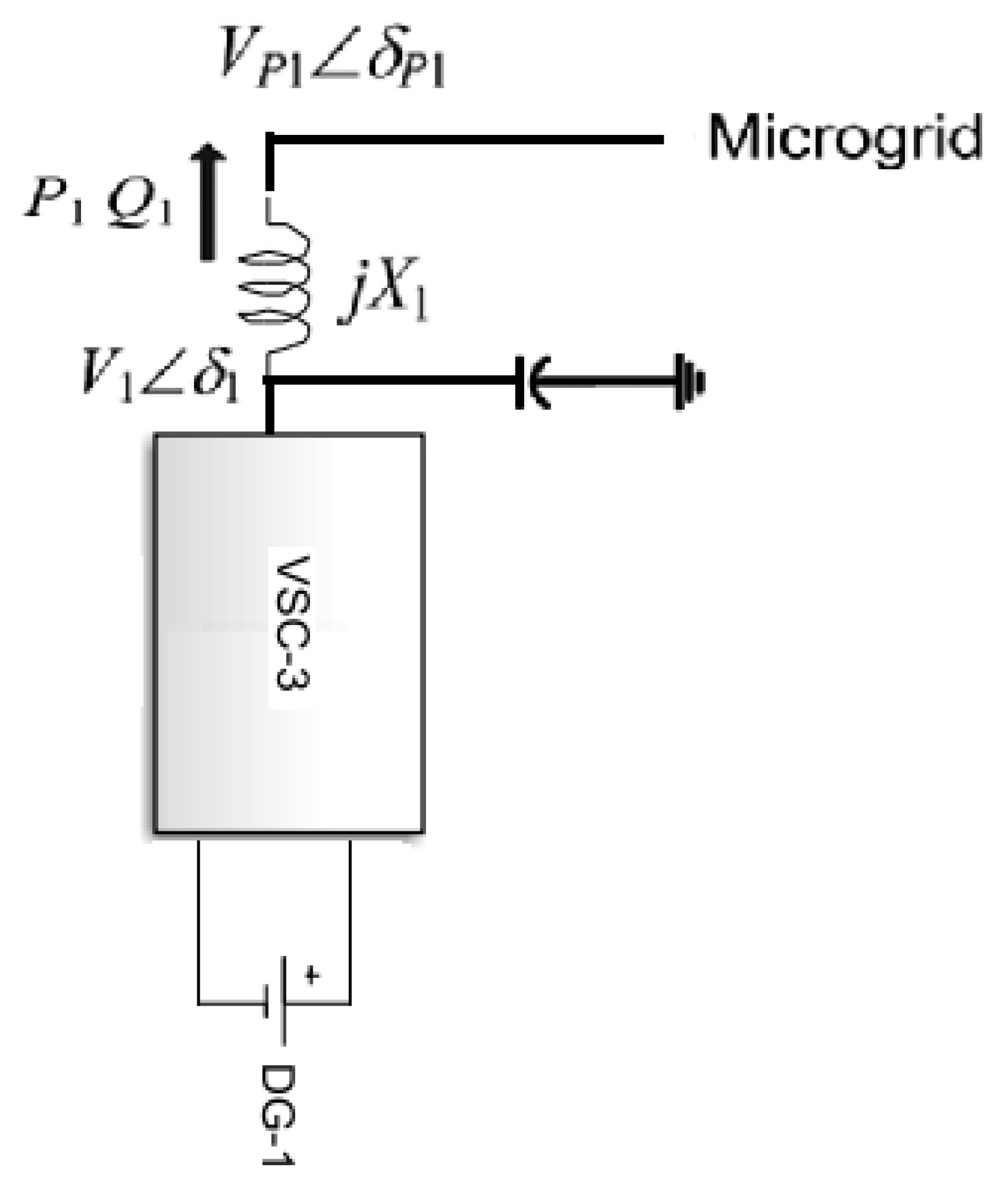
2.2. Back to Back Controlled Converter
2.3. DC Microgrid Method of Control
2.4. AC Microgrid Method of Control
2.5. Simulation of the Studied System
3. Results and Discussion
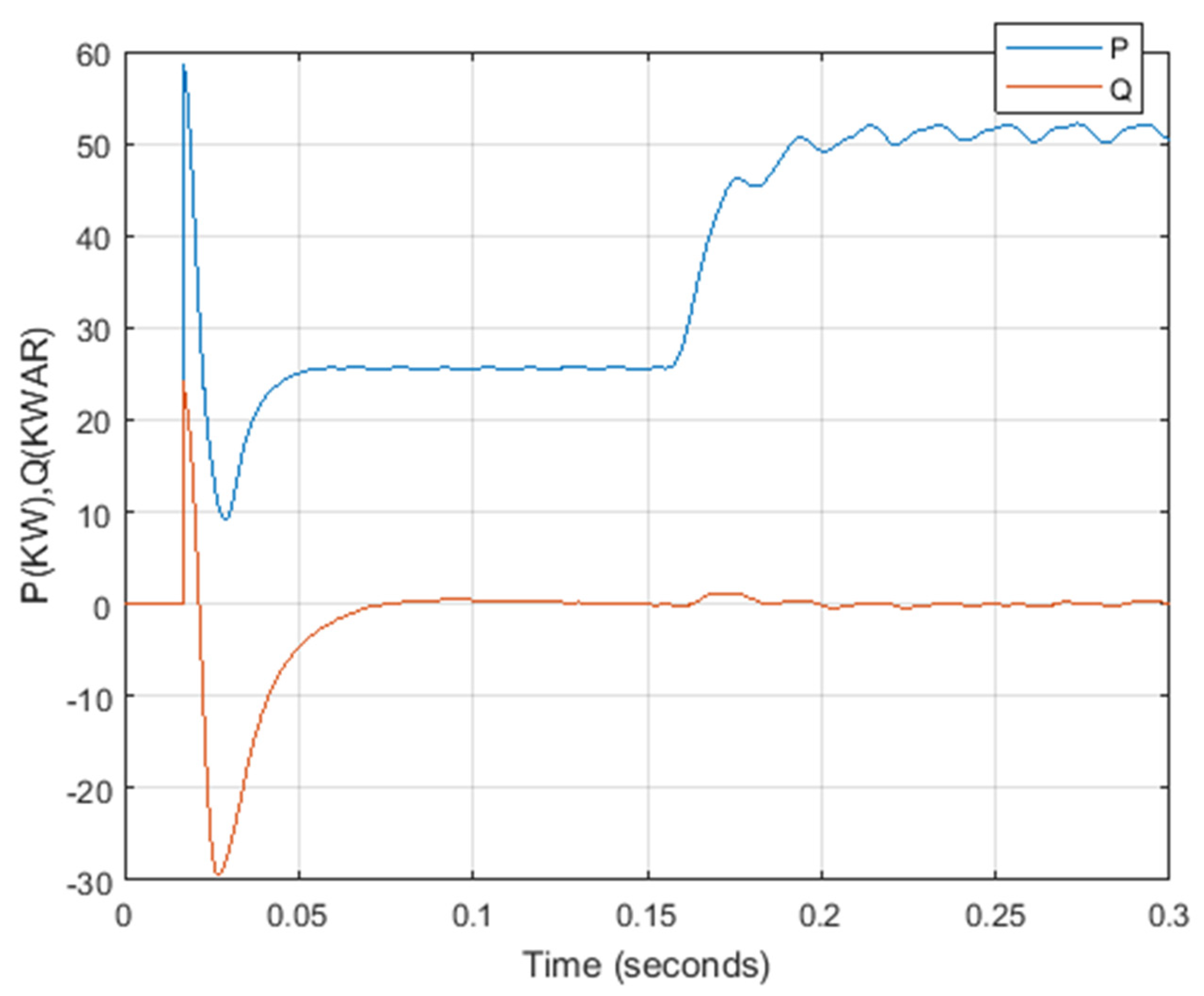
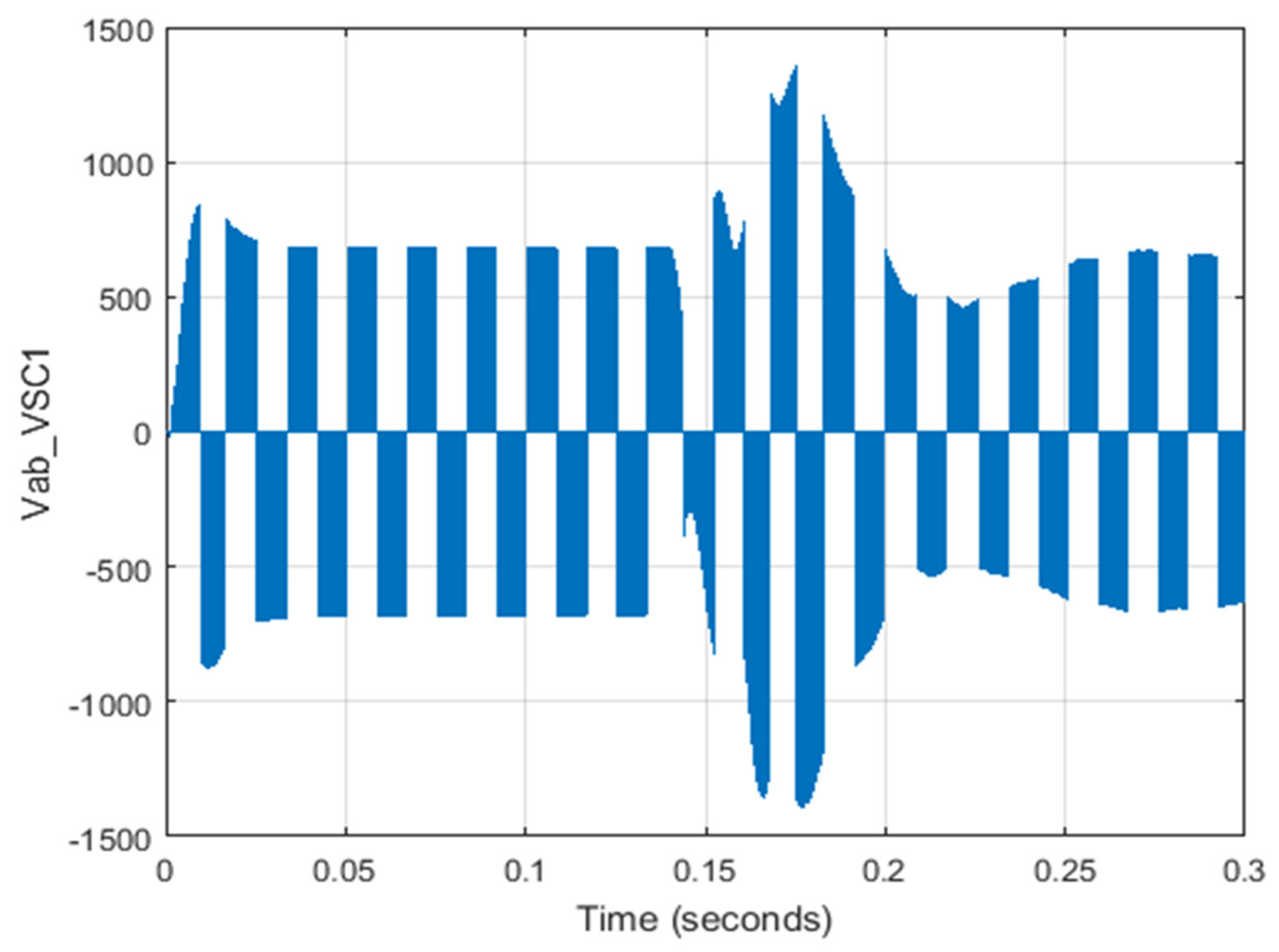
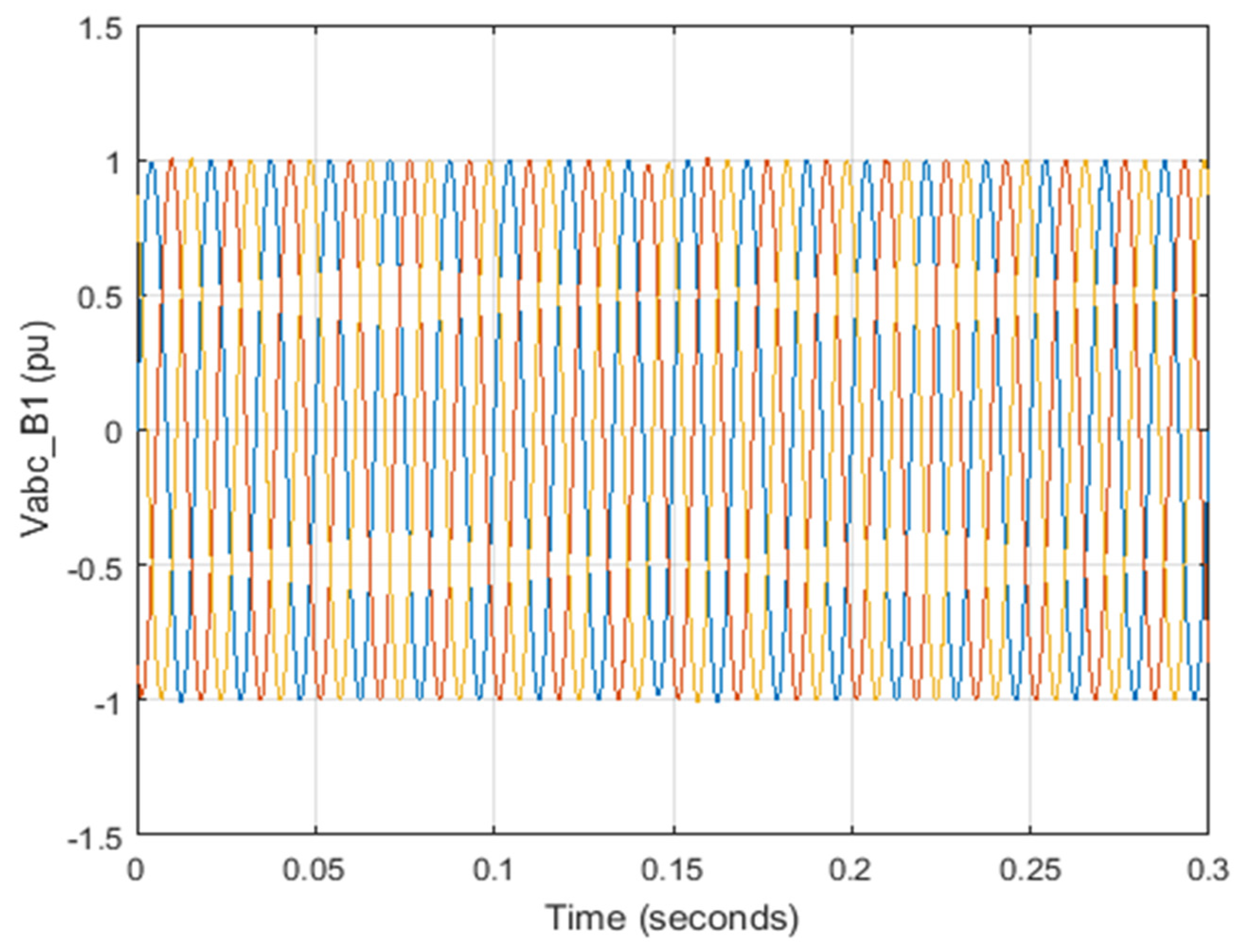
3.1. Scenario 1: Normal System Operation When AC Microgrid Receives Continuous Power from the National Grid and Vice-Versa
3.2. Scenario 2: AC Grid Error and Checking Microgrid Becoming Insular
3.3. Scenario 3: Error on the Microgrid Side and Checking Becoming Insular
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Khan, A.; Memon, S.; Sattar, T.P. Analyzing integrated renewable energy and smart-grid systems to improve voltage quality and harmonic distortion losses at electric-vehicle charging stations. IEEE Access 2018, 6, 26404–26415. [Google Scholar] [CrossRef]
- Makeen, P.; Ghali, H.A.; Memon, S. Experimental and theoretical analysis of the fast charging polymer lithium-ion battery based on Cuckoo Optimization Algorithm (COA). IEEE Access 2020, 8, 140486–140496. [Google Scholar] [CrossRef]
- Alayi, R.; Jahangeri, M.; Monfared, H. Optimal location of electrical generation from urban solid waste for biomass power plants. Anthropog. Pollut. J. 2020, 4, 44–51. [Google Scholar]
- Yang, L.; Yang, J. A robust dual-loop current control method with a delay-compensation control link for LCL-type shunt active power filters. IEEE Trans. Power Electron. 2018, 34, 6183–6199. [Google Scholar] [CrossRef]
- Alayi, R.; Sobhani, E.; Najafi, A. Analysis of Environmental Impacts on the Characteristics of Gas Released from Biomass. Anthropog. Pollut. J. 2020, 4, 1–14. [Google Scholar]
- He, J.; Li, Y.; Liang, B.; Wang, C. Inverse power factor droop control for decentralized power sharing in series-connected-microconverters-based islanding microgrids. IEEE Trans. Ind. Electron. 2017, 64, 7444–7454. [Google Scholar] [CrossRef]
- Alayi, R.; Rouhi, H. Techno-Economic Analysis of Electrical Energy Generation from Urban Waste in Hamadan, Iran. Int. J. Des. Nat. Ecodynamics 2020, 15, 337–341. [Google Scholar] [CrossRef]
- Alayi, R.; Jahanbin, F. Generation Management Analysis of a Stand-alone Photovoltaic System with Battery. Renew. Energy Res. Appl. 2020, 1, 205–209. [Google Scholar]
- Giraud, F.; Salameh, Z.M. Steady-state performance of a grid-connected rooftop hybrid wind-photovoltaic power system with battery storage. IEEE Trans. Energy Convers. 2001, 16, 1–7. [Google Scholar] [CrossRef]
- Shamel, A.; Marefati, M.; Alayi, R.; Gholaminia, B.; Rohl, H. Designing a PID controller to control a fuel cell voltage using the imperialist competitive algorithm. Adv. Sci. Technol. Res. J. 2016, 10, 176–181. [Google Scholar] [CrossRef] [Green Version]
- Ribeiro, P.F.; Johnson, B.K.; Crow, M.L.; Arsoy, A.; Liu, Y. Energy storage systems for advanced power applications. Proc. IEEE 2001, 89, 1744–1756. [Google Scholar] [CrossRef]
- Gurkaynak, Y.; Khaligh, A. Control and power management of a grid connected residential photovoltaic system with plug-in hybrid electric vehicle (PHEV) load. In Proceedings of the 2009 Twenty-Fourth Annual IEEE Applied Power Electronics Conference and Exposition, Washington, DC, USA, 15–19 February 2009; pp. 2086–2091. [Google Scholar]
- Alayi, R.; Kasaeian, A.; Najafi, A.; Jamali, E. Optimization and evaluation of a wind, solar and fuel cell hybrid system in supplying electricity to a remote district in national grid. Int. J. Energy Sect. Manag. 2020, 14, 408–418. [Google Scholar] [CrossRef]
- Alayi, R.; Harasii, H.; Pourderogar, H. Modeling and optimization of photovoltaic cells with GA algorithm. J. Robot. Control (JRC) 2021, 2, 35–41. [Google Scholar]
- Tan, K.T.; So, P.L.; Chu, Y.C.; Chen, M.Z.Q. Coordinated control and energy management of distributed generation inverters in a microgrid. IEEE Trans. Power Deliv. 2013, 28, 704–713. [Google Scholar] [CrossRef] [Green Version]
- Shahparasti, M.; Savaghebi, M.; Hosseinpour, M.; Rasekh, N. Enhanced circular chain control for parallel operation of inverters in ups systems. Sustainability 2020, 12, 8062. [Google Scholar] [CrossRef]
- Hoke, A.F.; Nelson, A.; Chakraborty, S.; Bell, F.; McCarty, M. An islanding detection test platform for multi-inverter islands using power HIL. IEEE Trans. Ind. Electron. 2018, 65, 7944–7953. [Google Scholar] [CrossRef]
- Haider, S.; Li, G.; Wang, K. A dual control strategy for power sharing improvement in islanded mode of AC microgrid. Prot. Control Mod. Power Syst. 2018, 3, 10. [Google Scholar] [CrossRef]
- Castilla, M.; de Vicuña, L.G.; Miret, J. Control of power converters in AC microgrids. In Microgrids Design and Implementation; Springer: Cham, Switzerland, 2019; pp. 139–170. [Google Scholar]
- Yue, Y.; Chen, Y.; Luo, A.; Ma, F.; Xu, Q.; He, Z. Robust predictive dual-loop control method based on Lyapunov function stability and energy equilibrium though double-core processors for active power filter. Int. J. Electr. Power Energy Syst. 2017, 89, 69–81. [Google Scholar] [CrossRef]
- Çelik, D.; Meral, M.E. Current control based power management strategy for distributed power generation system. Control Eng. Pract. 2019, 82, 72–85. [Google Scholar] [CrossRef]
- Li, L.; Sun, Y.; Liu, Y.; Han, H.; Ye, H.; Su, M.; Shi, G. Power factor consistency control for decentralized power sharing in islanded AC microgrids with cascaded inverters. In Proceedings of the IECON 2017-43rd Annual Conference of the IEEE Industrial Electronics Society, Beijing, China, 29 October–1 November 2017; pp. 1435–1440. [Google Scholar] [CrossRef]
- Das, P.P.; Chattopadhayay, S.; Palmal, M. A d–q voltage droop control method with dynamically phase-shifted phase-locked loop for inverter paralleling without any communication between individual inverters. IEEE Trans. Ind. Electron. 2017, 64, 4591–4600. [Google Scholar] [CrossRef]
- Kasaeian, A.; Shamel, A.; Alayi, R. Simulation and economic optimization of wind turbines and photovoltaic hybrid system with storage battery and hydrogen tank (case study the city of Yazd). J. Curr. Res. Sci. 2015, 3, 105. [Google Scholar]
- Alayi, R.; Ahmadi Kamarposhti, M.; Gharibi, M.; Abbasi zanghaneh, S.; Jahangiri, M. Design and simulation of a hybrid system based on renewable energy for hydrogen production. J. Power Technol. 2020, 100, 331–340. [Google Scholar]
- Alayi, R.; Khan, M.R.B.; Mohmammadi, M.S.G. Feasibility study of grid-connected PV system for peak demand reduction of a residential building in Tehran, Iran. Math. Model. Eng. Probl. 2020, 7, 563–567. [Google Scholar] [CrossRef]
- Ghosh, A.; Joshi, A. A new approach to load balancing and power factor correction in power distribution system. IEEE Trans. Power Deliv. 2000, 15, 417–422. [Google Scholar] [CrossRef]
- Majumder, R.; Ghosh, A.; Ledwich, G.; Zare, F. Power management and power flow control with back-to-back converters in a utility connected microgrid. IEEE Trans. Power Syst. 2009, 25, 821–834. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Wang, P.; Loh, P.C. A hybrid AC/DC microgrid and its coordination control. IEEE Trans. Smart Grid 2011, 2, 278–286. [Google Scholar]





















| Grid | Load | Permitted Output Power | Converter Losses | DC Voltage | Transformer | LC Filter |
|---|---|---|---|---|---|---|
| 25 kW and 10 MVA | 25 kW | 30 kW | R = 0.1 Ω per phase | 750 V | 25 kV/380 V | L = 5 mh, C = 8 kVar |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alayi, R.; Zishan, F.; Mohkam, M.; Hoseinzadeh, S.; Memon, S.; Garcia, D.A. A Sustainable Energy Distribution Configuration for Microgrids Integrated to the National Grid Using Back-to-Back Converters in a Renewable Power System. Electronics 2021, 10, 1826. https://doi.org/10.3390/electronics10151826
Alayi R, Zishan F, Mohkam M, Hoseinzadeh S, Memon S, Garcia DA. A Sustainable Energy Distribution Configuration for Microgrids Integrated to the National Grid Using Back-to-Back Converters in a Renewable Power System. Electronics. 2021; 10(15):1826. https://doi.org/10.3390/electronics10151826
Chicago/Turabian StyleAlayi, Reza, Farhad Zishan, Mahdi Mohkam, Siamak Hoseinzadeh, Saim Memon, and Davide Astiaso Garcia. 2021. "A Sustainable Energy Distribution Configuration for Microgrids Integrated to the National Grid Using Back-to-Back Converters in a Renewable Power System" Electronics 10, no. 15: 1826. https://doi.org/10.3390/electronics10151826
APA StyleAlayi, R., Zishan, F., Mohkam, M., Hoseinzadeh, S., Memon, S., & Garcia, D. A. (2021). A Sustainable Energy Distribution Configuration for Microgrids Integrated to the National Grid Using Back-to-Back Converters in a Renewable Power System. Electronics, 10(15), 1826. https://doi.org/10.3390/electronics10151826









