Modulation Performance Enhancement of Directly Modulated Injection-Locked Semiconductor Lasers Using an Equivalent Electrical Circuit
Abstract
:1. Introduction
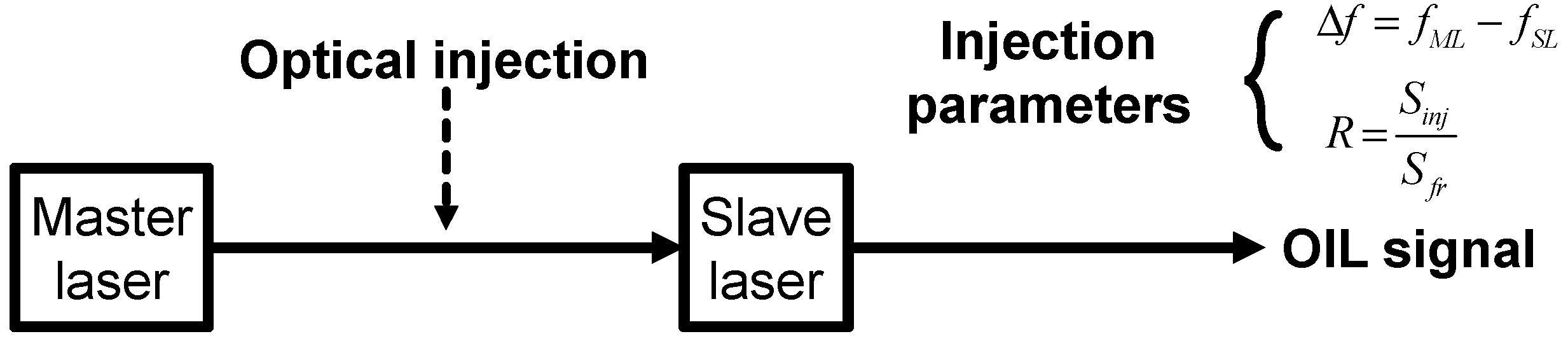
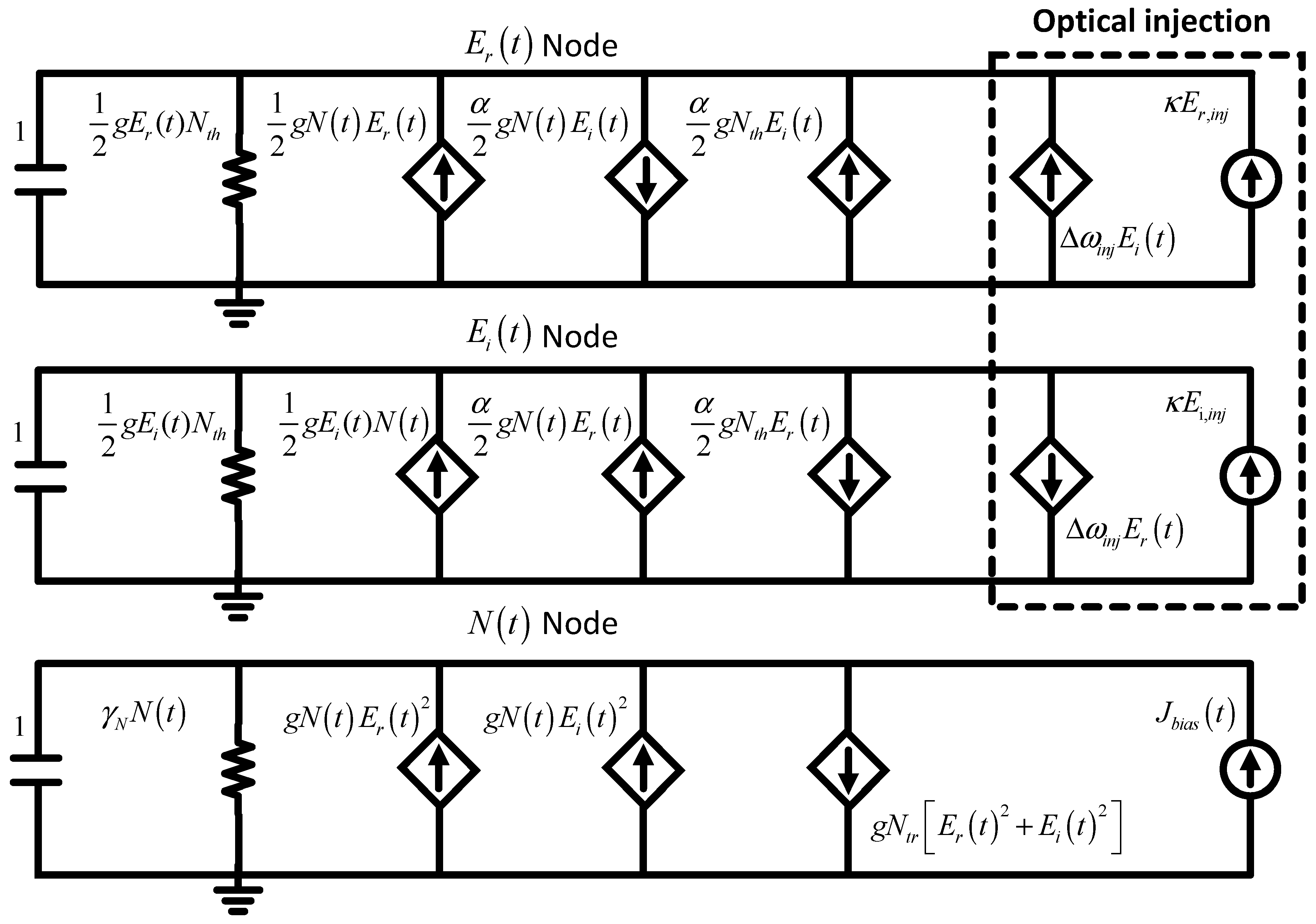
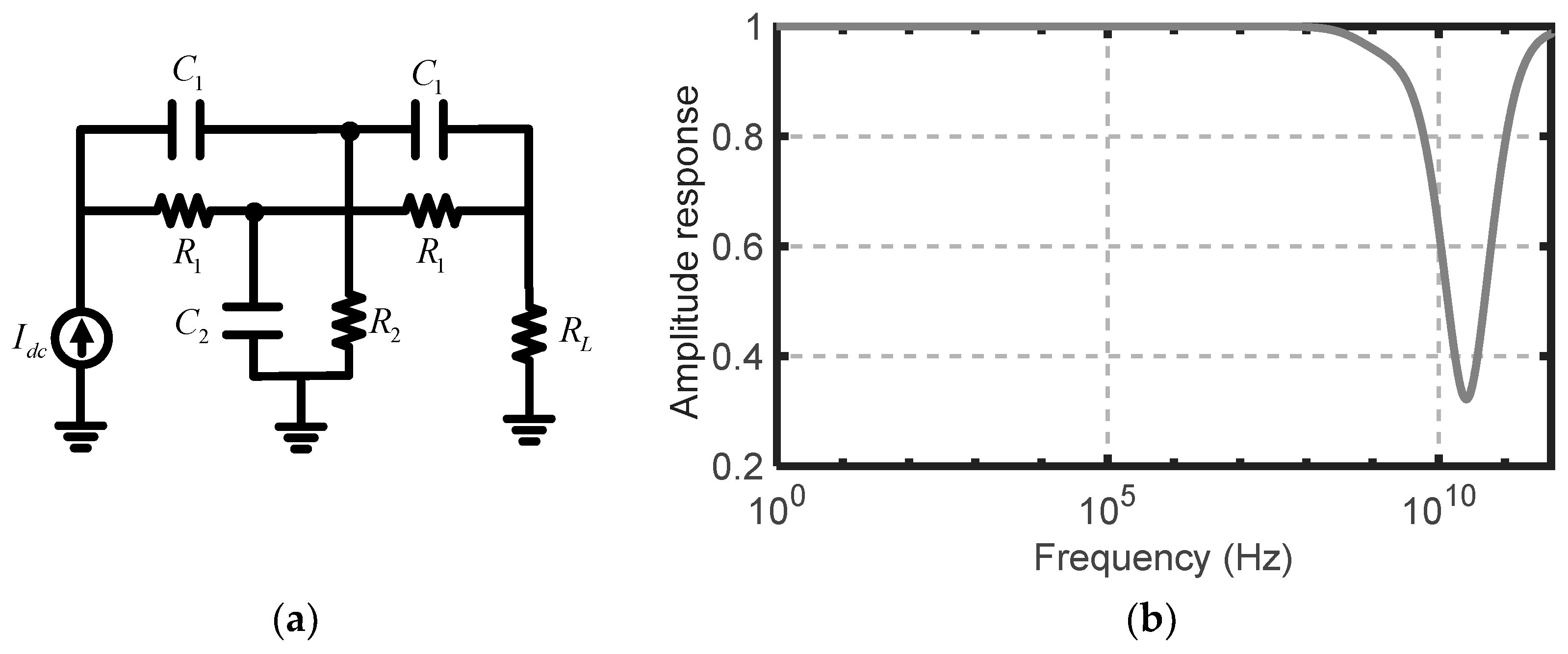
2. Equivalent Circuit Model of OIL Lasers
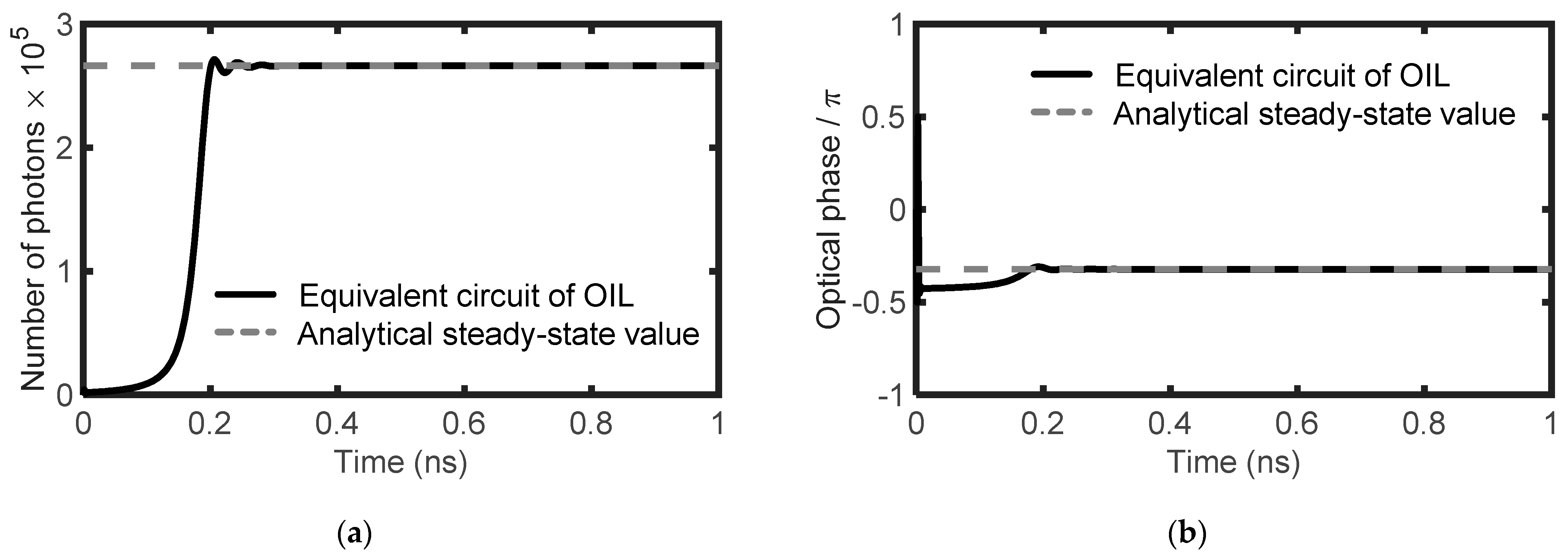
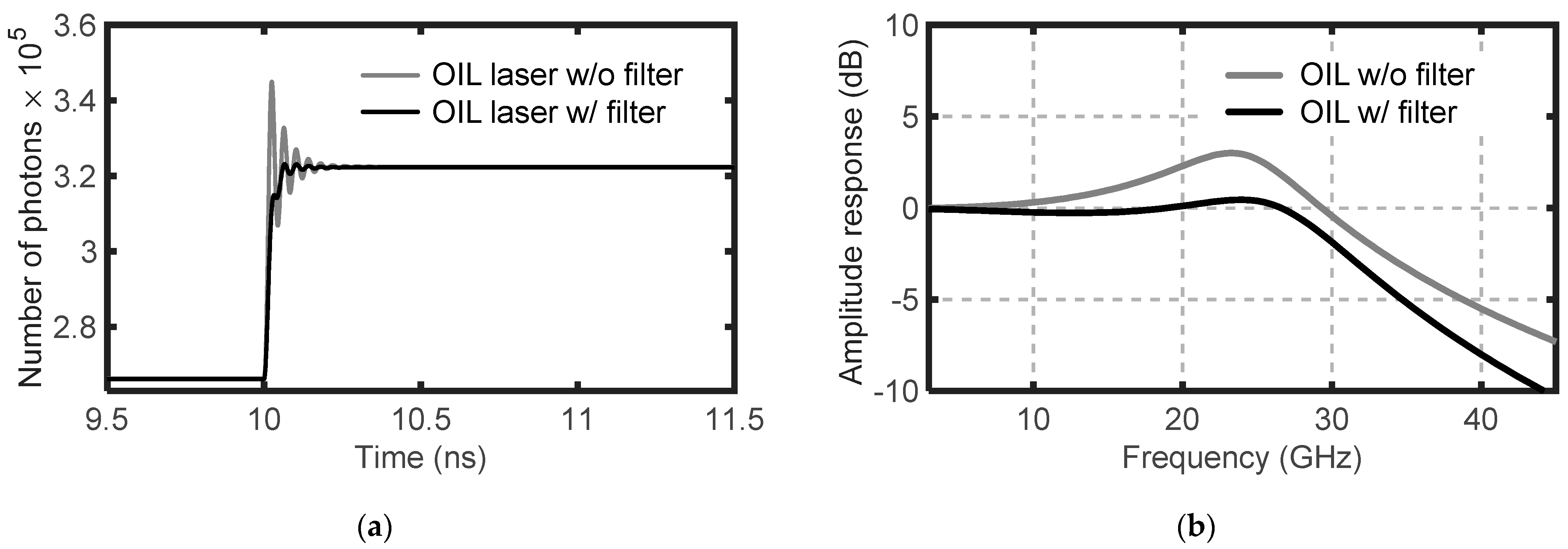
3. Simulation Results
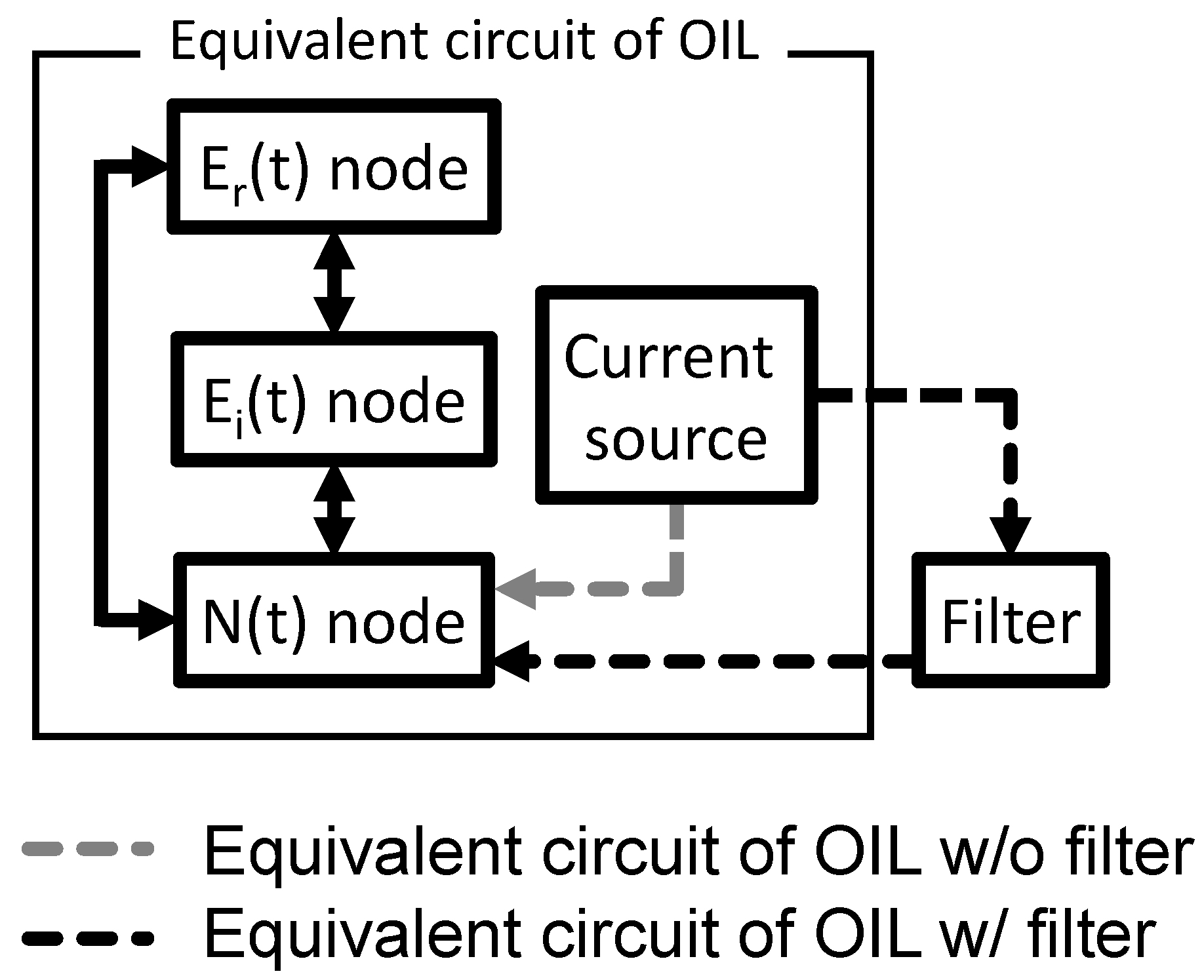
4. Modulation Performance Improvement
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Baek, Y.S. Technical trends of photonic integrated circuits for high speed, high capacity optical communication. Electron. Telcom. Trend. 2009, 24, 52–60. [Google Scholar]
- Baek, Y.S. Optical components for high speed optical communications. Korean J. Opt. Photon. 2013, 24, 297–310. [Google Scholar] [CrossRef]
- Smit, M.; Williams, K.; van der Tol, J. Past, present, and future of InP-based photonic integration. APL Photonics 2019, 4, 050901. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.J. Large-scale photonic integrated circuits. IEEE J. Sel. Top. Quantum Electron. 2006, 11, 1–14. [Google Scholar]
- Wang, J.; Haldar, M.K.; Mendis, F.V.C. Equivalent circuit model of injection-locked laser diodes. Microw. Opt. Technol. Lett. 1998, 18, 124–126. [Google Scholar] [CrossRef]
- Liu, Z.; Slavik, R. Optical injection locking: From principle to applications. J. Lightwave Technol. 2020, 38, 43–59. [Google Scholar] [CrossRef]
- Lau, E.; Sung, H.K.; Wu, M.C. Frequency response enhancement of optical injection-locked lasers. IEEE J. Quantum Electron. 2008, 44, 90–99. [Google Scholar] [CrossRef] [Green Version]
- Zhao, X.; Change-Hasnain, C.J.; Hofmann, W.; Amann, M.C. Modulation Efficiency Enhancement of 1.55-um Injection-Locked VCSELs. In Proceedings of the IEEE 20th International Semiconductor Laser Conference, Kohala Coast, HI, USA, 18–21 September 2006. [Google Scholar]
- Ramdane, A.C.; Gruning, P.; Roncin, V.; Du-Burck, F. Frequency stability transfer by optical injection locking into a semiconductor frequency comb. In Proceedings of the Joint Conference of the European Frequency and Time Forum and IEEE International Frequency Control Symposium, Besancon, France, 9–13 July 2017. [Google Scholar]
- Lau, E.K.; Wong, L.J.; Wu, M.C. Enhanced modulation characteristics of optical injection-locked lasers: A tutorial. IEEE J. Sel. Top. Quantum Electron. 2009, 15, 618–633. [Google Scholar] [CrossRef]
- Genest, J.; Chamberland, M.; Tremblay, P.; Tetu, M. Microwave signal generated by optical heterodyne between injection-locked semiconductor lasers. IEEE J. Quantum Electron. 1997, 33, 989–998. [Google Scholar] [CrossRef]
- Sung, H.K.; Lau, E.K.; Wu, M.C.; Tishinin, D.; Liou, K.Y.; Tasang, W.T. Large-Signal Analog Modulation response of Monolithic Opticcal Injection-Locked DFB Lasers. In Proceedings of the Conference on Laser and Electro-Optics, Baltimore, MD, USA, 22–27 May 2005. [Google Scholar]
- Perrott, A.H.; Caro, L.; Dernaika, M.; Peters, F.H. A Comparison between off and On-Chip Injection Locking in a Photonic Integrated Circuit. Photonics 2019, 6, 103. [Google Scholar] [CrossRef] [Green Version]
- Sorace, A.C.; Leu, J.; Watts, M.R.; Stojanovic, V. Electro-optical co-simulation for integrated CMOS photonic circuits with VerilogA. Opt. Express 2015, 23, 27180–27203. [Google Scholar] [CrossRef]
- Lim, D.W.; Cho, H.U.; Sung, H.K.; Yi, J.C. A PSPICE circuit modeling of strained AlGaInN laser diode based on the multilevel rate equations. J. Opt. Soc. Korea 2009, 13, 386–391. [Google Scholar] [CrossRef] [Green Version]
- Ganesh, M.M.; Rekha, E.; RajKumar, P. A comprehensive equivalnet circuit model for the study of thermal and spectral characteristics in laser diodes. Int. J. Lgts. Electron. Opt. 2013, 125, 3030–3036. [Google Scholar]
- Katz, J.; Margalit, S.; Harder, C.; Wilt, D.; Yariv, A. The intrinsic electrical equivalent circuit of a laser diode. IEEE J. Quantum Electron. 1981, 17, 4–9. [Google Scholar] [CrossRef]
- Smy, T.; Gunupudi, P. Robust simulation of opto-electronic systems by alternating complex envelope representations. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2012, 31, 1139–1143. [Google Scholar] [CrossRef]
- Mogensen, F.; Olesen, H.; Jacobsen, G. Locking conditions and stability properties for a semiconductor laser with external light injection. IEEE J. Quantum Electron. 1985, 21, 784–793. [Google Scholar] [CrossRef]
- Henry, C.; Olsson, N.; Dutta, N. Locking range and stability of injection locked 1.54 µm InGaAsp semiconductor lasers. IEEE J. Quantum Electron. 1985, 21, 1152–1156. [Google Scholar] [CrossRef]
- Lang, R. Injection locking properties of a semiconductor laser. IEEE J. Quantum Electron. 1982, 18, 976–983. [Google Scholar] [CrossRef]
- Murakami, A.; Kawashima, K.; Atsuki, K. Cavity resonance shift and bandwidth enhancement in semiconductor lasers with strong light injection. IEEE J. Quantum Electron. 2003, 39, 1196–1204. [Google Scholar] [CrossRef]
- Nguyen, A.H.; Cho, J.H.; Bae, H.J.; Sung, H.K. Side-lobe level reduction of an optical phased array using amplitude and phase modulation of array elements based on optically injection-locked semiconductor lasers. Photonics 2020, 7, 20. [Google Scholar] [CrossRef] [Green Version]
- Liu, Z.; Kakande, J.; Kelly, B.; O’Carroll, J.; Phelan, R.; Richardson, D.J.; Slavik, R. Modulator-free quadrature amplitude modulation. Nat. Commun. 2014, 5, 5911. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Smy, T.; Klein, J.; Jakubczyk, J. Self-consistent simulation of opto-electronic circuits using a modified nodal analysis formulation. IEEE Trans. Adv. Packag. 2010, 33, 4. [Google Scholar]
- Quarles, T.; Newton, A.; Pederson, D.; Sangiovanni-Vinentelli, A. SPICE 3 Version 3F5 User’s Manual, 1st ed.; UC Berkely EECS: Berkeley, CA, USA, 1994. [Google Scholar]
- Safwat, W.Z.M.; Moustafa, F.A.; Kamal, A.; Alaa, M. Estimation of parameters controlling direct modulation of semiconductor lasers. In Proceedings of the 26th National Radio Science Conference, El-Minia, Egypt, 17–19 March 2009. [Google Scholar]
- Lang, R.; Kobayashi, K. Suppression of the relaxation oscillation in the modulated output of semiconductor lasers. IEEE J. Quantum Electron. 1976, 12, 194–199. [Google Scholar] [CrossRef]
- Ahmed, M.; Mahmoud, S.W.Z.; Mahmoud, A.A. Influence of pseudorandom bit format on the direct modulation performance of semiconductor lasers. Pramana 2012, 79, 1443–1456. [Google Scholar] [CrossRef]
- Yildirim, R.; Schetzen, M. Applications of the single-mode laser-diode system theory. Opt. Commun. 2003, 219, 351–355. [Google Scholar] [CrossRef]
- Rizk, T.; Mahmoud, A.; Abdel-Rahman, M.; Ahemd, M. Correlation of Small-Signal Modulation with Digital Modulation Characteristics of High-Speed Semiconductor Lasers. Phys. Wave Phenom 2020, 28, 49–54. [Google Scholar] [CrossRef]

| Symbol | Quantity | Value | Unit |
|---|---|---|---|
| λ0 | Wavelength | 1550 | nm |
| g | Net stimulated gain | 4.7 × 104 | 1/s |
| Ntr | Transparency carrier number | 9.36 × 106 | - |
| Jth | Threshold current | 2 × 1016 | 1/s |
| Jbias | Bias current | 5 × Jth | 1/s |
| γP | Photon decay rate | 5 × 1011 | 1/s |
| γN | Carrier decay rate | 109 | 1/s |
| α | Linewidth enhancement factor | 5 | - |
| κ | Coupling ratio | 225 | 1/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bae, H.-J.; Cho, J.-H.; Sung, H.-K. Modulation Performance Enhancement of Directly Modulated Injection-Locked Semiconductor Lasers Using an Equivalent Electrical Circuit. Electronics 2021, 10, 2409. https://doi.org/10.3390/electronics10192409
Bae H-J, Cho J-H, Sung H-K. Modulation Performance Enhancement of Directly Modulated Injection-Locked Semiconductor Lasers Using an Equivalent Electrical Circuit. Electronics. 2021; 10(19):2409. https://doi.org/10.3390/electronics10192409
Chicago/Turabian StyleBae, Ho-Jun, Jun-Hyung Cho, and Hyuk-Kee Sung. 2021. "Modulation Performance Enhancement of Directly Modulated Injection-Locked Semiconductor Lasers Using an Equivalent Electrical Circuit" Electronics 10, no. 19: 2409. https://doi.org/10.3390/electronics10192409
APA StyleBae, H.-J., Cho, J.-H., & Sung, H.-K. (2021). Modulation Performance Enhancement of Directly Modulated Injection-Locked Semiconductor Lasers Using an Equivalent Electrical Circuit. Electronics, 10(19), 2409. https://doi.org/10.3390/electronics10192409







