An Efficient Design of High Step-Up Switched Z-Source (HS-SZSC) DC-DC Converter for Grid-Connected Inverters
Abstract
:1. Introduction
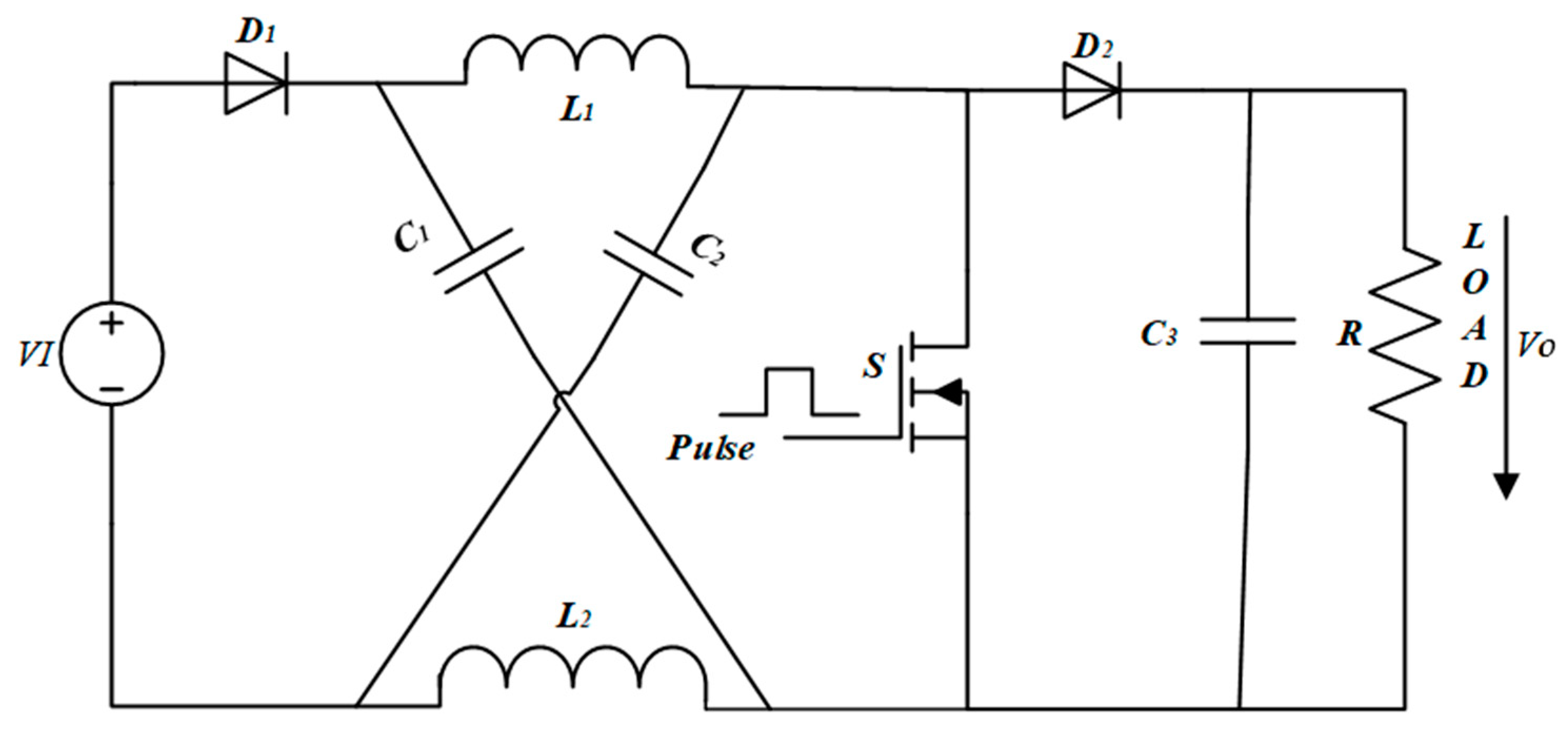
2. Configuration and Operating Principle of the HS-SZSC
3. Calculation of Gain Factor
- State-1
- State-2
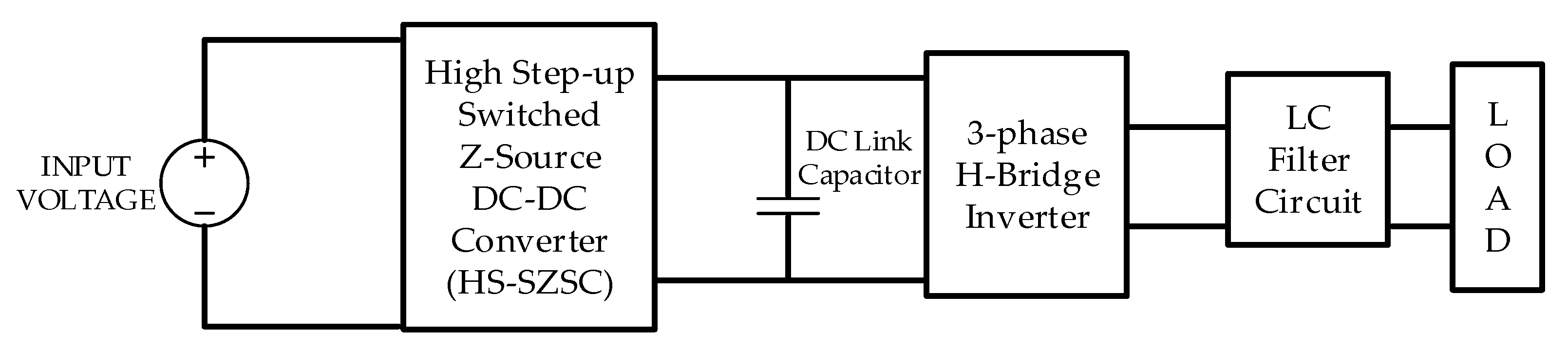
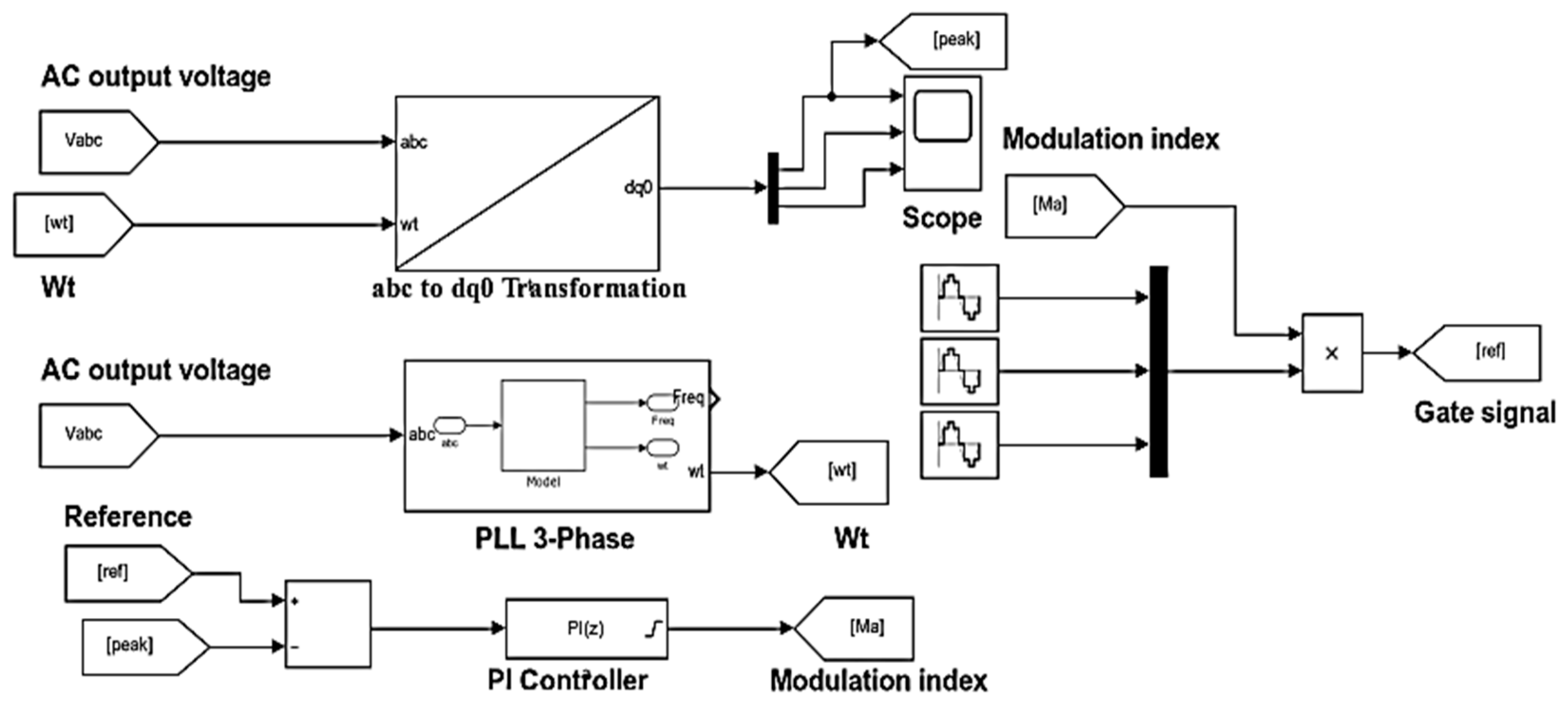
4. HS-SZSC Interfaced with 3-Phase Inverter (GCI)
- Phase matching.
- Reaction to the power outage: addressing an islanding issue.
4.1. Circuit Configuration
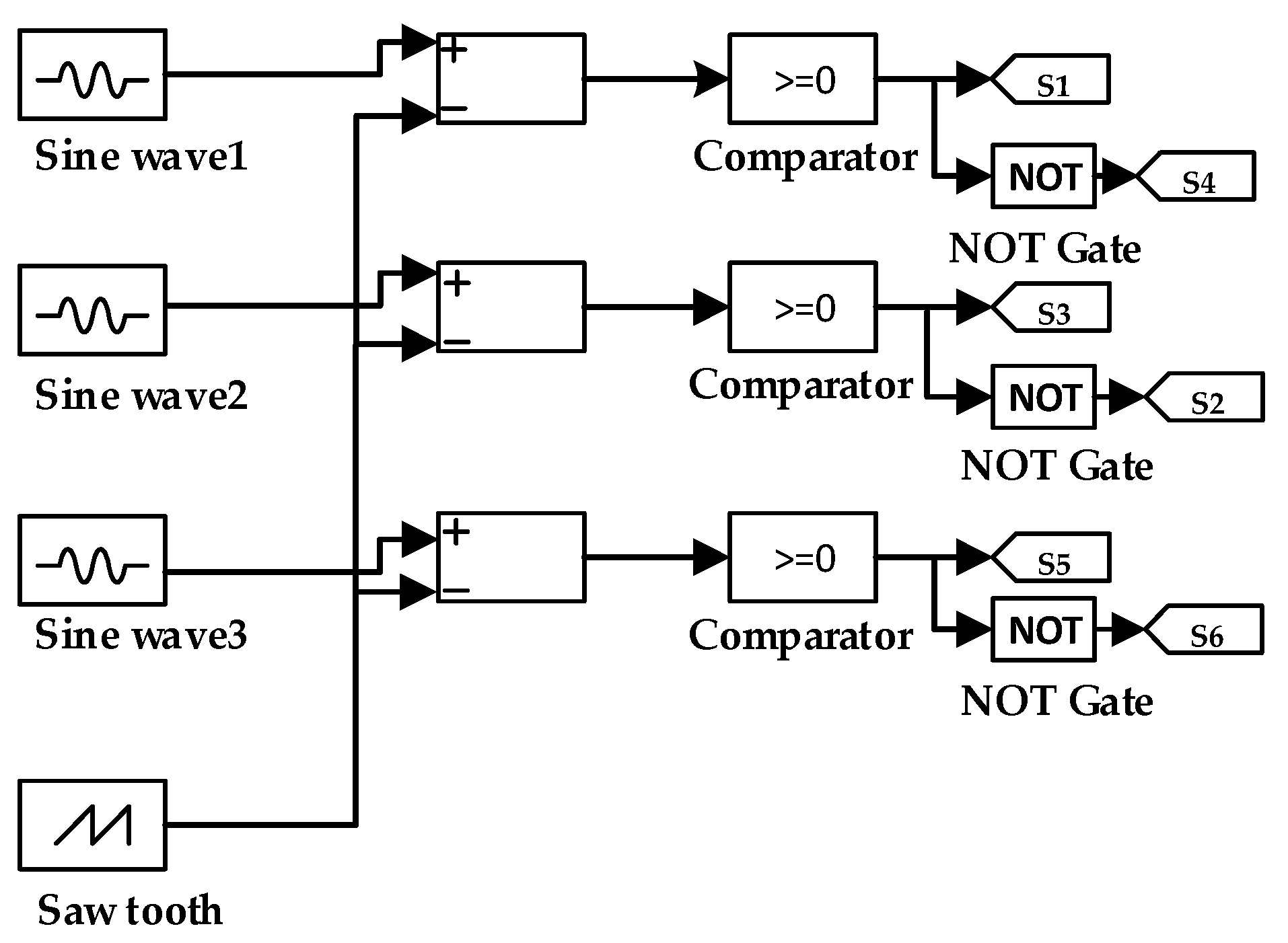
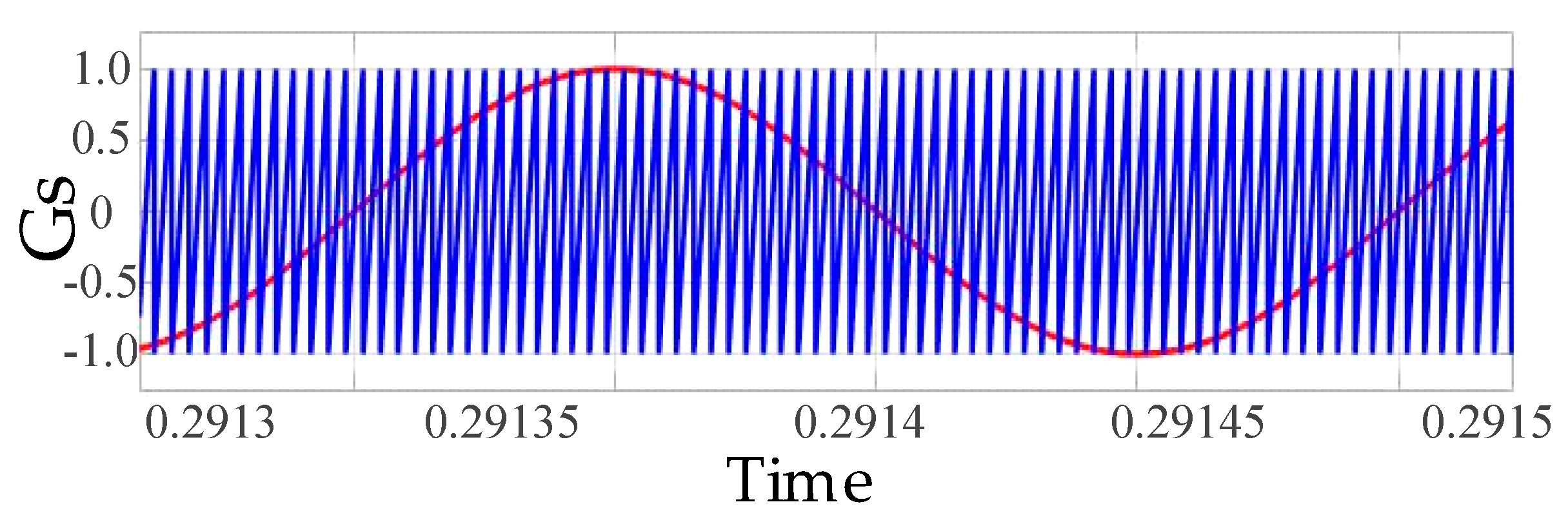
4.2. Sinusoidal Pulse Width Modulation (SPWM)
4.3. Inverter-Side Controller
5. Comparison of Voltage Gains
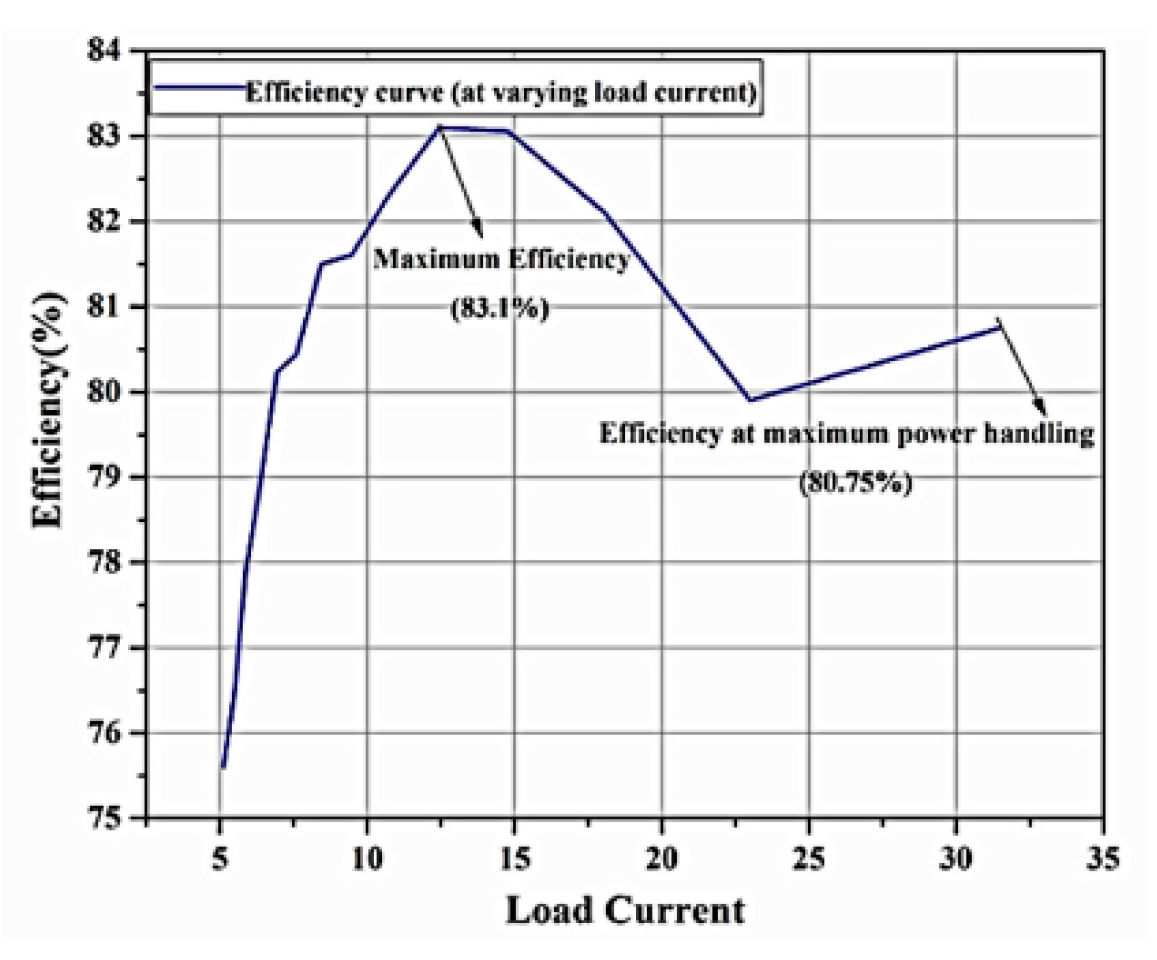
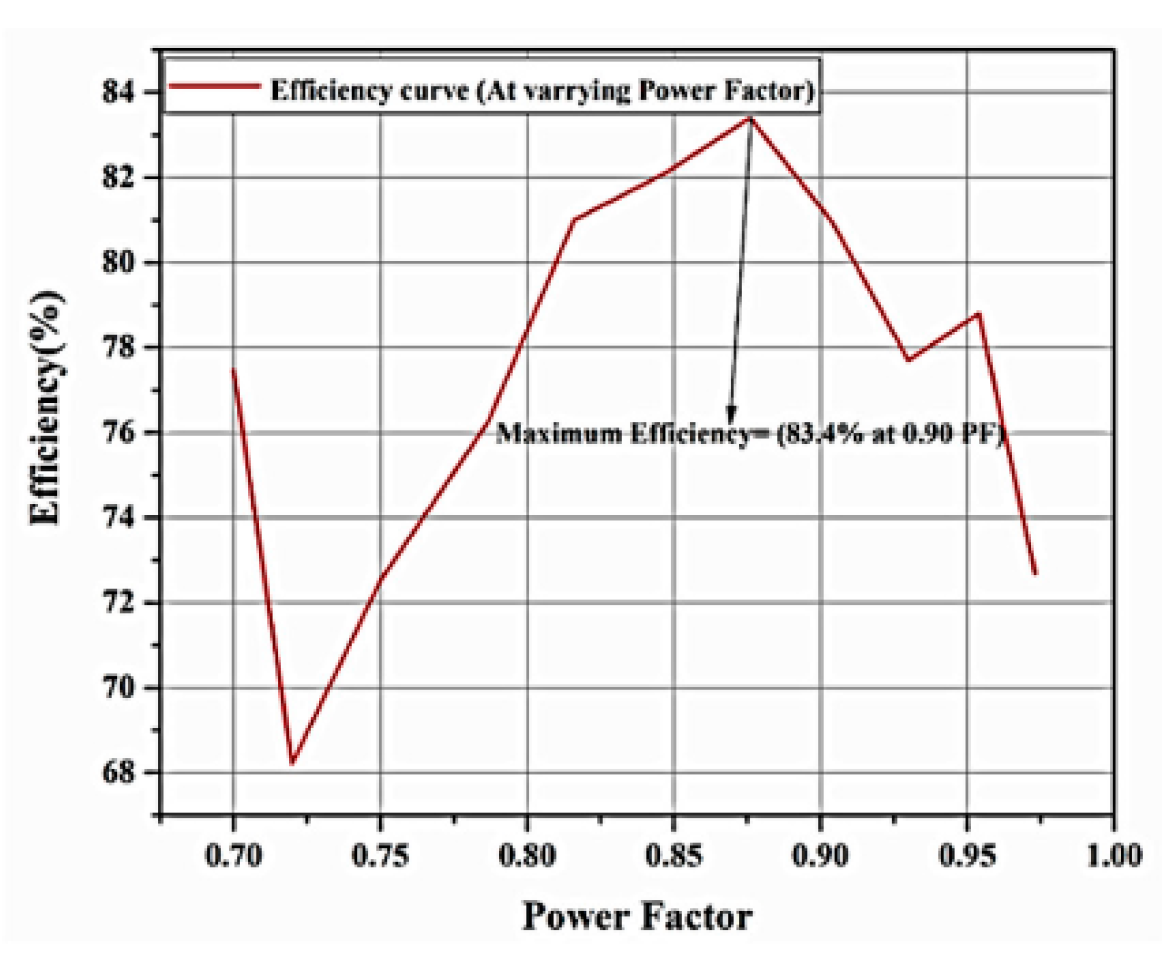
6. Results and Discussions
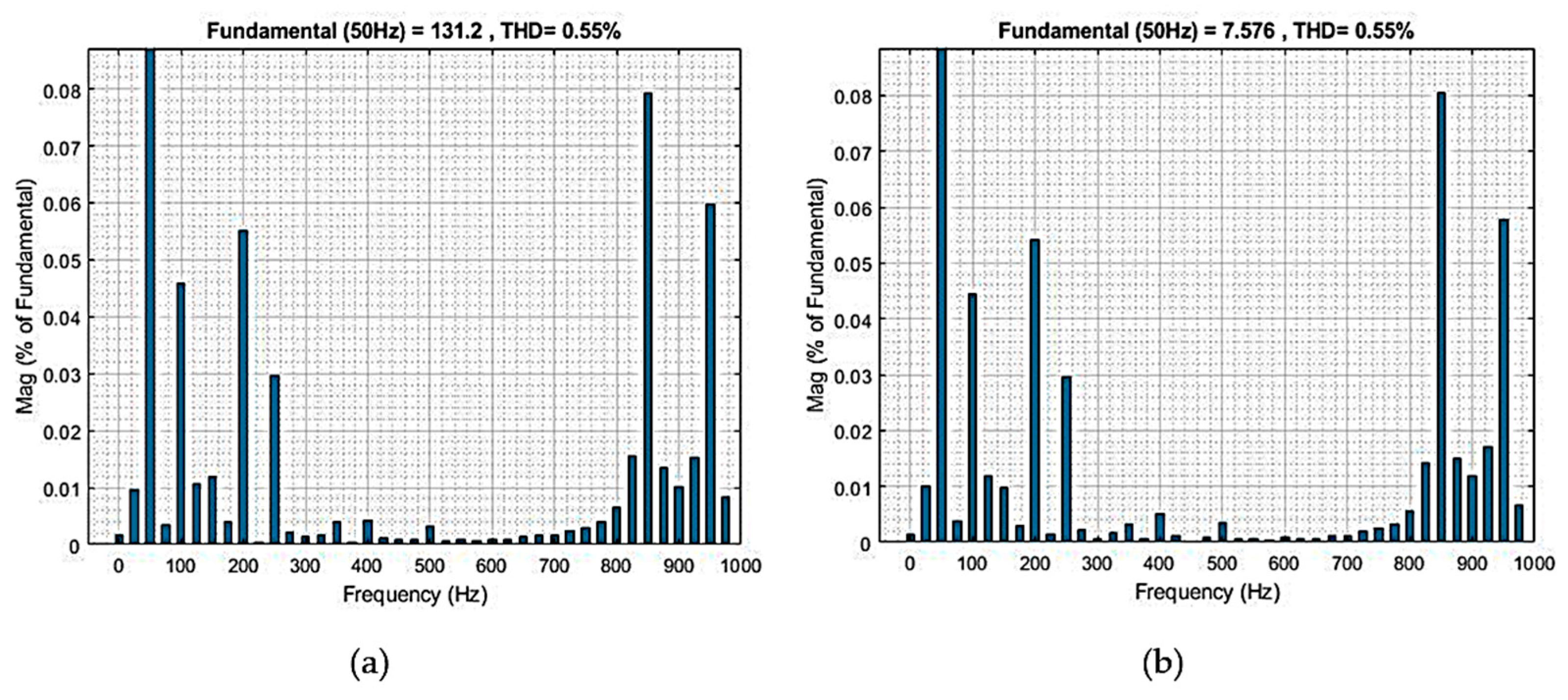
6.1. Case 1: Normal Conditions
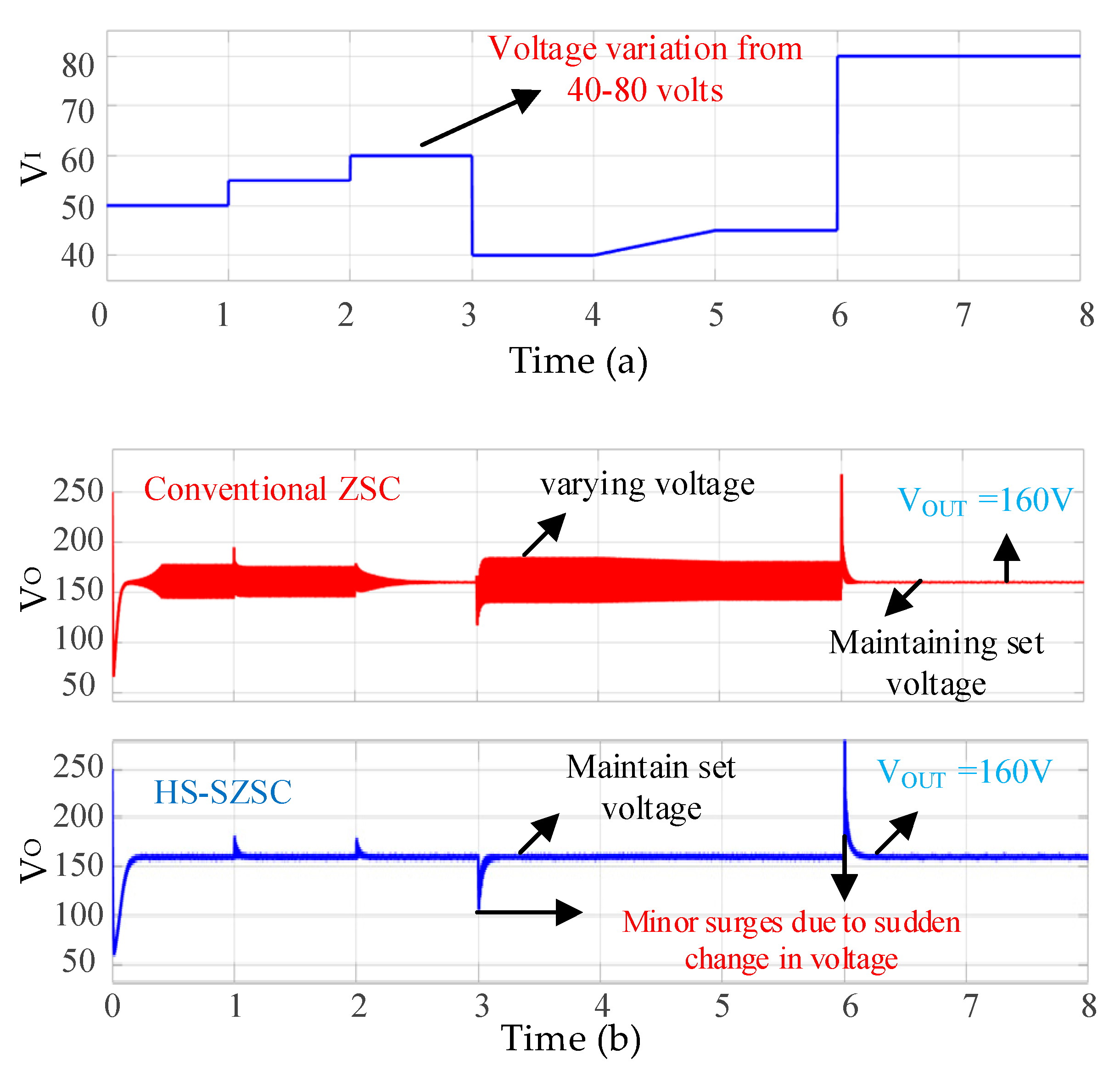
6.2. Case 2: Transient Conditions
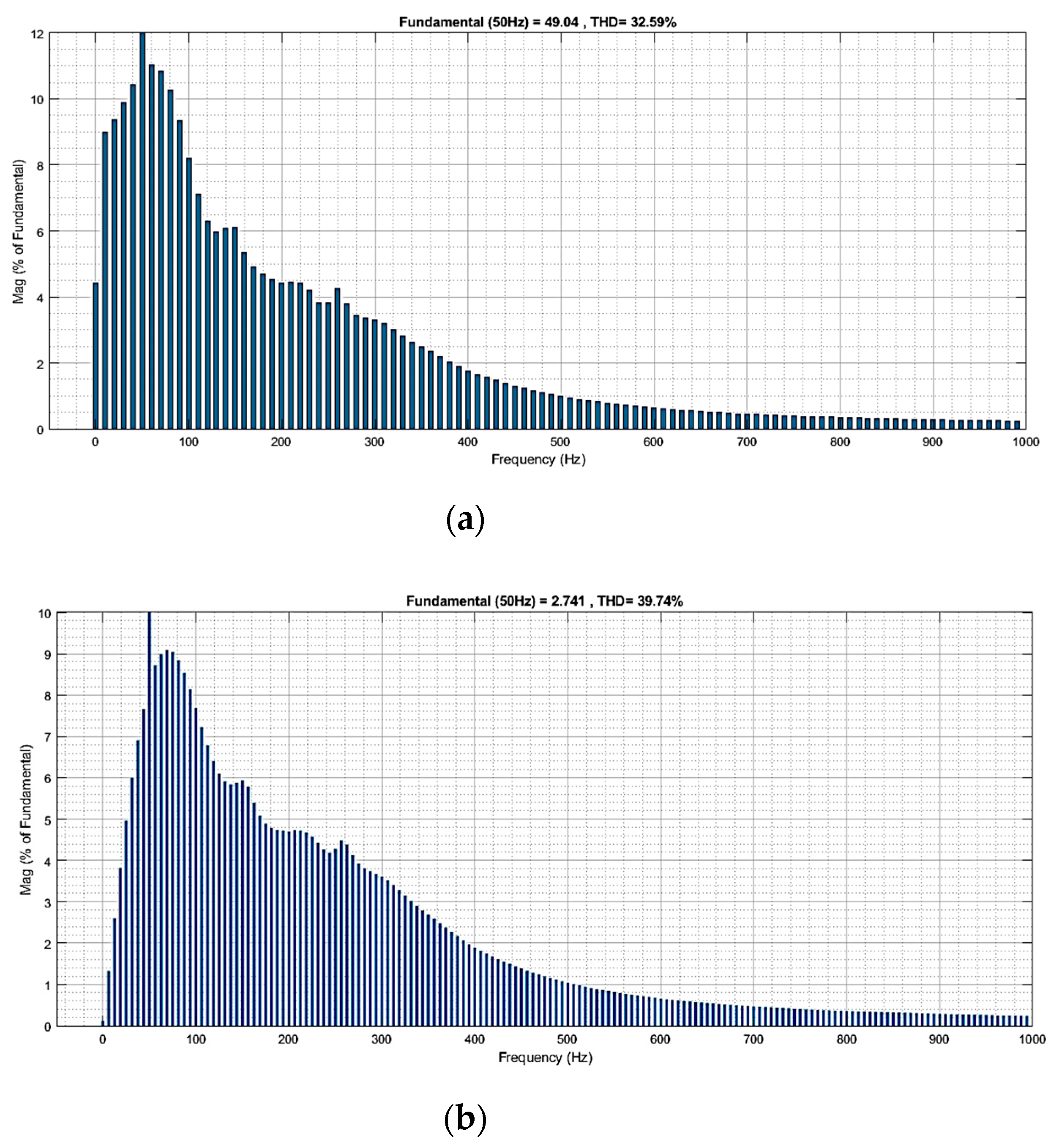
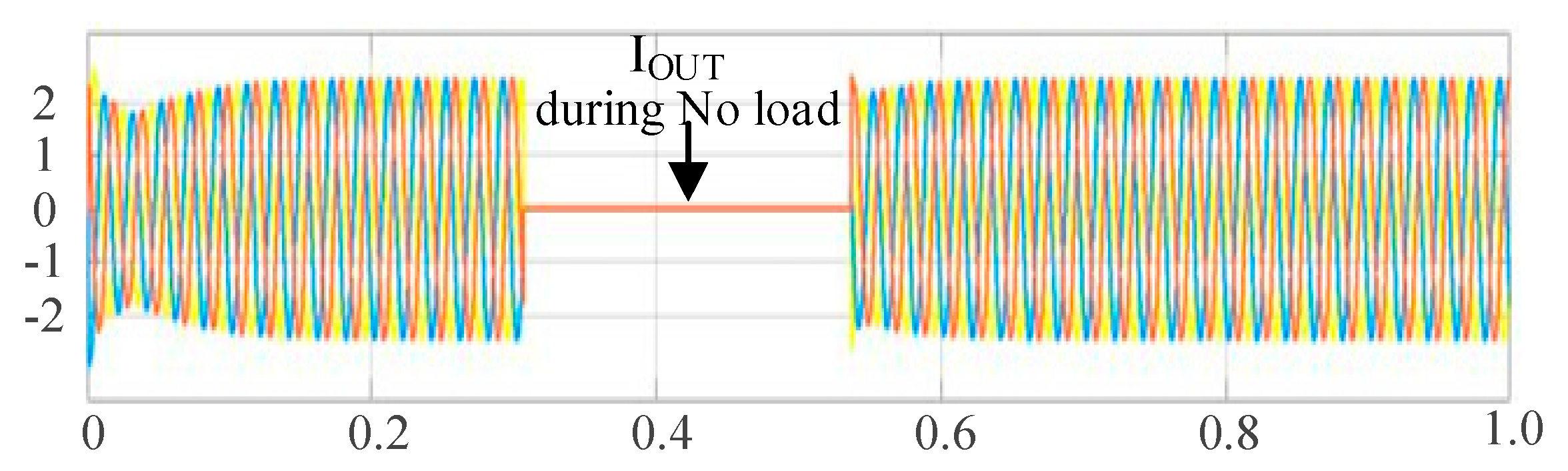
6.3. Case 3: Islanding Issues in GCIs
7. Conclusions and Future Works
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Revathi, B.S.; Prabhakar, M.; Gonzalez-Longatt, F. High-gain–high-power (HGHP) DC-DC converter for DC microgrid applications: Design and testing. Int. Trans. Electr. Energy Syst. 2018, 28, 1–45. [Google Scholar] [CrossRef] [Green Version]
- Kuang, Y.; Zhang, Y.; Zhou, B.; Li, C.; Cao, Y.; Li, L.; Zeng, L. A review of renewable energy utilization in islands. Renew. Sustain. Energy Rev. 2016, 59, 504–513. [Google Scholar] [CrossRef]
- Akinyele, D.O.; Rayudu, R.K.; Nair, N.K.C. Global progress in photovoltaic technologies and the scenario of development of solar panel plant and module performance estimation—Application in Nigeria. Renew. Sustain. Energy Rev. 2015, 48, 112–139. [Google Scholar] [CrossRef]
- Prabaharan, N.; Palanisamy, K. Analysis and integration of multilevel inverter configuration with boost converters in a photovoltaic system. Energy Convers. Manag. 2016, 128, 327–342. [Google Scholar] [CrossRef]
- Syam, M.S.; Kailas, T.S. Grid connected PV system using Cuk converter. In Proceedings of the 2013 Annual International Conference on Emerging Research Areas and 2013 International Conference on Microelectronics, Communications and Renewable Energy, Kanjirapally, India, 4–6 June 2013. [Google Scholar] [CrossRef]
- Sener, E.; Ertasgin, G. A 3-ph Grid-Connected Photovoltaic Inverter Utilizing Super-Lift Luo Converter. In Proceedings of the 2018 2nd International Symposium on Multidisciplinary Studies and Innovative Technologies (ISMSIT), Ankara, Turkey, 19–21 October 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Li, W.; He, X. Review of nonisolated high-step-up DC/DC converters in photovoltaic grid-connected applications. IEEE Trans. Ind. Electron. 2011, 58, 1239–1250. [Google Scholar] [CrossRef]
- Kim, J.K.; Moon, G.W. Derivation, analysis, and comparison of nonisolated single-switch high step-up converters with low voltage stress. IEEE Trans. Power Electron. 2015, 30, 1336–1344. [Google Scholar] [CrossRef]
- Choi, W.Y.; Lee, C.G. Photovoltaic panel integrated power conditioning system using a high efficiency step-up DC-DC converter. Renew. Energy 2012, 41, 227–234. [Google Scholar] [CrossRef]
- Wu, G.; Ruan, X.; Ye, Z. Nonisolated High Step-Up DC—DC Converters. IEEE Trans. Ind. Electron. 2015, 62, 383–393. [Google Scholar] [CrossRef]
- Poorali, B.; Torkan, A.; Adib, E. High step-up Z-source DC-DC converter with coupled inductors and switched capacitor cell. IET Power Electron. 2015, 8, 1394–1402. [Google Scholar] [CrossRef]
- Stauth, J.T. Pathways to mm-scale DC-DC converters: Trends, opportunities, and limitations. In Proceedings of the 2018 IEEE Custom Integrated Circuits Conference, CICC 2018, San Diego, CA, USA, 8–11 April 2018; pp. 1–8. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. Current-mode body-biased switch to increase performance of linear charge pumps. Int. J. Circuit Theory Appl. 2020, 48, 1864–1872. [Google Scholar] [CrossRef]
- Stauth, J.T.; Seeman, M.D.; Kesarwani, K. Resonant switched-capacitor converters for sub-module distributed photovoltaic power management. IEEE Trans. Power Electron. 2013, 28, 1189–1198. [Google Scholar] [CrossRef]
- Ballo, A.; Bottaro, M.; Grasso, A.D.; Palumbo, G. Regulated charge pumps: A comparative study by means of verilog-AMS. Electronics 2020, 9, 998. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G.; Tanzawa, T. Linear distribution of capacitance in Dickson charge pumps to reduce rise time. Int. J. Circuit Theory Appl. 2020, 48, 555–566. [Google Scholar] [CrossRef]
- Shams, I.; Mekhilef, S.; Tey, K.S. Advancement of voltage equalizer topologies for serially connected solar modules as partial shading mitigation technique: A comprehensive review. J. Clean. Prod. 2021, 285, 124824. [Google Scholar] [CrossRef]
- Ajami, A.; Ardi, H.; Farakhor, A. A Novel High Step-up DC/DC Converter Based on Integrating Coupled Inductor and Switched-Capacitor Techniques for Renewable Energy Applications. IEEE Trans. Power Electron. 2015, 30, 4255–4263. [Google Scholar] [CrossRef]
- Tang, Y.; Fu, D.; Wang, T.; Xu, Z. Hybrid switched-inductor converters for high step-up conversion. IEEE Trans. Ind. Electron. 2015, 62, 1480–1490. [Google Scholar] [CrossRef]
- Changchien, S.K.; Liang, T.J.; Chen, J.F.; Yang, L.S. Step-up DC-DC converter by coupled inductor and voltage-lift technique. IET Power Electron. 2010, 3, 369–378. [Google Scholar] [CrossRef] [Green Version]
- Sizkoohi, H.M.; Milimonfared, J.; Taheri, M.; Salehi, S. High step-up soft-switched dual-boost coupled-inductor-based converter integrating multipurpose coupled inductors with capacitor-diode stages. IET Power Electron. 2015, 8, 1786–1797. [Google Scholar] [CrossRef]
- Hu, X.; Gong, C. A high voltage gain DC-DC converter integrating coupled-inductor and diode-capacitor techniques. IEEE Trans. Power Electron. 2014, 29, 789–800. [Google Scholar] [CrossRef]
- Young, C.M.; Wu, S.F.; Chen, M.H.; Chen, S.J. Single-phase ac to high-voltage dc converter with soft-switching and diode-capacitor voltage multiplier. IET Power Electron. 2014, 7, 1704–1713. [Google Scholar] [CrossRef]
- Liang, T.J.; Chen, S.M.; Yang, L.S.; Chen, J.F.; Ioinovici, A. Ultra-large gain step-up switched-capacitor DC-DC converter with coupled inductor for alternative sources of energy. IEEE Trans. Circuits Syst. I Regul. Pap. 2012, 59, 864–874. [Google Scholar] [CrossRef]
- Saravanan, S.; Babu, N.R. Analysis and implementation of high step-up DC-DC converter for PV based grid application. Appl. Energy 2017, 190, 64–72. [Google Scholar] [CrossRef]
- Peng, F.Z. Z-source inverter. IEEE Trans. Ind. Appl. 2003, 39, 504–510. [Google Scholar] [CrossRef]
- Evran, F.; Aydemir, M.T. Z-source-based isolated high step-up converter. IET Power Electron. 2013, 6, 117–124. [Google Scholar] [CrossRef]
- Sharifi, S.; Monfared, M. Modified Series and Tapped Switched-Coupled-Inductors Quasi-Z-Source Networks. IEEE Trans. Ind. Electron. 2019, 66, 5970–5978. [Google Scholar] [CrossRef]
- Zhang, G.; Zhang, B.; Li, Z.; Qiu, D.; Yang, L.; Halang, W.A. A 3-Z-network boost converter. IEEE Trans. Ind. Electron. 2015, 62, 278–288. [Google Scholar] [CrossRef]
- Torkan, A.; Ehsani, M. A Novel Nonisolated Z-Source DC-DC Converter for Photovoltaic Applications. IEEE Trans. Ind. Appl. 2018, 54, 4574–4583. [Google Scholar] [CrossRef]
- Krishna, M.V.; Varma, L.T.V.; Kumar, M.A. Simulation Of High Step up Z-Source DC-DC Converter With Voltage Multiplier Using Coupled Inductors. IJRAR-Int. J. Res. Anal. Rev. 2018, 5, 594–598. [Google Scholar]
- MHaji-Esmaeili, M.; Babaei, E.; Sabahi, M. High Step-Up Quasi-Z Source DC-DC Converter. IEEE Trans. Power Electron. 2018, 33, 10563–10571. [Google Scholar] [CrossRef]
- Shen, H.; Zhang, B.; Qiu, D. Hybrid Z-Source Boost DC-DC Converters. IEEE Trans. Ind. Electron. 2017, 64, 310–319. [Google Scholar] [CrossRef]
- Zhang, G.; Iu, H.H.-C.; Zhang, B.; Li, Z.; Fernando, T.; Chen, S.-Z.; Zhang, Y. An Impedance Network Boost Converter with a High-Voltage Gain. IEEE Trans. Power Electron. 2017, 32, 6661–6665. [Google Scholar] [CrossRef]
- Florescu, A.; Stocklosa, O.; Teodorescu, M.; Radoi, C.; Stoichescu, D.A.; Rosu, S. The advantages, limitations and disadvantages of Z-source inverter. Proc. Int. Semicond. Conf. CAS 2010, 2, 483–486. [Google Scholar] [CrossRef]
- Subhani, N.; Kannan, R.; Porkumaran, K.; Prasath, S.; Srinath, M. An Improved Modified Capacitor-Assisted Z-Source Inverter with Reduced Capacitor Voltage Stress and Inrush Start-up Current. In Proceedings of the 2019 IEEE Conference on Energy Conversion (CENCON), Yogyakarta, Indonesia, 16–17 October 2019; pp. 182–187. [Google Scholar] [CrossRef]
- Subhani, N.; Kannan, R.; Mahmud, A. New Symmetric Enhanced-Boost Modified Z-Source Inverters with Switched Z-Impedance. In Proceedings of the 2020 IEEE International Conference on Power Electronics, Smart Grid and Renewable Energy (PESGRE2020), Cochin, India, 2–4 January 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Kumar, R.; Kannan, R.; Nor, N.B.M.; Mahmud, A. A high step-up switched z-source converter (Hs-szc) with minimal components count for enhancing voltage gain. Electronics 2021, 10, 924. [Google Scholar] [CrossRef]
- Kumar, R.; Kannan, R.; Bin, N.; Nor, M. A High Gain Switched Z-Source Converter with Reduced Passive Components. Solid State Technol. 2020, 63, 4160–4170. [Google Scholar]
- Kandasamy, K.V. Regulated DC-DC Converter for Grid Connected System during Hazardous Conditions. In Proceedings of the 2017 International Conference on Algorithms, Methodology, Models and Applications in Emerging Tech-nologies (ICAMMAET), Chennai, India, 16–18 February 2017. [Google Scholar]
- Aboadla, E.H.E.; Khan, S.; Habaebi, M.H.; Gunawan, T.; Hamidah, B.A.; Yaacob, M.B. Effect of Modulation Index of Pulse Width Modulation Inverter on Total Harmonic Distortion for Sinusoidal. In Proceedings of the 2016 International Conference on Intelligent Systems Engineering (ICISE), Islamabad, Pakistan, 15–17 January 2016. [Google Scholar]
- Zope, P.H.; Bhangale, P.G.; Sonare, P.; Suralkar, S.R. Design and Implementation of carrier based Sinusoidal PWM Inverter. Int. J. Adv. Res. Electr. Electron. Instrum. Eng. 2012, 1, 230–236. [Google Scholar]
- Ramesh, V.; Latha, Y.K. Performance improvement of grid connected PV system using new converter topologies. In Proceedings of the 2017 2nd IEEE International Conference on Electrical, Computer and Communication Technologies, ICECCT, Tamil Nadu, India, 22–24 February 2017. [Google Scholar] [CrossRef]
- Mawlikar, M.A.; Nair, S.S. A comparative analysis of Z source inverter and DC-DC converter fed VSI. In Proceedings of the 2017 International Conference on Nascent Technologies in Engineering (ICNTE), Vashi, India, 27–28 January 2017; pp. 1–6. [Google Scholar] [CrossRef]







| Converter Parameters | Inverter Parameters | ||
|---|---|---|---|
| L1–3 | 330 µH | fCr | 3000 Hz |
| C1–3 | 320 µF | Lf | H |
| VI | 35 V | Cf | F |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumar, R.; Kannan, R.; Singh, N.S.S.; Abro, G.E.M.; Mathur, N.; Baba, M. An Efficient Design of High Step-Up Switched Z-Source (HS-SZSC) DC-DC Converter for Grid-Connected Inverters. Electronics 2022, 11, 2440. https://doi.org/10.3390/electronics11152440
Kumar R, Kannan R, Singh NSS, Abro GEM, Mathur N, Baba M. An Efficient Design of High Step-Up Switched Z-Source (HS-SZSC) DC-DC Converter for Grid-Connected Inverters. Electronics. 2022; 11(15):2440. https://doi.org/10.3390/electronics11152440
Chicago/Turabian StyleKumar, Rahul, Ramani Kannan, Narinderjit Singh Sawaran Singh, Ghulam E. Mustafa Abro, Nirbhay Mathur, and Maveeya Baba. 2022. "An Efficient Design of High Step-Up Switched Z-Source (HS-SZSC) DC-DC Converter for Grid-Connected Inverters" Electronics 11, no. 15: 2440. https://doi.org/10.3390/electronics11152440
APA StyleKumar, R., Kannan, R., Singh, N. S. S., Abro, G. E. M., Mathur, N., & Baba, M. (2022). An Efficient Design of High Step-Up Switched Z-Source (HS-SZSC) DC-DC Converter for Grid-Connected Inverters. Electronics, 11(15), 2440. https://doi.org/10.3390/electronics11152440








