Multi-Physics Coupling Simulation Technique for Phase Stable Cables
Abstract
:1. Introduction
2. Theories of Multiple Physical Fields and Their Couplings
2.1. Electromagnetic Field
2.2. Thermal Flow Field
2.3. Thermal Mechanics Field
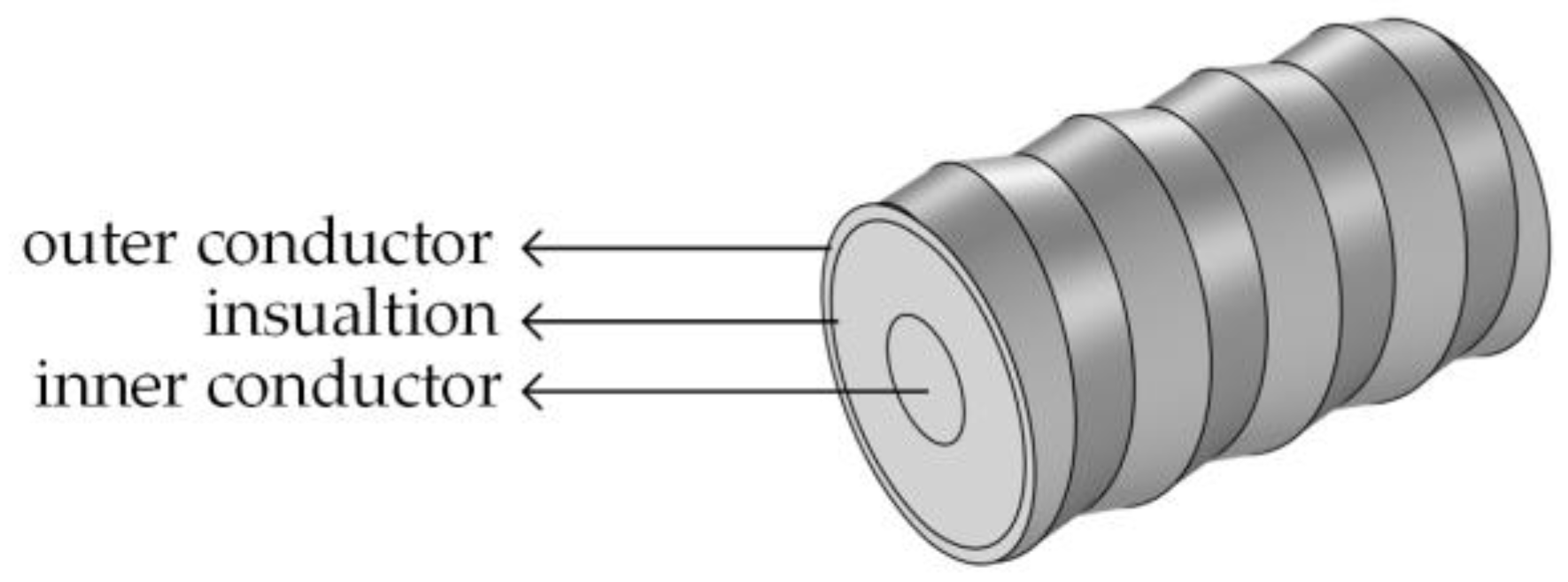
3. Modelling of Corrugated Phase Stable Cable
3.1. Geometric Model
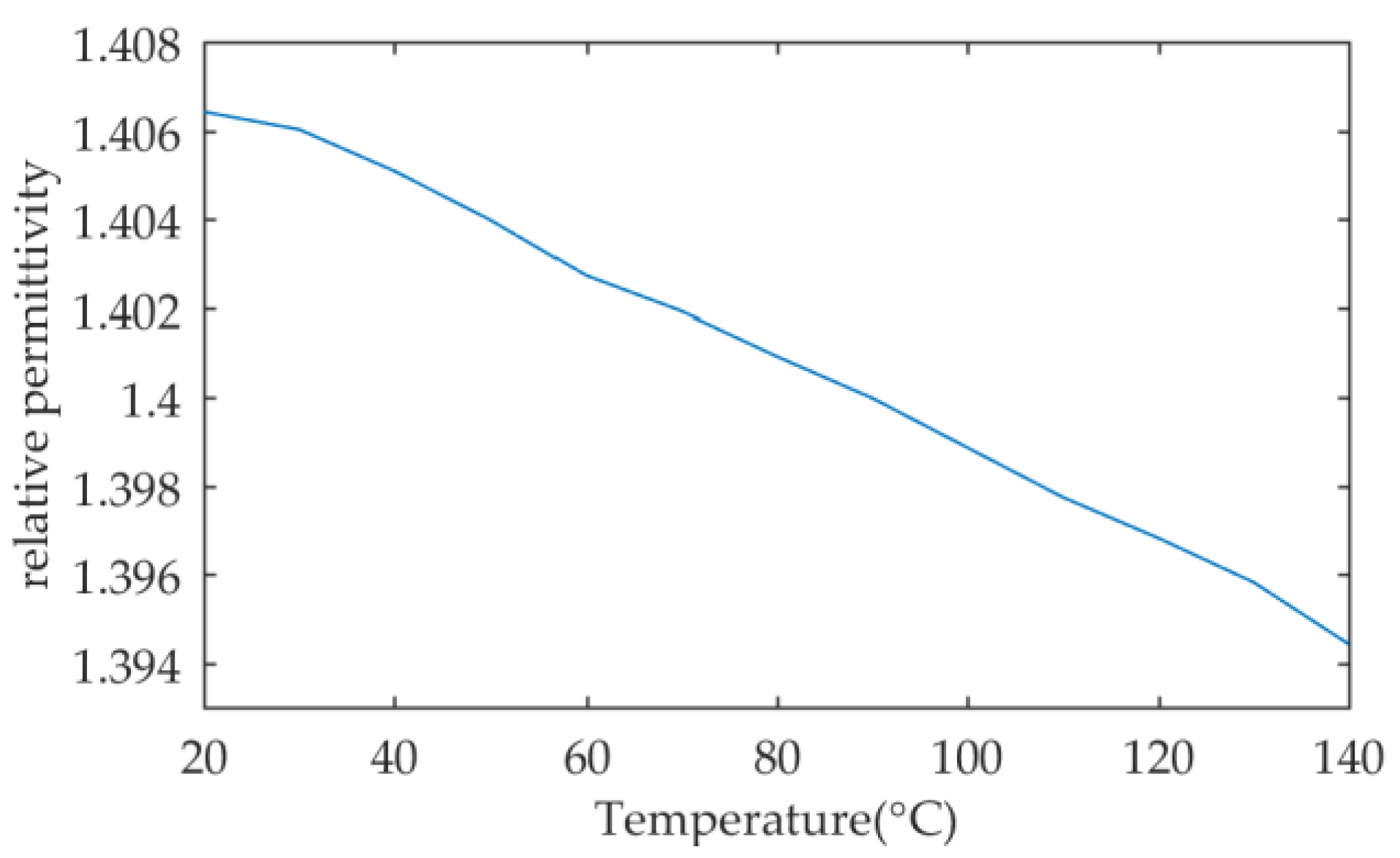
3.2. Physical Model
- As the skin depth is far less than radii and thicknesses of the conductors at frequencies in interest, the Joule heat dissipated from the conductors can be regarded to yield only on the laminas proximate to the insulation;
- The contact thermal resistance between the insulation and the conductors is ignored and the radiation heat transfer of the outer surface of the cable is ignored;
- The surrounding air has a temperature of 20 °C and the temperature of the upstream air of natural convection keeps 20 °C;
- The velocity of the surrounding air is limited and the flow mode is laminar flow;
- The surrounding air is regarded as viscous incompressible fluid, with a Mach number lower than 0.3;
- The deformation of the cable satisfies the infinitesimal deformation hypothesis such that linear solid mechanics always holds;
- The gravity is ignored when studying the thermal deformation of the cable in solid mechanics.
3.3. Simulation Model
- Calculate the electromagnetic field, the thermal field and, the flow field when transmitting electromagnetic wave of certain power by a frequency stationary study;
- Calculate the thermal deformation (solid mechanics field) of the corrugated cable by a stationary study;
- Calculate the electromagnetic field once again to attain the phase stability of the cable by a frequency domain study.
4. Analysis of Simulation Results
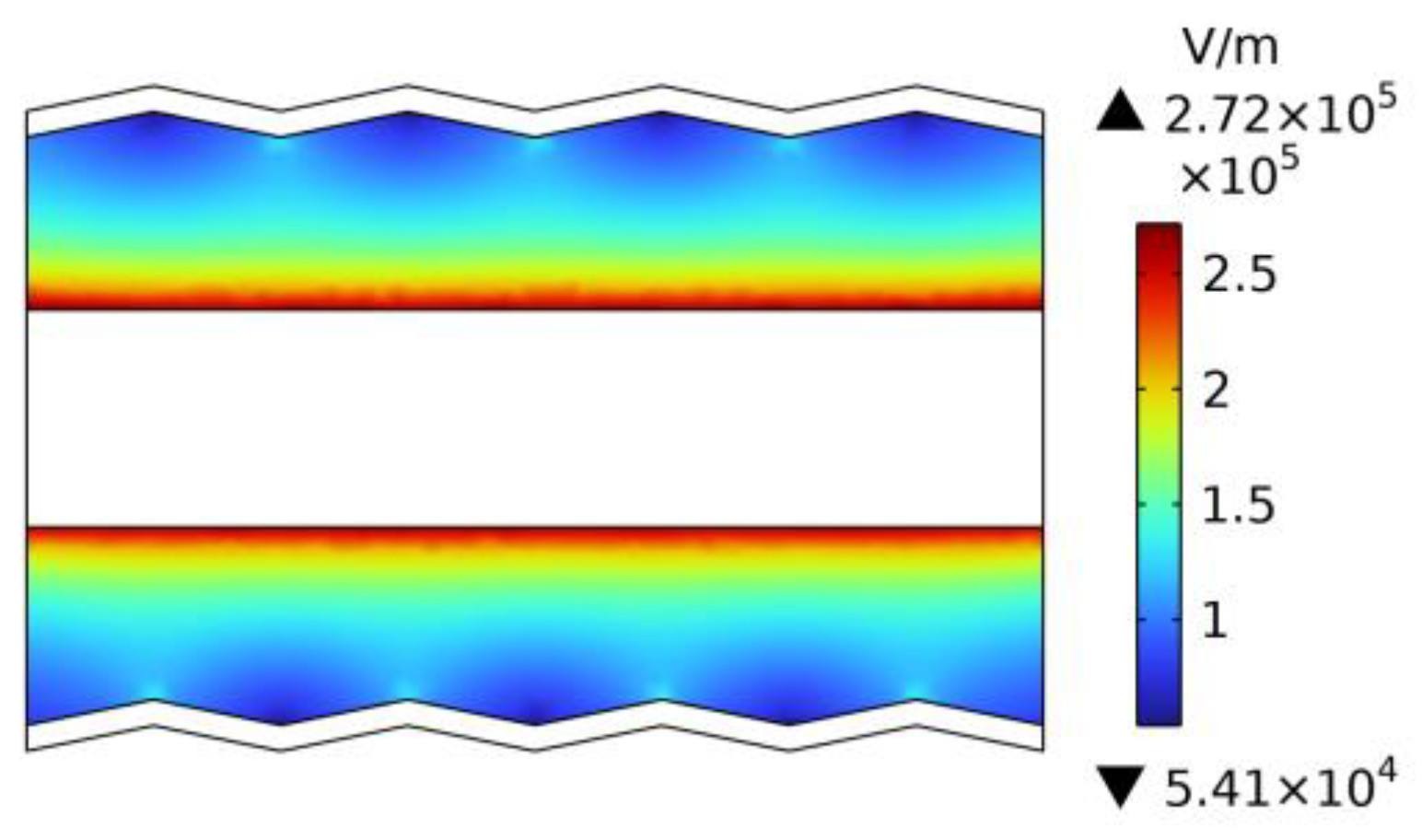
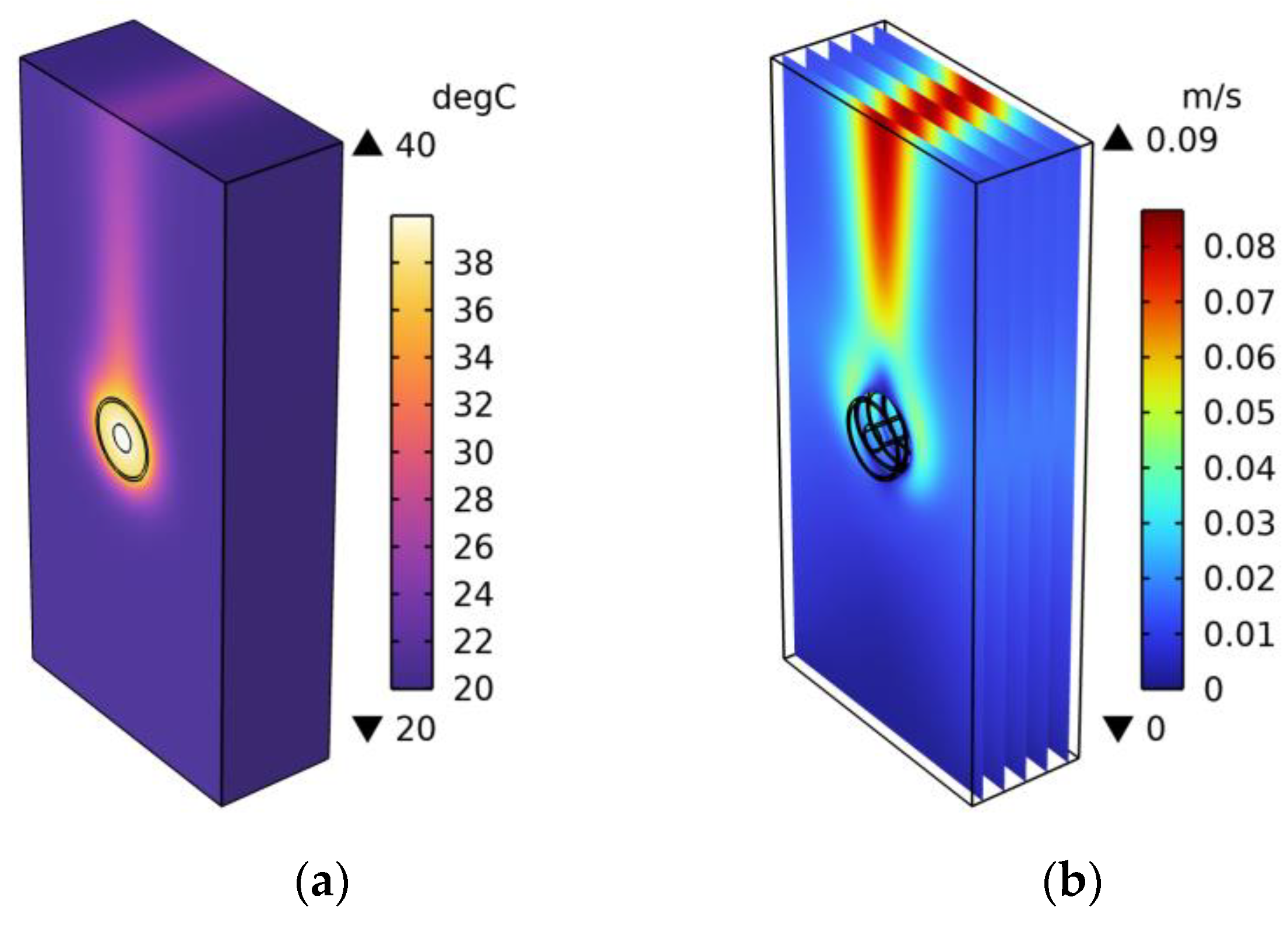
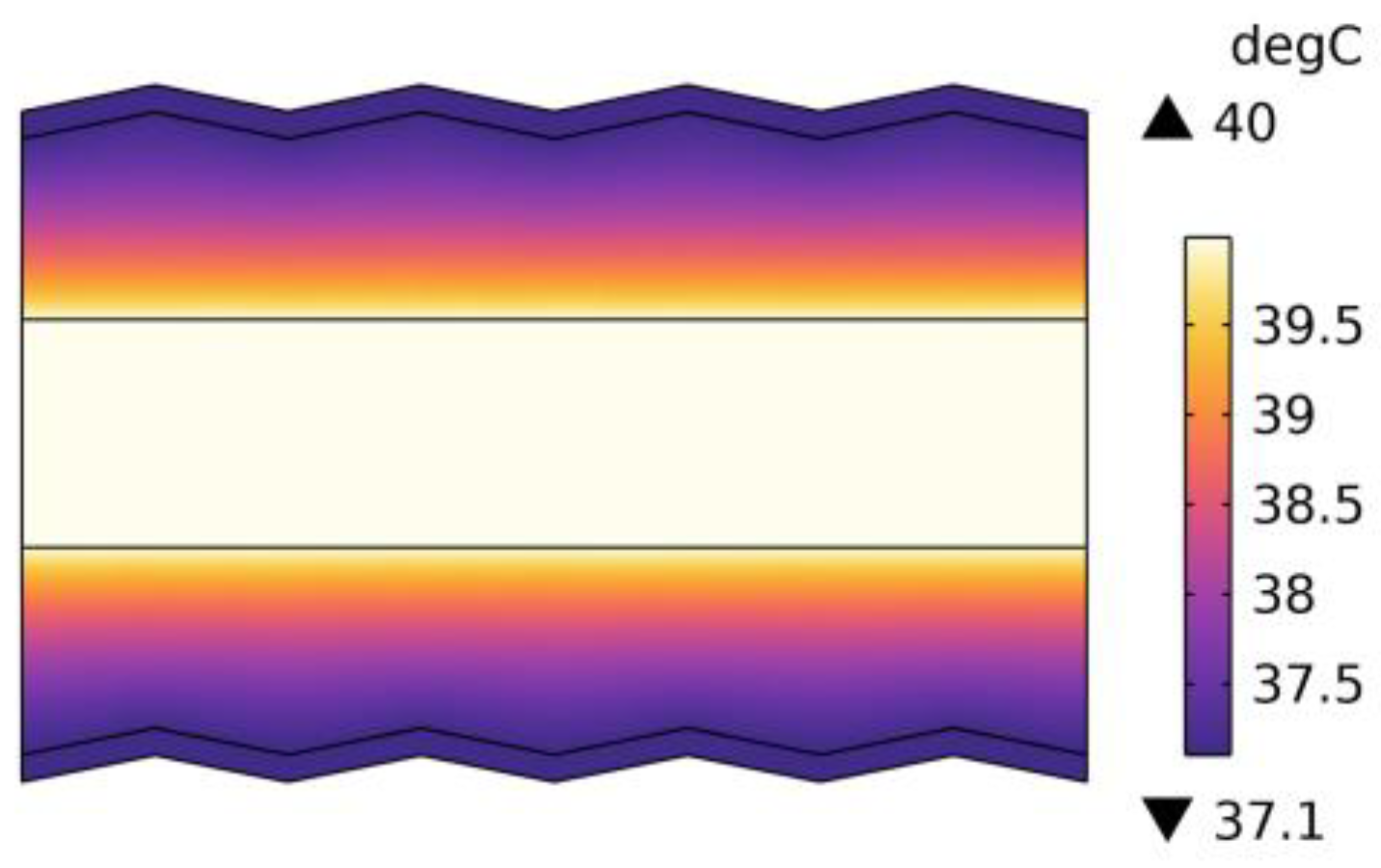
4.1. The Physical Fields
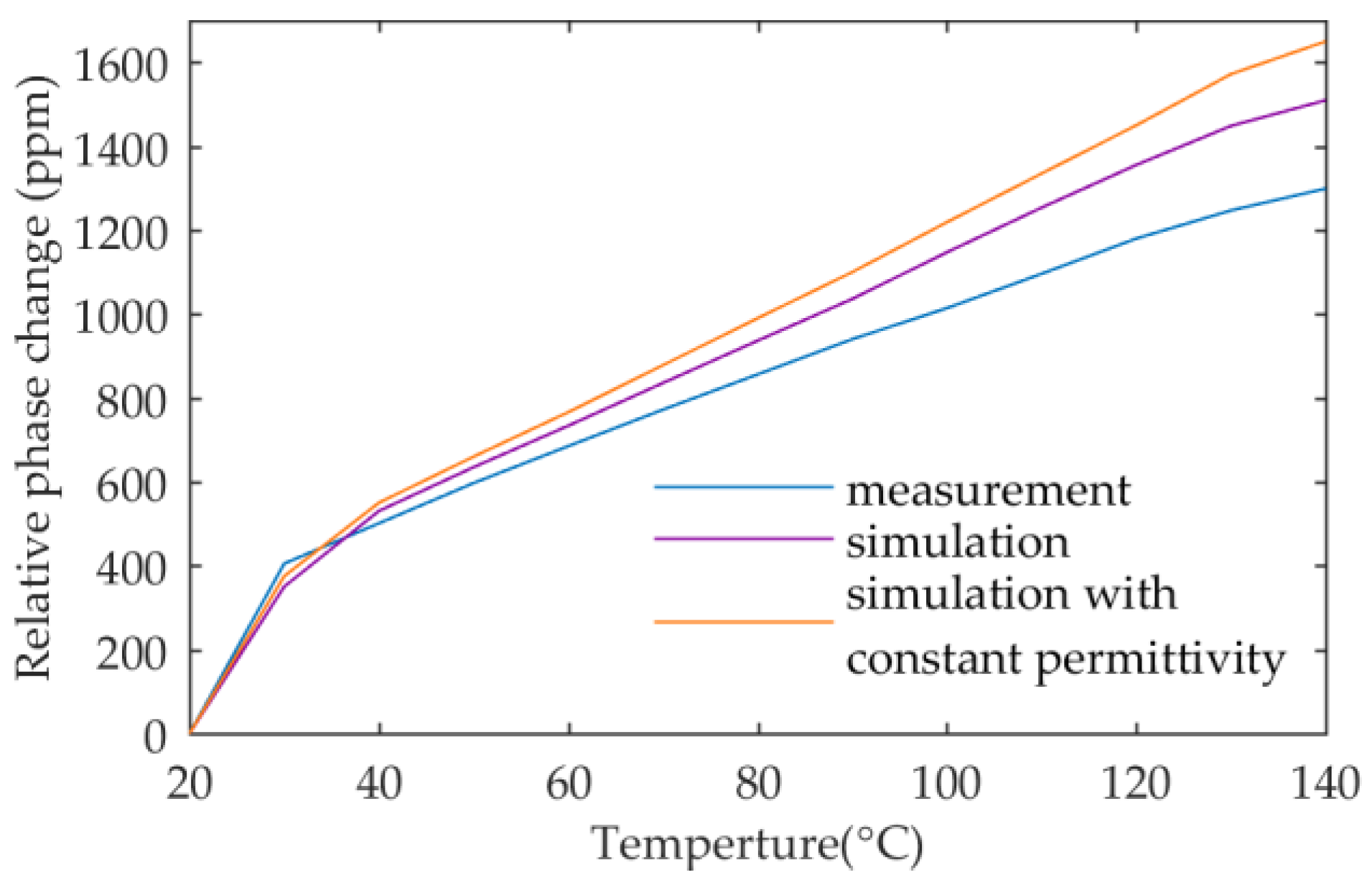
4.2. Phase Stability
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hejc, G.; Schafer, W.; Seidel, A.; Hess, M.-P.; Kehrer, J.; Santarelli, G.; de Vicente, J. Frequency Dependency of Phase Stability of RF Cables. In Proceedings of the 2007 IEEE International Frequency Control Symposium Joint with the 21st European Frequency and Time Forum, Geneva, Switzerland, 29 May 2007–1 June 2007; pp. 1309–1311. [Google Scholar]
- Mahiddini, F.; Ridel, M.; Reulet, P.; Donjat, D.; Millan, P. Coupled EMC-Thermal Modeling of Electrical Harnesses Within “More Electrical Aircraft”. In Proceedings of the 30th Congress of the International Council of the Aeronautical Sciences, Daejeon, Korea, 25–30 September 2016. [Google Scholar]
- Yang, Y.; Wang, Q.; Liu, Z. Simulation of Underground Cable Temperature Distribution Based on Multiphysics Modeling. In Proceedings of the 2021 11th International Conference on Power, Energy and Electrical Engineering (CPEEE), Shiga, Japan, 26–28 February 2021; pp. 26–31. [Google Scholar]
- Dai, D.; Hu, M.; Luo, L. Calculation of Thermal Distribution and Ampacity for Underground Power Cable System by Using Electromagnetic-Thermal Coupled Model. In Proceedings of the 2014 IEEE Electrical Insulation Conference (EIC), Philadelphia, PA, USA, 8–11 June 2014; pp. 303–306. [Google Scholar]
- Chen, W.; Zhou, M.; Yan, X.; Li, Z.; Lin, X. Study on Electromagnetic-Fluid-Temperature Multiphysics Field Coupling Model for Drum of Mine Cable Winding Truck. CES Trans. Electr. Mach. Syst. 2021, 5, 133–142. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, X.; Zhang, H.; Liu, J.; Zhang, C.; Jiao, J. Analysis on the Temperature Field and the Ampacity of XLPE Submarine HV Cable Based on Electro-Thermal-Flow Multiphysics Coupling Simulation. Polymers 2020, 12, 952. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bhatti, A.A.; Peng, X.; Yang, B.; Xie, C. Effect of Void on Temperature Distribution in XLPE Power Cables by COMSOL Multiphysics Simulation. In Proceedings of the 2021 IEEE 4th International Electrical and Energy Conference (CIEEC), Wuhan, China, 28–30 May 2021; pp. 1–4. [Google Scholar]
- Xia, Z.; Bhatti, A.A.; Peng, X.; Yang, B.; Zhou, H.; Zhao, C.; Xie, C. Electric Field and Temperature Distribution of High Voltage Cables with the Addition of Particles Based on COMSOL Simulation. In Proceedings of the 2020 IEEE International Conference on High Voltage Engineering and Application (ICHVE), Beijing, China, 6–10 September 2020; pp. 1–4. [Google Scholar]
- Orfanidis, S.J. Electromagnetic Waves and Antennas; Sophocles J. Orfanidis: Roma, Italy, 1999. [Google Scholar]
- Yang, S.; Tao, W. Heat Transfer, 3rd ed.; Northwest University of Technology Press: Xi’an, China, 2006. [Google Scholar]
- Chorin, A.; Marsden, J.E. A Mathematical Introduction to Fluid Mechanics; Springer: New York, NY, USA, 1992; ISBN 0-387-97300-1. [Google Scholar]
- Gorman, D.G.; Kennedy, W. Applied Solid Dynamics; Butterworth & Co. (Publishers) Ltd.: Sutton, Surrey, UK, 1988; ISBN 0-408-02309-0. [Google Scholar]
- Othman, M.; Lotfy, K. Two-Dimensional Problem of Generalized Magneto-Thermoelasticity with Temperature Dependent Elastic Moduli for Different Theories. Multidiscip. Model. Mater. Struct. 2009, 5, 235–242. [Google Scholar] [CrossRef]



| Notation | Meaning | Value |
|---|---|---|
| Rin | Radius of the inner conductor | 1.28 mm |
| Rout | Minimum inside radius of the outer conductor | 3.32 mm |
| h | Maximum difference of the outer conductor inside radius | 0.3 mm |
| l | Pitch of the corrugation | 3 mm |
| tout | Thickness of the outer conductor along the radial direction | 0.3 mm |
| Material | Copper | ePTFE | Air |
|---|---|---|---|
| Relative permittivity | 1 | εrP(T) | — |
| Relative permeability | 1 | 1 | — |
| Conductivity at 25 °C | 6 × 107 | — | — |
| Temperature coefficient of resistibility (K−1) | 3.8 × 10−3 | — | — |
| Dissipation factor | — | 1 × 10−4 | — |
| Density at 20 °C (kg∙m−3) | 8960 | 2180 | 1.24 |
| Average molar mass (g∙mol−1) | — | — | 29 |
| Thermal conductivity (W∙m−1∙K−1) | 400 | 0.24 | 0.027 |
| Specific heat capacity (J∙kg−1∙K−1) | 385 | 1000 | 1010 |
| Thermal expansion coefficient (K−1) | 1.7 × 10−5 | 1 × 10−4 | — |
| Young’s modulus (Pa) | 1.1 × 1011 | 4 × 108 | — |
| Poisson’s ratio | 0.35 | 0.46 | — |
| Kinematic viscosity (Pa∙s) | — | — | 4 × 10−5 |
| EMW Power (W) | Temperature (°C) | EMW Power (W) | Temperature (°C) |
|---|---|---|---|
| 61 | 30 | 519 | 90 |
| 100 | 40 | 602 | 100 |
| 185 | 50 | 684 | 110 |
| 270 | 60 | 752 | 120 |
| 354 | 70 | 827 | 130 |
| 435 | 80 | 897 | 140 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Chen, X.; Yang, D.; Wang, L.; He, X.; Zhang, Z. Multi-Physics Coupling Simulation Technique for Phase Stable Cables. Electronics 2023, 12, 1602. https://doi.org/10.3390/electronics12071602
Zhang G, Chen X, Yang D, Wang L, He X, Zhang Z. Multi-Physics Coupling Simulation Technique for Phase Stable Cables. Electronics. 2023; 12(7):1602. https://doi.org/10.3390/electronics12071602
Chicago/Turabian StyleZhang, Gang, Xiao Chen, Dazhi Yang, Lixin Wang, Xin He, and Zhehao Zhang. 2023. "Multi-Physics Coupling Simulation Technique for Phase Stable Cables" Electronics 12, no. 7: 1602. https://doi.org/10.3390/electronics12071602
APA StyleZhang, G., Chen, X., Yang, D., Wang, L., He, X., & Zhang, Z. (2023). Multi-Physics Coupling Simulation Technique for Phase Stable Cables. Electronics, 12(7), 1602. https://doi.org/10.3390/electronics12071602







