Efficient Strategies for Computing Euler Number of a 3D Binary Image
Abstract
:1. Introduction
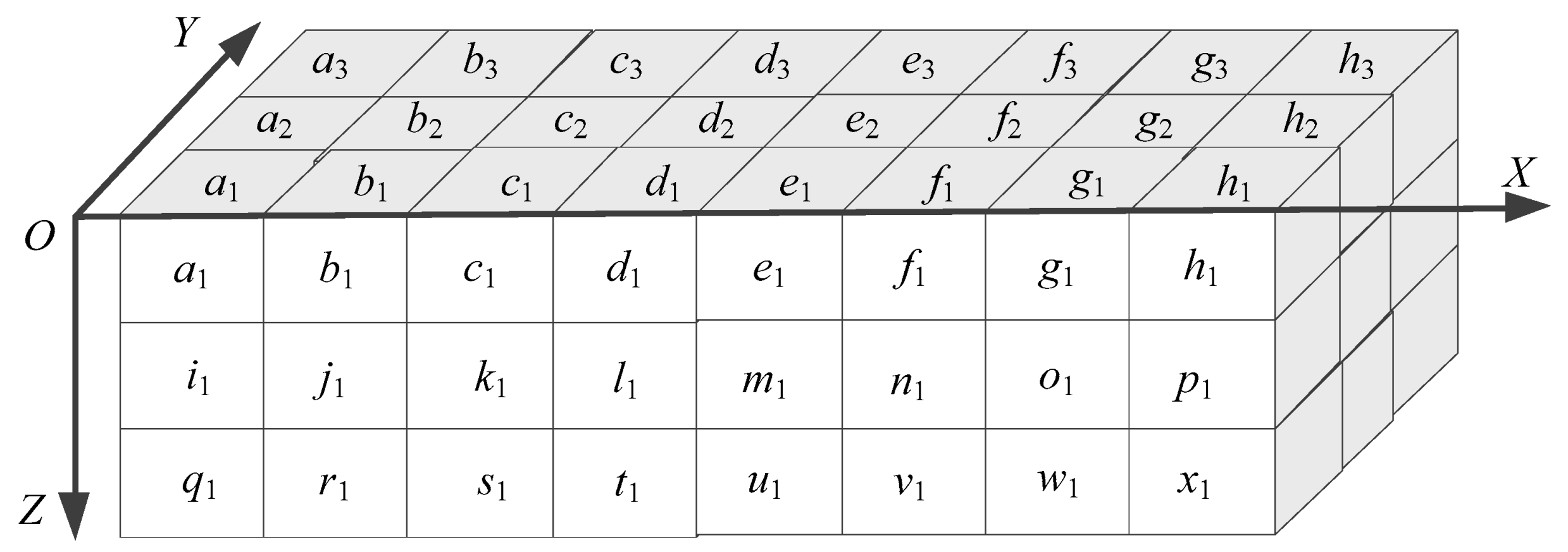
2. Reviews of Conventional Voxel-Pattern-Based Euler Number Computing Algorithm for a 3D Image
- Ψ1 = #[1];
- Ψ2 = #[2] + #[3] + #[4];
- Ψ3 = #[5] + #[6] + #[7];
- Ψ4 = #[8] + #[9] + #[10] + #[11] + #[12] + #[13] + #[14];
- Ψ5 = #[15] + #[16] + #[17];
- Ψ6 = #[18] + #[19] + #[20];
- Ψ7 = #[21];
- Ψ8 = #[22].
3. Our Proposed Strategies for Improving the VP Algorithm
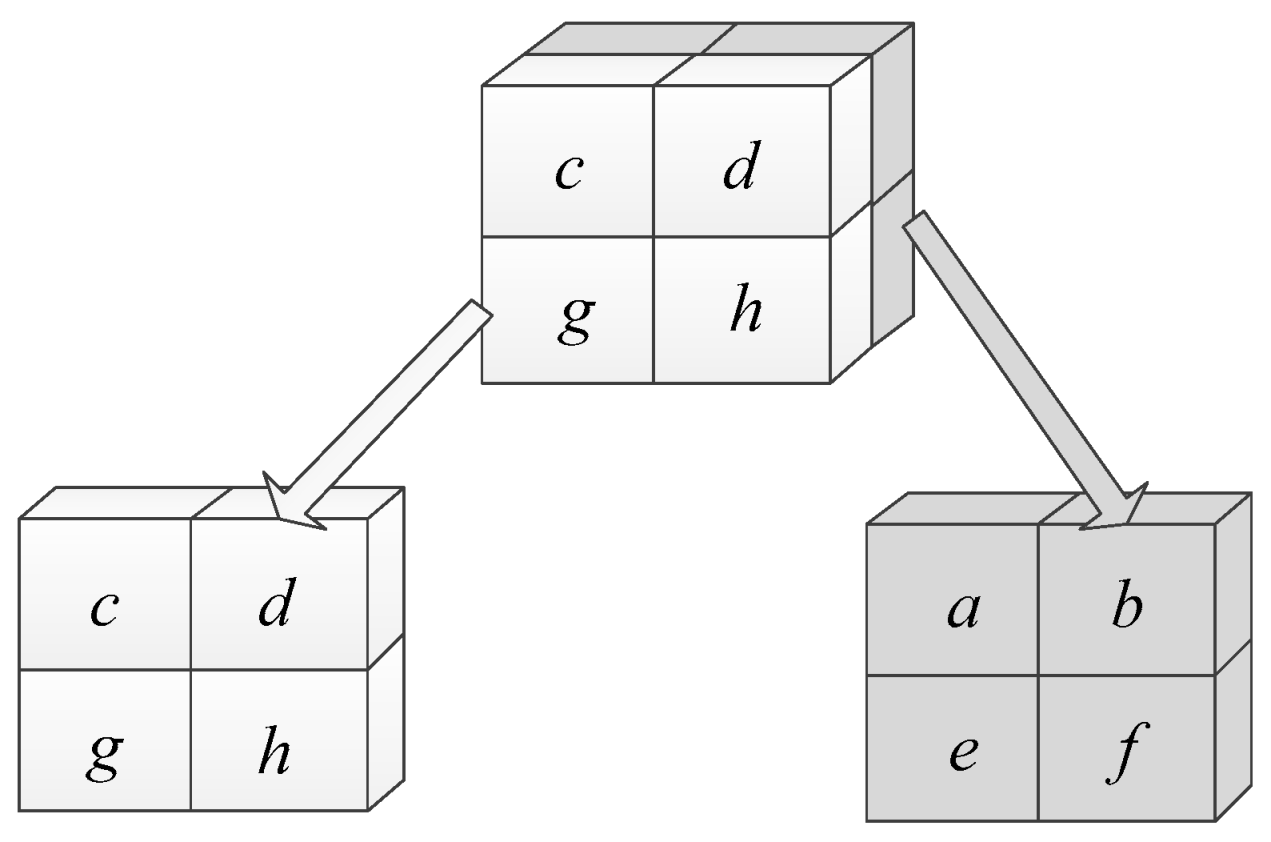
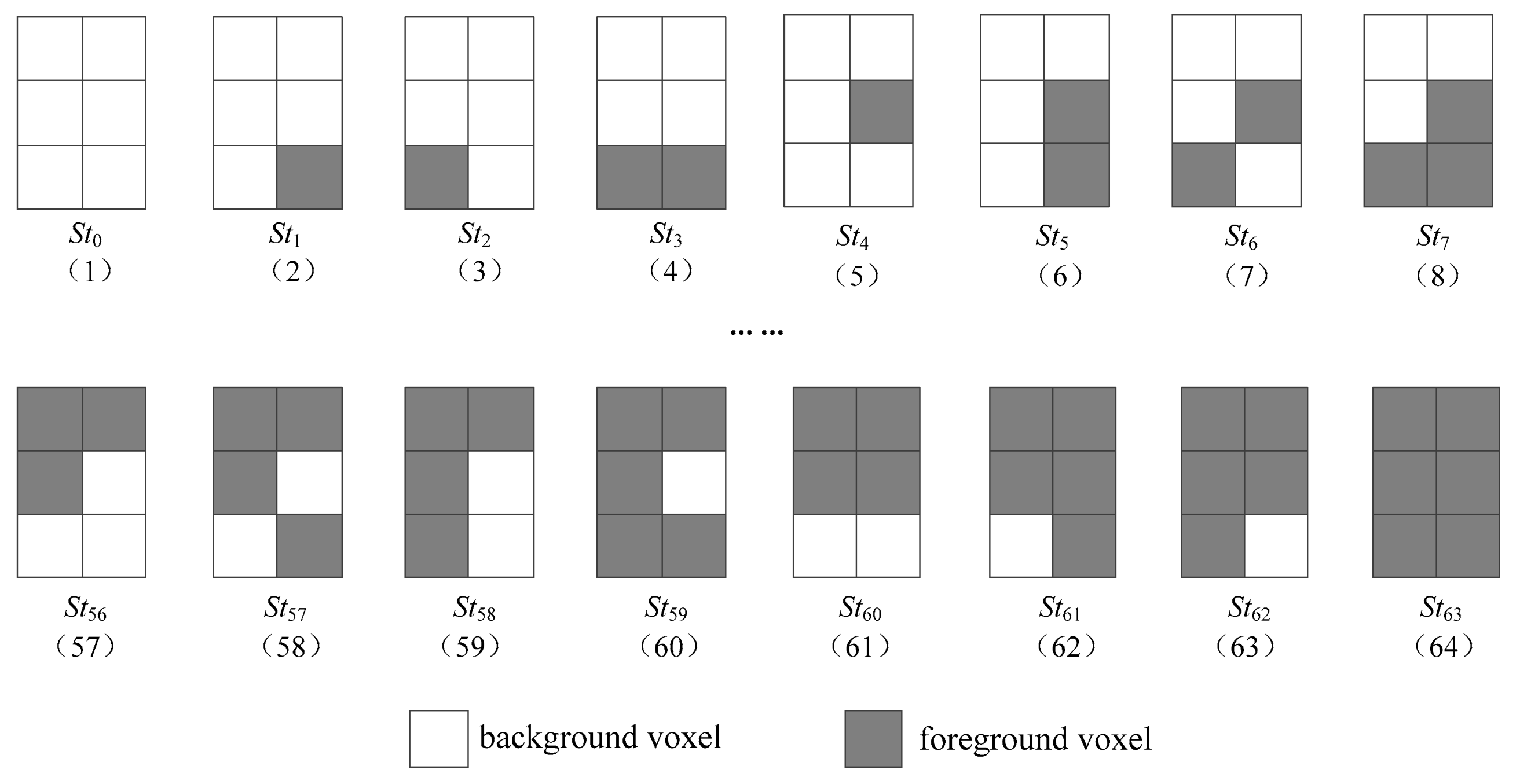
3.1. Strategy of State Transition
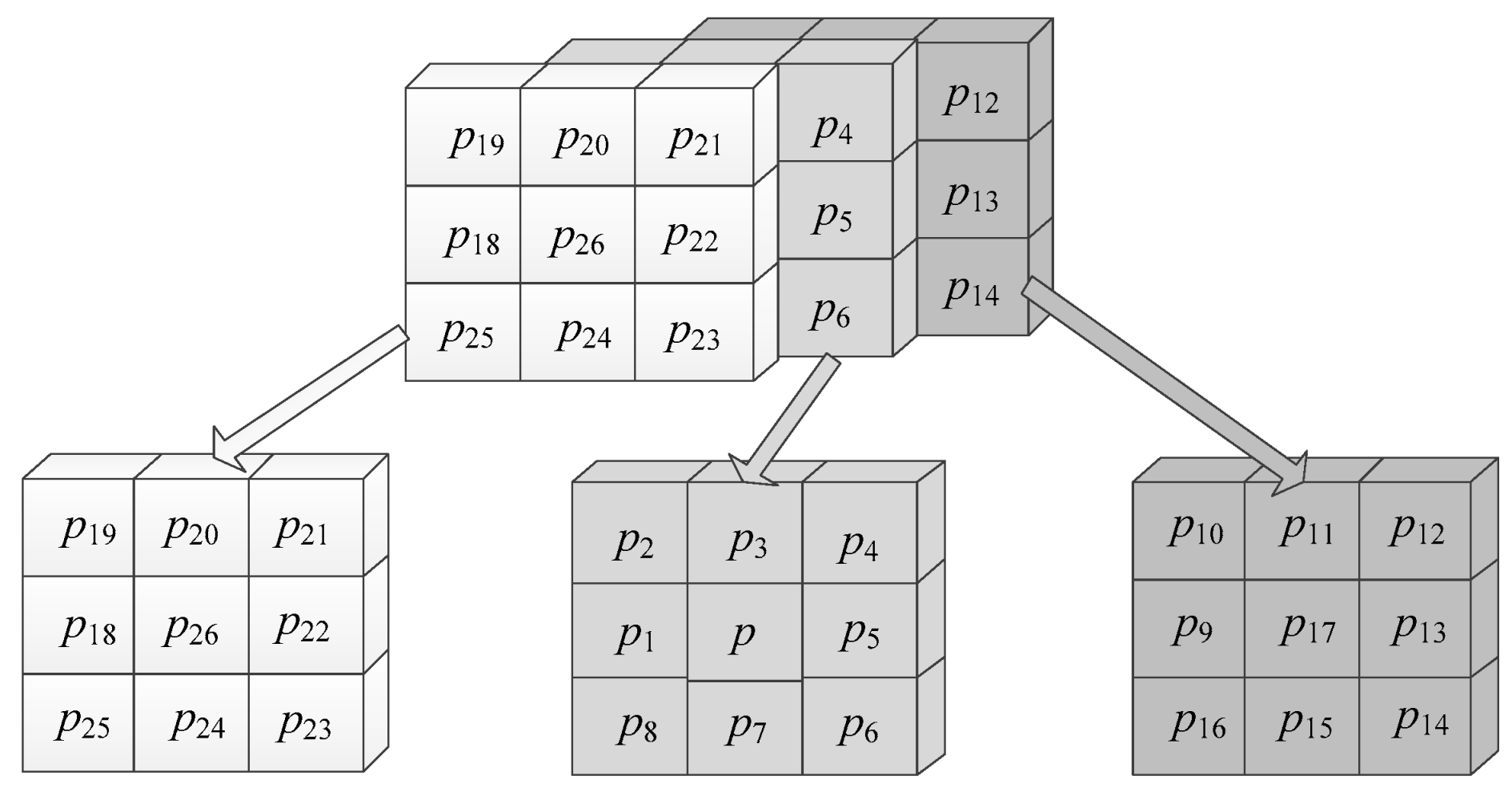
3.2. Scanning Every Two Rows and Processing Two Voxel Patterns Simultaneously
3.3. Strategy of Changing the Order of Accessing Voxels and Combining Similar Voxel Patterns
4. Experimental Results
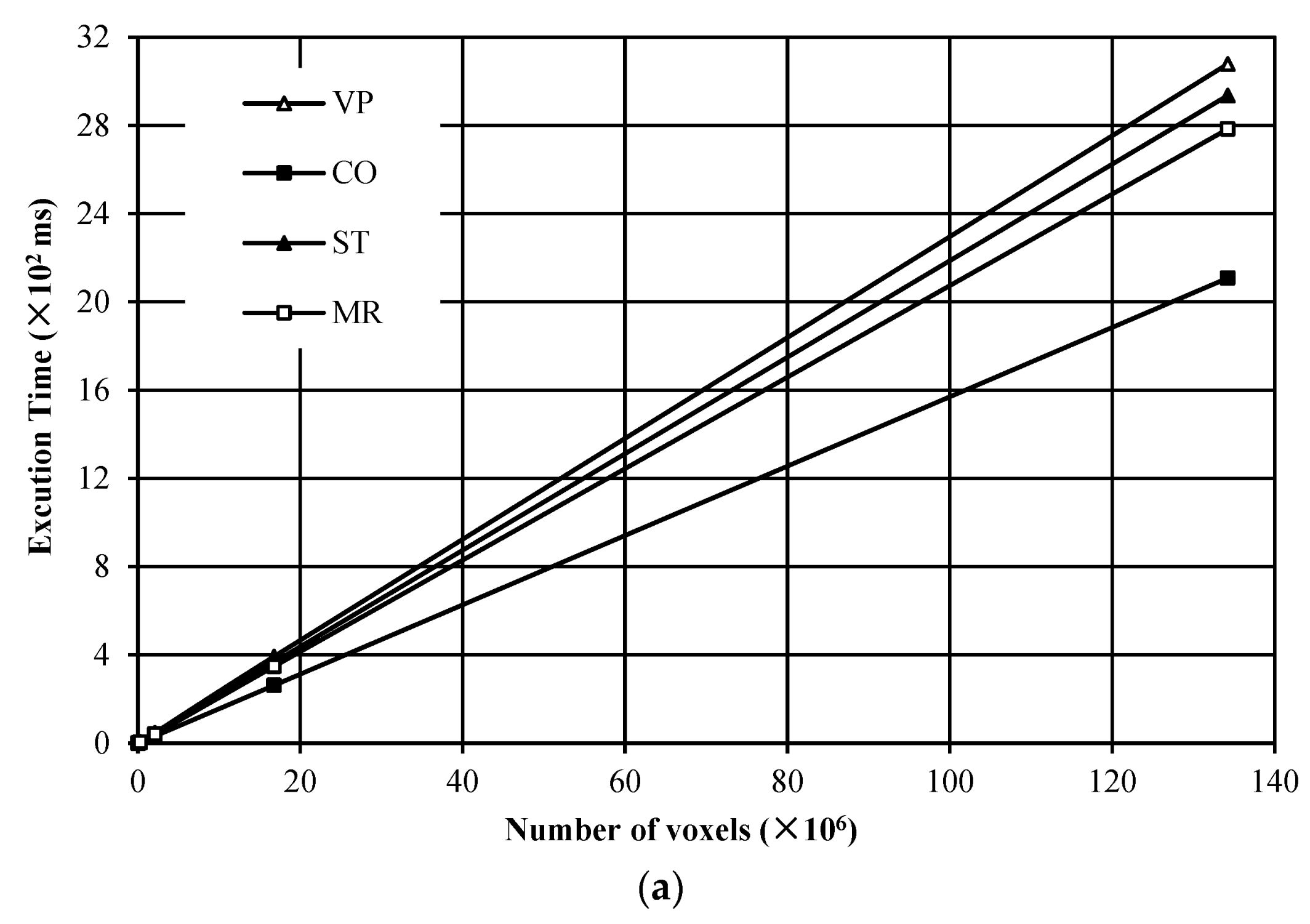
4.1. Execution Time versus the Number of Voxels in the Image
4.2. Comparison of the Number of Accessed Voxels in the Compared Algorithms
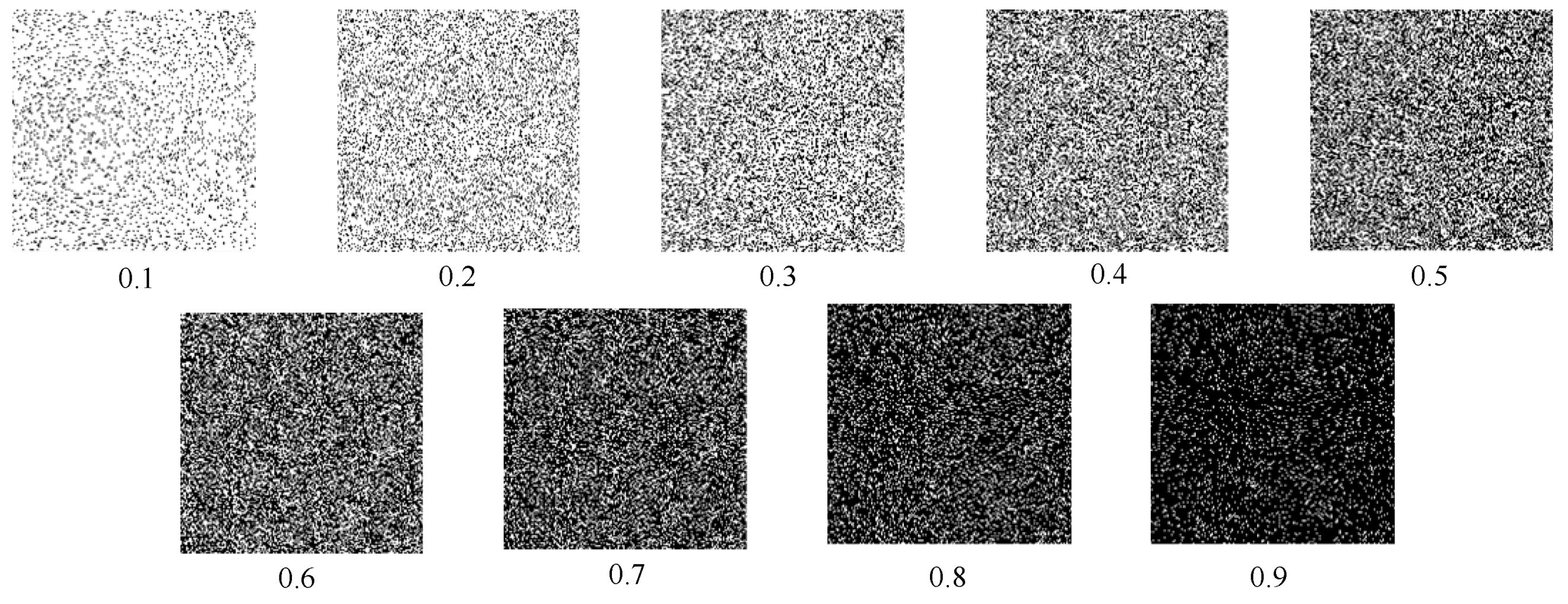
4.3. Execution Time versus Image Densities
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tekli, J. An overview of cluster-based image search result organization: Background, techniques, and ongoing challenges. Knowl. Inf. Syst. 2022, 64, 589–642. [Google Scholar] [CrossRef]
- Hashizume, A.; Suzuki, R.; Yokouchi, H.; Horiuchi, H.; Yamamoto, S. An algorithm of automated RBC classification and its evaluation. Bio Med. Eng. 1990, 28, 25–32. [Google Scholar]
- Nayar, S.K.; Bolle, R.M. Reflectance-based object recognition. Int. J. Comput. Vis. 1996, 17, 219–240. [Google Scholar] [CrossRef]
- Liu, Y.F.; Cho, S.; Spencer, B., Jr.; Fan, J.S. Concrete crack assessment using digital image processing and 3D scene reconstruction. J. Comput. Civ. Eng. 2016, 30, 04014124. [Google Scholar] [CrossRef]
- Bribiesca, E. Computation of the Euler number using the contact perimeter. Comput. Math. Appl. 2010, 60, 1364–1373. [Google Scholar] [CrossRef] [Green Version]
- Sossa, H.; Cuevas, E.; Zaldivar, D. Alternative way to compute the Euler number of a binary image. J. Appl. Res. Technol. 2011, 9, 335–341. [Google Scholar]
- Santiago, R.; López, A.; Ayala, A.P.; Jimenez, E.C.; Espino, E.R.; Juan, H. Alternative formulations to compute the binary shape Euler number. IET Comput. Vis. 2014, 8, 171–181. [Google Scholar]
- Gray, S.B. Local properties of binary images in two dimensions. IEEE Trans. Comput. 1971, C-20, 551–561. [Google Scholar] [CrossRef]
- Yao, B.; He, L.; Kang, S.; Zhao, X.; Chao, Y. Bit-quad-based Euler number computing. IEICE Trans. Inf. Syst. 2017, E100-D, 2197–2204. [Google Scholar] [CrossRef] [Green Version]
- Bishnu, A.; Bhattacharya, B.; Kundu, M.K.; Murthy, C.A.; Acharya, T. A pipeline architecture for computing the Euler number of a binary image. J. Syst. Archit. 2005, 51, 470–487. [Google Scholar] [CrossRef] [Green Version]
- Yao, B.; He, L.; Kang, S.; Zhao, X.; Chao, Y. A new run-based algorithm for Euler number computing. Pattern Anal. Appl. 2017, 20, 49–58. [Google Scholar] [CrossRef]
- Chen, M.; Yan, P. A fast algorithm to calculate the Euler number for binary images. Pattern Recognit. Lett. 1988, 8, 295–297. [Google Scholar] [CrossRef]
- Yao, B.; He, L.; Kang, S.; Chao, Y.; Zhao, X. A novel bit-quad-based Euler number computing algorithm. Springerplus 2015, 4, 1–16. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, L.; Chao, Y.; Suzuki, K. An algorithm for connected-component labeling, hole labeling and Euler number computing. J. Comput. Sci. Technol. 2013, 28, 468–478. [Google Scholar] [CrossRef]
- He, L.; Chao, Y. A very fast algorithm for simultaneously performing connected-component labeling and Euler number computing. IEEE Trans. Image Process. 2015, 24, 2725–2735. [Google Scholar]
- Velichko, A.; Holzapfel, C.; Siefers, A.; Schladitz, K.; Mücklich, F. Unambiguous classification of complex microstructures by their three-dimensional parameters applied to graphite in cast iron. Acta Mater. 2008, 56, 1981–1990. [Google Scholar] [CrossRef]
- Vogel, H.J.; Roth, K. Quantitative morphology and network representation of soil pore structure. Adv. Water Resour. 2001, 24, 233–242. [Google Scholar] [CrossRef]
- Park, C.; Rosenfeld, A. Connectivity and Genus in Three Dimensions; Computer Science Center, University of Maryland: College Park, MD, USA, 1971; Technical Report TR-156. [Google Scholar]
- Toriwaki, J.; Yonekura, T. Euler number and connectivity indexes of a three dimensional digital picture. Forma 2002, 17, 183–209. [Google Scholar]
- Akira, N.; Aizawa, K. On the recognition of properties of three-dimensional pictures. IEEE Trans. Pattern Anal. Mach. Intell. 1985, 7, 708–713. [Google Scholar]
- Lee, C.; Poston, T. Winding and Euler numbers for 2D and 3D digital images. Graph. Model. Image Process. 1991, 53, 522–537. [Google Scholar] [CrossRef]
- Saha, P.; Chaudhuri, B. A new approach to computing the Euler characteristic. Pattern Recognit. 1995, 28, 1955–1963. [Google Scholar] [CrossRef]
- Saha, P.; Chaudhuri, B. 3D digital topology under binary transformation with applications. Comput. Vis. Image Underst. 1996, 63, 418–429. [Google Scholar] [CrossRef] [Green Version]
- Lin, X.; Xiang, S.; Gu, Y. A new approach to compute the Euler number of 3D image. In Proceedings of the IEEE Conference on Industrial Electronics and Applications, Singapore, 3–5 June 2008; pp. 1543–1546. [Google Scholar]
- Lin, X.; Ji, J.; Huang, S.; Yang, J. A proof of new formula for 3D images Euler number. Pattern Recognit. Artif. Intell. 2010, 23, 52–58. [Google Scholar]
- Sánchez, H.; Sossa, H.; Braumann, U.-D.; Bribiesca, E. The Euler-Poincaré formula through contact surfaces of voxelized objects. J. Appl. Res. Technol. 2013, 11, 65–78. [Google Scholar] [CrossRef] [Green Version]
- Sossa, H.; Rubío, E.; Ponce, V.; Sánchez, H. Vertex codification applied to 3-D binary image Euler number computation. Adv. Soft Comput. 2019, 11835, 701–713. [Google Scholar]
- Sossa, J.; Santiago, R.; Pérez, M.; Rubío, E. Computing the Euler number of a binary image based on a vertex codification. J. Appl. Res. Technol. 2013, 11, 360–370. [Google Scholar] [CrossRef] [Green Version]
- Čomića, L.; Magillo, P. Surface-based computation of the Euler characteristic in the cubical grid. Graph. Model. 2020, 112, 101093. [Google Scholar] [CrossRef]
- Morgenthaler, D. Three-Dimensional Digital Image Processing; University of Maryland: College Park, MD, USA, 1981. [Google Scholar]





| Index of Voxel Patterns | ΔE | Index of Voxel Patterns | ΔE | Index of Voxel Patterns | ΔE |
|---|---|---|---|---|---|
| 00000010 | 1 | 00001001 | −1 | 00001011 | −1 |
| 00011000 | −1 | 00011001 | −1 | 00011010 | −1 |
| 00011011 | −1 | 00100001 | −1 | 00100011 | −1 |
| 00100100 | −1 | 00100101 | −1 | 00100110 | −1 |
| 00100111 | −1 | 00101000 | −1 | 00101001 | −2 |
| 00101010 | −1 | 00101011 | −2 | 00101100 | −1 |
| 00101101 | −1 | 00101110 | −1 | 00101111 | −1 |
| 00111000 | −1 | 00111001 | −1 | 00111010 | −1 |
| 00111011 | −1 | 10000001 | −1 | 10000011 | −1 |
| 10001001 | −1 | 10001011 | −1 | 10010100 | 1 |
| 10010101 | 1 | 10010110 | 1 | 10010111 | 1 |
| 10011100 | 1 | 10011101 | 1 | 10011110 | 1 |
| 10011111 | 1 | 10100001 | −1 | 10100011 | −1 |
| 10101001 | −1 | 10101011 | −1 | 10110100 | 1 |
| 10110101 | 1 | 10110110 | 1 | 10110111 | 1 |
| 10111100 | 1 | 10111101 | 1 | 10111110 | 1 |
| 10111111 | 1 | Others | 0 |
| The State of the Right Quad | Index | ΔE | The State of the Right Quad | Index | ΔE |
|---|---|---|---|---|---|
| St0 | 00101010 | −1 | St8 | 01101010 | 0 |
| St1 | 00101011 | −2 | St9 | 01101011 | 0 |
| St2 | 00101110 | −1 | St10 | 01101110 | 0 |
| St3 | 00101111 | −1 | St11 | 01101111 | 0 |
| St4 | 00111010 | −1 | St12 | 01111010 | 0 |
| St5 | 00111011 | −2 | St13 | 01111011 | 0 |
| St6 | 00111110 | 0 | St14 | 01111110 | 0 |
| St7 | 00111111 | 0 | St15 | 01111111 | 0 |
| State of Right Voxels | Voxel Pattern | Index | ΔE | State of Right Voxels | Voxel Pattern | Index | ΔE |
|---|---|---|---|---|---|---|---|
| St0 | VP1 | 00000010 | 1 | St1 | VP1 | 00000010 | 1 |
| VP2 | 00101010 | −1 | VP2 | 00101011 | −2 | ||
| St2 | VP1 | 00000010 | 1 | St3 | VP1 | 00000010 | 1 |
| VP2 | 00101110 | −1 | VP2 | 00101111 | −1 | ||
| St4 | VP1 | 00000011 | 0 | St5 | VP1 | 00000011 | 0 |
| VP2 | 00111010 | −1 | VP2 | 00111011 | −1 | ||
| St6 | VP1 | 00000011 | 0 | St7 | VP1 | 00000011 | 0 |
| VP2 | 00111110 | 0 | VP2 | 00111111 | 0 | ||
| …… | …… | ||||||
| …… | …… | ||||||
| St56 | VP1 | 01010110 | 0 | St57 | VP1 | 01010110 | 0 |
| VP2 | 01101010 | 0 | VP2 | 01101011 | 0 | ||
| St58 | VP1 | 01010110 | 0 | St59 | VP1 | 01010110 | 0 |
| VP2 | 01101110 | 0 | VP2 | 01101111 | 0 | ||
| St60 | VP1 | 01010111 | 0 | St61 | VP1 | 01010111 | 0 |
| VP2 | 01111010 | 0 | VP2 | 01111011 | 0 | ||
| St62 | VP1 | 01010111 | 0 | St63 | VP1 | 01010111 | 0 |
| VP2 | 01111110 | 0 | VP2 | 01111111 | 0 |
| Indexes of Voxel Patterns | ΔE | Indexes of Voxel Patterns | ΔE |
|---|---|---|---|
| 00011000–00011011 | −1 | 10010100–10010111 | 1 |
| 00100100–00100111 | −1 | 10011100–10011111 | 1 |
| 00101100–00101111 | −1 | 10110100–10110111 | 1 |
| 00111000–00111011 | −1 | 10111100–10111111 | 1 |
| Name | Version |
|---|---|
| Processor | Intel Core i7-6770 |
| Frequency | 3.20 GHz |
| Memory | 8 GB |
| Operating System | Ubuntu Linux 20.04.1 |
| GCC Compiler | 4.6.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, B.; He, H.; Kang, S.; Chao, Y.; He, L. Efficient Strategies for Computing Euler Number of a 3D Binary Image. Electronics 2023, 12, 1726. https://doi.org/10.3390/electronics12071726
Yao B, He H, Kang S, Chao Y, He L. Efficient Strategies for Computing Euler Number of a 3D Binary Image. Electronics. 2023; 12(7):1726. https://doi.org/10.3390/electronics12071726
Chicago/Turabian StyleYao, Bin, Haochen He, Shiying Kang, Yuyan Chao, and Lifeng He. 2023. "Efficient Strategies for Computing Euler Number of a 3D Binary Image" Electronics 12, no. 7: 1726. https://doi.org/10.3390/electronics12071726
APA StyleYao, B., He, H., Kang, S., Chao, Y., & He, L. (2023). Efficient Strategies for Computing Euler Number of a 3D Binary Image. Electronics, 12(7), 1726. https://doi.org/10.3390/electronics12071726







