Ultra-Short Pulsed Laser Annealing Effects on MoS2 Transistors with Asymmetric and Symmetric Contacts
Abstract
:1. Introduction
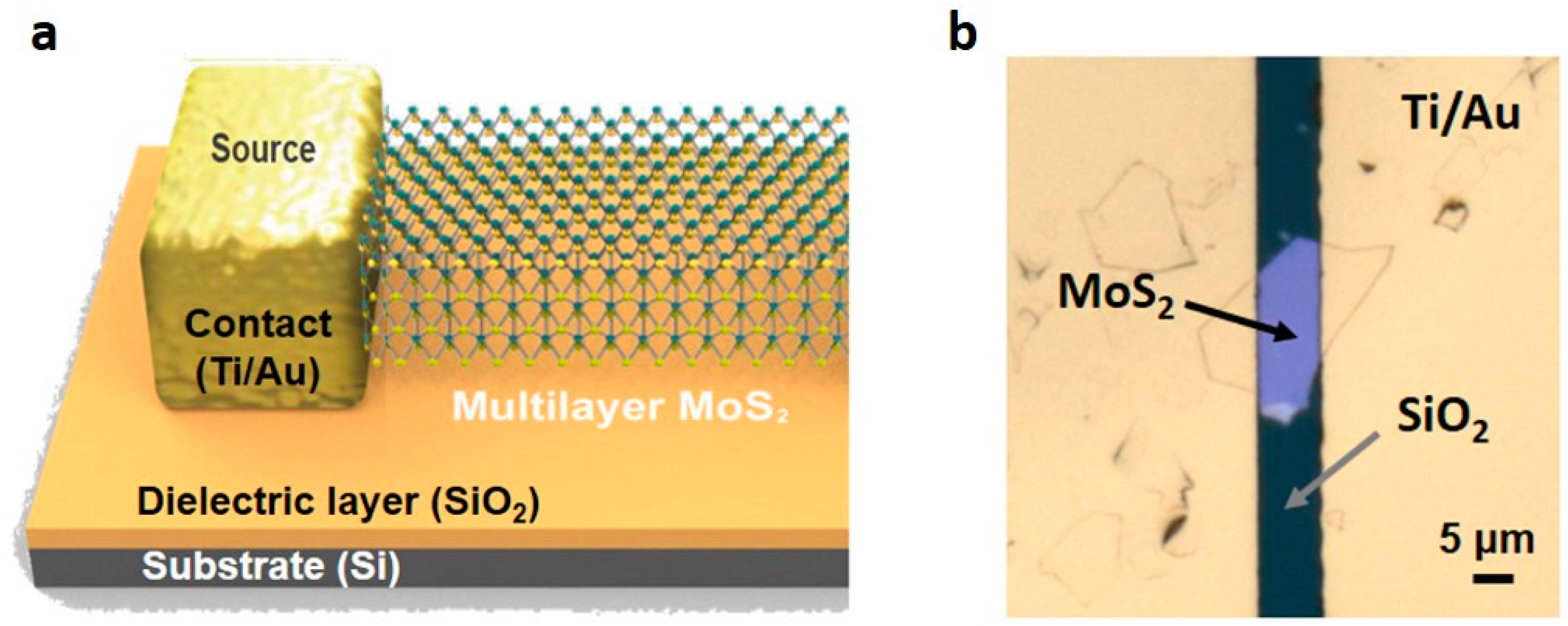
2. Experiments and Setup
3. Results and Discussion
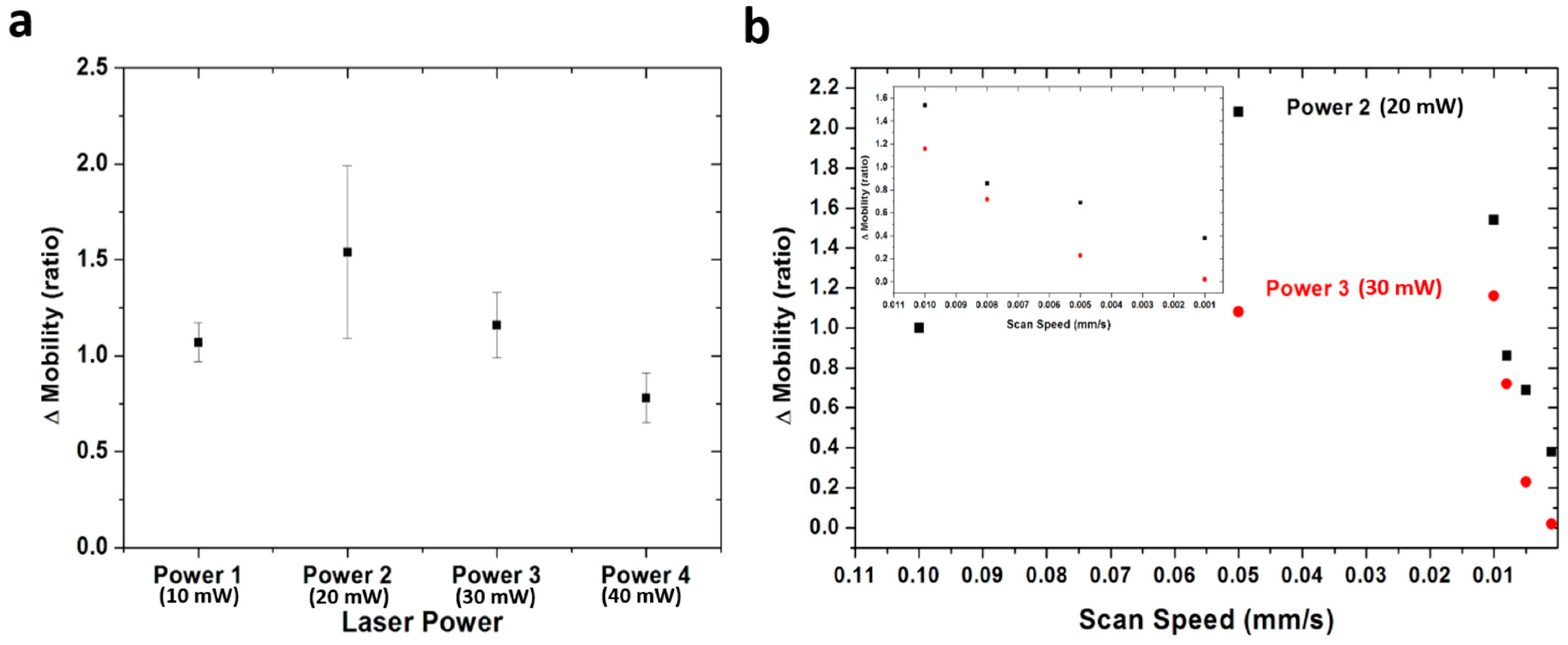
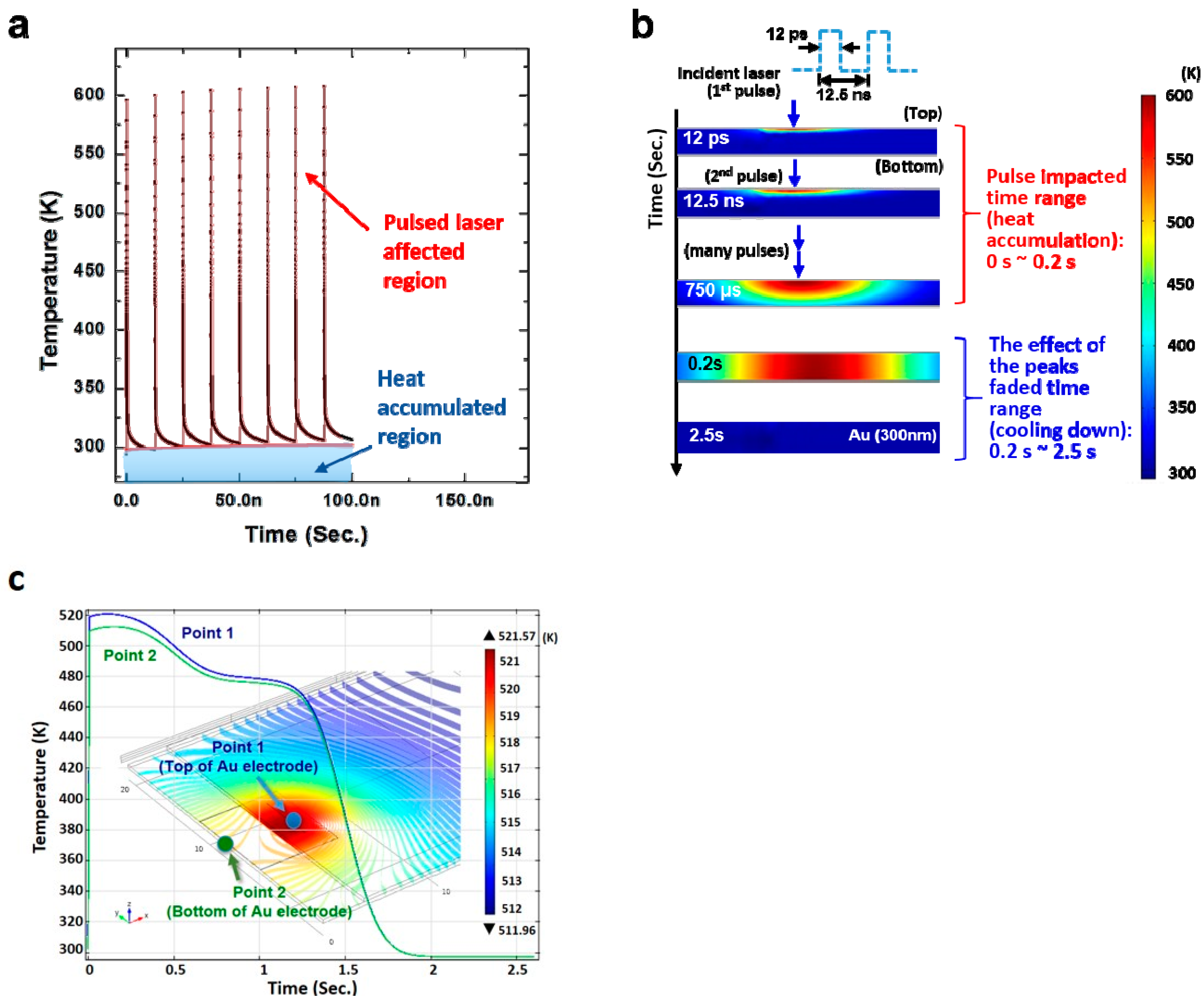
3.1. Thermal Analysis
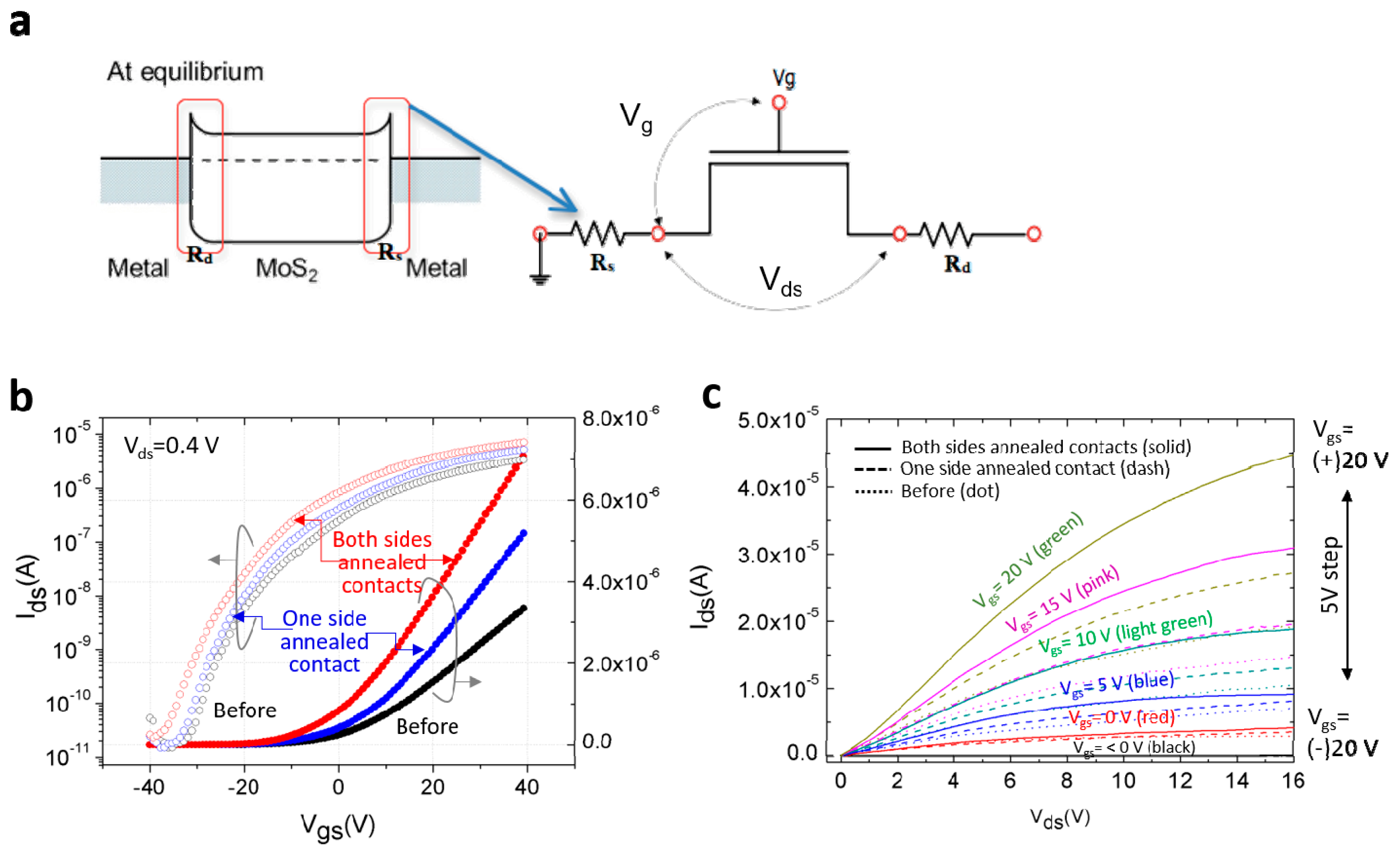
3.2. Electrical Characteristics
4. Summary and Outlook
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- MacDonald, W.A.; Looney, M.; MacKerron, D.; Eveson, R.; Adam, R.; Hashimoto, K.; Rakos, K. Latest advances in substrates for flexible electronics. J. Soc. Inf. Disp. 2007, 15, 1075–1083. [Google Scholar] [CrossRef]
- Kim, D.-H.; Lu, N.; Ma, R.; Kim, Y.-S.; Kim, R.-H.; Wang, S.; Wu, J.; Won, S.M.; Tao, H.; Islam, A. Epidermal electronics. Science 2011, 333, 838–843. [Google Scholar] [CrossRef] [PubMed]
- Akinwande, D.; Petrone, N.; Hone, J. Two-dimensional flexible nanoelectronics. Nat. Commun. 2014, 5, 5678. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Q.H.; Kalantar-Zadeh, K.; Kis, A.; Coleman, J.N.; Strano, M.S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotech. 2012, 7, 699. [Google Scholar] [CrossRef] [PubMed]
- Splendiani, A.; Sun, L.; Zhang, Y.; Li, T.; Kim, J.; Chim, C.-Y.; Galli, G.; Wang, F. Emerging photoluminescence in monolayer MoS2. Nano. Lett. 2010, 10, 1271–1275. [Google Scholar] [CrossRef] [PubMed]
- Mak, K.F.; Lee, C.; Hone, J.; Shan, J.; Heinz, T.F. Atomically thin MoS2: A new direct-gap semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [PubMed]
- Radisavljevic, B.; Radenovic, A.; Brivio, J.; Giacometti, I.V.; Kis, A. Single-layer MoS2 transistors. Nat. Nanotech. 2011, 6, 147. [Google Scholar] [CrossRef]
- Ganatra, R.; Zhang, Q. Few-layer MoS2: A promising layered semiconductor. ACS Nano. 2014, 8, 4074–4099. [Google Scholar] [CrossRef]
- Castellanos-Gomez, A.; Poot, M.; Steele, G.A.; van der Zant, H.S.; Agraït, N.; Rubio-Bollinger, G. Elastic properties of freely suspended MoS2 nanosheets. Adv. Mater. 2012, 24, 772–775. [Google Scholar] [CrossRef]
- Bertolazzi, S.; Brivio, J.; Kis, A. Stretching and breaking of ultrathin MoS2. ACS Nano. 2011, 5, 9703–9709. [Google Scholar] [CrossRef]
- Pu, J.; Yomogida, Y.; Liu, K.-K.; Li, L.-J.; Iwasa, Y.; Takenobu, T. Highly flexible MoS2 thin-film transistors with ion gel dielectrics. Nano. Lett. 2012, 12, 4013–4017. [Google Scholar] [CrossRef] [PubMed]
- Duerloo, K.-A.N.; Ong, M.T.; Reed, E.J. Intrinsic piezoelectricity in two-dimensional materials. J. Phys. Chem. Lett. 2012, 3, 2871–2876. [Google Scholar] [CrossRef]
- Popov, I.; Seifert, G.; Tománek, D. Designing electrical contacts to MoS2 monolayers: a computational study. Phys. Rev. Lwtt. 2012, 108, 156802. [Google Scholar]
- Fang, H.; Chuang, S.; Chang, T.C.; Takei, K.; Takahashi, T.; Javey, A. High-performance single layered WSe2 p-FETs with chemically doped contacts. Nano Lett. 2012, 12, 3788–3792. [Google Scholar] [CrossRef] [PubMed]
- Das, S.; Chen, H.-Y.; Penumatcha, A.V.; Appenzeller, J. High performance multilayer MoS2 transistors with scandium contacts. Nano Lett. 2012, 13, 100–105. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Ding, S.; Araki, T.; Jiu, J.; Sugahara, T.; Wang, J.; Vanfleteren, J.; Sekitani, T.; Suganuma, K. Facile fabrication of stretchable Ag nanowire/polyurethane electrodes using high intensity pulsed light. Nano. Res. 2016, 9, 401–414. [Google Scholar] [CrossRef] [Green Version]
- Kwon, H.; Choi, W.; Lee, D.; Lee, Y.; Kwon, J.; Yoo, B.; Grigoropoulos, C.P.; Kim, S. Selective and localized laser annealing effect for high-performance flexible multilayer MoS2 thin-film transistors. Nano Res. 2014, 7, 1137–1145. [Google Scholar] [CrossRef]
- Kwon, H.-J.; Jang, J.; Kim, S.; Subramanian, V.; Grigoropoulos, C.P. Electrical characteristics of multilayer MoS2 transistors at real operating temperatures with different ambient conditions. Appl. Phys. Lett. 2014, 105, 152105. [Google Scholar] [CrossRef]
- Yeh, P.-C.; Jin, W.; Zaki, N.; Kunstmann, J.; Chenet, D.; Arefe, G.; Sadowski, J.T.; Dadap, J.I.; Sutter, P.; Hone, J. Direct measurement of the tunable electronic structure of bilayer MoS2 by interlayer twist. Nano. Lett. 2016, 16, 953–959. [Google Scholar] [CrossRef]
- Py, M.; Haering, R. Structural destabilization induced by lithium intercalation in MoS2 and related compounds. Can. J. Phys. 1983, 61, 76–84. [Google Scholar] [CrossRef]
- Di Bartolomeo, A.; Genovese, L.; Giubileo, F.; Iemmo, L.; Luongo, G.; Foller, T.; Schleberger, M. Hysteresis in the transfer characteristics of MoS2 transistors. 2D Mater. 2017, 5, 015014. [Google Scholar] [CrossRef]
- Léonard, F.; Talin, A.A. Electrical contacts to one-and two-dimensional nanomaterials. Nat. Nanotech. 2011, 6, 773. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Wang, T.; Liu, L.; Du, K.; Liu, W.; Zhu, Z.; Li, M. Molybdenum disulfide and Au ultrasmall nanohybrids as highly active electrocatalysts for hydrogen evolution reaction. J. Mater. Chem. A 2017, 5, 4122–4128. [Google Scholar] [CrossRef]
- Eaton, S.M.; Zhang, H.; Herman, P.R.; Yoshino, F.; Shah, L.; Bovatsek, J.; Arai, A.Y. Heat accumulation effects in femtosecond laser-written waveguides with variable repetition rate. Opt. Express 2005, 13, 4708–4716. [Google Scholar] [CrossRef] [PubMed]
- Di Bartolomeo, A.; Grillo, A.; Urban, F.; Iemmo, L.; Giubileo, F.; Luongo, G.; Amato, G.; Croin, L.; Sun, L.; Liang, S.J. Asymmetric Schottky Contacts in Bilayer MoS2 Field Effect Transistors. Adv. Funct. Mater. 2018, 1800657. [Google Scholar] [CrossRef]
- Xu, Y.; Minari, T.; Tsukagoshi, K.; Chroboczek, J.; Ghibaudo, G. Direct evaluation of low-field mobility and access resistance in pentacene field-effect transistors. J. Appl. Phys. 2010, 107, 114507. [Google Scholar] [CrossRef]
- Ghibaudo, G. New method for the extraction of MOSFET parameters. Electron. Lett. 1988, 24, 543–545. [Google Scholar] [CrossRef]
- Chang, H.-Y.; Zhu, W.; Akinwande, D. On the mobility and contact resistance evaluation for transistors based on MoS2 or two-dimensional semiconducting atomic crystals. Appl. Phys. Lett. 2014, 104, 113504. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kwon, H.; Baik, S.; Jang, J.E.; Jang, J.; Kim, S.; Grigoropoulos, C.P.; Kwon, H.-J. Ultra-Short Pulsed Laser Annealing Effects on MoS2 Transistors with Asymmetric and Symmetric Contacts. Electronics 2019, 8, 222. https://doi.org/10.3390/electronics8020222
Kwon H, Baik S, Jang JE, Jang J, Kim S, Grigoropoulos CP, Kwon H-J. Ultra-Short Pulsed Laser Annealing Effects on MoS2 Transistors with Asymmetric and Symmetric Contacts. Electronics. 2019; 8(2):222. https://doi.org/10.3390/electronics8020222
Chicago/Turabian StyleKwon, Hyeokjin, Seunghun Baik, Jae Eun Jang, Jaewon Jang, Sunkook Kim, Costas P. Grigoropoulos, and Hyuk-Jun Kwon. 2019. "Ultra-Short Pulsed Laser Annealing Effects on MoS2 Transistors with Asymmetric and Symmetric Contacts" Electronics 8, no. 2: 222. https://doi.org/10.3390/electronics8020222
APA StyleKwon, H., Baik, S., Jang, J. E., Jang, J., Kim, S., Grigoropoulos, C. P., & Kwon, H.-J. (2019). Ultra-Short Pulsed Laser Annealing Effects on MoS2 Transistors with Asymmetric and Symmetric Contacts. Electronics, 8(2), 222. https://doi.org/10.3390/electronics8020222





