Design of an Intrinsically Safe Series-Series Compensation WPT System for Automotive LiDAR
Abstract
:1. Introduction
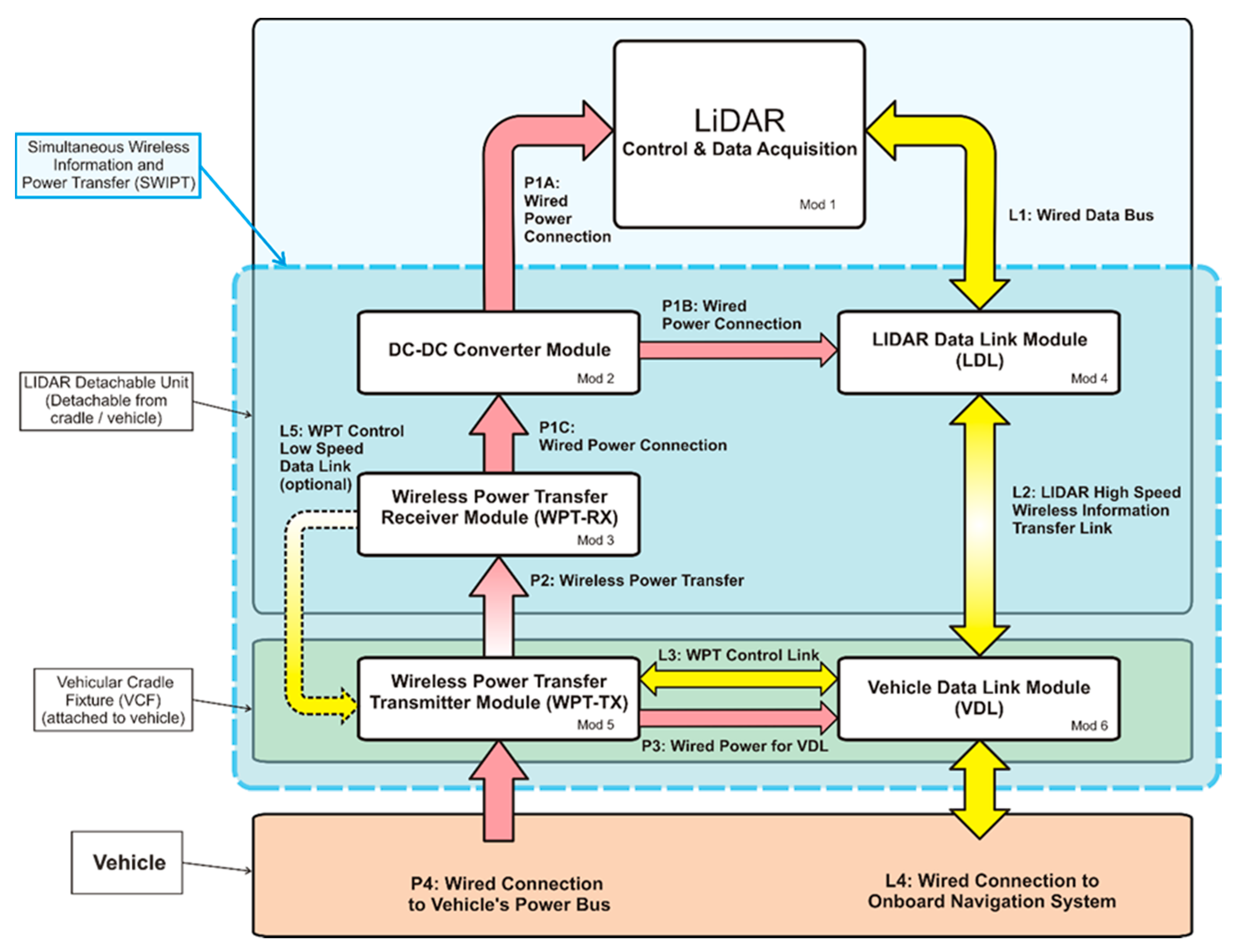
2. LiDAR Requirements for Simultaneous Information and Power Transfer
2.1. LiDAR Technology
2.2. LiDAR-Wireless Information and Power Transfer
3. WPT Model
3.1. Magnetic Ressoance and the Series-Series Impedance Compensated WPT
3.2. Estimation of the Maximum Power that Can Be Transferred over a Lossless Magnetic Link
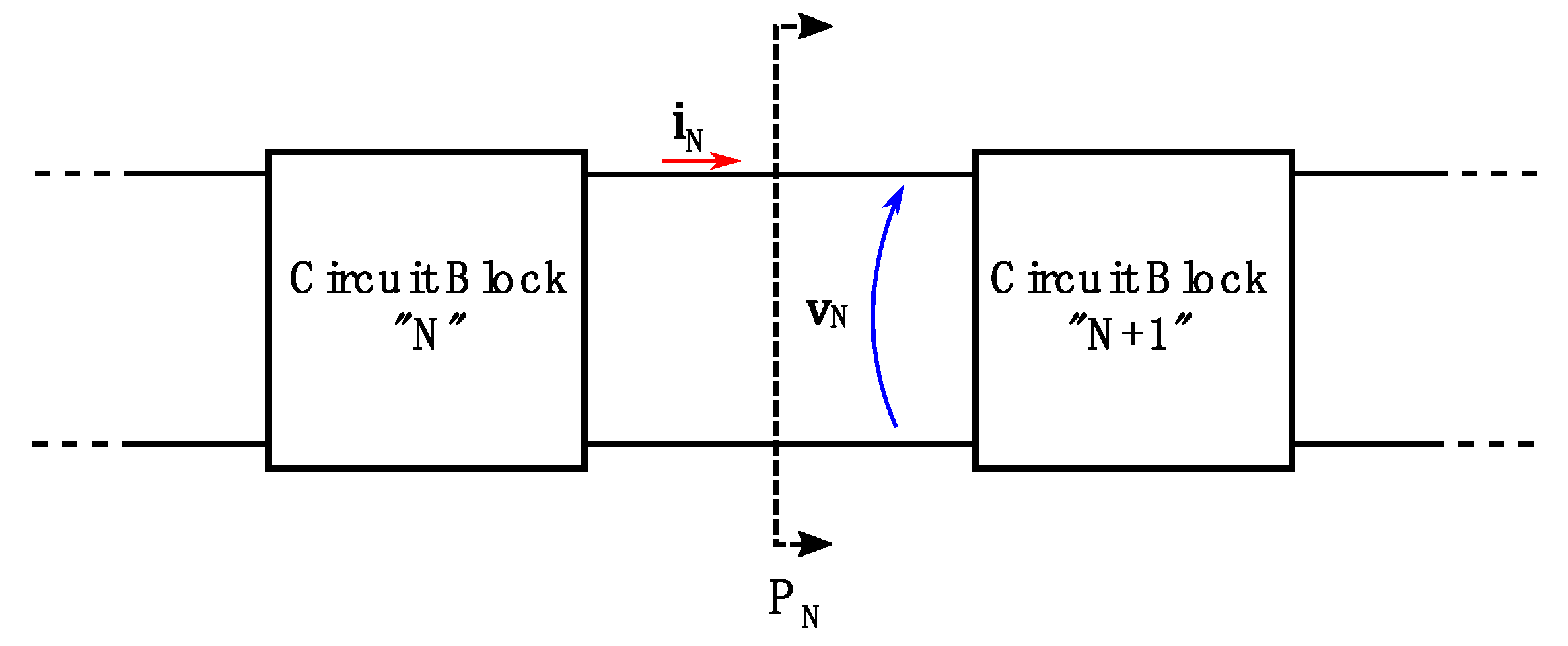
3.3. Magnetic Coupling Efficiency and the Overall DC-DC Efficiency
- , the power supplied by the primary DC source.
- , the power supplied to the compensation circuit by the primary DC-DC converter.
- , the power delivered at the terminals of the primary coil of the magnetic link.
- , the power transferred over the air-gap of the magnetic link.
- , the power delivered to the secondary circuit by the magnetic link.
- , the power delivered to the load-matching DC-DC converter in the secondary.
- , the power consumed by the load, .
- is the efficiency of the DC-DC at the input of the primary side.
- is the efficiency of the DC-AC converter driving the compensation circuit of the primary side, typically in the simpler WPT system, an H-bridge inverter.
- is the joint efficiency of the secondary compensation, the rectifier and DC filter on the secondary side.
- is the efficiency of the DC-DC converter that, if present, will feed the load.
3.4. WPT–Adotped Circuits
4. WPT Design and Simulation
4.1. Coil Selection
4.2. Impedance Compensation and DC Input Level
4.3. Open Secondary Safety Check
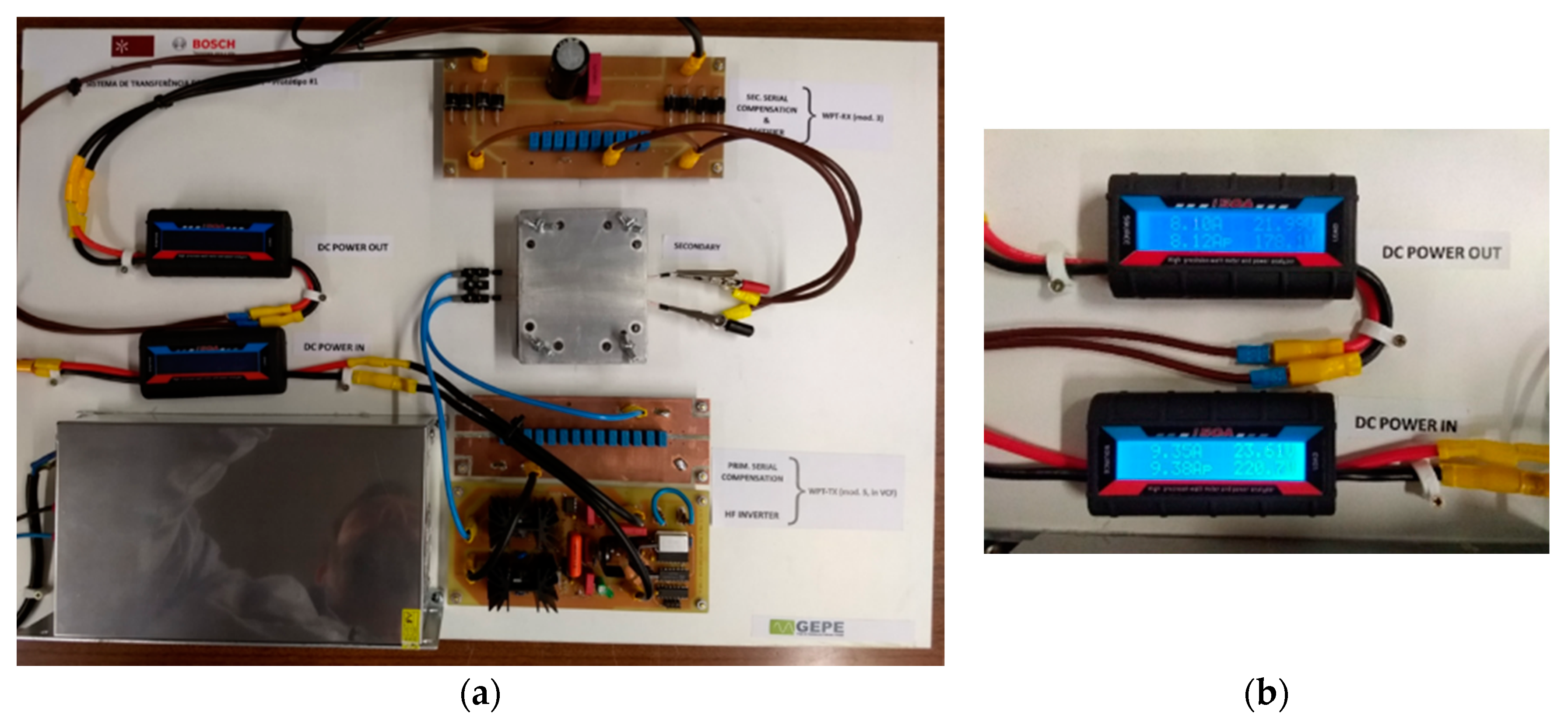
5. Results from Prototype
5.1. Output Power and Overall Efficiency
5.2. Open Secondary Safety Check
5.3. Power Transferred over the Air-Gap
5.4. Power and Efficiency Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Maxwell, J.C. A Treatise on Electricity and Magnetism, 3rd ed.; 1891; Dover Publications, Inc.: New York, NY, USA, 1873. [Google Scholar]
- Mulligan, J.F. Heinrich Hertz and the development of physics. Phys. Today 1989, 42, 50–57. [Google Scholar] [CrossRef]
- Helrich, C.S. The Classical Theory of Fields, 1st ed.; Needs, R., Rhodes, W.T., Stanley, H.E., Eds.; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- The Faraday centenary—A supplement to the bell system. Tech. J. 1931, 10, i–vii.
- Faraday, M. Experimental Researches in Electricity, 2nd ed.; 1849; Richard, E., John, E., Eds.; Richard and John Edward Taylor, Printers and Publishers to the University of London: London, UK, 1839; Volume 1. [Google Scholar]
- Coltman, J.W. The transformer historical overview. IEEE Ind. Appl. Mag. 2002, 8, 8–15. [Google Scholar] [CrossRef] [Green Version]
- Leyh, G.E.; Kennan, M.D. Efficient wireless transmission of power using resonators with coupled electric fields. In Proceedings of the 2008 40th North American Power Symposium, Calgary, AB, Canada, 28–30 September 2008; pp. 1–4. [Google Scholar]
- Tesla, N. Nikola Tesla on His Work With Alternating Currents and Their Application to Wireless Telegraphy, Telephony, and Transmission of Power—An Extended Interview (Tesla Presents Series, Part 1); Anderson, L.I., Ed.; Twenty First Century Books: Breckenridge, CO, USA, 1992. [Google Scholar]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljačić, M. Wireless power transfer via strongly coupled magnetic resonances. Science 2007, 317, 83–87. [Google Scholar] [CrossRef] [Green Version]
- Boys, J.T.; Covic, G.A. The inductive power transfer story at the University of Auckland. IEEE Circuits Syst. Mag. 2015, 15, 6–27. [Google Scholar] [CrossRef]
- Lidar, V. Velodyne’s HDL-64E: A high definition LiDAR sensor for 3D applications; White Paper—Velodyne LiDAR: San Jose, CA, USA, 2007; pp. 1–7. [Google Scholar]
- Varshney, L.R. Transporting information and energy simultaneously. In Proceedings of the 2008 IEEE International Symposium on Information Theory, Toronto, ON, Canada, 6–11 July 2008; pp. 1612–1616. [Google Scholar]
- Liu, L.; Zhang, R.; Chua, K. Wireless information transfer with opportunistic energy harvesting. IEEE Trans. Wirel. Commun. 2013, 12, 288–300. [Google Scholar] [CrossRef] [Green Version]
- Perera, T.D.P.; Jayakody, D.N.K.; De, S.; Ivanov, M.A. A Survey on simultaneous wireless information and power transfer. J. Phys. Conf. Ser. 2017, 803, 012113. [Google Scholar] [CrossRef]
- Berger, A.L.; Pilnick, B. Interface for Transferring Power and Data Between a Non-Rotating Body and a Rotating Body. World Intellectual Property Organization. U.S. Patent No. WO 2018/125709 Al, 5 July 2018. [Google Scholar]
- Worms, K.; Dreschmann, M. Lidar System, Operating Method for a Lidar Syetem and Working Device. Weltorganisation für Geistiges Eigentum. U.S. Patent No. WO 2019/158301 Al, 4 April 2019. [Google Scholar]
- Wu, M.C.; Lin, C.H.; Fang, M.H. Rotating Optical Range Finder. United States Patent Application Publication. U.S. Patent US 2016/0274221 A1, 22 September 2016. [Google Scholar]
- Pacala, A.; Yu, T.; Eldada, L. Cost-Effective Lidar Sensor for Multi-Signal Detection, Weak Signal Detection and Signal Disambiguation and Method of Using Same. U.S. Patent US20140211194A1, 31 July 2014. [Google Scholar]
- Hall, D.S. High definition LiDAR system. U.S. Patent US8767190B2, 1 July 2014. [Google Scholar]
- Niu, W.; Chu, J.; Gu, W.; Shen, A. Exact analysis of frequency splitting phenomena of contactless power transfer systems. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 1670–1677. [Google Scholar] [CrossRef]
- Zargham, M.; Gulak, P.G. Maximum achievable efficiency in near-field coupled power-transfer systems. IEEE Trans. Biomed. Circuits Syst. 2012, 6, 228–245. [Google Scholar] [CrossRef]
- Singh, B.; Singh, S.; Chandra, A.; Al-Haddad, K. Comprehensive study of single-phase ac-dc power factor corrected converters with high-frequency isolation. IEEE Trans. Ind. Inform. 2011, 7, 540–556. [Google Scholar] [CrossRef]
- Bellar, M.D.; Wu, T.S.; Tchamdjou, A.; Mahdavi, J.; Ehsani, M. A review of soft-switched DC-AC converters. IEEE Trans. Ind. Appl. 1998, 34, 847–860. [Google Scholar] [CrossRef]
- Samanta, S.; Rathore, A.K. Wireless power transfer technology using full-bridge current-fed topology for medium power applications. IET Power Electron. 2016, 9, 1903–1913. [Google Scholar] [CrossRef]
- Mallik, A.; Khaligh, A. Maximum efficiency tracking of an integrated two-staged AC–DC converter using variable DC-link voltage. IEEE Trans. Ind. Electron. 2018, 65, 8408–8421. [Google Scholar] [CrossRef]
- Qian, H.; Lai, J.; Zhang, J.; Yu, W. High-efficiency bidirectional AC-DC converter for energy storage systems. In Proceedings of the 2010 IEEE Energy Conversion Congress and Exposition, Atlanta, Georgia, 12–16 September 2010; pp. 3224–3229. [Google Scholar]
- Rothmund, D.; Guillod, T.; Bortis, D.; Kolar, J.W. 99.1% efficient 10 kV SiC-based medium-voltage ZVS bidirectional single-phase PFC AC/DC stage. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 779–797. [Google Scholar] [CrossRef]
- Lai, J.-S.; Peng, F.Z. Multilevel converters-a new breed of power converters. IEEE Trans. Ind. Appl. 1996, 32, 509–517. [Google Scholar]
- Rodriguez, J.; Lai, J.-S.; Peng, F.Z. Multilevel inverters: A survey of topologies, controls, and applications. IEEE Trans. Ind. Electron. 2002, 49, 724–738. [Google Scholar] [CrossRef] [Green Version]
- Park, S.-J.; Kang, F.-S.; Lee, M.H.; Kim, C.-U. A new single-phase five-level PWM inverter employing a deadbeat control scheme. IEEE Trans. Power Electron. 2003, 18, 831–843. [Google Scholar] [CrossRef]
- Marchesoni, M.; Mazzucchelli, M.; Tenconi, S. A non conventional power converter for plasma stabilization. In Proceedings of the PESC ’88 Record: 19th Annual IEEE Power Electronics Specialists Conference, Kyoto, Japan, 11–14 April 1988; Volume 1, pp. 122–129. [Google Scholar]
- Khoucha, F.; Lagoun, M.S.; Kheloui, A.; Benbouzid, M.E.H. A comparison of symmetrical and asymmetrical three-phase H-bridge multilevel inverter for DTC induction motor drives. IEEE Trans. Energy Convers. 2011, 26, 64–72. [Google Scholar] [CrossRef] [Green Version]
- Zhang, L.; Sun, K.; Feng, L.; Wu, H.; Xing, Y. A Family of neutral point clamped full-bridge topologies for transformerless photovoltaic grid-tied inverters. IEEE Trans. Power Electron. 2013, 28, 730–739. [Google Scholar] [CrossRef]
- Teixeira, C.A.; Holmes, D.G.; McGrath, B.P. Single-Phase Semi-Bridge Five-Level Flying-Capacitor Rectifier. IEEE Trans. Ind. Appl. 2013, 49, 2158–2166. [Google Scholar] [CrossRef]
- Ahmed, R.A.; Mekhilef, S.; Ping, H.W. New multilevel inverter topology with minimum number of switches. In Proceedings of the TENCON 2010: 2010 IEEE Region 10 Conference, Fukuoka, Japan, 21–24 November 2010; pp. 1862–1867. [Google Scholar]
- Buticchi, G.; Barater, D.; Lorenzani, E.; Concari, C.; Franceschini, G. A nine-level grid-connected converter topology for single-phase transformerless PV systems. IEEE Trans. Ind. Electron. 2014, 61, 3951–3960. [Google Scholar] [CrossRef]
- Liu, J.; Cheng, K.W.E.; Ye, Y. A cascaded multilevel inverter based on switched-capacitor for high-frequency AC power distribution system. IEEE Trans. Power Electron. 2014, 29, 4219–4230. [Google Scholar] [CrossRef]
- Babaei, E.; Alilu, S.; Laali, S. A new general topology for cascaded multilevel inverters with reduced number of components based on developed H-bridge. IEEE Trans. Ind. Electron. 2014, 61, 3932–3939. [Google Scholar] [CrossRef]
- Babaei, E.; Laali, S.; Alilu, S. Cascaded multilevel inverter with series connection of novel H-bridge basic units. IEEE Trans. Ind. Electron. 2014, 61, 6664–6671. [Google Scholar] [CrossRef]
- Society of Automobile Engineers (SAE). SAE Technical Information Report J2954—Wireless Power Transfer for Light-Duty Plug-In/Electric Vehicles and Alignment Methodology; SAE: Warrendale, PA, USA, 2016. [Google Scholar]














| Component Specification | Measured Values * (gap = 3 mm) | Energy Parameter | Estimated Transferrable Power @ | |||
|---|---|---|---|---|---|---|
| Coil Model | L (self-inductance) | (A) | (μH) | (μH) | (μH.A2) | (W) |
| Würth Elektronik 760308102142 | 5.8 ± 10% | 18 | 7.04 ± 2% | 4.97 ± 2% | 805 ± 10% (coil model) | 273 ± 10% (coil model) |
| Parameter | Design Value | |
|---|---|---|
| Air-gap, d | 3 mm | |
| Frequency of Operation, | 84.75 kHz | |
| Coil | Stand Alone Inductance, | 5.8 μH |
| Coupled Leakage Inductance, | 7.04 μH | |
| Mutual Inductance, | 4.97 μH | |
| Q-factor @ , | 85 | |
| Primary compensation capacitor, | 1.4 μF | |
| Secondary compensation capacitor, | 1.5 μF | |
| Primary DC power source, | 24 V | |
| Load resistance, | ≥2.5 Ω | |
| Maximum DC load power, | ≥150 W | |
| DC-DC overall peak efficiency, | ≥80% | |
| Over the air-gap peak efficiency, | ≤96.7% | |
Input Power (W) | Peak Primary Current (A) | Output Power (W) | Overall DC Efficiency (%) | |
|---|---|---|---|---|
| 1.19 ∗ | 395.2 | 27.6 | 300.4 | 76.2 |
| 2.55 | 225.7 | 17.8 | 184.0 | 81.5 |
| 2.71 | 220.7 | 17.4 | 178.1 | 80.7 |
| 3.16 | 193.8 | 16.4 | 154.8 | 79.9 |
| 4.57 | 149.6 | 14.5 | 114.3 | 76.4 |
| 7.86 | 100.4 | 13.1 | 70.8 | 70.5 |
| 16.1 | 63.4 | 13.3 | 36.4 | 54.6 |
| 1 | 199.156098 | 0 | 199.16 |
| 2 | 0.090544 | −33.4 | 199.25 |
| 3 | −1.694581 | −20.7 | 197.55 |
| 4 | 0.013378 | −41.7 | 197.57 |
| 5 | −0.410383 | −26.9 | 197.16 |
| 6 | 0.001274 | −51.9 | 197.16 |
| ⇨ 7 | −0.005496 | −45.6 | 197.15 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cardoso, L.A.L.; Monteiro, V.; Pinto, J.G.; Nogueira, M.; Abreu, A.; Afonso, J.A.; Afonso, J.L. Design of an Intrinsically Safe Series-Series Compensation WPT System for Automotive LiDAR. Electronics 2020, 9, 86. https://doi.org/10.3390/electronics9010086
Cardoso LAL, Monteiro V, Pinto JG, Nogueira M, Abreu A, Afonso JA, Afonso JL. Design of an Intrinsically Safe Series-Series Compensation WPT System for Automotive LiDAR. Electronics. 2020; 9(1):86. https://doi.org/10.3390/electronics9010086
Chicago/Turabian StyleCardoso, Luiz A. Lisboa, Vítor Monteiro, José Gabriel Pinto, Miguel Nogueira, Adérito Abreu, José A. Afonso, and João L. Afonso. 2020. "Design of an Intrinsically Safe Series-Series Compensation WPT System for Automotive LiDAR" Electronics 9, no. 1: 86. https://doi.org/10.3390/electronics9010086
APA StyleCardoso, L. A. L., Monteiro, V., Pinto, J. G., Nogueira, M., Abreu, A., Afonso, J. A., & Afonso, J. L. (2020). Design of an Intrinsically Safe Series-Series Compensation WPT System for Automotive LiDAR. Electronics, 9(1), 86. https://doi.org/10.3390/electronics9010086









