System for Stabilizing an Operating Point of a Remote Electro-Optical Modulator Powered by Optical Fiber
Abstract
:1. Introduction
- (a)
- long cable sections without signal regeneration devices;
- (b)
- intrinsic safety, no current leakage;
- (c)
- electromagnetic compatibility is ensured due to:
- lack of galvanic connection between the receiver and the transmitter;
- absence of metal conductors, which are parasitic transmitting and receiving antennas for radiation in the radio frequency range.
- (d)
- corrosion resistance;
- (e)
- longer fiber-optic cables have a smaller cross-section, weight, and also lower cost compared with equivalent cables with copper or aluminum conductors.
2. Power Over Fiber System for a Remote Electro-Optical Modulator
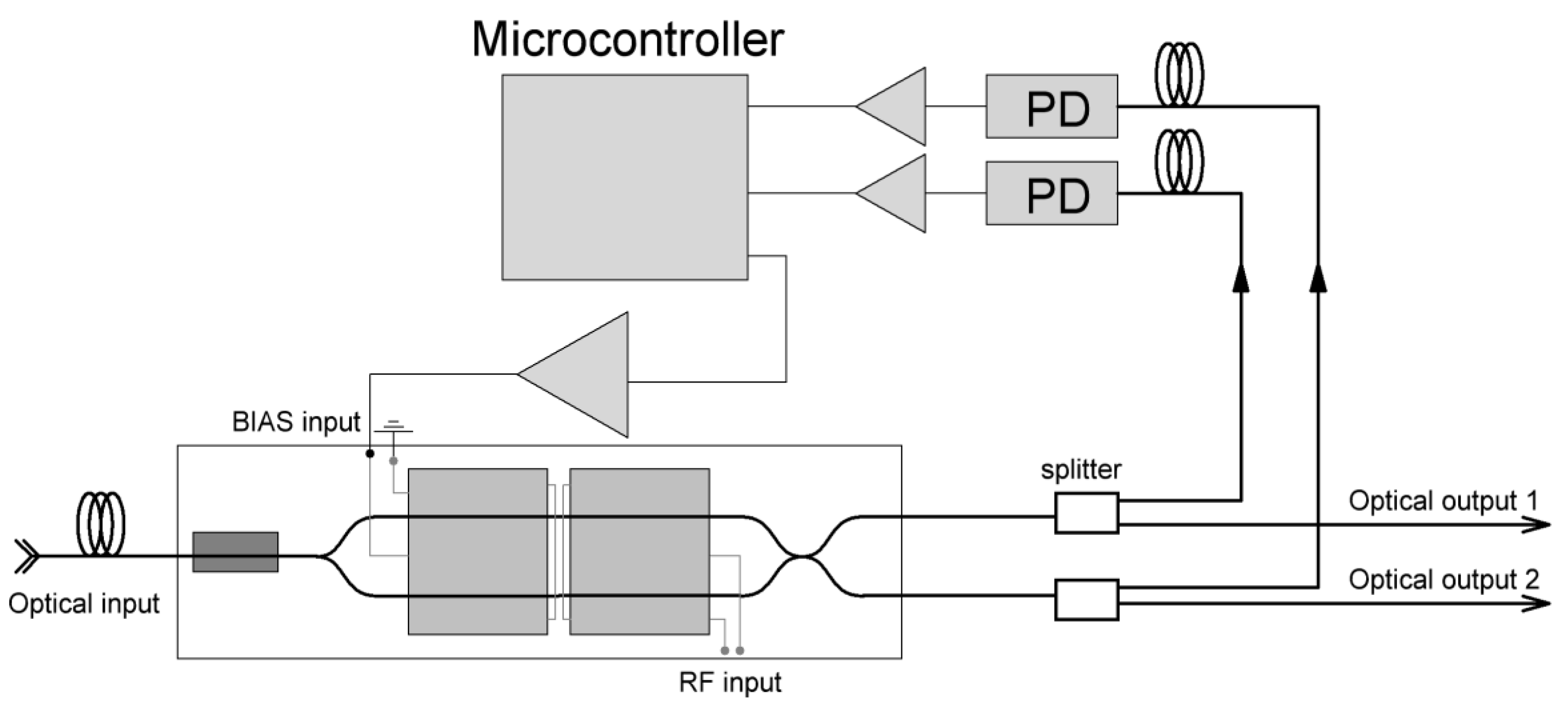
2.1. System Architecture
2.2. Low-Power-Consumption Stabilization System of an Operating Point
2.3. Algorithm for Stabilizing the Operating Point
- Calculation of the signal error function by analyzing the difference between the input and the reference signal.
- Formation of the control signal as the sum of three components (proportional, integral, and differential components).
- Subtracting the control signal from the input signal.
- Re-analysis of the error and repetition of the cycle from 1–3.
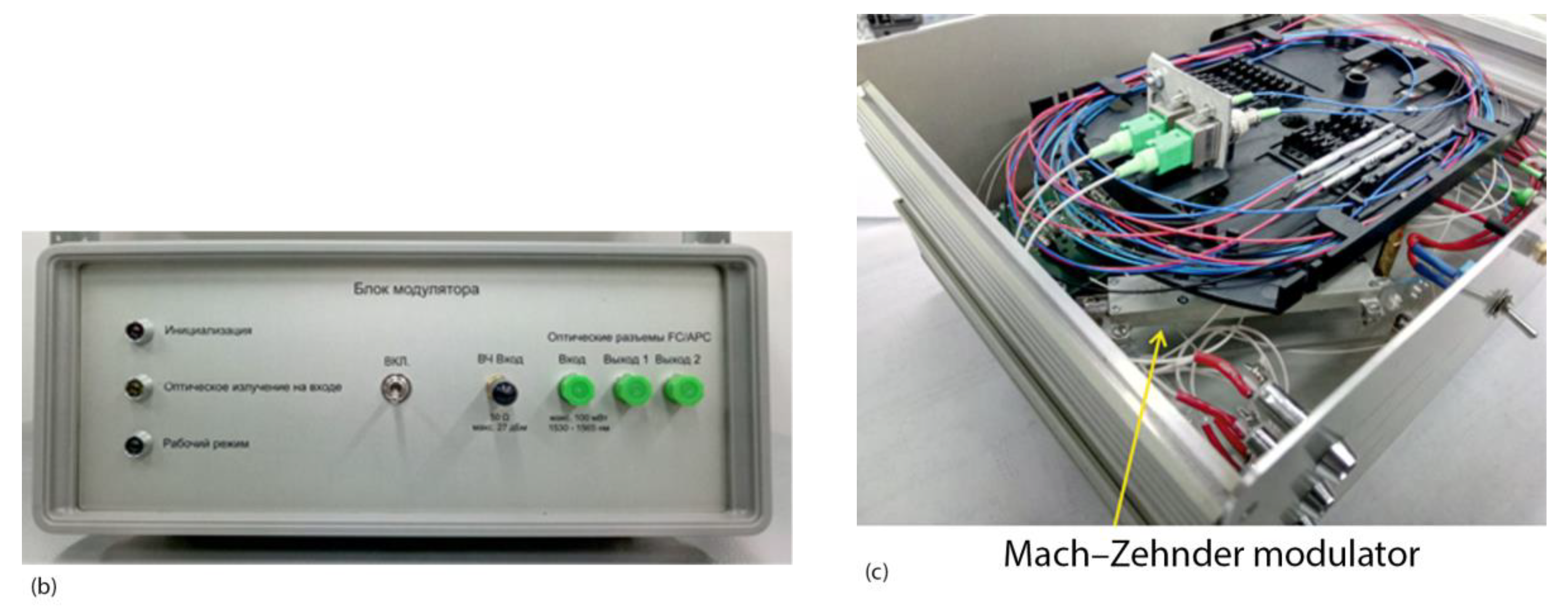
3. Experimental Demonstration
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Waterhouse, R.; Novack, D. Realizing 5G: Microwave Photonics for 5G Mobile Wireless Systems. IEEE Microw. Mag. 2015, 16, 84–92. [Google Scholar] [CrossRef]
- Chen, A.; Murphy, E.J. Broadband Optical Modulators: Science, Technology and Applications; CRC Press: Boca Raton, FL, USA, 2012; p. 568. [Google Scholar]
- Urick, V.J.; Williams, K.J.; McKinney, J.D. Fundamentals of Microwave Photonics; John Wiley & Sons: Hoboken, NJ, USA, 2015; p. 496. [Google Scholar]
- Lebedev, V.V.; Il’Ichev, I.V.; Agruzov, P.M.; Shamray, A.V. The influence of the current-carrying electrode material on the characteristics of integral optical microwave modulators. Tech. Phys. Lett. 2014, 40, 743–746. [Google Scholar] [CrossRef]
- Petrov, A. Radiophotonics Module to Increase the Distance of the Remote Antenna. In Proceedings of the 2019 IEEE International Conference on Electrical Engineering and Photonics (EExPolytech), Saint Petersburg, Russia, 17–18 October 2019; pp. 348–350. [Google Scholar]
- Petrov, A.; Velichko, E.; Lebedev, V.; Ilichev, I.; Agruzov, P.; Parfenov, M.; Varlamov, A.; Shamrai, A. Broad-Band Fiber Optic Link with a Stand-Alone Remote External Modulator for Antenna Remoting and 5G Wireless Network Applications. In Lecture Notes in Computer Science; Springer: Cham, Switzerland, 2019; pp. 727–733. [Google Scholar]
- Deloach, B.C.; Miller, R.C.; Kaufman, S. Sound Alerter Powered Over an Optical Fiber. Bell Syst. Tech. J. 1978, 57, 3309–3316. [Google Scholar] [CrossRef]
- Werthen, J.-G. Powering Next Generation Networks by Laser Light over Fiber. In Proceedings of the OFC/NFOEC 2008—2008 Conference on Optical Fiber Communication/National Fiber Optic Engineers Conference, San Diego, CA, USA, 24–28 February 2008; pp. 1–3. [Google Scholar]
- Tamura, T. Transcutaneous optical power converter for implantable devices. Proc. SPIE 1994, 2084, 99–104. [Google Scholar]
- Dumke, M.; Heiserich, G.; Franke, S.; Schulz, L.; Overmeyer, L. Power Transmission by Optical Fibers for Component Inherent Communication. Syst. Cybern. Inform. 2010, 8, 55. [Google Scholar]
- Basanskaya, A. Electricity over Glass. IEEE Spectr. 2005, 40, 18. [Google Scholar] [CrossRef]
- Cohen, M. Power-over-fiber drives remote data exchange. OPTO Laser Eur. 2006, 140, 27–29. [Google Scholar]
- Yasui, T.; Ohwaki, J.; Mino, M.; Sakai, T. A STable 2-W Supply Optical-Powering System. In Proceedings of the 28th Photovoltaic Specialists Conference, Anchorage, AK, USA, 15–22 September 2000; pp. 1614–1617. [Google Scholar]
- Hou, V. Update on Interim Results of Fiber Optic System Field Failure Analysis. Proc. NFOEC 1991, 1, 539–545. [Google Scholar]
- Zediker, M.S.; Rinzler, C.C.; Faircloth, B.O.; Moxley, J.F. Optical Fiber Cable for Transmission of High Power Laser Energy Over Great Distances. U.S. Patent 9,347,271, 24 May 2016. [Google Scholar]
- Tajima, N.; Kamiyama, D.; Matsuura, M. 150-Watt Power-over-Fiber Feed for Bidirectional Radio-over-Fiber Systems Using a Double-Clad Fiber. In Proceedings of the Optical Fiber Communication Conference (OFC), San Diego, CA, USA, 3–7 March 2019; p. W1I-7. [Google Scholar]
- Cox, C.; Lu, X.; Piprek, J.; Bowers, J.E.; Betts, G.E.; Howerton, M.M.; Burns, W.K.; Van Eck, T.; Yu, P.; Wu, M.C.; et al. RF Photonic Technology in Optical Fiber Links; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Burns, W.K.; Moeller, R.P.; Bulmer, C.H.; Greenblatt, A.S. Depolarized source for fiber-optic applications. Opt. Lett. 1991, 16, 381–383. [Google Scholar] [CrossRef] [PubMed]
- Sisto, M.M.; LaRochelle, S.; Rusch, L.A. Gain Optimization by Modulator-Bias Control in Radio-Over-Fiber Links. J. Light. Technol. 2006, 24, 4974–4982. [Google Scholar] [CrossRef]
- Bulmer, C.; Burns, W. Linear interferometric modulators in Ti: LiNbO3. J. Light. Technol. 1984, 2, 512–521. [Google Scholar] [CrossRef]
- Petrov, A.; Lebedev, V.; Agruzov, P.; Ilichev, P.; Shamrai, A.; Liokumovich, L.; Kudryashova, T.; Sorotsky, V. Lithium Niobate Direction Coupler Modulator for Linearization of Analog Optical Signal Transmission. In Proceedings of the 2020 IEEE International Conference on Electrical Engineering and Photonics (EExPolytech), Saint Petersburg, Russia, 15–16 October 2020; pp. 80–84. [Google Scholar]
- Petrov, A.; Parfenov, M.; Lebedev, V.; Ilichev, I.; Agruzov, P.; Tronev, A.; Shamrai, A. Dynamic Range Improvement of Broad-band Analog Fiber Optic Links with Special Lithium Niobate Integrated Optical Modulators. In Internet of Things, Smart Spaces, and Next Generation Networks and Systems; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Chen, F.S. Optically Induced Change of Refractive Indices in LiNbO3 and LiTaO3. J. Appl. Phys. 1969, 40, 3389–3396. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petrov, A.; Tronev, A.; Agruzov, P.; Shamrai, A.; Sorotsky, V. System for Stabilizing an Operating Point of a Remote Electro-Optical Modulator Powered by Optical Fiber. Electronics 2020, 9, 1861. https://doi.org/10.3390/electronics9111861
Petrov A, Tronev A, Agruzov P, Shamrai A, Sorotsky V. System for Stabilizing an Operating Point of a Remote Electro-Optical Modulator Powered by Optical Fiber. Electronics. 2020; 9(11):1861. https://doi.org/10.3390/electronics9111861
Chicago/Turabian StylePetrov, Aleksei, Aleksandr Tronev, Peter Agruzov, Aleksandr Shamrai, and Vladimir Sorotsky. 2020. "System for Stabilizing an Operating Point of a Remote Electro-Optical Modulator Powered by Optical Fiber" Electronics 9, no. 11: 1861. https://doi.org/10.3390/electronics9111861
APA StylePetrov, A., Tronev, A., Agruzov, P., Shamrai, A., & Sorotsky, V. (2020). System for Stabilizing an Operating Point of a Remote Electro-Optical Modulator Powered by Optical Fiber. Electronics, 9(11), 1861. https://doi.org/10.3390/electronics9111861





