Bayesian Statistics Improves Biological Interpretability of Metabolomics Data from Human Cohorts
Abstract
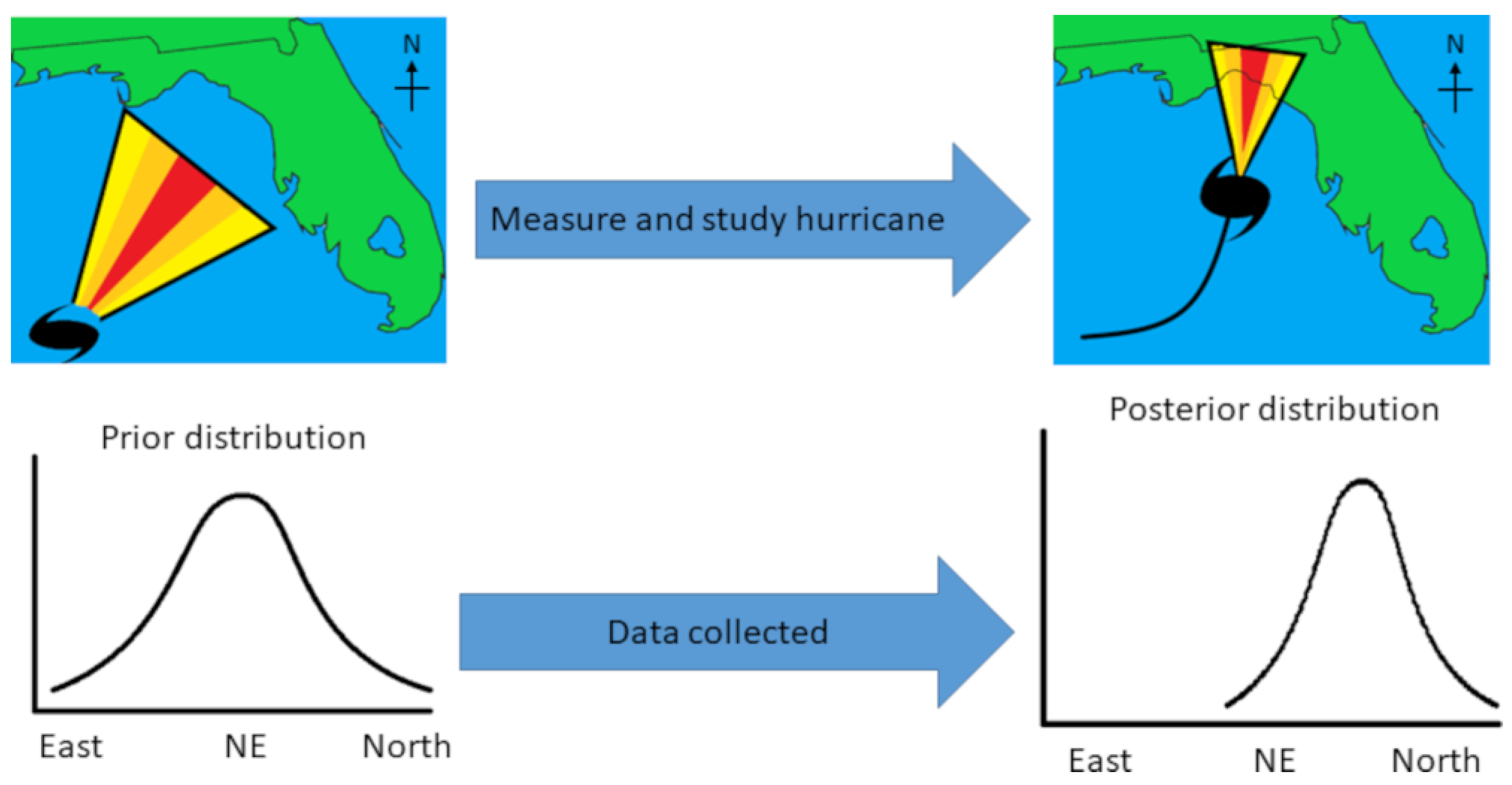
:1. Introduction
2. Materials and Methods
2.1. Metabolomics Datasets
2.2. Statistical Analyses
3. Results
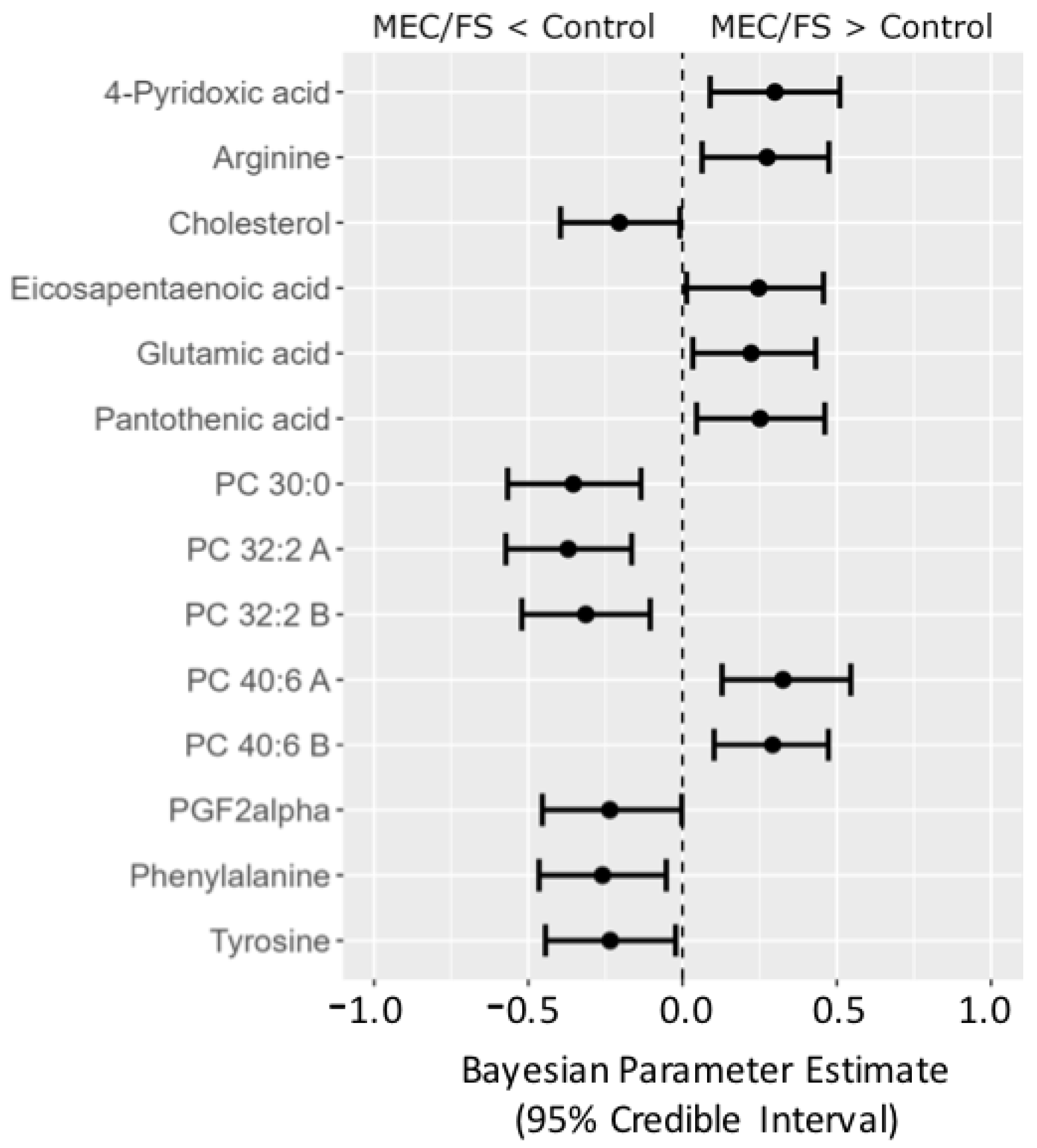
3.1. Classic Univariate Statistics Analyses
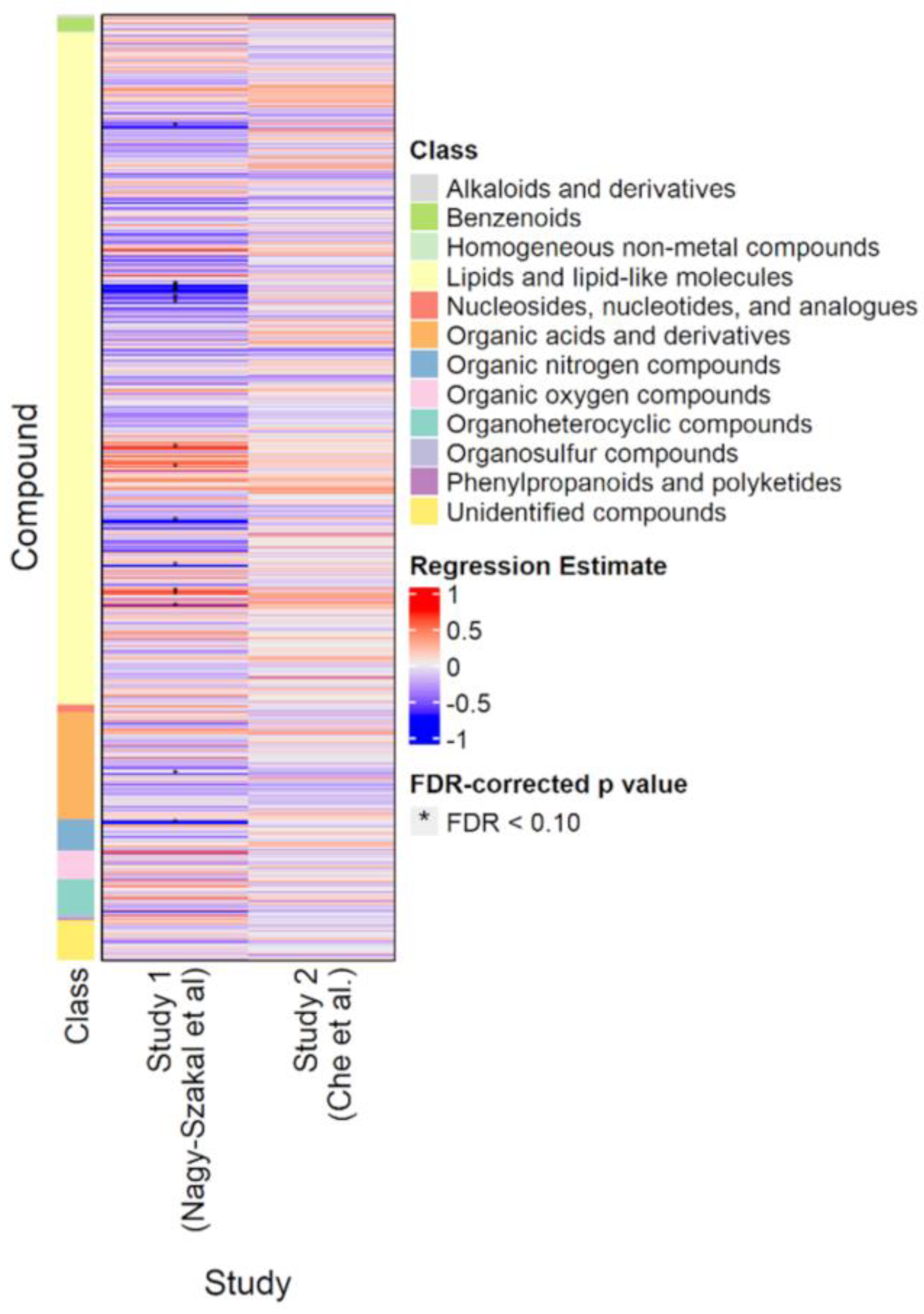
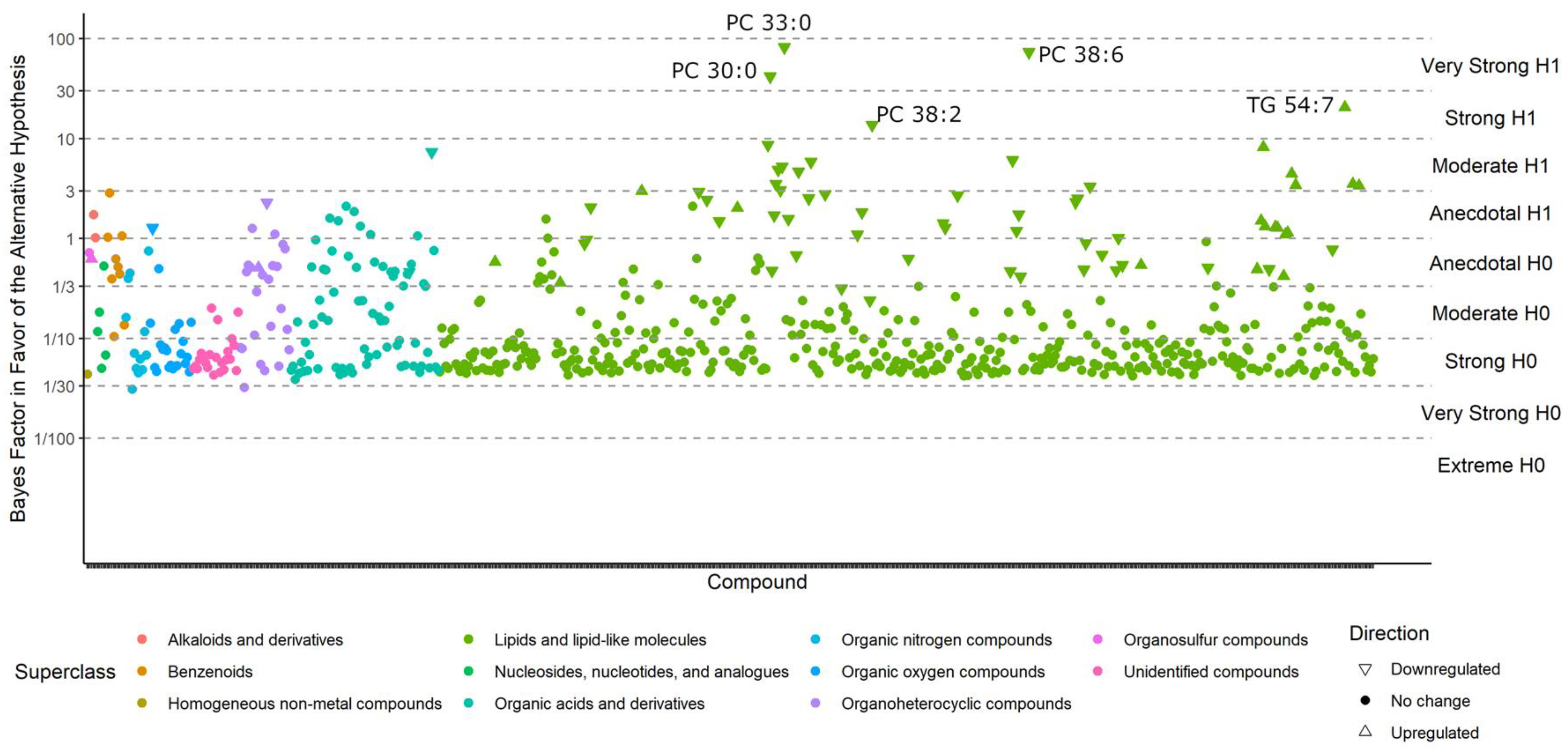
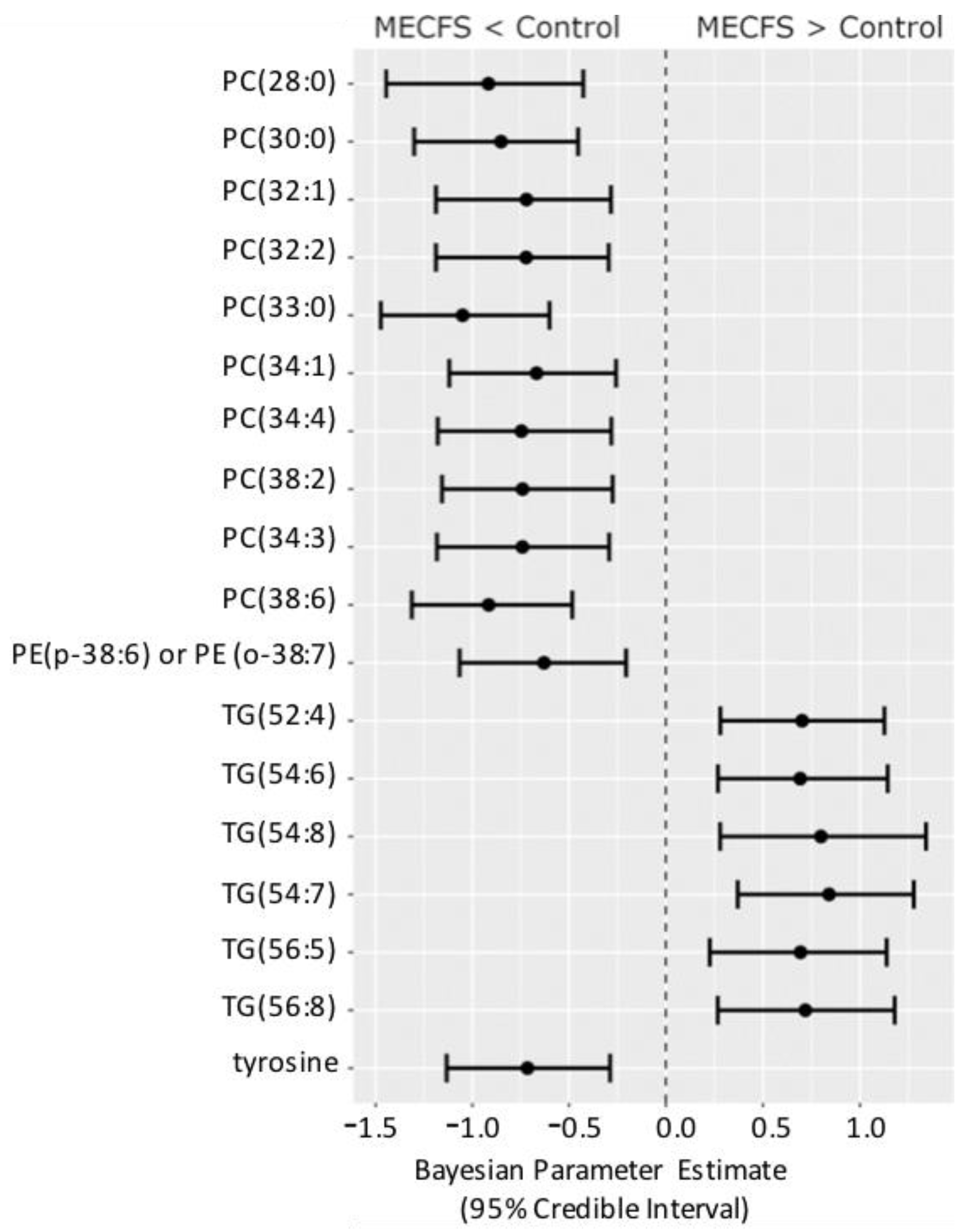
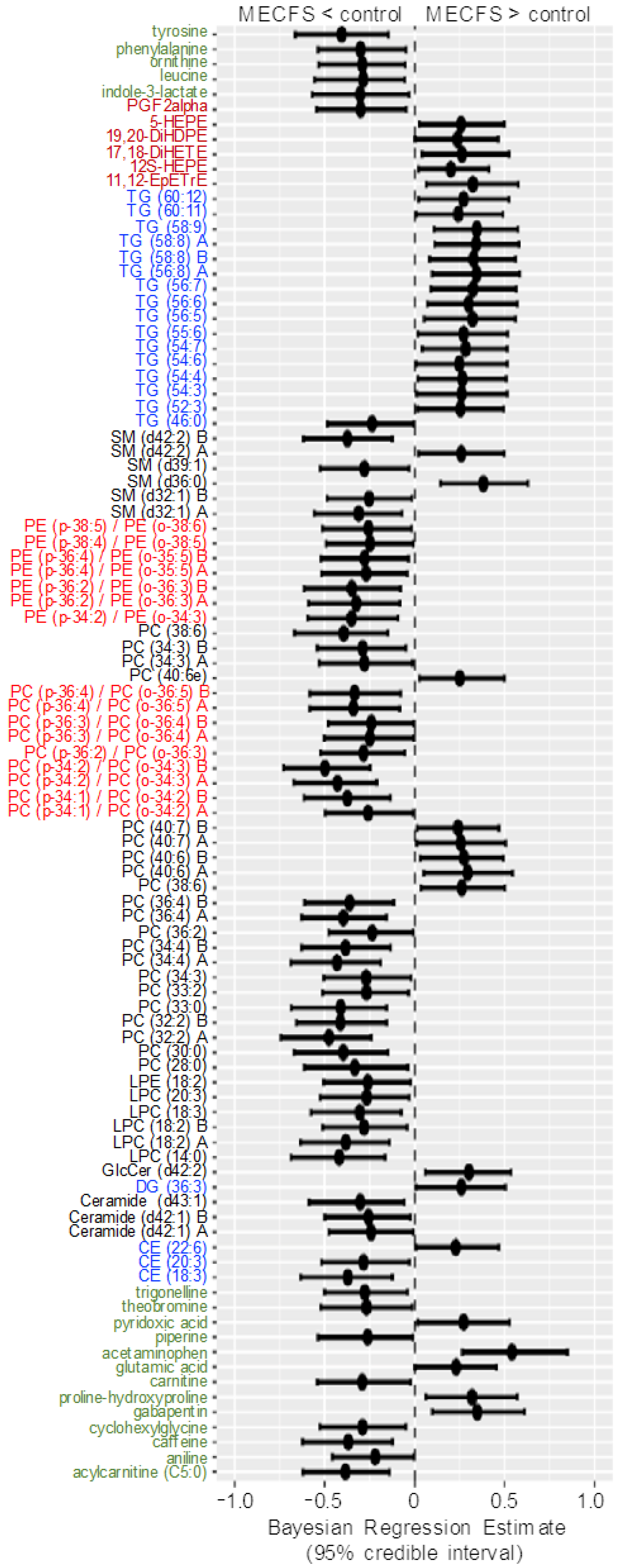
3.2. Bayesian Analyses
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gigerenzer, G. Mindless statistics. J. Socio-Econ. 2004, 33, 587–606. [Google Scholar] [CrossRef]
- Gigerenzer, G. Statistical rituals: The replication delusion and how we got there. Adv. Methods Pract. Psychol. Sci. 2018, 1, 198–218. [Google Scholar] [CrossRef]
- Greenland, S.; Senn, S.J.; Rothman, K.J.; Carlin, J.B.; Poole, C.; Goodman, S.N.; Altman, D.G. Statistical tests, P values, confidence intervals, and power: A guide to misinterpretations. Eur. J. Epidemiol. 2016, 31, 337–350. [Google Scholar] [CrossRef] [PubMed]
- Wagenmakers, E.J. A practical solution to the pervasive problems of p values. Psychon. Bull. Rev. 2007, 14, 779–804. [Google Scholar] [CrossRef] [PubMed]
- Wasserstein, R.L.; Lazar, N.A. The ASA statement on p-values: Context, process, and purpose. Am. Stat. 2016, 70, 129–133. [Google Scholar] [CrossRef]
- Benjamin, D.J.; Berger, J.O.; Johannesson, M.; Nosek, B.A.; Wagenmakers, E.J.; Berk, R.; Bollen, K.A.; Brembs, B.; Brown, L.; Camerer, C.; et al. Redefine statistical significance. Nat. Hum. Behav. 2018, 2, 6–10. [Google Scholar] [CrossRef] [PubMed]
- Lakens, D.; Adolfi, F.G.; Albers, C.J.; Anvari, F.; Apps, M.A.; Argamon, S.E.; Baguley, T.; Becker, R.B.; Benning, S.D.; Bradford, D.E.; et al. Justify your alpha. Nat. Hum. Behav. 2018, 2, 168–171. [Google Scholar] [CrossRef]
- McShane, B.B.; Gal, D.; Gelman, A.; Robert, C.; Tackett, J.L. Abandon statistical significance. Am. Stat. 2019, 73, 235–245. [Google Scholar] [CrossRef]
- Dienes, Z. Bayesian versus orthodox statistics: Which side are you on? Perspect. Psychol. Sci. 2011, 6, 274–290. [Google Scholar] [CrossRef]
- Krueger, J.I.; Heck, P.R. The heuristic value of p in inductive statistical inference. Front. Psychol. 2017, 9, 908. [Google Scholar] [CrossRef]
- Goodman, S.N. Toward evidence-based medical statistics. 2: The Bayes factor. Ann. Intern. Med. 1999, 130, 1005–1013. [Google Scholar] [CrossRef] [PubMed]
- Wagenmakers, E.J.; Marsman, M.; Jamil, T.; Ly, A.; Verhagen, J.; Love, J.; Selker, R.; Gronau, Q.F.; Šmíra, M.; Epskamp, S.; et al. Bayesian inference for psychology. Part I: Theoretical advantages and practical ramifications. Psychon. Bull. Rev. 2018, 25, 35–57. [Google Scholar] [CrossRef] [PubMed]
- Silver, N. The Signal and the Noise: Why So Many Predictions Fail-but Some Don’t; Penguin: New York, NY, USA, 2012. [Google Scholar]
- Kruschke, J.K.; Liddell, T.M. Bayesian data analysis for newcomers. Psychon. Bull. Rev. 2018, 25, 155–177. [Google Scholar] [CrossRef] [PubMed]
- Nagy-Szakal, D.; Barupal, D.K.; Lee, B.; Che, X.; Williams, B.L.; Kahn, E.J.; Ukaigwe, J.E.; Bateman, L.; Klimas, N.G.; Komaroff, A.L.; et al. Insights into myalgic encephalomyelitis/chronic fatigue syndrome phenotypes through comprehensive metabolomics. Sci. Rep. 2018, 8, 10056. [Google Scholar] [CrossRef] [PubMed]
- Sud, M.; Fahy, E.; Cotter, D.; Azam, K.; Vadivelu, I.; Burant, C.; Edison, A.; Fiehn, O.; Higashi, R.; Nair, K.S.; et al. Metabolomics Workbench: An international repository for metabolomics data and metadata, metabolite standards, protocols, tutorials and training, and analysis tools. Nucleic Acids Res. 2016, 44, D463–D470. [Google Scholar] [CrossRef] [PubMed]
- Che, X.; Brydges, C.R.; Yu, Y.; Price, A.; Joshi, S.; Roy, A.; Lee, B.; Barupal, D.K.; Cheng, A.; Palmer, D.M.; et al. Metabolomic Evidence for Peroxisomal Dysfunction in Myalgic Encephalomyelitis/Chronic Fatigue Syndrome. Int. J. Mol. Sci. 2022, 23, 7906. [Google Scholar] [CrossRef] [PubMed]
- Naviaux, R.K.; Naviaux, J.C.; Li, K.; Bright, A.T.; Alaynick, W.A.; Wang, L.; Baxter, A.; Nathan, N.; Anderson, W.; Gordon, E. Metabolic features of chronic fatigue syndrome. Proc. Natl. Acad. Sci. USA 2016, 113, E5472–E5480. [Google Scholar] [CrossRef] [PubMed]
- Fahy, E.; Subramaniam, S. RefMet: A reference nomenclature for metabolomics. Nat. Methods 2020, 17, 1173–1174. [Google Scholar] [CrossRef]
- Goodrich, B.; Gabry, J.; Ali, I.; Brilleman, S. Rstanarm: Bayesian Applied Regression Modeling via Stan. R Package Version. 2020. Available online: https://mc-stan.org/rstanarm/ (accessed on 29 August 2023).
- Makowski, D.; Ben-Shachar, M.S.; Lüdecke, D. bayestestR: Describing effects and their uncertainty, existence and significance within the Bayesian framework. J. Open Source Softw. 2019, 4, 1541. [Google Scholar] [CrossRef]
- Gelman, A.; Jakulin, A.; Pittau, M.G.; Su, Y.S. A weakly informative default prior distribution for logistic and other regression models. Ann. Appl. Stat. 2008, 2, 1360–1383. [Google Scholar] [CrossRef]
- Muth, C.; Oravecz, Z.; Gabry, J. User-friendly Bayesian regression modeling: A tutorial with rstanarm and shinystan. Quant. Methods Psychol. 2018, 14, 99–119. [Google Scholar] [CrossRef]
- Barupal, D.K.; Fiehn, O. Chemical Similarity Enrichment Analysis (ChemRICH) as alternative to biochemical pathway mapping for metabolomic datasets. Sci. Rep. 2017, 7, 14567. [Google Scholar] [CrossRef]
- Makowski, D.; Ben-Shachar, M.S.; Chen, S.H.; Lüdecke, D. Indices of effect existence and significance in the Bayesian framework. Front. Psychol. 2019, 10, 2767. [Google Scholar] [CrossRef] [PubMed]
- Lee, M.D.; Wagenmakers, E.J. Bayesian Cognitive Modeling: A Practical Course; Cambridge University Press: New York, NY, USA, 2014. [Google Scholar]
- Jeffreys, H. The Theory of Probability; Oxford University Press: Oxford, UK, 1961. [Google Scholar]
- Ackermann, M.; Strimmer, K. A general modular framework for gene set enrichment analysis. BMC Bioinform. 2009, 10, 47. [Google Scholar] [CrossRef] [PubMed]
- Hung, J.H.; Yang, T.H.; Hu, Z.; Weng, Z.; DeLisi, C. Gene set enrichment analysis: Performance evaluation and usage guidelines. Brief. Bioinform. 2012, 13, 281–291. [Google Scholar] [CrossRef]
- Canavan, C.; West, J.; Card, T. The epidemiology of irritable bowel syndrome. Clin. Epidemiol. 2014, 6, 71. [Google Scholar]
- Prince, N.; Stav, M.; Cote, M.; Chu, S.H.; Vyas, C.M.; Okereke, O.I.; Palacios, N.; Litonjua, A.A.; Vokonas, P.; Sparrow, D.; et al. Metabolomics and Self-Reported Depression, Anxiety, and Phobic Symptoms in the VA Normative Aging Study. Metabolites 2023, 13, 851. [Google Scholar] [CrossRef] [PubMed]
- Panyard, D.J.; McKetney, J.; Deming, Y.K.; Morrow, A.R.; Ennis, G.E.; Jonaitis, E.M.; Van Hulle, C.A.; Yang, C.; Sung, Y.J.; Ali, M.; et al. Large-scale proteome and metabolome analysis of CSF implicates altered glucose and carbon metabolism and succinylcarnitine in Alzheimer’s disease. Alzheimer’s Dement. 2023; in press. [Google Scholar] [CrossRef]
- Hoel, F.; Hoel, A.; Pettersen, I.K.; Rekeland, I.G.; Risa, K.; Alme, K.; Sørland, K.; Fosså, A.; Lien, K.; Herder, I.; et al. A map of metabolic phenotypes in patients with myalgic encephalomyelitis/chronic fatigue syndrome. JCI Insight 2021, 6, e149217. [Google Scholar] [CrossRef]
- Bartel, J.; Krumsiek, J.; Theis, F.J. Statistical methods for the analysis of high-throughput metabolomics data. Comput. Struct. Biotechnol. J. 2013, 4, e201301009. [Google Scholar] [CrossRef]
- Lamichhane, S.; Sen, P.; Dickens, A.M.; Hyötyläinen, T.; Orešič, M. An overview of metabolomics data analysis: Current tools and future perspectives. Compr. Anal. Chem. 2018, 82, 387–413. [Google Scholar]
- Saccenti, E.; Hoefsloot, H.C.; Smilde, A.K.; Westerhuis, J.A.; Hendriks, M.M. Reflections on univariate and multivariate analysis of metabolomics data. Metabolomics 2014, 10, 361–374. [Google Scholar] [CrossRef]
- Livera, A.M.; Olshansky, M.; Speed, T.P. Statistical analysis of metabolomics data. In Metabolomics Tools for Natural Product Discovery; Humana Press: Totowa, NJ, USA, 2013; pp. 291–307. [Google Scholar]
- Lakens, D.; McLatchie, N.; Isager, P.M.; Scheel, A.M.; Dienes, Z. Improving inferences about null effects with Bayes factors and equivalence tests. J. Gerontol. Ser. B 2020, 75, 45–57. [Google Scholar] [CrossRef] [PubMed]
- Etz, A.; Haaf, J.M.; Rouder, J.N.; Vandekerckhove, J. Bayesian inference and testing any hypothesis you can specify. Adv. Methods Pract. Psychol. Sci. 2018, 1, 281–295. [Google Scholar] [CrossRef]
- Haaf, J.M.; Rouder, J.N. Developing constraint in Bayesian mixed models. Psychol. Methods 2017, 22, 779. [Google Scholar] [CrossRef] [PubMed]
- Morey, R.D.; Hoekstra, R.; Rouder, J.N.; Lee, M.D.; Wagenmakers, E.J. The fallacy of placing confidence in confidence intervals. Psychon. Bull. Rev. 2016, 23, 103–123. [Google Scholar] [CrossRef] [PubMed]
- Dienes, Z.; Mclatchie, N. Four reasons to prefer Bayesian analyses over significance testing. Psychon. Bull. Rev. 2018, 25, 207–218. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brydges, C.; Che, X.; Lipkin, W.I.; Fiehn, O. Bayesian Statistics Improves Biological Interpretability of Metabolomics Data from Human Cohorts. Metabolites 2023, 13, 984. https://doi.org/10.3390/metabo13090984
Brydges C, Che X, Lipkin WI, Fiehn O. Bayesian Statistics Improves Biological Interpretability of Metabolomics Data from Human Cohorts. Metabolites. 2023; 13(9):984. https://doi.org/10.3390/metabo13090984
Chicago/Turabian StyleBrydges, Christopher, Xiaoyu Che, Walter Ian Lipkin, and Oliver Fiehn. 2023. "Bayesian Statistics Improves Biological Interpretability of Metabolomics Data from Human Cohorts" Metabolites 13, no. 9: 984. https://doi.org/10.3390/metabo13090984
APA StyleBrydges, C., Che, X., Lipkin, W. I., & Fiehn, O. (2023). Bayesian Statistics Improves Biological Interpretability of Metabolomics Data from Human Cohorts. Metabolites, 13(9), 984. https://doi.org/10.3390/metabo13090984







